Numerical Simulation and Experimental Validation of the Flow-Induced Excitation Characteristics of a Four-Way Reversing Valve
Abstract
:1. Introduction
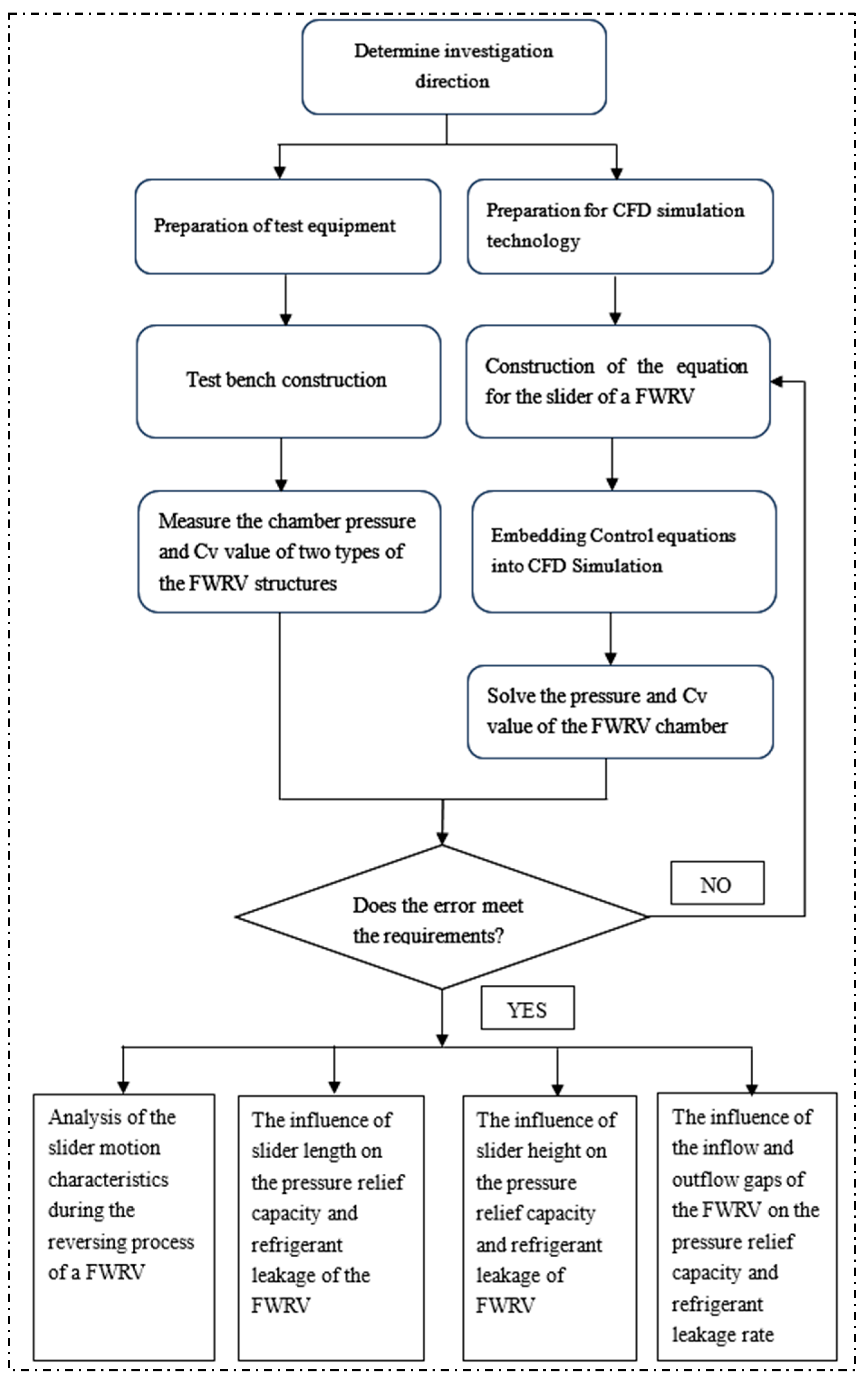
2. Materials and Methods
2.1. Analysis of the Problem in the Reversing Process of the FWRV
2.2. Materials and Methods
2.2.1. Establishment of Mathematical Model for the Motion Process of Sliders
- The physical parameters of air do not change during reversing, that is, the density and viscosity are constant;
- During the reversing process, the pressure at the inlet and outlet of the FWRV is stable;
- Ignore the influence of ambient temperature on the four-way reversing valve.
- (1)
- Force balance equation of sliding
where PD is the inlet pressure of the D pipe, f is the friction coefficient of the capillary, v is the flow rate of fluid in the capillary, L is the length of the capillary, d is the diameter of the capillary, λ is the friction coefficient between the slider and the valve seat, FP is the pressure exerted on the slider, A1 is the cross-sectional area of the capillary, A2 is the cross-sectional area of the valve chamber, u is the fluid viscosity, ε is the absolute roughness, Re is the Reynolds number, and μ is the dynamic viscosity.- (2)
- Equation of slider motion
where m is the mass of moving parts such as the slider and bracket, w is the moving speed of the slider, t is the time, Ft is the propulsive force, and Ff is the frictional force.
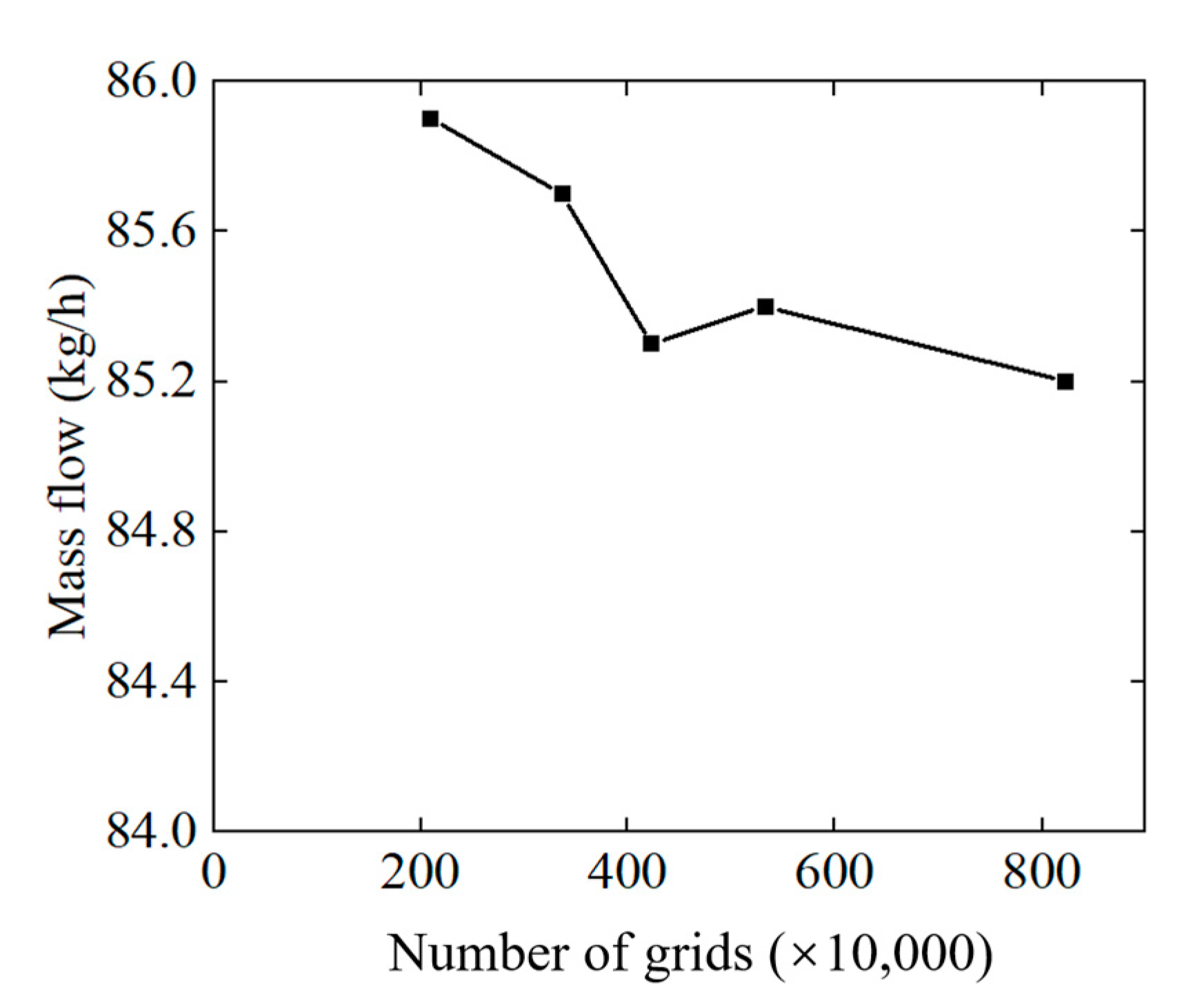
2.2.2. Establishment of Grid Model for Four-Way Reversing Valve
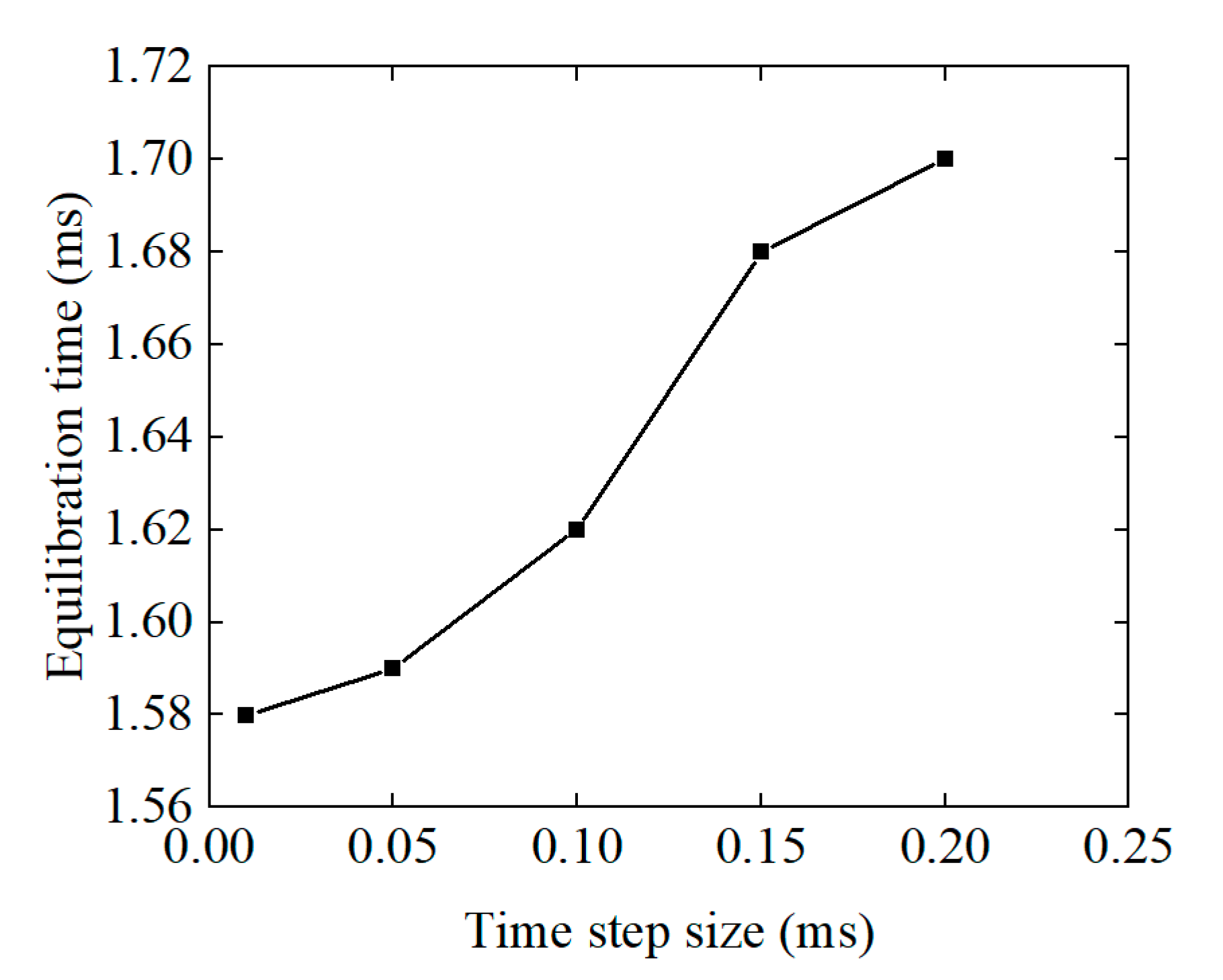
2.2.3. Parameter Settings for the Transient Solution Process of the Four-Way Reversing Valve
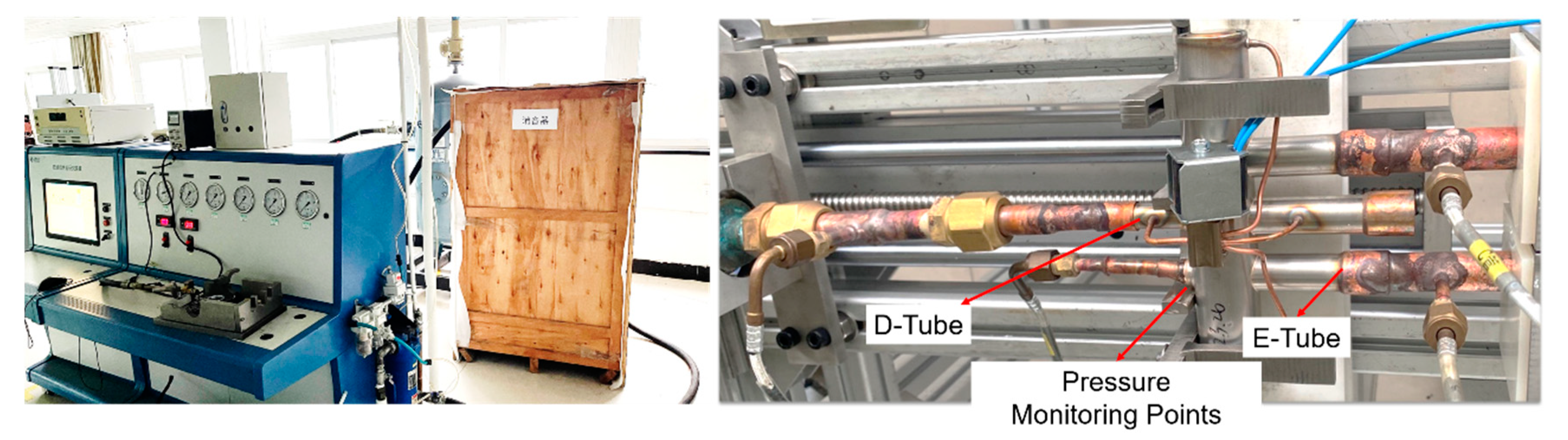
2.2.4. Model Experiment Validation
2.2.5. Deviation and Uncertainty Analysis
3. Discussion
3.1. Transient Characteristics Analysis of FWRV
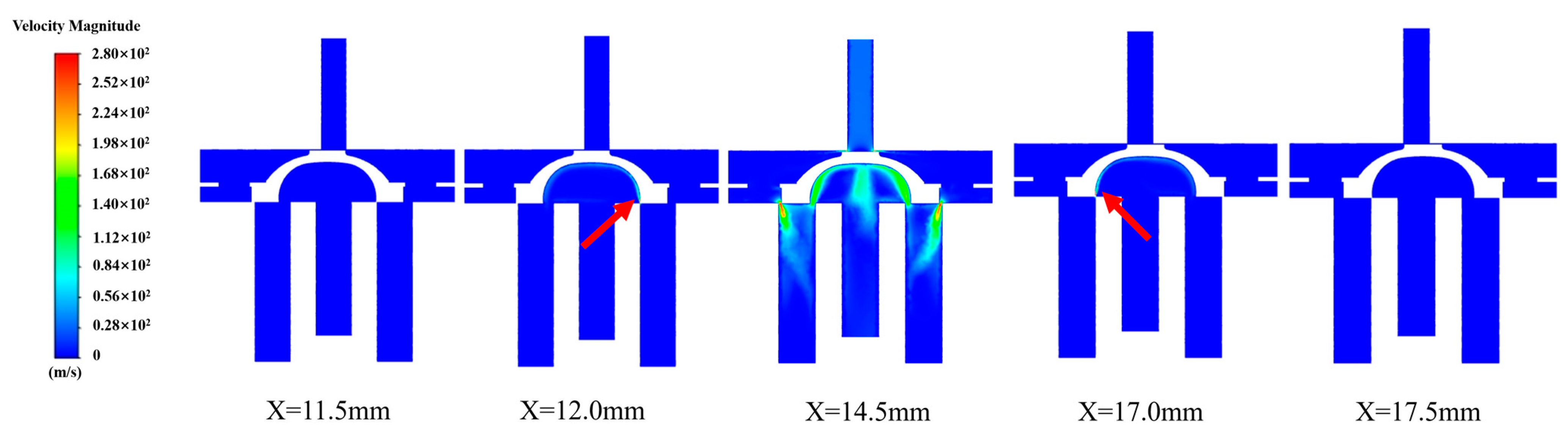
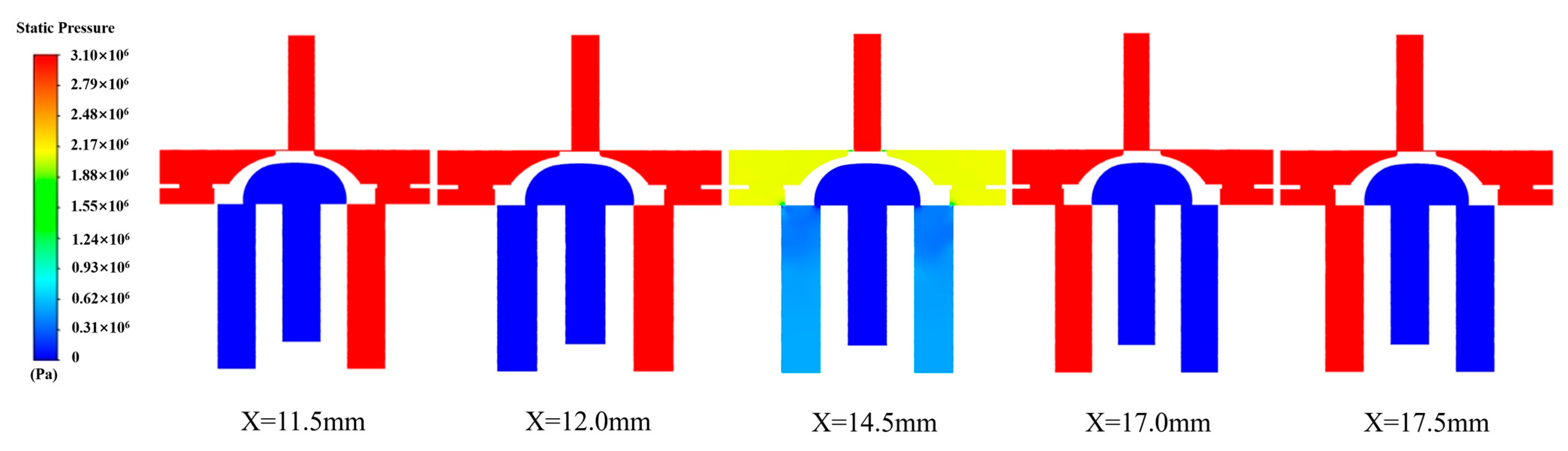
3.1.1. The Variation in Velocity and Pressure Fields inside the Valve with the Motion Process
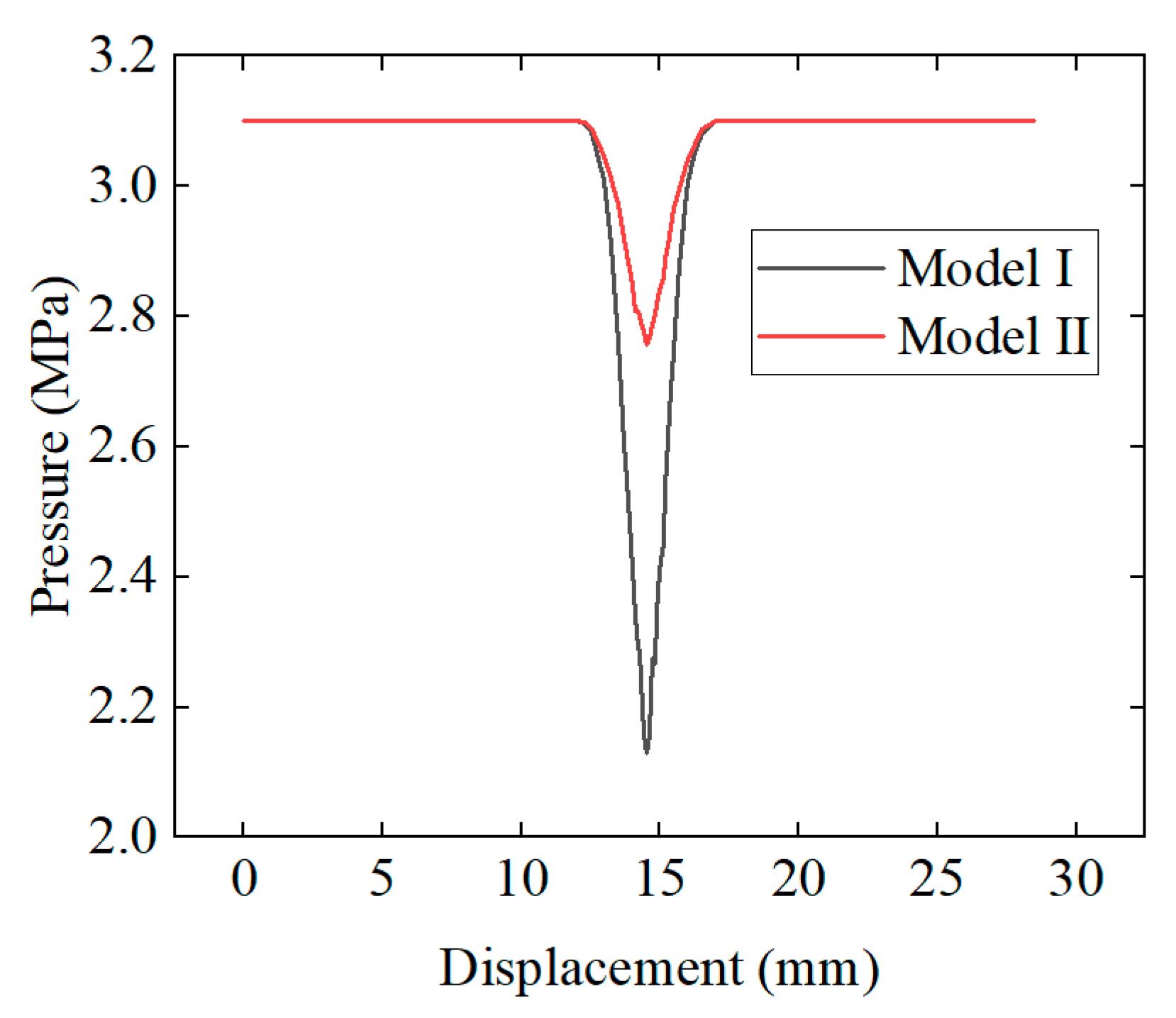
3.1.2. The Variation in Pressure in the Valve Chamber with the Reversing Process
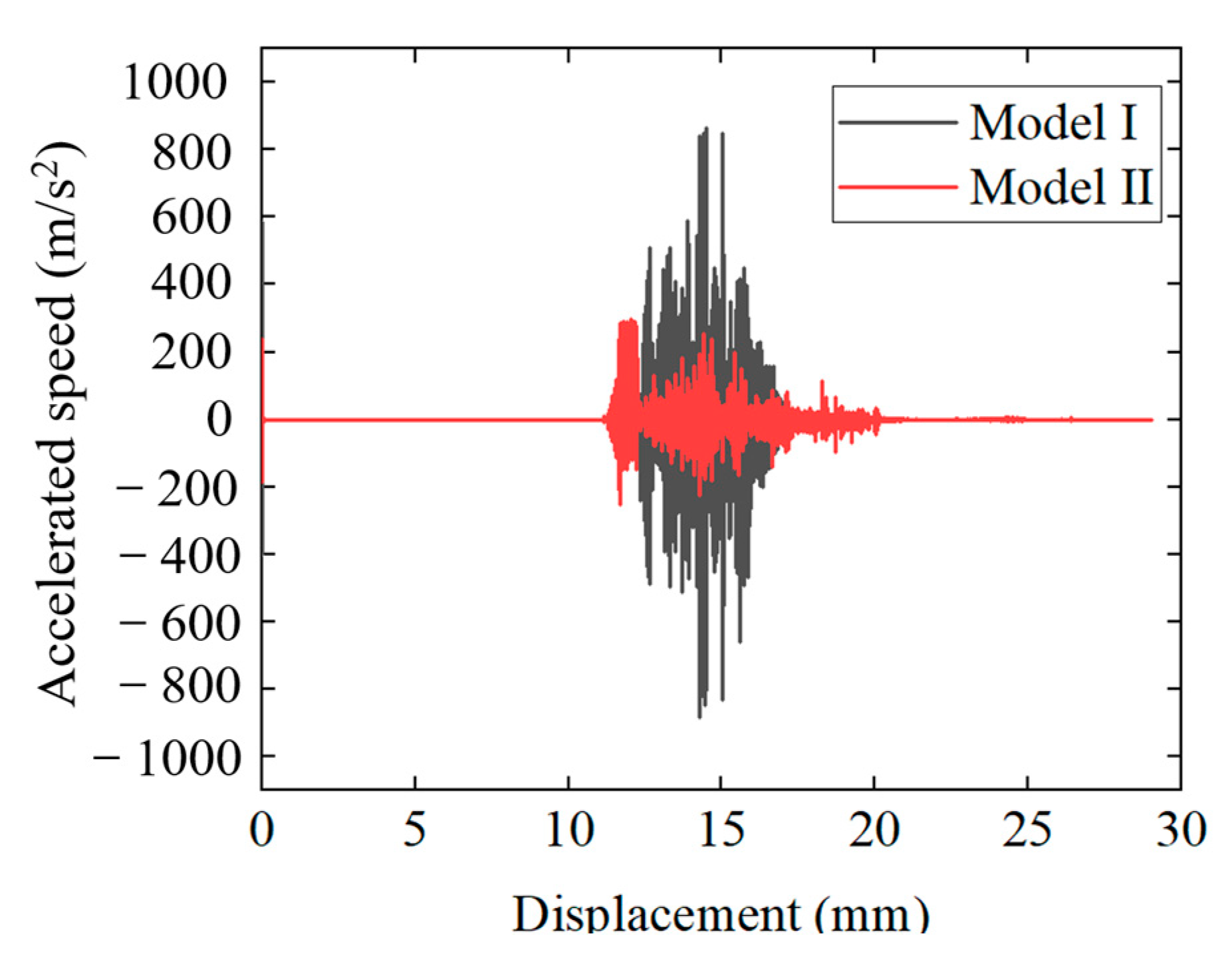
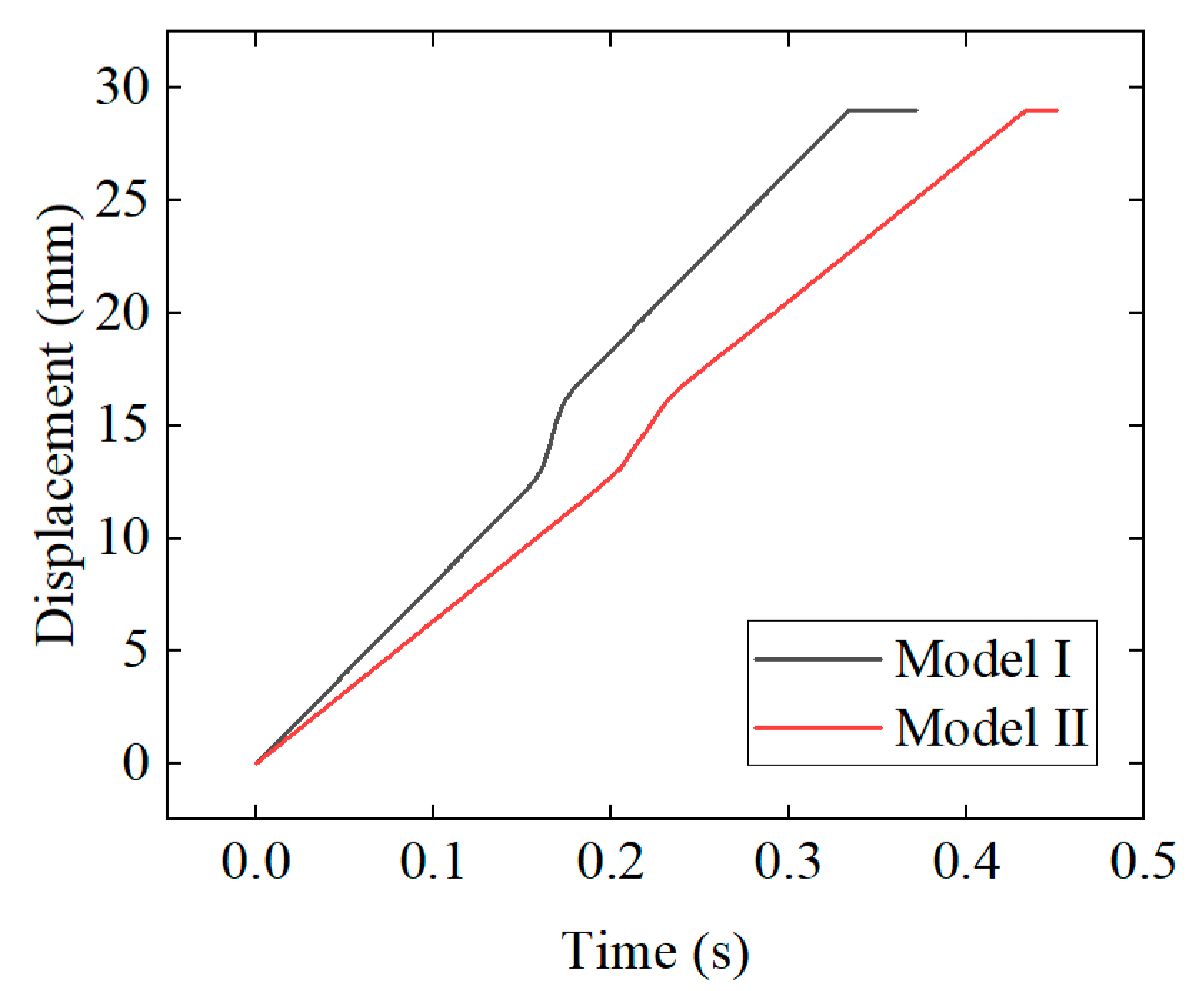
3.1.3. Acceleration and Velocity Changes in Slider Movement
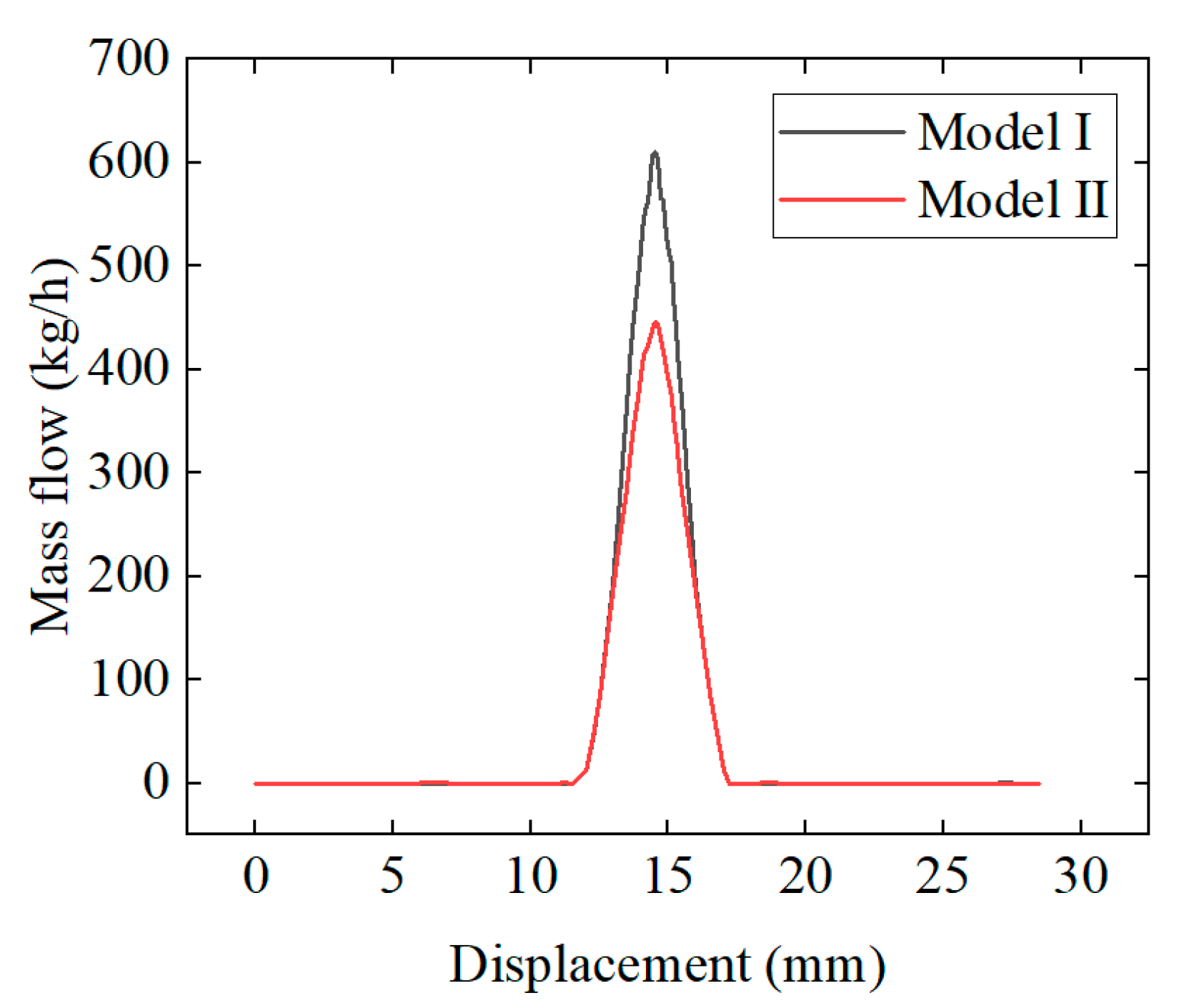
3.1.4. Change in Leakage Amount with Reversing Process
3.1.5. Analysis of Leakage Capacity during Reversing Process
3.2. Analysis of the Slider Structure of an FWRV
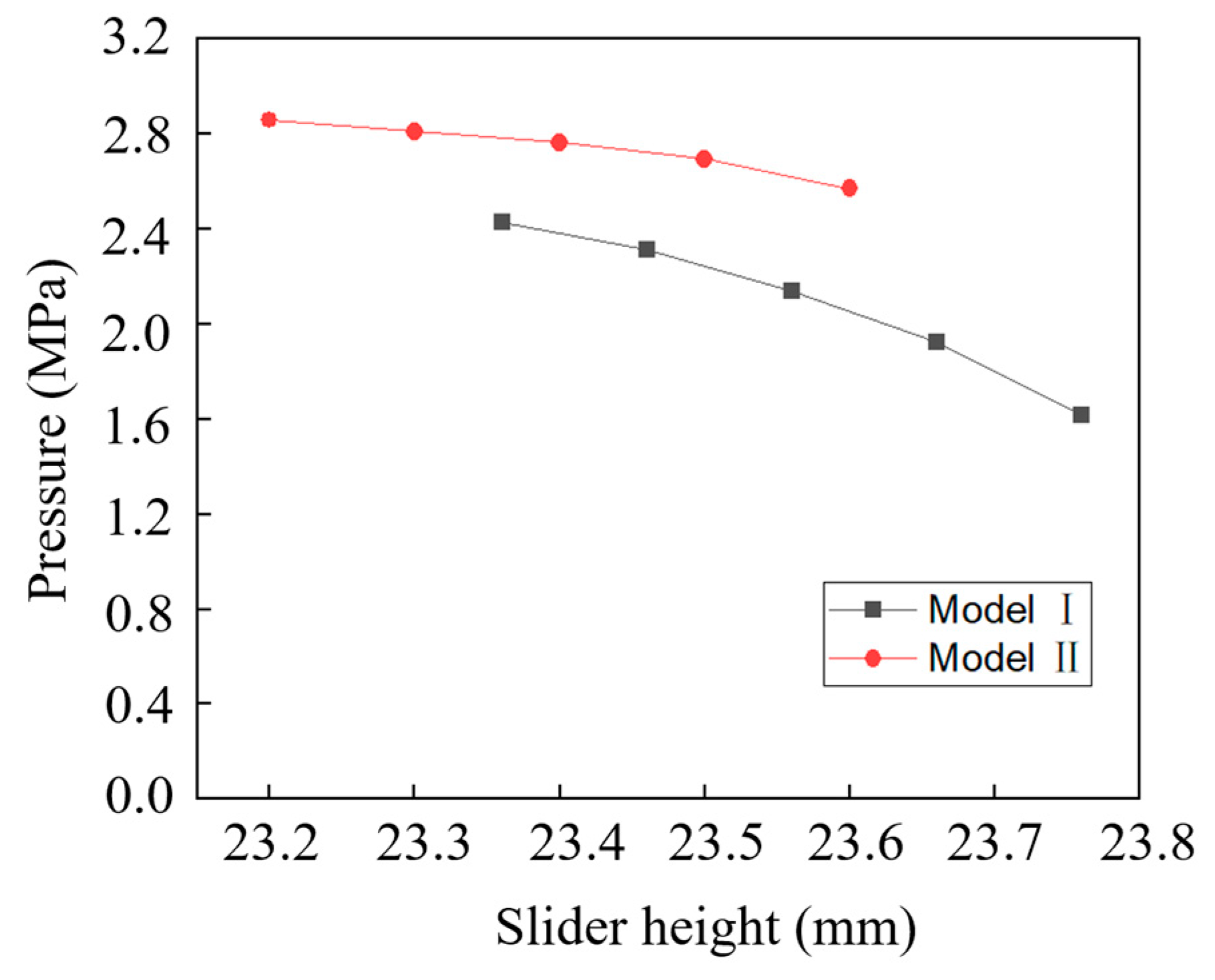
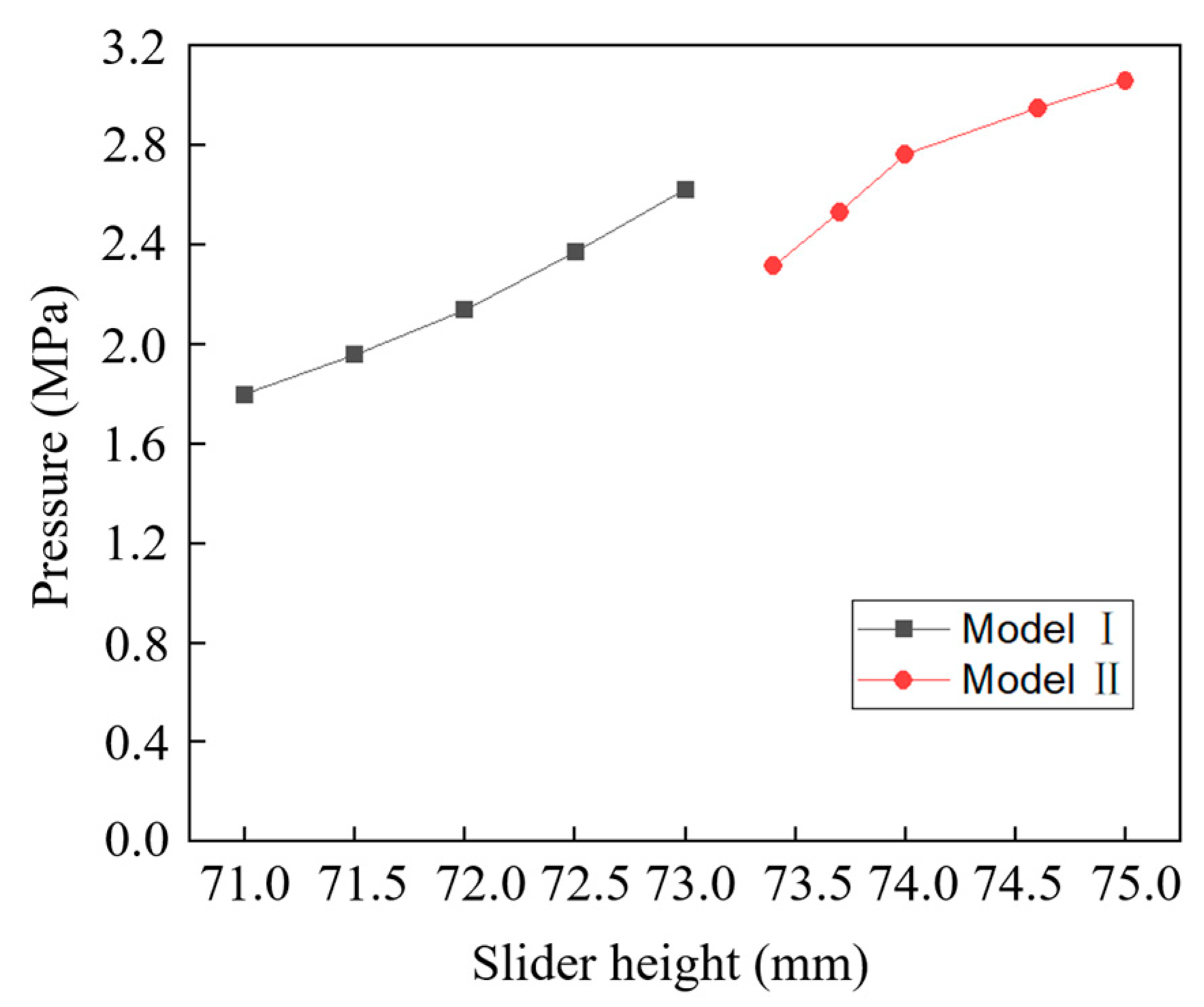
3.2.1. The Influence of Slider Height and Length on Pressure Relief Capacity
3.2.2. The Influence of Slider Height and Length on Refrigerant Leakage Capacity
4. Conclusions
- Based on the analysis of the transient characteristics of the reversing process of the FWRV, it is shown that the flow field and pressure field in the valve chamber are extremely unstable when the slider is in the initial and intermediate positions during the reversing process of the four-way reversing valve, which can easily induce slider vibration and increase the probability of reversing failure.
- Based on the analysis of the impact of slider height on pressure relief capacity and refrigerant leakage capacity, it is shown that increasing the height of the slider is not only beneficial for improving the pressure relief capacity of the FWRV, protecting the slider from damage caused by high-pressure gas impact, and ensuring the smooth operation of the directional process, but it also reduces the leakage of refrigerant during the directional process, thereby improving the working efficiency of the heat pump air conditioning system.
- Based on the analysis of the relationship between the length of the slider and the pressure relief capacity and refrigerant leakage capacity, it is shown that reducing the length of the slider may improve the pressure relief capacity of the FWRV, but it will increase the leakage of refrigerant and thereby reduce the working efficiency of the heat pump air conditioning system.
- Overall, when designing the slider of the FWRV, the height and length of the slider should be increased as much as possible to improve its working performance.
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| PD | Inlet pressure of D pipe (MPa) |
| v | Flow velocity of fluid in capillaries (m/s) |
| L | Length of capillary (m) |
| d | Diameter of capillary (m) |
| Fp | Pressure exerted on the slider (N) |
| A1 | Cross-sectional area of the capillary (m2) |
| A2 | Cross-sectional area of the valve chamber (m2) |
| u | Fluid viscosity (Pa·s) |
| m | Mass of moving parts such as slider and bracket (kg) |
| w | Moving speed of slider (m/s) |
| t | Time (s) |
| Ft | Propulsive force (N) |
| Ff | Frictional force (N) |
| f | Friction coefficient of capillary |
| λ | Friction coefficient between the slider and the valve seat |
| Cv0 | Flow coefficients of the four-way reversing valve |
| Cv1 | Flow coefficients of the inflow gap |
| Cv2 | Flow coefficients of the outflow gap |
References
- Asim, T.; Oliveira, A.; Charlton, M.; Mishra, R. Improved Design of a Multi-Stage Continuous-Resistance Trim for minimum Energy Loss in Control Valves. Energy 2019, 174, 954–971. [Google Scholar] [CrossRef]
- Dong, X.; Wu, J.; Li, J.; Xu, X.; Su, H.; Shang, J.; Li, Q.; Gong, L. Impact of external heat transfer on the performance of a cold compressor used in superfluid helium system. Cryogenics 2020, 110, 103141. [Google Scholar] [CrossRef]
- Arpagaus, C.; Bless, F.; Schiffmann, J.; Bertsch, S.S. Multi-temperature heat pumps: A literature review. Int. J. Refrig. 2016, 69, 437–465. [Google Scholar] [CrossRef] [Green Version]
- Wallerand, A.S.; Kermani, M.; Kantor, I.; Maréchal, F. Optimal heat pump integration in industrial processes. Appl. Energy 2018, 219, 68–92. [Google Scholar] [CrossRef] [Green Version]
- Lin, Z.H.; Li, J.Y.; Jin, Z.J.; Qian, J.Y. Fluid dynamic analysis of liquefied natural gas flow through a cryogenic ball valve in liquefied natural gas receiving stations. Energy 2021, 226, 120376. [Google Scholar] [CrossRef]
- Chen, D.; Luo, Y.; Yuan, X. Refrigeration System Synthesis by Continuous Temperature Level Optimization Considering the Sub-Cooler Configuration. Comput. Chem. Eng. 2020, 141, 107031. [Google Scholar] [CrossRef]
- Zhang, X.; Xie, Y.; Han, J.; Wang, Y. Design of control valve with low energy consumption based on Isight platform. Energy 2022, 239, 122328. [Google Scholar] [CrossRef]
- Wang, Z.; Yang, H.; Chen, S. Study on the operating performance of cross hot-gas bypass defrosting system for air-to-water screw heat pumps. Appl. Therm. Eng. 2013, 59, 398–404. [Google Scholar] [CrossRef]
- Choi, H.J.; Kim, B.S.; Kang, D.; Kim, K.C. Defrosting method adopting dual hot gas bypass for an air-to-air heat pump. Appl. Energy 2011, 88, 4544–4555. [Google Scholar] [CrossRef]
- Wang, H.; Lai, Z.; Wu, D.; Zhang, K.; Zheng, M. Investigation of the friction-induced vibration of a novel four-way reversing valve using spectral kurtosis and number of peaks spectrum. Mech. Syst. Signal Process. 2022, 166, 108425. [Google Scholar] [CrossRef]
- Liu, W.; Ni, H.; Wang, P.; Zhao, B. Analytical investigation of the friction reduction performance of longitudinal vibration based on the modified elastoplastic contact model. Tribol. Int. 2020, 146, 106237. [Google Scholar] [CrossRef]
- Gutowski, P.; Leus, M. Computational model for friction force estimation in sliding motion at transverse tangential vibrations of elastic contact support. Tribol. Int. 2015, 90, 455–462. [Google Scholar] [CrossRef]
- Song, X. Study on Commutation Characteristics of Household Heat Pump Air Conditioner and Dynamic Characteristics of Refrigeration System; Shanghai Jiao Tong University: Shanghai, China, 1997. [Google Scholar]
- Dong, J.K.; Jiang, Y.Q.; Yao, Y.; Song, M.J. Experiment study on the influence of four-way reversing valve leakage on heat pump performance. J. Harbin Inst. Technol. 2011, 43, 80–83. [Google Scholar]
- Shang, P.J.; Dong, Y.J.; Yuan, X.L. Experimental Investigation on Four-way Reversing Valve. Refrig. Air Cond. Electr. Power Mach. 2006, 2, 7–10. [Google Scholar]
- Marks, R.T. The Effect of Different Materials on Heat Transfer of Reversing Valves. ASHRAE Trans. 1986, 92, 2B. [Google Scholar]
- Damasceno, G.; Lee, W.; White, L. Heat transfer pressure drop and leakage in reversing valves characterizing parameters. ASHRAE Trans. 1986, 92, 61–70. [Google Scholar]
- Damasceno, G.S.; Lee, W.T.; Rooke, S.P.; Goldschmidt, V.W. Performance of heat pump reversing valves and comparison through characterizing parameters. ASHRAE Trans. 1988, 94, 50–56. [Google Scholar]
- Chen, H.R.; Li, D.P.; He, C. Study on application of four-way valve on stainless steel. Electr. Appl. 2015, 12, 53–58. [Google Scholar]
- Chai, T.; Hu, H.T.; Ding, G.L. Energy-saving Effect of Using New Material in Reversing Valve. J. Refrig. 2011, 33, 49–53. [Google Scholar]
- Chai, T.; Hu, H.T.; Ding, G.L. Optimization Design of Reversing Valve Based on Heat Loss Analysis. Chin. J. Refrig. Technol. 2012, 32, 33–38. [Google Scholar]
- Young, D.J. Development of a northern climate residential air-source heat pump. ASHREA Trans. 1980, 86, 671–684. [Google Scholar]
- Hargraves, D.P. A refrigerant enthalpy method for measuring reversing valve heat transfer. ASHREA Trans. 1986, 95, 88–93. [Google Scholar]
- Deng, N.; Gao, J.; Cai, R.; Jing, X.; Zhang, Y.; Hao, R.; Li, M. Experimental investigation on matching a conventional water source heat pump with different refrigerants for supplying high temperature water. Appl. Therm. Eng. 2020, 166, 114668. [Google Scholar] [CrossRef]
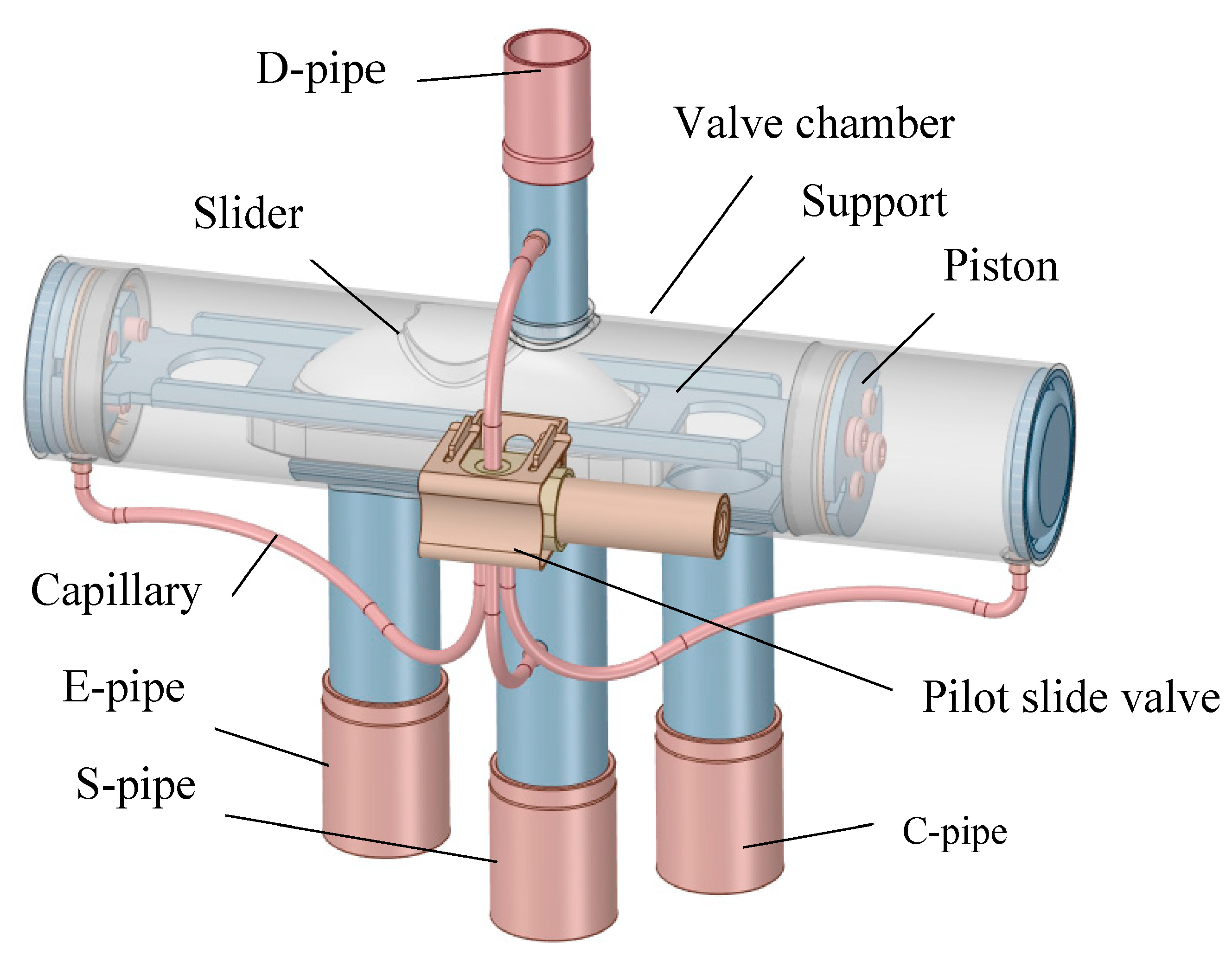

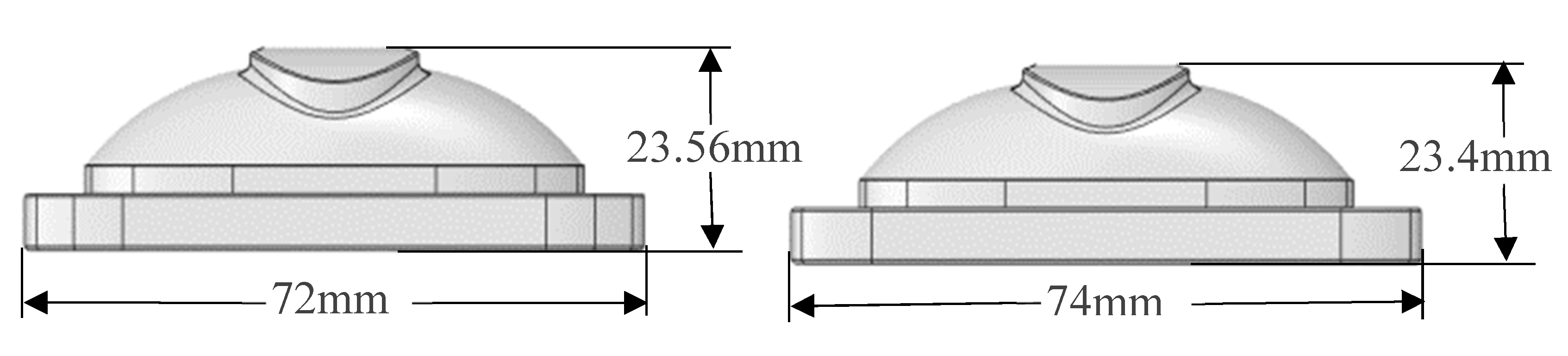
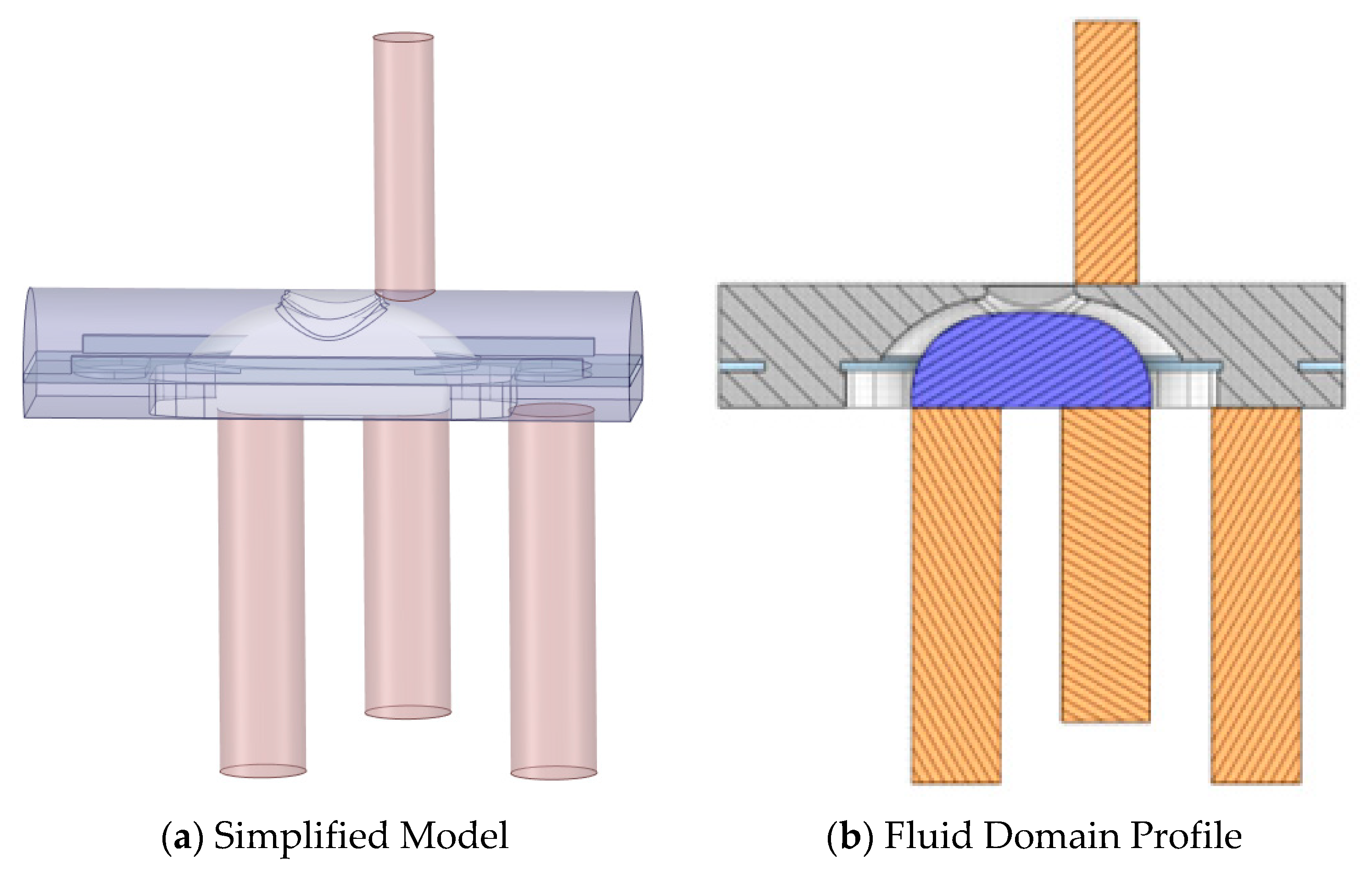





| Model I | Model II | |
|---|---|---|
| D pipe diameter | 12 | 12 |
| E pipe diameter | 17.15 | 17.15 |
| S pipe diameter | 17.15 | 17.15 |
| C pipe diameter | 17.15 | 17.15 |
| Inner diameter of valve chamber | 32 | 32 |
| Slider length | 72 | 74 |
| Slider height | 23.56 | 23.4 |
| Experimental Equipment | Specifications |
|---|---|
| Pressure sensor UNIK5000 | Operating voltage: 7~32 VDC; measuring range: 0~4 MPa; output signal: 4~20 am; Measurement accuracy: 0.2%; response time: 5 ms |
| Gas receiver | Maximum pressure: 4.0 MPa; volume: 0.3 m3 |
| Pressure--regulating valve TESCOM | Regulation precision: ±0.5 bar |
| Mass flowmeter D07-60BM | Operating voltage: ±15 VDC; measuring range: 1500 SLM; output signal: 0–5 VDC; Measurement accuracy: ±2%; response time: <10 s |
| Control console ADAM-3968 | / |
| Model Ⅰ | Model Ⅱ | |||
|---|---|---|---|---|
| Pressure (MPa) | Cv | Pressure (MPa) | Cv | |
| Simulation | 2.12 | 0.68 | 2.78 | 0.50 |
| Test | 2.41 | 0.64 | 3.06 | 0.49 |
| Deviation | 12.03% | 6.25% | 9.15% | 2.04% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, K.; Wu, D.; Yuan, Y.; Zhu, J.; Feng, Z.; Li, J.; Xuan, L.; Xiong, Y. Numerical Simulation and Experimental Validation of the Flow-Induced Excitation Characteristics of a Four-Way Reversing Valve. Processes 2023, 11, 2177. https://doi.org/10.3390/pr11072177
Zhang K, Wu D, Yuan Y, Zhu J, Feng Z, Li J, Xuan L, Xiong Y. Numerical Simulation and Experimental Validation of the Flow-Induced Excitation Characteristics of a Four-Way Reversing Valve. Processes. 2023; 11(7):2177. https://doi.org/10.3390/pr11072177
Chicago/Turabian StyleZhang, Kepeng, Dazhuan Wu, Yadong Yuan, Jiafeng Zhu, Zhongbo Feng, Jianjun Li, Lihua Xuan, and Yunjun Xiong. 2023. "Numerical Simulation and Experimental Validation of the Flow-Induced Excitation Characteristics of a Four-Way Reversing Valve" Processes 11, no. 7: 2177. https://doi.org/10.3390/pr11072177
APA StyleZhang, K., Wu, D., Yuan, Y., Zhu, J., Feng, Z., Li, J., Xuan, L., & Xiong, Y. (2023). Numerical Simulation and Experimental Validation of the Flow-Induced Excitation Characteristics of a Four-Way Reversing Valve. Processes, 11(7), 2177. https://doi.org/10.3390/pr11072177







