Analysis of Heat Transfer of the Gas Head Cover of Diaphragm Compressors for Hydrogen Refueling Stations
Abstract
1. Introduction
2. Analysis of the Heat Transfer Related to the Gas Head Cover
2.1. Heat Transfer from Compressed Gas to the Surface of the Gas Cavity
2.2. Heat Transfer from the Outer Surface of the Gas Head Cover to the Environment
2.3. Heat Transfer between the Gas and the Surface of Suction and Discharge Holes
2.4. Heat Transfer between the Suction/Discharge Nozzles and the Gas Head Cover
3. Finite Element Analysis of the Temperature Field
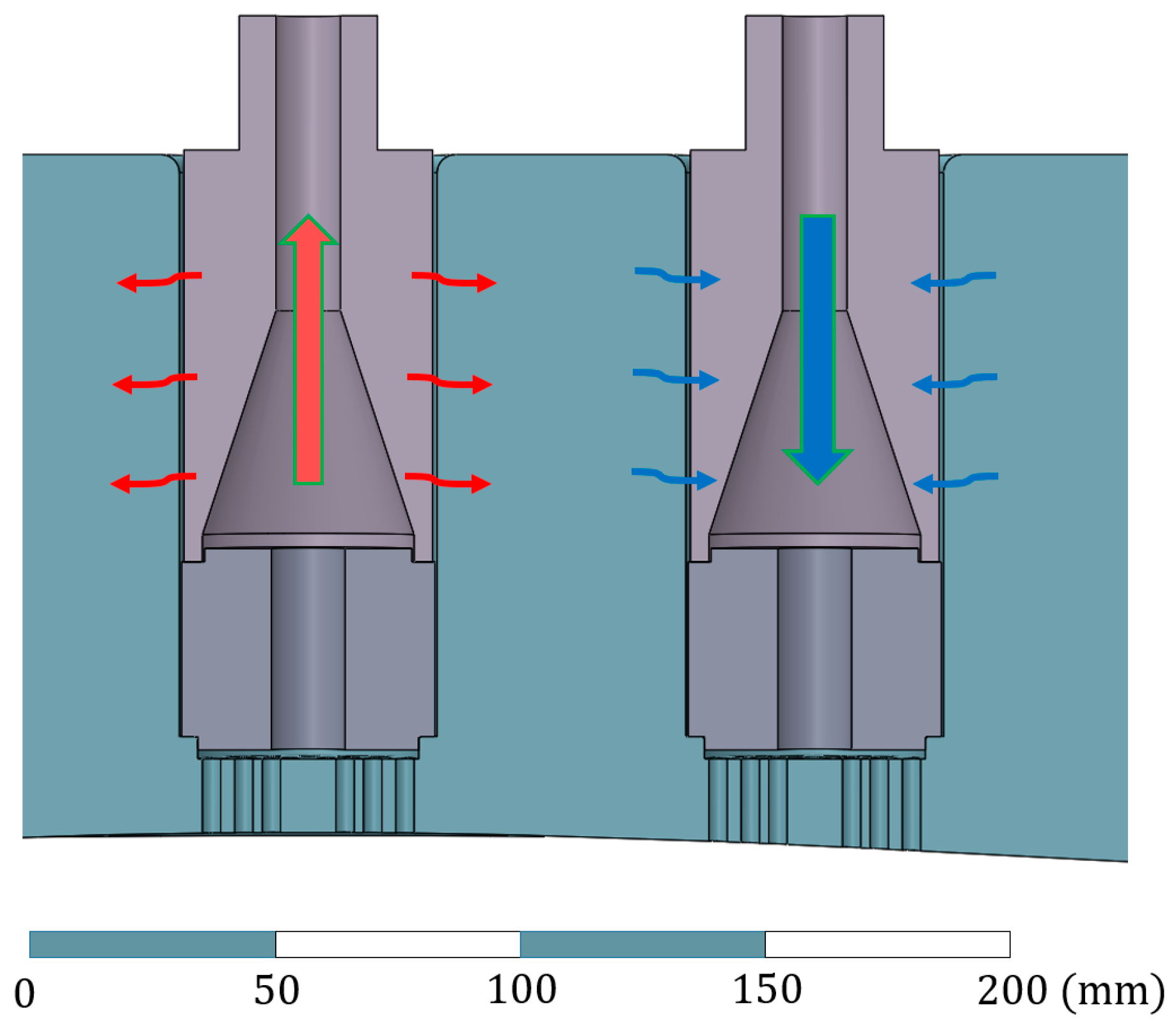
3.1. Geometric Model of the Gas Head Cover
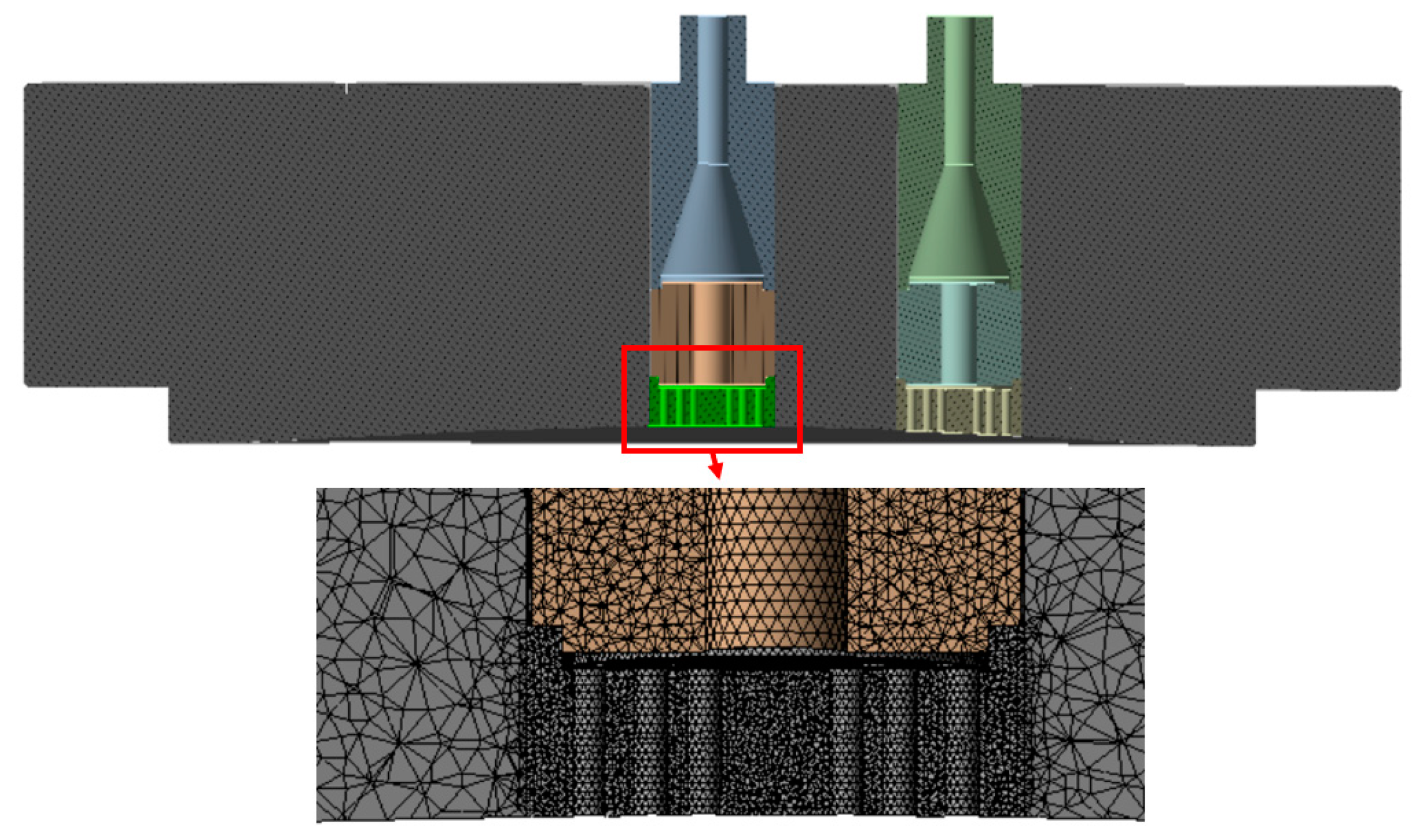
3.2. Mesh Generation
3.3. Thermal Loads
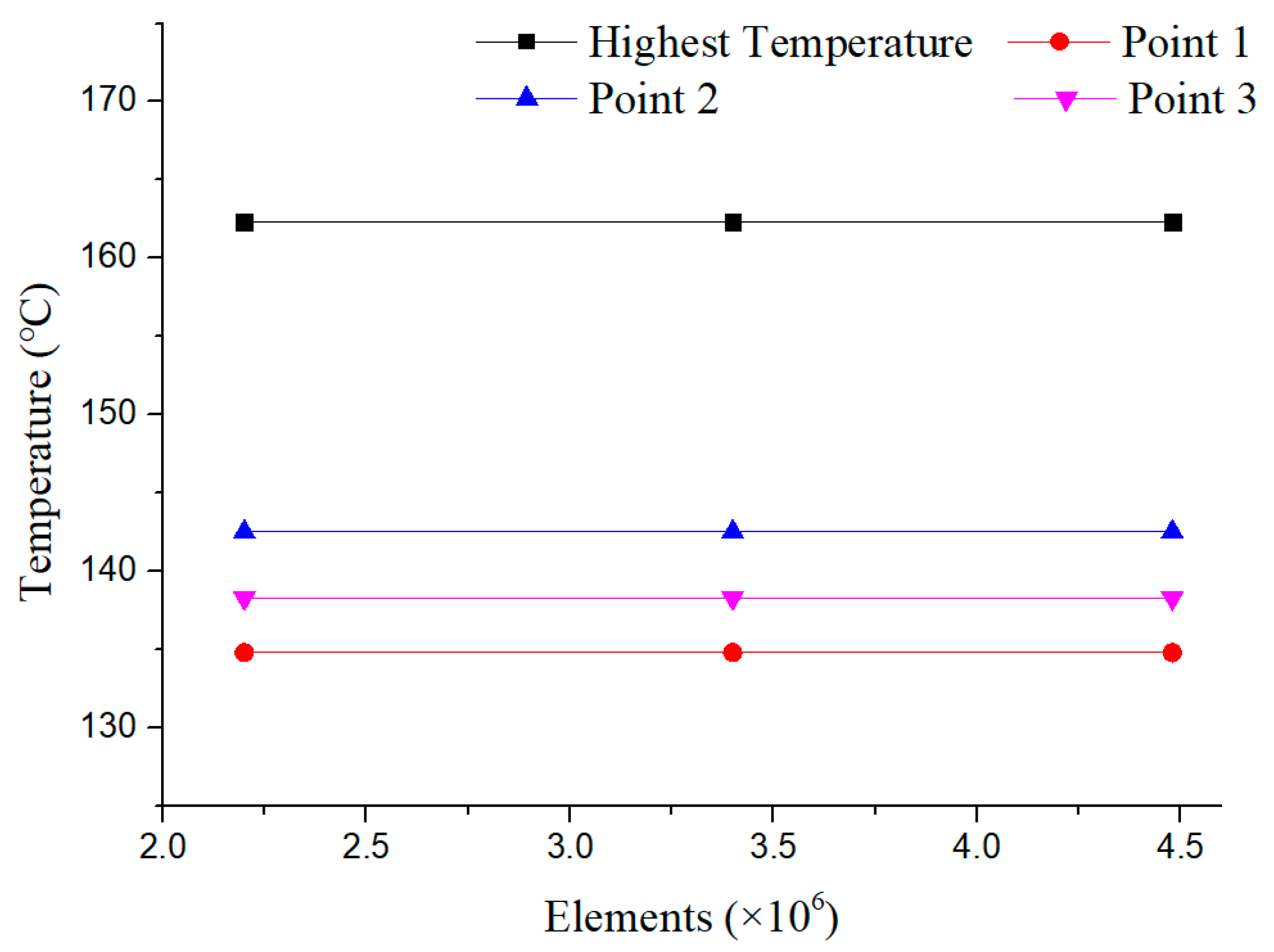
3.4. Mesh Independence Verification
4. Experimental Validation
5. Results and Discussion
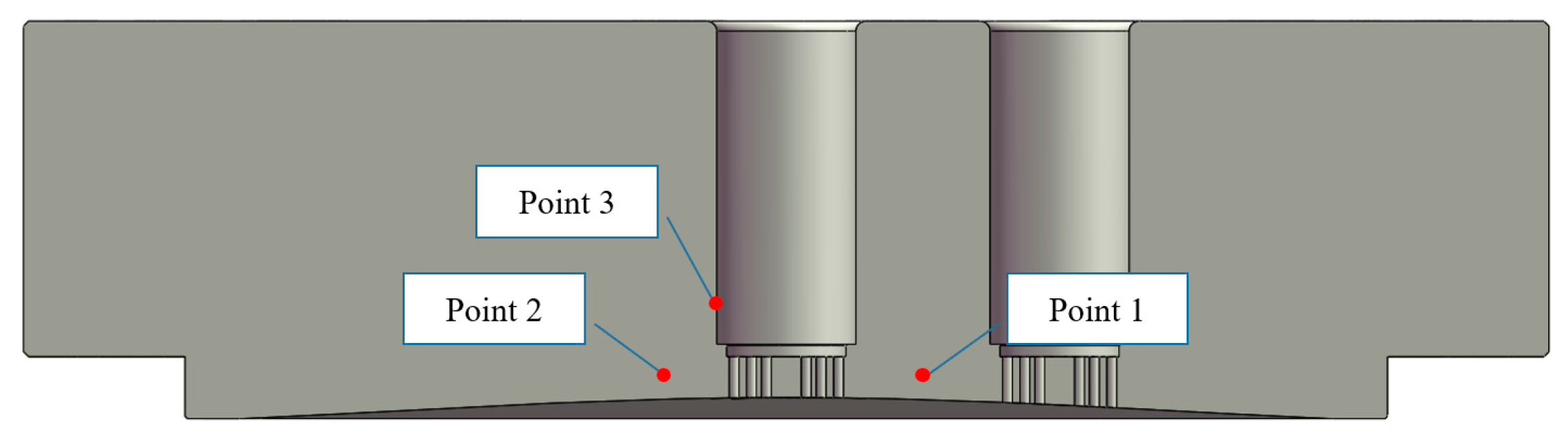
5.1. Finite Element Analysis Results and Comparison with Experimental Values
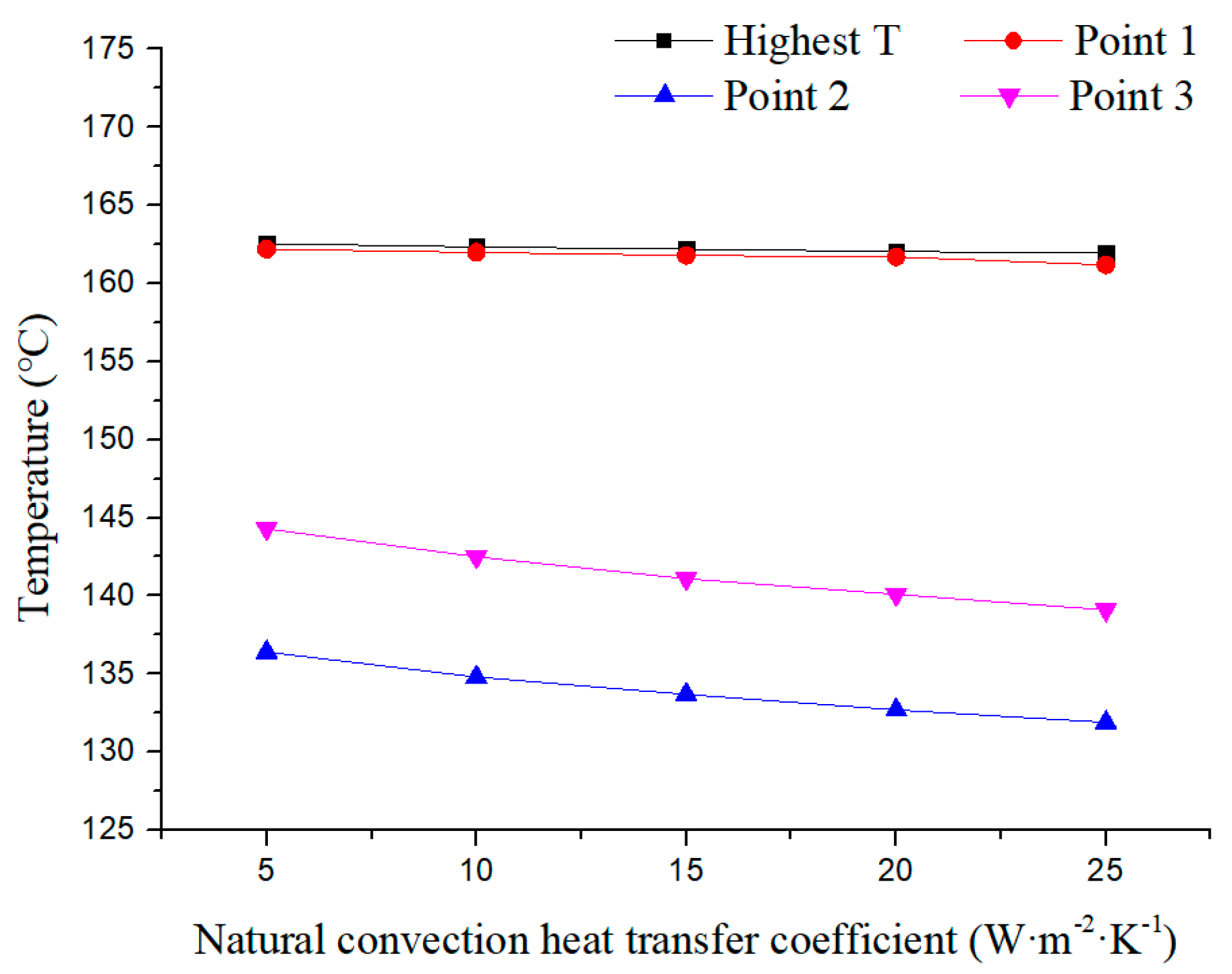
5.2. Influence of the Outer Surface Heat Transfer Coefficient on the Temperature Field of the Gas Head Cover
5.3. Effect of Augmented Heat Transfer of the Outer Surface and Core High-Temperature Zone
6. Conclusions
- The temperature field simulation analysis model established in this paper can calculate and has high accuracy to analyze the temperature distribution characteristics of the diaphragm compressor gas head cover of hydrogen refueling stations. The deviation between the measured temperature and the simulated value of each special measuring point is less than 9.1%.
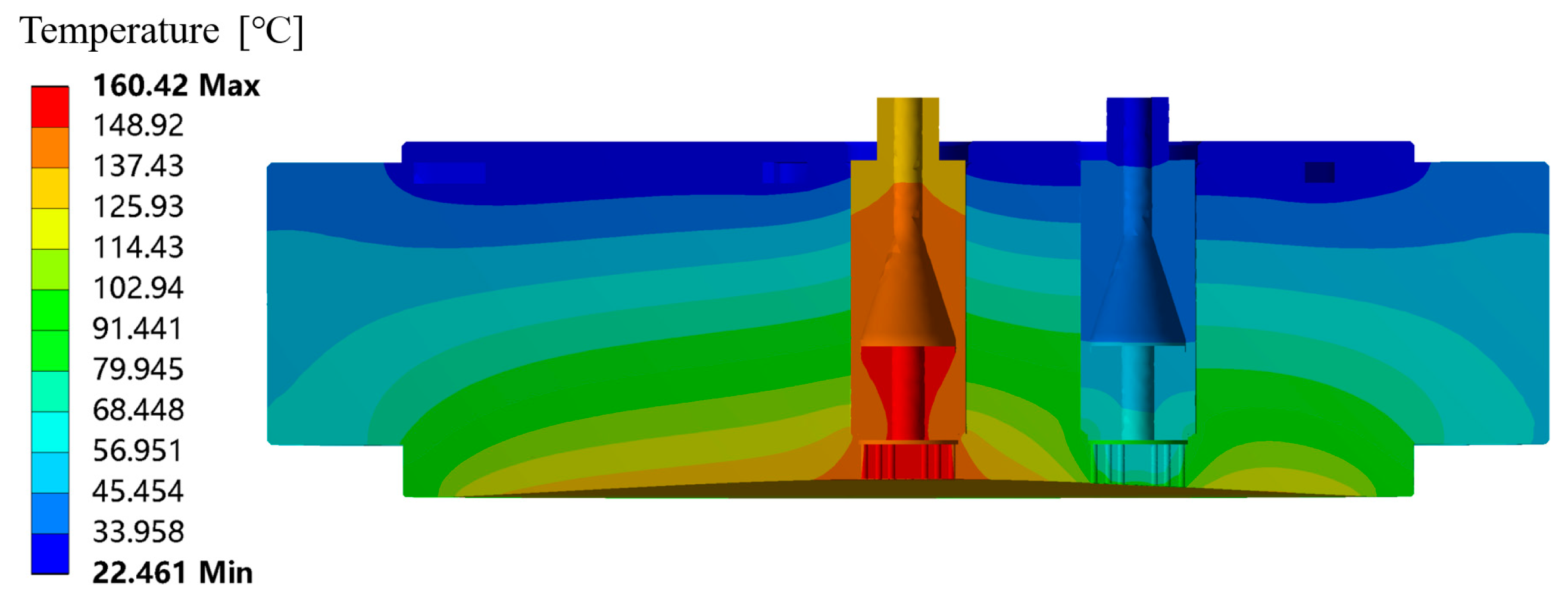
- The region of the discharge holes is the core high-temperature zone of the diaphragm compressor, and the temperature exceeds 150 °C under the mother hydrogen refueling station conditions with suction pressure of 5 MPa and discharge pressure of 22 Mpa. From the discharge holes to the surroundings, the temperature gradient decreases. This is so because the central part has the maximum thermal resistance to the outside air and the highest temperature of the gas in contact with it.
- The temperature field simulation results of the two enhanced heat exchange head cover structures with different enhancing heat transfer methods are in good agreement with the measured values. This simulation method can be used to evaluate the heat dissipation effect of the head cover structure.
- The highest temperature and discharge are reduced by 14.1 °C and 16.1 °C with colling water around the discharge holes, respectively. This method successfully lowers the thermal resistance of heat dissipation in the middle high-temperature area, and significantly lowers the temperature, which can effectively solve the problem of the local excessive temperature of diaphragm compressors and enhance the reliability of diaphragm compressors for hydrogen refueling stations.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| Discharge temperature, K | |
| Suction temperature, K | |
| Discharge pressure, MPa | |
| Suction pressure, MPa | |
| Temperature adiabatic index | |
| Average temperature, K | |
| Convective heat transfer coefficient, W·m−2·K−1 | |
| Rate of decrease of heat transfer coefficient | |
| Radius of the gas cavity | |
| r | Radius |
| Blackness | |
| Stefan-Boltzmann constant | |
| Temperature of the outer surface, K | |
| Temperature of the environment, K | |
| Heat transfer coefficient of heat radiation, W·m−2·K−1 | |
| Heat transfer coefficient of air natural convective, W·m−2·K−1 | |
| Flow velocity of the gas flowing through the suction holes, m/s | |
| Flow velocity of the gas flowing through the discharge holes, m/s | |
| Volume flow rate in standard conditions, m3/h | |
| Pressure in standard conditions, MPa | |
| Temperature in standard conditions, K | |
| Compressibility factors in suction conditions | |
| Compressibility factors in discharge conditions | |
| Total sectional areas of suction holes, m2 | |
| Total sectional areas of discharge holes, m2 | |
| Reynolds numbers | |
| Kinematic viscosity | |
| Diameter of the small holes, m2 | |
| Nusselt number | |
| Prandtl number | |
| Thermal conductivity | |
| Exponent of the Prandtl number | |
| Heat transfer capacity of thermal radiation | |
| Heat transfer capacity of heat conduction | |
| Total heat transfer capacity | |
| Length of the nozzle, m | |
| Thermal conductivity of air |
References
- Zheng, F.; Zhou, X.; Rahat, B.; Rubbaniy, G. Carbon neutrality target for leading exporting countries: On the role of economic complexity index and renewable energy electricity. J. Environ. Manag. 2021, 299, 113558. [Google Scholar]
- Qin, L.; Kirikkaleli, D.; Hou, Y.; Miao, X.; Tufail, M. Carbon neutrality target for G7 economies: Examining the role of environmental policy, green innovation and composite risk index. J. Environ. Manag. 2021, 295, 113119. [Google Scholar]
- Espegren, K.; Damman, S.; Pisciella, P.; Graabak, I.; Tomasgard, A. The role of hydrogen in the transition from a petroleum economy to a low-carbon society. Int. J. Hydrogen Energy 2021, 46, 23125–23138. [Google Scholar]
- Muradov, N.Z.; Veziroğlu, T.N. “Green” path from fossil-based to hydrogen economy: An overview of carbon-neutral technologies. Int. J. Hydrogen Energy 2008, 33, 6804–6839. [Google Scholar]
- Winter, C. Hydrogen energy—Abundant, efficient, clean: A debate over the energy-system-of-change. Int. J. Hydrogen Energy 2009, 34, S1–S52. [Google Scholar]
- Acar, C.; Dincer, I. The potential role of hydrogen as a sustainable transportation fuel to combat global warming. Int. J. Hydrogen Energy 2020, 45, 3396–3406. [Google Scholar]
- Liu, F.; Zhao, F.; Liu, Z.; Hao, H. The impact of fuel cell vehicle deployment on road transport greenhouse gas emissions: The China case. Int. J. Hydrogen Energy 2018, 43, 22604–22621. [Google Scholar]
- Sherif, S.A.; Goswami, D.Y.; Stefanakos, E.L.; Steinfeld, A. (Eds.) Handbook of Hydrogen Energy; CRC Press: Boca Raton, FL, USA, 2014. [Google Scholar]
- Tarhan, C.; Çil, M.A. A study on hydrogen, the clean energy of the future: Hydrogen storage methods. J. Energy Storage 2021, 40, 102676. [Google Scholar]
- Kikukawa, S.; Yamaga, F.; Mitsuhashi, H. Risk assessment of Hydrogen fueling stations for 70 MPa FCVs. Int. J. Hydrogen Energy 2008, 33, 7129–7136. [Google Scholar]
- Chalk, S.G.; Miller, J.F.; Wagner, F.W. Challenges for fuel cells in transport applications. J. Power Source 2000, 86, 40–51. [Google Scholar]
- Bhogilla, S.S.; Niyas, H. Design of a hydrogen compressor for hydrogen fueling stations. Int. J. Hydrogen Energy 2019, 44, 29329–29337. [Google Scholar] [CrossRef]
- Weinert, J.X. A Near-Term Economic Analysis of Hydrogen Fueling Stations; Working Paper Series; Institute of Transportation Studies: Berkeley, CA, USA, 2005. [Google Scholar]
- Sdanghi, G.; Maranzana, G.; Celzard, A.; Fierro, V. Review of the current technologies and performances of hydrogen compression for stationary and automotive applications. Renew. Sustain. Energy Rev. 2019, 102, 150–170. [Google Scholar]
- Tian, Z.; Lv, H.; Zhou, W.; Zhang, C.; He, P. Review on equipment configuration and operation process optimization of hydrogen refueling station. Int. J. Hydrogen Energy 2022, 47, 3033–3053. [Google Scholar]
- Kermani, N.A.; Petrushina, I.; Nikiforov, A.; Jensen, J.O.; Rokni, M. Corrosion behavior of construction materials for ionic liquid hydrogen compressor. Int. J. Hydrogen Energy 2016, 41, 16688–16695. [Google Scholar]
- Reddi, K.; Elgowainy, A.; Sutherland, E. Hydrogen refueling station compression and storage optimization with tube-trailer deliveries. Int. J. Hydrogen Energy 2014, 39, 19169–19181. [Google Scholar] [CrossRef]
- Altukhov, S.M.; Kuznetsov, V.D.; Levin, G.A. Calculation of the volumetric characteristics of diaphragm compressors. Chem. Pet. Eng. 1971, 7, 671–675. [Google Scholar] [CrossRef]
- Lei, J.; Lin, Z.; Qian, Z.; Han, G.; Peng, L. Analysis of Diaphragm Compressor Exhausts Volume Decrease. In Proceedings of the 2013 Fifth International Conference on Measuring Technology and Mechatronics Automation, Hong Kong, China, 16–17 January 2013; pp. 233–235. [Google Scholar]
- Park, H.W.; Shin, Y.I.; Lee, Y.J.; Song, J.H.; Jeon, C.H. A Numerical Analysis on a Dependence of Hydrogen Diaphragm Compressor Performance on Hydraulic Oil Conditions. Trans. Korean Hydrog. New Energy Soc. 2009, 20, 471–478. [Google Scholar]
- Jia, X.; Zhao, Y.; Chen, J.; Peng, X. Research on the flowrate and diaphragm movement in a diaphragm compressor for a hydrogen refueling station. Int. J. Hydrogen Energy 2016, 41, 14842–14851. [Google Scholar]
- Ren, S.; Jia, X.; Jiang, J.; Zhang, S.; Zhao, B.; Peng, X. Effect of hydraulic oil compressibility on the volumetric efficiency of a diaphragm compressor for hydrogen refueling stations. Int. J. Hydrogen Energy 2022, 47, 15224–15235. [Google Scholar]
- Li, X.; Chen, J.; Wang, Z.; Jia, X.; Peng, X. A non-destructive fault diagnosis method for a diaphragm compressor in the hydrogen refueling station. Int. J. Hydrogen Energy 2019, 44, 24301–24311. [Google Scholar]
- Antonova, L.I.; Kalashnikov, V.F.; Degtyareva, T.S. Calculation of the reliability of the parametric and functional elements of diaphragm compressors. Chem. Pet. Eng. 1987, 23, 153–155. [Google Scholar]
- Lu, W.; Wang, X.; Cong, Y. Analysis on factors influencing diaphragm life of diaphragm compressors. China Sci. Technol. Overv. 2019, 4, 93–94. [Google Scholar]
- Jia, X.; Chen, J.; Wu, H.; Peng, X. Study on the diaphragm fracture in a diaphragm compressor for a hydrogen refueling station. Int. J. Hydrogen Energy 2016, 41, 6412–6421. [Google Scholar]
- Hu, Y.; Xu, X.; Wang, W. A new cavity profile for a diaphragm compressor used in hydrogen fueling stations. Int. J. Hydrogen Energy 2017, 42, 24458–24469. [Google Scholar]
- Li, J.Y.; Chen, J.H.; Zhao, H.L.; Wang, S.Y.; Jia, X.H. Theoretical analysis of diaphragm fracture in diaphragm compresssor. Eng. Mech. 2015, 32, 192–197. [Google Scholar]
- Li, J.; Jia, X.; Wu, Z.; Peng, X. The cavity profile of a diaphragm compressor for a hydrogen refueling station. Int. J. Hydrogen Energy 2014, 39, 3926–3935. [Google Scholar]
- Li, J.; Lin, L.; Jia, X.; Peng, X. A new generatrix of the cavity profile of a diaphragm compressor. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2014, 228, 1754–1766. [Google Scholar]
- Li, J.Y.; Jia, X.H.; Jiang, R.M.; Peng, X.Y. Optimization Design of Diaphragm Compressor Cavity Contour with New Generatrix. Compress. Technol. 2013, 6–10. [Google Scholar] [CrossRef]
- Li, J.; Zhao, X.; Jia, X.; Peng, X. Design and experimental verification for diaphragm compressor cavity profile by two exponential terms generatrix. J. Xi’an Jiaotong Univ. 2014, 48, 127–132. [Google Scholar]
- Wang, T.; Jia, X.; Li, X.; Ren, S.; Peng, X. Thermal-structural coupled analysis and improvement of the diaphragm compressor cylinder head for a hydrogen refueling station. Int. J. Hydrogen Energy 2020, 45, 809–821. [Google Scholar]
- Wang, T.; Tang, Z.; Jia, X. Study on the stress and deformation of a diaphragm compressor cylinder head under extreme conditions. IOP Conf. Ser. Mater. Sci. Eng. 2019, 604, 12029. [Google Scholar] [CrossRef]
- Arasteh, H.; Mashayekhi, R.; Ghaneifar, M.; Toghraie, D.; Afrand, M. Heat transfer enhancement in a counter-flow sinusoidal parallel-plate heat exchanger partially filled with porous media using metal foam in the channels’ divergent sections. J. Therm. Anal. Calorim. 2020, 141, 1669–1685. [Google Scholar]
- El-Shorbagy, M.A.; Eslami, F.; Ibrahim, M.; Barnoon, P.; Xia, W.; Toghraie, D. Numerical investigation of mixed convection of nanofluid flow in a trapezoidal channel with different aspect ratios in the presence of porous medium. Case Stud. Therm. Eng. 2021, 25, 100977. [Google Scholar]
- Ahmadi, G.; Toghraie, D.; Akbari, O.A. Technical and environmental analysis of repowering the existing CHP system in a petrochemical plant: A case study. Energy 2018, 159, 937–949. [Google Scholar]
- Yang, S.; Tao, W. Heat Transfer; Higher Education Press: Beijing, China, 2006; pp. 246–249. [Google Scholar]
- Barthélémy, H. Effects of pressure and purity on the hydrogen embrittlement of steels. Int. J. Hydrogen Energy 2011, 36, 2750–2758. [Google Scholar] [CrossRef]
- Shin, H.; Custodio, N.A.; Baek, U. Numerical analysis for characterizing hydrogen embrittlement behaviors induced in STS316L stainless steel using an in-situ small-punch test. Theor. Appl. Fract. Mech. 2021, 116, 103139. [Google Scholar]





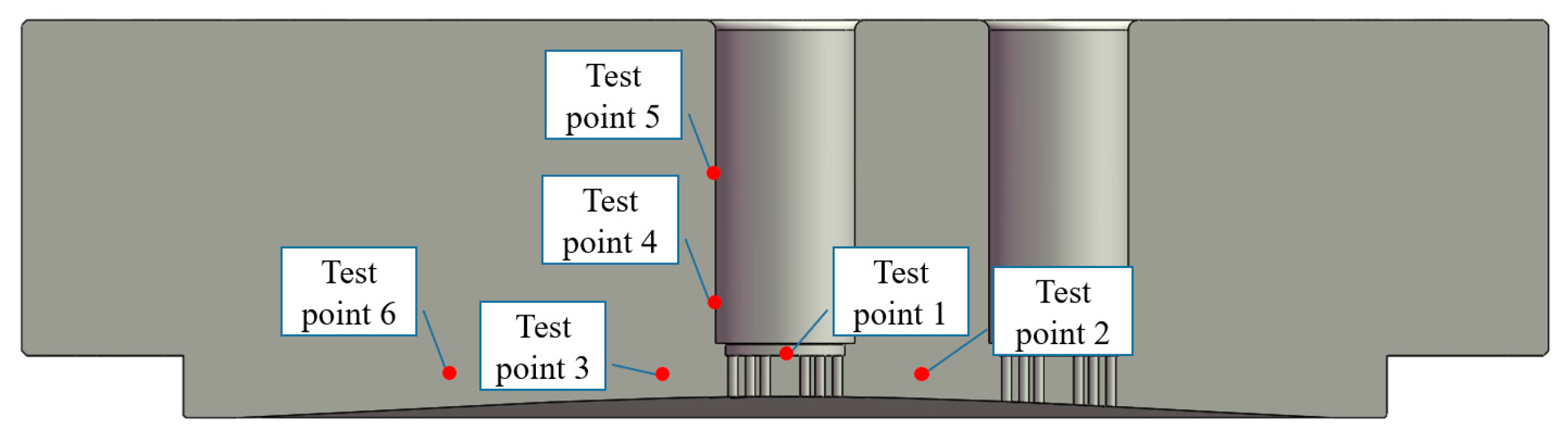




| Parameters | Values/mm |
|---|---|
| Diameter of the gas head cover | 615 |
| Thickness of the gas head cover | 160 |
| Diameter of the nozzles | 54 |
| Diameter of the hole in the gas head cover | 56 |
| Diameter of the suction and discharge small holes | 4 |
| Positions | Point 1 | Point 2 | Point 3 | Point 4 | Point 5 | Point 6 |
|---|---|---|---|---|---|---|
| Measured temperature (°C) | 165.2 | 123.5 | 132.3 | 146.3 | 134.2 | 124.6 |
| Simulated temperature (°C) | 162 | 134.8 | 142.5 | 138.3 | 125.1 | 135.4 |
| Deviation | 1.8% | 9.1% | 7.7% | 5.5% | 6.8% | 8.7% |
| Structures | No Heat Transfer Enhancement | Augmented Heat Transfer of Outer Surface | Augmented Heat Transfer of Core High-Temperature Zone |
|---|---|---|---|
| Measured discharge temperature (°C) | 171.5 | 169.2 | 155.4 |
| Simulated highest temperature (°C) | 162.2 | 160.4 | 148.2 |
| Point 1 Simulated/Measured (°C) | 162/165.2 | 160.1/164.7 | 147.9/147.2 |
| Point 2 Simulated/Measured (°C) | 134.8/123.5 | 121.9/112.5 | 88.6/82.6 |
| Point 3 Simulated/Measured (°C) | 142.5/132.3 | 127.4/121.8 | 93.9/89.6 |
| Point 4 Simulated/Measured (°C) | 138.3/146.3 | 122.2/131.1 | 46.8/50.2 |
| Point 5 Simulated/Measured (°C) | 125.1/134.2 | 72.7/75.1 | 37.9/40.3 |
| Point 6 Simulated/Measured (°C) | 135.4/124.6 | 117.7/105.5 | 119.6/111.9 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ren, S.; Jia, X.; Zhang, J.; Xin, D.; Peng, X. Analysis of Heat Transfer of the Gas Head Cover of Diaphragm Compressors for Hydrogen Refueling Stations. Processes 2023, 11, 2274. https://doi.org/10.3390/pr11082274
Ren S, Jia X, Zhang J, Xin D, Peng X. Analysis of Heat Transfer of the Gas Head Cover of Diaphragm Compressors for Hydrogen Refueling Stations. Processes. 2023; 11(8):2274. https://doi.org/10.3390/pr11082274
Chicago/Turabian StyleRen, Shengdong, Xiaohan Jia, Jiatong Zhang, Dianbo Xin, and Xueyuan Peng. 2023. "Analysis of Heat Transfer of the Gas Head Cover of Diaphragm Compressors for Hydrogen Refueling Stations" Processes 11, no. 8: 2274. https://doi.org/10.3390/pr11082274
APA StyleRen, S., Jia, X., Zhang, J., Xin, D., & Peng, X. (2023). Analysis of Heat Transfer of the Gas Head Cover of Diaphragm Compressors for Hydrogen Refueling Stations. Processes, 11(8), 2274. https://doi.org/10.3390/pr11082274








