Microparticles’ Lateral Oscillation Motion in Serpentine Micro-Channels without Inertial Lift Effects
Abstract
1. Introduction
2. Numerical Simulation Method
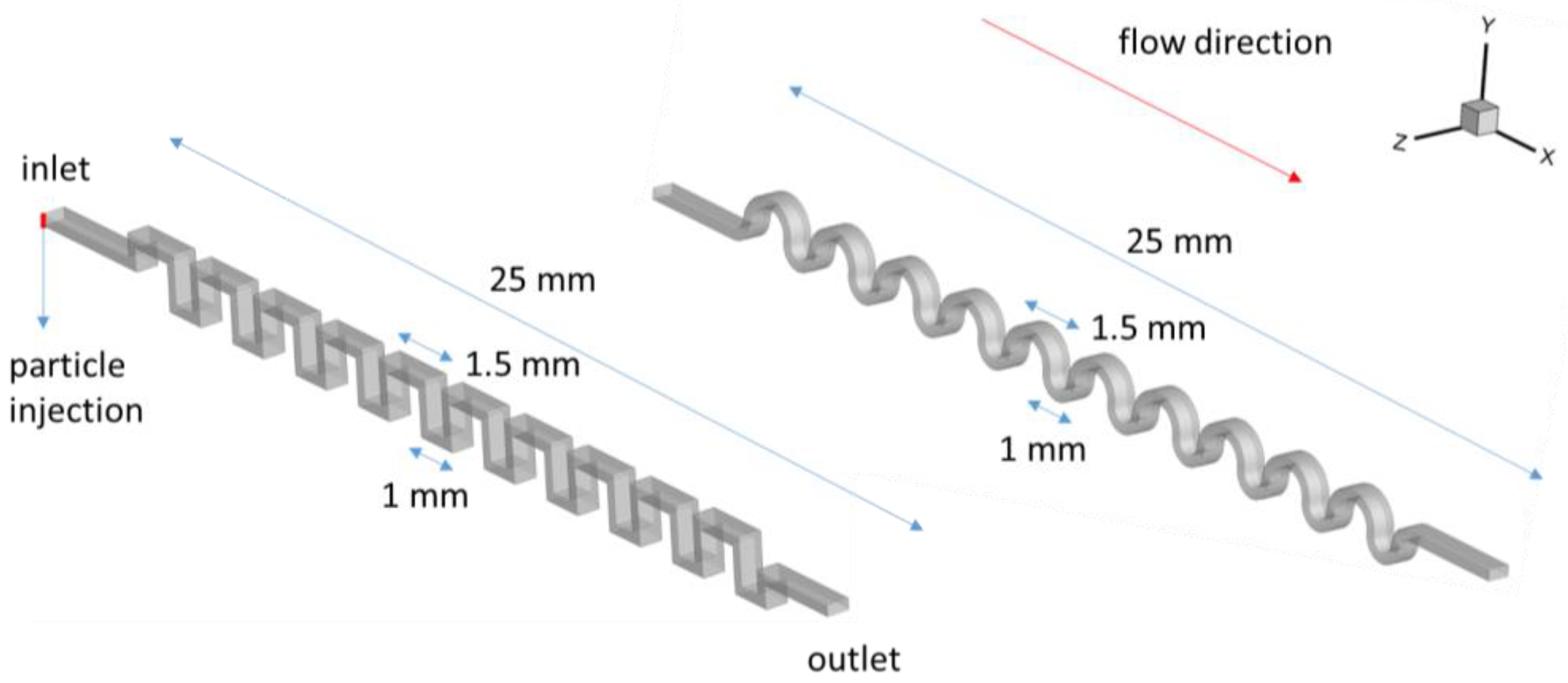
2.1. Calculation Scheme
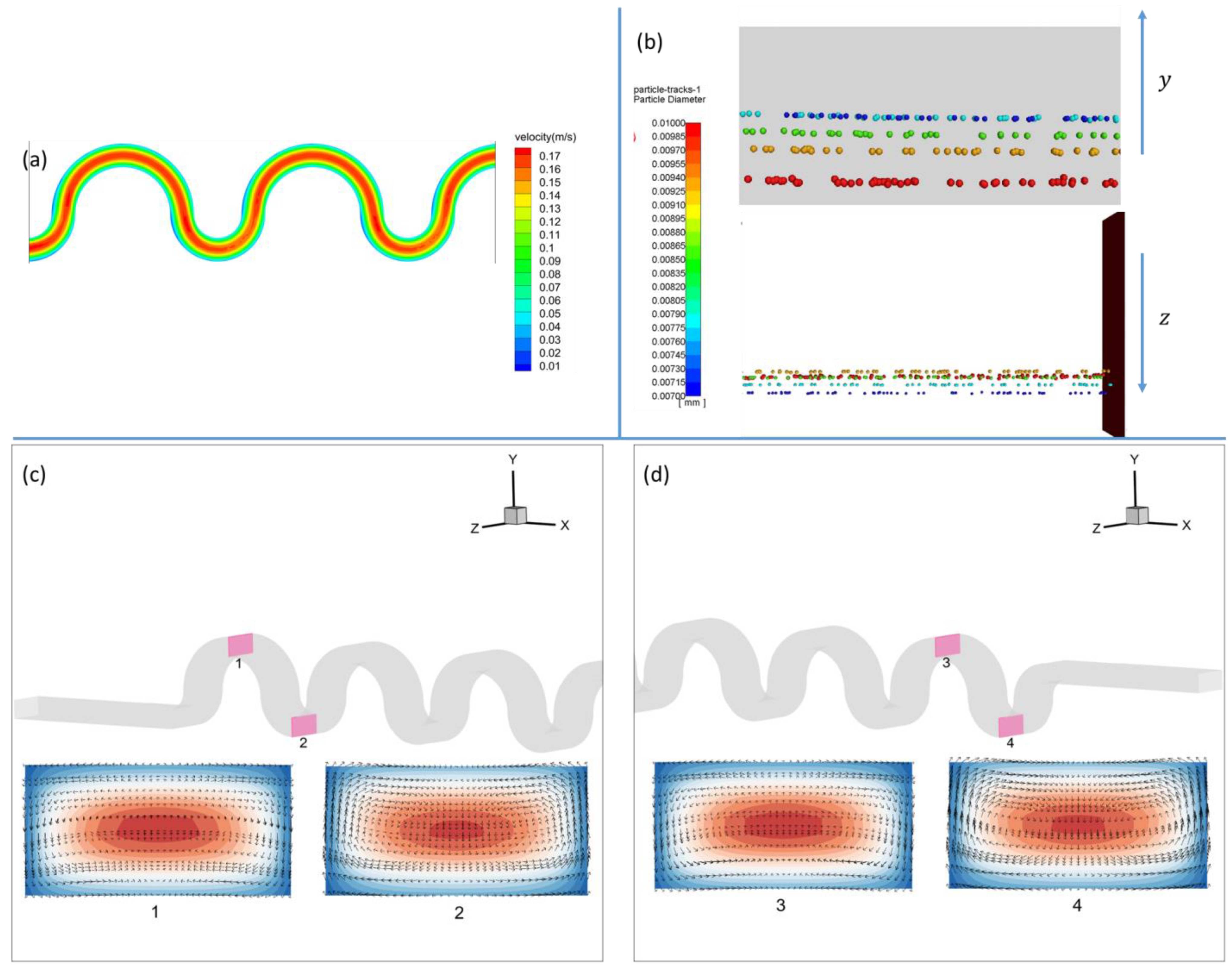
2.2. Software Settings and Physical Problem
2.3. Non-Newtonian Fluid
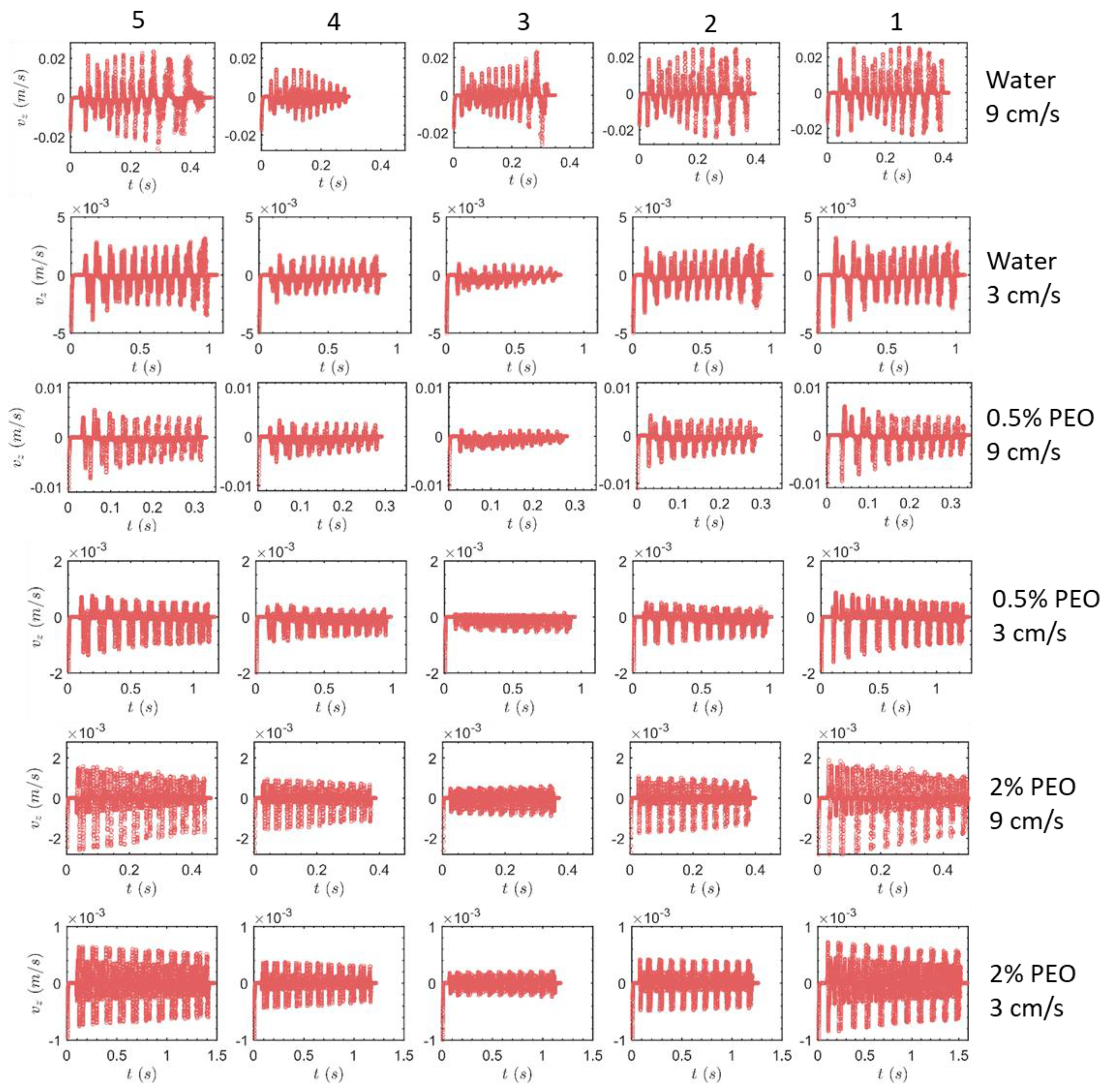
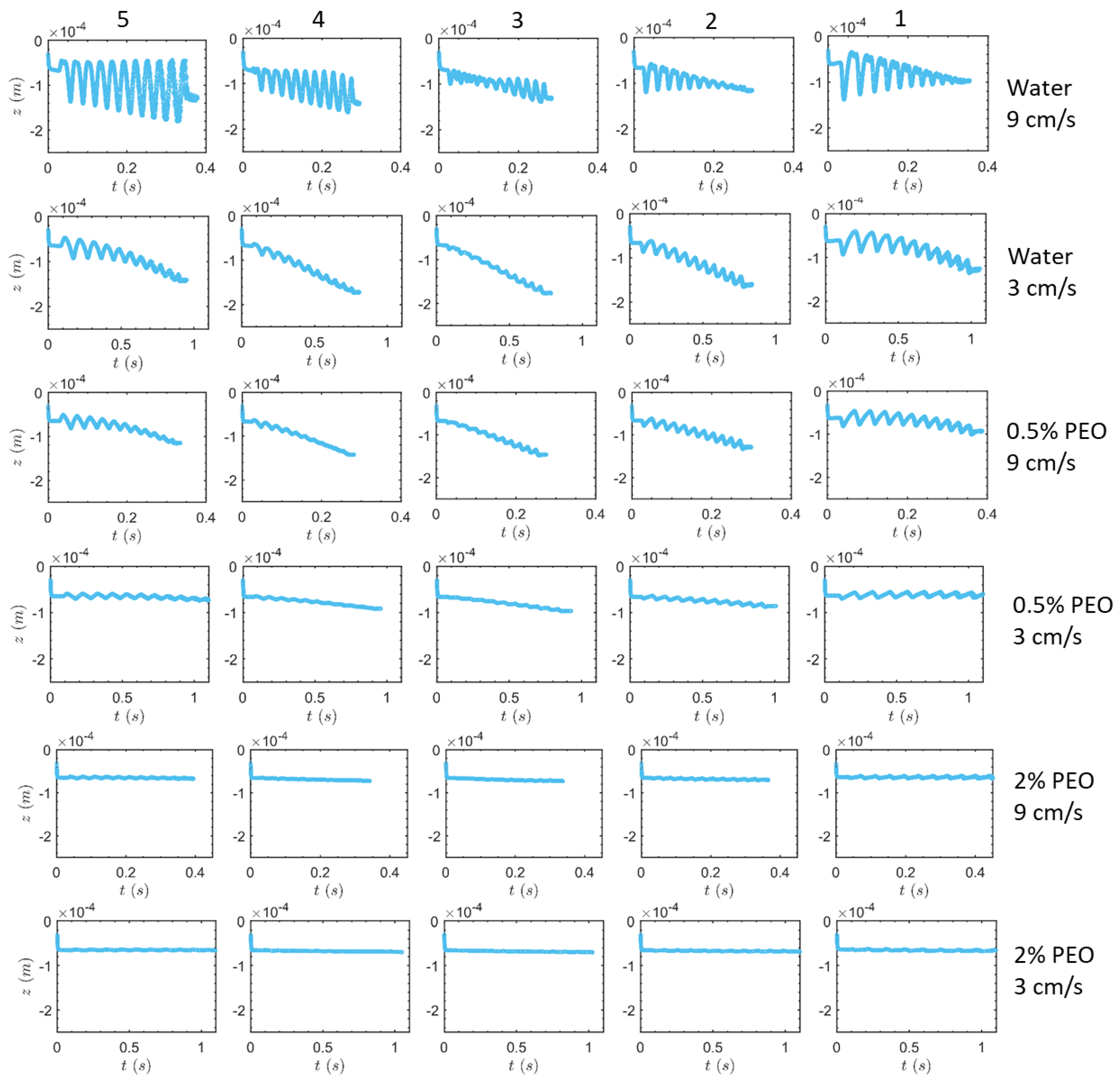
3. Results and Discussion
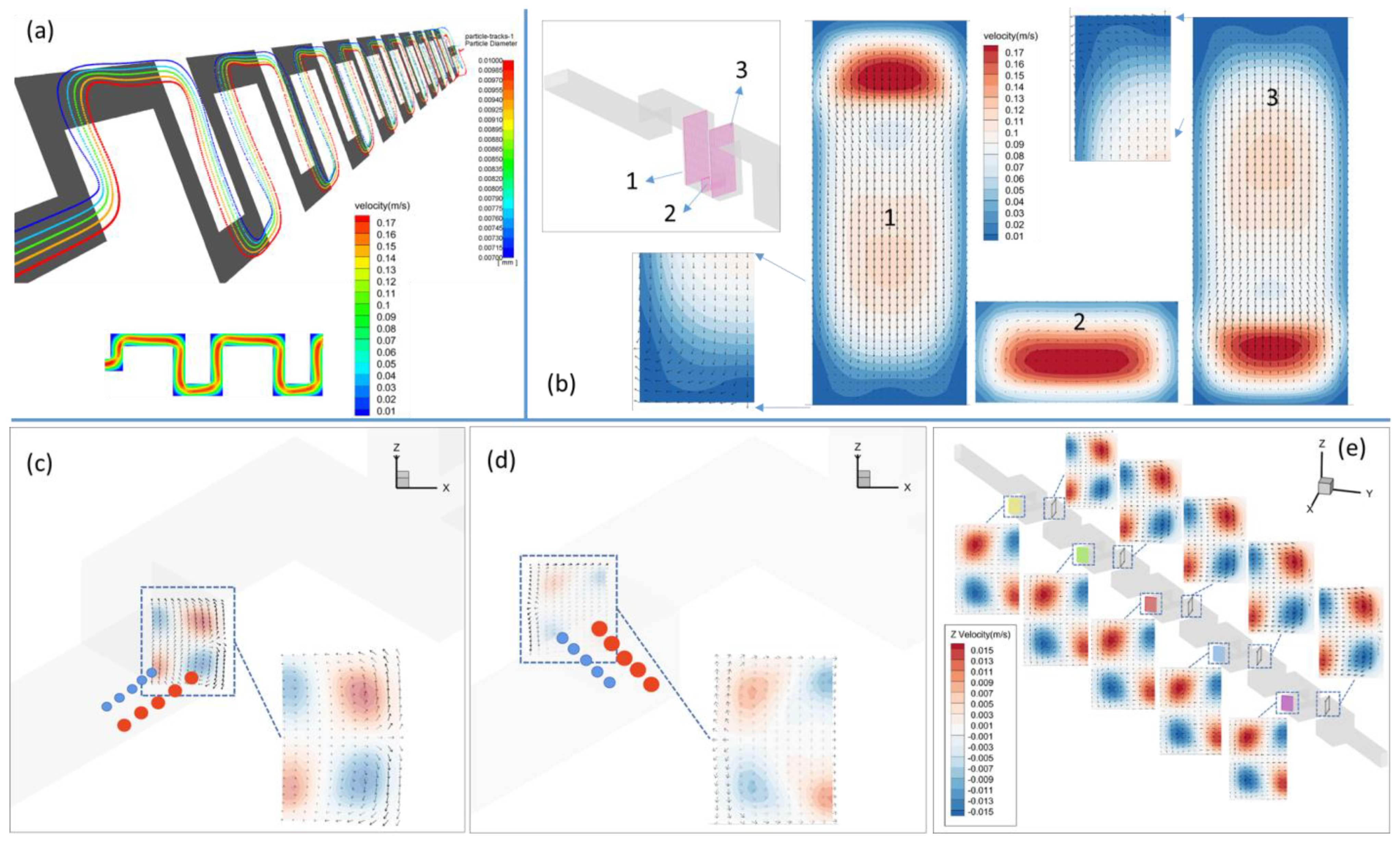
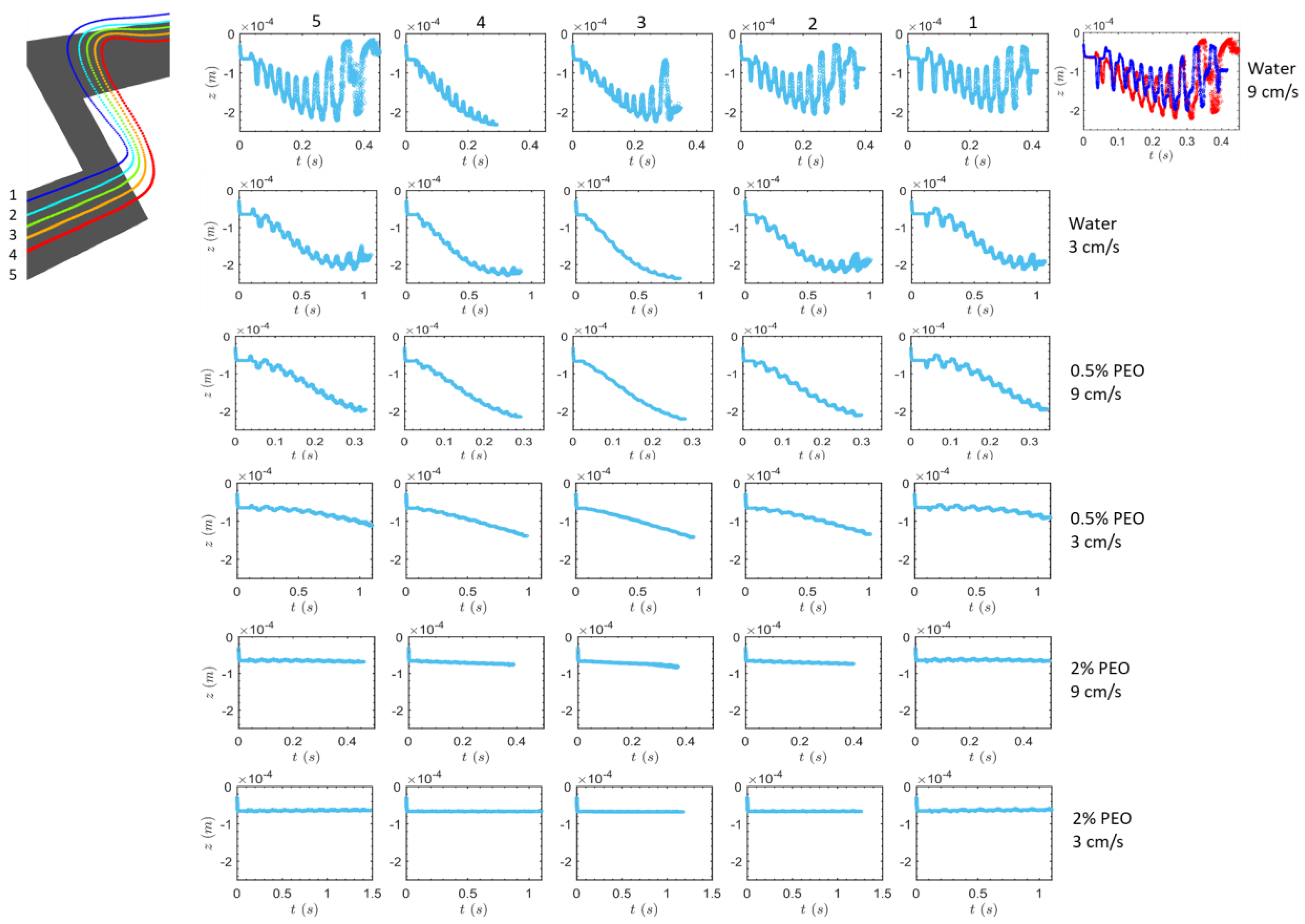
3.1. Transverse Motion
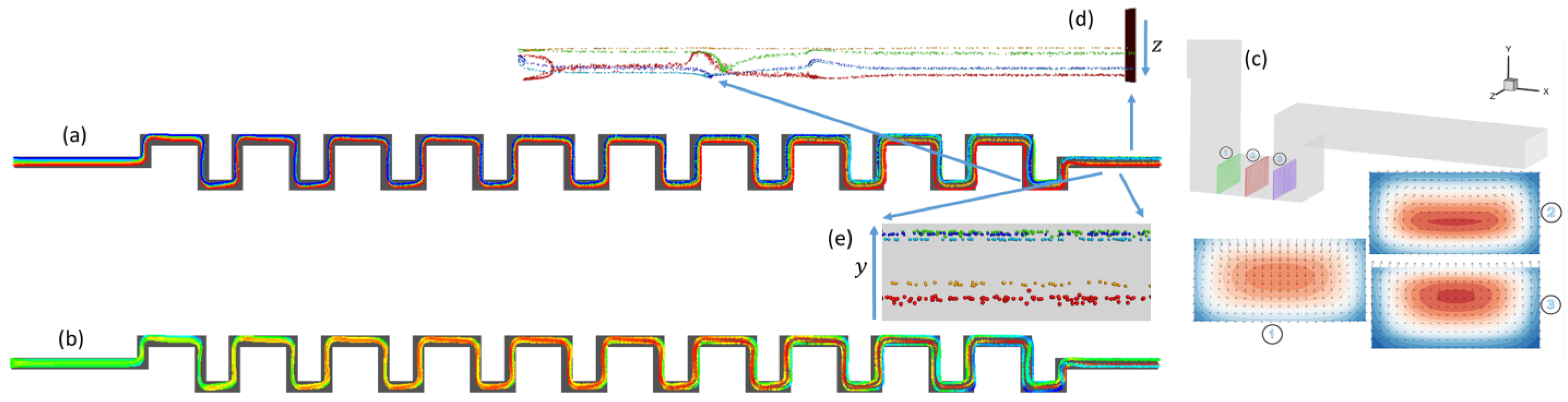
3.1.1. Square-Segment Serpentine Channel
3.1.2. Half-Circle-Segment Serpentine Channel
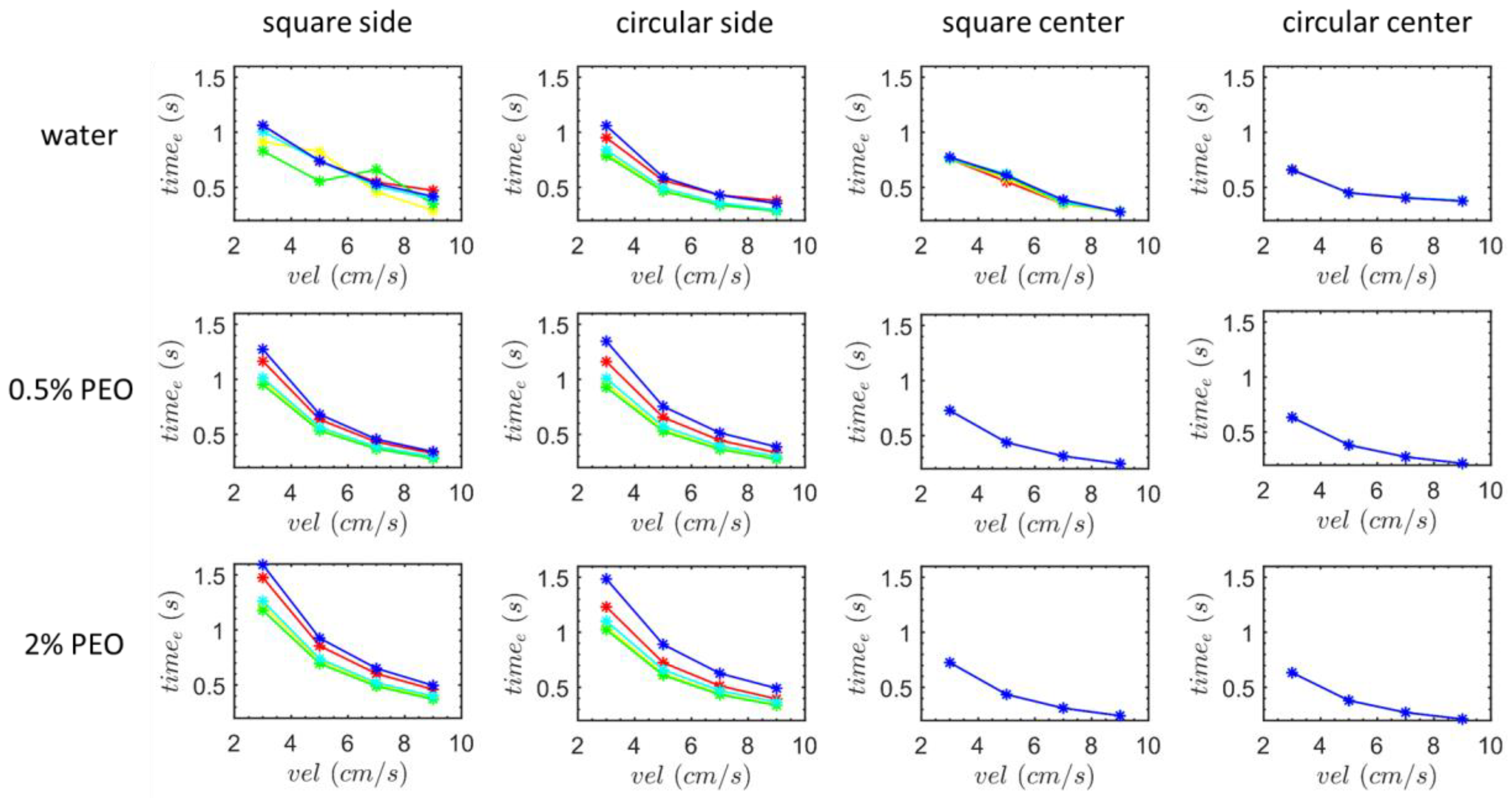
3.2. Particle Positions at the Outlet
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Inertial lift force | |
| Fluid density | |
| Maximum velocity | |
| Particle diameter | |
| Channel height | |
| Hydraulic radius | |
| Curvature radius | |
| Dimensionless inertial lift coefficient | |
| Particle mass | |
| Gravitational acceleration | |
| Particle velocity | |
| Moment of inertia of particles | |
| Interaction force between particles and fluid | |
| Collision contact force | |
| Angular velocity of particles | |
| Time | |
| Pressure gradient force | |
| Drag coefficient | |
| Particle volume | |
| Fluid velocity | |
| Volume fraction of fluid | |
| Fluid viscosity | |
| Normal component of the spring stiffness | |
| Tangential component of the spring stiffness | |
| Normal component of the damping coefficient | |
| Tangential component of the damping coefficient | |
| Normal overlap of the two particles | |
| Tangential overlap of the two particles | |
| Equivalent Young’s modulus | |
| Equivalent diameter | |
| Young’s modulus of particles | |
| Poisson’s ratio of particles | |
| Equivalent shear modulus | |
| Equivalent mass | |
| Fluid pressure | |
| Stress tensor of the fluid | |
| Particle density | |
| Reynolds number | |
| Dean number | |
| Elastic lift force | |
| Dimensionless coefficient of elastic lift | |
| First normal stress difference | |
| Viscosity of PEO at infinite shear rate | |
| Viscosity of PEO at zero shear rate | |
| Constant of the shear rate in the Cross model | |
| Shear rate | |
| Curvature ratio | |
| Radial fluid velocity | |
| Particle velocity in primary direction |
References
- Xu, J.; Liao, K.; Yang, X.; Wu, C.; Wu, W. Using single-cell sequencing technology to detect circulating tumor cells in solid tumors. Mol. Cancer 2021, 20, 104. [Google Scholar] [CrossRef]
- Xu, C.; He, X.; Ren, X.; Cheng, S. Direct detection of intracellular miRNA in living circulating tumor cells by tumor targeting nanoprobe in peripheral blood. Biosens. Bioelectron. 2021, 190, 113401. [Google Scholar] [CrossRef]
- Sinkala, E.; Sollier-Christen, E.; Renier, C.; Rosas-Canyelles, E.; Che, J.; Heirich, K.; Herr, A.E. Profiling protein expression in circulating tumour cells using microfluidic western blotting. Nat. Commun. 2017, 8, 14622. [Google Scholar] [CrossRef]
- Warkiani, M.E.; Khoo, B.L.; Wu, L.; Tay, A.K.P.; Bhagat, A.A.S.; Han, J.; Lim, C.T. Ultra-fast, label-free isolation of circulating tumor cells from blood using spiral microfluidics. Nat. Protoc. 2016, 11, 134–148. [Google Scholar] [CrossRef]
- Warkiani, M.E.; Guan, G.; Luan, K.B.; Lee, W.C.; Bhagat, A.A.S.; Chaudhuri, P.K.; Han, J. Slanted spiral microfluidics for the ultra-fast, label-free isolation of circulating tumor cells. Lab Chip 2014, 14, 128–137. [Google Scholar] [CrossRef] [PubMed]
- Jubery, T.; Srivastava, S.K.; Dutta, P. Dielectrophoretic separation of bioparticles in microdevices: A review. Electrophoresis 2014, 35, 691–713. [Google Scholar] [CrossRef]
- Zhang, H.; Chang, H.; Neuzil, P. DEP-on-a-chip: Dielectrophoresis applied to microfluidic platforms. Micromachines 2019, 10, 423. [Google Scholar] [CrossRef] [PubMed]
- Xuan, X. Recent advances in direct current electrokinetic manipulation of particles for microfluidic applications. Electrophoresis 2019, 40, 2484–2513. [Google Scholar] [CrossRef]
- Plouffe, B.D.; Murthy, S.K.; Lewis, L.H. Fundamentals and application of magnetic particles in cell isolation and enrichment: A review. Rep. Prog. Phys. 2014, 78, 016601. [Google Scholar] [CrossRef] [PubMed]
- Ding, X.; Lin, S.C.S.; Lapsley, M.I.; Li, S.; Guo, X.; Chan, C.Y.; Huang, T.J. Standing surface acoustic wave (SSAW) based multichannel cell sorting. Lab Chip 2012, 12, 4228–4231. [Google Scholar] [CrossRef] [PubMed]
- Liu, Y.; Lim, K.M. Particle separation in microfluidics using a switching ultrasonic field. Lab Chip 2011, 11, 3167–3173. [Google Scholar] [CrossRef] [PubMed]
- Carlo, D.D.; Irimia, D.; Tompkins, R.G.; Toner, M. Continuous inertial focusing, ordering, and separation of particles in microchannels. Proc. Natl. Acad. Sci. USA 2007, 104, 18892–18897. [Google Scholar] [CrossRef] [PubMed]
- Tang, W.; Zhu, S.; Jiang, D.; Zhu, L.; Yang, J.; Xiang, N. Channel innovations for inertial microfluidics. Lab Chip 2020, 20, 3485–3502. [Google Scholar] [CrossRef]
- Liu, C.; Guo, J.; Tian, F.; Yang, N.; Yan, F.; Ding, Y.; Sun, J. Field-free isolation of exosomes from extracellular vesicles by microfluidic viscoelastic flows. ACS Nano 2017, 11, 6968–6976. [Google Scholar] [CrossRef] [PubMed]
- Fallahi, H.; Zhang, J.; Phan, H.P.; Nguyen, N.T. Flexible microfluidics: Fundamentals, recent developments, and applications. Micromachines 2019, 10, 830. [Google Scholar]
- Liu, Y.; Zhao, W.; Cheng, R.; Puig, A.; Hodgson, J.; Egan, M.; Mao, L. Label-free inertial-ferrohydrodynamic cell separation with high throughput and resolution. Lab Chip 2021, 21, 2738–2750. [Google Scholar] [CrossRef]
- Wu, Z.; Chen, Y.; Wang, M.; Chung, A.J. Continuous inertial microparticle and blood cell separation in straight channels with local microstructures. Lab Chip 2016, 16, 532–542. [Google Scholar] [CrossRef]
- Sun, J.; Xianyu, Y.; Li, M.; Liu, W.; Zhang, L.; Liu, D.; Liu, C.; Hu, G.; Jiang, X. A microfluidic origami chip for synthesis of functionalized polymeric nanoparticles. Nanoscale 2013, 5, 5262–5265. [Google Scholar] [CrossRef]
- Bazaz, S.R.; Mihandust, A.; Salomon, R.; Joushani, H.A.N.; Li, W.; Amiri, H.A.; Warkiani, M.E. Zigzag microchannel for rigid inertial separation and enrichment (Z-RISE) of cells and particles. Lab Chip 2022, 22, 4093–4109. [Google Scholar] [CrossRef]
- Chung, A.J.; Gossett, D.R.; Carlo, D.D. Three dimensional, sheathless, and high-throughput microparticle inertial focusing through geometry-induced secondary flows. Small 2013, 9, 685–690. [Google Scholar] [CrossRef]
- Xiang, N.; Chen, K.; Dai, Q.; Jiang, D.; Sun, D.; Ni, Z. Inertia-induced focusing dynamics of microparticles throughout a curved microfluidic channel. Microfluid. Nanofluid. 2015, 18, 29–39. [Google Scholar] [CrossRef]
- Oakey, J.; Applegate, R.W.; Arellano, E.; Carlo, D.D.; Graves, S.W.; Toner, M. Particle focusing in staged inertial microfluidic devices for flow cytometry. Anal. Chem. 2010, 82, 3862–3867. [Google Scholar] [CrossRef] [PubMed]
- Chung, A.J.; Pulido, D.; Oka, J.C.; Amini, H.; Masaeli, M.; Carlo, D.D. Microstructure-induced helical vortices allow single-stream and long-term inertial focusing. Lab Chip 2013, 13, 2942–2949. [Google Scholar] [CrossRef] [PubMed]
- Segre, G.; Silberberg, A. Behaviour of macroscopic rigid spheres in Poiseuille flow Part 2. Experimental results and interpretation. J. Fluid Mech. 1962, 14, 136–157. [Google Scholar] [CrossRef]
- Segre, G.; Silberberg, A. Radial particle displacements in Poiseuille flow of suspensions. Nature 1961, 189, 209–210. [Google Scholar] [CrossRef]
- Amini, H.; Lee, W.; Di Carlo, D. Inertial microfluidic physics. Lab Chip 2014, 14, 2739–2761. [Google Scholar] [CrossRef] [PubMed]
- Shen, S.; Tian, C.; Li, T.; Xu, J.; Chen, S.W.; Tu, Q.; Wang, J. Spiral microchannel with ordered micro-obstacles for continuous and highly-efficient particle separation. Lab Chip 2017, 17, 3578–3591. [Google Scholar] [CrossRef]
- Ghadami, S.; Kowsari-Esfahan, R.; Saidi, M.S.; Firoozbakhsh, K. Spiral microchannel with stair-like cross section for size-based particle separation. Microfluid. Nanofluid. 2017, 21, 1–10. [Google Scholar] [CrossRef]
- Bhagat, A.A.S.; Kuntaegowdanahalli, S.S.; Papautsky, L. Enhanced particle filtration in straight microchannels using shear-modulated inertial migration. Phys. Fluids 2008, 20, 101702–101704. [Google Scholar] [CrossRef]
- Karampelas, I.H.; Gómez-Pastora, J. Novel approaches concerning the numerical modeling of particle and cell separation in microchannels: A review. Processes 2022, 10, 1226. [Google Scholar] [CrossRef]
- Seo, J.; Lean, M.H.; Kole, A. Membraneless microseparation by asymmetry in curvilinear laminar flows. J. Chromatogr. A 2007, 1162, 126–131. [Google Scholar] [CrossRef] [PubMed]
- Li, D.; Lu, X.; Song, Y.; Wang, J.; Li, D.; Xuan, X. Sheathless electrokinetic particle separation in a bifurcating microchannel. Biomicrofluidics 2016, 10, 054104. [Google Scholar] [CrossRef] [PubMed]
- Bhagat, A.A.S.; Kuntaegowdanahalli, S.S.; Papautsky, L. Inertial microfluidics for continuous particle filtration and extraction. Microfluid. Nanofluid. 2009, 7, 217–226. [Google Scholar] [CrossRef]
- Choi, Y.; Seo, K.; Lee, S. Lateral and cross-lateral focusing of spherical particles in a square microchannel. Lab Chip 2011, 11, 460–465. [Google Scholar] [CrossRef] [PubMed]
- Hur, S.; Henderson-MacLennan, N.K.; McCabe, E.R.B.; Carlo, D.D. Deformability-based cell classification and enrichment using inertial microfluidics. Lab Chip 2011, 11, 912–920. [Google Scholar] [CrossRef]
- Gossett, D.R.; Tse, H.; Dudani, J.; Goda, K.; Woods, T.A.; Graves, S.W.; Di Carlo, D. Inertial manipulation and transfer of microparticles across laminar fluid streams. Small 2012, 8, 2757–2764. [Google Scholar] [CrossRef]
- Di Carlo, D.; Edd, J.F.; Humphry, K.J.; Stone, H.A.; Toner, M. Particle Segregation and Dynamics in Confined Flows. Phys. Rev. Lett. 2009, 102, 1–4. [Google Scholar] [CrossRef]
- Hur, S.; Tse, H.; Di Carlo, D. Sheathless inertial cell ordering for extreme throughput flow cytometry. Lab Chip 2010, 10, 274–280. [Google Scholar] [CrossRef]
- Vasseur, P.; Cox, R.G. The lateral migration of a spherical particle in two-dimensional shear flows. J. Fluid Mech. 1976, 78, 385–413. [Google Scholar] [CrossRef]
- Feng, J.; Hu, H.H.; Joseph, D.D. Direct simulation of initial value problems for the motion of solid bodies in a Newtonian fluid Part 1. Sedimentation. J. Fluid Mech. 1994, 261, 95–134. [Google Scholar] [CrossRef]
- Edd, J.F.; Di Carlo, D.; Humphry, K.J.; Köster, S.; Irimia, D.; Weitz, D.A.; Toner, M. Controlled encapsulation of single-cells into monodisperse picolitre drops. Lab Chip 2008, 8, 1262–1264. [Google Scholar] [CrossRef]
- Gossett, D.R.; Di Carlo, D. Particle focusing mechanisms in curving confined flows. Anal. Chem. 2009, 81, 8459–8465. [Google Scholar] [CrossRef]
- Lee, M.G.; Shin, J.H.; Bae, C.Y.; Choi, S.; Park, J.K. Label-free cancer cell separation from human whole blood using inertial microfluidics at low shear stress. Anal. Chem. 2013, 85, 6213–6218. [Google Scholar] [CrossRef]
- Zhang, J.; Li, W.; Li, M.; Alici, G.; Nguyen, N.T. Particle inertial focusing and its mechanism in a serpentine microchannel. Microfluid. Nanofluid. 2014, 17, 305–316. [Google Scholar] [CrossRef]
- Jiang, D.; Huang, D.; Zhao, G.; Tang, W.; Xiang, N. Numerical simulation of particle migration in different contraction–expansion ratio microchannels. Microfluid. Nanofluid. 2019, 23, 7. [Google Scholar] [CrossRef]
- Elghobashi, S. On predicting particle-laden turbulent flows. Appl. Sci. Res. 1994, 52, 309–329. [Google Scholar] [CrossRef]
- Zhang, S.; Zhang, B.; Liu, X.; Shan, J. Application of a particle-grid hybrid method in multiphase flow calculation. J. Nucl. Sci. Technol. 2020, 57, 1199–1214. [Google Scholar] [CrossRef]
- Kidanemariam, A.G.; Uhlmann, M. Formation of sediment patterns in channel flow: Minimal unstable systems and their temporal evolution. J. Fluid Mech. 2017, 818, 716–743. [Google Scholar] [CrossRef]
- Fernandes, C.; Semyonov, D.; Ferrás, L.L.; Nóbrega, J.M. Validation of the CFD-DPM solver DPMFoam in OpenFOAM® through analytical, numerical and experimental comparisons. Granul. Matter 2018, 20, 64. [Google Scholar] [CrossRef]
- Sippola, P.; Kolehmainen, J.; Ozel, A.; Liu, X.; Saarenrinne, P.; Sundaresan, S. Experimental and numerical study of wall layer development in a tribocharged fluidized bed. J. Fluid Mech. 2018, 849, 860–884. [Google Scholar] [CrossRef]
- Shalaby, H.; Wozniak, K.; Wozniak, G. Numerical calculation of particle-laden cyclone separator flow using LES. Eng. Appl. Comput. Fluid Mech. 2008, 2, 382–392. [Google Scholar] [CrossRef]
- Song, C.; Pei, B.; Jiang, M.; Wang, B.; Xu, D.; Chen, Y. Numerical analysis of forces exerted on particles in cyclone separators. Powder Technol. 2016, 294, 437–448. [Google Scholar] [CrossRef]
- Liu, C.; Hu, G.; Jiang, X.; Sun, J. Inertial focusing of spherical particles in rectangular microchannels over a wide range of Reynolds numbers. Lab Chip 2015, 15, 1168–1177. [Google Scholar] [CrossRef]
- Zhu, H.; Zhou, Z.; Yang, R.; Yu, A. Discrete particle simulation of particulate systems: A review of major applications and findings. Chem. Eng. Sci. 2008, 63, 5728–5770. [Google Scholar]
- Tsuji, Y.; Tanaka, T.; Ishida, T. Lagrangian numerical simulation of plug flow of cohesionless particles in a horizontal pipe. Powder Technol. 1992, 71, 239–250. [Google Scholar] [CrossRef]
- Mindlin, R.D. Compliance of elastic bodies in contact. J. Appl. Mech. 1949, 16, 259–268. [Google Scholar] [CrossRef]
- Lien, C.C.; Wu, M.C.; Ay, C. Study on the Young’s modulus of red blood cells using atomic force microscope. Appl. Mech. Mater. 2014, 627, 197–201. [Google Scholar] [CrossRef]
- Javanmardi, Y.; Colin-York, H.; Szita, N.; Fritzsche, M.; Moeendarbary, E. Quantifying cell-generated forces: Poisson’s ratio matters. Commun. Phys. 2021, 4, 237. [Google Scholar] [CrossRef]
- Grover, W.H.; Bryan, A.K.; Diez-Sliva, M.; Manalis, S.R. Measuring single-cell density. Proc. Natl. Acad. Sci. USA 2011, 108, 10992–10996. [Google Scholar] [CrossRef]
- Liu, C.; Xue, C.; Chen, X.; Shan, L.; Tian, Y.; Hu, G. Size-based separation of particles and cells utilizing viscoelastic effects in straight microchannels. Anal. Chem. 2015, 87, 6041–6048. [Google Scholar] [CrossRef]
- Crowley, M.M.; Zhang, F.; Koleng, J.J.; McGinity, J.W. Stability of polyethylene oxide in matrix tablets prepared by hot-melt extrusion. Biomaterials 2002, 23, 4241–4248. [Google Scholar] [CrossRef] [PubMed]
- Ebagninin, K.W.; Benchabane, A.; Bekkour, K. Rheological characterization of poly (ethylene oxide) solutions of different molecular weights. J. Colloid Interface Sci. 2009, 336, 360–367. [Google Scholar] [CrossRef] [PubMed]
- Zhu, J.; Tzeng, T.R.; Hu, G.; Xuan, X. DC dielectrophoretic focusing of particles in a serpentine microchannel. Microfluid. Nanofluid. 2009, 7, 751–756. [Google Scholar] [CrossRef]



| Concentration (%) | ||||
|---|---|---|---|---|
| 0.5 | 0.0006 | 0.79 | 0.005 | 0.003 |
| 2 | 0.0033 | 0.73 | 0.049 | 0.008 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, Y.; Hu, X.; Ma, J.; Gao, F.; Gao, Y.; Yan, L. Microparticles’ Lateral Oscillation Motion in Serpentine Micro-Channels without Inertial Lift Effects. Processes 2023, 11, 2411. https://doi.org/10.3390/pr11082411
Liu Y, Hu X, Ma J, Gao F, Gao Y, Yan L. Microparticles’ Lateral Oscillation Motion in Serpentine Micro-Channels without Inertial Lift Effects. Processes. 2023; 11(8):2411. https://doi.org/10.3390/pr11082411
Chicago/Turabian StyleLiu, Yang, Xintao Hu, Jiayuan Ma, Feng Gao, Yanan Gao, and Linbo Yan. 2023. "Microparticles’ Lateral Oscillation Motion in Serpentine Micro-Channels without Inertial Lift Effects" Processes 11, no. 8: 2411. https://doi.org/10.3390/pr11082411
APA StyleLiu, Y., Hu, X., Ma, J., Gao, F., Gao, Y., & Yan, L. (2023). Microparticles’ Lateral Oscillation Motion in Serpentine Micro-Channels without Inertial Lift Effects. Processes, 11(8), 2411. https://doi.org/10.3390/pr11082411





