Differential Evolution of Reservoir Permeability Under Dip Angle Control During Coalbed Methane Production
Abstract
1. Introduction
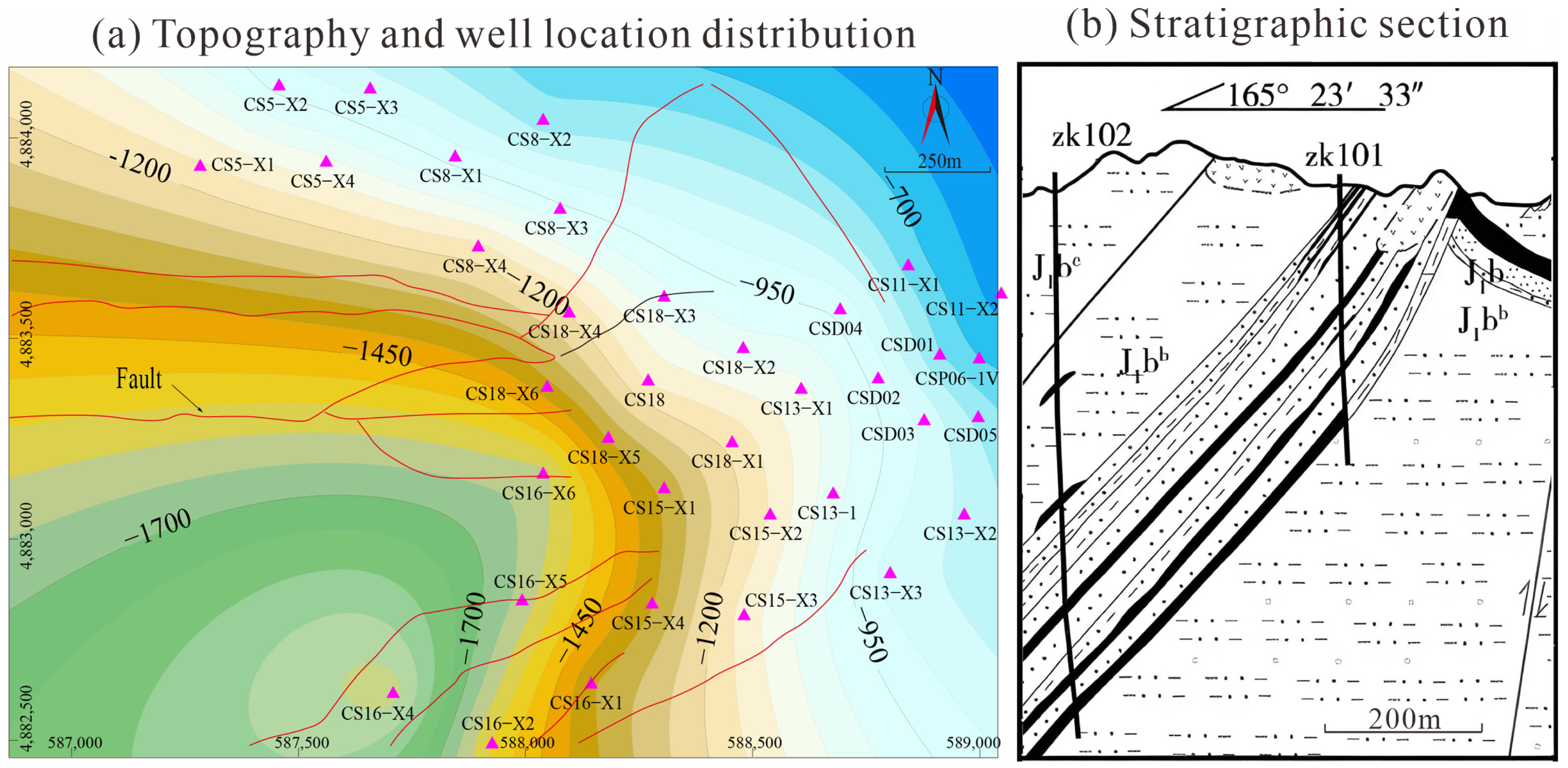
2. Research Background
3. Simulation for CBM Production
3.1. Evolution of Permeability During Gas Production
3.2. Parameter Determination
4. Results and Discussion
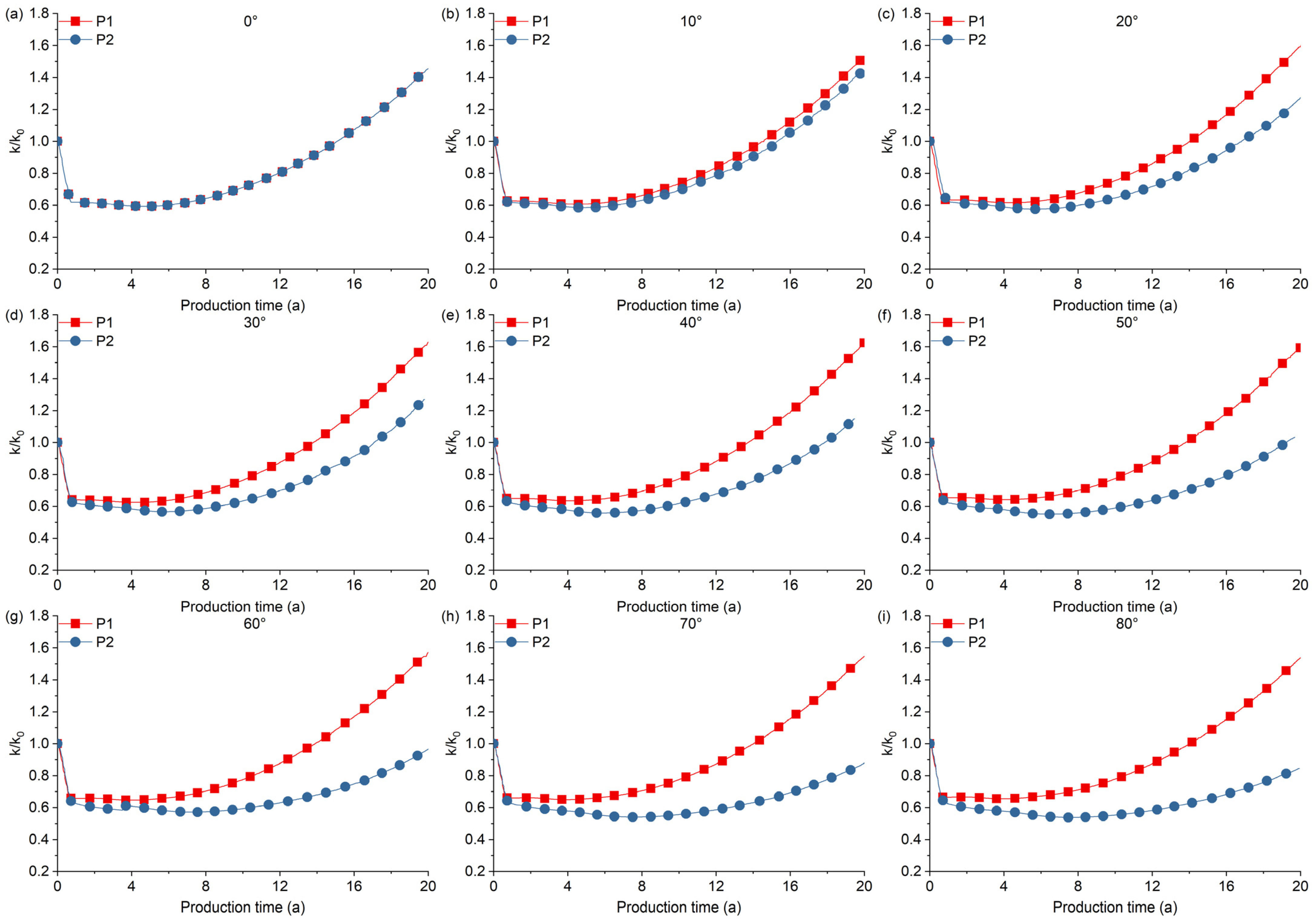
4.1. Spatio-Temporal Evolution Characteristics of Permeability in SICRs
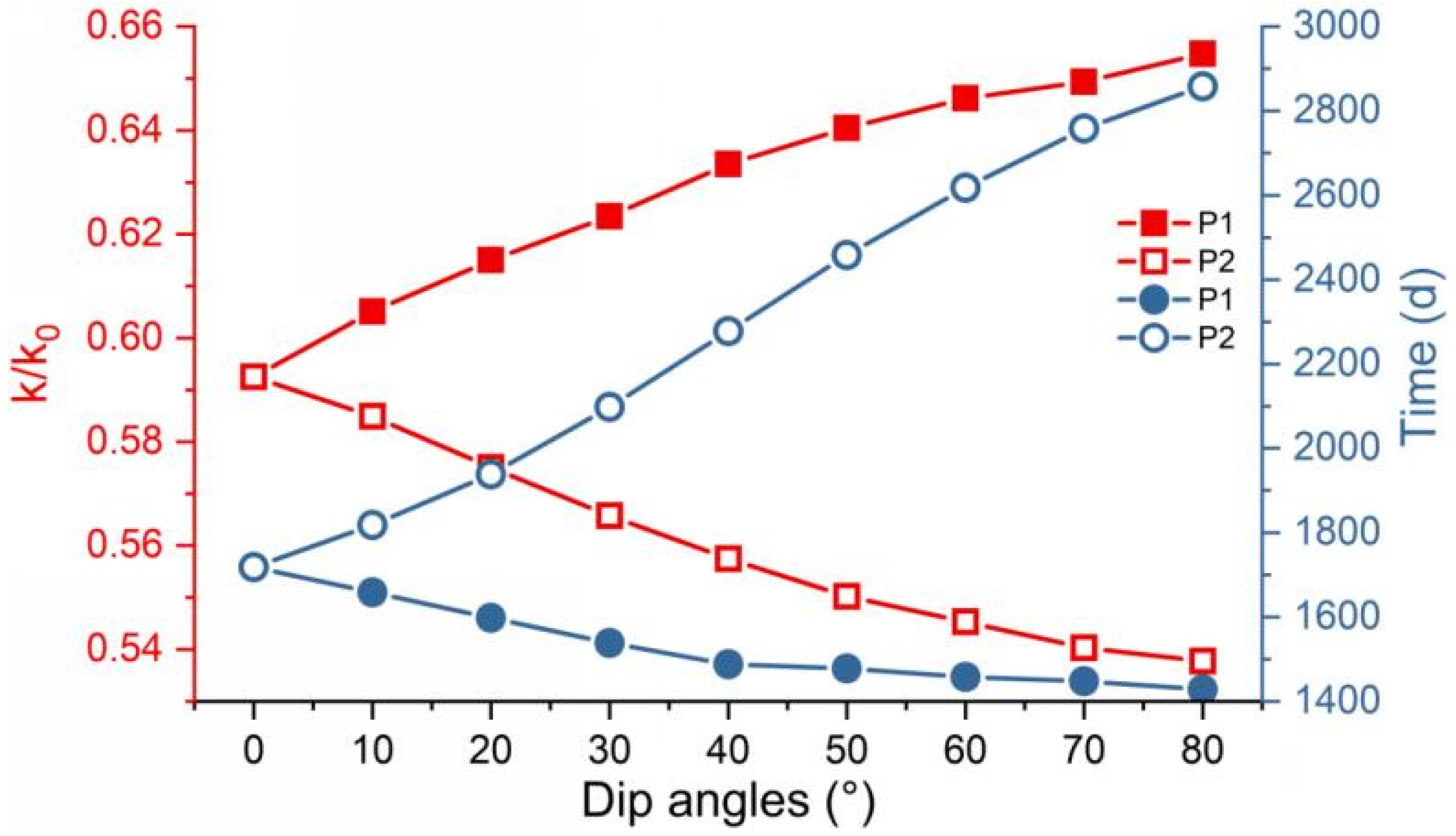
4.2. Different Characteristics of Permeability in Reservoirs with Different Dip Angles
4.3. Influence of Permeability Change on Gas Production
4.4. Evolution Mechanism of Permeability Difference in SICRs
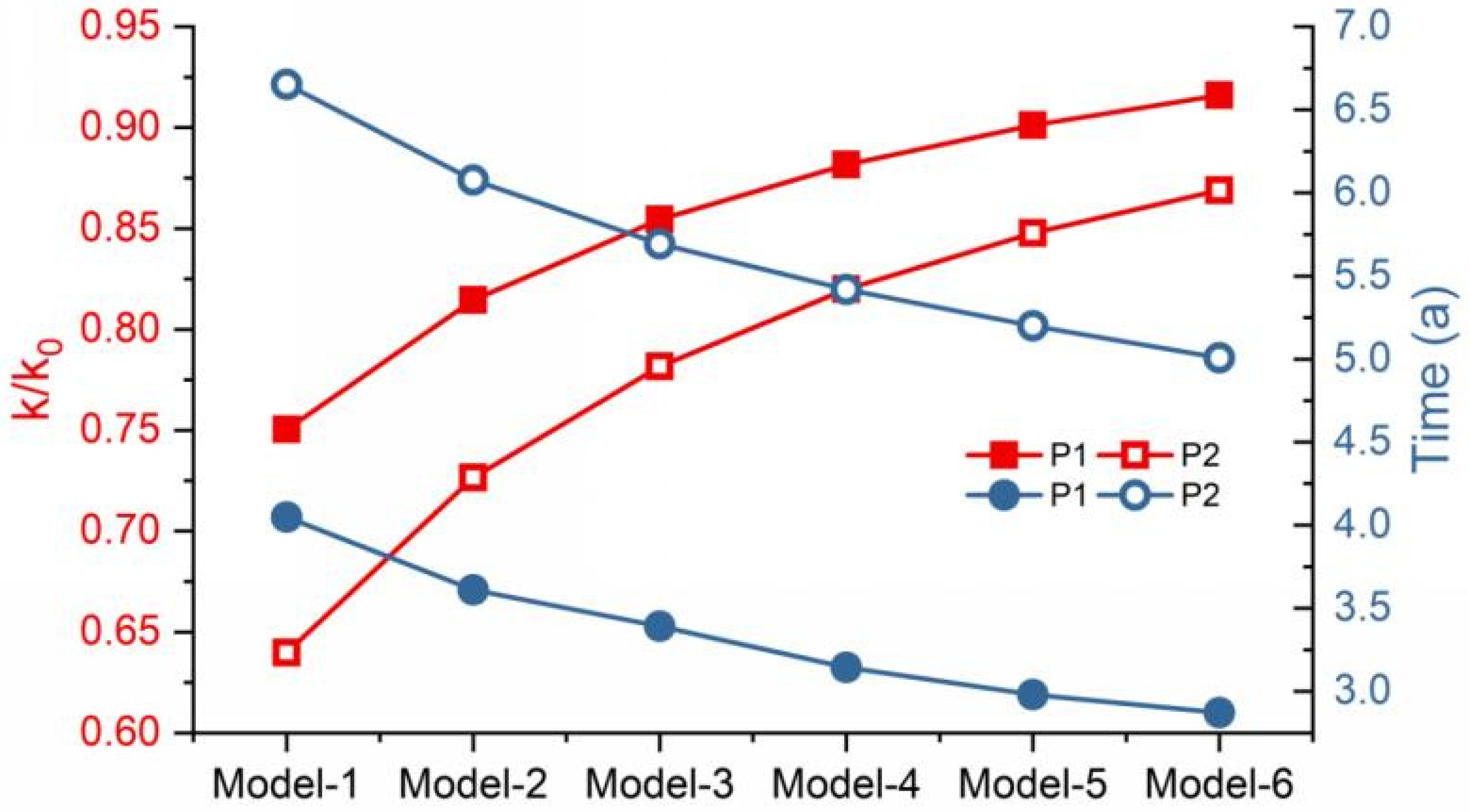
4.5. Influence of Elastic Mechanical Parameters on Permeability
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| SICR | steeply inclined coal reservoir | v | Poisson’s ratio | V | gas content in wellbore |
| UD | up-dip direction | β | strain pressure | Vgra | gas content gradient |
| DD | down-dip direction | kD | k0x vs. depth | VL | Langmuir volume |
| ES | effective stress | φf0 | porosity | pL | Langmuir pressure |
| MS | matrix shrinkage | τ | desorption time | k0x | permeability in X direction |
| k | real-time permeability | ε | Langmuir strain constant | k0y | permeability in Y direction |
| k0 | initial permeability | β | Langmuir strain constant | k0y | permeability in Z direction |
| p | reservoir pressure | μg | dynamic viscosity of CH4 | Sw | initial water saturation |
| p0 | initial reservoir pressure | μw | dynamic viscosity of water | Swr | irreducible water saturation |
| pc | critical desorption pressure | H | burial depth | Sgr | residual gas saturation |
| ε | maximum adsorption strain | h | coal thickness | η | tortuosity coefficient |
| E | Young’s modulus | pgra | reservoir pressure gradient | ρc | coal density |
References
- Luo, X.; Wang, Z.; Zhang, L.; Yang, W.; Liu, L. Overpressure generation and evolution in a compressional tectonic setting, the southern margin of Junggar basin, northwestern China. AAPG Bull. 2007, 91, 1123–1139. [Google Scholar] [CrossRef]
- Li, X.; Fu, X.; Yang, X.; Ge, Y.; Quan, F. Coalbed methane accumulation and dissipation patterns: A case study of the Junggar Basin, NW China. J. Asian Earth Sci. 2018, 160, 13–26. [Google Scholar] [CrossRef]
- Song, Y.; Liu, H.; Hong, F.; Qin, S.; Liu, S.; Li, G.; Zhao, M. Syncline reservoir pooling as a general model for coalbed methane (CBM) accumulations: Mechanisms and case studies. J. Pet. Sci. Eng. 2012, 88, 5–12. [Google Scholar] [CrossRef]
- Kang, J.; Fu, X.; Gao, L.; Liang, S. Production profile characteristics of large dip angle coal reservoir and its impact on coalbed methane production: A case study on the Fukang west area, southern Junggar Basin, China. J. Pet. Sci. Eng. 2018, 171, 99–114. [Google Scholar] [CrossRef]
- Kang, J.; Fu, X.; Liang, S.; Li, X.; Chen, X.; Wang, Z. A numerical simulation study on the characteristics of the gas production profile and its formation mechanisms for different dip angles in coal reservoirs. J. Pet. Sci. Eng. 2019, 181, 106198. [Google Scholar] [CrossRef]
- Pan, Z.; Connell, L.; Camilleri, M. Laboratory characterisation of coal reservoir permeability for primary and enhanced coalbed methane recovery. Int. J. Coal Geol. 2010, 82, 252–261. [Google Scholar] [CrossRef]
- Pan, Z.; Connell, L. Modelling of anisotropic coal swelling and its impact on permeability behaviour for primary and enhanced coalbed methane recovery. Int. J. Coal Geol. 2011, 85, 257–267. [Google Scholar] [CrossRef]
- Liu, J.; Chen, Z.; Elsworth, D.; Miao, X.; Mao, X. Evolution of coal permeability from stress-controlled to displacement-controlled swelling conditions. Fuel 2011, 90, 2987–2997. [Google Scholar] [CrossRef]
- Tao, S.; Wang, Y.; Tang, D.; Xu, H.; Lv, Y.; He, W.; Li, Y. Dynamic variation effects of coal permeability during the coalbed methane development process in the Qinshui Basin, China. Int. J. Coal Geol. 2012, 93, 16–22. [Google Scholar] [CrossRef]
- Liang, S.; Han, H.; Elsworth, D.; Fu, X.; Yao, Q.; Kang, J.; Li, X.; Li, X. Evolution of production and transport characteristics of steeply-dipping ultra-thick coalbed methane reservoirs. Energies 2020, 13, 5081. [Google Scholar] [CrossRef]
- Qin, Q.; Li, X.; Peng, R.; Peng, S.; Liu, Y.; Zhou, X.; Yang, J. Coalbed methane reservoir dynamic simulation comparisons using the actual steeply inclined model and ideal steeply inclined model. Front. Earth Sci. 2022, 10, 999516. [Google Scholar] [CrossRef]
- Pan, Z.; Connell, L. Modelling permeability for coal reservoirs: A review of analytical models and testing data. Int. J. Coal Geol. 2012, 92, 1–44. [Google Scholar] [CrossRef]
- Eclipse. User’s Guide and Reference Guide; Eclipse Version 2013; Eclipse: Brussels, Belgium, 2013. [Google Scholar]
- CMG. User’s Guide and Reference Guide; CMG Version 2019; CMG: Beijing, China, 2019. [Google Scholar]
- Robertson, E.; Christiansen, R. A permeability model for coal and other fractured, sorptive-elastic media. SPE J. 2008, 13, 314–324. [Google Scholar] [CrossRef]
- Meng, Y.; Liu, S.; Li, Z. Experimental study on sorption induced strain and permeability evolutions and their implications in the anthracite coalbed methane production. J. Pet. Sci. Eng. 2018, 164, 515–522. [Google Scholar] [CrossRef]
- Wu, Y.; Liu, J.; Elsworth, D.; Miao, X.; Mao, X. Development of anisotropic permeability during coalbed methane production. J. Nat. Gas Sci. Eng. 2010, 2, 197–210. [Google Scholar] [CrossRef]
- Zou, M.; Wei, C.; Yu, H.; Song, L. Modeling and application of coalbed methane recovery performance based on a triple porosity/dual permeability model. J. Nat. Gas Sci. Eng. 2015, 22, 679–688. [Google Scholar] [CrossRef]
- Sun, Z.; Shi, J.; Zhang, T.; Wu, K.; Feng, D.; Sun, F.; Huang, L.; Hou, C.; Li, X. A fully-coupled semi-analytical model for effective gas/water phase permeability during coal-bed methane production. Fuel 2018, 223, 44–52. [Google Scholar] [CrossRef]
- Pashin, J. Variable gas saturation in coalbed methane reservoirs of the black warrior basin: Implications for exploration and production. Int. J. Coal Geol. 2010, 82, 135–146. [Google Scholar] [CrossRef]
- Palmer, I.; Mansoori, J. How permeability depends on stress and pore pressure in coalbeds: A new model. In Proceedings of the SPE Annual Technical Conference and Exhibition, Denver, CO, USA, 6–9 October 1996. [Google Scholar] [CrossRef]
- Fan, C.; Elsworth, D.; Li, S.; Zhou, L.; Yang, Z.; Song, Y. Thermo-hydro-mechanical- chemical couplings controlling CH4 production and CO2 sequestration in enhanced coalbed methane recovery. Energy 2019, 173, 1054–1077. [Google Scholar] [CrossRef]
- Fan, C.; Elsworth, D.; Li, S.; Chen, Z.; Luo, M.; Song, Y.; Zhang, H. Modelling and optimization of enhanced coalbed methane recovery using CO2/N2 mixtures. Fuel 2019, 253, 1114–1129. [Google Scholar] [CrossRef]
- Omotilewa, O.; Panja, P.; Vega-Ortiz, C.; McLennan, J. Evaluation of enhanced coalbed methane recovery and carbon dioxide sequestration potential in high volatile bituminous coal. J. Nat. Gas Sci. Eng. 2021, 91, 103979. [Google Scholar] [CrossRef]
- Wang, J.; Kabir, A.; Liu, J.; Chen, Z. Effects of non-Darcy flow on the performance of coal seam gas wells. Int. J. Coal Geol. 2012, 93, 62–74. [Google Scholar] [CrossRef]
- Ostojic, J.; Rezaee, R.; Bahrami, H. Production performance of hydraulic fractures in tight gas sands, a numerical simulation approach. J. Pet. Sci. Eng. 2012, 88, 75–81. [Google Scholar] [CrossRef]
- Salmachi, A.; Sayyafzadeh, M.; Haghighi, M. Infill well placement optimization in coal bed methane reservoirs using genetic algorithm. Fuel 2013, 111, 248–258. [Google Scholar] [CrossRef]
- Herckenrath, D.; Doherty, J.; Panday, S. Incorporating the effect of gas in modelling the impact of CBM extraction on regional groundwater systems. J. Hydrol. 2015, 523, 587–601. [Google Scholar] [CrossRef]
- Sayyafzadeh, M.; Keshavarz, A.; Alias, A.; Dong, K.; Manser, M. Investigation of varying-composition gas injection for coalbed methane recovery enhancement: A simulation-based study. J. Nat. Gas Sci. Eng. 2015, 27, 1205–1212. [Google Scholar] [CrossRef]
- Moore, T. Coalbed methane: A review. Int. J. Coal Geol. 2012, 101, 36–81. [Google Scholar] [CrossRef]
- Shi, J.; Durucan, S. A model for changes in coalbsed permeability during primary and enhanced methane recovery. SPE Reserv. Eval. Eng. 2005, 8, 291–299. [Google Scholar] [CrossRef]
- Shi, J.; Durucan, S. Modelling laboratory horizontal stress and coal permeability data using S&D permeability model. Int. J. Coal Geol. 2014, 131, 172–176. [Google Scholar] [CrossRef]
- Cui, X.; Bustin, R. Volumetric strain associated with methane desorption and its impact on coalbed gas production from deep coal seams. AAPG Bull. 2005, 89, 1181–1202. [Google Scholar] [CrossRef]
- Palmer, I. Coalbed methane completions: A world view. Int. J. Coal Geol. 2010, 82, 184–195. [Google Scholar] [CrossRef]
- An, F.; Cheng, Y.; Wang, L.; Li, W. A numerical model for outburst including the effect of adsorbed gas on coal deformation and mechanical properties. Comput. Geotech. 2013, 54, 222–231. [Google Scholar] [CrossRef]
- Moffat, D.; Weale, K. Sorption by coal of methane at high pressures. Fuel 1955, 34, 449–462. [Google Scholar]










| Parameter | Unit | Value | Parameter | Unit | Value |
|---|---|---|---|---|---|
| H | m | 1143 | τ | d | 10 |
| h | m | 17.6 | ε | 1 | 0.17 |
| p | MPa | 11.8 | β | MPa−1 | 0.5 |
| pgra | MPa/m | 0.01 | μg | Pa·s | 1.03 × 10−5 |
| V | m3/t | 9.61 | μw | Pa·s | 1.01 × 10−3 |
| Vgra | m3/t/m | 0.0032 | Sw | 1 | 1.0 |
| VL | m3/t | 15.71 | Swr | 1 | 0.3 |
| pL | MPa | 3.29 | Sgr | 1 | 0 |
| k0x | mD | 0.205 | η | 1 | 2.5 |
| k0y | mD | k0x | ρc | kg/m3 | 1350 |
| k0y | mD | k0x/10 | E | MPa | 1493 |
| kD | mD | k0x = 18,750 × e−0.008 × H | v | 1 | 0.42 |
| φf0 | 1 | 0.0423 |
| Dip Angle/° | 0 | 10 | 20 | 30 | 40 | 50 | 60 | 70 | 80 | Avg |
|---|---|---|---|---|---|---|---|---|---|---|
| ADGP-C/m3 | 1665 | 1686 | 1699 | 1696 | 1678 | 1653 | 1628 | 1606 | 1591 | 1656 |
| ADGP-D/m3 | 2006 | 2028 | 2045 | 2042 | 2019 | 1986 | 1952 | 1923 | 1903 | 1989 |
| Change rate/% | 20.49 | 20.29 | 20.37 | 20.40 | 20.34 | 20.15 | 19.91 | 19.70 | 19.57 | 20.14 |
| Peak-C/m3 | 3509 | 3522 | 3451 | 3312 | 3115 | 2907 | 2715 | 2600 | 2479 | 3081 |
| Peak-D/m3 | 3398 | 3428 | 3409 | 3324 | 3178 | 3021 | 2874 | 2764 | 2690 | 3120 |
| Change rate/% | −3.16 | −2.65 | −1.22 | 0.36 | 2.05 | 3.90 | 5.87 | 6.29 | 8.53 | 2.22 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Duan, C.; Kang, J.; Fu, X.; Wang, Y.; Lai, P. Differential Evolution of Reservoir Permeability Under Dip Angle Control During Coalbed Methane Production. Processes 2025, 13, 1147. https://doi.org/10.3390/pr13041147
Duan C, Kang J, Fu X, Wang Y, Lai P. Differential Evolution of Reservoir Permeability Under Dip Angle Control During Coalbed Methane Production. Processes. 2025; 13(4):1147. https://doi.org/10.3390/pr13041147
Chicago/Turabian StyleDuan, Chaochao, Junqiang Kang, Xuehai Fu, Yibing Wang, and Peng Lai. 2025. "Differential Evolution of Reservoir Permeability Under Dip Angle Control During Coalbed Methane Production" Processes 13, no. 4: 1147. https://doi.org/10.3390/pr13041147
APA StyleDuan, C., Kang, J., Fu, X., Wang, Y., & Lai, P. (2025). Differential Evolution of Reservoir Permeability Under Dip Angle Control During Coalbed Methane Production. Processes, 13(4), 1147. https://doi.org/10.3390/pr13041147






