Analysis and Control of Abnormal Wear of Reciprocating Compressors in Natural Gas Underground Storage Caverns
Abstract
:1. Introduction
2. Experiment
2.1. Operation Principles and Structure of Compression Cylinder
2.2. Experiments to Determine Composition of “Grinding Paste” in Compression Cylinder
2.3. Measurement of Compression Cylinder Wear
2.4. Sliding Wear Test
3. Numerical Simulation of Wear Rate
4. Results and Discussion
4.1. Determination of Compression Cylinder Wear Rate
4.1.1. Physical Property Analysis of “Grinding Paste”
4.1.2. Determination of Coefficient of Sliding Friction
4.1.3. Changes in Shear Stress
4.1.4. Concentration Distribution of Grinding Paste
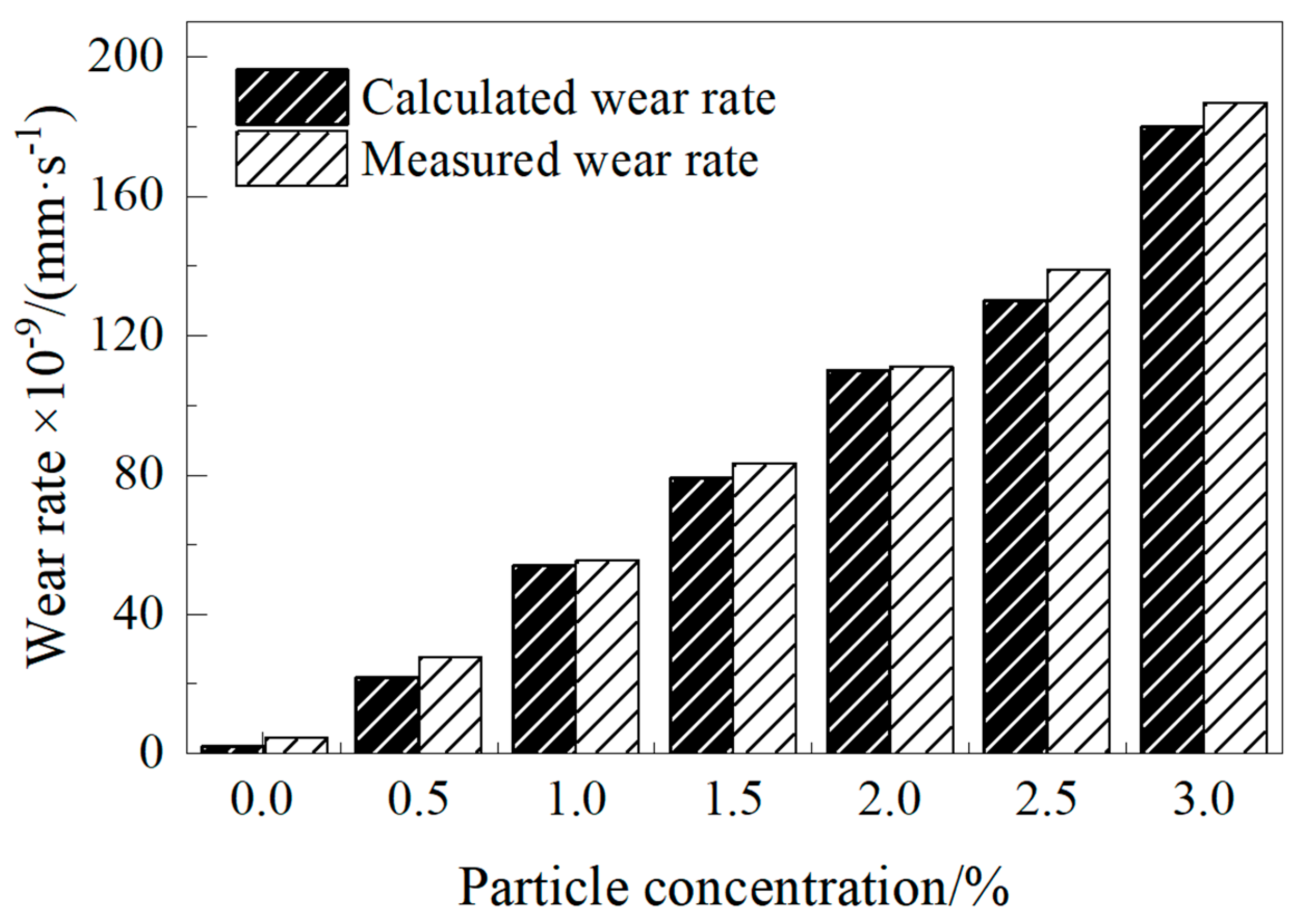
4.1.5. Determination of Wear Rate
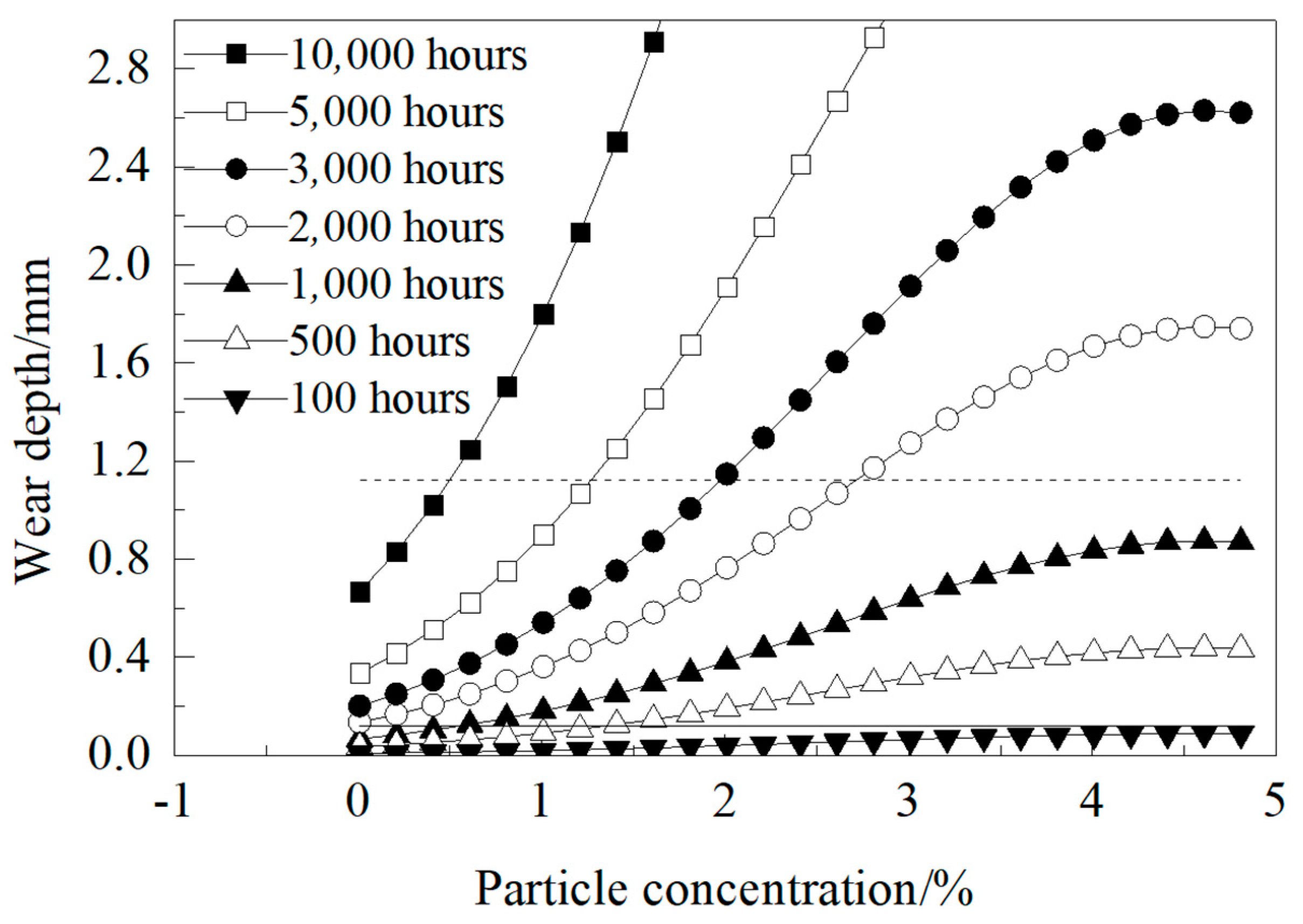
4.2. Determination of Compression Cylinder’s Operation Monitoring Criteria
4.3. Lubricating Oil Viscosity Reduction Experiment Results
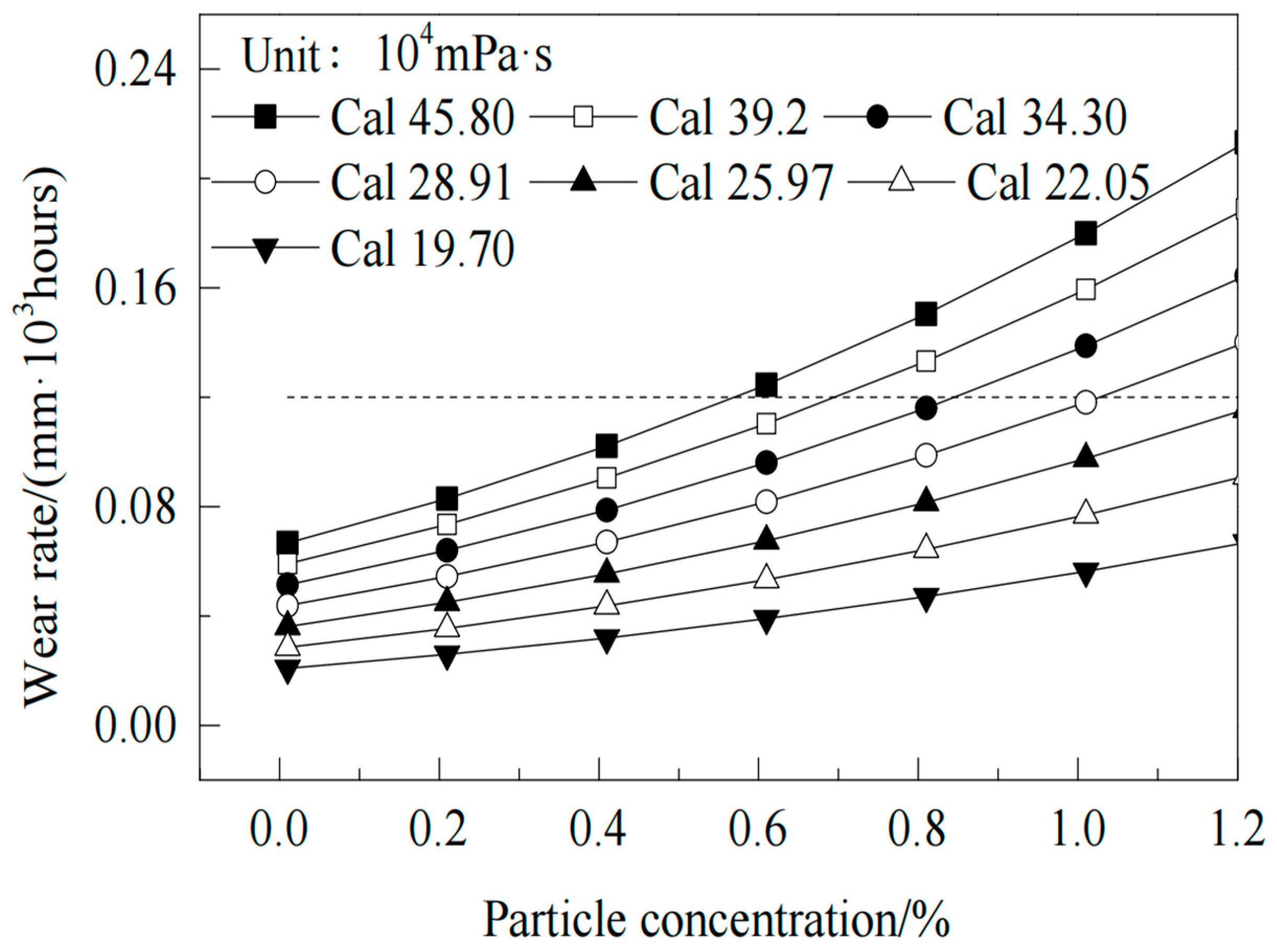
4.3.1. Relationship Between Lubricating Oil Viscosity and Wear Rate
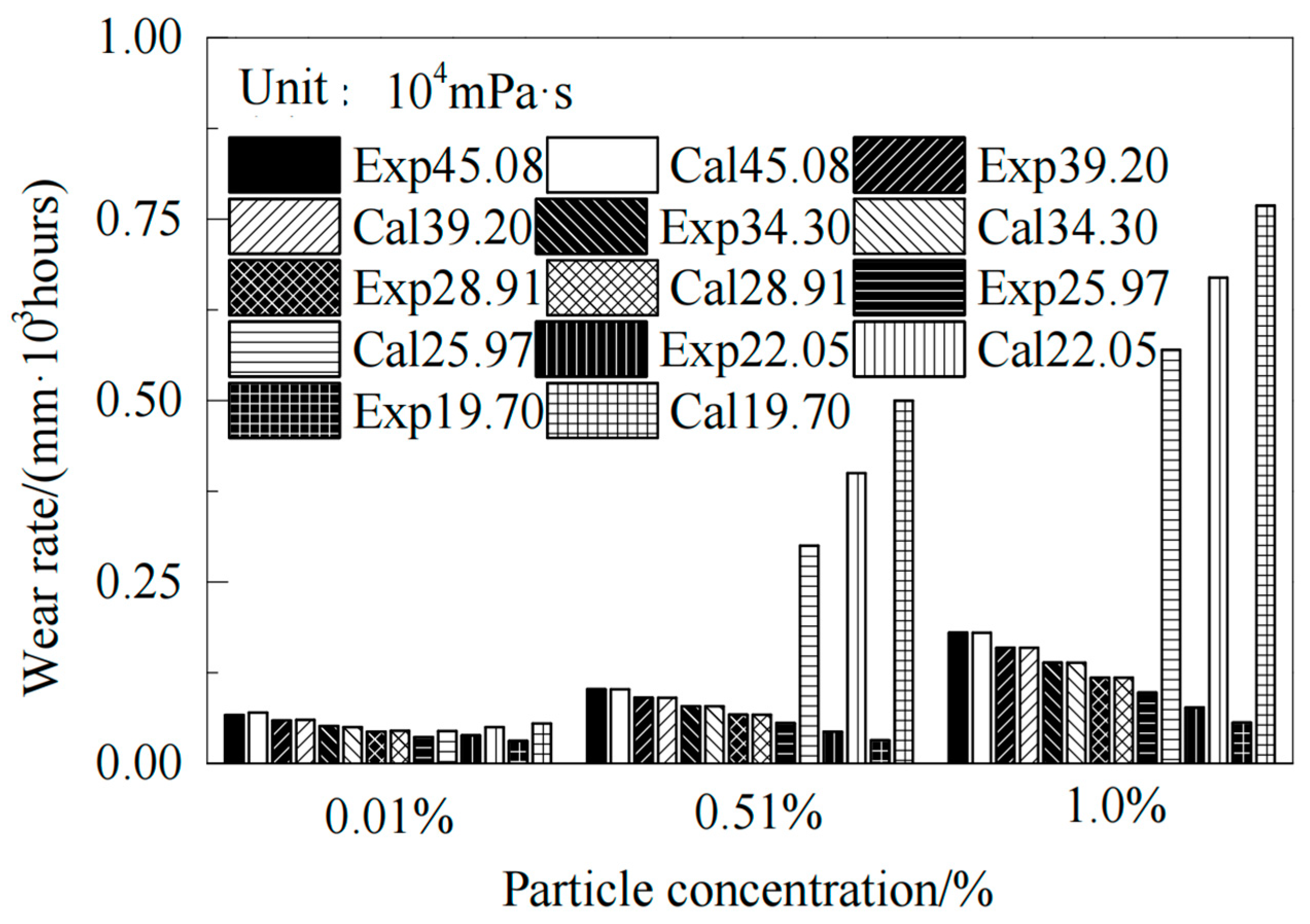
4.3.2. Viscosity Reduction Effect
5. Conclusions
- (1)
- The lubrication conditions in the compression cylinder became worse as the mass percentage of dust in the lubricating oil increased. The viscosity of the grinding paste rose from 450,800 mPa·s to 1,274,000 mPa·s, and the density increased from 890 kg/m3 to 980 kg/m3.
- (2)
- Specifically, the cylinder wall experienced a minimal shear stress of 0.0001 MPa at both the left and right endpoints of the stroke, since the piston did not come into contact with it during the reciprocating cycle of the intake and exhaust process. However, the concentration of the “grinding paste” adhering to the piston remained significantly high. This can be ascribed to the adhesive properties of “grinding paste”, which has a high viscosity and density. When the piston is positioned near the stroke’s midpoint, the concentration of “grinding paste” is lower for two reasons. First of all, the piston ring inhibits the transfer of the “grinding paste” to the opposite side. Second, the minimal gap between the piston and the cylinder results in a low mass concentration of the “grinding paste” that can be retained.
- (3)
- The error in the calculation of wear rate when utilizing the CFD method may be maintained below 10%. It was revealed that for every 1000 h of operation without repairing the compression cylinder (wear less than 0.13 mm), the dust mass concentration should be controlled below 0.6%; for every 5000 h and 10,000 h of operation without replacing the cylinder (wear less than 1.16 mm), the dust mass concentration should be kept below 1.3% and 0.4%, respectively.
- (4)
- It was observed that the wear rate of the seven lubricants with viscosities of 450,800 mPa·s, 392,000 mPa·s, 343,000 mPa·s, 289,100 mPa·s, 259,700 mPa·s, 220,500 mPa·s, and 196,980 mPa·s decreased gradually with increasing lubricant viscosity at a dust mass concentration of 0.01%; however, at dust mass concentrations of 0.51% and 1.0%, the wear rate increased sharply when the lubricant viscosity was 259,700 mPa·s, 220,500 mPa·s, and 196,980 mPa·s.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Pawar, P.B. Compressor, pneumatic components, and accessories in fluid system. In Industrial Hydraulics and Pneumatics; Sankalp Publication: Bilaspur, Chhattisgarh, 2020. [Google Scholar]
- Almasi, A. Latest technologies of rotating machine lubrication and lubricant selection. Aust. J. Mech. Eng. 2015, 9, 171–183. [Google Scholar] [CrossRef]
- Jurayev, R.; Xatamova, D.; Pardayeva, S. Increasing the operating efficiency of mining compressor installations on the basis of improving the cooling, lubrication and air suction system. E3S Web Conf. 2023, 417, 03016. [Google Scholar] [CrossRef]
- Almeida, I.M.G.; Barbosa, C.R.F.; Fontes, F.D.A.O. Methods of wear tests for hermetic reciprocating compressors: An overview. Holos 2016, 4, 86–104. [Google Scholar] [CrossRef]
- Elhaj, M.; Almrabet, M.; Rgeai, M.; Ehtiwesh, I. A combined practical approach to condition monitoring of reciprocating compressors using IAS and dynamic pressure. Int. J. Mech. Mechatron. Eng. 2010, 4, 287–293. [Google Scholar]
- Grabon, W.; Pawlus, P.; Sep, J. Tribological characteristics of one-process and two-process cylinder liner honed surfaces under reciprocating sliding conditions. Tribol. Int. 2010, 43, 1882–1892. [Google Scholar] [CrossRef]
- Milojević, S.; Pešić, R.; Taranović, D. Tribological principles of constructing the reciprocating machines. Tribol. Ind. 2015, 37, 13–19. [Google Scholar]
- Silva, E.; Dhutra, T. Piston trajectory optimization of a reciprocating compressor. Int. J. Refrig. 2021, 121, 159–167. [Google Scholar] [CrossRef]
- Su, J.; Zhang, T.F.; Ke, L.L. Fretting wear behavior of silicone rubber under quartz sand abrasives. Wear 2025, 564–565, 205721. [Google Scholar] [CrossRef]
- Cho, I. Dynamic behavior characteristics of piston in reciprocating compressor. Korean Soc. Tribol. Lubr. Eng. 2013, 29, 105–110. [Google Scholar]
- Manepatil, S.; Yadava, G.S.; Nakra, B.C. Modelling and computer simulation of reciprocating compressor with faults. J. Inst. Eng. Mech. Eng. Div. 2000, 81, 108–116. [Google Scholar]
- Elhaj, M.; Gu, F.; Ball, A.D.; Albarbar, A.; Al-Qattan, M.; Naid, A. Numerical simulation and experimental study of a two-stage reciprocating compressor for condition monitoring. Mech. Syst. Signal Process. 2008, 22, 374–389. [Google Scholar] [CrossRef]
- Rao, X.; Sheng, C.; Guo, Z.; Yuan, C. Influence of surface groove width on tribological performance for cylinder liner–piston ring components. Tribol. Trans. 2019, 62, 239–248. [Google Scholar] [CrossRef]
- Cheng, S. Dynamic analysis of reciprocating compressor with a translational joint clearance. Vibroeng. Procedia 2018, 18, 36–40. [Google Scholar] [CrossRef]
- Zhang, J.J.; Song, C.Y.; Lei, F.C.; Wang, Y.; Zhi, H.; Liu, F. Research on the dynamic response of connecting rod bearing bush wear of reciprocating machine under variable working conditions. High Technol. Lett. 2023, 29, 148–158. [Google Scholar]
- Jarang, H.G.; Deshpande DR, S. The survey on reciprocating gas compressor—A review. Int. J. Sci. Res. 2022, 11, 389–393. [Google Scholar] [CrossRef]
- Shcherba, V.; Pavlyuchenko, E.; Nosov, E.; Bulgakova, I.Y. Approximation of the compression process to isothermal in a reciprocating compressor with a liquid piston. Appl. Therm. Eng. 2022, 207, 118151. [Google Scholar] [CrossRef]
- Burhanuddin, F.; Yuwono, A.H.; Yuliati, D. Improved Maintenance Strategy for Reciprocating Compressor Based on Reliability and Safety. Int. J. Reliab. Risk Saf. Theory Appl. 2024, 7, 73–81. [Google Scholar]
- Zhao, Z.; Ma, Y.; Ju, P.; Wu, Y.; Chen, L.; Zhou, H.; Chen, J. Improved wear resistance and environmental adaptability of a polysiloxane/molybdenum disulfide composite lubricating coating using polyhedral oligomeric silsesquioxane. ACS Appl. Mater. Interfaces 2022, 14, 36105–36115. [Google Scholar] [CrossRef]
- Xu, C.; Liu, Z.; Zhou, L.; Zhao, Y. Development of a DEM method for predicting wear distribution on particle scale. Powder Technol. 2024, 432, 119126. [Google Scholar] [CrossRef]
- Zhao, E.H.; Ma, B.; Li, H.Y. Study on the high temperature friction and wear behaviors of Cu-based friction pairs in wet clutches by pin-on-disc tests. Adv. Mater. Sci. Eng. 2017, 2017, 6373190. [Google Scholar] [CrossRef]
- Pereira, E.L.; Santos, C.J.; Deschamps, C.J.; Kremer, R. A simplified CFD model for simulation of the suction process of reciprocating compressors. In Proceedings of the International Compressor Engineering Conference at Purdue, West Lafayette, IN, USA, 16–19 July 2012; pp. 234–289. [Google Scholar]
- Pereira, E.; Deschamps, C.J.; Ribas, F.A. Performance analysis of reciprocating compressors through computational fluid dynamics. Arch. Proc. Inst. Mech. Eng. Part E J. Process Mech. Eng. 2008, 222, 183–192. [Google Scholar] [CrossRef]
- Chang, X.; Ma, R.; Zhang, L.; He, X.; Li, M. Further study on the geometric conservation law for finite volume method on dynamic unstructured mesh. Comput. Fluids 2015, 120, 98–110. [Google Scholar] [CrossRef]
- Zhang, Y.; Reuterfors, E.; McLaury, B.; Shirazi, S.; Rybicki, E. Comparison of Computed and Measured Particle Velocities and Erosion in Water and Air Flows. Wear 2007, 263, 330–338. [Google Scholar] [CrossRef]
- Beinert, S.; Fragnière, G.; Schilde, C.; Kwade, A. Analysis and modelling of bead contacts in wet-operating stirred media and planetary ball mills with CFD–DEM simulations. Chem. Eng. Sci. 2015, 134, 648–662. [Google Scholar] [CrossRef]
- Tomanik, E. Friction and wear bench tests of different engine liner surface finishes. Tribol. Int. 2008, 41, 1032–1038. [Google Scholar] [CrossRef]
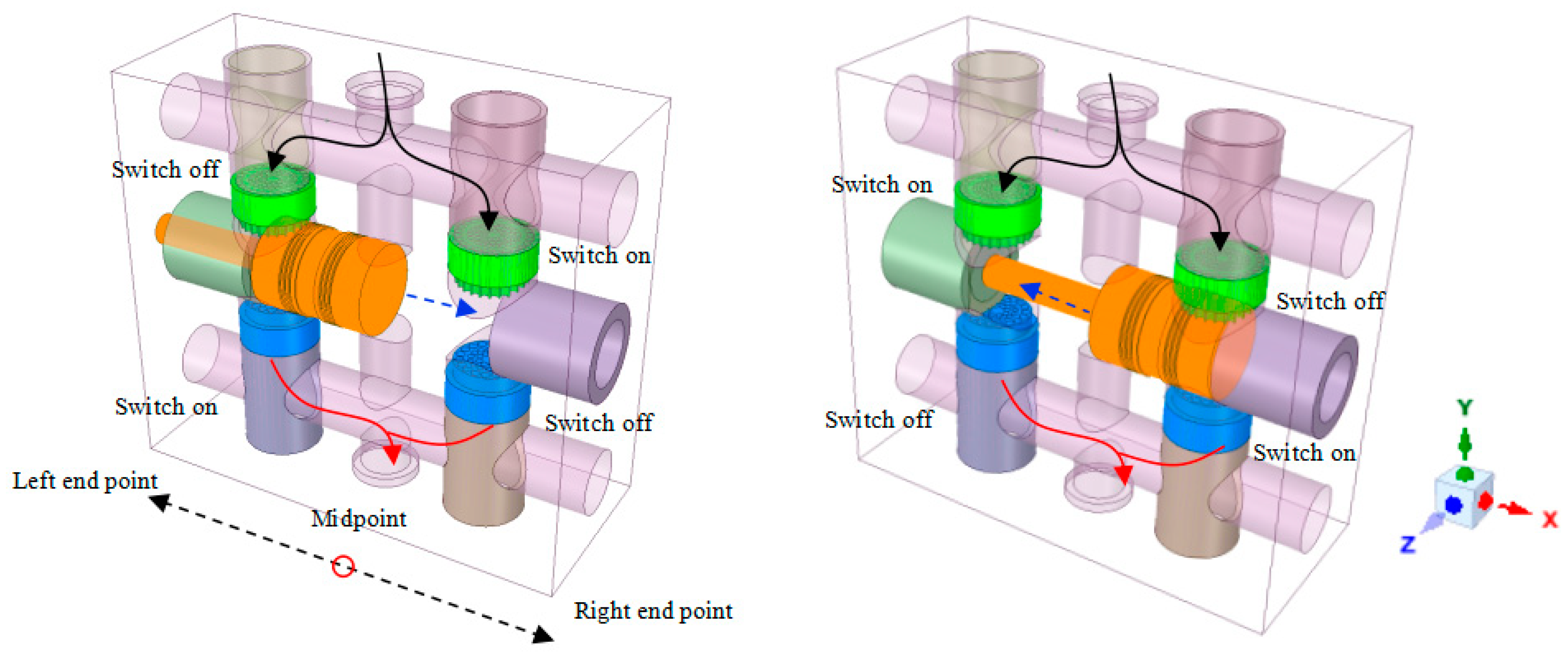

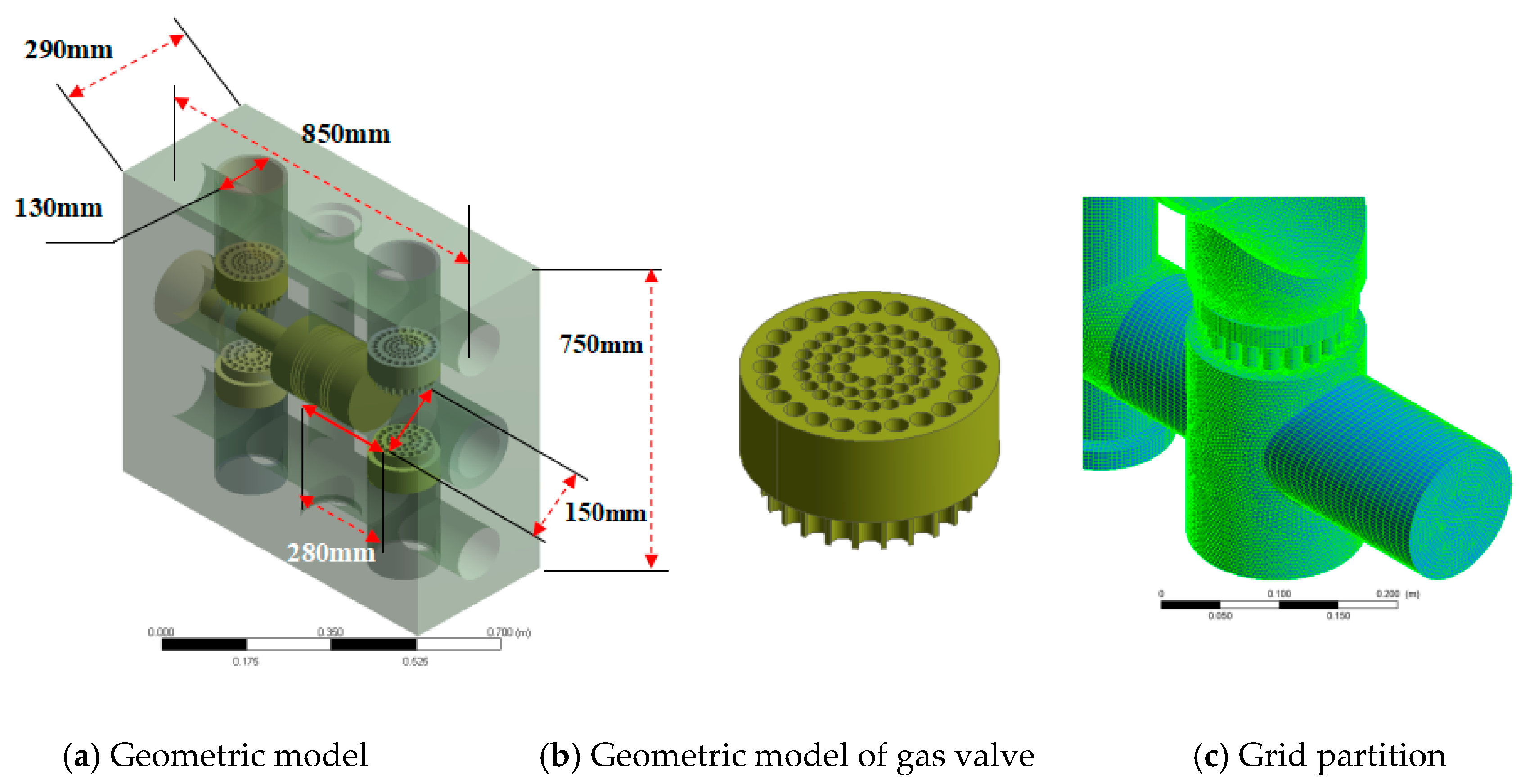


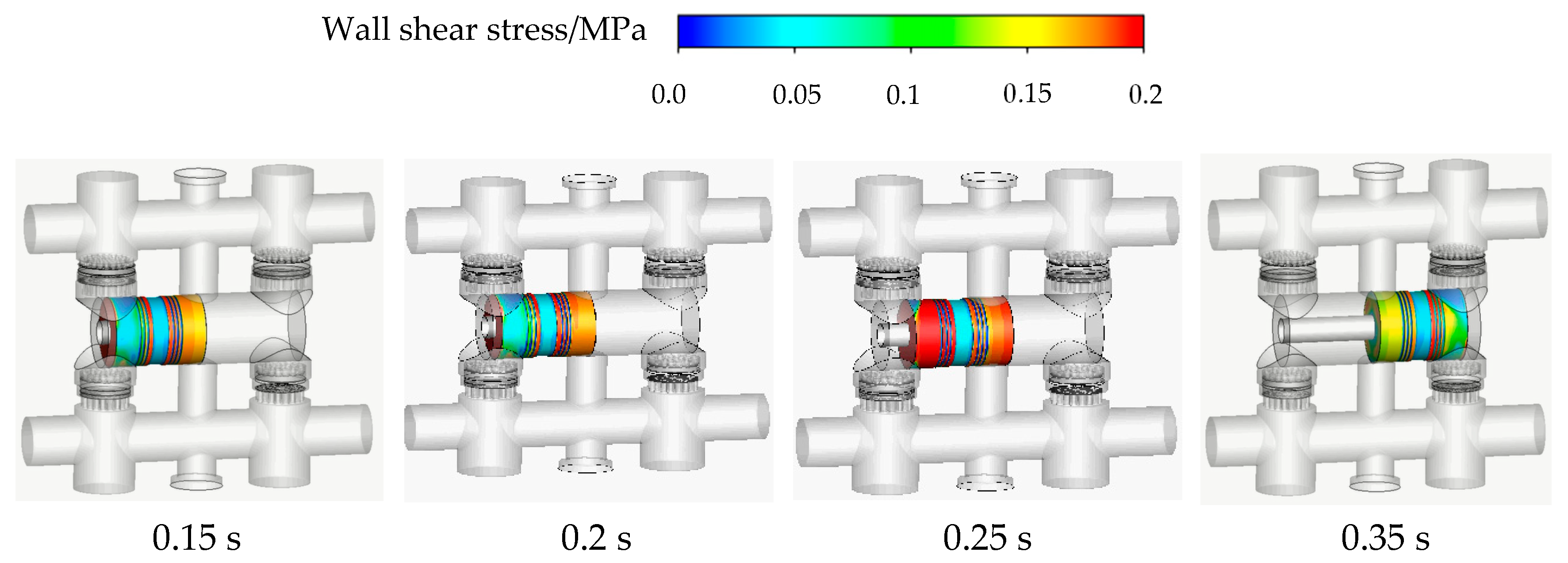
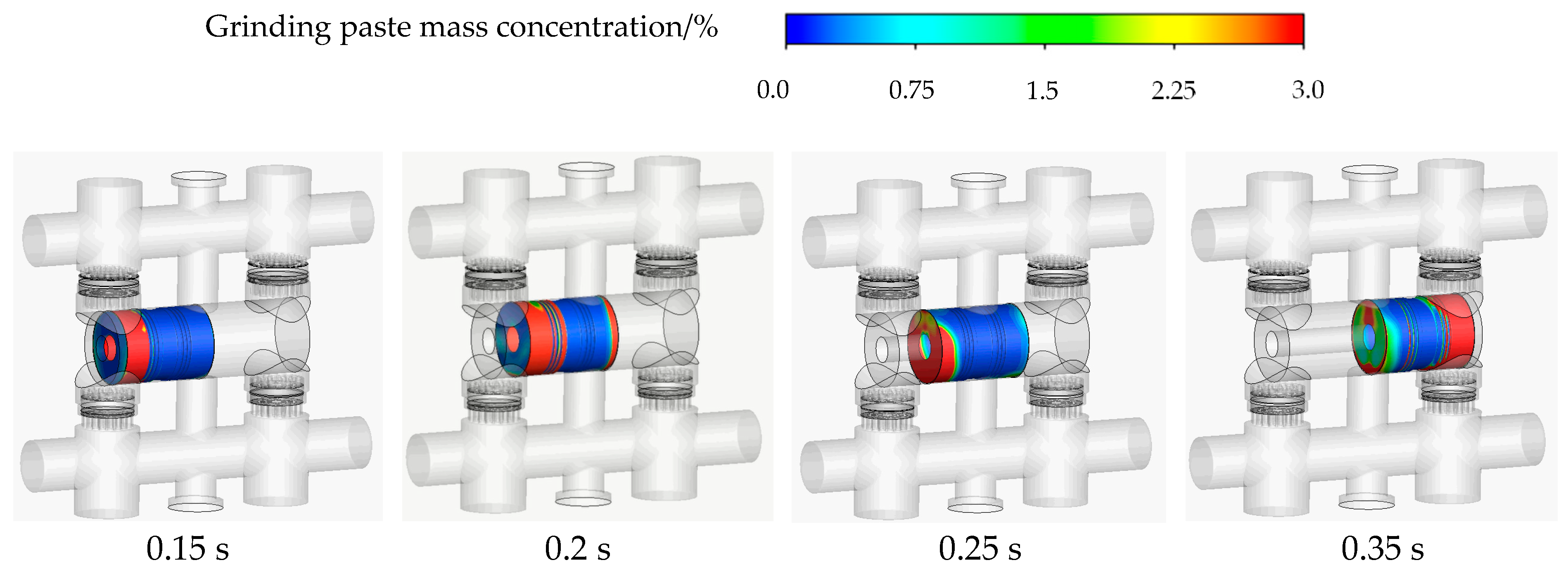


| No. | Dust Mass Concentration /% | Lubricating Oil Viscosity /mPa·s | Dust Diameter /μm |
|---|---|---|---|
| 1 | 0.01 | 450,800 | 10~50 |
| 2 | 0.5 | 450,800 | 10~50 |
| 3 | 1.0 | 450,800 | 10~50 |
| 4 | 1.5 | 450,800 | 10~50 |
| 5 | 2.0 | 450,800 | 10~50 |
| 6 | 2.5 | 450,800 | 10~50 |
| 7 | 3.0 | 450,800 | 10~50 |
| No. | Dust Mass Concentration /% | Lubricating Oil Viscosity /mPa·s | No. | Dust Mass Concentration /% | Lubricating Oil Viscosity /mPa·s | No. | Dust Mass Concentration /% | Lubricating Oil Viscosity /mPa·s |
|---|---|---|---|---|---|---|---|---|
| 8.1 | 0.01 | 450,800 | 9.1 | 0.51 | 450,800 | 10.1 | 1.00 | 450,800 |
| 8.2 | 0.01 | 392,000 | 9.2 | 0.51 | 392,000 | 10.2 | 1.00 | 392,000 |
| 8.3 | 0.01 | 343,000 | 9.3 | 0.51 | 343,000 | 10.3 | 1.00 | 343,000 |
| 8.4 | 0.01 | 289,100 | 9.4 | 0.51 | 289,100 | 10.4 | 1.00 | 289,100 |
| 8.5 | 0.01 | 259,700 | 9.5 | 0.51 | 259,700 | 10.5 | 1.00 | 259,700 |
| 8.6 | 0.01 | 220,500 | 9.6 | 0.51 | 220,500 | 10.6 | 1.00 | 220,500 |
| 8.7 | 0.01 | 196,980 | 9.7 | 0.51 | 196,980 | 10.7 | 1.00 | 196,980 |
| Dust mass concentration/% | 0.01 | 0.50 | 1.00 | 1.50 | 2.00 | 2.50 | 3.00 |
| Grinding paste viscosity/(mPa·s) | 450,800 | 518,300 | 608,600 | 719,200 | 993,300 | 1,128,400 | 1,274,000 |
| Grinding paste density/(kg/m3) | 890 | 890 | 895 | 899 | 903 | 910 | 980 |
| Dust mass concentration/% | 0.01 | 0.50 | 1.00 | 1.50 | 2.00 | 2.50 | 3.00 |
| Friction coefficient μ | 0.08 | 0.124 | 0.129 | 0.163 | 0.18 | 0.205 | 0.245 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zheng, S.; Dai, Z.; Wang, F.; Wang, F.; Wang, Y.; Bie, Q.; Jiang, W.; Chen, J.; Peng, Z.; Sun, J. Analysis and Control of Abnormal Wear of Reciprocating Compressors in Natural Gas Underground Storage Caverns. Processes 2025, 13, 996. https://doi.org/10.3390/pr13040996
Zheng S, Dai Z, Wang F, Wang F, Wang Y, Bie Q, Jiang W, Chen J, Peng Z, Sun J. Analysis and Control of Abnormal Wear of Reciprocating Compressors in Natural Gas Underground Storage Caverns. Processes. 2025; 13(4):996. https://doi.org/10.3390/pr13040996
Chicago/Turabian StyleZheng, Sijia, Zhixiang Dai, Fei Wang, Feng Wang, Yongbo Wang, Qin Bie, Wei Jiang, Jingdong Chen, Zicheng Peng, and Jie Sun. 2025. "Analysis and Control of Abnormal Wear of Reciprocating Compressors in Natural Gas Underground Storage Caverns" Processes 13, no. 4: 996. https://doi.org/10.3390/pr13040996
APA StyleZheng, S., Dai, Z., Wang, F., Wang, F., Wang, Y., Bie, Q., Jiang, W., Chen, J., Peng, Z., & Sun, J. (2025). Analysis and Control of Abnormal Wear of Reciprocating Compressors in Natural Gas Underground Storage Caverns. Processes, 13(4), 996. https://doi.org/10.3390/pr13040996








