Experimental Study and Numerical Simulation of Sediment’s Promoting Effect on Cavitation Based on Flow Field Analysis

Abstract

Sediment-laden water significantly exacerbates the cavitation damage in hydraulic machinery compared to clear water, underscoring the importance of investigating the effects of sediment on cavitation. This study examines cavitation in sediment-laden water using a Venturi flow channel and a high-speed camera system. Natural river sand samples with a median diameter of 0.05, 0.07, and 0.09 mm are selected, and sediment-laden water with a concentration of 10, 30, and 50 g/L is prepared. The results indicate that increasing the sediment concentration or reducing the sediment size intensifies cavitation, and the influence of the sediment concentration is significantly greater than that of the sediment size. Meanwhile, the numerical simulation is conducted based on a gas–liquid–solid phase mixing model. The findings indicate that a higher sediment concentration corresponds to a greater shearing effect near the wall, which raises the drag on the attached sheet-like cavitation clouds and enhances the re-entrant jet which can intensify the shedding of cavitation clouds. Furthermore, sediment particles contribute to more vortices. Therefore, for hydraulic machinery operating in sediment-laden water of high concentration, the relative velocity should be reduced to mitigate the shearing effect, thereby weakening the synergy of cavitation and sediment erosion at the turbine runner.

1. Introduction

Hydropower is one of the most important types of renewable energy, and its power generation accounts for 70% of the total energy production. The excessive river sedimentation in regions such as the Himalayas and Alps poses a severe challenge to sustainable hydropower development [1]. China has the highest hydropower development potential in the world, accounting for 43% of the total number of dams in the world. China’s installed hydropower capacity accounts for 27% of the world’s hydropower installed capacity [2]. At the same time, China is facing the problem of sedimentation. Moreover, hydraulic machinery (i.e., pumps, turbines, and reversible pump turbines) often operate under cavitation conditions. The synergistic effect of cavitation and sediment erosion can cause more serious damage as shown in Figure 1 [3,4].

Among the hydropower stations that have been built in China, 40% of the turbines have been severely affected by the synergistic effect of cavitation and sediment erosion, which will reduce the turbine efficiency, shorten the service life, and increase the operation and maintenance costs. However, the synergistic wear mechanisms are barely understood, and the conclusions reached by studies differ from each other. The synergistic bubble particle effect is manifested in the dual inhibition or promotion mechanism [5,6]. There are many factors affecting the promotion or inhibition of the synergistic effect between abrasion and cavitation, including the solid–liquid mixture [7,8] and particle properties [9,10].

Raichenko, Byakova [11], and Morch [12] suggested that inhomogeneities within the liquid can function as nucleation centers for bubbles and developed models to analyze the bubble formation process. Shen et al. [13] found that the effect on single cavitation bubble dynamics could be negligible for small viscosities, but it should be considered for relatively high viscosities. Liu et al. [14] found that fine particles increased the fluid’s viscosity, and the strong liquid viscosity produced higher resistance to the growth and collapse of bubbles, lowering the particle velocity and suppressing cavitation.

The properties of particles including size, concentration, shape, and type are key influencing factors for the damage from cavitation and abrasion.

Lian et al. [10] reported that the critical particle diameter was approximately 0.035–0.048 mm. When the sediment had a particle size smaller than the critical value, the damage from the combined wear was slightly less severe than that from cavitation in clear water. Using a specialized vibrating device, Wu and Gou [15] discussed the combined wear under three sediment concentration cases (25, 50, and 85 kg/m³) and five particle diameter scenarios (0.531, 0.253, 0.063, 0.042, 0.026 mm). They revealed that when the particle diameter was smaller than the critical value (0.048 mm), the wear effect weakened as the concentration increased. Chen et al. [16] found that the generated damage was less severe than that in clear water when the average sediment size was smaller than 0.120 mm, and Zhong and Minemura [17] suggested that for sediment with a size less than 1 mm, the flow would only cause cavitation erosion. In general, when the particle size is smaller than the threshold, cavitation is suppressed; otherwise, it is intensified [18,19,20]. For particles smaller than the threshold size, the medium sand and clay in the particle-laden flow have non-Newtonian fluid features.

In the presence of sand, the cavitation pressure threshold rises proportionately to the sediment concentration. Zhu et al. [21] indicated that the sediment concentration was linearly related to the critical cavitation value, with higher particle concentrations corresponding to more possible occurrences of cavitation. This was because many gas nuclei with different sizes existed within the particle surface cracks; increasing the sediment concentration inevitably generated more gas nuclei in the flow [22,23]. However, the conclusion that an increased particle concentration monotonically promotes or inhibits the combined erosion was contradicted by some other reports, which suggested a particle concentration threshold. As the concentration increased under a fixed particle diameter, the combined wear initially weakened and then strengthened [24,25].

In addition, the shape and type of particle will affect the cavitation [26,27,28,29,30]. However, the size and concentration of particles are crucial factors affecting cavitation, and their different combinations can inhibit or promote cavitation. Based on scholars’ previous research [10,15], natural river sand with a median diameter of 0.05, 0.07, and 0.09 mm is selected, and sediment-laden water with a concentration of 10, 30, and 50 g/L is prepared for the study of how different sediment conditions promote cavitation in a Venturi structure flow channel in this paper.

The mechanism of the inhibition or promotion of cavitation has been studied. Li and Zhang et al. [31,32] noticed that the particle shooting effect (acceleration of particles when bubbles expanded and after bubbles and particles detached) was more pronounced as the particle-to-bubble size ratio was reduced. Li et al. [33] claimed that bubble–particle interactions were heavily dependent on the particle-to-bubble size ratio and particle-to-liquid density ratio. Poulain et al. [34] reported a quantitative relation between sand movement due to cavitation bubbles and their distance. Arora et al. [35,36] discovered that cavitation bubbles’ collapse would accelerate the nearby sand particles, possibly aggravating abrasion and damage.

The current research on the synergistic mechanisms focuses on the theoretical analyses of individual cavitation bubbles or the combined effects of sediment and cavitation through material erosion assessments. Meanwhile, significant achievements have been made in the impact of cavitation on particles. However, there is a dearth of studies that directly analyze sediment-laden flow fields to understand the sediment–bubbles relationship. This study explores the effect of sediment-laden flow on cavitation evolution. The viscosity of sediment-laden water varies with the sediment size and concentration, and has influences on the flow field and will affect the formation, development, and collapse of cavitation. We obtained the viscosity of sediment-laden water through a viscometer and apply it to subsequent numerical calculations and analysis of the flow field.

There are relatively few visual experimental studies on the cavitation characteristics of sediment-laden water in the existing research. Due to its simple structure, the cavitation mechanism of the Venturi is consistent with hydraulic machinery such as pumps and turbines, making it important experimental equipment for studying the cavitation mechanisms and characteristics. This study employed a high-speed camera system to capture the morphology of cavitation and the complete developmental process within a Venturi structure flow channel containing sediment-laden water. Meanwhile, some scholars have used the three-phase flow (solid–liquid–vapor) model to analyze the erosion of the wall by sediment particles in the presence of cavitation bubbles. However, there is a lack of research on the cavitation flow field affected by sediment. In this paper, based on sediment–water two-phase flow, we consider the occurrence and development of cavitation, and compare the impact of sediment particles on the cavitation cycle.

2. Visual Cavitation Experiment

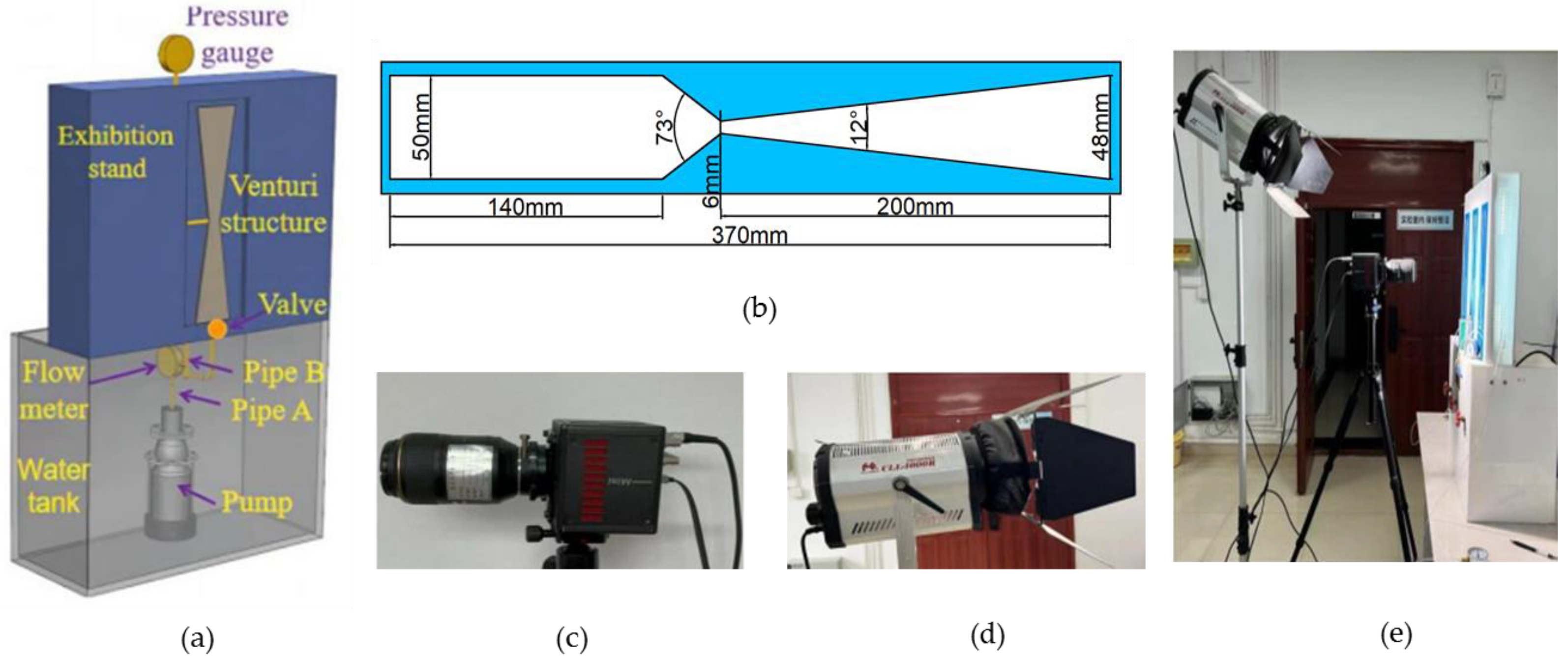
2.1. Experimental Apparatus

The experimental apparatus is a small desktop-integrated self-circulating system, equipped with a 40 L water storage tank, a pump with a head of 20 m, a T4-type light source, a display screen, and a Venturi flow channel made of Seiko transparent acrylic material as shown in Figure 2a. The dimensions of the equipment are 550 mm in length, 300 mm in width, and 1100 mm in height. The working voltage is 220 volts, and the power is 300 watts. The throat pressure can be read through a vacuum gauge connected to the throat. The experimental apparatus has been modified to read the inlet and outlet pressures: a hole is drilled in the display screen at a position 1 cm downstream of the inlet, and a pressure gauge with a measuring range of 0 to 0.16 MPa is attached. A hole is drilled in the display screen at a position 3 cm away from the outlet, and a vacuum gauge with a measuring range of −0.1 to 0 MPa is attached.

The Venturi flow channel comprises four distinct sections: an inlet straight section, a contraction section, a throat, and a diffusion section. As the fluid traverses the throat of the Venturi channel, the reduction in cross-sectional area results in an increase in fluid velocity, leading to a decrease in static pressure. This pressure drop induces the vacuum condition, creating the requisite environment for cavitation formation. The dimensions of the Venturi channel are illustrated in Figure 2b. A Fastcam AX-100 high-speed camera (Photron, Japan) equipped with a Nikon lens (Nikon, Japan) with a focal length of 105 mm is used to record the cavitation phenomena as shown in Figure 2c. To enhance the quality of the cavitation cloud images, a photographic light source is utilized for additional illumination and is shown in Figure 2d. The experimental site is shown in Figure 2e. The Photron FASTCAM Viewer software (version 3.6.9.1) is utilized to precisely control the frame rate, recording duration, and resolution of the high-speed photography system, which can achieve a 20,000 fps frame rate at the maximum. During the experiments, the frame rate was set to 4000 fps to ensure that the captured images maintain sufficient detail, with an image resolution of 1024 × 1024 pixels.

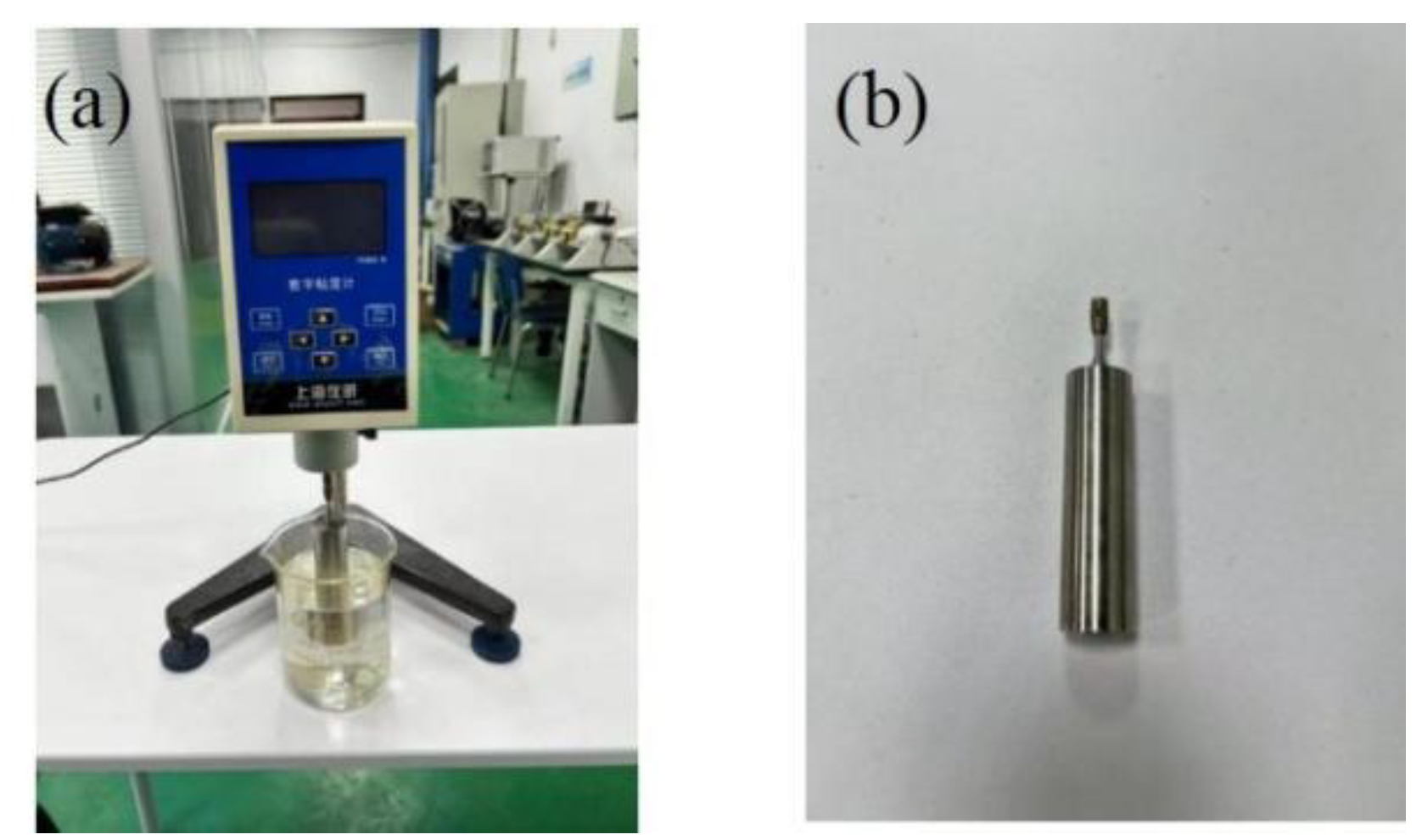
2.2. Measurement of Viscosity

The viscosity of a liquid significantly influences its flow behavior and cavitation characteristics. In sediment-laden water, viscosity differences arise from the variations in particle concentration and size compared to clear water. These viscosity variations impact the cavitation behavior, which is the primary concern of the present study. To measure the sediment-laden water’s viscosity, an NDJ-8S digital viscometer (Shanghai Zhongchen Digital Technology Equipment Co., Ltd., Shanghai, China) was employed, as illustrated in Figure 3a. This instrument drives the rotor to rotate at a constant speed via an electric motor. When the rotor spins in the liquid, the liquid exerts a viscous torque on the rotor. A sensor detects and collects data on this viscous torque, which are then processed by a computer to determine the viscosity of the measured liquid. Assuming laminar flow and negligible slip between the liquid and the measuring spindle’s surface, the viscosity of the liquid can be calculated using the appropriate formula.

$$\mu ={\displaystyle \frac{M}{4\pi h\omega }}\left({\displaystyle \frac{1}{r^2}}-{\displaystyle \frac{1}{R^2}}\right)$$

(1)

In this context, M represents the torque exerted by the internal friction of the liquid on the rotating spindle, r denotes the inner cylinder radius, and R is the outer cylinder radius. h indicates the immersed height of the liquid surrounding the inner cylinder, and ω signifies the angular velocity of the rotating spindle. This instrument is equipped with an over range alarm function: when the test value exceeds 100% of the measuring range, the display will show “over”. To ensure the measurement accuracy, the percentage of the measuring range is controlled between 10% and 90%. Rotor 0 has the smallest measuring range, with a full scale of 20 mPa·s at a rotation speed of 60 rpm. Therefore, Rotor 0 was selected in this study.

The viscosity of clear water measured with this instrument is 1.13 mPa·s when the water temperature is 15 degrees Celsius, and the viscosity measurements for sediment-laden water are summarized in

Table 1. Viscosity of sand water μ (mPa·s).

	S (g/L)	10	30	50
d (mm)
0.09		1.132	1.141	1.219
0.07		1.134	1.153	1.275
0.05		1.139	1.165	1.756

. Notably, at a low sediment concentration (S = 10 g/L), the viscosity matches that of clear water, regardless of the particle size. However, as the sediment concentration increases, the viscosity rises, with the particle size playing a more significant role. Smaller particles contribute to a greater increase in viscosity. For example, with a particle diameter of d = 0.09 mm, the viscosity rises from 1.132 mPa·s to 1.219 mPa·s, reflecting a 7.68% increase. In contrast, with a particle diameter of d = 0.05 mm, the viscosity escalates from 1.139 mPa·s to 1.756 mPa·s, a notable increase of 54.17%. Thus, an increase in the sediment concentration with finer particles leads to a substantial rise in the viscosity compared to clear water, underscoring the importance of the cavitation behavior under such conditions.

2.3. Experiment Results

2.3.1. Comparison of the Cavitation Intensity

Since cavitation initiates and then develops at the wall of the throat, the cavitation number at the throat is adopted to reflect the intensity of the cavitation. In the experimental Venturi structure flow channel, the transition from the converging flow section to the diverging flow section occurs abruptly at a certain cross-section, and the throat is exactly this abrupt cross-section. The pressure measuring points are arranged on the inner wall of the throat, and the pressure at the measuring points is used to reflect the pressure at the throat. Based on the experimentally measured average pressure at the throat, the cavitation numbers for various sediment-laden water samples are presented in

Table 2. Cavitation number of sand water.

d (mm)	S (g/L)	Pthroat (Pa)	σ
0.09	10	22,325	0.27
	30	14,325	0.17
	50	8325	0.08
0.07	10	19,325	0.24
	30	11,825	0.14
	50	7325	0.07
0.05	10	17,325	0.21
	30	10,325	0.12
	50	6325	0.06

. The findings demonstrate that the cavitation number decreases at higher sediment concentrations, indicating the enhanced cavitation intensity. As the water flow decelerates from the throat to the diffusion section, the drag effect of the sediment particles slows the decrease in flow velocity and leads to a more gradual increase in pressure. Consequently, this results in a lower pressure at the throat, effectively promoting cavitation. A higher concentration corresponds to a more pronounced particle drag effect.

The effect of particle dimension on the cavitation number is notably less significant than that of the sediment concentration. For example, at d = 0.09 mm, increasing the sediment concentration from 10 g/L to 50 g/L results in a reduction in the cavitation number from 0.27 to 0.08, a decrease of 70.37%. Conversely, in sediment-laden water with a concentration of 50 g/L, reducing the particle size from d = 0.09 mm to d = 0.05 mm only decreases the cavitation number from 0.08 to 0.05, reflecting a reduction of only 12.5%.

The relationship between the cavitation number and viscosity is illustrated in Figure 4, which is supported by the data in Table 1. The graph demonstrates that, in sediment-laden water, an increased viscosity correlates with a smaller cavitation number and an increased cavitation intensity. When the sediment size exceeds the critical value, an increase in concentration or a decrease in sediment size will promote cavitation, and the promoting effect of concentration is more significant than that of the sediment size. Therefore, the cavitation of sediment-laden water with a high concentration (S = 50 g/L) should be taken seriously.

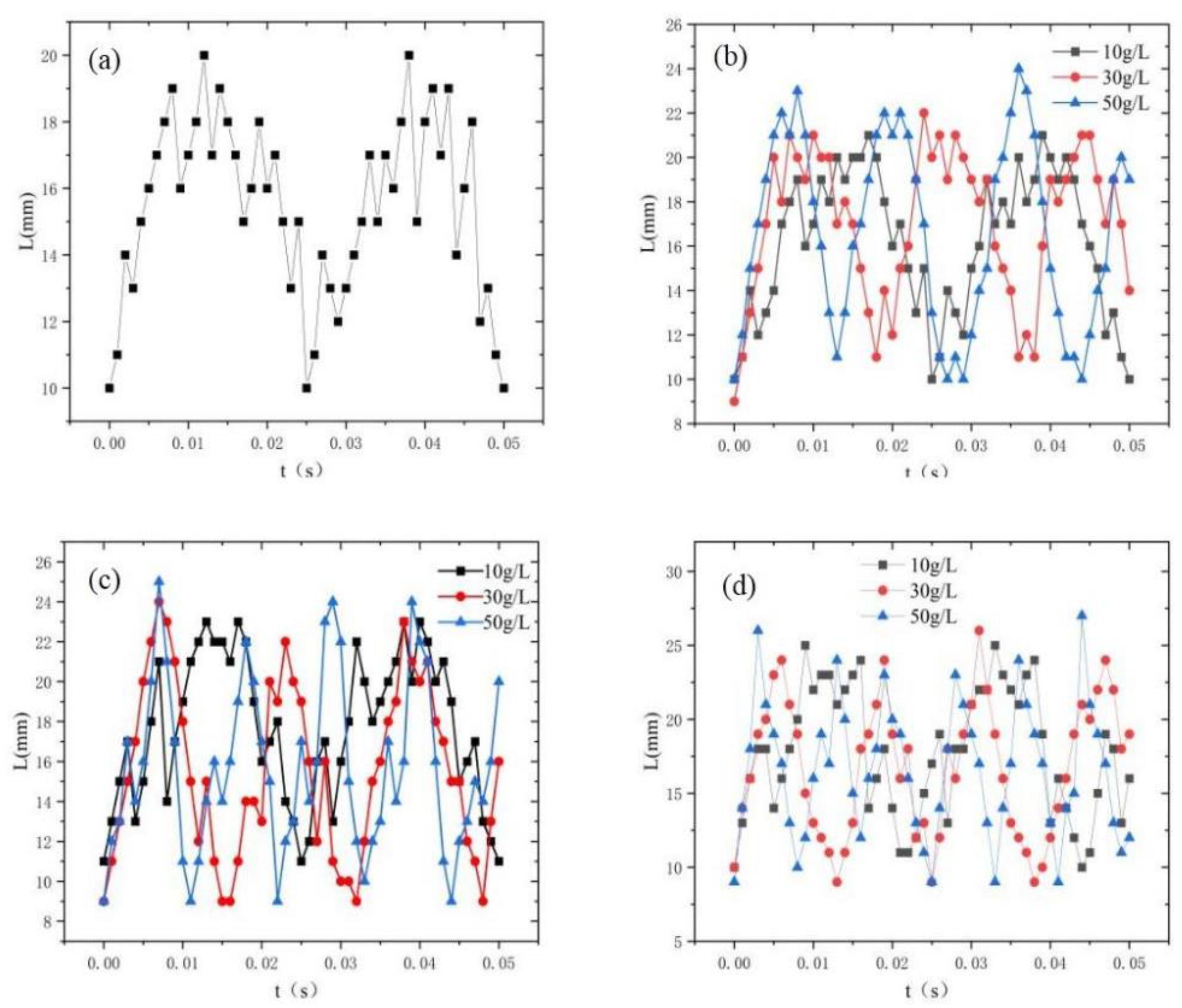
2.3.2. Evolution Process of the Cavitation Cloud

During the cavitation experiments, high flow rates revealed a distinct phenomenon of periodic cavitation bubble shedding at the cloud tail. For instance, at a flow rate of Q = 294 mL/s and a sediment concentration of S = 50 g/L with different sediment sizes, the cavitation cloud evolution over a selected cycle, denoted as T, is analyzed and depicted. The captured raw images underwent MATLAB (version 9.13 R2022b) processing including background subtraction, binarization, and denoising, as demonstrated in Figure 5.

The cavitation bubbles at the tail of the cloud undergo three distinct stages: growth, separation, and collapse. During cavitation growth, the cloud extends downstream on both the upper and lower sides. During the separation stage, noticeable breaking and detachment of bubbles occur at both sides of the tail. Finally, in the collapse stage, the separated bubbles rapidly collapse. The cloud length before detaching from the wall is defined as the cavitation cloud length (L), and its periodic variation over time is illustrated in Figure 6.

To examine the development cycle of cavitation clouds in both clear water and sediment-laden water, ten development cycles were selected for analysis. An arithmetic average was computed to determine the average period. The cavitation period for clear water is T = 0.0255 s. The impacts of the sediment size and concentration on the cavitation cloud evolution are presented in Figure 7.

Notably, when the sediments have a very low concentration (S = 10 g/L), the cavitation cloud’s evolution cycle closely resembles that of clear water and is minimally affected by the particle size. However, as the sediment concentration increases, shorter cycles become more pronounced with smaller particle sizes. For instance, when the sediment concentration S = 50 g/L and the particle diameter d = 0.09 mm, the cycle is T = 0.0123 s; with d = 0.05 mm, the cycle is T = 0.0075 s, showing a 39% reduction. The impact of the sediment concentration on the cavitation cloud’s evolution cycle is even more significant. With a particle diameter of d = 0.09 mm, increasing the sediment concentration from S = 10 g/L to 50 g/L reduces the cycle from T = 0.0254 s to 0.0123 s, a 51.57% decrease. When the particle diameter is d = 0.05 mm, the cycle decreases from T = 0.0252 s to 0.0075 s, a reduction of 70.24% when the sediment concentration grows from 10 g/L to 50 g/L. It is found that a decreasing sediment size or increasing sediment concentration shortens the development cycle of cavitation, and the effect of concentration is more significant than that of size.

This phenomenon can be attributed to the cavitation cloud shedding induced by the near-wall re-entrant jet. Sediment particles, characterized as non-wettable solids, exhibit a greater tensile stress than water fractures. Consequently, the re-entrant jet transporting the sediment particles possesses enhanced tearing and destructive capabilities compared to that in clear water, thereby accelerating the shedding of the cavitation cloud. The destructive potential of the re-entrant jet becomes increasingly pronounced at higher sediment concentrations.

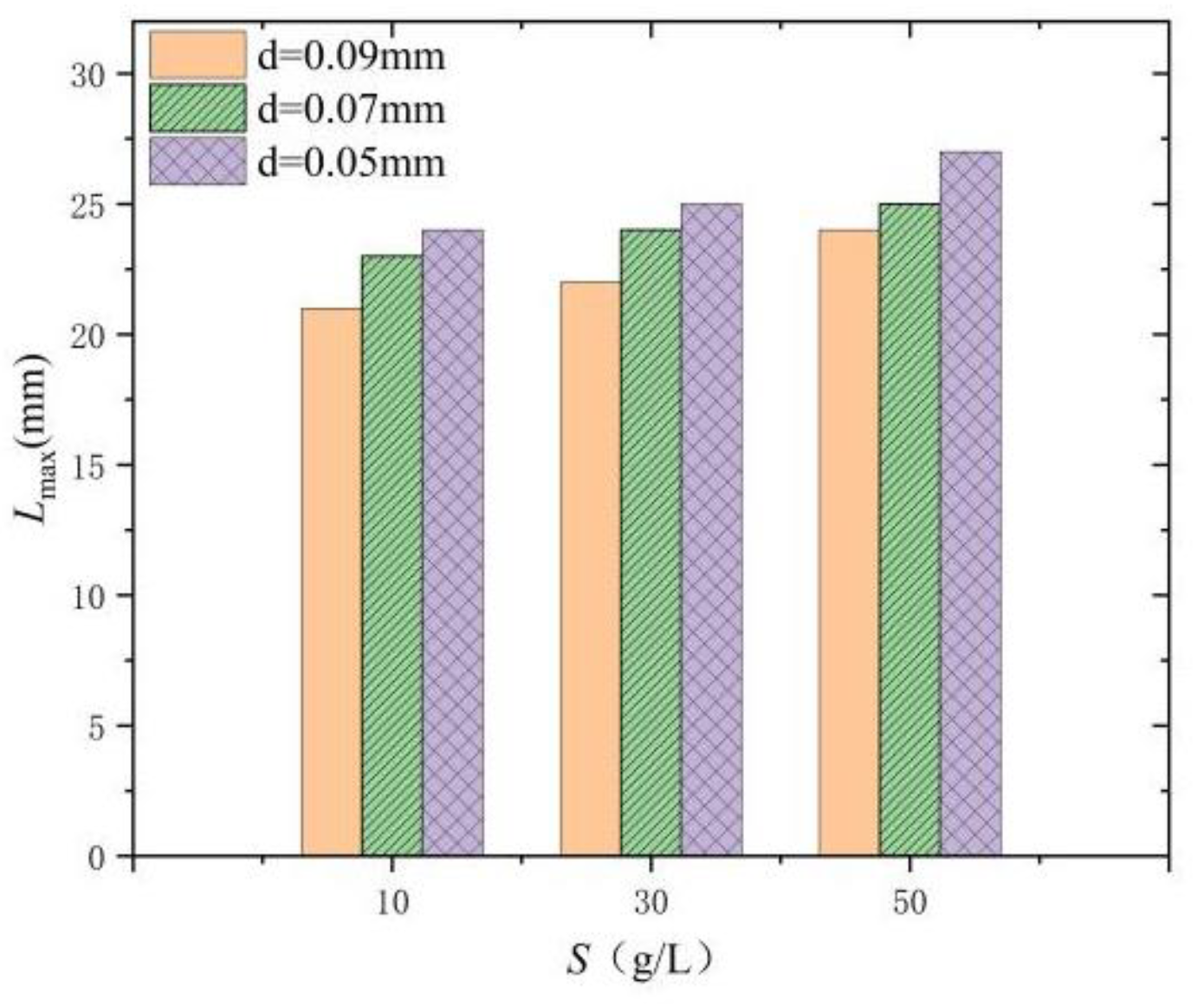
2.3.3. Maximum Length of the Wall-Bounded Cavitation Cloud

The average maximum cavitation cloud length L_max before shedding was calculated over 10 cycles at a flow of 294 mL/s, yielding L_max = 19 mm in clear water. The influences of the sediment size and sediment concentration on this length are illustrated in Figure 8. It is evident that both increasing sediment concentration and decreasing sediment size contribute to an expansion of the cavitation cloud, as indicated by the increased length. Notably, the magnitude of these increases is comparable, suggesting that the sediment size and concentration exert similar influences on the cavitation cloud length.

As shown in Table 2 and Figure 8, L increases with the sediment-laden water’s viscosity. The sediment facilitates the formation of additional cavitation nuclei compared to a clear water medium, which subsequently leads to the generation of more cavitation bubbles and promotes cavitation effectively. Smaller sediment generates a greater number of cavitation nuclei, further enhancing the development of cavitation.

3. Numerical Calculation

3.1. Mathematical Model

3.1.1. Solid–Liquid Mixture Model

The mixture model is employed to manage solid–liquid flow by accounting for the mixed states of multiple substances (phases), which is used to analyze the movement of sediment-laden water flow in this paper. In the model, the average velocity is defined as follows:

$${\stackrel{\rightharpoonup }{u}}_m={\displaystyle \frac{{\alpha }_w{\rho }_w{\stackrel{\rightharpoonup }{u}}_w+{\alpha }_s{\rho }_s{\stackrel{\rightharpoonup }{u}}_s}{{\rho }_m}}$$

(2)

where ${\rho }_m={\alpha }_w{\rho }_w+{\alpha }_s{\rho }_s$ is the density of sediment-laden water, ${\stackrel{\rightharpoonup }{u}}_m$ is the velocity of sediment-laden water, ${\alpha }_w$ and ${\alpha }_s$ denote the water’s and sediment’s volume fraction, respectively, ${\rho }_w$ and ${\rho }_s$ represent the water’s and sediment’s density, respectively, and ${\stackrel{\rightharpoonup }{u}}_w$ and ${\stackrel{\rightharpoonup }{u}}_s$ signify the water’s and sediment’s velocity, respectively.

The continuity equation is:

$${\displaystyle \frac{\partial {\rho }_m}{\partial t}}+\mathsf{\nabla }\cdot \left({\rho }_m{\stackrel{\rightharpoonup }{u}}_m\right)=0$$

(3)

The momentum equation is:

$$\begin{array}{l}{\displaystyle \frac{\partial }{\partial t}}\left({\rho }_m{\stackrel{\rightharpoonup }{u}}_m\right)+\mathsf{\nabla }\cdot \left({\rho }_m{\stackrel{\rightharpoonup }{u}}_m{\stackrel{\rightharpoonup }{u}}_m\right)\\ =-\mathsf{\nabla }P+\mathsf{\nabla }\cdot \left[{\mu }_m\left(\mathsf{\nabla }{\stackrel{\rightharpoonup }{u}}_m+\mathsf{\nabla }{\stackrel{\rightharpoonup }{u}}_m^T\right)\right]+{\rho }_m\stackrel{\rightharpoonup }{g}+\mathsf{\nabla }\cdot \left({\displaystyle \sum _{i=1}^n}{\alpha }_p{\rho }_p{\stackrel{\rightharpoonup }{u}}_{drt}{\stackrel{\rightharpoonup }{u}}_{drt}\right)\end{array}$$

(4)

where n represents the number of phases, ${\mu }_m$ is the viscosity of sediment-laden which can be obtained from Table 1, ${\alpha }_p$, ${\rho }_p$, and ${\stackrel{\rightharpoonup }{u}}_p$ represent the volume fraction, density and velocity of phase p, respectively, and ${\stackrel{\rightharpoonup }{u}}_{drt}={\stackrel{\rightharpoonup }{u}}_p-{\stackrel{\rightharpoonup }{u}}_m$ represents the drift velocity of phase p.

The volume fraction equation is:

$${\displaystyle \frac{\partial \left({\alpha }_p{\rho }_p\right)}{\partial t}}+\mathsf{\nabla }\cdot \left({\alpha }_p{\rho }_p{\stackrel{\rightharpoonup }{u}}_p\right)=0$$

(5)

3.1.2. Solid–Liquid–Vapor Mixture Model

After completing the numerical calculation of the water–sand flow, cavitation is taken into account, and a three-phase calculation of solid–liquid–vapor is carried out. Here, the sediment-laden water is regarded as a whole, and it is combined with vapor to form a mixture model. Equation (2) is rewritten as:

$${\stackrel{\rightharpoonup }{u}}_{mv}={\displaystyle \frac{{\alpha }_{ws}{\rho }_{ws}{\stackrel{\rightharpoonup }{u}}_{ws}+{\alpha }_v{\rho }_v{\stackrel{\rightharpoonup }{u}}_v}{{\rho }_{mv}}}$$

(6)

where ${\rho }_{mv}={\alpha }_{ws}{\rho }_m+{\alpha }_v{\rho }_v$ is the mixed density of sediment-laden water and vapor, ${\stackrel{\rightharpoonup }{u}}_{mv}$ is the velocity of sediment-laden water and vapor, ${\alpha }_{ws}$ and ${\alpha }_v$ denote the sediment-laden water’s and vapor’s volume fraction, respectively, ${\rho }_{ws}$ and ${\rho }_v$ represent the sediment-laden water’s and vapor’s density, respectively, and ${\stackrel{\rightharpoonup }{u}}_{ws}$ and ${\stackrel{\rightharpoonup }{u}}_v$ signify the sediment-laden water’s and vapor’s velocity, respectively.

The continuity equation is rewritten as:

$${\displaystyle \frac{\partial {\rho }_{mv}}{\partial t}}+\mathsf{\nabla }\cdot \left({\rho }_{mv}{\stackrel{\rightharpoonup }{u}}_{mv}\right)=0$$

(7)

The momentum equation is:

$$\begin{array}{l}{\displaystyle \frac{\partial }{\partial t}}\left({\rho }_{mv}{\stackrel{\rightharpoonup }{u}}_{mv}\right)+\mathsf{\nabla }\cdot \left({\rho }_{mv}{\stackrel{\rightharpoonup }{u}}_{mv}{\stackrel{\rightharpoonup }{u}}_{mv}\right)\\ =-\mathsf{\nabla }P+\mathsf{\nabla }\cdot \left[{\mu }_{mv}\left(\mathsf{\nabla }{\stackrel{\rightharpoonup }{u}}_{mv}+\mathsf{\nabla }{\stackrel{\rightharpoonup }{u}}_{mv}^T\right)\right]+{\rho }_{mv}\stackrel{\rightharpoonup }{g}+\mathsf{\nabla }\cdot \left({\displaystyle \sum _{p=1}^n}{\alpha }_p{\rho }_p{\stackrel{\rightharpoonup }{u}}_{drt}{\stackrel{\rightharpoonup }{u}}_{drt}\right)\end{array}$$

(8)

where n represents the number of phases, ${\mu }_{mv}={\alpha }_{ws}{\mu }_m+{\alpha }_v{\mu }_v$ is the mixed viscosity of sediment-laden and vapor, the phase p denotes the sediment-laden and vapor, and ${\stackrel{\rightharpoonup }{u}}_{drt}={\stackrel{\rightharpoonup }{u}}_p-{\stackrel{\rightharpoonup }{u}}_{mv}$ represents the drift velocity of the phase p.

The Zwart-Gerber-Belamri cavitation model is used to determine the volume fraction of cavitation bubbles. The transport equation for the mass transfer between vapor and sediment-laden water is:

$${\displaystyle \frac{\partial \left({\alpha }_v{\rho }_v\right)}{\partial t}}+\mathsf{\nabla }\cdot \left({\alpha }_v{\rho }_v{\stackrel{\rightharpoonup }{u}}_v\right)=\dot{R}$$

(9)

where the subscript v is the vapor phase, ${\alpha }_v$ is the volume fraction of the vapor, ${\rho }_v$ is the density of the vapor, ${\stackrel{\rightharpoonup }{u}}_v$ represents the velocity of the vapor, and $\dot{R}$ is the net mass transfer rate between the vapor and the sediment-laden water.

$${\dot{R}}_e=F_{\nu ap}{\displaystyle \frac{{\rho }_{\nu }{\rho }_{ws}}{{\rho }_{mv}}}{\alpha }_v(1-{\alpha }_v){\displaystyle \frac{3}{R_B}}\sqrt{{\displaystyle \frac{2\left(p_v-p\right)}{{\rho }_{ws}}}}\left(p_v>p\right)$$

(10)

$${\dot{R}}_c=F_{cond}{\displaystyle \frac{{\rho }_v{\rho }_{ws}}{{\rho }_{mv}}}{\alpha }_v(1-{\alpha }_v){\displaystyle \frac{3}{R_B}}\sqrt{{\displaystyle \frac{2\left(p_v-p\right)}{{\rho }_{ws}}}}\left(p_v<p\right)$$

(11)

where R_B is the radius of the cavitation bubble, ${\rho }_{ws}$ is the density of sediment-laden water, $F_{\nu ap}$ is the evaporation coefficient, and $F_{\nu ap}=50$, $F_{cond}$ is the condensation coefficient, $F_{cond}=0.2$.

In the early stage of our research, the SST k-ω and RNG k-ε models were used to carry out the numerical calculation of cavitation in clear water. It was found that the maximum length value of the cavitation cloud calculated by the RNG k-ε model was closer to the experimental result. Therefore, when calculating the cavitation of sand-laden water, the RNG k-ε model is still adopted.

3.2. Grid Generation

Considering the symmetry of the flow channel, a half geometric model was established as shown in Figure 9a. The geometric model presented herein features a regular structure and employs a structured mesh. Given that cavitation occurs near the wall, and reverse flow is present in that region, it is essential to refine the mesh near the wall during the meshing process to enhance the simulation accuracy. The first layer of mesh nodes closest to the wall was set to y⁺ = 1, comprising a total of 27 boundary layer mesh layers. Furthermore, since cavitation typically occurs in the throat of the Venturi structure, the mesh in this area was also refined, as depicted in Figure 9b.

Grid independence verification was performed to reduce the dependency of the calculation results on the grid. Numerical calculations were carried out using five grid schemes for a clear water flow rate of 294 mL/s, with the grid configurations listed in

Table 3. Grid scheme.

Scheme	1	2	3	4	5
Grid number	2,351,584	3,569,521	5,665,841	7,670,400	8,641,851

. The experimental measurements obtained inlet and outlet pressures of 144,325 Pa and 92,325 Pa, respectively. Seven monitoring points were selected starting from the throat, 0.2 mm away from the wall, and at 3 mm intervals along the flow direction, as shown in Figure 10a. The pressures at these seven points at time t = T/2 were plotted to show the pressure distribution along the flow path, as depicted in Figure 10b. According to the results in Figure 10b, the pressure values of Grid 4 and Grid 5 were very close, with their pressure curves basically overlapping. Further increasing the number of grids led to almost no noticeable change in the pressure at the monitoring points. Therefore, the grid with 7,670,400 cells was selected.

3.3. Boundary Conditions and Parameter Settings

In the cavitation experiments of sediment-laden water, the outlet pressure remains constant while the inlet pressure varies with the particle size and sediment concentration. When d = 0.05 mm and Q = 294 mL/s, for the sediment concentrations of 30 g/L and 50 g/L, the measured corresponding inlet pressures were 146,360 Pa and 152,340 Pa, respectively. The inlet boundary condition adopts a velocity inlet, with vin = 1.44 m/s, and the inlet pressure is taken as the experimental value. The outlet boundary condition is set as a pressure outlet, with pout = 92,325 Pa. The turbulent intensities at the inlet and outlet were set according to the hydraulic diameter. The turbulent intensity at the inlet was 5%, and the turbulent viscosity ratio was 10. The backflow turbulent intensity was 5%, and the backflow turbulent viscosity ratio was 10. The time step was set to be 1 × 10⁻³ s. The pressure–velocity coupling equations were solved based on the SIMPLEC algorithm, and the residual was set to be 1 × 10⁻³. The discretization format of pressure is PRESTO!, the discretization format of the gradient is Least Squares Cell-Based, and the discretization format of the remaining parameters was selected as the first-order upwind scheme.

The vapor density ${\rho }_{\mathrm{v}}=0.017{\mathrm{kg}/\mathrm{m}}^3$, vapor viscosity ${\mu }_{\mathrm{v}}=1.00\times {10}^{-2}\mathrm{mPa}\cdot \mathrm{s}$, saturated vapor pressure $p_v=2236\mathrm{Pa}$, bubble radius R_B = 1 × 10⁻⁵ m, temperature T = 293.15 K, the sand density ${\rho }_s=2600{ \mathrm{kg}/\mathrm{m}}^3$, and the viscosity of the sediment-laden water were determined based on the experimental values.

3.4. Analysis of the Calculation Results

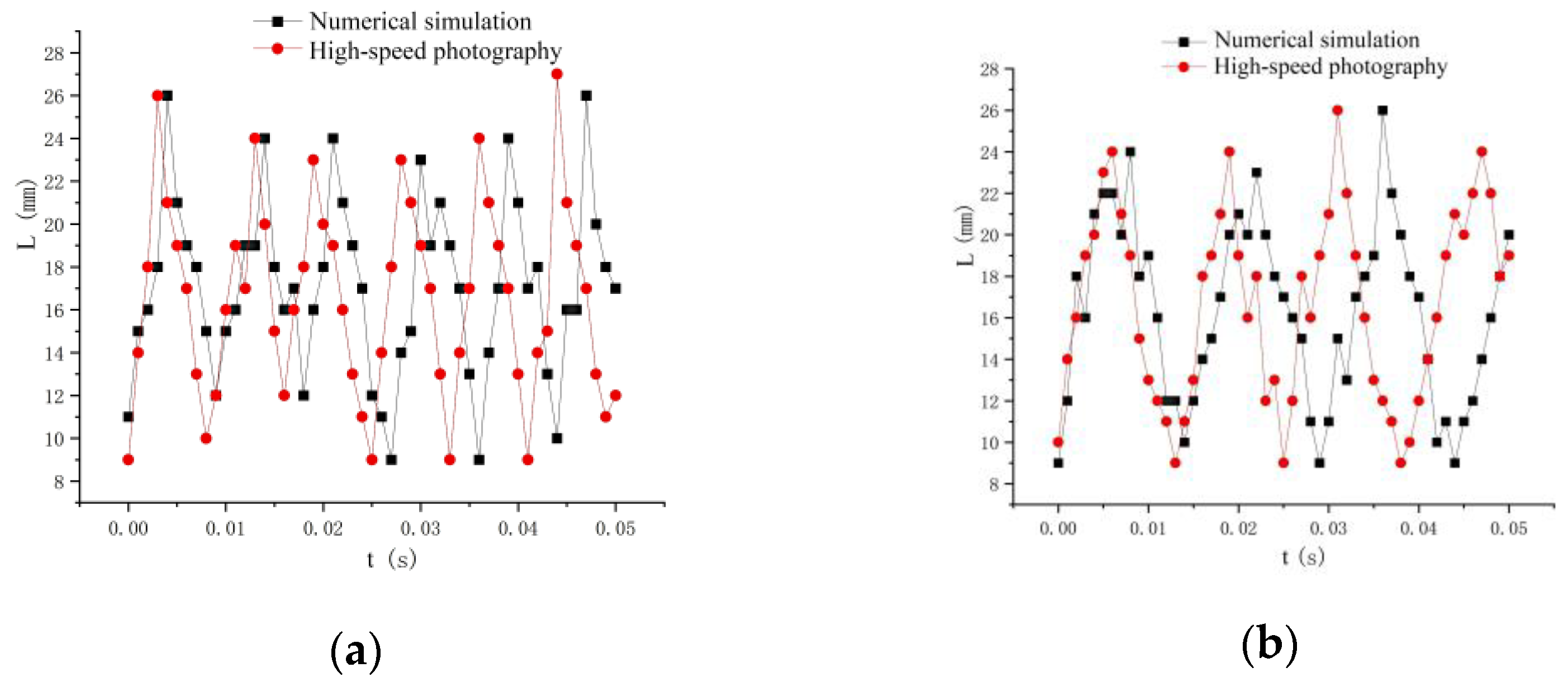
3.4.1. Comparison of the Cavitation Cloud Morphology and Periodicity

The experimental photos and numerical simulation images of cavitation alongside the vapor volume fraction distribution from numerical simulations of sediment-laden water, with sediment sizes of d = 0.05 mm and sediment concentrations of S = 50 g/L and S = 30 g/L over one cycle are shown in Figure 11. Cavitation initiates at the throat, and as the cycle progresses, the wall-attached cavitation cloud’s length and thickness increase, thereby expanding the cavitation region. During the interval from 2/6T to 5/6T, shedding cavitation clouds form at the tail, moving with the flow before gradually collapsing. Alongside the periodic development of cavitation, the vapor–liquid re-entrant jet at the tail during cavitation collapse exhibits similar periodicity. The static pressure near the collapsing cavitation bubbles at the tail increases rapidly, resulting in the formation of a concave jet structure. A portion of the liquid at the tail, driven by the concave jet, moves in the opposite direction to the main liquid flow, generating a vapor–liquid coexisting re-entrant jet. The numerical simulations capture the periodicity of the cavitation process, which undergoes cyclical phases of contraction, expansion, and retraction. Notably, the shape of the wall-attached cavitation region in the simulations aligns with the non-shed cavitation cloud observed in the experiments, and the extent of the cavitation region closely resembles the experimental findings.

The length of the cavitation cloud L at the corresponding moment can be found from Figure 11. The ratio of this length L to the maximum length of the cavitation cloud Lmax that appears within a cycle is defined as Lc (i.e., Lc = L/Lmax). The Lc values from the experiment and numerical simulation are compared, as shown in

Table 4. Comparison of Lc between experiment and numerical simulation.

S (g/L)	t	Experiment Lc	Numerical Simulation Lc	Error (%)
50	0	0.36	0.39	8.33
50	1/6T	0.59	0.56	−5.08
50	2/6T	0.87	0.98	12.64
50	4/6T	0.65	0.43	−12.31
50	5/6T	0.41	0.36	−12.20
30	0	0.35	0.28	−8.57
30	1/6T	0.67	0.58	−13.43
30	2/6T	0.83	0.80	−3.61
30	4/6T	0.77	0.75	−2.60
30	5/6T	0.34	0.32	−5.88

. The error range is between 2.6% and 13.43%, and it is considered that the numerical simulation is consistent with the variation in the actual cavitation cloud length.

The values of L_max in sediment-laden water before shedding, were compared experimentally and numerically, as shown in Figure 12. It is found that the numerically calculated cavitation cloud length closely aligns with the experimental results; however, the evolution cycle of the cavitation cloud in the simulation is slightly longer than that observed in the experiments. For a sediment concentration of S = 50 g/L, the experimental cycle is T = 0.0075 s, while the calculated cycle is T = 0.0089 s, indicating an 18.6% difference. Conversely, at a sediment concentration of S = 30 g/L, the experimental cycle is T = 0.0130 s, and the calculated cycle is T = 0.0151 s, resulting in a 16.1% difference. As the sediment concentration increases, the cavitation cloud cycle shortens while the cavitation region expands. The numerical results agree with the experiment outcomes.

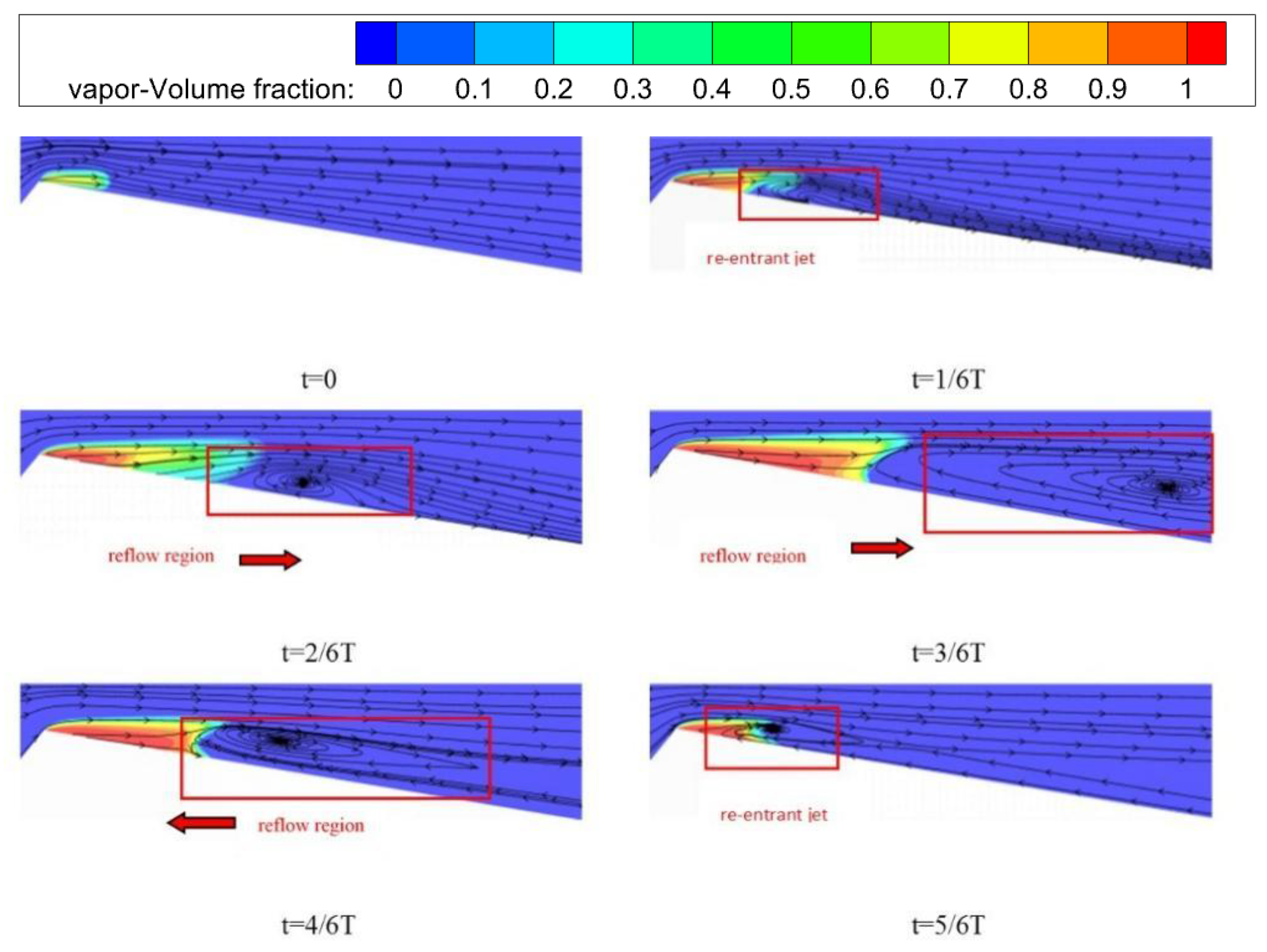
3.4.2. Re-Entrant Jet and Reflow

The reverse pressure gradient of the diffusion section generates a re-entrant jet which causes the detachment of bubbles at the tail end of the cavitation cloud. The streamline diagrams of sediment-laden water cavitation at a sediment concentration of S = 50 g/L are shown in Figure 13. At t = 1/6 T, a re-entrant jet emerges at the wall near the cavitation cloud tail, resulting in an upward lift of the tail. At t = 2/6 T, as the cavitation cloud expands and the tail continues to rise, the reflow region is obvious. By t = 3/6T, the cavitation cloud attains its maximum length, with the reflow region moving further away and expanding to its greatest extent. At t = 4/6T, due to the collapse of the detached cavitation cloud, the reflow region is pushed back and the cavitation cloud near the wall begins to contract. By t = 5/6T, the contraction of the cavitation cloud persists, and the reflow region continues to shift upstream toward the tail, further reducing in size, and it finally becomes a re-entrant jet.

A comparative analysis of the streamlines of cavitation flows, maintaining the same flow rate for sediment-laden water at a sediment concentration of S = 30 g/L and S = 50 g/L (Figure 14) reveals that an increased sediment concentration correlates with a larger reflow region at the tail of the cavitation cloud.

From the throat to the downstream diffusion section, the flow velocity decreases along the way. The density and inertia of the sediment-laden water increase when the concentration is raised. Under the same flow rate conditions, the trend of decreasing flow velocity slows down. Therefore, the velocity gradient from the edge wall to the central flow area rises accordingly.

Meanwhile starting from the throat, a small amount of sediment settles and accumulates on the side walls, while most of the sediment particles are suspended in the water and carried downstream by the water flow, forming the main flow area. There are strip-shaped areas with a low sediment content between the subsidence zone and the mainstream. As the overall sediment concentration decreases, this strip-shaped area approaches clear water (Figure 15).

Based on the analysis of the velocity gradient and sediment distribution, the shearing stress $\tau =\mu {\displaystyle \frac{\partial v_x}{\partial y}}$ near the wall enlarges, and the drag on bubbles enhances with the concentration increasing, which results in an increase in the length of the sheet-like cavitation cloud. Therefore, the reverse pressure gradient at the cavitation cloud tail increases, leading to an expanded re-entrant jet as shown in Figure 14a. The collapse of detached bubbles pushes the reflow region upstream, causing the cavitation cloud to contract. The higher the sediment content, the more obvious the driving effect, and the reflow region increases significantly as shown in Figure 14b.

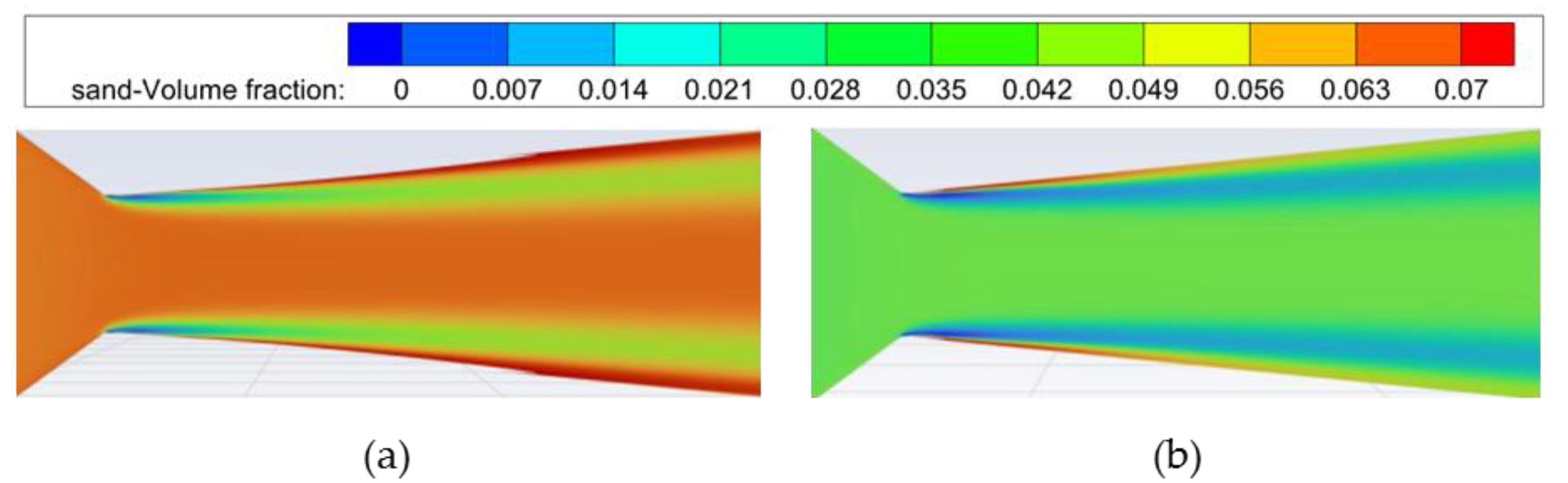
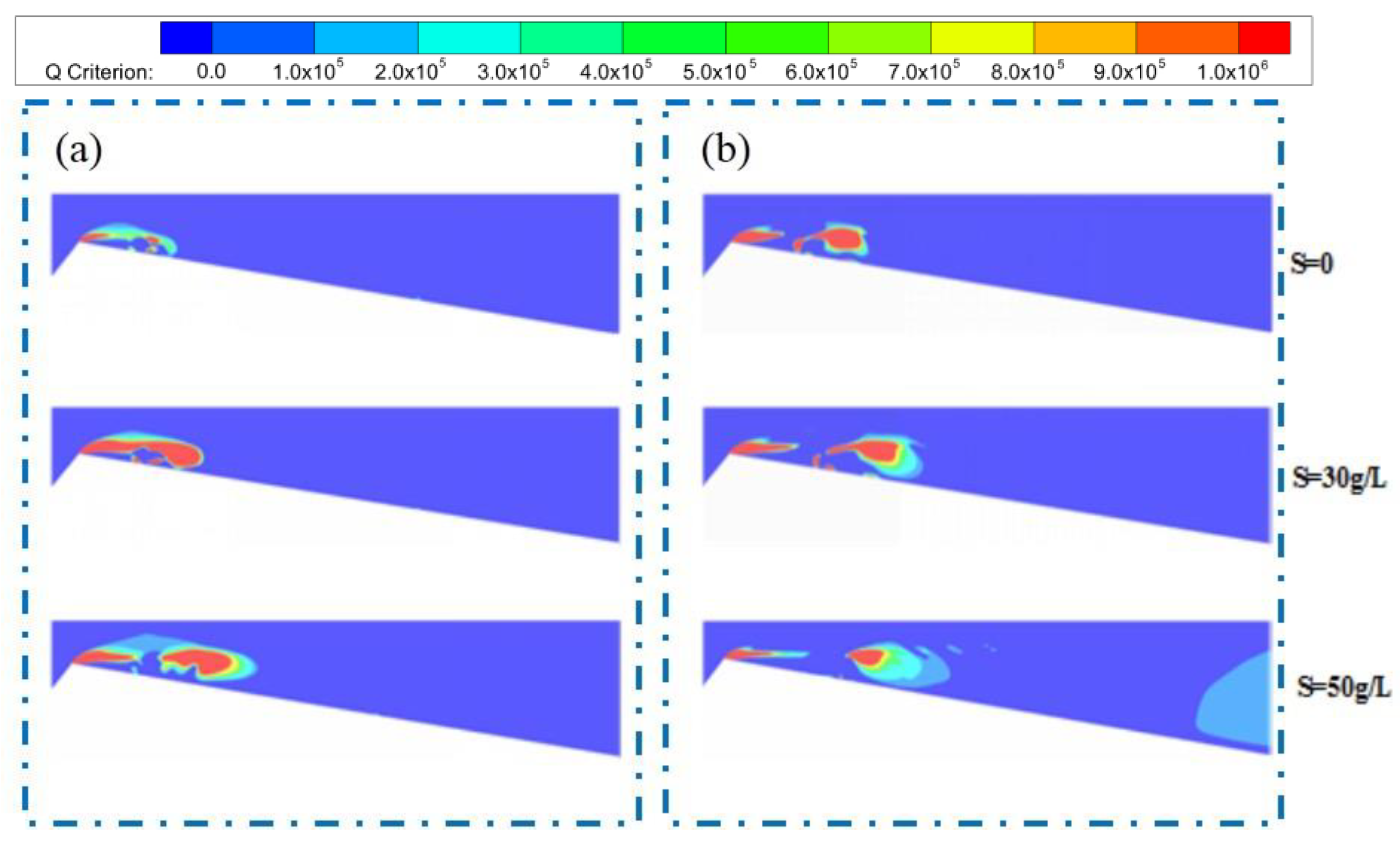
3.4.3. Vortex Distribution and Evolution

The cavitation behavior significantly affects the vortex structure. Because of the growth and shedding process of bubbles, the vortex structure changes over time, and the absolute value of vorticity near the wall is relatively large. This originates from the flow’s strong shearing effect near the wall. The smooth vortex structure grows with the development of bubbles, and there is a high vorticity value at the position where the reflow advances, which disturbs the bubbles, enhances turbulent fluctuations, and causes the vortex structure to lift up. This indicates that the reflow impacts the surface of the bubbles, ultimately leading to the detachment of cloud bubbles.

The vorticity diagrams for cavitation in sediment-laden water with S = 50 g/L—calculated using the Q-criterion—are shown in Figure 16. The sheet-like vortices at the front of the cavitation cloud are relatively smooth and stable, whereas the vortices at the tail exhibit considerable periodic fluctuations due to the influence of the reflow, undergoing a cycle of shedding, downstream movement, return, and merging. From t = 1/6T to t = 3/6T, the reflow impacts the surfaces of the sheet-like cavitation bubbles, ultimately leading to cavitation cloud shedding. As the cloud progresses downstream, large-scale vortices are generated, complicating the vorticity characteristics. After t = 4/6T, the reflow propels the vortices to return, with larger vortex structures being supplanted by smaller-scale vortices.

Figure 17 compares the cavitation vorticity of clear water and sediment-laden water with a sediment concentration of 30 g/L and 50 g/L, all under identical flow rates. It is observed that during the vortices shedding phase, higher sediment concentrations enhance the shear effect near the wall, leading to an increase in vorticity. The re-entrant jet causes the shedding of vortices, and higher sediment concentrations lead to a stronger impact of the jet on the sheet-like vortices, causing more vortices to detach and the boundaries of the vortices to become more turbulent as shown in Figure 17a. During the vortices return phase, larger-scale vortices transform into small-scale vortices which exhibit greater energy consumption due to energy dissipation. In sediment-laden water with a higher sediment concentration, viscous damping is more pronounced, leading to more reduction in vorticity and more small-scale vortices as shown in Figure 17b.

4. Conclusions

This study investigates the cavitation phenomenon in sediment-laden water using a Venturi-based hydraulic cavitation device, integrating both numerical simulations and experimental methods. By comparing the numerical results with the experimental data, the study investigates cavitation patterns, analyzes the flow field characteristics associated with cavitation in sediment-laden water, and explores the influences of the sediment size and concentration on cavitation, drawing the following conclusions:

• The impact of the sediment concentration on the viscosity is more pronounced than that of the particle size. As the sediment concentration increases, the viscosity rises significantly, with this trend becoming more accentuated as the particle size decreases.
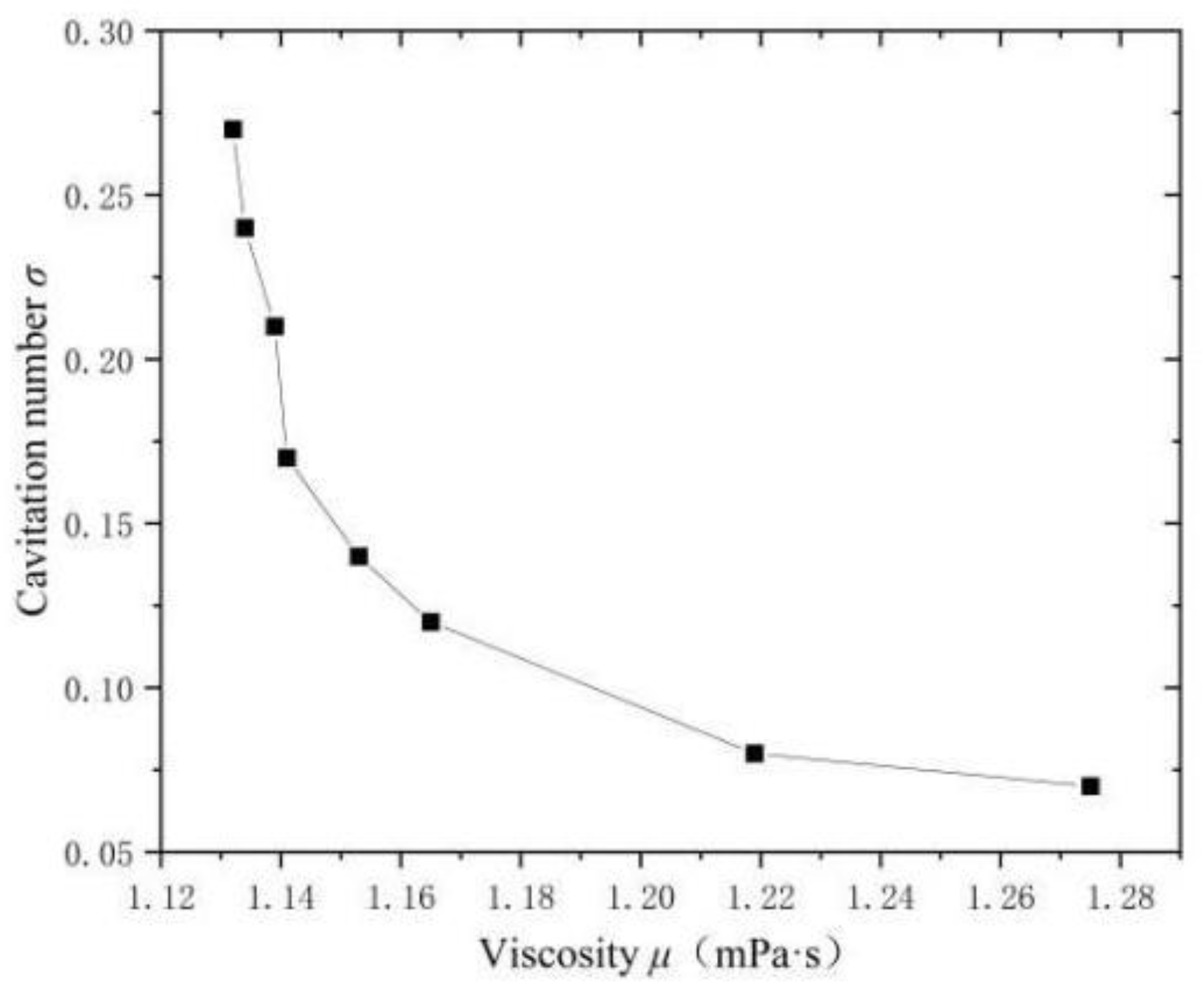
• The results of the experiment and numerical simulation show that an increase in the sediment concentration or a decrease in the sediment size leads to a shorter evolution cycle of the cavitation cloud and a higher frequency of cavitation cloud shedding, with the sediment concentration exerting a more substantial influence. The cavitation number decreases as well, with the sediment concentration having a more significant effect. When the concentration reaches 50 g/L, the cavitation number is less than 0.1. Therefore, attention should be paid to the cavitation generated when the sediment concentration is high during the operation of hydraulic machinery.
• The numerical analyses of the flow field of the cavitation in sediment-laden water reveal that the sediment expands the reflow region and enhances the re-entrant jet, thereby promoting cavitation cloud shedding due to the more pronounced shear effect. Additionally, the sediment contributes to a more complex vortex structure: on one hand, it enhances the interaction with smooth, sheet-like vortices attached to the wall, leading to increased vortex shedding; on the other hand, it consumes more energy during the vortex return, thus forming more small-scale vortices.
• When the sediment concentration increases, it can increase the viscosity of the sediment-laden water. On the one hand, it increases the pressure gradient, leading to the enhancement of the re-entrant jet and accelerating the shedding of the cavitation cloud. At the same time, the viscous resistance is strengthened, which expands the range of the cavitation zone. In engineering, the geometric shape of the runner blades of a turbine can be optimized to reduce the relative flow velocity of the fluid, thereby decreasing the pressure gradient and weakening the shear effect.

The channel depth in this article is only 4 mm, which is much smaller than the channel length and width, so a sidewall effect will occur. Sidewall effects can boost the flow resistance and pressure differentials, facilitating the occurrence of cavitation. In future research, we need to analyze the impact of depth and refine the results accordingly.

Since the fluid is regarded as incompressible in the calculation process, in fact, the shedding and collapse of cavitation bubbles should be a compressible flow process. In the follow-up, the compression factor should be taken into account to refine the calculation model. At the same time, due to the narrow channel, the influence of the roughness of the side wall on cavitation should also be considered, which also needs to be optimized and improved in the subsequent calculations.