Bubble Motion and Interfacial Phenomena during Bubbles Crossing Liquid–Liquid Interfaces
Abstract
:1. Introduction
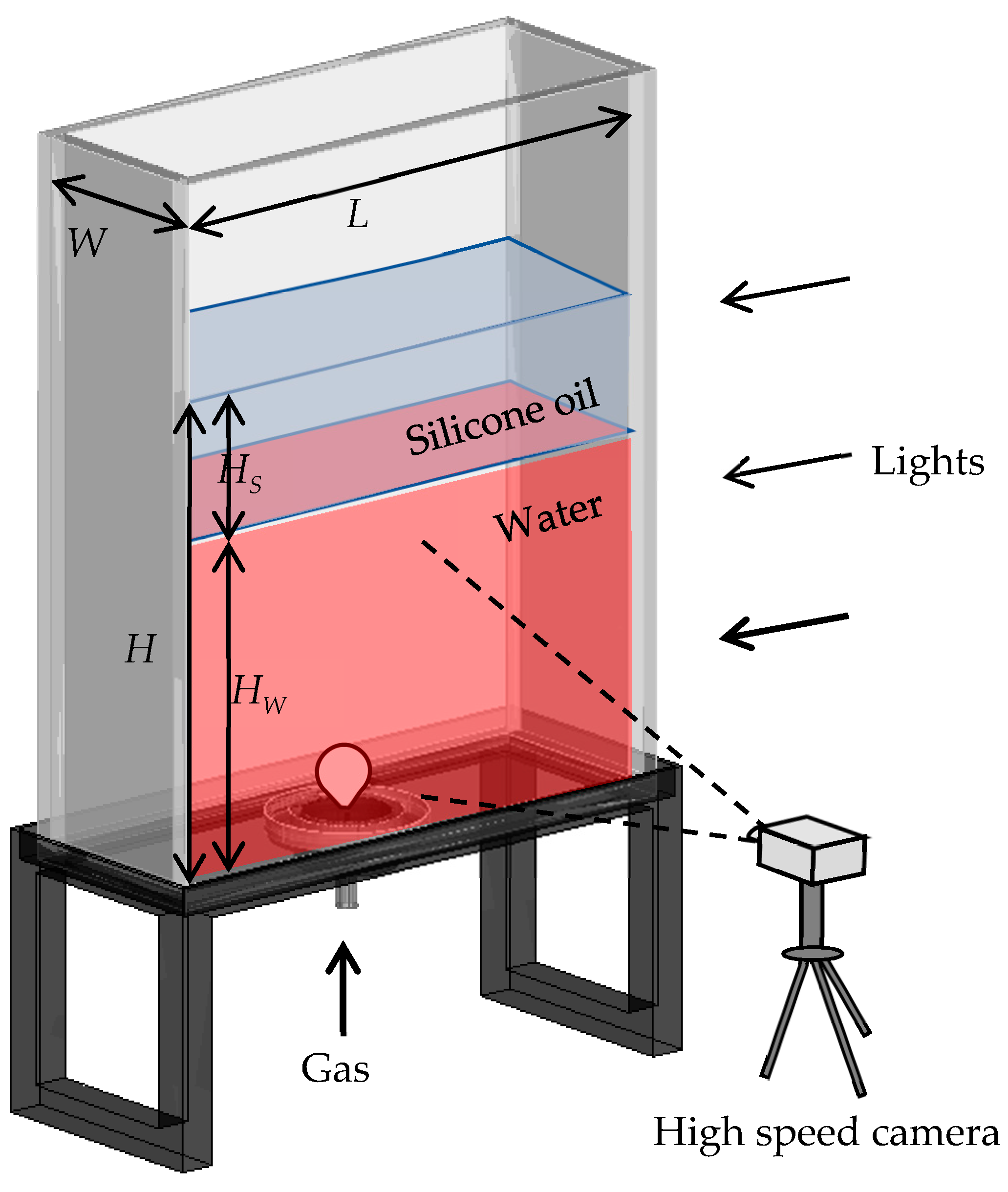
2. Experimental
3. Results and Discussions
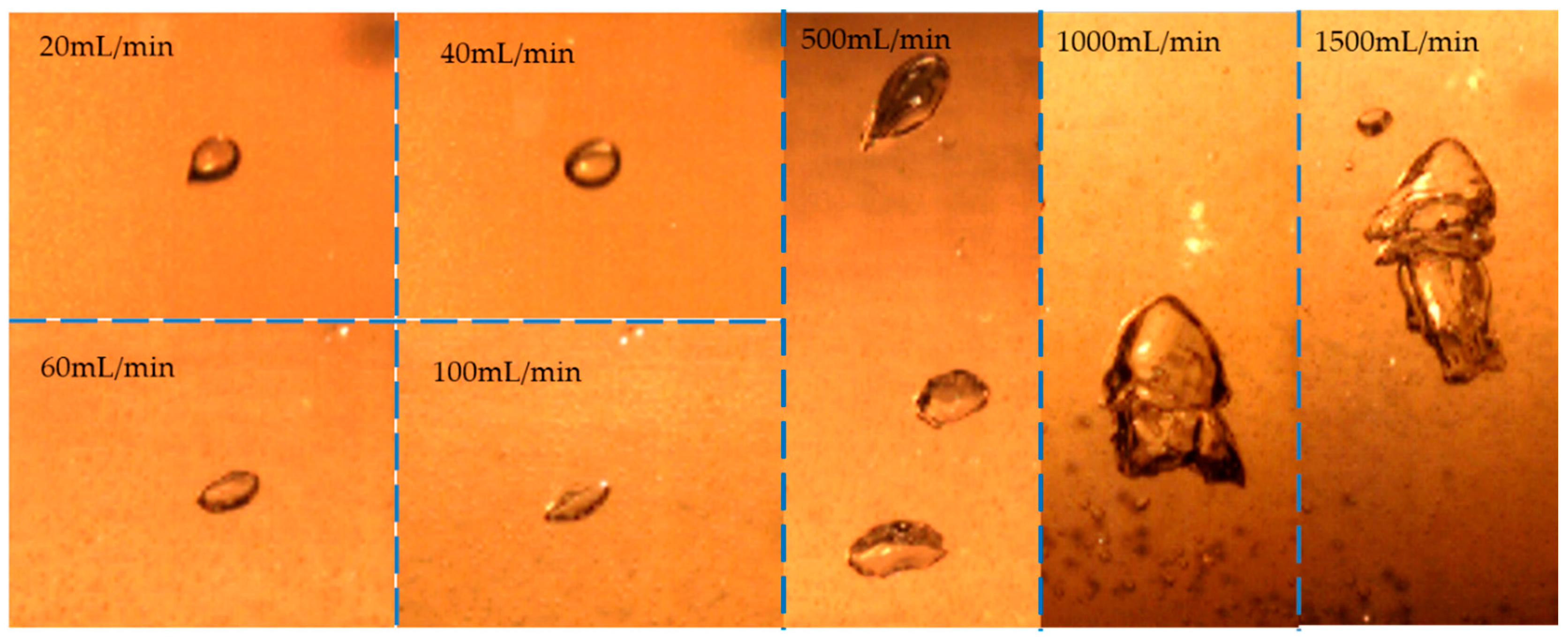
3.1. Bubble Shape and Moving Path
3.2. Bubble Residence Time at Liquid–Liquid Interface
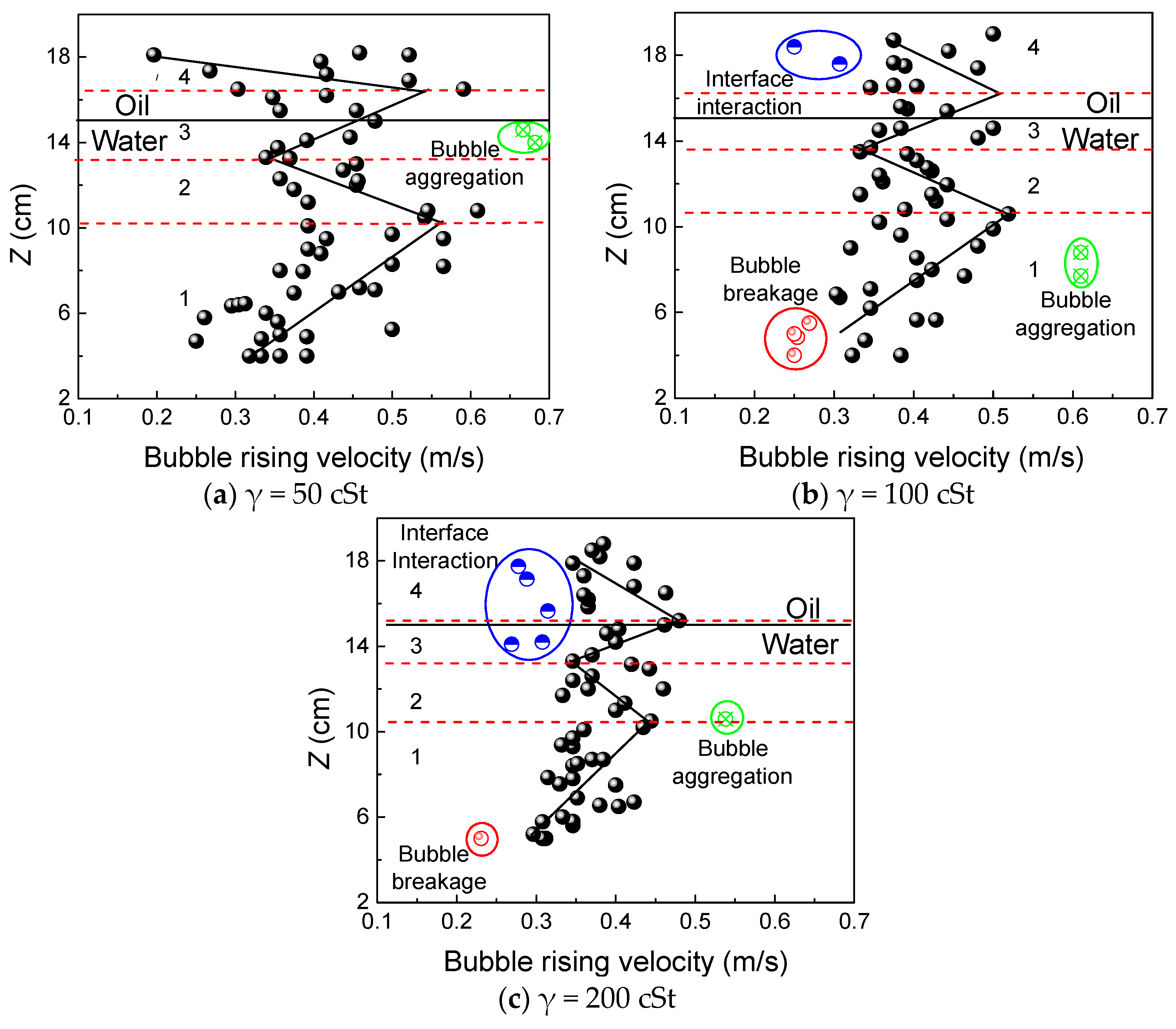
3.3. Bubble Rising Velocity
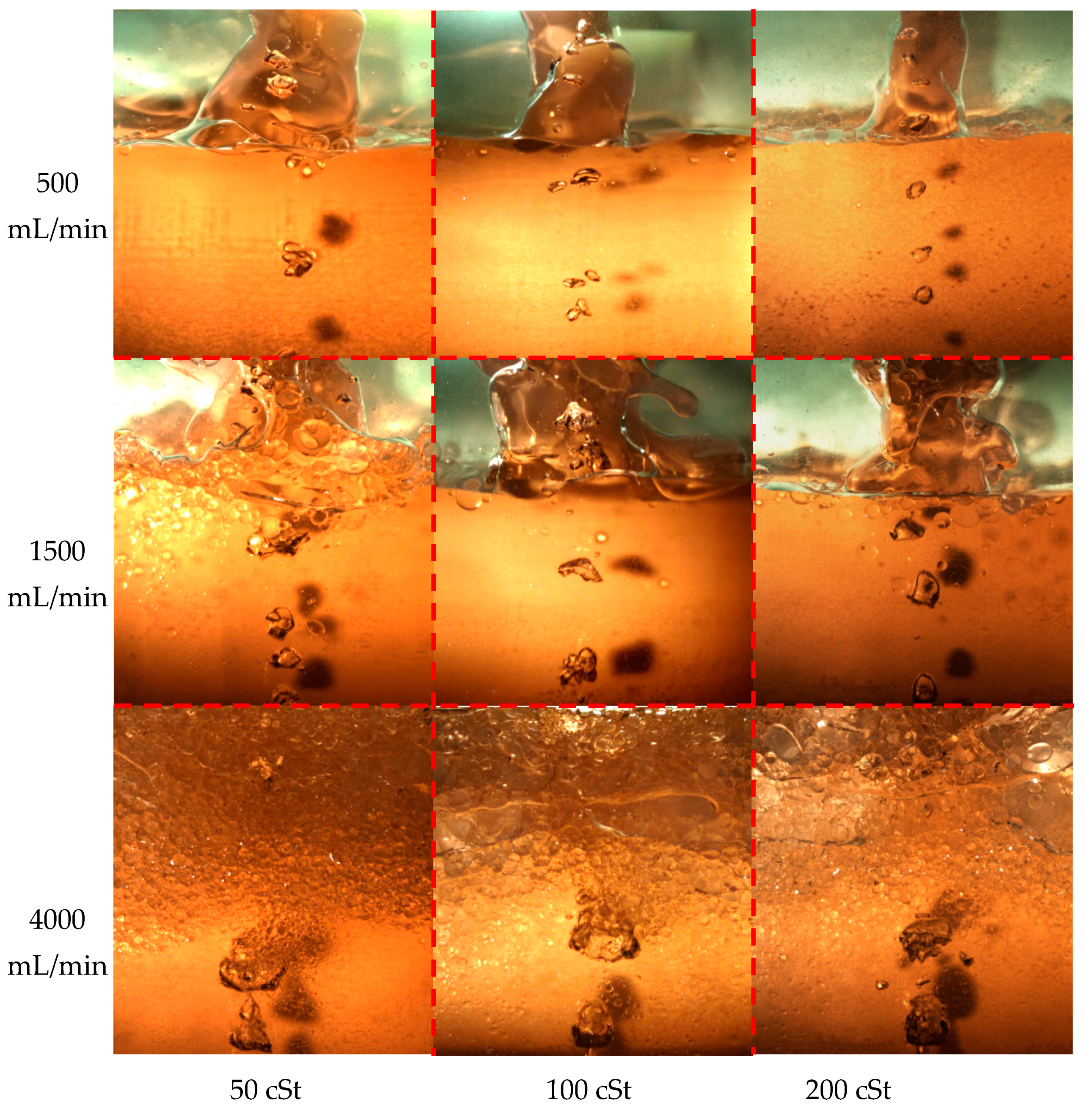
3.4. Liquid–Liquid Interface Distribution and Phase Entrainment
- (a)
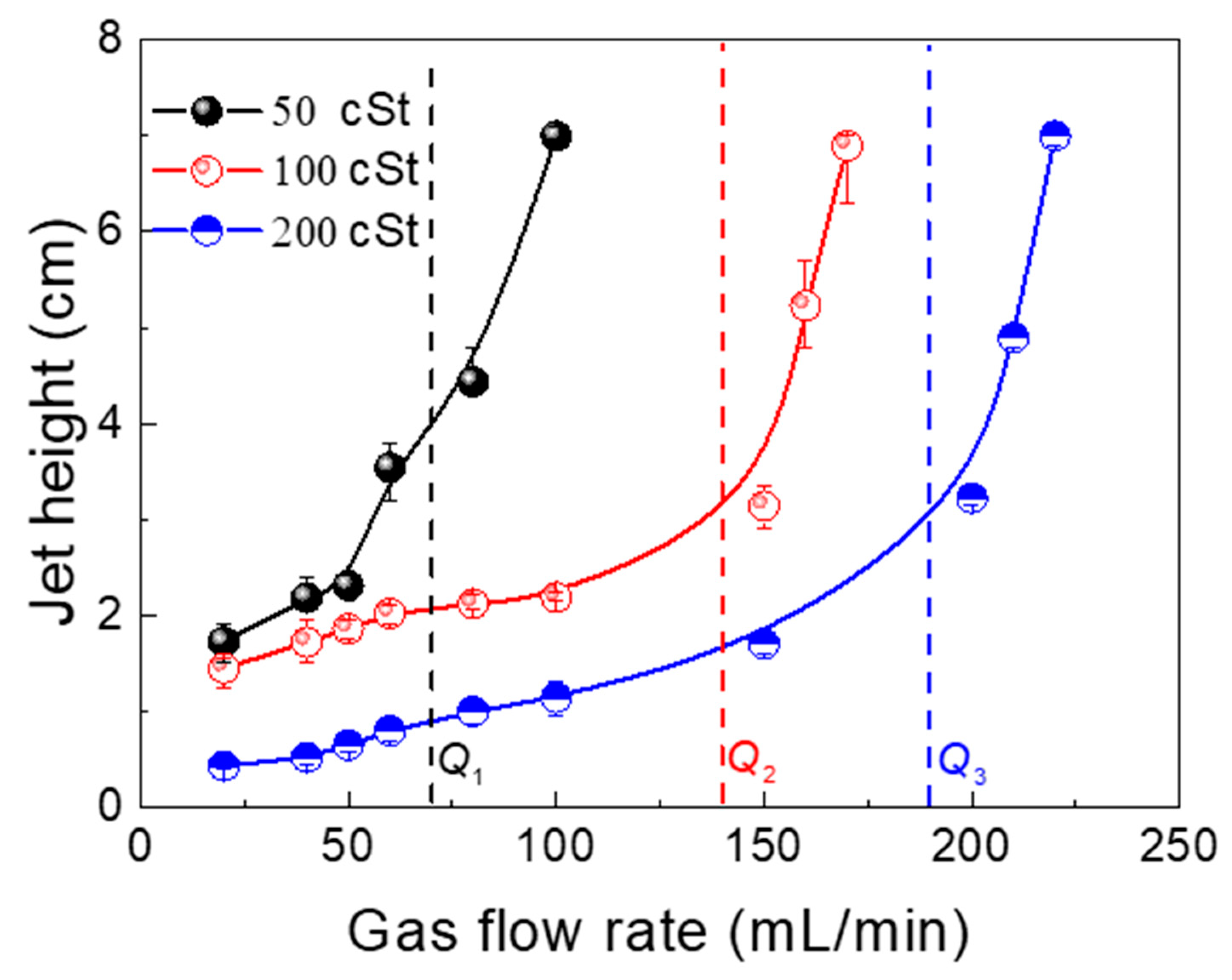
- Firstly, as shown in Figure 7a, the micro water-phase entrainment in the oil phase was generated by the interfacial fracture when a bubble crosses the interface at a low gas flow rate (Q < 50 mL/min). The micro water-phase entrainment generated in this case was sensitively disturbed by the fluid flow and dropped very slowly without gas injection. The micro water-phase entrainment is difficult to separate from the oil phase layer. When increasing oil viscosity, the micro water-phase entrainment will be intensified.
- (b)
- The second mechanism of water-phase entrainment at the mesoscale was an oil film escaping from the bubble surface, as shown in Figure 7b. This path was the main entrainment generation method at gas flow rates of <100 mL/min. The size of the mesoscale entrainment increases when increasing the gas flow rate and separates from the oil phase layer quickly without gas injection.
- (c)
- As shown in Figure 7c, at large gas flow rates (100–500 mL/min), the unstable water/oil interface generated would reach the top surface of the oil phase. Large-scale water-phase entrainment occurred via breakage of the column interface. The large-scale entrainment in this case would quickly drop to the water phase. Increasing the oil viscosity could strengthen the water/oil interface, which is more difficult to break.
- (d)
- The last mechanism was associated with higher gas flow rates (>1000 mL/min). The oil and water mixed and penetrated each other with strong stirring of the gas phase, as shown in Figure 7d. There was no clear water/oil interface. The mixed entrainment took a long time to separate.
3.5. Phase Distribution
4. Conclusions
- (1).
- The second phase (oil phase) can reduce the rise rate of a bubble as it passes through the liquid–liquid interface. The greater the viscosity, the more time required for a bubble to pass through the interface.
- (2).
- The bubble rise rate decreases sharply as it crosses the interface because it must overcome interfacial tension and viscous drag during this process. Therefore, when the atmospheric amount is used, the bubble is mainly affected by the “liquid column” and deformation during ascension. The velocity distribution is M-shaped at this time.
- (3).
- Phase entrainment can occur via four mechanisms, and increasing the viscosity of the second phase (oil phase) can suppress the generation of the fine entrained phase.
Author Contributions
Funding
Conflicts of Interest
References
- Su, C.J.; Chou, J.M.; Liu, S.H. Effect of gas bottom blowing condition on mixing molten iron and slag inside ironmaking smelter. Mater. Trans. 2009, 50, 1502–1509. [Google Scholar] [CrossRef]
- Ma, J.; Sun, Y.S.; Li, B.W. Spectral collocation method for transient thermal analysis of coupled conductive, convective and radiative heat transfer in the moving plate with temperature dependent properties and heat generation. Int. J. Heat Mass Transf. 2017, 114, 469–482. [Google Scholar] [CrossRef]
- Ma, J.; Sun, Y.S.; Li, B.W. Simulation of combined conductive, convective and radiative heat transfer in moving irregular porous fins by spectral element method. Int. J. Therm. Sci. 2017, 118, 475–487. [Google Scholar] [CrossRef]
- Han, Z.; Holappa, L. Mechanisms of iron entrainment into slag due to rising gas bubbles. ISIJ Int. 2003, 43, 292–297. [Google Scholar] [CrossRef]
- Beskow, K.; Dayal, P.; Björkvall, J.; Nzotta, M.; Sichen, D. A new approach for the study of Slag–Metal interface in steelmaking. Ironmak. Steelmak. 2006, 33, 74–80. [Google Scholar] [CrossRef]
- Reiter, G.; Schwerdtfeger, K. Characteristics of entrainment at liquid/liquid interfaces due to rising bubbles. ISIJ Int. 1992, 32, 57–65. [Google Scholar] [CrossRef]
- Reiter, G.; Schwerdtfeger, K. Observations of physical phenomena occurring during passage of bubbles through liquid/liquid Interfaces. ISIJ Int. 1992, 32, 50–56. [Google Scholar] [CrossRef]
- Dietrich, N.; Poncin, S.; Pheulpin, S.; Li, H.Z. Passage of a bubble through a Liquid–Liquid interface. AICHE J. 2008, 54, 594–600. [Google Scholar] [CrossRef]
- Delobelle, P.; Oytana, C. Study of Slag-Metal interface in ladle treatment. Ironmak. Steelmak. 2006, 33, 454–464. [Google Scholar]
- Kobayashi, S. Iron droplet formation due to bubbles passing through molten iron/slag interface. ISIJ Int. 1993, 33, 577–582. [Google Scholar] [CrossRef]
- Ueda, Y.; Kochi, N.; Uemura, T.; Ishii, T.; Iguchi, M. Numerical observation of flow field around the water column behind a rising bubble through an oil water interface. ISIJ Int. 2011, 51, 1940–1942. [Google Scholar] [CrossRef]
- Kochi, N.; Ueda, Y.; Uemura, T.; Ishii, T.; Iguchi, M. Numerical simulation on penetration stage of a rising bubble through an Oil-Water Interface. ISIJ Int. 2011, 51, 1011–1013. [Google Scholar] [CrossRef]
- Grenier, N.; Touzé, D.L.; Colagrossi, A.; Antuono, M.; Colicchio, G. Viscous bubbly flows simulation with an interface SPH model. Ocean. Eng. 2013, 69, 88–102. [Google Scholar] [CrossRef]
- Natsui, S.; Takai, H.; Kumagai, T.; Kikuchi, T.; Suzuki, R.O. Multiphase particle simulation of gas bubble passing through liquid/liquid interfaces. Mater. Trans. 2014, 55, 1707–1715. [Google Scholar] [CrossRef]
- Dehkordi, P.B.; Colombo, L.P.M.; Mohammadian, E.; Arnone, D.; Azdarpour, A.; Sotgia, G. Study of viscous Oil-Water-Gas slug flow in a horizontal pipe. J. Pet. Sci. Eng. 2019, 178, 1–13. [Google Scholar] [CrossRef]
- Kajero, O.T.; Mukhtar, A.; Lokman, A.; James, A.B. Experimental study of viscous effects on flow pattern and bubble behavior in small diameter bubble column. Phys. Fluids 2018, 30, 093101. [Google Scholar] [CrossRef]




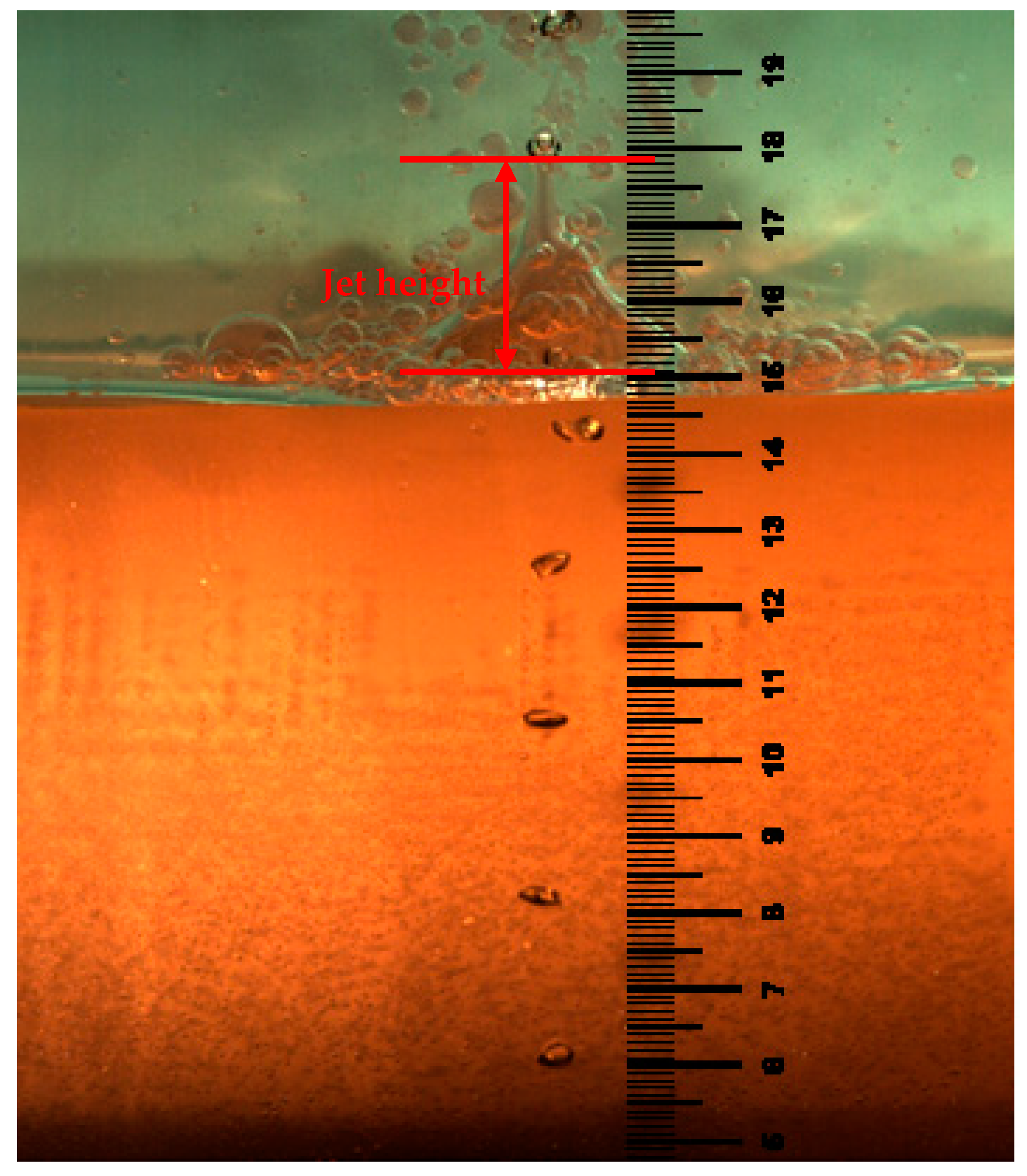
| No. | Kinematic Viscosity, cSt | Density, kg/m3 | Interface Tension, mN/m |
|---|---|---|---|
| 1 | 50 | 956 | 30.6 |
| 2 | 100 | 957 | 30.7 |
| 3 | 200 | 961 | 36.9 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, H.; Wang, J.; Zhang, W.; Xie, M.; Liu, F.; Cao, X. Bubble Motion and Interfacial Phenomena during Bubbles Crossing Liquid–Liquid Interfaces. Processes 2019, 7, 719. https://doi.org/10.3390/pr7100719
Zhao H, Wang J, Zhang W, Xie M, Liu F, Cao X. Bubble Motion and Interfacial Phenomena during Bubbles Crossing Liquid–Liquid Interfaces. Processes. 2019; 7(10):719. https://doi.org/10.3390/pr7100719
Chicago/Turabian StyleZhao, Hongliang, Jingqi Wang, Wanlong Zhang, Mingzhuang Xie, Fengqin Liu, and Xiaochang Cao. 2019. "Bubble Motion and Interfacial Phenomena during Bubbles Crossing Liquid–Liquid Interfaces" Processes 7, no. 10: 719. https://doi.org/10.3390/pr7100719
APA StyleZhao, H., Wang, J., Zhang, W., Xie, M., Liu, F., & Cao, X. (2019). Bubble Motion and Interfacial Phenomena during Bubbles Crossing Liquid–Liquid Interfaces. Processes, 7(10), 719. https://doi.org/10.3390/pr7100719





