Eulerian Multiphase Simulation of the Particle Dynamics in a Fluidized Bed Opposed Gas Jet Mill
Abstract
:1. Introduction
2. Materials and Methods
2.1. Fluidized Bed Opposed Jet Mill Unit and Material Properties
2.2. Eulerian Multiphase Modeling
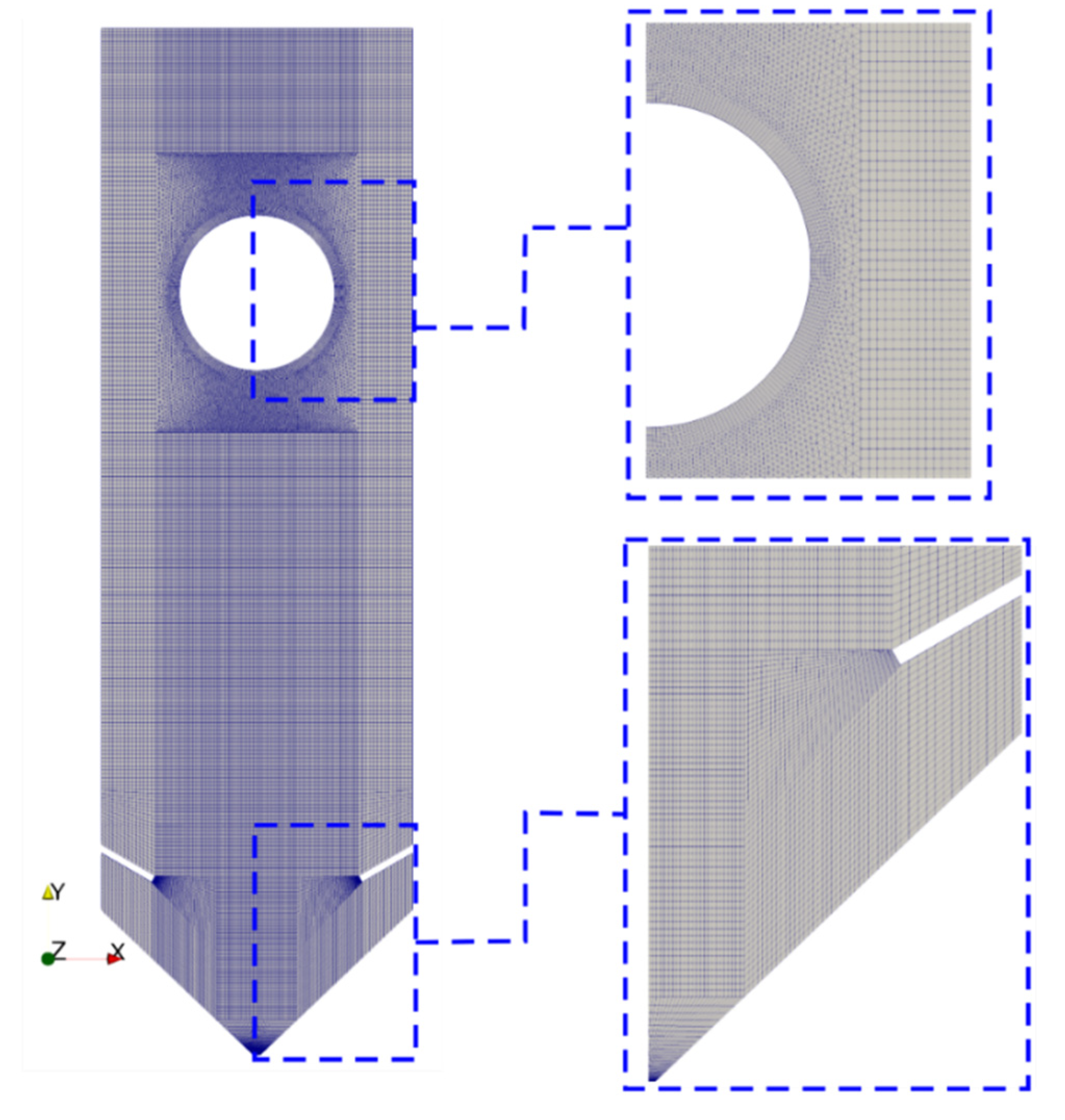
2.3. Numerical and Boundary Conditions
3. Results and Discussion
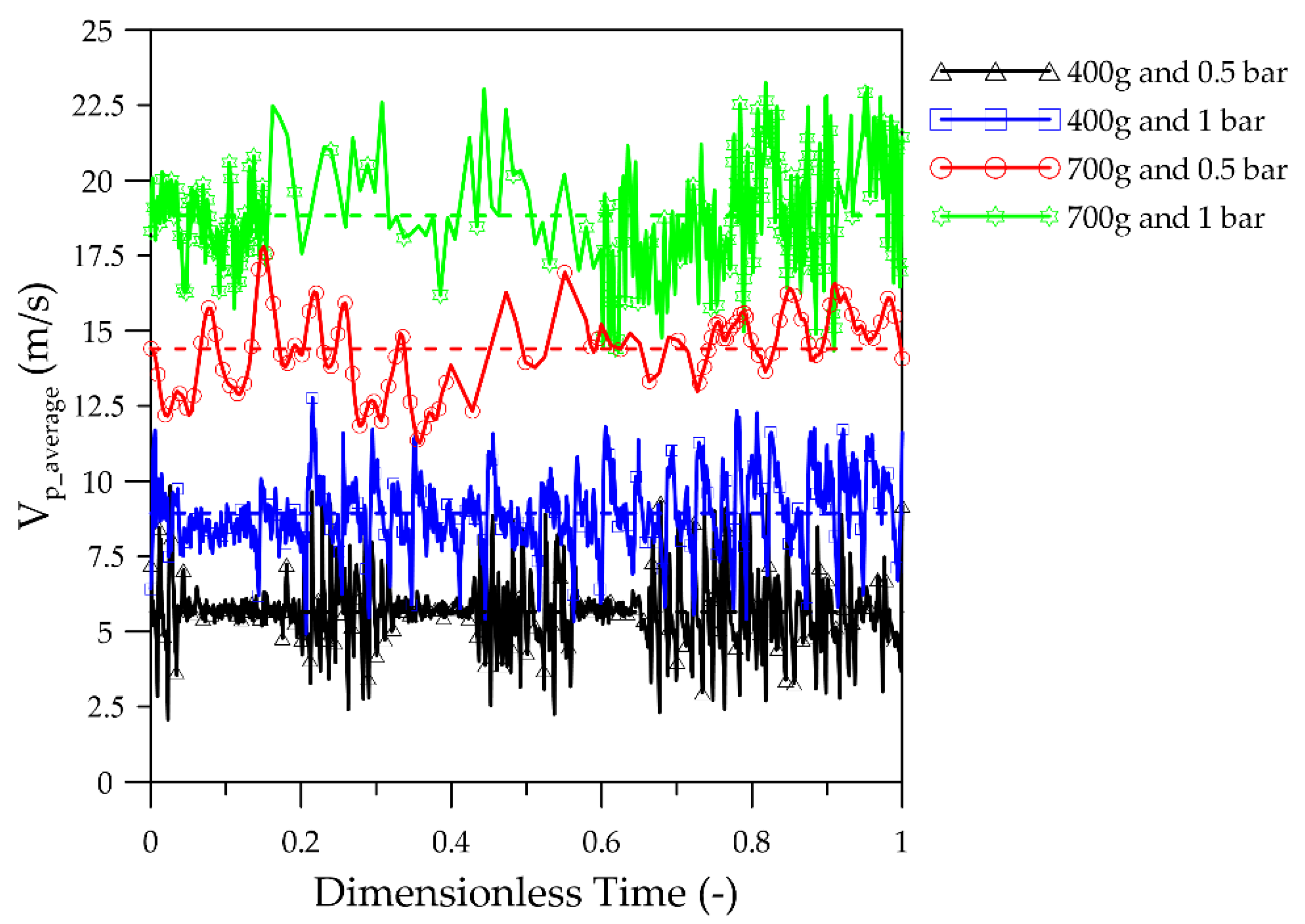
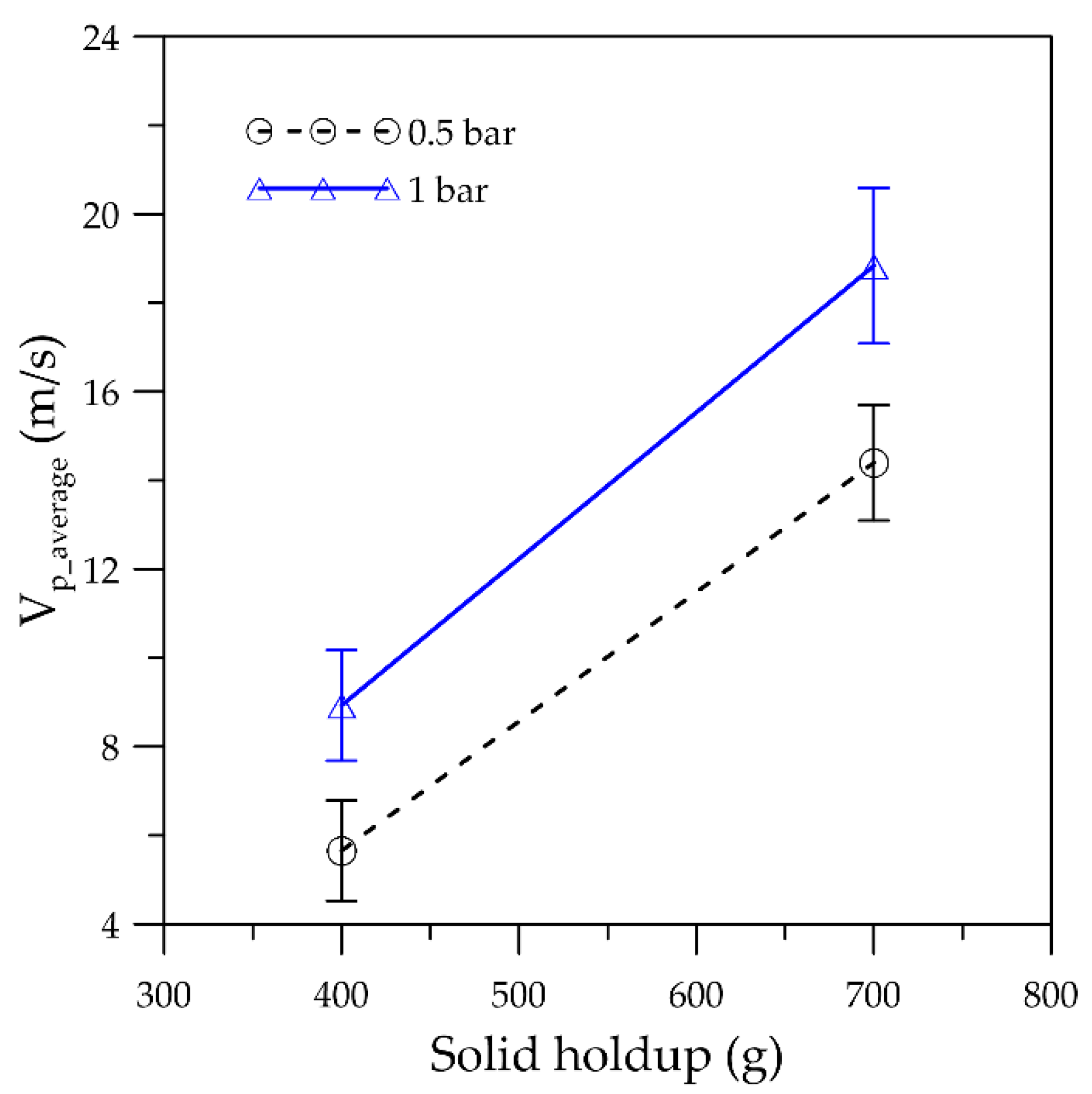
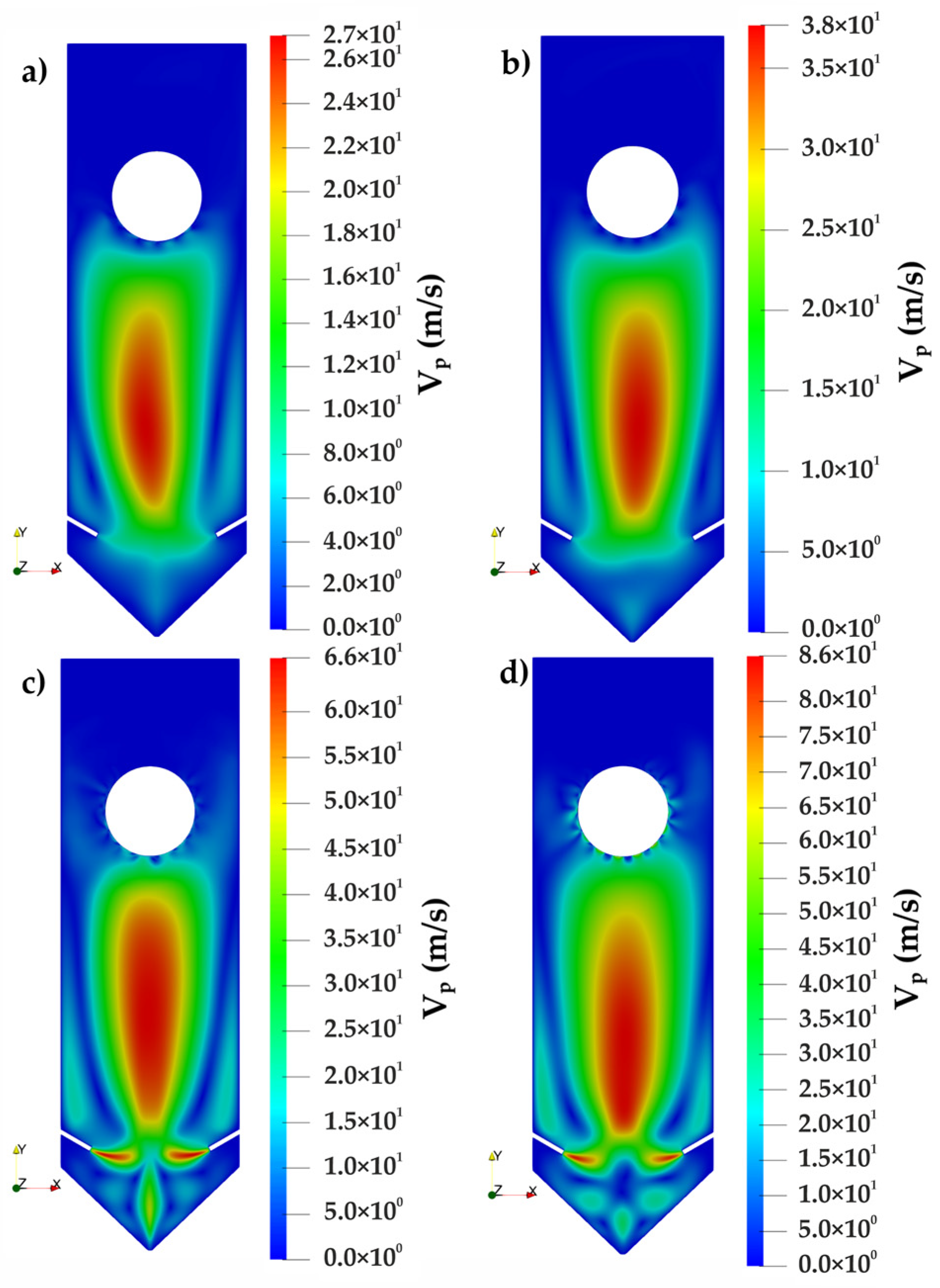
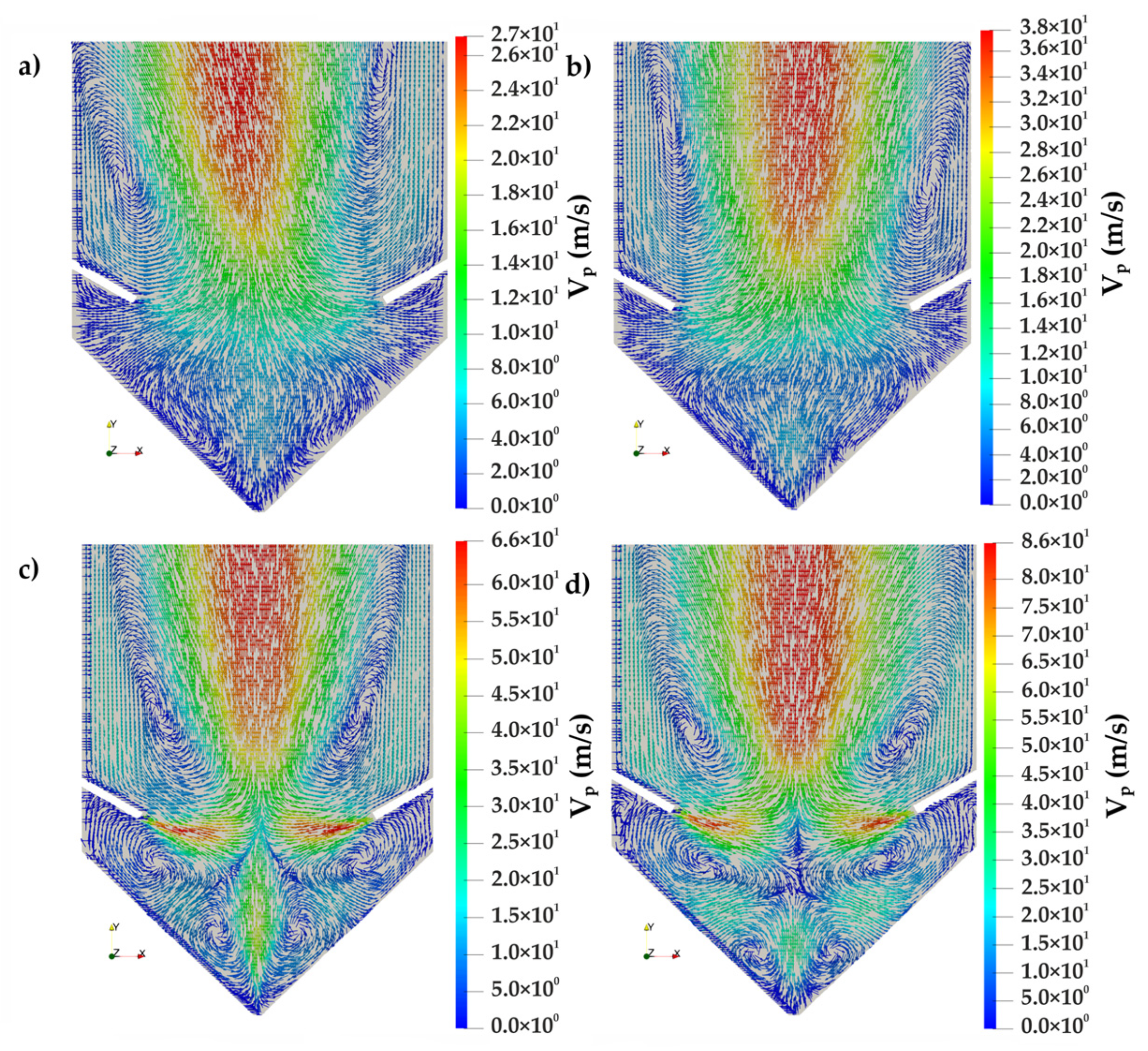
3.1. Influence of the Solid Holdup and the Nozzle Inlet Pressure on the Volumetric Average Particle Velocities
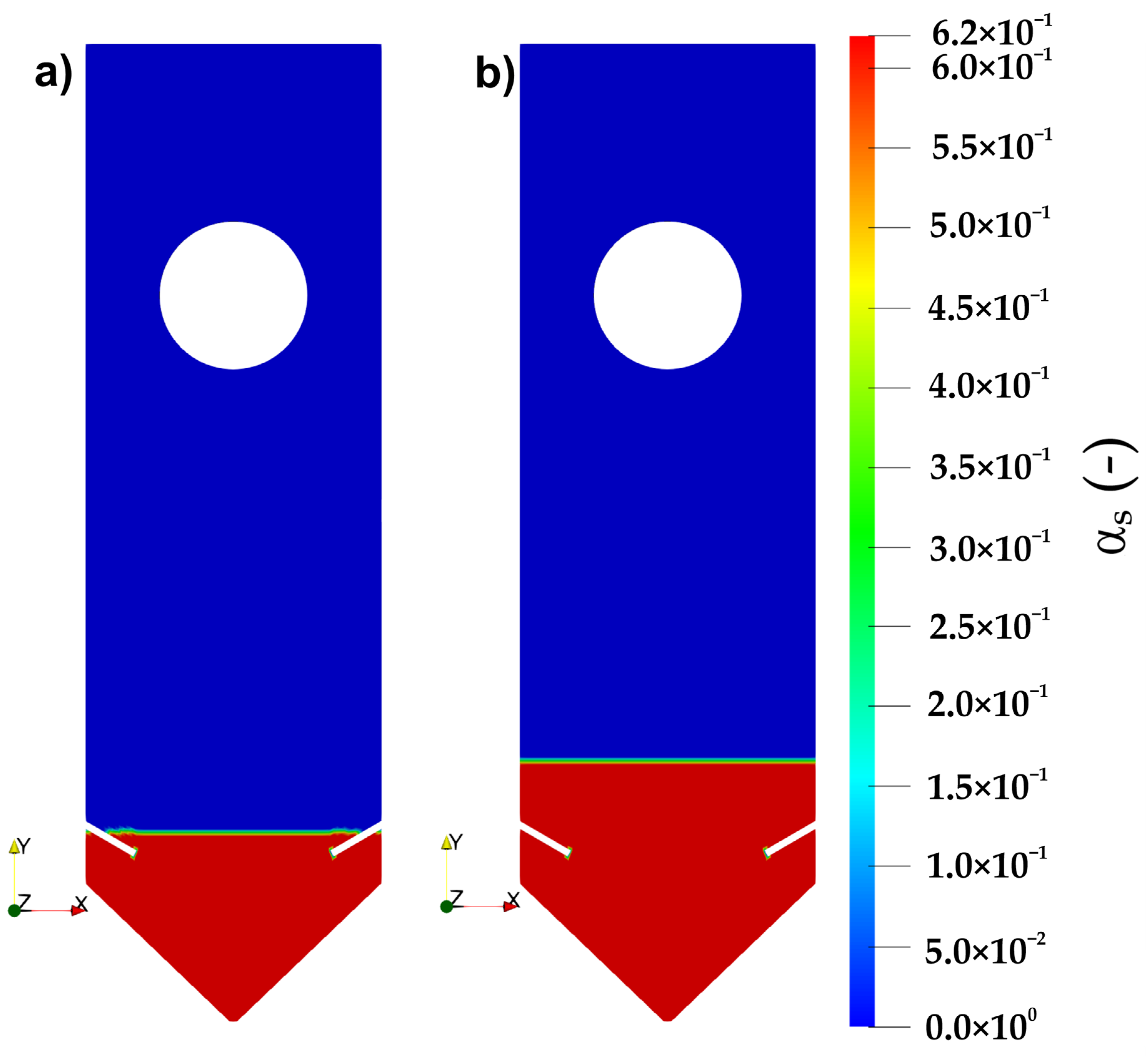
3.2. Influence of the Solid Holdup and the Nozzle Inlet Pressure on the Time-Averaged Particle Volume Fraction Distribution
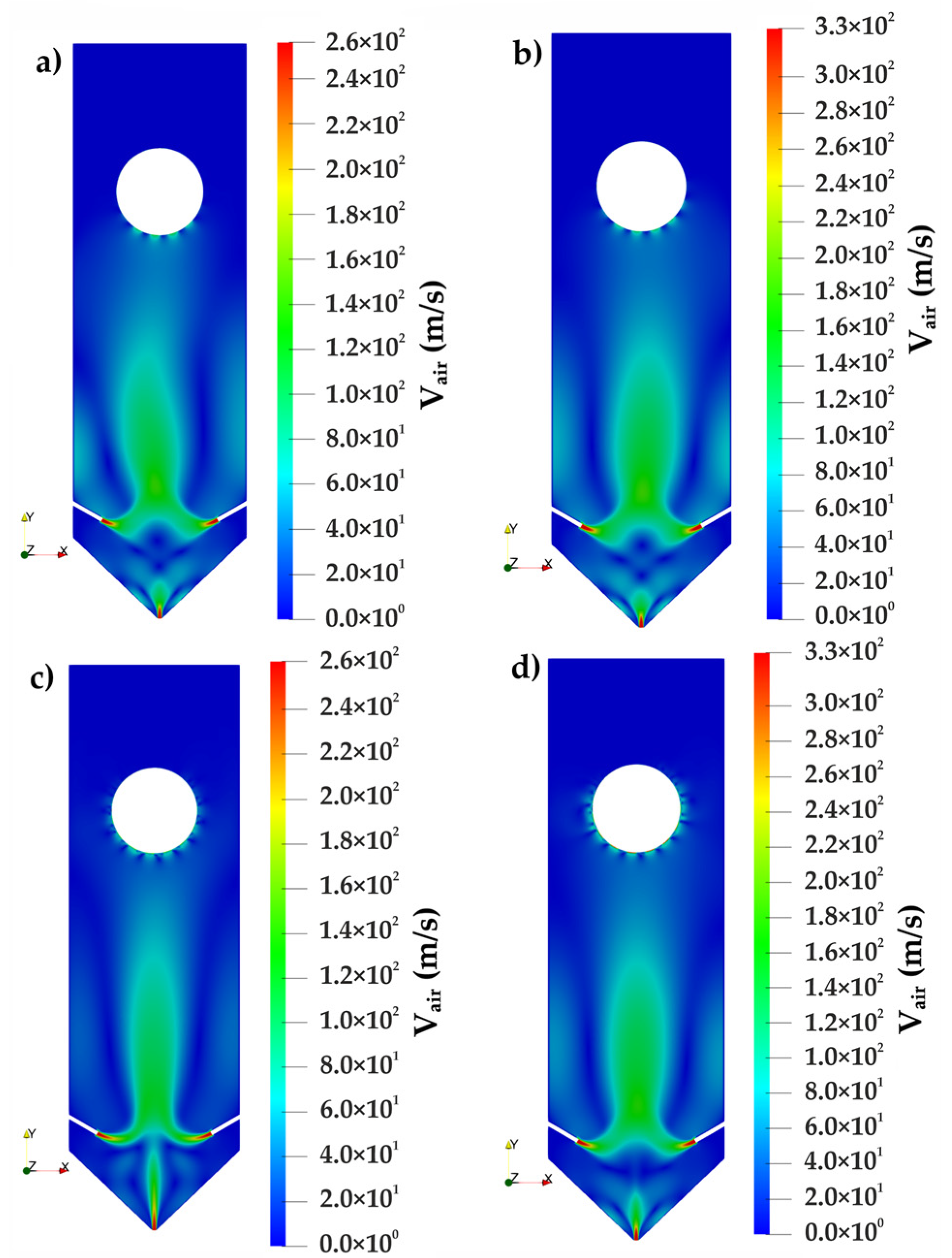
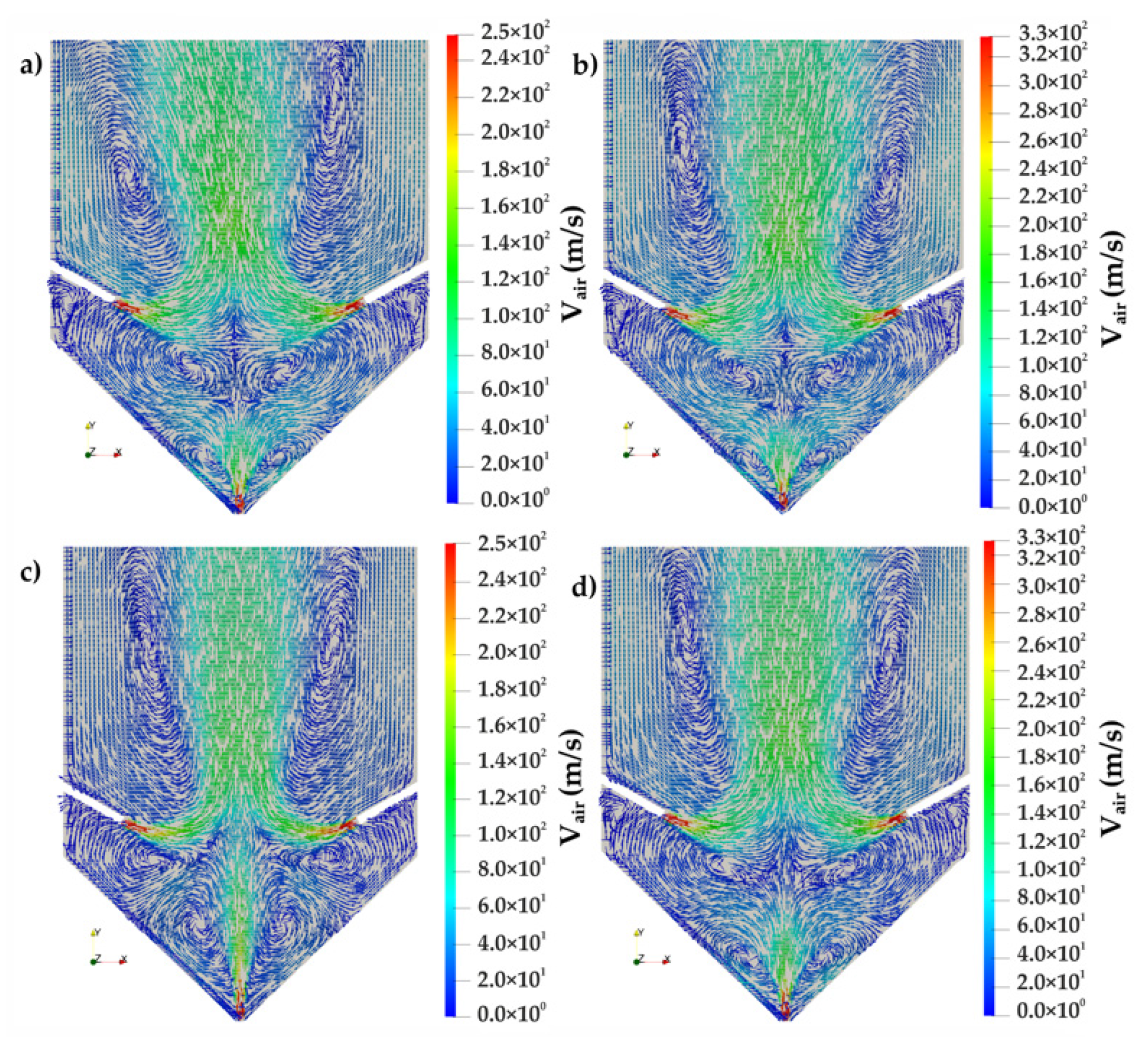
3.3. Analysis of the Particle–Fluid Dynamics Inside the Fluidized Bed Opposed Jet Mill
4. Conclusions
- Simulation results are in qualitative agreement with respect to published experimental results, not just in terms of movement and distribution but also with respect to particle collision velocity. The significant influence of the classifier on solids movement observed in operation of these mills was also observed in the model. This enables further study on solids flow around the classifier and determine process limits of classification with respect to solids over-loading and decreases process performance;
- Furthermore, asymmetric behavior of the particle and fluid flows were observed, indicating spatial and temporal periodic behavior within the apparatus. These oscillations may affect the particle residence time and stressing conditions in the grinding region and the transport of the particles towards the classifier. Onset and magnitude of these oscillations can be investigated by the proposed model, utilizing methods from system dynamics, leading to regime maps that may be linked to product properties;
- Apart from the particle–particle interactions in the grinding region, significant particle–particle interactions at the walls of the grinding chamber, the cylindrical lateral’s wall, and the classifier’s wall were observed, indicating that not only the air jets but also the equipment walls are contributing to particle breakage and comminution efficiency.
Author Contributions
Funding
Conflicts of Interest
References
- Wang, Y.; Peng, F. Parameter effects on dry fine pulverization of alumina particles in a fluidized bed opposed jet mill. Powder Technol. 2011, 214, 269–277. [Google Scholar] [CrossRef]
- Köninger, B.; Hensler, T.; Romeis, S.; Peukert, W.; Wirth, K.-E. Dynamics of fine grinding in a fluidized bed opposed jet mill. Powder Technol. 2018, 327, 346–357. [Google Scholar] [CrossRef]
- Köninger, B.; Spoetter, C.; Romeis, S.; Weber, A.P.; Wirth, K.-E. Classifier performance during dynamic fine grinding in fluidized bed opposed jet mills. Adv. Powder Technol. 2019, 30, 1678–1686. [Google Scholar] [CrossRef]
- Köninger, B.; Kögl, T.; Hensler, T.; Arlt, W.; Wirth, K.-E. Solid distribution in fluidized and fixed beds with horizontal high speed gas jets. Powder Technol. 2018, 336, 57–69. [Google Scholar] [CrossRef]
- Köninger, B.; Hensler, T.; Schug, S.; Arlt, W.; Wirth, K.-E. Horizontal secondary gas injection in fluidized beds: Solid concentration and velocity in multiphase jets. Powder Technol. 2017, 316, 49–58. [Google Scholar] [CrossRef]
- Strobel, A.; Köninger, B.; Romeis, S.; Schott, F.; Wirth, K.-E.; Peukert, W. Assessing stress conditions and impact velocities in fluidized bed opposed jet mills. Particuology 2020. [Google Scholar] [CrossRef]
- Jiang, Z.; Hagemeier, T.; Bück, A.; Tsotsas, E. Color-PTV measurement and CFD-DEM simulation of the dynamics of poly-disperse particle systems in a pseudo-2D fluidized bed. Chem. Eng. Sci. 2018, 6, 115–132. [Google Scholar] [CrossRef]
- Benedito, W.M.; Duarte, C.R.; Barrozo, M.A.S.; Santos, D.A. An investigation of CFD simulations capability in treating non-sphericalparticle dynamics in a rotary drum. Powder Technol. 2018, 332, 171–177. [Google Scholar] [CrossRef]
- Lun, C.K.K.; Savage, S.B.; Jeffrey, D.J.; Chepurniy, N. Kinetic theories for granular flow: Inelastic particles in coquette flow and singly inelastic particles in a general flow field. J. Fluid Mech. 1984, 140, 223–256. [Google Scholar] [CrossRef]
- Zhou, Z.Y.; Kuang, S.B.; Chu, K.W.; Yu, A.B. Discrete particle simulation of particle–fluid flow: Model formulations and their applicability. J. Fluid Mech. 2010, 661, 482–510. [Google Scholar] [CrossRef]
- Lin, W.; Guoli, Q.; Ming, T.; Xuemin, L.; Hassan, M.; Huilin, L. Simulated pulsed flow of gas and particles in a horizontal oppose-pulsed gas jets of bubbling fluidized bed. Adv. Powder Technol. 2018, 29, 3507–3519. [Google Scholar] [CrossRef]
- Santos, D.A.; Alves, G.C.; Duarte, C.R.; Barrozo, M.A.S. Disturbances in the Hydrodynamic Behavior of a Spouted Bed Caused by an Optical Fiber Probe: Experimental and CFD Study. Ind. Eng. Chem. Res. 2012, 51, 3801–3810. [Google Scholar] [CrossRef]
- Shi, P.; Rzehak, R. Solid-liquid flow in stirred tanks: Euler-Euler/RANS modeling. Chem. Eng. Sci. 2020, 227, 1–23. [Google Scholar] [CrossRef]
- Rajeswari, M.S.R.; Azizli, K.A.M.; Hashim, S.F.S.; Abdullah, M.K.; Mujeebu, M.A.; Abdullah, M.Z. CFD simulation and experimental analysis of flow dynamics and grinding performance of opposed fluidized bed air jet mill. Int. J. Miner. Process. 2011, 98, 94–105. [Google Scholar] [CrossRef]
- Lee, H.W.; Song, S.; Kim, H.T. Improvement of pulverization efficiency for micro-sized particles grinding by uncooled high-temperature air jet mill using a computational simulation. Chem. Eng. Sci. 2019, 207, 1140–1147. [Google Scholar] [CrossRef]
- Teng, S.; Wang, P.; Zhu, L.; Young, M.-W.; Gogos, C.G. Experimental and numerical analysis of a lab-scale fluid energy mill. Powder Technol. 2009, 195, 31–39. [Google Scholar] [CrossRef]
- Teng, S.; Wang, P.; Zhang, Q.; Gogos, C. Analysis of Fluid Energy Mill by gas-solid two-phase flow simulation. Powder Technol. 2011, 208, 684–693. [Google Scholar] [CrossRef]
- Brosh, T.; Kalman, H.; Levy, A.; Peyron, I. Francois R. DEM-CFD simulation of particle comminution in jet-mill. Powder Technol. 2014, 257, 104–112. [Google Scholar] [CrossRef]
- Rodnianski, V.; Levy, A.; Kalman, H. A new method for simulation of comminution process in jet mills. Powder Technol. 2019, 343, 867–879. [Google Scholar] [CrossRef]
- Bnà, S.; Ponzini, R.; Cestari, M.; Cavazzoni, C.; Cottini, C.; Benassi, A. Investigation of particle dynamics and classification mechanism in a spiral jet mill through computational fluid dynamics and discrete element methods. Powder Technol. 2020, 364, 746–773. [Google Scholar] [CrossRef]
- Schaeffer, G. Instability in the evolution equations describing incompressible granular flow. J. Differ. Equ. 1987, 66, 19–50. [Google Scholar] [CrossRef] [Green Version]
- Johnson, P.C.; Jackson, R. Frictional-collisional constitutive relations for granular materials with application to plane shearing. J. Fluid Mech. 1987, 176, 67–93. [Google Scholar] [CrossRef]
- Gidaspow, D. Multiphase Flow and Fluidization. Continuum and Kinetic Theory Descriptions; Elsevier Science: San Diego, CA, USA, 1994; ISBN 978-0-08-051226-6. [Google Scholar] [CrossRef]
- Cokljat, D.; Ivanov, V.A.; Sarasola, F.J.; Vasquez, S.A. Multiphase k-epsilon Models for Unstructured Meshes. In ASME 2000 Fluids Engineering Division Summer Meeting; ASME: Boston, MA, USA, 2000; pp. 749–754. ISBN 0791819795. [Google Scholar]
- Elghobashi, S.E.; Abou-Arab, T.W. A two-equation turbulence model for two-phase flows. Phys. Fluids 1983, 26, 931–938. [Google Scholar] [CrossRef]
- Launder, B.E.; Spalding, D.B. The numerical computation of turbulent flows. Comput. Methods Appl. Mech. Eng. 1974, 3, 269–289. [Google Scholar] [CrossRef]
- Ranz, W.E.; Marshall, W.R. Evaporation from drops. Chem. Eng. Prog. 1952, 48, 141–146. [Google Scholar]
- Wen, C.Y.; Yu, Y.H. Mechanics of fluidization. Chem. Eng. Prog. 1966, 62, 100–111. [Google Scholar]
- Ergun, S. Fluid flow through packed columns. Chem. Eng. Prog. 1952, 48, 89–94. [Google Scholar]
- Batista, J.N.M.; Santos, D.A.; Béttega, R. Determination of the physical and interaction properties of sorghum grains: Application to computational fluid dynamics–discrete element method simulation of the fluid dynamics of a conical spouted bed. Particuology 2020. [Google Scholar] [CrossRef]
- Kobayashi, T.; Tanaka, T.; Shimada, N.; Kawaguchi, T. DEM-CFD analysis of fluidization behavior of Geldart Group A particles using a dynamic adhesion force model. Powder Technol. 2013, 248, 143–152. [Google Scholar] [CrossRef]
- Upadhyay, M.; Kim, A.; Kim, H.; Lim, D.; Lim, H. An Assessment of Drag Models in Eulerian-Eulerian CFD Simulation of Gas-Solid Flow Hydrodynamics in Circulating Fluidized Bed Riser. ChemEngineering 2020, 4, 37. [Google Scholar] [CrossRef]
- Barton, I.E. Comparison of SIMPLE- and PISO-type algorithms for transient flows. Int. J. Numer. Methods Fluids 1998, 26, 459–483. [Google Scholar] [CrossRef]
- Goldhirsch, I. Introduction to granular temperature. Powder Technol. 2008, 182, 130–136. [Google Scholar] [CrossRef]




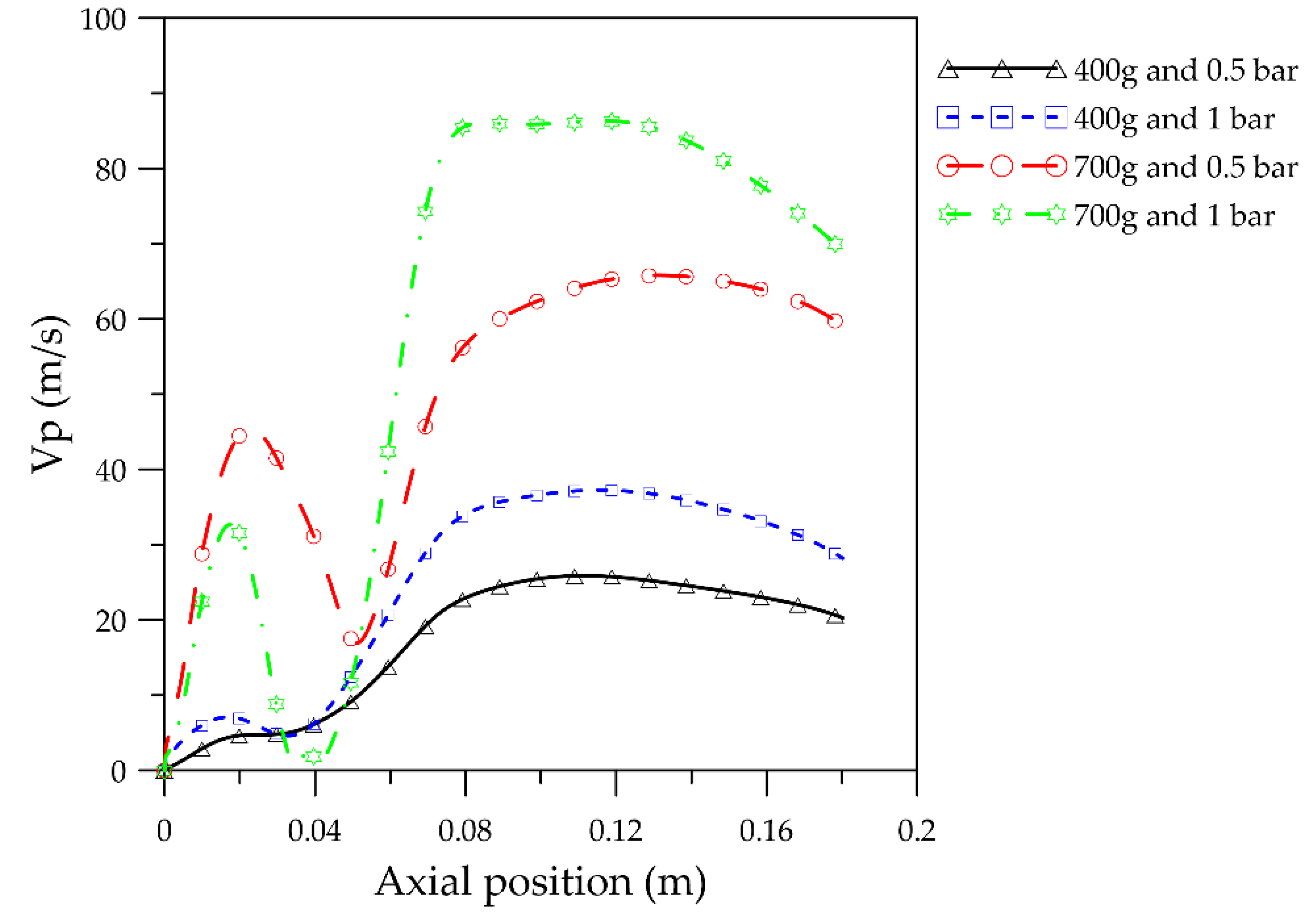
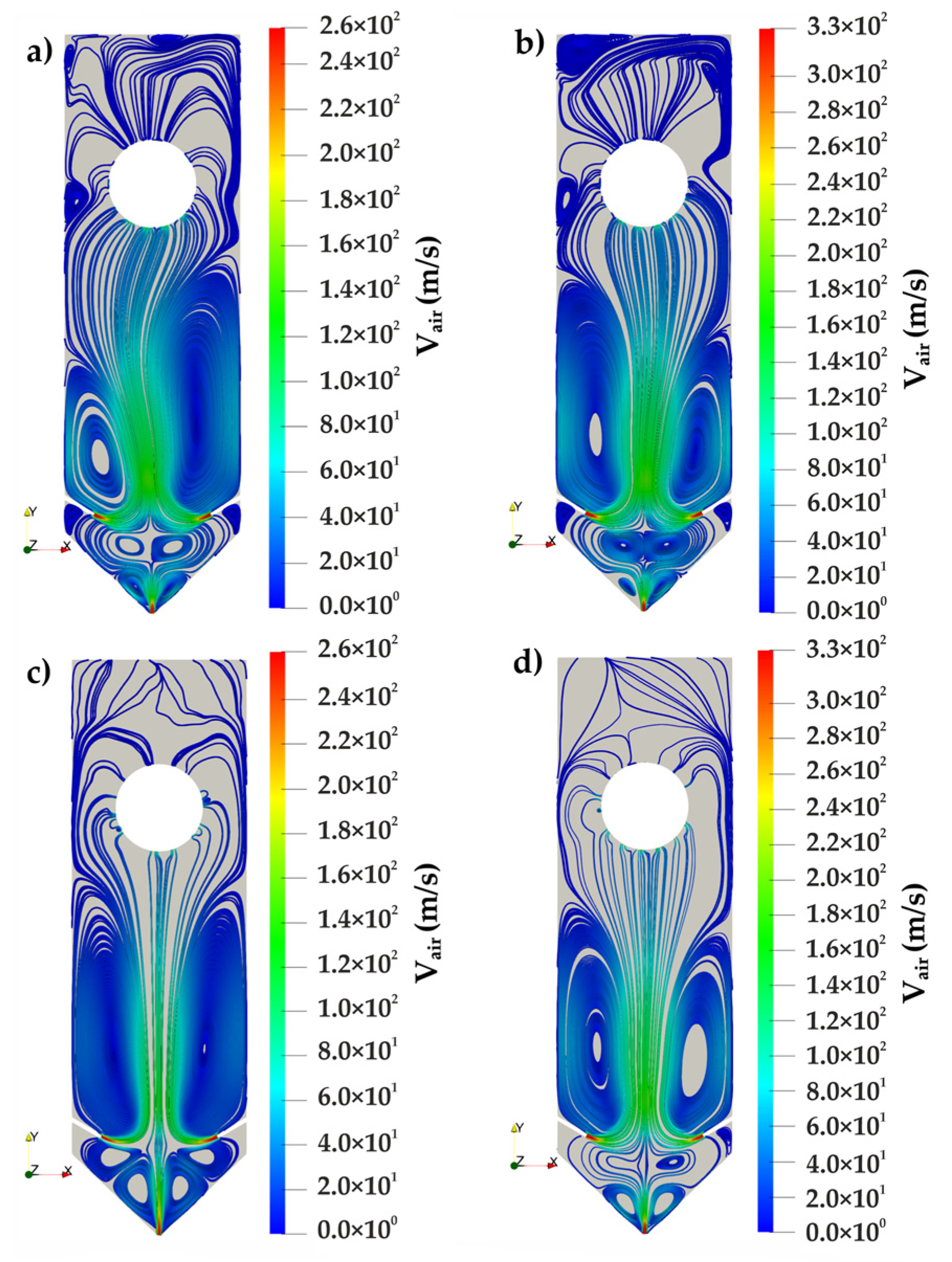
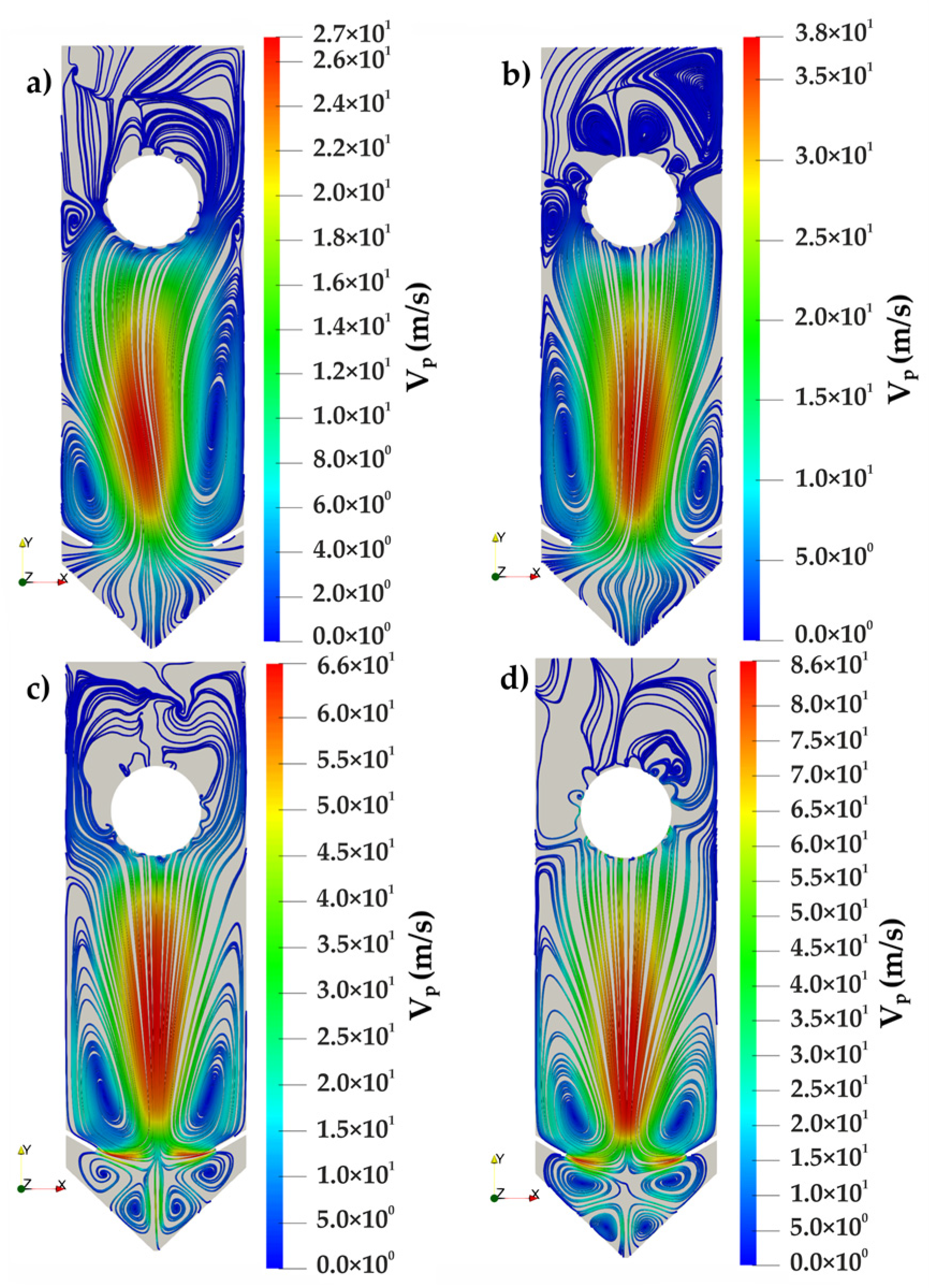

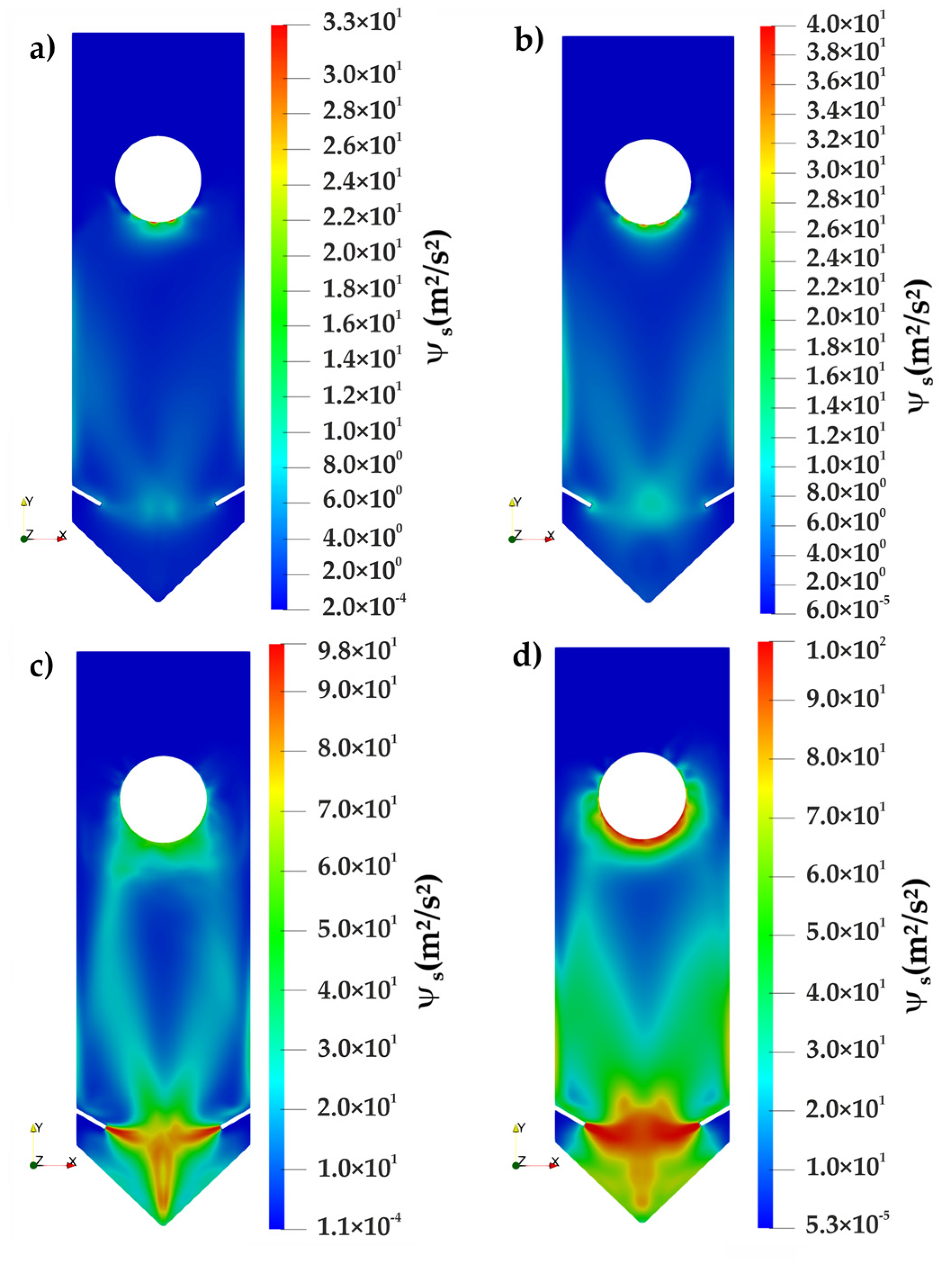
| Parameter | Value | Parameter | Value | Parameter | Value |
|---|---|---|---|---|---|
| L1 | 0.1985 m | L4 | 0.0654 m | D2 | 0.0500 m |
| L2 | 0.0465 m | L5 | 0.0850 m | θ | 30° |
| L3 | 0.0662 m | D1 | 0.1000 m | Dnozzle (exit) | 2.4 mm |
| Constitutive Equations: | |
|---|---|
| Viscous stress tensor of the fluid phase: | (5) |
| Viscous stress tensor of the solid phase: | (6) |
| Total granular viscosity: | (7) |
| Kinetic () and collisional () granular viscosity: | (8) |
| (9) | |
| Frictional viscosity (Schaeffer’s model [21]): | (10) |
| Total granular pressure: | (11) |
| Kinetic () and collisional () granular pressure: | (12) |
| Frictional pressure (Johnson and Jackson’s model [22]): | (13) |
| Radial distribution function: | (14) |
| Solid bulk viscosity: | (15) |
| Conductivity of granular temperature: | (16) |
| Kinetic energy dissipation due to inelastic collisions: | (17) |
| Kinetic energy dissipation due to fluid friction: | (18) |
| Solid–gas momentum exchange coefficient (Gidaspow’s model [23]): | |
| (19) | |
| (20) | |
| (21) |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Araújo dos Santos, D.; Baluni, S.; Bück, A. Eulerian Multiphase Simulation of the Particle Dynamics in a Fluidized Bed Opposed Gas Jet Mill. Processes 2020, 8, 1621. https://doi.org/10.3390/pr8121621
Araújo dos Santos D, Baluni S, Bück A. Eulerian Multiphase Simulation of the Particle Dynamics in a Fluidized Bed Opposed Gas Jet Mill. Processes. 2020; 8(12):1621. https://doi.org/10.3390/pr8121621
Chicago/Turabian StyleAraújo dos Santos, Dyrney, Shivam Baluni, and Andreas Bück. 2020. "Eulerian Multiphase Simulation of the Particle Dynamics in a Fluidized Bed Opposed Gas Jet Mill" Processes 8, no. 12: 1621. https://doi.org/10.3390/pr8121621
APA StyleAraújo dos Santos, D., Baluni, S., & Bück, A. (2020). Eulerian Multiphase Simulation of the Particle Dynamics in a Fluidized Bed Opposed Gas Jet Mill. Processes, 8(12), 1621. https://doi.org/10.3390/pr8121621







