Closed-Cell Powder Metallurgical Aluminium Foams Reinforced with 3 vol.% SiC and 3 vol.% Graphite
Abstract
:1. Introduction
2. Materials and Methods
3. Results and Discussion
3.1. Composite Foams Microstructure
3.2. Damping and Modulus of Elasticity
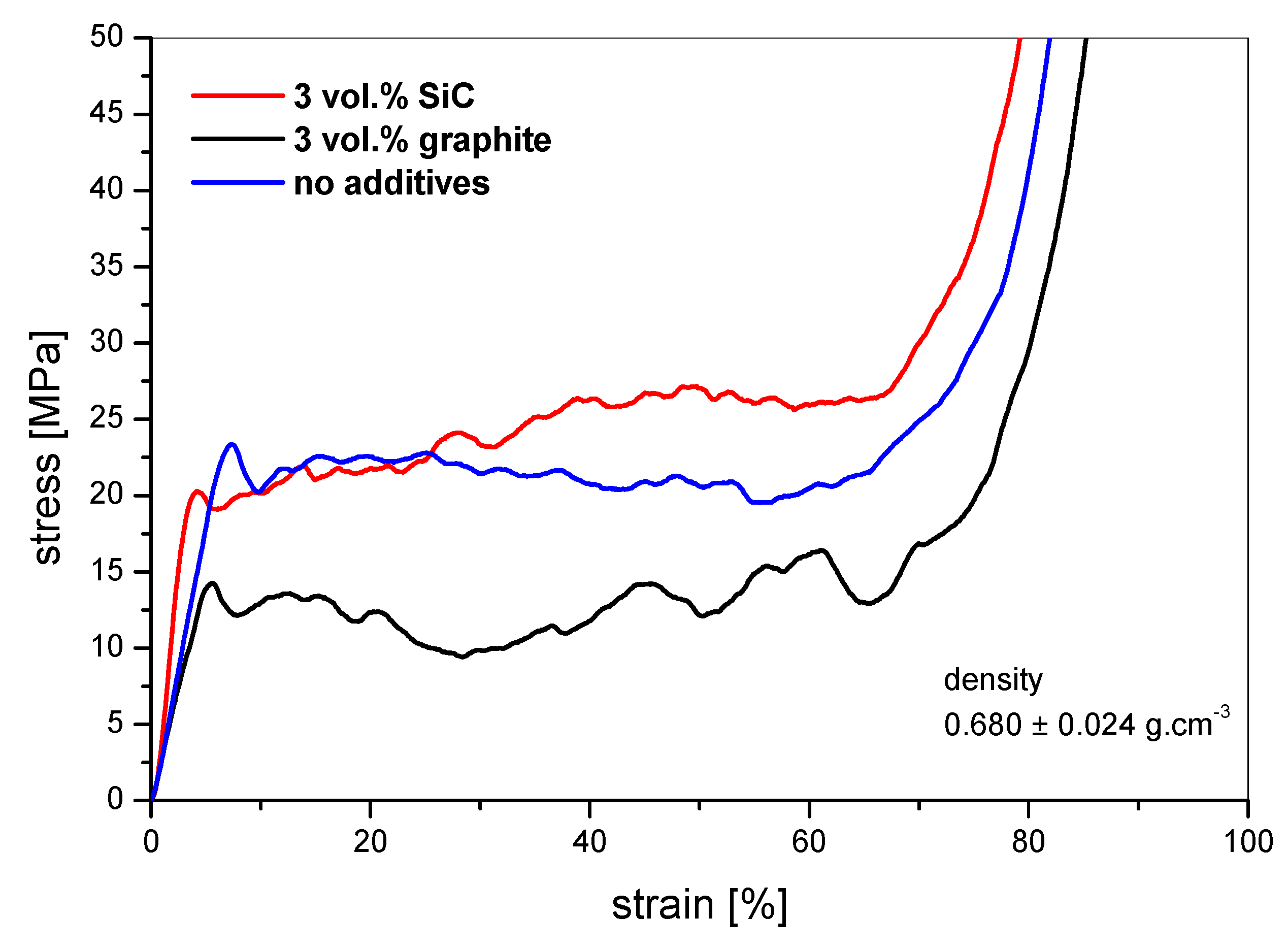
3.3. Compression Properties
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Simančík, F.; Rajner, W.; Laag, R. Alulight—Aluminum foam for lightweight construction. SAE Tech. Pap. 2000. 2000-01-0337. [Google Scholar] [CrossRef]
- Linul, E.; Pietras, D.; Sadowski, T.; Marşavina, L.; Rajak, D.P.; Kováčik, J. Crashworthiness performance of lightweight Composite Metallic Foams at high temperatures. Compos. Part A 2021, 149, 106516. [Google Scholar] [CrossRef]
- Movahedi, N.; Linul, E. Radial crushing response of ex-situ foam-filled tubes at elevated temperatures. Compos. Struct. 2021, 277, 114634. [Google Scholar] [CrossRef]
- Khezrzadeh, O.; Mirzaee, O.; Emadoddin, E.; Linul, E. Anisotropic Compressive Behavior of Metallic Foams under Extreme Temperature Conditions. Materials 2020, 13, 2329. [Google Scholar] [CrossRef]
- Das, S.; Rajak, D.K.; Khanna, S.; Mondal, D.P. Energy Absorption Behavior of Al-SiC-Graphene Composite Foam under a High Strain Rate. Materials 2020, 13, 783. [Google Scholar] [CrossRef] [Green Version]
- Yu, Y.; Cao, Z.; Wang, J.; Tu, G.; Mu, Y. Compressive Behavior of Al-TiB2 Composite Foams Fabricated under Increased Pressure. Materials 2021, 14, 5112. [Google Scholar] [CrossRef] [PubMed]
- Liu, H.; Pan, W.; Si, F.; Huang, K.; Liu, Y.; Liu, J. Enhanced Compressive Property of Al Composite Foams at Elevated Temperatures via Plasma Electrolytic Oxidation. Metals 2018, 8, 118. [Google Scholar] [CrossRef] [Green Version]
- Epasto, G.; Distefano, F.; Mozafari, H.; Linul, E.; Crupi, V. Nondestructive Evaluation of Aluminium Foam Panels Subjected to Impact Loading. Appl. Sci. 2021, 11, 1148. [Google Scholar] [CrossRef]
- Linul, E.; Khezrzadeh, O. Axial crashworthiness performance of foam-based composite structures under extreme temperature conditions. Compos. Struct. 2021, 271, 114156. [Google Scholar] [CrossRef]
- Kaczyński, P.; Ptak, M.; Gawdzińska, K. Energy absorption of cast metal and composite foams tested in extremely low and high-temperatures. Mater. Des. 2020, 196, 109114. [Google Scholar] [CrossRef]
- Jiejun, W.; Chenggong, L.; Dianbin, W.; Manchang, G. Damping and sound absorption properties of particle reinforced Al matrix composite foams. Compos. Sci. Technol. 2003, 63, 569–574. [Google Scholar] [CrossRef]
- Mepura. Data Sheets; Mepura GmbH.: Ranshofen, Austria, 1995. [Google Scholar]
- Kováčik, J.; Tobolka, P.; Simančík, F. Noise attenuation using aluminium foams. In Metal. Foams and Porous Metal. Structures; Banhart, J., Ashby, M.F., Fleck, N.A., Eds.; Verlag MIT Publishing: Bremen, Germany, 1999; pp. 405–408. [Google Scholar]
- Degischer, H.P.; Kriszt, B. Handbook of Cellular Metals, Production, Processing, Applications, 1st ed.; Degischer, H.P., Kriszt, B., Eds.; Wiley-VCH: Weinheim, Germany, 2002; pp. 215–241. [Google Scholar]
- Parveez, B.; Jamal, N.A.; Maleque, A.; Yusof, F.; Jamadon, N.H.; Adzila, S. Review on advances in porous Al composites and the possible way forward. J. Mater. Res. Technol. 2021, 14, 2017–2038. [Google Scholar] [CrossRef]
- Matli, P.R.; Sheng, J.G.Y.; Parande, G.; Manakari, V.; Chua, B.W.; Wong, S.C.K.; Gupta, M. A Novel Method of Light Weighting Aluminium Using Magnesium Syntactic Composite Core. Crystals 2020, 10, 917. [Google Scholar] [CrossRef]
- Campana, G.; Ascari, A.; Fortunato, A. Laser foaming for joining aluminum foam cores inside a hollow profile. Opt. Laser Technol. 2013, 48, 331–336. [Google Scholar] [CrossRef]
- Ghiani, C.; Linul, E.; Porcu, M.C.; Marsavina, L.; Movahedi, N.; Aymerich, F. Metal Foam-Filled Tubes as Plastic Dissipaters in Earthquake-Resistant Steel Buildings. 2018 IOP Conf. Ser. Mater. Sci. Eng. 2018, 416, 012051. [Google Scholar] [CrossRef]
- Rajak, D.K.; Linul, E. Crushing response of Composite Metallic Foams: Density and High Strain Rate effects. J. Alloy. Compd. 2021, 871, 159614. [Google Scholar] [CrossRef]
- Bauer, B.; Kralj, S.; Busic, M. Production and application of metal foams in casting technology. Teh. Vjesn. 2013, 20, 1095–1102. [Google Scholar]
- Ubertalli, G.; Ferraris, S. Al-Based Metal Foams (AMF) as Permanent Cores in Casting: State-of-the-Art and Future Perspectives. Metals 2020, 10, 1592. [Google Scholar] [CrossRef]
- Nammi, S.K.; Edwards, G.; Shirvani, H. Effect of cell-size on the energy absorption features of closed-cell aluminium foams. Acta Astronaut. 2016, 128, 243–250. [Google Scholar] [CrossRef]
- Maiorano, L.P.; Molina, J.M. Guiding heat in active thermal management: One-pot incorporation of interfacial nano-engineered aluminium/diamond composites into aluminium foams. Compos. Part A 2020, 133, 105859. [Google Scholar] [CrossRef]
- Sunar, T.; Cetin, M. Manufacturing of B4C particle reinforced A360 aluminium cellular composite materials by the integration of stir casting and space holder methods. J. Compos. Mater. 2021, 55, 3763–3773. [Google Scholar] [CrossRef]
- Gopinathan, A.; Jerz, J.; Kováčik, J.; Dvorák, T. Investigation of the Relationship between Morphology and Thermal Conductivity of Powder Metallurgically Prepared Aluminium Foams. Materials 2021, 14, 3623. [Google Scholar] [CrossRef]
- Bruel & Kjaer. Mechanical Vibration and Shock Measurements, 2nd ed.; K. Larsen & Son: Soborg, Denmark, 1980. [Google Scholar]
- Kaptay, G.; Bader, E.; Bolyan, L. Interfacial Forces and Energies Relevant to Production of Metal Matrix Composites. Mater. Sci. Forum 2000, 329–330, 151–156. [Google Scholar] [CrossRef]
- Hoch, M.; Johnston, H.L. Formation, Stability and Crystal Structure of the Solid Aluminum Suboxides: Al2O and AlO1. J. Am. Chem. Soc. 1954, 76, 2560–2561. [Google Scholar] [CrossRef]
- Gibson, L.; Ashby, M. Cellular Solids: Structure and Properties, 2nd ed.; Cambridge University Press: Cambridge, UK, 1997. [Google Scholar] [CrossRef]
- Deshpande, V.S.; Ashby, M.F.; Fleck, N.A. Foam topology: Bending versus stretching dominated architectures. Acta Mater. 2001, 49, 1035–1040. [Google Scholar] [CrossRef]
- Wang, A.J.; McDowell, D.L. In-Plane Stiffness and Yield Strength of Periodic Metal Honeycombs. J. Eng. Mater. Technol. 2004, 126, 137–156. [Google Scholar] [CrossRef]
- Benedetti, M.; Klarin, J.; Johansson, F.; Fontanari, V.; Luchin, V.; Zappini, G.; Molinari, A. Study of the Compression Behaviour of Ti6Al4V Trabecular Structures Produced by Additive Laser Manufacturing. Materials 2019, 12, 1471. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Simančík, F. Reproducibility of aluminium foam properties. In Metal. Foams and Porous Metal. Structures; Banhart, J., Ashby, M.F., Fleck, N.A., Eds.; Verlag MIT Publishing: Bremen, Germany, 1999; p. 235. [Google Scholar]
- Uzun, A. Production of aluminium foams reinforced with silicon carbide and carbon nanotubes prepared by powder metallurgy method. Compos. Part B 2019, 172, 206–217. [Google Scholar] [CrossRef]
- Li, W.T.; Yang, X.D.; He, C.N.; Sha, J.W.; Shi, C.S.; Li, J.J.; Zhao, N.Q. Compressive responses and strengthening mechanisms of aluminum composite foams reinforced with graphene nanosheets. Carbon 2019, 153, 396–406. [Google Scholar] [CrossRef]
- Kováčik, J.; Jerz, J.; Mináriková, N.; Marsavina, L.; Linul, E. Scaling of compression strength in disordered solids: Metallic foam. Frat. Integrità Strutt. 2016, 10, 55–62. [Google Scholar] [CrossRef] [Green Version]
- Krizik, P.; Balog, M.; Matko, I.; Svec, P.; Cavojsky, M.; Simancik, F. The effect of a particle–matrix interface on the Young’s modulus of Al–SiC composites. J. Compos. Mater. 2016, 50, 99–108. [Google Scholar] [CrossRef]
- Mazloum, A.; Kováčik, J.; Emmer, Š.; Sevostianov, I. Copper–graphite composites: Thermal expansion, thermal and electrical conductivities, and cross-property connections. J. Mater. Sci. 2016, 51, 7977–7990. [Google Scholar] [CrossRef]
- Guden, M.; Yüksel, S. SiC-particulate aluminum composite foams produced from powder compacts: Foaming and compression behavior. J. Mater. Sci. 2006, 41, 4075–4084. [Google Scholar] [CrossRef] [Green Version]
- Aldoshan, A.; Khanna, S. Effect of relative density on the dynamic compressive behavior of carbon nanotube reinforced aluminum foam. Mater. Sci. Eng. A 2017, 689, 17–24. [Google Scholar] [CrossRef]
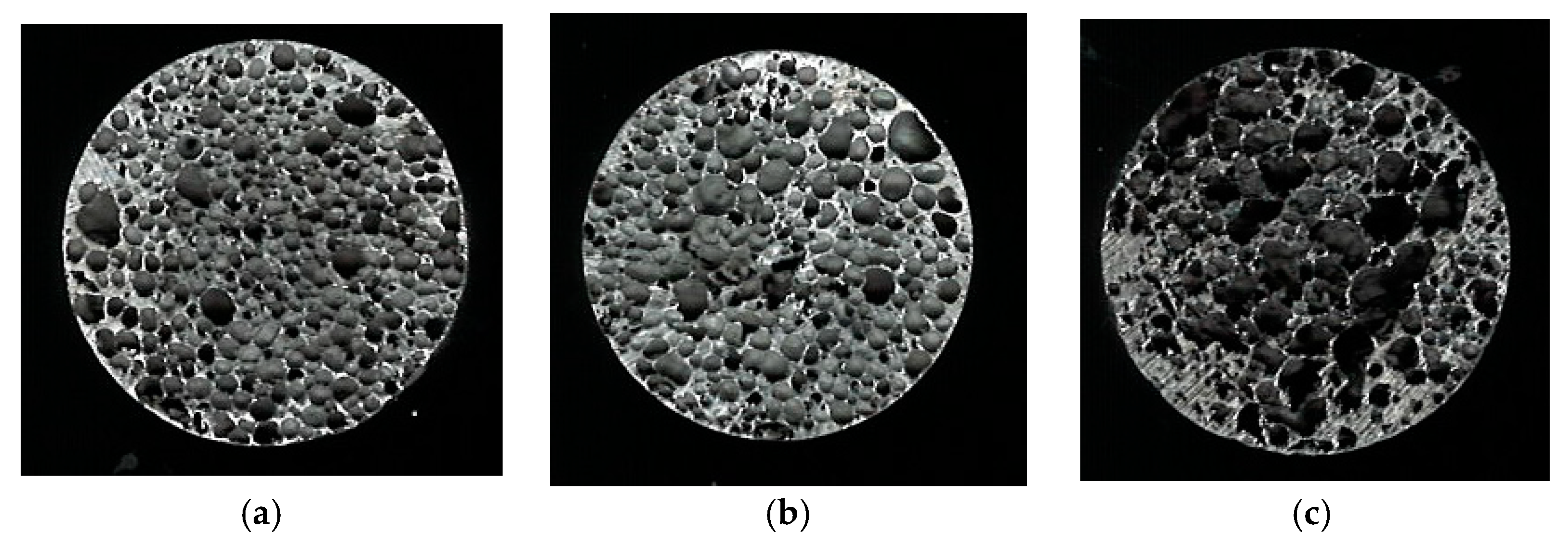
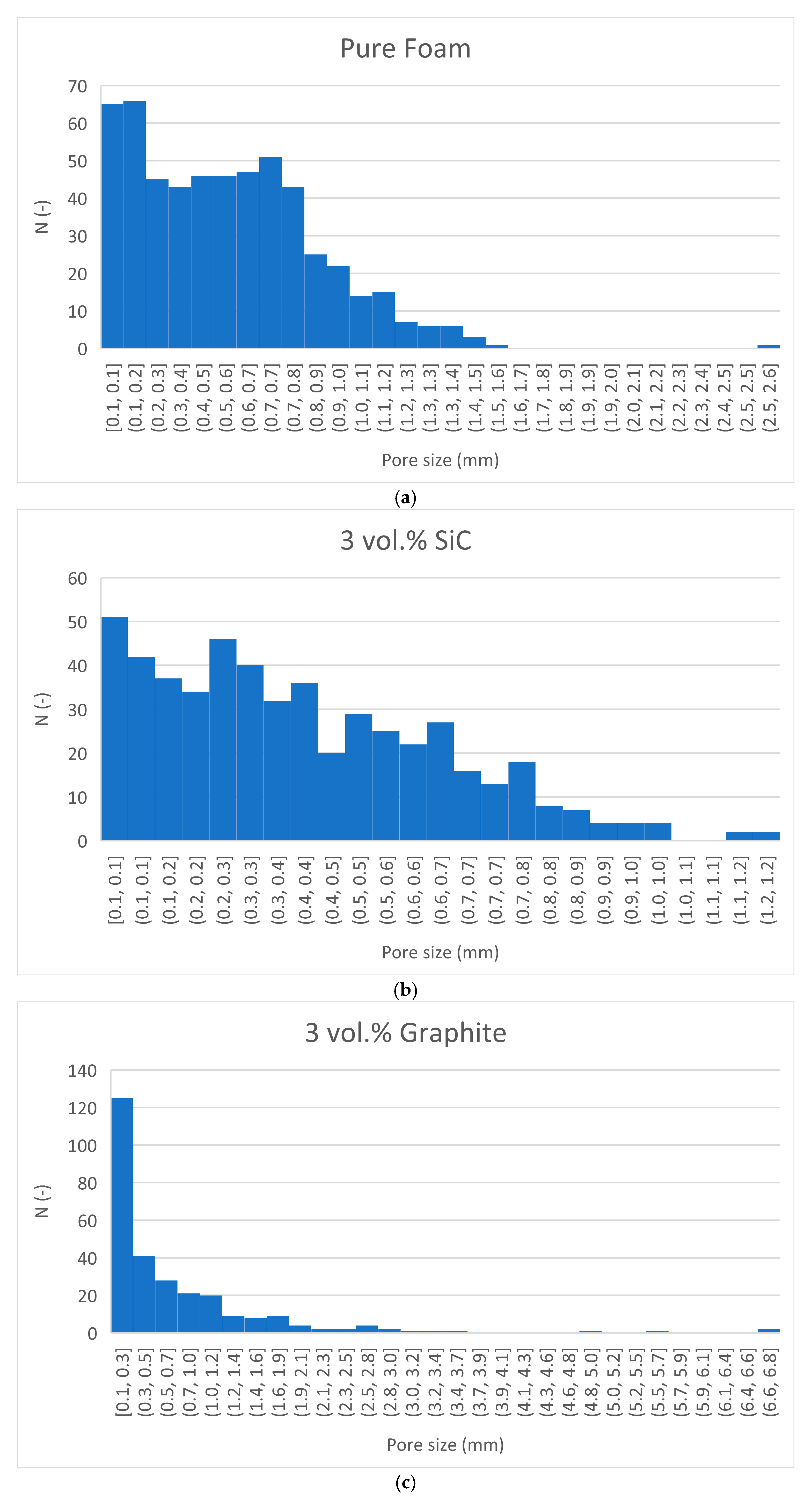
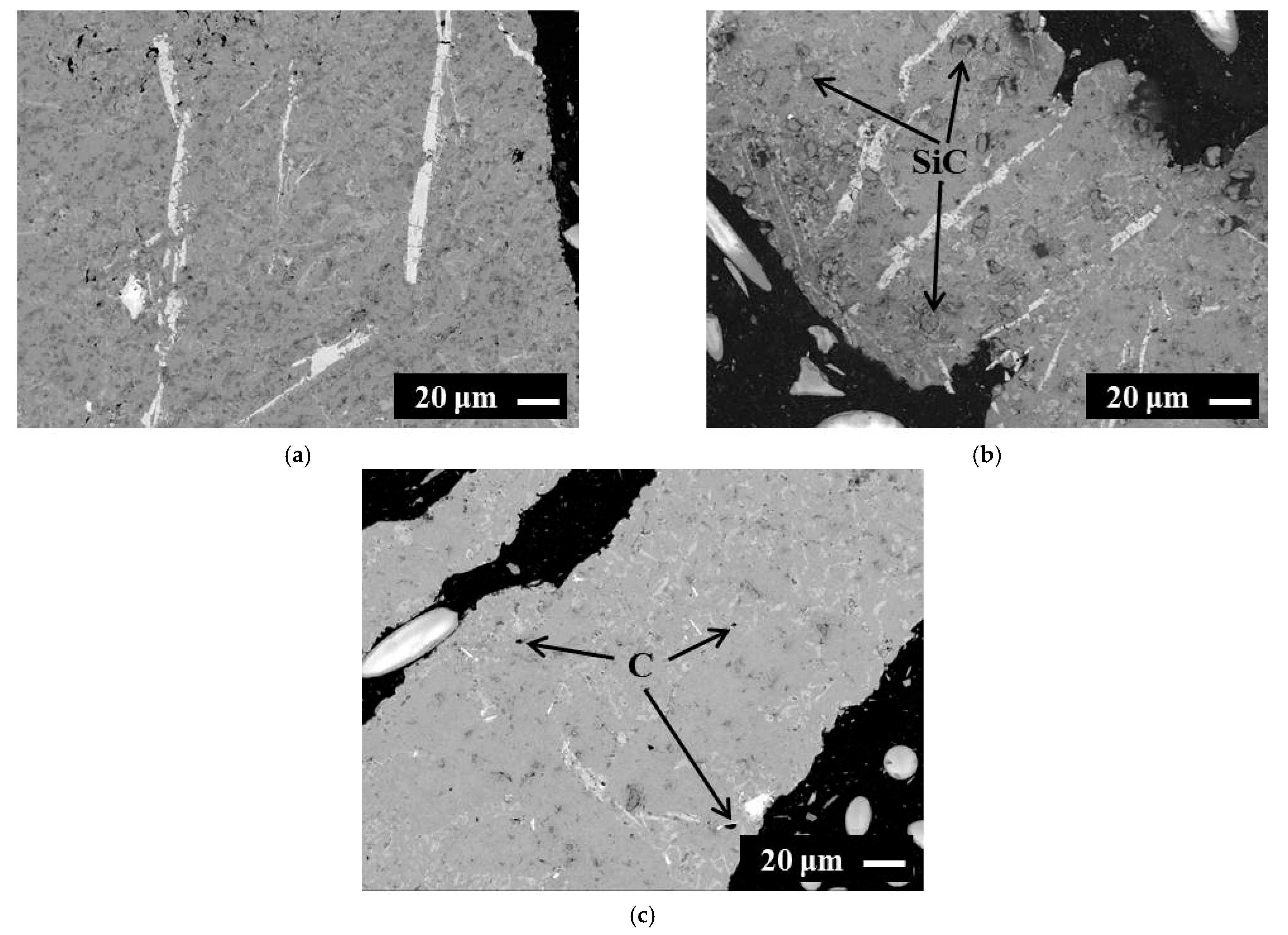

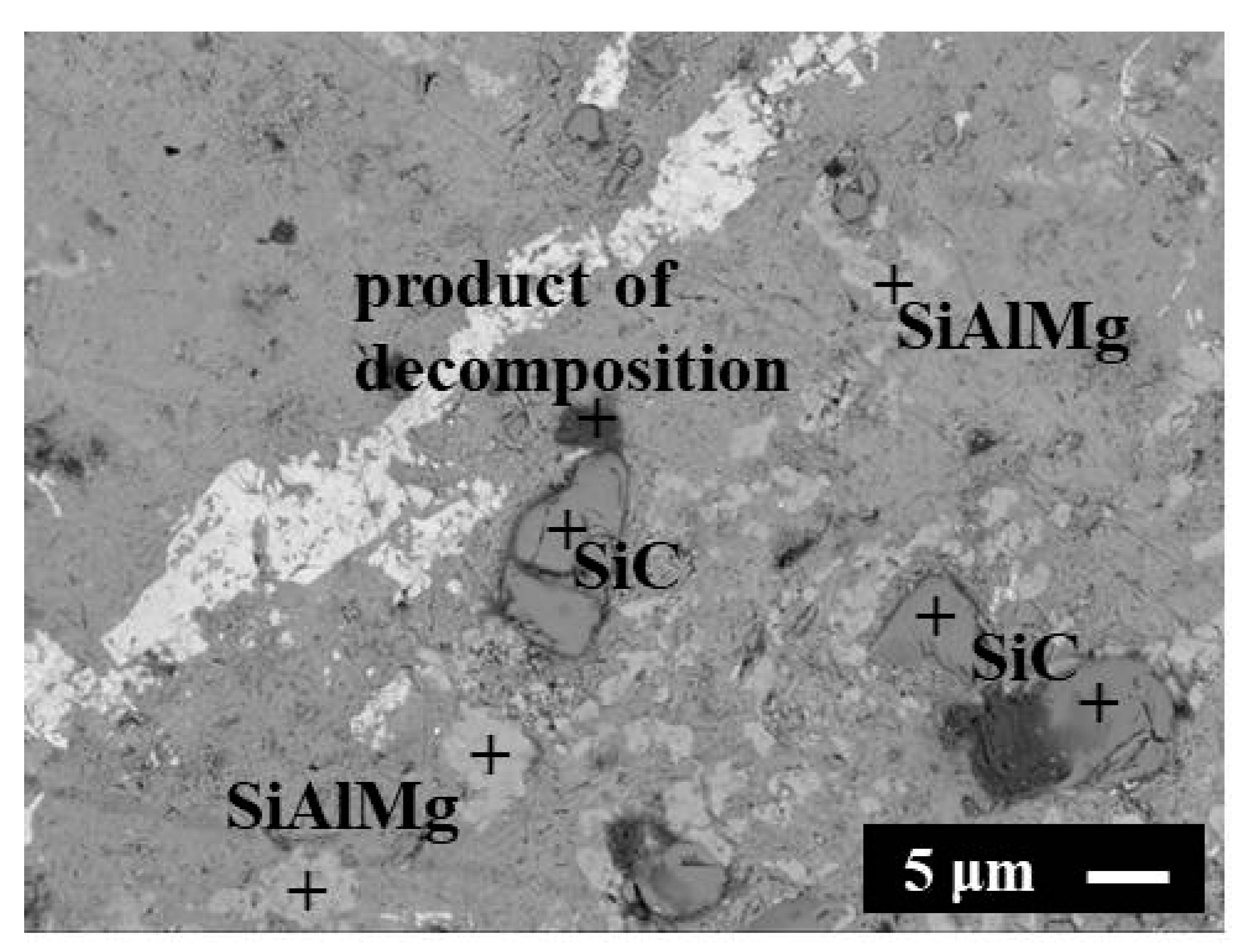
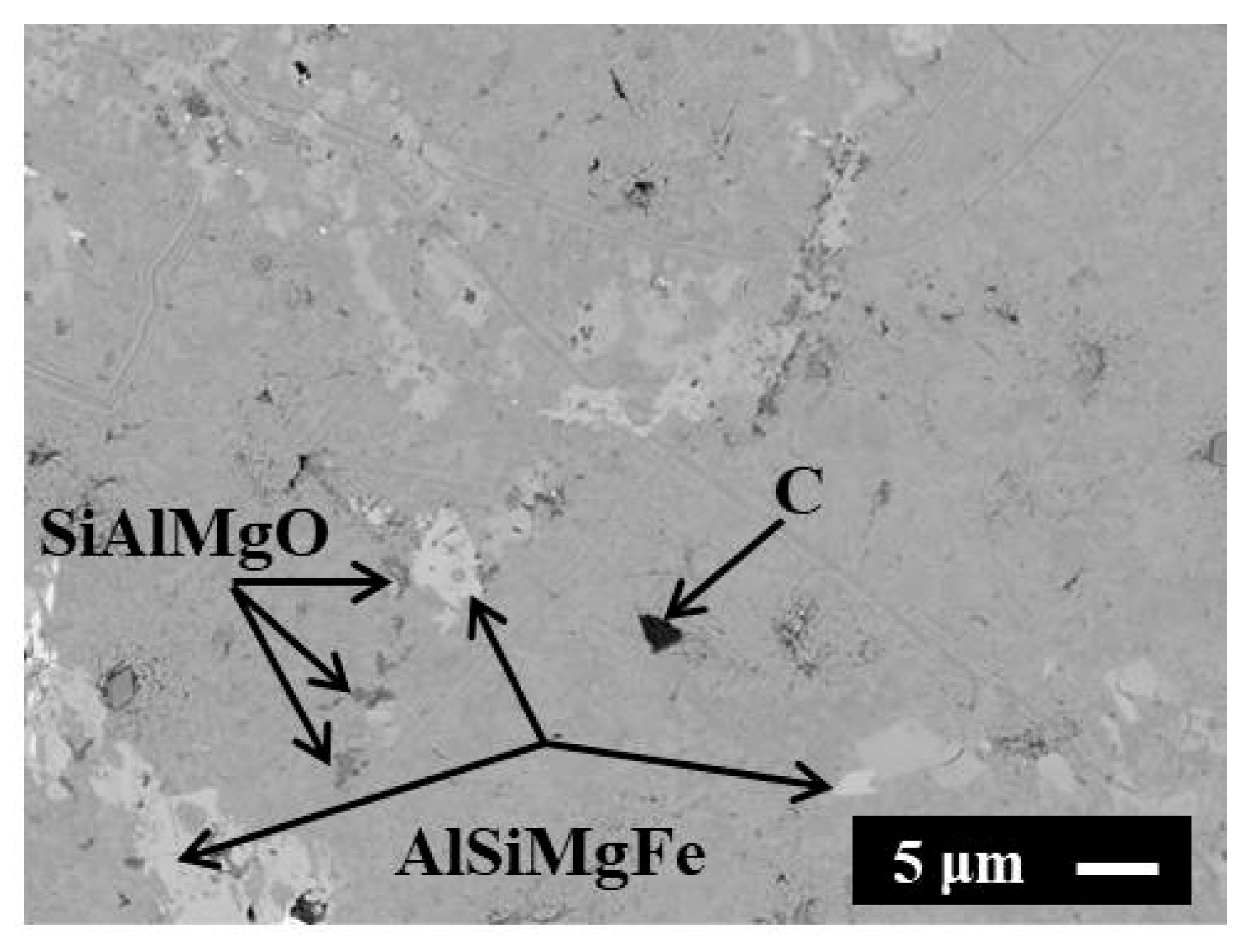


| Foam | Density | Average Diameter | Number of Pores | Average Pore Size |
|---|---|---|---|---|
| Type | (g·cm−3) | (mm) | (-) | (mm) |
| No additives | 0.52 | 0.53 ± 0.35 | 551 | 1.03 |
| 3 vol.% SiC | 0.59 | 0.39 ± 0.24 | 518 | 0.69 |
| 3 vol.% graphite | 0.64 | 0.69 ± 0.92 | 281 | 4.22 |
| Foam | Density | Resonant Frequency (Hz) | η | E | EAshby (C = 1) | C | ||
|---|---|---|---|---|---|---|---|---|
| Type | (g·cm−3) | f1 | f2 | f3 | (-) | (GPa) | (GPa) | (-) |
| No additives | 0.52 | 822 | 2368 | 4545 | 0.0023 ± 0.0007 | 1.26 ± 0.23 | 5.55 | 0.56 |
| No additives | 0.76 | 1065 | 2676 | 4823 | 0.0038 ± 0.0014 | 3.10 ± 0.18 | 2.60 | 0.49 |
| 3 vol.% SiC | 0.59 | 1002 | 2765 | 5132 | 0.0017 ± 0.0004 | 2.15 ± 0.32 | 3.84 | 0.56 |
| 3 vol.% graphite | 0.64 | 767 | 2225 | 4143 | 0.0034 ± 0.0020 | 1.36 ± 0.17 | 4.03 | 0.34 |
| Foam | Density | σcs | Strain@CS | Length of Plateau | σDs |
|---|---|---|---|---|---|
| Type | (g·cm−3) | (MPa) | (%) | (GPa) | (MPa) |
| Pure | 0.652 | 23.34 | 7.23 | 60.38 | 23.28 |
| 3 vol.% SiC | 0.694 | 20.27 | 4.16 | 63.52 | 27.18 |
| 3 vol.% graphite | 0.694 | 14.26 | 5.61 | 63.69 | 16.29 |
| Pure | 0.558 | 16.90 | 5.20 | 63.89 | 21.09 |
| 3 vol.% SiC | 0.754 | 17.95 | 3.69 | 67.16 | 25.39 |
| 3 vol.% SiC | 0.785 | 22.39 | 4.44 | 67.19 | 36.82 |
| 3 vol.% SiC | 0.818 | 26.43 | 5.37 | 60.74 | 26.46 |
| 3 vol.% graphite | 0.823 | 21.59 | 4.42 | 69.06 | 29.36 |
| 3 vol.% graphite | 0.884 | 27.55 | 5.49 | 65.76 | 27.63 |
| Foam | Density | EA@25% Strain | EA@50% Strain | EA@75% Strain |
|---|---|---|---|---|
| Type | (g·cm−3) | (MJ·m−3) | (MJ·m−3) | (MJ·m−3) |
| Pure | 0.652 | 4.83 | 10.14 | 15.71 |
| 3 vol.% SiC | 0.694 | 4.83 | 11.15 | 18.12 |
| 3 vol.% graphite | 0.694 | 2.87 | 5.76 | 9.53 |
| Pure | 0.558 | 3.73 | 7.74 | 12.63 |
| 3 vol.% SiC | 0.754 | 3.89 | 9.13 | 15.28 |
| 3 vol.% SiC | 0.785 | 5.94 | 13.89 | 22.79 |
| 3 vol.% SiC | 0.818 | 5.79 | 11.27 | 17.16 |
| 3 vol.% graphite | 0.823 | 5.32 | 10.73 | 16.74 |
| 3 vol.% graphite | 0.884 | 5.04 | 8.06 | 12.71 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kováčik, J.; Nosko, M.; Mináriková, N.; Simančík, F.; Jerz, J. Closed-Cell Powder Metallurgical Aluminium Foams Reinforced with 3 vol.% SiC and 3 vol.% Graphite. Processes 2021, 9, 2031. https://doi.org/10.3390/pr9112031
Kováčik J, Nosko M, Mináriková N, Simančík F, Jerz J. Closed-Cell Powder Metallurgical Aluminium Foams Reinforced with 3 vol.% SiC and 3 vol.% Graphite. Processes. 2021; 9(11):2031. https://doi.org/10.3390/pr9112031
Chicago/Turabian StyleKováčik, Jaroslav, Martin Nosko, Natália Mináriková, František Simančík, and Jaroslav Jerz. 2021. "Closed-Cell Powder Metallurgical Aluminium Foams Reinforced with 3 vol.% SiC and 3 vol.% Graphite" Processes 9, no. 11: 2031. https://doi.org/10.3390/pr9112031
APA StyleKováčik, J., Nosko, M., Mináriková, N., Simančík, F., & Jerz, J. (2021). Closed-Cell Powder Metallurgical Aluminium Foams Reinforced with 3 vol.% SiC and 3 vol.% Graphite. Processes, 9(11), 2031. https://doi.org/10.3390/pr9112031







