Regenerative Shaper of Ultrashort Light Pulses
Abstract
1. Introduction
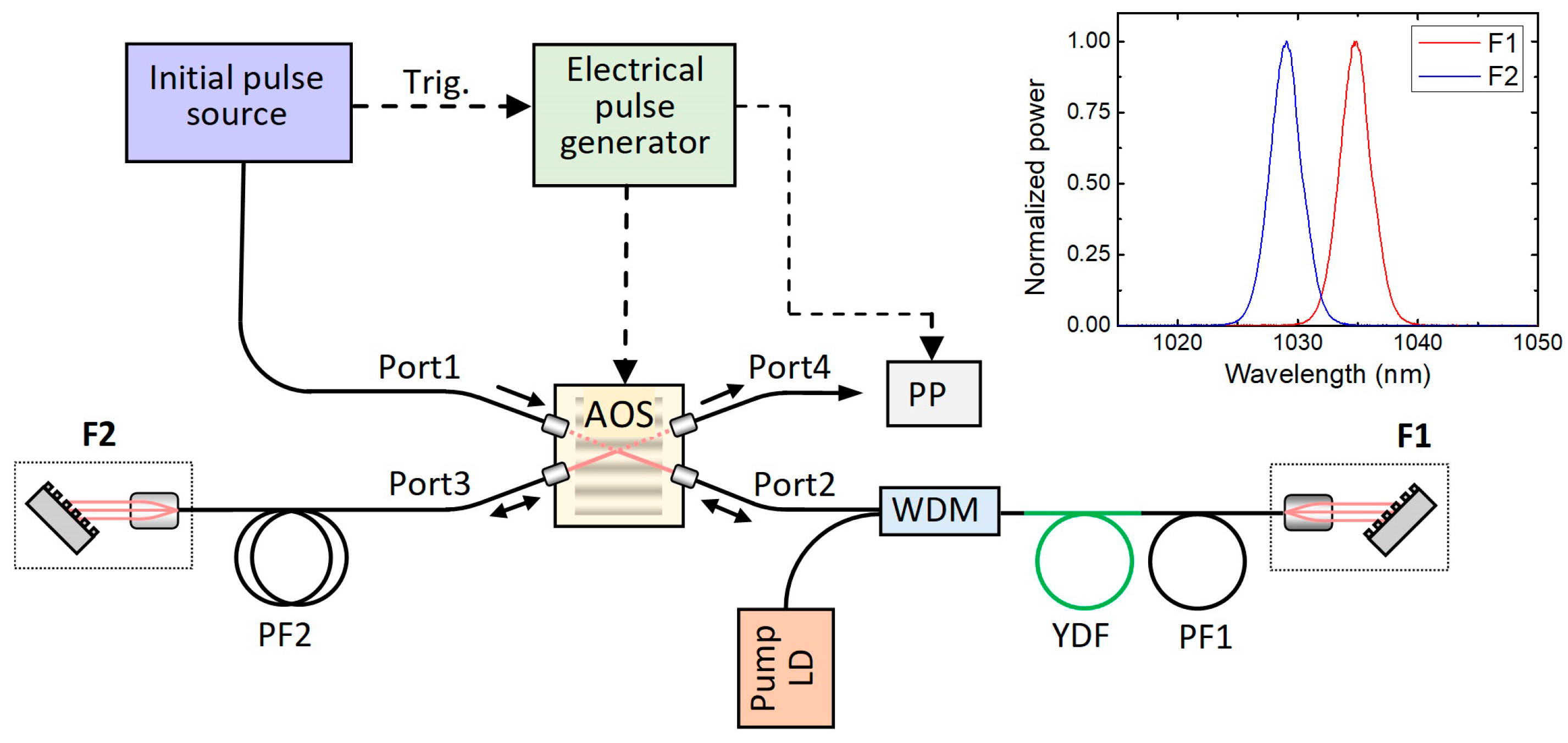
2. Experimental Setup and Numerical Simulations
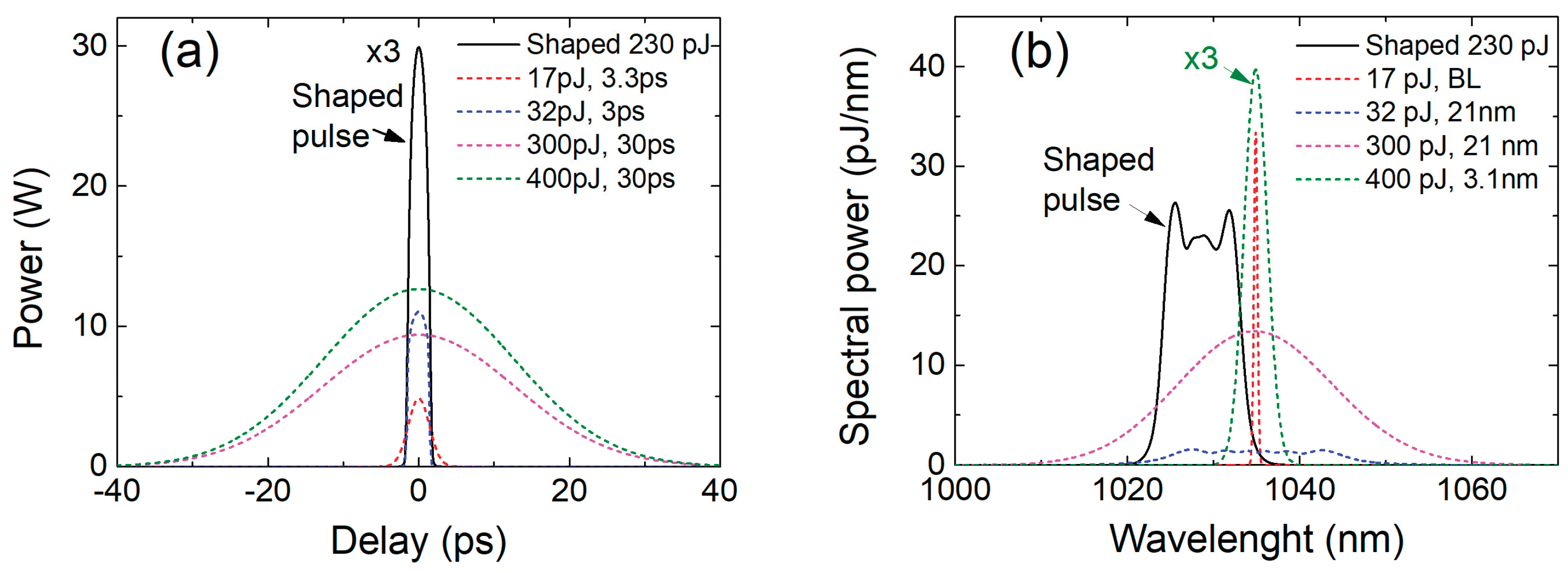
3. Experiment Results and Discussion
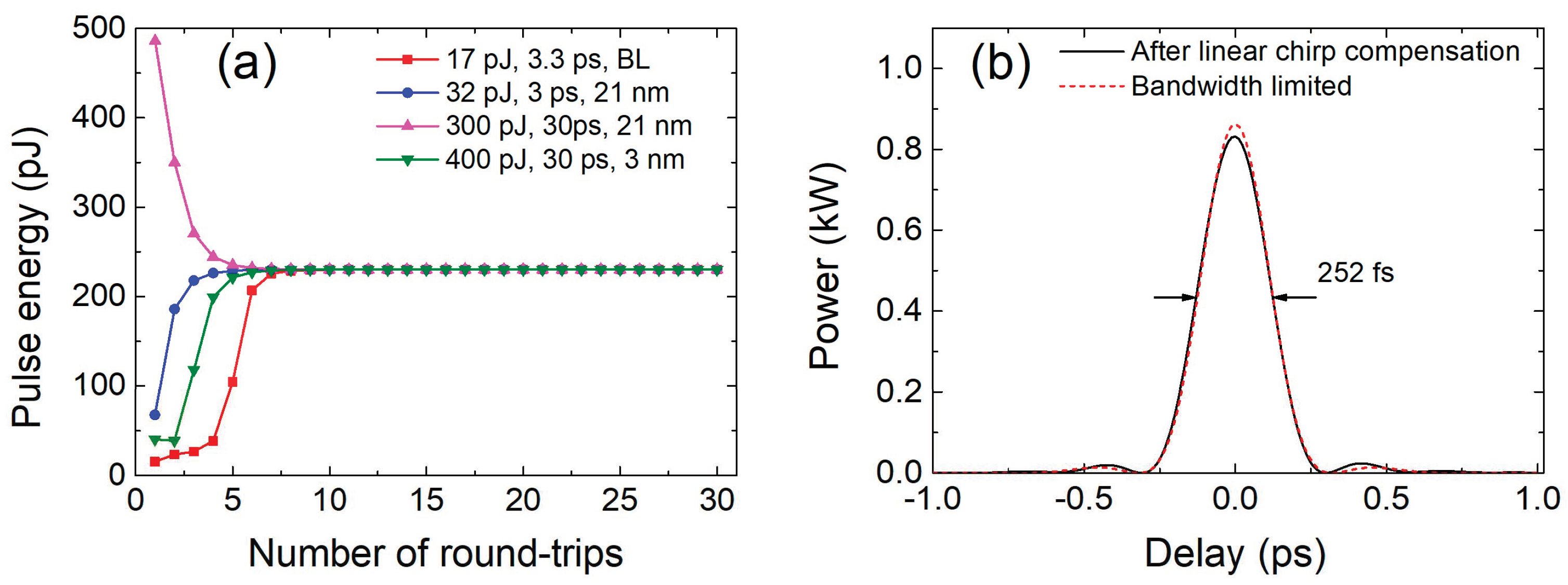
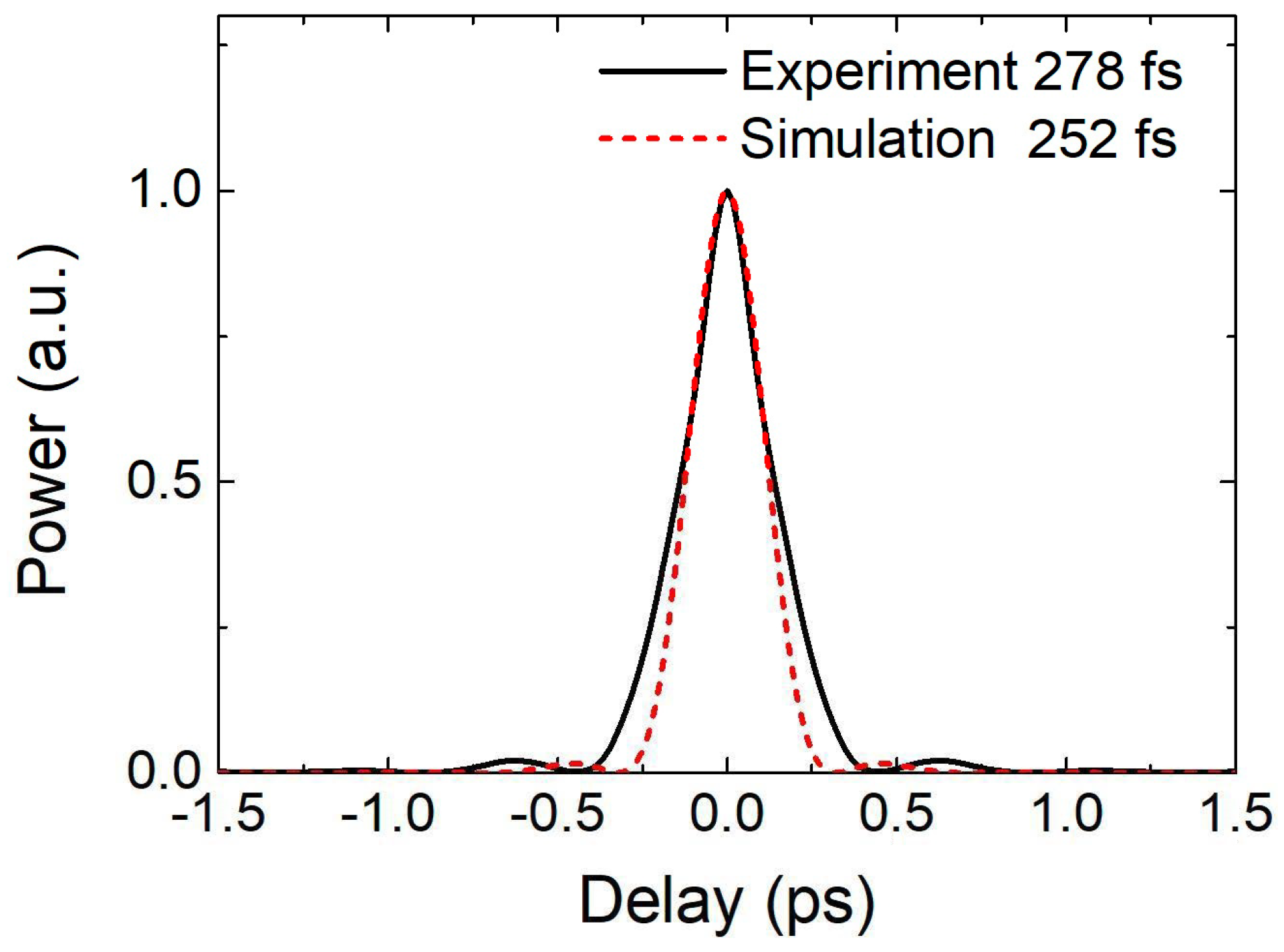
3.1. Regenerative Shaping at a Fixed Round-Trip Number
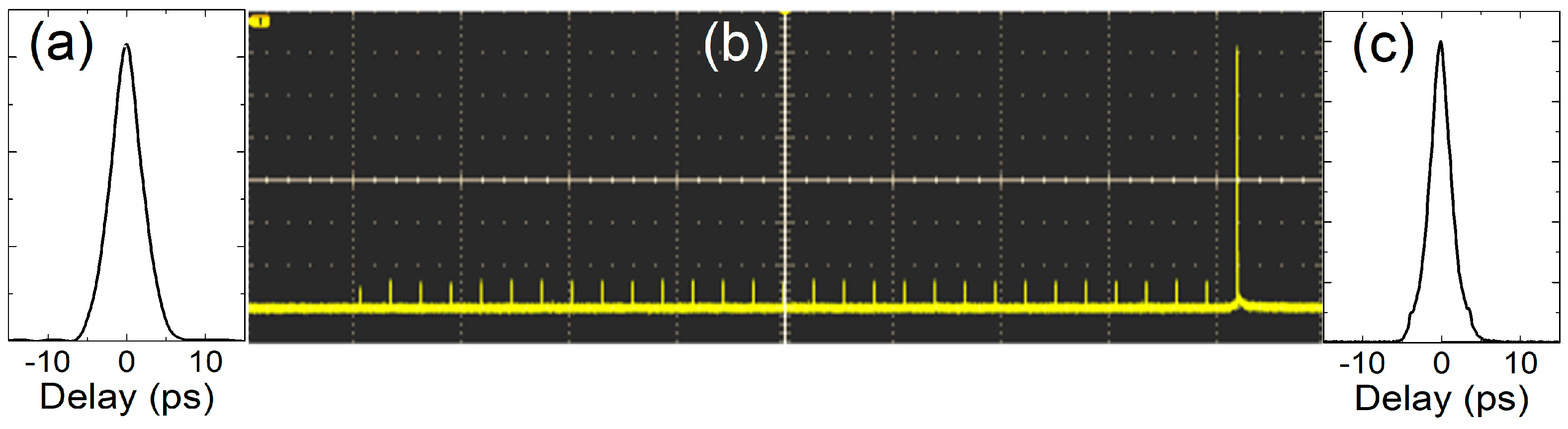
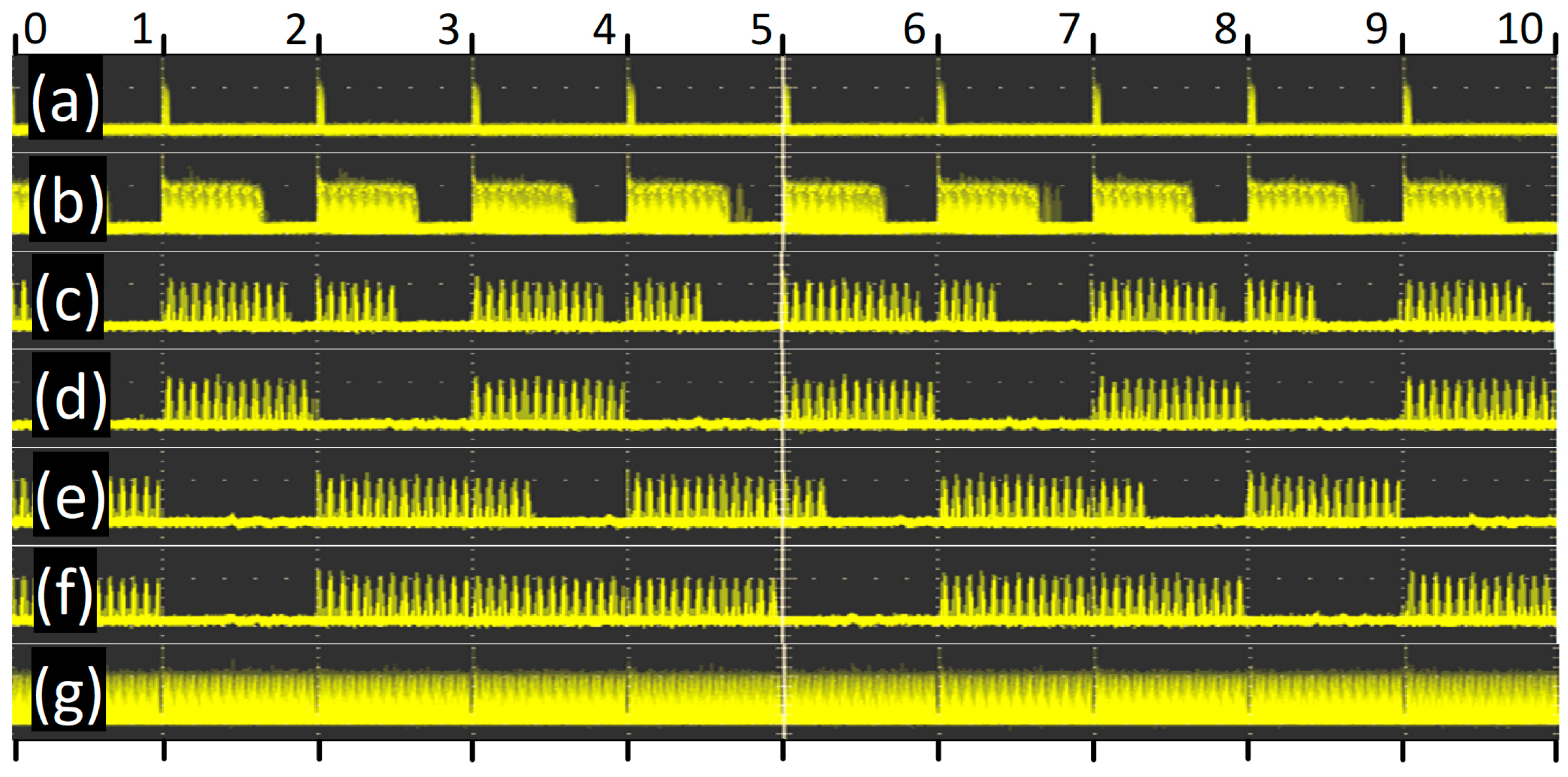
3.2. Generation of Periodic Pulse Sequences with Limited Pump Power
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Hargrove, L.E.; Fork, R.L.; Pollack, M.A. Locking of He–Ne laser modes induced by synchronous intracavity modulation. Appl. Phys. Lett. 1964, 5, 4–5. [Google Scholar] [CrossRef]
- Ippen, E.P.; Shank, C.V.; Dienes, A. Passive mode locking of the cw dye laser. Appl. Phys. Lett. 1972, 21, 348–350. [Google Scholar] [CrossRef]
- Haus, H.A. Mode-locking of lasers. J. Sel. Top. Quantum Electron. 2000, 6, 1173–1185. [Google Scholar] [CrossRef]
- Gautam, V.; Drury, J.; Choy, J.M.C.; Stricker, C.; Bachor, H.A.; Daria, V.R. Improved two-photon imaging of living neurons in brain tissue through temporal gating. Biomed. Opt. Express. 2015, 6, 402–4036. [Google Scholar] [CrossRef] [PubMed]
- Charan, K.; Li, B.; Wang, M.; Lin, C.P.; Xu, C. Fiber-based tunable repetition rate source for deep tissue two-photon fluorescence microscopy. Biomed. Opt. Express. 2018, 9, 2304–2311. [Google Scholar] [CrossRef] [PubMed]
- Petkovšek, R.; Agrež, V.; Petelin, J.; Černe, L.; Bünting, U.; Podobnik, B. Pulses on demand in fibre and hybrid lasers. J. Mech. Eng. 2019, 65, 680–689. [Google Scholar] [CrossRef]
- Lau, K.Y. Gain switching of semiconductor injection lasers. Appl. Phys. Lett. 1988, 52, 257–259. [Google Scholar] [CrossRef]
- Lin, C.; Liu, P.L.; Damen, T.C.; Eilenberger, D.J.; Hartman, R.L. Simple picosecond pulse generation scheme for injection lasers. Electron. Lett. 1980, 16, 600–602. [Google Scholar] [CrossRef]
- Paulus, P.; Langenhorst, R.; Jager, D. Generation and optimum control of picosecond optical pulses from gain-switched semiconductor lasers. IEEE J. Quant. Electron. 1988, 24, 1519–1523. [Google Scholar] [CrossRef]
- Chen, K.K.; Price, J.H.V.; Alam, S.-u.; Hayes, J.R.; Lin, D.; Malinowski, A.; Richardson, D.J. Polarisation maintaining 100 W Yb-fiber MOPA producing µJ pulses tunable in duration from 1 to 21 ps. Opt. Express 2010, 18, 14385–14394. [Google Scholar] [CrossRef] [PubMed]
- Fu, W.; Wright, L.G.; Wise, F.W. High-power femtosecond pulses without a modelocked laser. Optica 2017, 4, 831–834. [Google Scholar] [CrossRef] [PubMed]
- Mamyshev, P.V. All-optical data regeneration based on self-phase modulation effect. In Proceedings of the 24th European Conference on Optical Communication. ECOC’98 (IEEE Cat. No.98TH8398), Madrid, Spain, 20–24 September 1998; Volume 1, pp. 475–476. [Google Scholar] [CrossRef]
- Närhi, M.; Fedotov, A.; Aksenova, K.; Fiebrandt, J.; Schönau, T.; Gerecke, M.; Gumenyuk, R. Design guidelines for ultrashort pulse generation by a Mamyshev regenerator. Opt. Express 2021, 29, 15699–15710. [Google Scholar] [CrossRef] [PubMed]
- Mamyshev, P.V. Method and Apparatus for All-Optical Data Regeneration. U.S. Patent 6,141,129, 31 October 2000. [Google Scholar]
- Regelskis, K.; Želudevičius, J.; Viskontas, K.; Račiukaitis, G. Ytterbium-doped fiber ultrashort pulse generator based on self-phase modulation and alternating spectral filtering. Opt. Lett. 2015, 40, 5255–5258. [Google Scholar] [CrossRef] [PubMed]
- Liu, Z.; Ziegler, Z.M.; Wright, L.G.; Wise, F.W. Megawatt peak power from a Mamyshev oscillator. Optica 2017, 4, 649–654. [Google Scholar] [CrossRef] [PubMed]
- Želudevičius, J.; Mickus, M.; Regelskis, K. Investigation of different configurations and operation regimes of fiber pulse generators based on nonlinear spectral re-shaping. Opt. Express 2018, 26, 27247–272624. [Google Scholar] [CrossRef] [PubMed]
- Samartsev, I.; Bordenyuk, A.; Gapontsev, V. Environmentally stable seed source for high power ultrafast laser. In Proceedings of the Components and Packaging for Laser Systems III, San Francisco, CA, USA, 2 February 2017; Volume 10085, p. 100850S. [Google Scholar] [CrossRef]
- Sidorenko, P.; Fu, W.; Wright, L.G.; Olivier, M.; Wise, F.W. Self-seeded, multi-megawatt, Mamyshev oscillator. Opt. Lett. 2018, 43, 2672–2675. [Google Scholar] [CrossRef] [PubMed]
- Zheng, J.C.; Yang, S.; Lau, K.Y.; Zhu, Z.W.; Li, L. Recent research progress of Mamyshev oscillator for high energy and ultrashort pulse generation. Opt. Fiber Technol. 2021, 67, 102691. [Google Scholar] [CrossRef]
- Repgen, P.; Wandt, D.; Morgner, U.; Neumann, J.; Kracht, D. Amplification of ultrafast pulses in an extended Mamyshev regenerator. In Proceedings of the Fiber Lasers XVII: Technology and Systems, San Francisco, CA, USA, 21 February 2020; Volume 11260, p. 112600P. [Google Scholar] [CrossRef]
- Agrawal, G. Nonlinear Fiber Optics, 3rd ed.; Academic Press: San Diego, CA, USA, 2001; pp. 51–55. [Google Scholar]
- Lægsgaard, J. Control of fibre laser mode-locking by narrow-band Bragg gratings. J. Phys. B-At. Mol. Opt. 2008, 41, 095401. [Google Scholar] [CrossRef]





Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Regelskis, K.; Liaugminas, G.; Želudevičius, J. Regenerative Shaper of Ultrashort Light Pulses. Photonics 2023, 10, 836. https://doi.org/10.3390/photonics10070836
Regelskis K, Liaugminas G, Želudevičius J. Regenerative Shaper of Ultrashort Light Pulses. Photonics. 2023; 10(7):836. https://doi.org/10.3390/photonics10070836
Chicago/Turabian StyleRegelskis, Kęstutis, Gustas Liaugminas, and Julijanas Želudevičius. 2023. "Regenerative Shaper of Ultrashort Light Pulses" Photonics 10, no. 7: 836. https://doi.org/10.3390/photonics10070836
APA StyleRegelskis, K., Liaugminas, G., & Želudevičius, J. (2023). Regenerative Shaper of Ultrashort Light Pulses. Photonics, 10(7), 836. https://doi.org/10.3390/photonics10070836



