Quarter-Wave Plate Meta-Atom Metasurfaces for Continuous Longitudinal Polarization Modulation of Hybrid Poincaré Sphere Beams

Abstract

Quarter-wave plate (QWP) metasurfaces provide a novel approach for generating three-dimensional (3D) hybrid-order Poincaré sphere (HyOPS) beams and enabling longitudinal polarization modulation, owing to their unique spin-decoupling properties. In this work, we designed a set of QWP meta-atom metasurfaces that generate 3D HyOPS beams with continuously varying polarization states along the propagation direction. The third-, fourth- and fifth-order HyOPS beams are generated by three metasurface devices, respectively. The HyOPS beams exhibit a focal depth of 30 μm, a stable longitudinal propagation, and a continuously evolving polarization state. Notably, complete polarization evolution along the equator of the HyOPS occurs within a depth of 20 μm. Numerical calculations in MATLAB R2022b validated the feasibility of the designed QWP metasurfaces. The finite-difference time-domain (FDTD) simulations further confirmed the stable propagation and continuous polarization evolution of the longitudinal light field. Additionally, the concentric arrangement of the QWP meta-atoms on the metasurface effectively mitigates scattering crosstalk caused by abrupt edge phase variations. This work offers new insights into the generation and control of HyOPS light fields and contributes significantly to the development of miniaturized, functionally integrated high-performance nanophotonics.

1. Introduction

Polarization, an intrinsic property of light, plays an important role in light–matter interactions [1]. A vector light field with inhomogeneous polarization distribution can be generated by superimposing left circularly polarized (LCP) light and right circularly polarized (RCP) light, both carrying spin angular momentum (SAM) σℏ (σ = ±1) [2]. Increasing attention has been given to precisely controlling the polarization distribution of vector light fields for applications in quantum state engineering [3], the high-resolution control of twisted vector light fields [4], and optical data storage [5]. In addition to retaining vector polarization properties, vector vortex beams carry the orbital angular momentum (OAM) of lℏ (where l is the topological charge), making it a vital tool in advanced applications such as particle manipulation [6] and optical communication [7]. The polarization state of the vector vortex field can be depicted on the higher-order Poincaré sphere (HOPS) visualized by Stokes parameters [8]. The hybrid-order Poincaré sphere (HyOPS), an extension of the HOPS sphere, has been developed to describe the spin–orbit angular momentum and polarization evolution of light more generally [9]. Theoretically, HyOPS beams can be generated by superimposing two orthogonal circular polarization (CP) vortex beams with distinct topological charges [6] and have been employed in focusing [10], nonlinear optics [11], and atmospheric turbulence beam propagation [12]. Among the various methods for generating HyOPS beams, metasurfaces composed of subwavelength structures have garnered increasing attention due to their unique ability to control amplitude, polarization, and phase [13,14,15,16,17]. They have emerged as a new platform for the generation and manipulation of HyOPS beams at the nanoscale [18,19,20,21]. To address the need for multifunctional HyOPS beams, phase modulation methods of metasurfaces have advanced from single-phase modulation to composite-phase modulation, combining propagation and geometric phases [22,23,24]. With its flexible phase modulation capability, the composite-phase metasurface shows significant advantages in various fields, such as security inspection imaging [25], vector-based depth sensing [26], free-space quantum imaging [27], and holography [28].

Leveraging the flexible phase modulation capabilities of composite-phase metasurfaces, significant progress has been made in the modulation of vector vortex light fields across multiple dimensions, gradually expanding from two-dimensional (2D) to three-dimensional (3D) spatial modulation [29,30,31]. Continuous longitudinal polarization modulation introduces a new dimension to longitudinal detection and volumetric laser processing [32,33]. Significant progress has been made in generating curved propagation Bessel beams with longitudinally various OAM [26] as well as in generating HOPS beams of arbitrary polarization along the propagation direction [34,35] by half-wave plate (HWP) meta-atom metasurfaces. Since HWP metasurfaces can only modulate the cross-polarized component, their capacity for light field manipulation is inherently limited. In order to modulate the light field more flexibly, the quarter-wave plate (QWP) metasurface was employed to generate longitudinally evolving HOPS beams of arbitrary order, because of its capability to independently control co- and cross-polarized components [36,37]. However, the polarization state of the light field could only be modulated in a limited number of transverse planes. Moreover, HyOPS beams generated by using two optical vortices with different topological charges are prone to collapse during propagation [14]. Generating a stable multi-order HyOPS beam and regulating its polarization distribution remains a challenge, but it offers new prospects for the flexible control of optical forces and particle motion.

In this work, we employed a metasurface composed of QWP meta-atoms to generate 3D HyOPS beams and achieved the continuous modulation of their longitudinal polarization states. By leveraging the QWP’s ability to independently control co-polarized and cross-polarized components and the axicon phase’s capacity to confine the vortex beam radius, we generated 3D HyOPS beams with a focal depth of 30 μm using orthogonal circularly polarized beams carrying different topological charges, ensuring stable longitudinal propagation. Moreover, within a focal depth of only 20 μm, we demonstrated the continuous evolution of the polarization state along the HyOPS equator. Numerical calculations using MATLAB R2022b, corroborated by finite-difference time-domain (FDTD) simulations, confirmed the stable propagation of third- to fifth-order HyOPS beams with continuously varying polarization states along the longitudinal direction. Moreover, the concentric arrangement of the QWP meta-atoms in the designed metasurface effectively mitigates scattering crosstalk induced by phase discontinuities at the edges. This work provides a new perspective for manipulating the polarization states of vector vortex light fields and holds significant promise for advancing applications in optical tweezers and optical communication.

2. Results

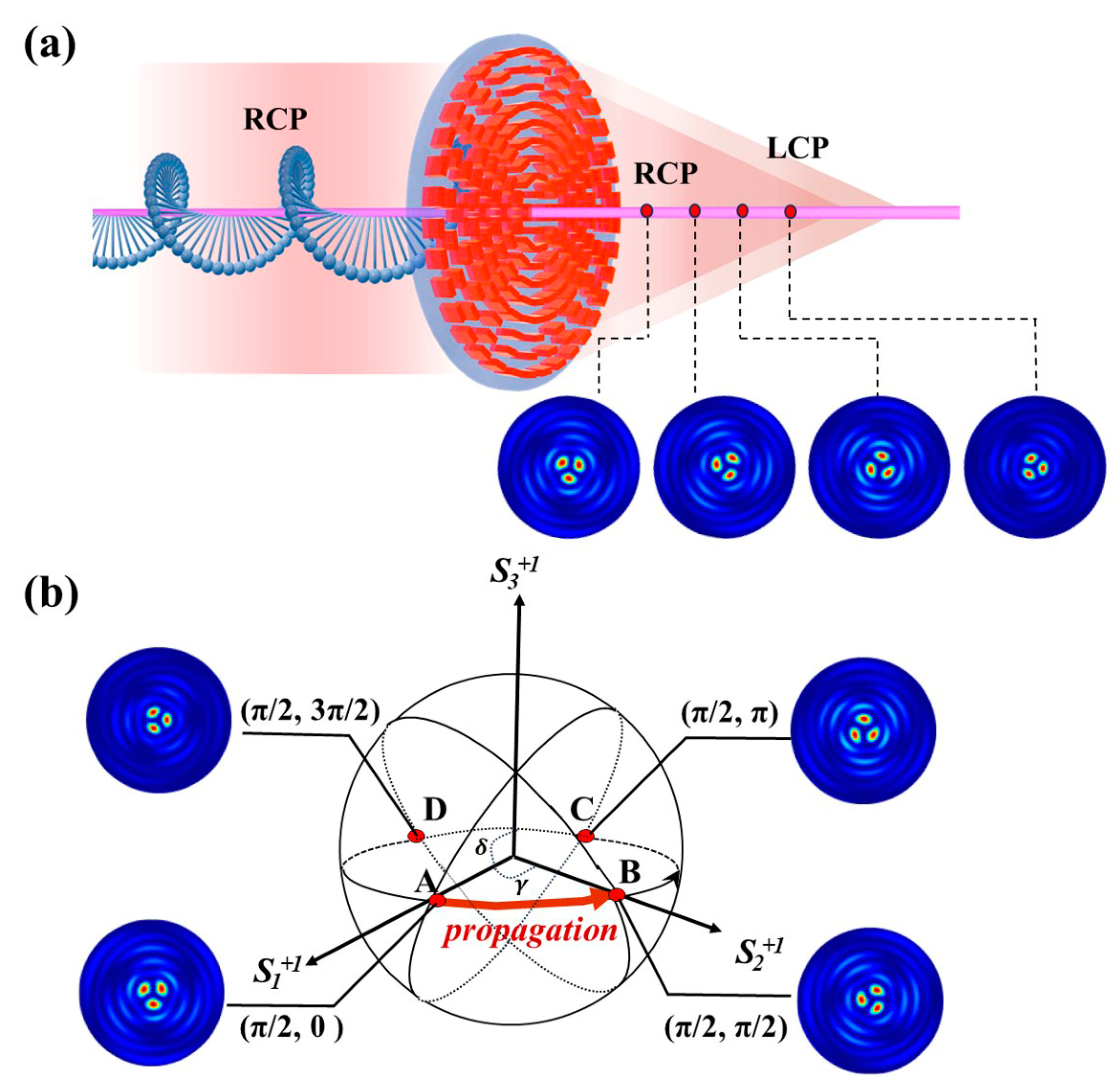
2.1. Concept of 3D HyOPS Beam

Figure 1a illustrates the longitudinal polarization modulation of HyOPS beams generated using a QWP meta-atom metasurface. In this configuration, RCP is vertically incident on the metasurface. The RCP and LCP components of the output light field were meticulously designed (as detailed in Section 2.2) to ensure that RCP and LCP beams with distinct topological charges combine within a common focal depth to synthesize HyOPS beams. These orthogonal CP vortices exhibit different Bessel light cone angles. By exploiting the symmetric photon spin–orbit interaction, the two opposite spin-state lights possess different propagation constants. Consequently, the phase difference between them continuously varies with increasing propagation distance, leading to the polarization state of the synthesized HyOPS beam to continuously evolute in a longitudinal way.

On the surface of the HyOPS, the equator represents a cylindrically symmetric, linearly polarized vector vortex beam. Specifically, the coordinates (π/2, 0) and (π/2, π) on the equator denote radial and azimuthal polarization states, respectively. As the trajectory approaches the north and south poles, the polarization states transition to LCP and RCP configurations, with the ellipticity progressively decreasing. At the poles, these states manifest as RCP and LCP vortex beams with distinct topological charges. Within the focal depth of the 3D HyOPS beam, the polarization states at distinct spatial positions approximate linear polarization. As shown in Figure 1b, as the light propagates, the polarization direction of the HyOPS beam rotates counterclockwise, corresponding to movement along the equator of the HyOPS. This dynamic evolution underscores the beam’s intrinsic polarization–vortex coupling.

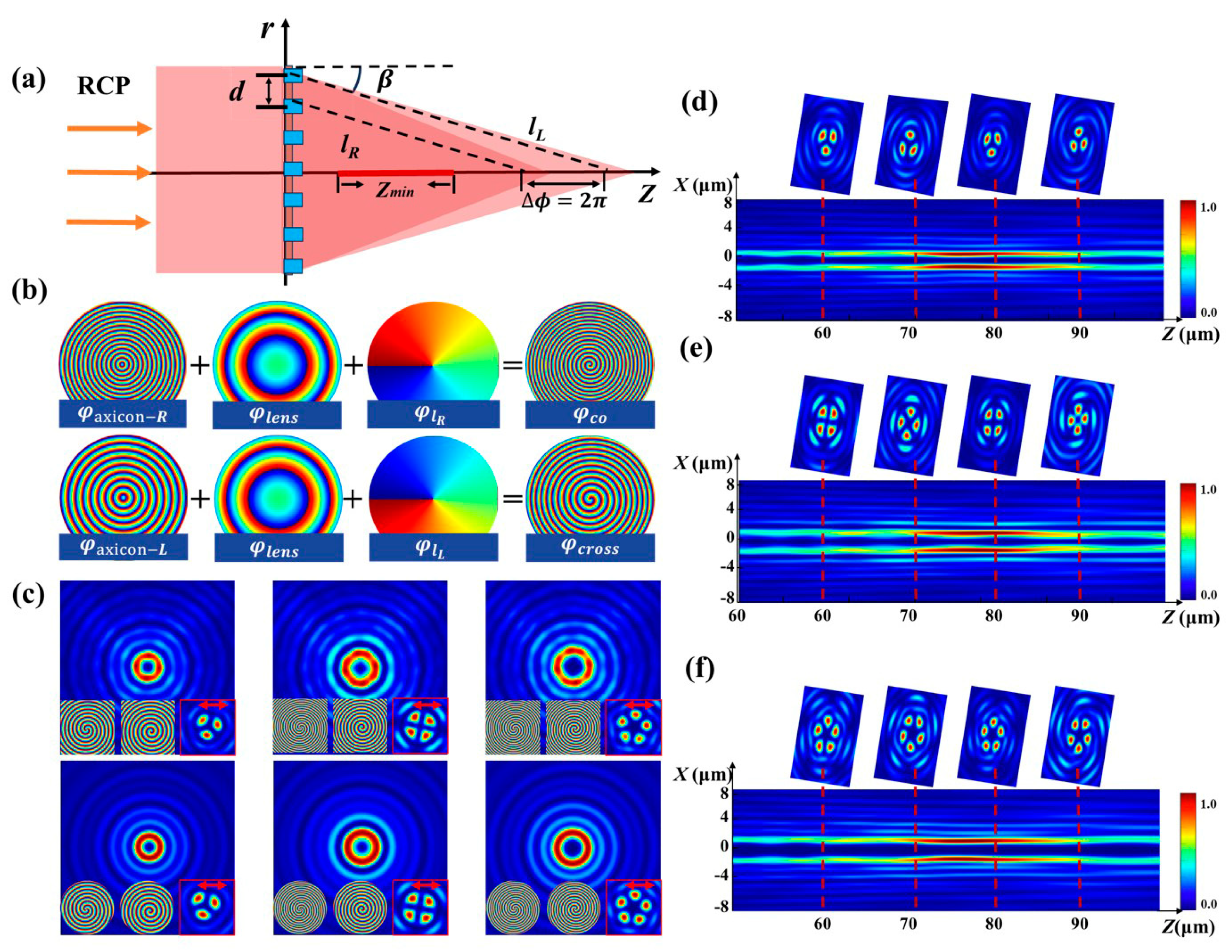
2.2. Working Principle and Numerical Calculation

Here, we detail the generation method for HyOPS beams and examine the associated variations in their polarization states. Based on the principles of HyOPS theory [9], a monochromatic paraxial beam can be effectively described using two orthogonal CP bases as follows:

$$\left|{\mathsf{\Psi }}_l(\alpha ,\beta )\right.⟩=\cos ({\displaystyle \frac{\delta }{2}})e^{i\gamma /2}\left|R_{l_R}⟩\right.+\sin ({\displaystyle \frac{\delta }{2}})e^{-i\gamma /2}\left|L_{l_L}⟩\right.$$

(1)

where $\left|R_{l_a}⟩\right.$ and $\left|L_{l_b}⟩\right.$ represent the RCP and LCP vortex beams with distinct topological charges denoted by l_R and l_L, respectively. ${\phi }_l={\tan }^{-1}(y/x)$ is used to describe the phase of the vortex beam; the term $\cos ({\displaystyle \frac{\delta }{2}})$ and $\sin ({\displaystyle \frac{\delta }{2}})$ respectively correspond to the amplitudes of the RCP and LCP vortex beams; and γ represents the phase difference between the RCP and LCP states, with $\delta$∈[0, π], $\gamma$∈[0, 2π].

As illustrated in Figure 2a, when the RCP light is incident along the Z-axis, the transmitted field’s RCP and LCP components carry distinct topological charges and exhibit different Bessel cone angles β, enabling a deflection of the beam at an angle β along the propagation direction. For a Bessel cone with angle β, the phase delay increases linearly with the radial distance from the center, thereby forming axicon phase distribution [38]:

$${\phi }_{axicon}={\displaystyle \frac{2\pi }{\lambda }}r\sin \beta$$

(2)

where d represents the radial period of the axicons, and $r=\sqrt{x^2+y^2}$. When the radial coordinate varies by d, a phase shift of 2π is introduced, expressed as follows:

$$\mathsf{\Delta }\varphi ={\displaystyle \frac{2\pi }{\lambda }}d\sin \beta =2\pi$$

(3)

In this context, d represents the radial period of the axicons. Substituting Equation (3) into Equation (2), the axicon phase distribution can be expressed as follows:

$${\phi }_{axicon}=2\pi {\displaystyle \frac{r}{d}}$$

(4)

Utilizing the zero-order diffraction approximation of a grating, we have $\lambda =d\sin \beta \approx {\displaystyle \frac{dr}{z}}$. Substituting this into Equation (4), the axicon phase distribution can be further expressed as follows:

$${\phi }_{axicon}=2\pi {\displaystyle \frac{\lambda z}{d^2}}$$

(5)

At this juncture, the axicon phase factor can be redefined as a parameter dependent on the propagation direction z. For the RCP and LCP components, which exhibit distinct radial periods d_R and d_L (d_R < d_L), the phase distribution of the axicon is given by the following equation:

$${\phi }_{axicon-R}=2\pi {\displaystyle \frac{\lambda z}{d_R^2}}$$

(6)

$${\phi }_{axicon-L}=2\pi {\displaystyle \frac{\lambda z}{d_L^2}}$$

(7)

Utilizing the axicon phase to generate perfect vortex beams has been reported, where the axicon period d and longitudinal distance z directly determine the radius of the vortex beam [39]. By designing appropriate axicon periods d_R and d_L, vortex beams carrying different topological charges can be generated with similar radii, allowing them to overlap within a common depth of focus and form stable 3D HyOPS beams during propagation. In addition, as the light field propagates in the z direction, a phase difference emerges between RCP and LCP, which is dependent on the propagation distance:

$$\mathsf{\Delta }\phi \left(z\right)=2\pi {\displaystyle \frac{\lambda z(d_L^2-d_R^2)}{d_R^2{d}_L^2}}$$

(8)

In this context, generating HyOPS beams with continuously varying polarization states along the longitudinal direction is feasible. Furthermore, to achieve a complete variation of the polarization state along the entire equator of the HyOPS, the RCP and LCP components must induce a phase change of at least π within the range from z₁ to z₂, expressed as $\mathsf{\Delta }\phi \left(z_{min}\right)=\pi$, where z_min = z₂ − z₁. Under these conditions, the following relationship holds:

$$z_{min}={\displaystyle \frac{d_R^2{d}_L^2}{2\lambda (d_L^2-d_R^2)}}$$

(9)

Given the limited common focal depth of RCP and LCP light, achieving a phase change of π within this confined focal depth is challenging. To enable their phase difference to change by π over a shorter focal depth range, a lens phase ${\phi }_{lens}={\displaystyle \frac{2\pi }{\lambda }}(f-\sqrt{r^2+f^2})$ is introduced to accelerate the rate of phase difference change along the propagation direction. Here, f denotes the focal location in the lens phase. The focusing of the optical field is predominantly modulated by the axicon phase, while the lens phase primarily facilitates the evolution of the phase difference. If the parameter f is set too large, its ability to modulate the phase difference is weakened; conversely, if f is set too small, it dominates the focusing process, significantly shortening the depth of focus and thereby failing to provide a sufficiently long focal region for the complete evolution of the polarization state. Considering these factors, we have set f = 400 nm.

In order to verify the science and feasibility of the above theory of light field modulation, the phase distribution of the metasurface was coded with MATLAB R2022b, and the diffraction of the light field was calculated. Based on the above theory, the phase distribution of the LCP and RCP components in the z = 0 plane can be expressed as follows:

$${\phi }_R(x,y)=2\pi {\displaystyle \frac{\sqrt{x^2+y^2}}{d_R}}+{\displaystyle \frac{2\pi }{\lambda }}\left(f-\sqrt{x^2+y^2+f^2}\right)+l_R{\tan }^{-1}(y/x)$$

(10)

$${\phi }_L(x,y)=2\pi {\displaystyle \frac{\sqrt{x^2+y^2}}{d_L}}+{\displaystyle \frac{2\pi }{\lambda }}\left(f-\sqrt{x^2+y^2+f^2}\right)+l_L{\tan }^{-1}(y/x)$$

(11)

Figure 2b presents the phase distribution profiles obtained from our MATLAB R2022b numerical calculations. For the metasurface (incident surface), we established a 2D grid spanning from −38 μm to 38 μm along both the X and Y axes, divided into 200 × 200 pixels. This grid represents a metasurface composed of QWP meta-atoms arranged with a period P of 380 nm, enabling an overall metasurface area of 76 μm × 76 μm. RCP light with a wavelength of 800 nm was used for illumination. To minimize edge diffraction effects inherent to the rectangular grid, complex amplitude values were assigned only to circular coordinate meshes within a radius of 38 μm, while the remaining areas were set to zero. The phase distribution of the incident plane was partitioned into two distinct components: one representing the phase distribution of the co-polarization component as described by Equation (10), and the other representing the phase distribution of the cross-polarization component as described by Equation (11). The diffraction calculation was performed using the Rayleigh–Sommerfeld diffraction formula, as detailed in Equation (10).

$$U\left(x_0,y_0\right)=-{\displaystyle \frac{i}{\lambda }}\iint U\left(x,y\right){\displaystyle \frac{F}{\rho }}{\displaystyle \frac{e^{ik\rho }}{\rho }}dxdy$$

(12)

where $\left(x_0,y_0\right)$ denotes a fixed point on the diffraction observation plane, whereas $\left(x,y\right)$ represents an arbitrary point on the metasurface. The term F represents the position of the observation plane, and $\rho =\sqrt{{(x-x_0)}^2+{(y-y_0)}^2+z^2}$ represents the distance between a point on the metasurface and a fixed point on the diffraction observation plane, and z denotes the distance from the plane of incidence (metasurface) to the diffraction observation screen.

The observation plane was defined to have dimensions comparable to the radial extent of the vortex vector beam and was set to 16 μm. The pixel resolution of the observation plane is a critical factor that determines the clarity and definition of the diffraction pattern, thereby influencing the computational accuracy. Consequently, the pixel resolution of the observation plane was set at 256 × 256. Initial observations were conducted on the X–Z plane, where an appropriate longitudinal range was selected to identify the common focal depth position with a high overlap of the RCP and LCP components. Figure 2c presents the numerical calculation results of HyOPS beams generated using both rectangular and circular phase distributions. From left to right, the beams correspond to the third-, fourth-, and fifth-order HyOPS beams. The insets, arranged from left to right, display the phase distributions of the co- and cross-polarized components, as well as the X-component of the electric field intensity. In the first row of Figure 2c, where the rectangular phase distribution is employed, significant edge diffraction effects are observed. These effects distort the HyOPS beams, which no longer exhibit an ideal “doughnut” shape but instead display an intensity profile with irregular contours and wavelike ring structures. In contrast, the second row of Figure 2c—calculated using a circular phase distribution—reveals HyOPS beams with smooth surfaces and a strictly defined doughnut-shaped intensity distribution. Therefore, the circular phase distribution is essential to greatly reduce the edge effect and maintain a uniform distribution of the light field. Subsequently, the polarization state of the HyOPS beam was characterized on the X–Y plane, as shown in Figure 2d–f. Figure 2d illustrates the electric field distribution of the HyOPS beam at various directions within the range of 50–100 μm. Specifically, within the range of 60–90 μm, the RCP and LCP components exhibit a common focal depth with good overlap. At z = 60 μm, the X-component of the electric field displays a three-petal intensity distribution. As the light field propagates along the z-direction, the petal-like field strength continues to rotate, indicating the evolution of the vector light field from radial polarization to azimuthal polarization. At z = 80 μm, it reverts to a radial polarization state, signifying the change in the polarization state of the vector vortex beam along the entire equator of the HyOPS. Beyond this region, the field intensity remains stable while the polarization state continues to evolve along the longitudinal direction. To further demonstrate the fidelity and universality of our method for other-order HyOPS beams and to enhance the information capacity, we performed numerical calculations for the fourth-and fifth-order HyOPS beams, as shown in Figure 2e,f. For higher-order HyOPS beams, this method still exhibits excellent light field control capabilities. Numerical calculation intensity patterns on the X–Z and X–Y planes are provided at different propagation distances z. The polarization state can be clearly observed to change as z increases, rotating counterclockwise as expected. Consistent with the previous observations, the evolution of the vector mode on the entire equator of the HyOPS is achieved in the range of z = 60 μm to z = 80 μm. The 3D HyOPS beams generated using this method demonstrate robust polarization control capabilities and propagation stability, as well as the universality of a series of HyOPS beams.

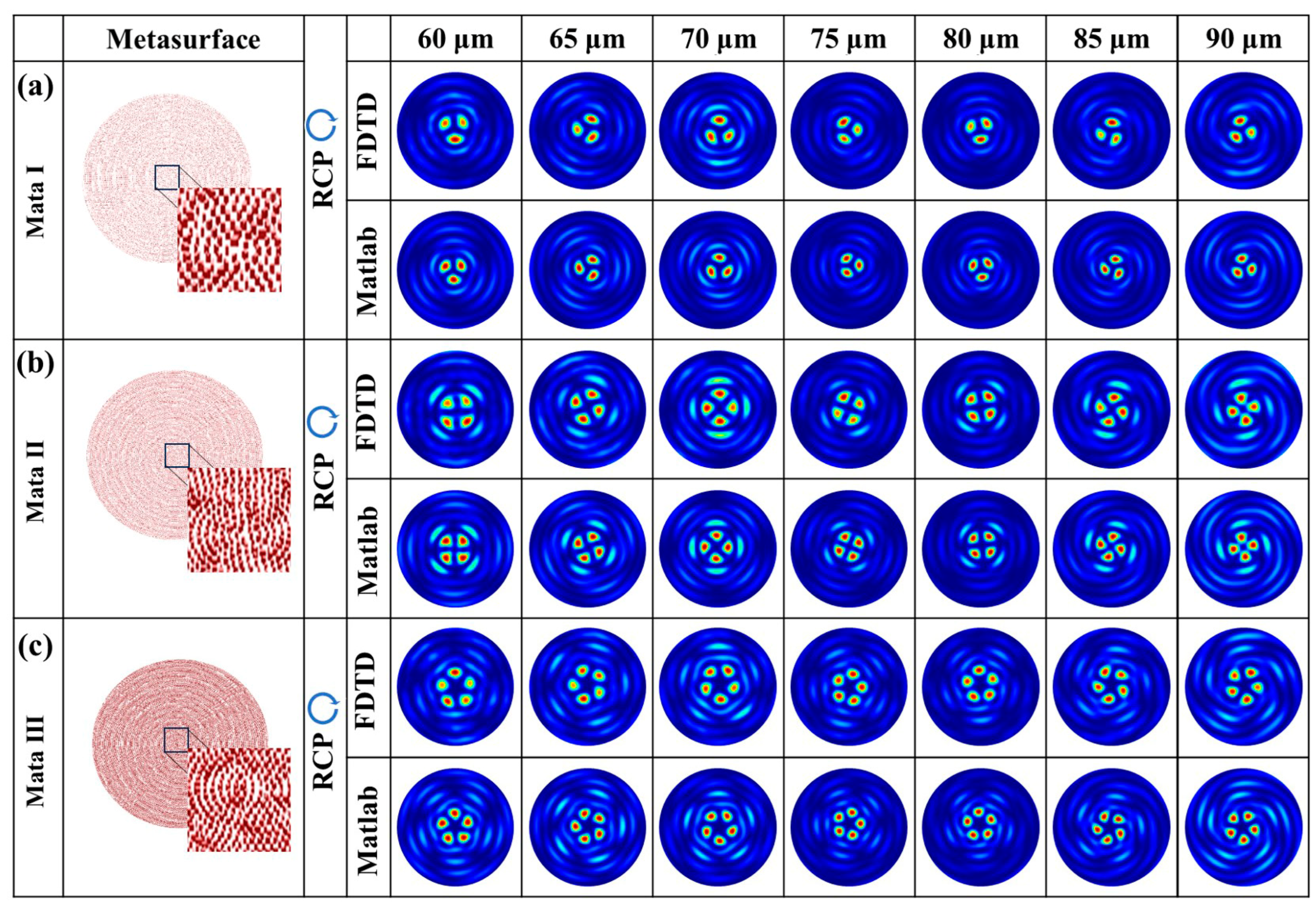
2.3. Design of Meta-Atom and Metasurface

Building on the theoretical basis, the necessary phase distribution is obtained by using a composite-phase QWP meta-atom metasurface. The Jones matrix serves as a powerful tool to characterize the polarization-conversion capabilities of the meta-atoms for the incident light. When the geometric angle θ of the anisotropic meta-atom relative to the optical axis is given, the Jones matrix is expressed as follows:

$$J\left(x,y\right)=R\left(-\theta \right)\left[\begin{array}{cc}{t}_{xx}& 0\\ 0& t_{yy}\end{array}\right]R\left(\theta \right)$$

(13)

where t_xx and t_yy represent the complex amplitudes of the anisotropic meta-atoms along the long and short axes, respectively. When the transmittance of the meta-atoms along the principal axis direction is approximately united, t_xx and t_yy can be expressed as $t_{xx}=e^{i{\phi }_x},t_{yy}=e^{i{\phi }_y}$, where ${\phi }_x$ and ${\phi }_y$ denote the phase modulation imparted by the meta-atoms along the respective axes. The rotation matrix $R\left(\theta \right)$ is defined as follows:

$$R\left(\theta \right)=\left[\begin{array}{cc}\cos \theta & \sin \theta \\ -\sin \theta & \cos \theta \end{array}\right]$$

(14)

When the phase delay of the anisotropic meta-atom along the long and short axes is $∆=\left|{\phi }_x(x,y)-{\phi }_y(x,y)\right|$, and the phase distribution ${\phi }_x$ along the long axis of the anisotropic meta-atom is selected as the transmission phase, Equation (13) is then transformed into the Jones matrix of the meta-atom with arbitrary phase delay $∆$:

$$J\left(x,y\right)=e^{i{\phi }_x(x,y)}\left[\begin{array}{cc}{\cos }^2\theta +e^{i∆}{\sin }^2\theta & (1-e^{i∆})\sin \theta \cos \theta \\ (1-e^{i∆})\sin \theta \cos \theta & {\sin }^2\theta +e^{i∆}{\cos }^2\theta \end{array}\right]$$

(15)

When the QWP meta-atom with phase delay $∆$ = π/2 is incident by CP, the transmitted field can be represented as follows:

$$J\left(x,y\right)\left|R⟩\right.=e^{i{\phi }_1(x,y)}\left|R⟩\right.+e^{i{\phi }_2(x,y)}\left|L⟩\right.$$

(16)

$$J\left(x,y\right)\left|L⟩\right.=e^{i{\phi }_1(x,y)}\left|L⟩\right.+e^{i{\phi }_3(x,y)}\left|R⟩\right.$$

(17)

where $\left|R⟩\right.={\left[\begin{array}{cc}1& -i\end{array}\right]}^T$ represents RCP light, and $\left|L⟩\right.={\left[\begin{array}{cc}1& i\end{array}\right]}^T$ represents LCP light. Here, ${\phi }_1\left(x,y\right)={\phi }_2(x,y)$, indicating that the phase of the co-polarized component of the QWP meta-atom is exclusively modulated by the propagation phase. For the phase distributions of the cross-polarized components, ${\phi }_2(x,y)$ and ${\phi }_3(x,y)$, their modulation is influenced by both the propagation phase ${\phi }_x(x,y)$ and the rotation angle $\theta (x,y)$ of the fast axis relative to the coordinate axes. Consequently, the target phase allows for the determination of the meta-atom’s propagation phase and rotation angle, which can be expressed as follows:

$${\phi }_x\left(x,y\right)={[\phi }_2\left(x,y\right)+{\phi }_3(x,y)]/2+\pi /2$$

(18)

$${\phi }_y\left(x,y\right)={[\phi }_2\left(x,y\right)+{\phi }_3(x,y)]/2+\pi$$

(19)

$$\theta \left(x,y\right)={[\phi }_3\left(x,y\right)-{\phi }_2(x,y)]/4$$

(20)

To enable multifunctional optical responses, we systematically designed the phase distributions of a QWP metasurface through hybrid modulation mechanisms. ${\phi }_1={\phi }_x$ modulated by the propagation phase includes three distinct phase profiles: ${\phi }_{axicon-R}$, ${\phi }_{lens}$ and ${\phi }_{lR}$. ${\phi }_2={\phi }_x-2{\phi }_g$ arises from the synergistic modulation of both the transmission and geometric phases, including ${\phi }_{axicon-L}$, ${\phi }_{lens}$ and ${\phi }_{lL}$. Here, the geometric phase term ${\phi }_g\left(x,y\right)$ is defined as ${\phi }_g\left(x,y\right)=2\sigma \theta (x,y)$, where σ = ±1 corresponds to the spin angular momentum of incident circularly polarized light. The spatially varying orientation angle $\theta (x,y)$ follows $\theta (x,y)={\theta }_0+m\beta (x,y)$, with ${\theta }_0$ as the meta-atom’s initial orientation and m as the topological charge governing rotational symmetry. These relationships yield the following complete phase profile expressions:

$${\phi }_1(x,y)=2\pi {\displaystyle \frac{\sqrt{x^2+y^2}}{d_R}}+{\displaystyle \frac{2\pi }{\lambda }}\left(f-\sqrt{x^2+y^2+f^2}\right)+l_R{\tan }^{-1}(y/x)$$

(21)

$${\phi }_2(x,y)=2\pi {\displaystyle \frac{\sqrt{x^2+y^2}}{d_L}}+{\displaystyle \frac{2\pi }{\lambda }}\left(f-\sqrt{x^2+y^2+f^2}\right)+l_L{\tan }^{-1}(y/x)$$

(22)

Upon illumination of a QWP meta-atom metasurface by RCP light $\left|R⟩\right.={\left[\begin{array}{cc}1& -i\end{array}\right]}^T$, the resulting co- and cross-polarized components of the transmitted light field emerge as vortex beams carrying topological charges l_R and l_L, respectively. Through the synergistic modulation of the axicon phase and the lens phase, a 3D HyOPS beam is produced within the shared focal depth.

3. Simulation Results and Discussion

Lumerical FDTD provides a comprehensive suite of dispersive material models and robust quantum optical support, making it a widely preferred tool for simulating complex light field distributions and nano-optical optimization. Compared to other simulation software such as CST Studio Suite, COMSOL Multiphysics, and Ansys HFSS, FDTD demonstrates superior computational accuracy and efficiency, particularly for simulating large-area devices composed of nanostructures. Therefore, we selected FDTD 2024R1 for our simulations to ensure results that are both realistic and precise.

Figure 3a illustrates a schematic of the metasurface and its unit cell structure that was applied in practice. On a fused silica SiO₂ substrate, we designed anisotropic rectangular dielectric a-Si: H pillars with a height of h = 480 nm and period P of 380 nm to serve as the meta-atoms of the metasurface. At a wavelength of λ = 800 nm, the refractive index and extinction coefficient of a-Si: H are n = 3.744 and κ = 0.000, respectively. By varying the lengths L₁ and L₂ in two orthogonal directions, we could control its phase change to cover the entire 2π range. Additionally, by rotating the rectangular silicon pillar, an extra geometric phase could be generated. Using FDTD, we conducted a parameter sweep of the meta-atom with a unit cell length, width, and height of 380 nm, 380 nm, and 4000 nm with corresponding boundary conditions of “Periodic”, “Periodic”, and “PML”. Linearly polarized (LP) light was used as incident light, illuminating the meta-atom along the x-and y-directions. Then, based on the sweep results, we selected eight meta-atoms that could cover the transmission phase from 0 to 2π with an increment of approximately π⁄4, as shown in Figure 3b. This linearly increasing phase arrangement facilitates the design of the metasurface phase profile. Additionally, Figure 3b shows that the eight meta-atoms we selected had a transmittance of over 90% in both the x-and y-directions, which greatly ensures the uniformity of the transmitted light. To achieve independent control of the co- and cross-polarized components using both propagation and geometric phases, the phase delay of each meta-atom is $\left|{\phi }_x(x,y)-{\phi }_y(x,y)\right|=\pi ⁄2$, at which point the meta-atom acts as a QWP.

Based on the definitions of the transmission phase profile and geometric phase profile of the metasurface in Equations (21) and (22), we selected appropriately sized QWP meta-atoms and assigned them specific orientation angles θ to design the metasurface structure. We then carried out simulations of the longitudinal evolution of the polarization state of the HyOPS beam for the designed metasurface. The three designed metasurfaces (referred to as Meta I, Meta II, and Meta III) each had a radius of 38 μm. The simulation region was set to a range of 80 μm × 80 μm × 3 μm, with the x, y, and z directions configured with PML boundary conditions. It is worth mentioning that to save computational space and improve computational speed, the calculation range in the z-direction does not need to cover the entire light field transmission range. Instead, projecting the light field data from the monitor to the far field is sufficient to obtain the wave field within the observation plane, and the monitor in FDTD was placed at 1.5 wavelengths from the metasurface.

Figure 4 illustrates the FDTD simulation results of the three samples along with a comparison to the numerical calculation results. The second column of Figure 4 presents both the full view and locally magnified images of the metasurfaces of QWP meta- atoms corresponding to the three samples. The first row of Figure 4a illustrates the simulation results of the longitudinal evolution of the polarization state of the third-order HyOPS beam for Meta I under RCP incidence. It is evident that as z increases, the polarization state evolves, with the X-polarized component of the beam forming a three-petal pattern that rotates in a counterclockwise direction. Similar to Meta I, Meta II and Meta III also demonstrate highly flexible polarization-state modulation capabilities under the same incidence conditions, as shown in Figure 4b,c. The simulation results are in excellent agreement with the numerical calculations. In the simulation, the vector vortex beam transforms from radial polarization to azimuthal polarization and back to radial polarization, achieving the comprehensive modulation of the polarization state along the equator of the HyOPS. Moreover, the simulation demonstrates stable propagation of the HyOPS beam over a considerable depth of field, as shown in Figure 5a–c. The overall quality of the HyOPS beam in the simulation is satisfactory, and the strong agreement between the simulation and numerical results further confirms the correctness of the theory and methodology. Compared to the 3D vector vortex beams reported for HWP meta-atom metasurfaces, the simulation realization of higher-order HyOPS sphere beams carrying richer OAM indicates the great potential of QWP meta-atom metasurfaces for further enhancing information capacity.

4. Conclusions

In this work, we designed metasurfaces composed of QWP meta-atoms to generate 3D HyOPS beams with polarization states that continuously vary along the propagation direction of light, leveraging a strong spin-decoupling capability of QWP meta-atoms. By precisely engineering the co- and cross-polarized components of the QWP metasurface, third-, fourth-, and fifth-order HyOPS beams with continuously varying polarization states over an extended focal depth of 30 μm under RCP light illumination were generated. Notably, the complete evolution of the polarization state along the equator of the HyOPS is achieved within a range of 20 μm. MATLAB R2022b numerical calculations and FDTD simulations confirmed the reliability of this approach for stable beam propagation and continuous longitudinal polarization modulation. This research not only expands the potential applications of 3D HyOPS light fields but also demonstrates significant promise for more complex vector light fields, such as full-Poincaré sphere vector beams and multi-singularity vector beams.