Far-Field Topological Structure of the Second Harmonic from Higher-Order Poincaré Sphere Beam
Abstract
:1. Introduction
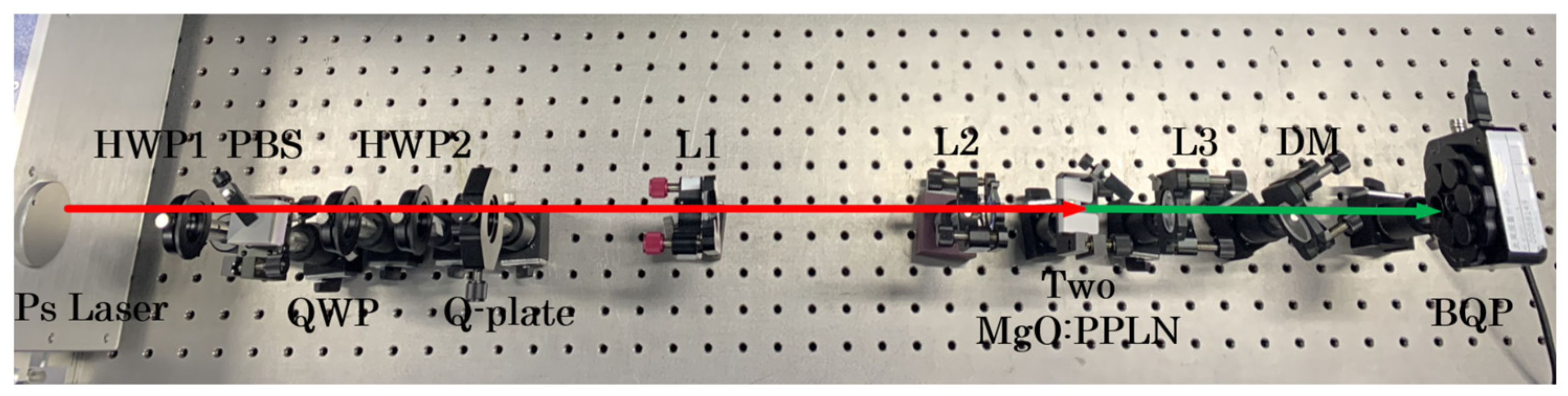
2. Theory
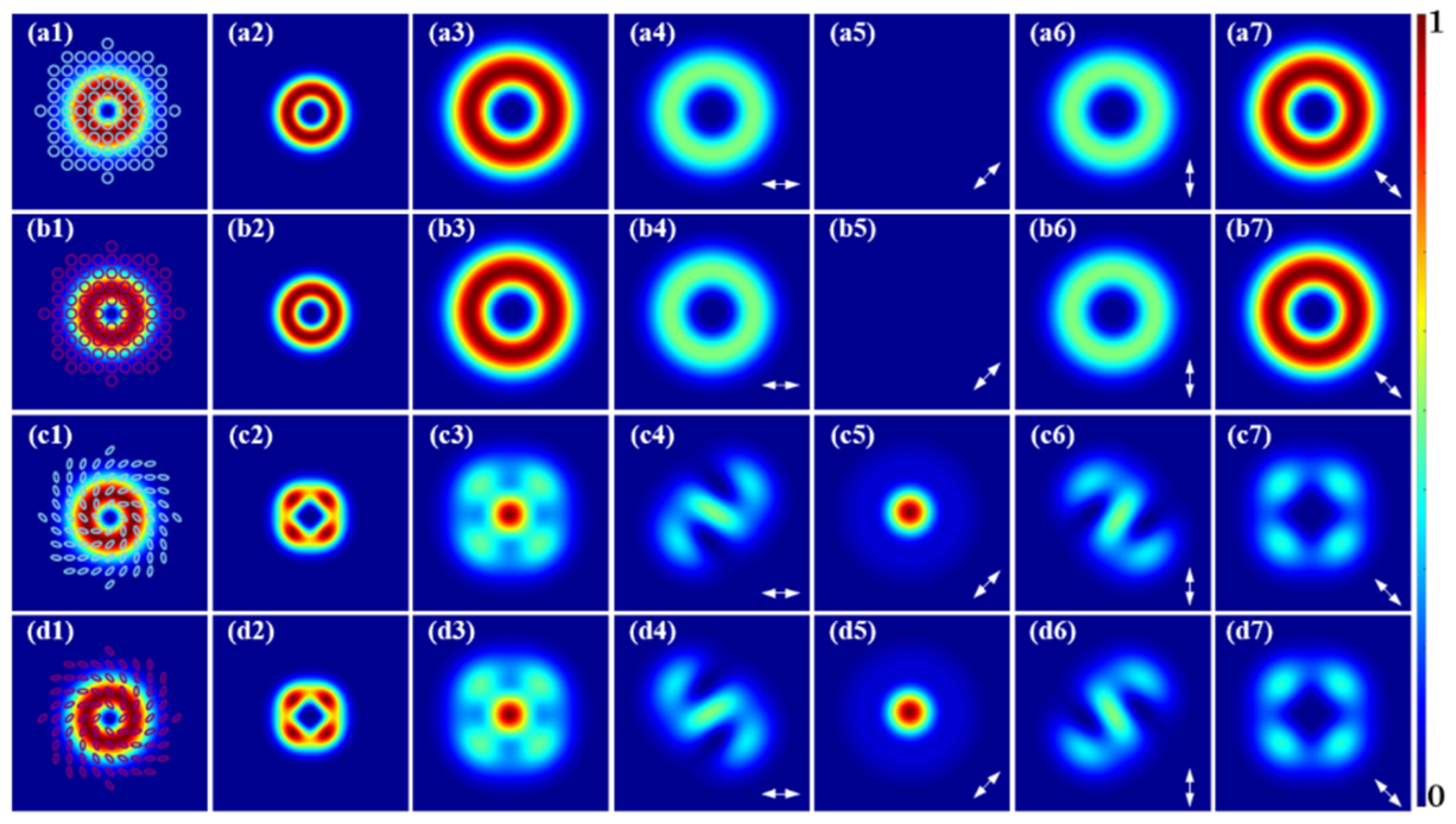
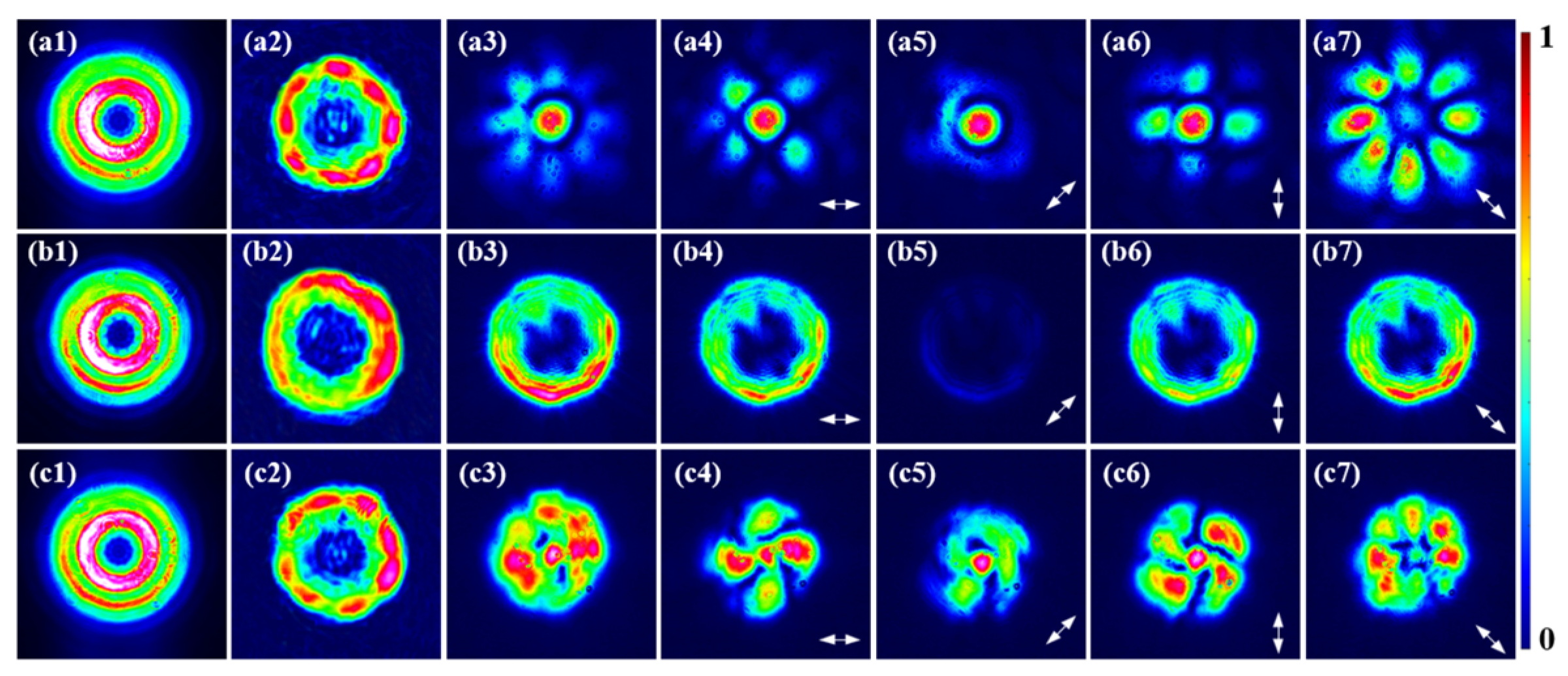
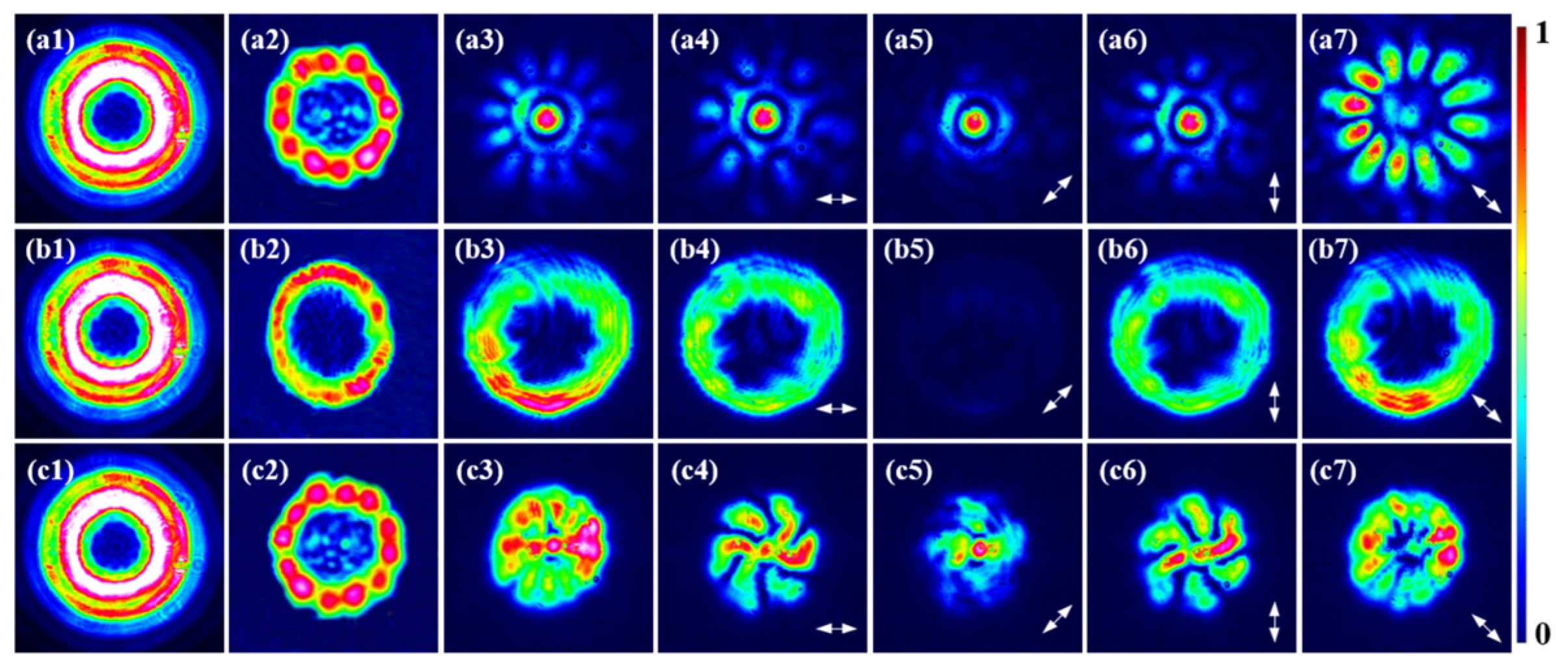
3. Results and Discussions
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Milione, G.; Sztul, H.I.; Nolan, D.A.; Alfano, R.R. Higher-Order Poincaré Sphere, Stokes Parameters, and the Angular Momentum of Light. Phys. Rev. Lett. 2011, 107, 053601. [Google Scholar] [CrossRef] [PubMed]
- Chen, S.Z.; Zhou, X.X.; Liu, Y.C.; Wen, S.C. Generation of arbitrary cylindrical vector beams on the higher order Poincaré sphere. Opt. Lett. 2014, 39, 5274–5276. [Google Scholar] [CrossRef]
- Zhang, S.Y.; Zhao, W.Q.; Dou, J.T.; Hu, Y.Y. Full higher-order Poincaré sphere beam mode-locked fiber laser. Opt. Laser Technol. 2024, 176, 110933. [Google Scholar] [CrossRef]
- Forbes, A.; Oliveira, M.D.; Dennis, M.R. Structured light. Nat. Photonics 2021, 15, 253–262. [Google Scholar] [CrossRef]
- Rui, G.H.; Zhan, Q.W. Trapping of resonant metallic nanoparticles with engineered vectorial optical field. Nanophotonics 2014, 3, 351–361. [Google Scholar] [CrossRef]
- Wang, Q.; Tu, C.H.; He, H.; Xia, Z.C.; Hou, X.Z.; Li, Y.N.; Wang, H.T. Local angular momentum induced dual orbital effect. APL Photonics 2022, 7, 086102. [Google Scholar] [CrossRef]
- Carruthers, A.E.; Walker, J.S.; Casey, A.; Orr-Ewing, A.J.; Reid, J.P. Selection and characterization of aerosol particle size using a Bessel beam optical trap for single particle analysis. Phys. Chem. Chem. Phys. 2012, 14, 6741–6748. [Google Scholar] [CrossRef]
- Drevinskas, R.; Zhang, J.Y.; Beresna, M.; Gecevičius, M.; Kazanskii, A.G.; Svirko, Y.P.; Kazansky, P.G. Laser material processing with tightly focused cylindrical vector beams. Appl. Phys. Lett. 2016, 108, 221107. [Google Scholar] [CrossRef]
- Liu, M.; Lei, Y.; Yu, L.; Fang, X.; Ma, Y.; Liu, L.; Zheng, J.; Gao, P. Super-resolution optical microscopy using cylindrical vector beams. Nanophotonics 2022, 11, 3395–3420. [Google Scholar] [CrossRef]
- Xiao, F.J.; Shang, W.Y.; Zhu, W.R.; Han, L.; Premaratne, M.; Mei, T.; Zhao, J.L. Cylindrical vector beam-excited frequency-tunable second harmonic generation in a plasmonic octamer. Photonics Res. 2018, 6, 157–161. [Google Scholar] [CrossRef]
- Zhang, L.; Lin, F.; Qiu, X.D.; Chen, L.X. Full vectorial feature of second-harmonic generation with full Poincaré beams. Chin. Opt. Lett. 2019, 17, 091901. [Google Scholar] [CrossRef]
- Saripalli, R.K.; Ghosh, A.; Chaitanya, N.A.; Samanta, G.K. Frequency-conversion of vector vortex beams with space-variant polarization in single-pass geometry. Appl. Phys. Lett. 2019, 115, 051101. [Google Scholar] [CrossRef]
- Buono, W.T.; Forbes, A. Nonlinear optics with structured light. Opto-Electron. Adv. 2022, 5, 210174. [Google Scholar] [CrossRef]
- Jiang, Q.N.; Zhao, M.L.; Wang, Y.X.; Wang, S.L.; Dou, J.T.; Liu, J.; Li, B.; Hu, Y.Y. Second harmonic of higher-order Poincaré sphere beam with two orthogonal 5% MgO: PPLN crystals. APL Photonics 2024, 9, 056111. [Google Scholar] [CrossRef]
- Liu, H.G.; Li, H.; Zheng, Y.L.; Chen, X.F. Nonlinear frequency conversion and manipulation of vector beams. Opt. Lett. 2018, 43, 5981–5984. [Google Scholar] [CrossRef]
- Chaitanya, N.A.; Jabir, M.V.; Samanta, G.K. Efficient nonlinear generation of high power, higher order, ultrafast “perfect” vortices in green. Opt. Lett. 2016, 41, 1348–1351. [Google Scholar] [CrossRef] [PubMed]
- Tang, Y.T.; Li, K.F.; Zhang, X.C.; Deng, J.H.; Li, G.X.; Brasselet, E. Harmonic spin–orbit angular momentum cascade in nonlinear optical crystals. Nat. Photonics 2020, 14, 658–662. [Google Scholar] [CrossRef]
- Fickler, R.; Lapkiewicz, R.; Ramelow, S.; Zeilinger, A. Quantum entanglement of complex photon polarization patterns in vector beams. Phys. Rev. A 2014, 89, 060301. [Google Scholar] [CrossRef]
- Bliokh, K.Y.; Fortuño, F.J.R.; Nori, F.; Zayats, A.V. Spin–orbit interactions of light. Nat. Photonics 2015, 9, 796–808. [Google Scholar] [CrossRef]
- Cardano, F.; Marrucci, L. Spin-orbit photonics. Nat. Photonics 2015, 9, 776–778. [Google Scholar] [CrossRef]
- Fatemi, F.K. Cylindrical vector beams for rapid polarization-dependent measurements in atomic systems. Opt. Express 2011, 19, 25143–25150. [Google Scholar] [CrossRef] [PubMed]
- He, C.; Shen, Y.J.; Forbes, A. Towards higher-dimensional structured light. Light Sci. Appl. 2022, 11, 205. [Google Scholar] [CrossRef] [PubMed]
- Pereira, L.J.; Buono, W.T.; Tasca, D.S.; Dechoum, K.; Khoury, A.Z. Orbital-angular-momentum mixing in type-II second-harmonic generation. Phys. Rev. A 2017, 96, 053856. [Google Scholar] [CrossRef]
- Rao, A.S.; Miamoto, K.; Omatsu, T. Ultraviolet intracavity frequency-doubled Pr3+:LiYF4 orbital Poincaré laser. Opt. Express 2020, 28, 37397–37405. [Google Scholar] [CrossRef]
- Wu, H.J.; Yang, H.R.; Rosales-Guzmán, C.; Gao, W.; Shi, B.S.; Zhu, Z.H. Vectorial nonlinear optics: Type-II second-harmonic generation driven by spin-orbit-coupled fields. Phys. Rev. A 2019, 100, 053840. [Google Scholar] [CrossRef]
- Wu, H.J.; Zhou, Z.Y.; Gao, W.; Shi, B.S.; Zhu, Z.H. Dynamic tomography of the spin-orbit coupling in nonlinear optics. Phys. Rev. A 2019, 99, 023830. [Google Scholar] [CrossRef]
- Zhou, Z.Y.; Zhu, Z.H.; Liu, S.L.; Li, Y.H.; Shi, S.; Ding, D.S.; Chen, L.X.; Gao, W.; Guo, G.C.; Shi, B.S. Quantum twisted double-slits experiments: Confirming wavefunctions’ physical reality. Sci. Bull. 2017, 62, 1185–1192. [Google Scholar] [CrossRef]
- Zhen, W.; Ren, Z.C.; Wang, X.L.; Ding, J.; Wang, H.T. Polarization structure transition of C-point singularities upon reflection. Sci. China Phys. Mech. 2025, 68, 244211. [Google Scholar] [CrossRef]
- Neugebauer, M.; Banzer, P.; Bauer, T.; Orlov, S.; Lindlein, N.; Aiello, A.; Leuchs, G. Geometric spin Hall effect of light in tightly focused polarization-tailored light beams. Phys. Rev. A 2014, 89, 013840. [Google Scholar] [CrossRef]
- Hu, Y.Y.; Ma, Z.Y.; Zhao, W.Q.; Zhao, J.; Liu, J.; Jing, Q.L.; Dou, J.T.; Li, B. Controlled generation of mode-switchable nanosecond pulsed vector vortex beams from a Q-switched fiber laser. Opt. Express 2022, 30, 33195–33207. [Google Scholar] [CrossRef]
- Ling, X.H.; Guan, F.X.; Cai, X.D.; Ma, S.J.; Xu, H.X.; He, Q.; Xiao, S.Y.; Zhou, L. Topology-Induced Phase Transitions in Spin-Orbit Photonics. Laser Photonics Rev. 2021, 15, 2000492. [Google Scholar] [CrossRef]
- Ma, Z.Y.; Zhao, W.Q.; Zhao, J.; Liu, J.; Jing, Q.L.; Dou, J.T.; Li, B.; Hu, Y.Y. Generation of arbitrary higher-order Poincaré sphere beam from a ring fiber laser with cascaded Q-plates. Opt. Laser Technol. 2022, 156, 108552. [Google Scholar] [CrossRef]
- Duque, C.B.; Sucerquia, J.G. Non-approximated Rayleigh–Sommerfeld diffraction integral: Advantages and disadvantages in the propagation of complex wave fields. Appl. Opt. 2019, 58, G11–G18. [Google Scholar] [CrossRef]
- Collins, S.A. Lens-System Diffraction Integral Written in Terms of Matrix Optics. J. Opt. Soc. Am. 1970, 60, 1168–1177. [Google Scholar] [CrossRef]
- Cai, Y.J.; He, S.L. Propagation of hollow Gaussian beams through apertured paraxial optical systems. J. Opt. Soc. Am. A 2006, 23, 1410–1418. [Google Scholar] [CrossRef]
- Zhang, L.; Qiu, X.D.; Li, F.S.; Liu, H.G.; Chen, X.F.; Chen, L.X. Second harmonic generation with full Poincaré beams. Opt. Express 2018, 26, 11678–11684. [Google Scholar] [CrossRef]
- Wen, Z.X.; Wang, Y.X.; Dou, J.T.; Fan, L.; Li, B.; Hu, Y.Y. Controlled generation of double-ring-shaped generalized cylindrical vector beams from a YVO4/Nd: YVO4 laser with ring-shaped defect mirror. Opt. Laser Technol. 2025, 186, 112718. [Google Scholar] [CrossRef]
- Zhan, Q.W. Cylindrical vector beams: From mathematical concepts to applications. Adv. Opt. Photon. 2009, 1, 1–57. [Google Scholar] [CrossRef]
- Zhang, Z.L.; Gao, Y.; Li, X.J.; Wang, X.; Zhao, S.Y.; Liu, Q.; Zhao, C.M. Second harmonic generation of laser beams in transverse mode locking states. Adv. Photonics 2022, 4, 026002. [Google Scholar] [CrossRef]
- Liu, S.L.; Zhou, Z.Y.; Liu, S.K.; Li, Y.H.; Li, Y.; Yang, C.; Xu, Z.H.; Liu, Z.D.; Guo, G.C.; Shi, B.S. Coherent manipulation of a three-dimensional maximally entangled state. Phys. Rev. A 2018, 98, 062316. [Google Scholar] [CrossRef]
- Forbes, A. Sculpting electric currents with structured light. Nat. Photonics 2020, 14, 656–657. [Google Scholar] [CrossRef]
- Cao, L.Y.; Zhang, M.M.; Dou, J.T.; Zhao, J.; Hu, Y.Y.; Li, B. Controlled generation of order-switchable cylindrical vector beams from a Nd: YAG laser. Chin. Opt. Lett. 2023, 21, 101401. [Google Scholar] [CrossRef]
- da Silva, N.R.; de Oliveira, A.G.; Arruda, M.F.Z.; de Araújo, R.M.; Soares, W.; Walborn, S.; Gomes, R.; Ribeiro, P.S. Stimulated Parametric Down-Conversion with Vector Vortex Beams. Phys. Rev. Appl. 2021, 15, 024039. [Google Scholar] [CrossRef]
- Arnold, S.F.; Barnett, S.M.; Yao, E.; Leach, J.; Courtial, J.; Padgett, M. Uncertainty principle for angular position and angular momentum. New J. Phys. 2004, 6, 103. [Google Scholar] [CrossRef]
- Sun, X.; Liu, L.; Zhu, Q.; Huang, W.; Zhang, Y.; Wang, W.; Chen, Y.; Geng, Y. Unitary transformation in polarization of vector beams via biaxial cascade crystals. J. Opt. 2020, 22, 025602. [Google Scholar] [CrossRef]
- Wang, W.; Zhou, L.J.; Dou, J.T.; Li, B.; Hu, Y.Y. Controlled generation of OAM-switchable vortex beams at 639 nm from a Pr3+: YLF laser. Opt. Commun. 2024, 563, 130576. [Google Scholar] [CrossRef]
- Wu, H.J.; Yu, B.S.; Zhu, Z.H.; Gao, W.; Ding, D.S.; Zhou, Z.Y.; Hu, X.P.; Rosales-Guzmán, C.; Shen, Y.; Shi, B.S. Conformal frequency conversion for arbitrary vectorial structured light. Optica 2022, 9, 187–196. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, Y.; Zhu, Z.; Wang, Y.; Dou, J.; Fan, L.; Li, B.; Hu, Y. Far-Field Topological Structure of the Second Harmonic from Higher-Order Poincaré Sphere Beam. Photonics 2025, 12, 407. https://doi.org/10.3390/photonics12050407
Li Y, Zhu Z, Wang Y, Dou J, Fan L, Li B, Hu Y. Far-Field Topological Structure of the Second Harmonic from Higher-Order Poincaré Sphere Beam. Photonics. 2025; 12(5):407. https://doi.org/10.3390/photonics12050407
Chicago/Turabian StyleLi, Yangyang, Ziping Zhu, Yuanxiang Wang, Jiantai Dou, Li Fan, Bo Li, and Youyou Hu. 2025. "Far-Field Topological Structure of the Second Harmonic from Higher-Order Poincaré Sphere Beam" Photonics 12, no. 5: 407. https://doi.org/10.3390/photonics12050407
APA StyleLi, Y., Zhu, Z., Wang, Y., Dou, J., Fan, L., Li, B., & Hu, Y. (2025). Far-Field Topological Structure of the Second Harmonic from Higher-Order Poincaré Sphere Beam. Photonics, 12(5), 407. https://doi.org/10.3390/photonics12050407




