Assessment of a New Trifocal Diffractive Corneal Inlay for Presbyopia Correction Using an Adaptive Optics Visual Simulator
Abstract
:1. Introduction
2. Materials and Methods
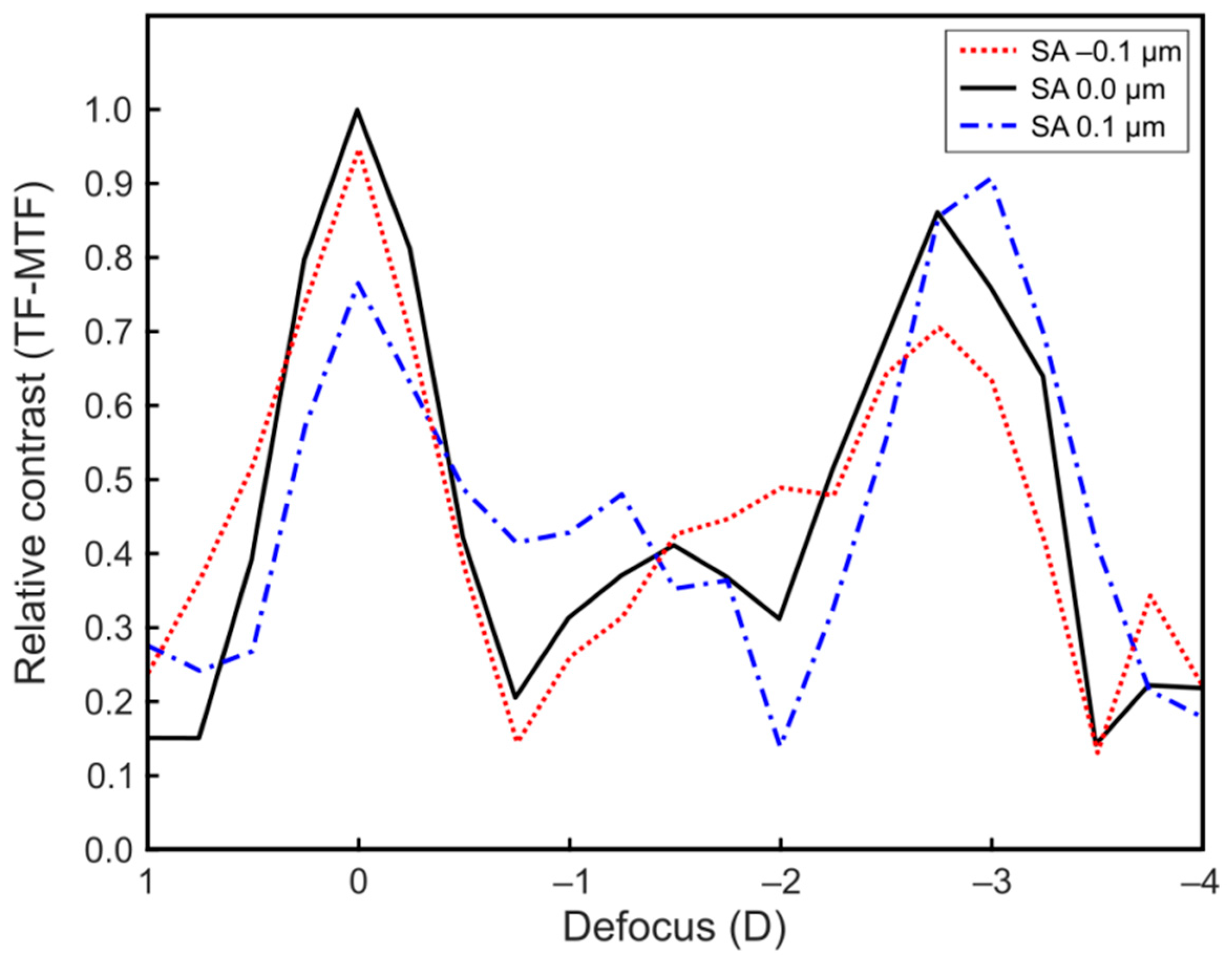
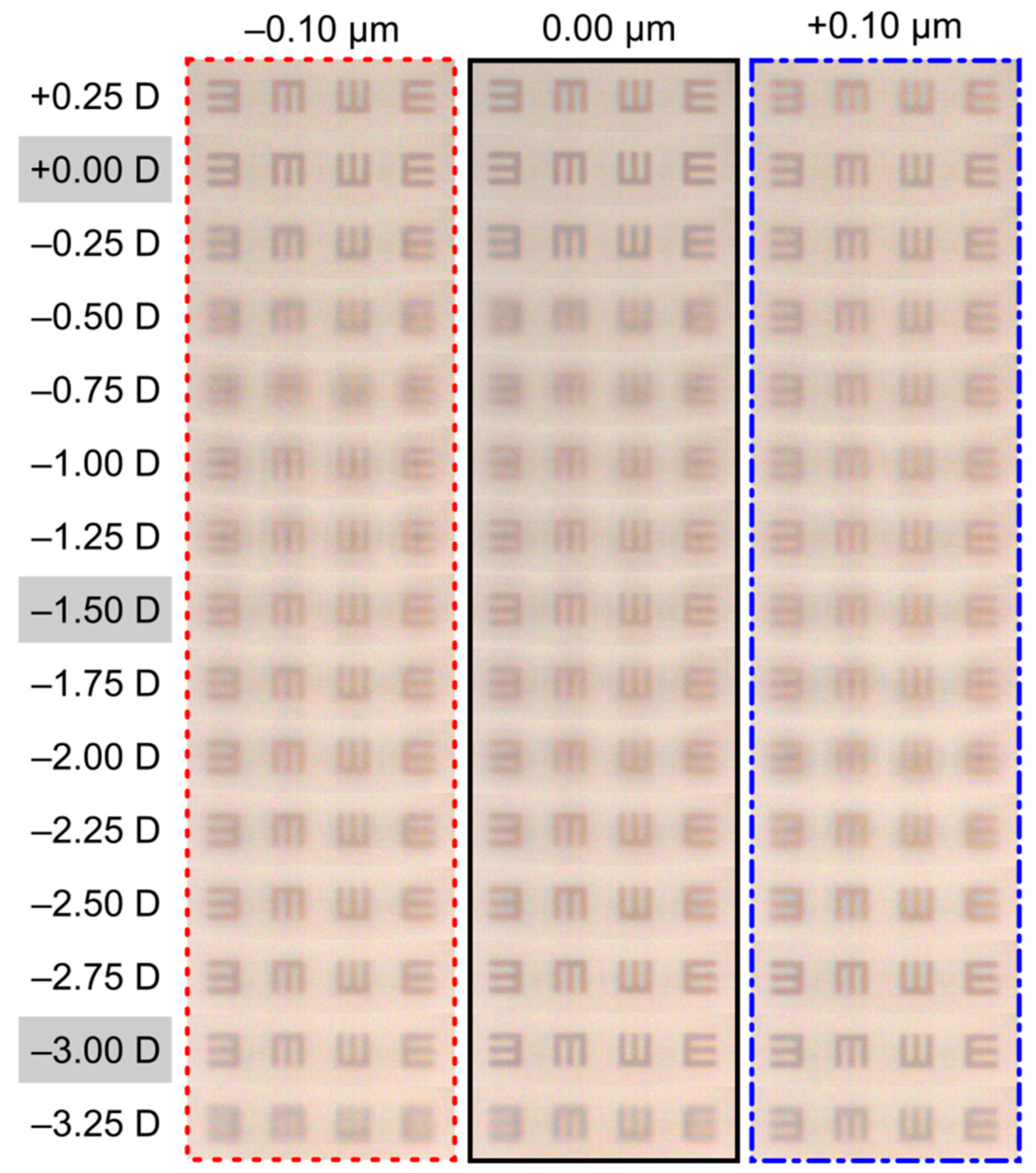
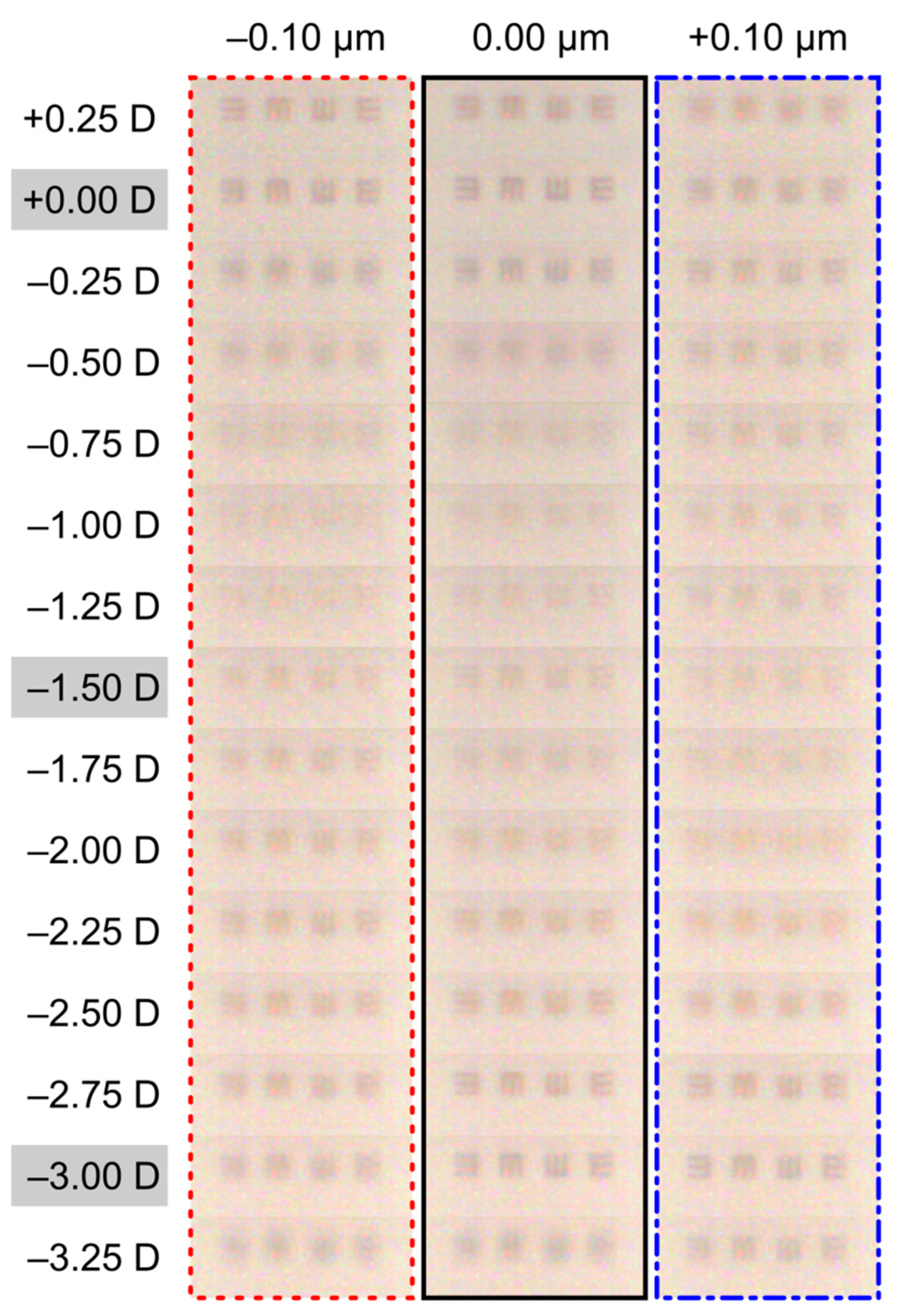
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Fricke, T.R.; Tahhan, N.; Resnikoff, S.; Papas, E.; Burnett, A.; Ho, S.M.; Naduvilath, T.; Naidoo, K.S. Global prevalence of presbyopia and vision impairment from uncorrected presbyopia: Systematic review, meta-analysis, and modelling. Ophthalmology 2018, 125, 1492–1499. [Google Scholar] [CrossRef] [PubMed]
- Charman, W.N. Developments in the correction of presbyopia I: Spectacle and contact lenses. Ophthalmic Physiol. Opt. 2014, 34, 8–29. [Google Scholar] [CrossRef] [PubMed]
- Charman, W.N. Developments in the correction of presbyopia II: Surgical approaches. Ophthalmic Physiol. Opt. 2014, 34, 397–426. [Google Scholar] [CrossRef] [PubMed]
- Bababekova, Y.; Rosenfield, M.; Hue, J.E.; Huang, R.R. Font size and viewing distance of handheld smart phones. Optom. Vis. Sci. 2011, 88, 795–797. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wang, C.; Wang, X.; Jin, L.; Tang, B.; Zhu, W.; Zhang, G.; Chen, T.; McAneney, H.; Kassalow, J.; Congdon, N. Influence of presbyopia on smartphone usage among Chinese adults: A population study. Clin. Exp. Ophthalmol. 2019, 47, 909–917. [Google Scholar] [CrossRef]
- Wolffsohn, J.S.; Davies, L.N. Presbyopia: Effectiveness of correction strategies. Prog. Retin. Eye Res. 2019, 68, 124–143. [Google Scholar] [CrossRef] [Green Version]
- Charman, W.N. Non-surgical treatment options for presbyopia. Expert Rev. Ophthalmol. 2018, 13, 219–231. [Google Scholar] [CrossRef]
- Hossain, P.; Barbara, R. The future of refractive surgery: Presbyopia treatment, can we dispense with our glasses? Eye 2021, 35, 359–361. [Google Scholar] [CrossRef]
- Lindstrom, R.L.; Macrae, S.M.; Pepose, J.S.; Hoopes, P.C. Corneal inlays for presbyopia correction. Curr. Opin. Ophthalmol. 2013, 24, 281–287. [Google Scholar] [CrossRef]
- Moarefi, M.A.; Bafna, S.; Wiley, W. A review of presbyopia treatment with corneal inlays. Opthamol. Ther. 2017, 6, 55–65. [Google Scholar] [CrossRef] [Green Version]
- Beer, S.M.C.; Werner, L.; Nakano, E.M.; Santos, R.T.; Hirai, F.; Nitschke, E.J.; Francesconi Benicio, C.; Campos, M.S. A 3-year follow-up study of a new corneal inlay: Clinical results and outcomes. Br. J. Ophthalmol. 2020, 104, 723–728. [Google Scholar] [CrossRef] [PubMed]
- Limnopoulou, A.N.; Bouzoukis, D.I.; Kymionis, G.D.; Panagopoulou, S.I.; Plainis, S.; Pallikaris, A.I.; Feingold, V.; Pallikaris, I.G. Visual outcomes and safety of a refractive corneal inlay for presbyopia using femtosecond laser. J. Refract. Surg. 2013, 29, 12–19. [Google Scholar] [CrossRef] [PubMed]
- Malandrini, A.; Martone, G.; Menabuoni, L.; Catanese, A.M.; Tosi, G.M.; Balestrazzi, A.; Corsani, C.; Fantozzi, M. Bifocal refractive corneal inlay implantation to improve near vision in emmetropic presbyopic patients. J. Cataract Refract. Surg. 2015, 41, 1962–1972. [Google Scholar] [CrossRef] [PubMed]
- Garza, E.B.; Gomez, S.; Chayet, A.; Dishler, J. One-year safety and efficacy results of a hydrogel inlay to improve near vision in patients with emmetropic presbyopia. J. Refract. Surg. 2013, 29, 166–172. [Google Scholar] [CrossRef] [Green Version]
- Waring, G.O. Correction of presbyopia with a small aperture corneal inlay. J. Refract. Surg. 2011, 27, 842–845. [Google Scholar] [CrossRef]
- Vilupuru, S.; Lin, L.; Pepose, J.S. Comparison of contrast sensitivity and through focus in small-aperture inlay, accommodating intraocular lens, or multifocal intraocular lens subjects. Am. J. Ophthalmol. 2015, 160, 150–162. [Google Scholar] [CrossRef]
- Vukich, J.A.; Durrie, D.S.; Pepose, J.S.; Thompson, V.; van de Pol, C.; Lin, L. Evaluation of the small-aperture intracorneal inlay: Three-year results from the cohort of the US Food and Drug Administration clinical trial. J. Cataract Refract. Surg. 2018, 44, 541–556. [Google Scholar] [CrossRef] [PubMed]
- Seyeddain, O.; Bachernegg, A.; Riha, W.; Rückl, T.; Reitsamer, H.; Grabner, G.; Dexl, A.K. Femtosecond laser–assisted small-aperture corneal inlay implantation for corneal compensation of presbyopia: Two-year follow-up. J. Cataract Refract. Surg. 2013, 39, 234–241. [Google Scholar] [CrossRef]
- Tabernero, J.; Schwarz, C.; Fernández, E.J.; Artal, P. Binocular visual simulation of a corneal inlay to increase depth of focus. Investig. Ophthalmol. Vis. Sci. 2011, 52, 5273–5277. [Google Scholar] [CrossRef]
- Plainis, S.; Petratou, D.; Giannakopoulou, T.; Radhakrishnan, H.; Pallikaris, I.G.; Charman, W.N. Small-aperture monovision and the Pulfrich experience: Absence of neural adaptation effects. PLoS ONE 2013, 8, e75987. [Google Scholar] [CrossRef] [Green Version]
- Furlan, W.D.; García-Delpech, S.; Udaondo, P.; Remón, L.; Ferrando, V.; Monsoriu, J.A. Diffractive corneal inlay for presbyopia. J. Biophotonics 2017, 10, 1110–1114. [Google Scholar] [CrossRef] [PubMed]
- Kipp, L.; Skibowski, M.; Johnson, R.L.; Berndt, R.; Adelung, R.; Harm, S.; Seemann, R. Sharper images by focusing soft X-rays with photon sieves. Nature 2001, 414, 184–188. [Google Scholar] [CrossRef] [PubMed]
- Montagud-Martínez, D.; Ferrando, V.; Monsoriu, J.A.; Furlan, W.D. Proposal of a new diffractive corneal inlay to improve near vision in a presbyopic eye. Appl. Opt. 2020, 59, D54–D58. [Google Scholar] [CrossRef] [PubMed]
- Furlan, W.D.; Montagud-Martínez, D.; Ferrando, V.; García-Delpech, S.; Monsoriu, J.A. A new trifocal corneal inlay for presbyopia. Sci. Rep. 2021, 11, 6620. [Google Scholar] [CrossRef]
- Leray, B.; Cassagne, M.; Soler, V.; Villegas, E.A.; Triozon, C.; Perez, G.M.; Letsch, J.; Chapotot, E.; Artal, P.; Malecaze, F. Relationship between induced spherical aberration and depth of focus after hyperopic LASIK in presbyopic patients. Ophthalmology 2015, 122, 233–243. [Google Scholar] [CrossRef]
- Liou, H.-L.; Brennan, N.A. Anatomically accurate, finite model eye for optical modeling. J. Opt. Soc. Am. A 1997, 14, 1684–1695. [Google Scholar] [CrossRef]
- VAO. Voptica. Available online: https://voptica.com/vao/ (accessed on 29 September 2021).
- Manzanera, S.; Prieto, P.M.; Ayala, D.B.; Lindacher, J.M.; Artal, P. Liquid crystal Adaptive Optics Visual Simulator: Application to testing and design of ophthalmic optical elements. Opt. Express 2007, 15, 16177–16188. [Google Scholar] [CrossRef]
- Hervella, L.; Villegas, E.A.; Robles, C.; Artal, P. Spherical aberration customization to extend the depth of focus with a clinical adaptive optics visual simulator. J. Refract. Surg. 2020, 36, 223–229. [Google Scholar] [CrossRef]
- Fernández, E.J.; Prieto, P.M.; Artal, P. Wave-aberration control with a liquid crystal on silicon (LCOS) spatial phase modulator. Opt. Express 2009, 17, 11013–11025. [Google Scholar] [CrossRef]
- Calatayud, A.; Remón, L.; Martos, J.; Furlan, W.D.; Monsoriu, J.A. Imaging quality of multifocal intraocular lenses: Automated assessment setup. Ophthal. Physiol. Opt. 2013, 33, 420–426. [Google Scholar] [CrossRef]
- Rahmania, N.; Salah, I.; Rampat, R.; Gatinel, D. Clinical Effectiveness of Laser-Induced Increased Depth of Field for the Simultaneous Correction of Hyperopia and Presbyopia. J. Refract. Surg. 2021, 37, 16–24. [Google Scholar] [CrossRef] [PubMed]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Martínez-Espert, A.; Montagud-Martínez, D.; Ferrando, V.; Furlan, W.D.; Monsoriu, J.A. Assessment of a New Trifocal Diffractive Corneal Inlay for Presbyopia Correction Using an Adaptive Optics Visual Simulator. Photonics 2022, 9, 135. https://doi.org/10.3390/photonics9030135
Martínez-Espert A, Montagud-Martínez D, Ferrando V, Furlan WD, Monsoriu JA. Assessment of a New Trifocal Diffractive Corneal Inlay for Presbyopia Correction Using an Adaptive Optics Visual Simulator. Photonics. 2022; 9(3):135. https://doi.org/10.3390/photonics9030135
Chicago/Turabian StyleMartínez-Espert, Anabel, Diego Montagud-Martínez, Vicente Ferrando, Walter D. Furlan, and Juan A. Monsoriu. 2022. "Assessment of a New Trifocal Diffractive Corneal Inlay for Presbyopia Correction Using an Adaptive Optics Visual Simulator" Photonics 9, no. 3: 135. https://doi.org/10.3390/photonics9030135
APA StyleMartínez-Espert, A., Montagud-Martínez, D., Ferrando, V., Furlan, W. D., & Monsoriu, J. A. (2022). Assessment of a New Trifocal Diffractive Corneal Inlay for Presbyopia Correction Using an Adaptive Optics Visual Simulator. Photonics, 9(3), 135. https://doi.org/10.3390/photonics9030135





