Composition-Dependent Phonon and Thermodynamic Characteristics of C-Based X_xY_1−xC (X, Y ≡ Si, Ge, Sn) Alloys

Abstract

Novel zinc-blende (zb) group-IV binary XC and ternary X_xY_1−xC alloys (X, Y ≡ Si, Ge, and Sn) have recently gained scientific and technological interest as promising alternatives to silicon for high-temperature, high-power optoelectronics, gas sensing and photovoltaic applications. Despite numerous efforts made to simulate the structural, electronic, and dynamical properties of binary materials, no vibrational and/or thermodynamic studies exist for the ternary alloys. By adopting a realistic rigid-ion-model (RIM), we have reported methodical calculations to comprehend the lattice dynamics and thermodynamic traits of both binary and ternary compounds. With appropriate interatomic force constants (IFCs) of XC at ambient pressure, the study of phonon dispersions ${\omega }_j\left(\overrightarrow{\mathit{q}}\right)$ offered positive values of acoustic modes in the entire Brillouin zone (BZ)—implying their structural stability. For X_xY_1−xC, we have used Green’s function (GF) theory in the virtual crystal approximation to calculate composition x, dependent ${\omega }_j\left(\overrightarrow{\mathit{q}}\right)$ and one phonon density of states $g\left(\omega \right)$. With no additional IFCs, the RIM GF approach has provided complete ${\omega }_j\left(\overrightarrow{\mathit{q}}\right)$ in the crystallographic directions for both optical and acoustical phonon branches. In quasi-harmonic approximation, the theory predicted thermodynamic characteristics (e.g., Debye temperature Θ_D(T) and specific heat C_v(T)) for X_xY_1−xC alloys. Unlike SiC, the GeC, SnC and Ge_xSn_1−xC materials have exhibited weak IFCs with low [high] values of Θ_D(T) [C_v(T)]. We feel that the latter materials may not be suitable as fuel-cladding layers in nuclear reactors and high-temperature applications. However, the XC and X_xY_1−xC can still be used to design multi-quantum well or superlattice-based micro-/nano devices for different strategic and civilian application needs.

1. Introduction

Since the invention of Si-based transistors [1], the evolution to include them in radio frequency (RF) devices for microelectronics has been remarkable [2,3,4,5,6,7,8,9,10,11]. With the low processing costs, Si-technology has led to many achievements by incorporating electrodes, dielectrics, and other elements in different integrated circuits (ICs). With truly monolithic optoelectronic functionality, Si has offered substantial cost benefits as well as long-term performance gains in the optoelectronic microsensor systems [12,13,14,15,16] and photovoltaic cells. These achievements have given tremendous opportunities for both the scientists and engineers to successfully incorporate different devices in energy harvesting and gas-sensing needs. Earlier accomplishments on RF devices and ICs have quickly grown to become valuable for wireless sensor networks (WSNs) [17,18]. These devices are now employed in a wide range of applications including photonics, optoelectronics, environment monitoring, medical diagnostics, forensic, spintronics, cellular base phone transceivers, amplifiers, Gigabit wireless local as well as personal area networks [12,13,14,15,16,17,18,19,20,21,22,23,24,25,26,27,28,29,30,31,32].

The progress in Si-based ICs and WSNs is the outcome of many scientific research reports published on different topics where materials’ perspectives played increasingly important roles. New wavelength regimes are now extended from 1.55 μm to 5.0 μm for the operations of Si-based photonic and opto-electronic ICs [10]. While many multiple-quantum-wells (MQWs) and superlattices (SLs) based III-V semiconductors [19,20,21,22] have revealed extremely inspiring results, the high cost and incompatibility of III-Vs with the Si platform has been, and still is, the main impediment that prevented the large-scale commercial production of different opto-electronic devices [23,24,25,26,27,28,29,30,31,32,33,34,35,36,37,38].

Despite the technological achievements of group-IV materials, the IV-IV binary XC and ternary X_xY_1−xC alloys with X, Y (≡Si, Ge, and Sn) have recently become quite attractive in preparing different heterostructures for bandgap and strain engineering [29,30,31,32]. At room temperature (RT), the C, Si, Ge, and α-Sn of diamond crystal structures are known to exhibit indirect band gap energies $E_g$ [≡5.47 eV, 1.12 eV, 0.66 eV and 0 eV]. Higher mismatch between the lattice constants, $a_o$ [≡C: 3.56 Å, Si: 5.43 Å, Ge: 5.66 Å, α-Sn: 6.49 Å] and low solubility of C has caused complications in the earlier designs of XC/Si MQWs and SLs [33,34,35,36,37,38,39,40,41,42,43,44,45]. However, the differences in their electronegativity now play a vital role in carefully optimizing parameters to achieve epitaxial growth of heterostructures apposite for diverse device applications. Recently, the use of Si/SiGe has gained considerable attention for designing heterojunction bipolar transistors with cut-off frequencies > 10 GHz [11].

Tremendous efforts have also been formulated in preparing novel C-based binary XC and/or ternary X_xY_1−xC alloys by taking advantage of the unique and exciting properties of group-IV materials [12,13,14,15,16,17,18,19,20,21,22,23,24,25,26,27,28,29,30,31,32,33,34,35,36,37]. Good structural stability of zinc-blende (zb) binary and/or ternary compounds with different $E_g$, hardness, high stiffness, melting point, and high thermal conductivity is considered particularly favorable for applications [38,39,40,41] in blue/ultraviolet (UV) light-emitting diodes (LEDs), laser diodes (LDs), photodetectors and solar cells [12,13,14,15,16], etc. Due to large lattice mismatch and the differences in thermal expansion coefficients between X_xY_1−xC epilayers and Si substrate, one would expect the possibilities of observing structural and/or intrinsic defects near the interfaces [29]. However, appropriate use of buffer layers acquiring load through the relaxation of mechanical stresses has helped improve the structural qualities of MQWs and SLs. While there remain a few intrinsic issues, which could constrain the design of opto-electronic device structures, solutions to these problems are not impossible and can be resolved by exploiting suitable experimental (e.g., growth, [42,43,44,45,46,47,48,49,50,51,52,53,54,55,56,57,58,59,60,61,62,63,64,65,66,67,68,69,70,71,72,73,74,75,76,77,78,79,80,81,82,83,84,85,86,87,88,89,90,91,92,93] characterization [94,95,96,97,98,99,100,101,102,103,104,105,106,107]) and theoretical [108,109,110,111,112,113,114,115,116,117,118,119,120,121,122,123,124,125,126,127,128,129,130,131,132,133,134,135,136,137] methods.

One must note that by employing the pulsed supersonic free jets techniques [42,43,44,45], an inverse heteroepitaxial growth of Si on SiC has been demonstrated to achieve multilayer structures [46,47,48,49,50,51,52,53,54,55,56,57,58,59,60,61,62,63,64,65]. A novel arc plasma C gun source is incorporated in the molecular beam epitaxial (MBE) methods to grow ultrathin MQWs and SLs [66,67,68,69,70]. Ultrahigh chemical vapor deposition (UH-CVD), reduced pressure RP-CVD and metalorganic (MOCVD) techniques are also successfully used [71,72,73,74,75,76,77,78,79,80,81,82,83,84,85,86,87,88,89,90,91,92,93] to prepare different Si_1−xGe_xC/Si, Ge_1−xSn_xC/Si, GeC/SiC epilayers. For commercial applications of these materials, the RP-CVD method has been preferred due to the balance between good epitaxial quality and relatively high growth rates [76]. Certainly, the progress made in the growth of complex and exotic C-based materials has set challenges for both the physicists and engineers in investigating their fundamental properties.

Although binary compounds are used in many technological applications, considerably less attention is paid to ternary alloys despite the successful growth of ultrathin epifilms. A variety of characterization techniques are also applied for analyzing/ monitoring their fundamental properties [94,95,96,97,98,99,100,101,102,103,104,105,106,107]. The classification of such methods includes reflection high-energy electron diffraction (RHEED) [94,95], Auger electron spectroscopy (AES) [96], He⁺ Rutherford backscattering spectrometry (RBS) [97], atomic force microscopy (AFM) [98,99], high-resolution X-ray diffraction (HR-XRD) [100,101,102], transmission electron microscopy (XTEM) [103], photoluminescence (PL) [104], absorption, Fourier transform infrared (FTIR) spectroscopy [100,101,102], Raman scattering spectroscopy (RSS) [105,106,107] and spectroscopic ellipsometry (SE) techniques [107], etc. It is to be noted that not only have these techniques validated their crystal structures but also helped in assessing the film thickness, strain, intrinsic electrical, and optical traits. Despite the existence of numerous experimental studies on structural and electrical properties, there are limited IR absorption and RSS measurements [100,101,102,103,104,105,106,107] for evaluating their phonon characteristics.

From a theoretical standpoint, several calculations are performed to understand the structural, electronic, and optical properties of the binary compounds using full potential linear augmented plane wave (FP-LAPW), first-principles (ab initio) and molecular dynamical (MD) methods [108,109,110,111,112,113,114,115,116,117,118,119,120,121,122,123,124,125,126]. For the ternary X_xY_1−xC alloys, however, no systematic studies are known for comprehending their lattice dynamical and/or thermodynamic characteristics. To accomplish major technological applications of the C-based heterostructures [e.g., Si/Si(Ge)C, GeSnC/GeC MQWs and (SiC)_m/(Ge(Sn)C)_n SLs], such calculations are necessary to obtain the complete phonon dispersions of XC and X_xY_1−xC materials from realistic lattice dynamical models. One reason for this requisite is that the dynamical response of polar lattices affects the key electronic properties, including the exciton-binding energies and charge-carrier mobilities. Examining the dynamical response of crystals and its impact on the dielectric environment provides a major step in realizing their structural characteristics. The other reason for its need is that, in MQWs and SLs, the phonon density of states (DOS) of binary/ternary alloys has played crucial roles in evaluating thermodynamic traits, including the thermal expansion coefficients α(T), Debye temperature Θ_D(T), heat capacity C_v(T), Grüneisen constants γ(T), entropy, lattice thermal conductivity, etc.

The purpose of this work is to use a realistic rigid-ion model (RIM) [127] and report the methodical results of our comprehensive study to assess the structural, lattice dynamical (cf. Section 2.1), and thermodynamic (cf. Section 2.2) characteristics of binary XC and ternary (cf. Section 2.3) X_1−xY_xC alloys. For the tetrahedral (${\mathrm{T}}_{\mathrm{d}}^2$: $\mathrm{F}\overline{4}3\mathrm{m}$) binary materials, we have carefully optimized the RIM interatomic force constants (IFCs) by exercising [128] (cf. Section 2.1.1) successive least-square fitting procedures. In these processes, the phonon frequencies at high critical points (Γ, X, and L) are used as input while exploiting their lattice—$a_o$, and elastic constants ${\mathrm{C}}_{\mathrm{i}\mathrm{j}}$ as constraints (see

Table 1. Different parameters for XC (X ≡ Si, Ge and Sn) materials, viz., lattice constants $a_0 (\mathrm{i}\mathrm{n} \AA )$; elastic constants ${\mathrm{c}}_{\mathrm{i}\mathrm{j}}\left(\mathrm{i}\mathrm{n} {10}^{11}\frac{\mathrm{d}\mathrm{y}\mathrm{n}}{{\mathrm{c}\mathrm{m}}^2}\right)$ and phonon frequencies (cm⁻¹) at Γ, X, and L critical points. These are required for evaluating the necessary interatomic force constants of the rigid-ion model (see: text).

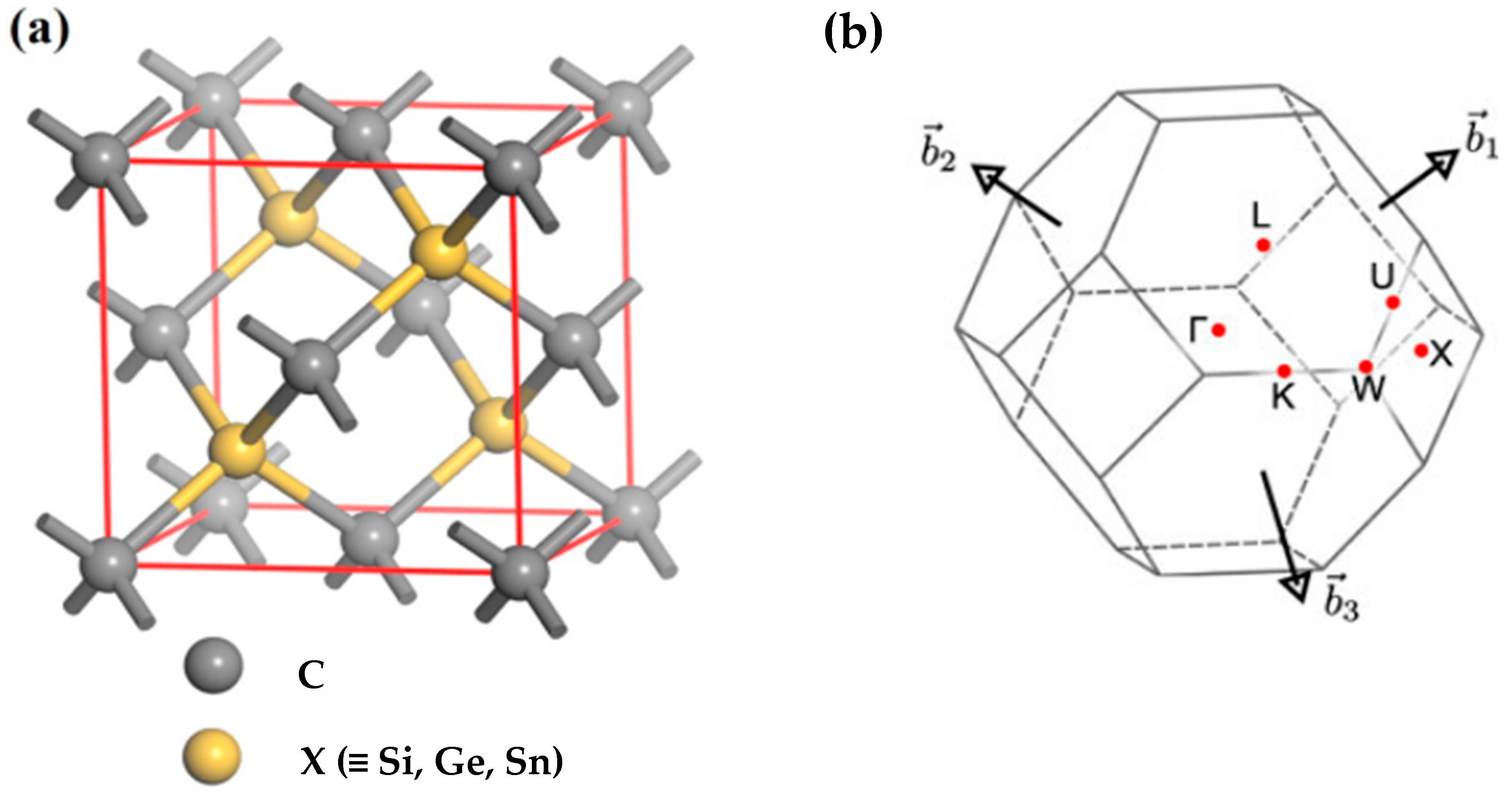
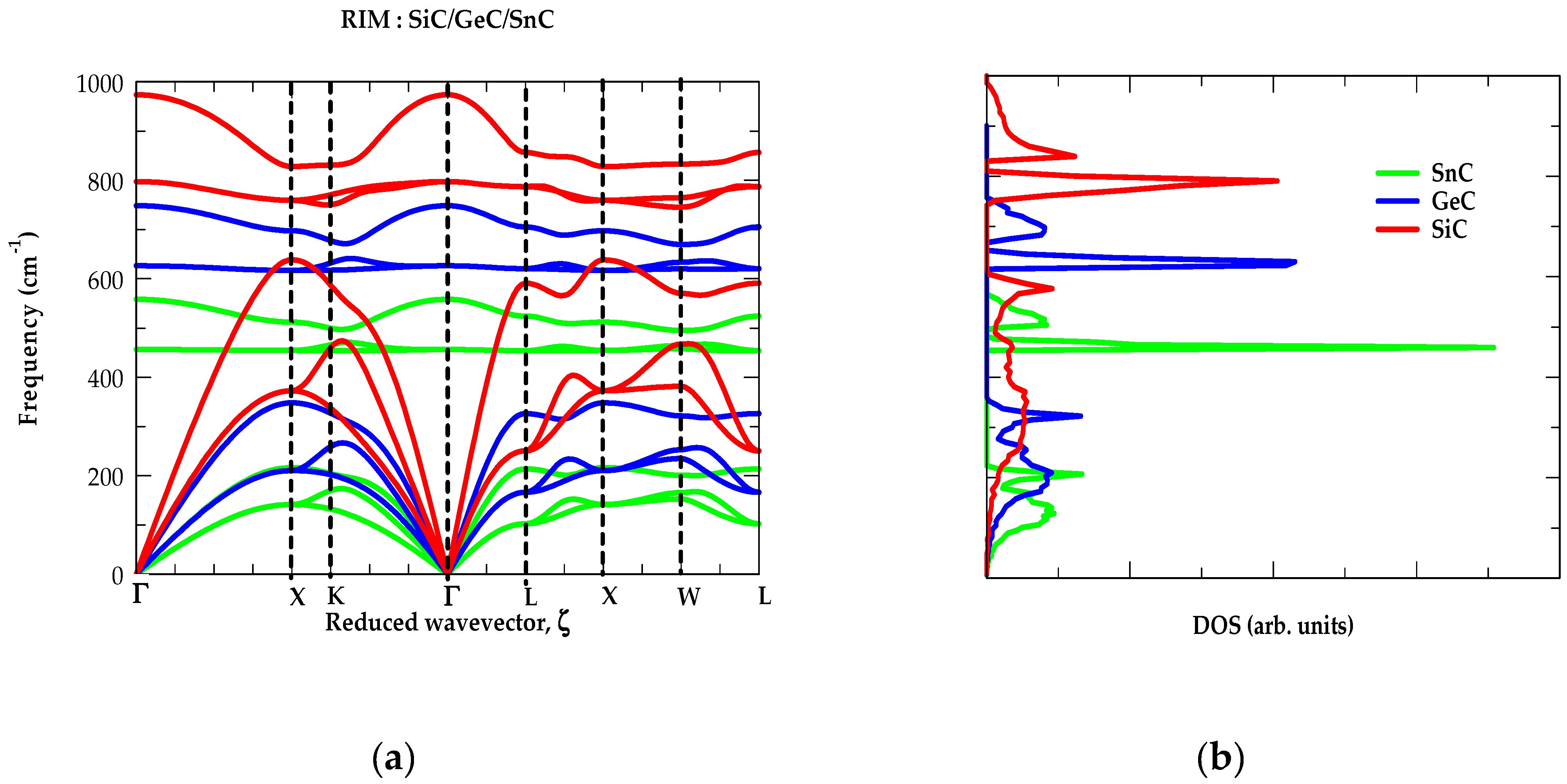
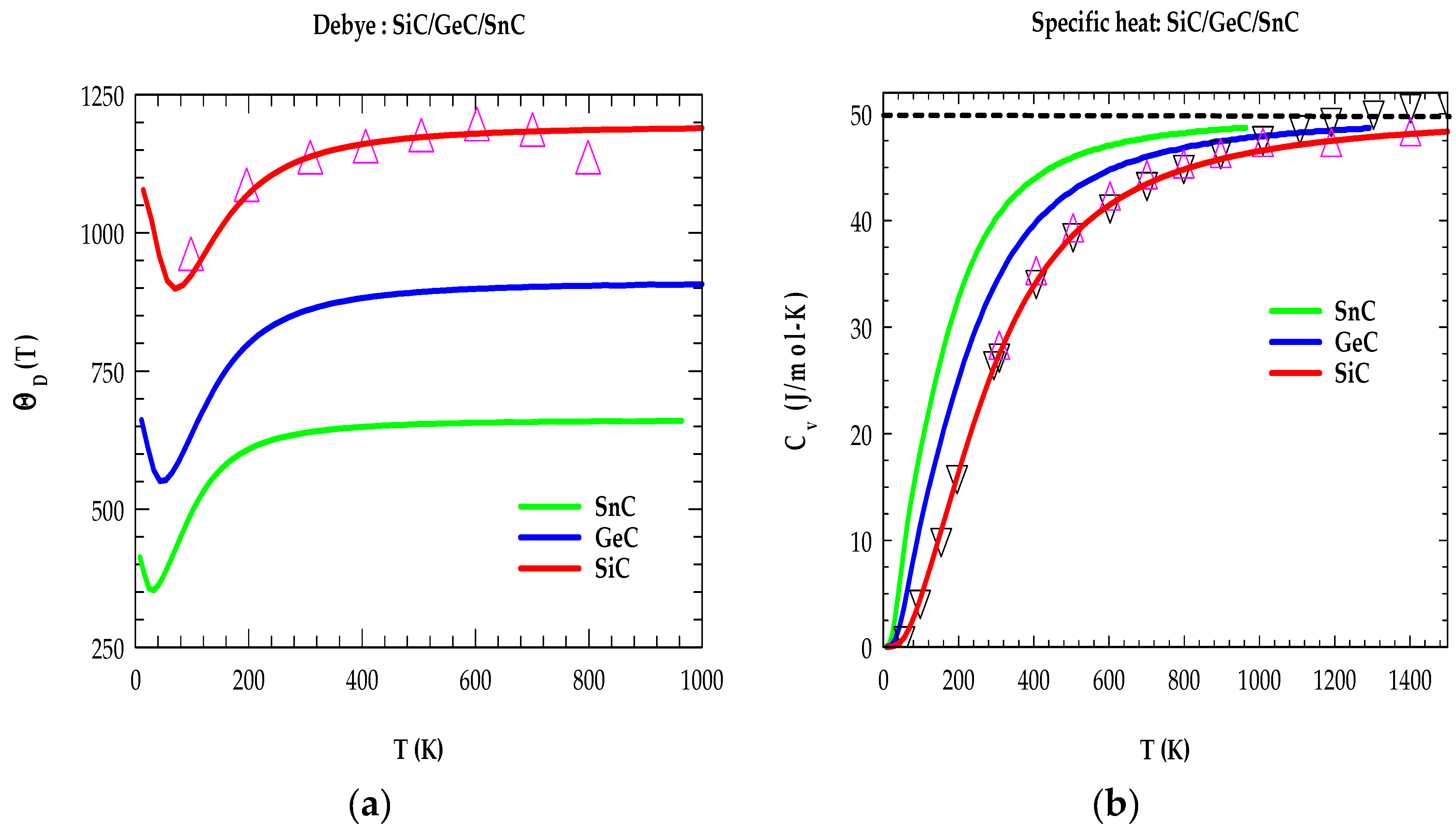
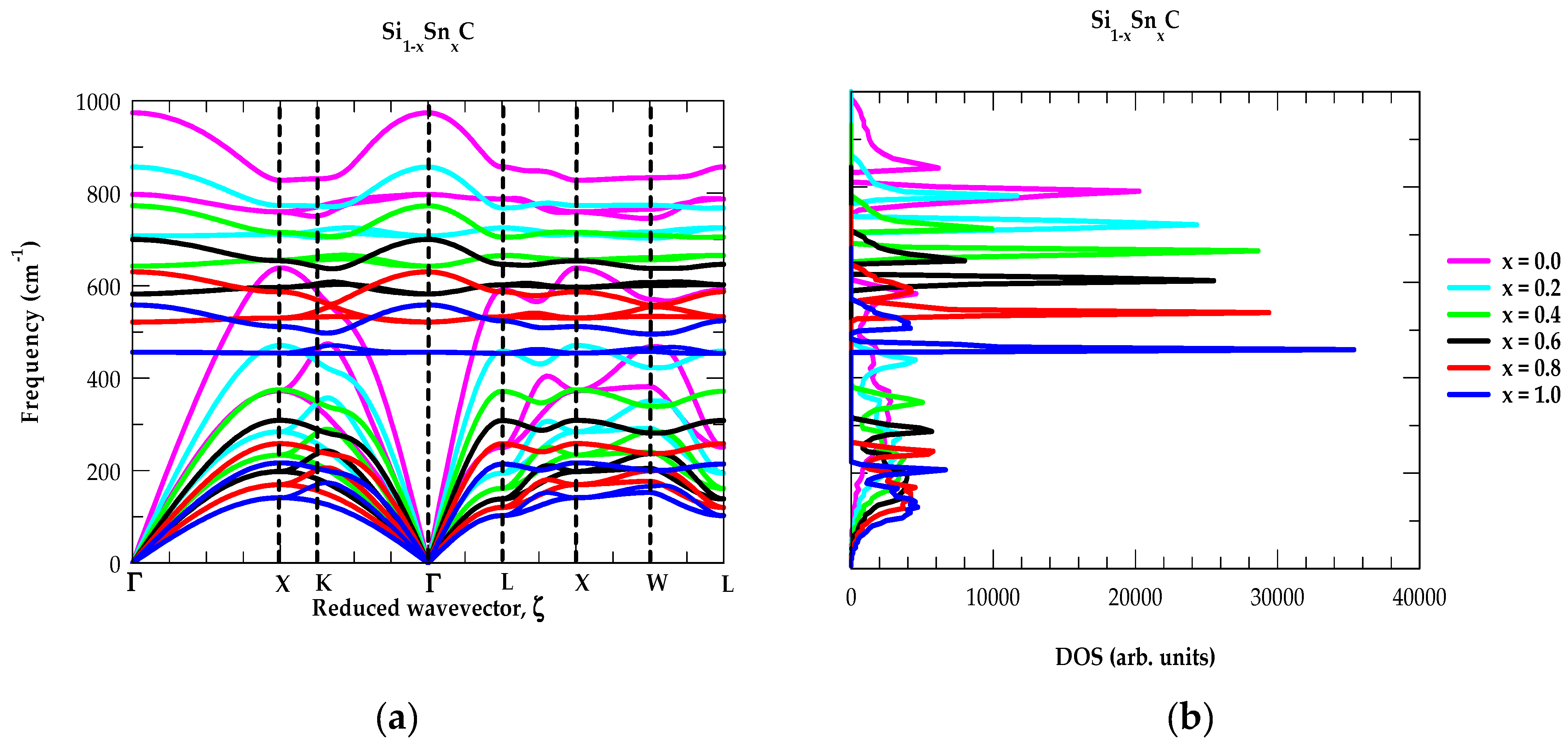
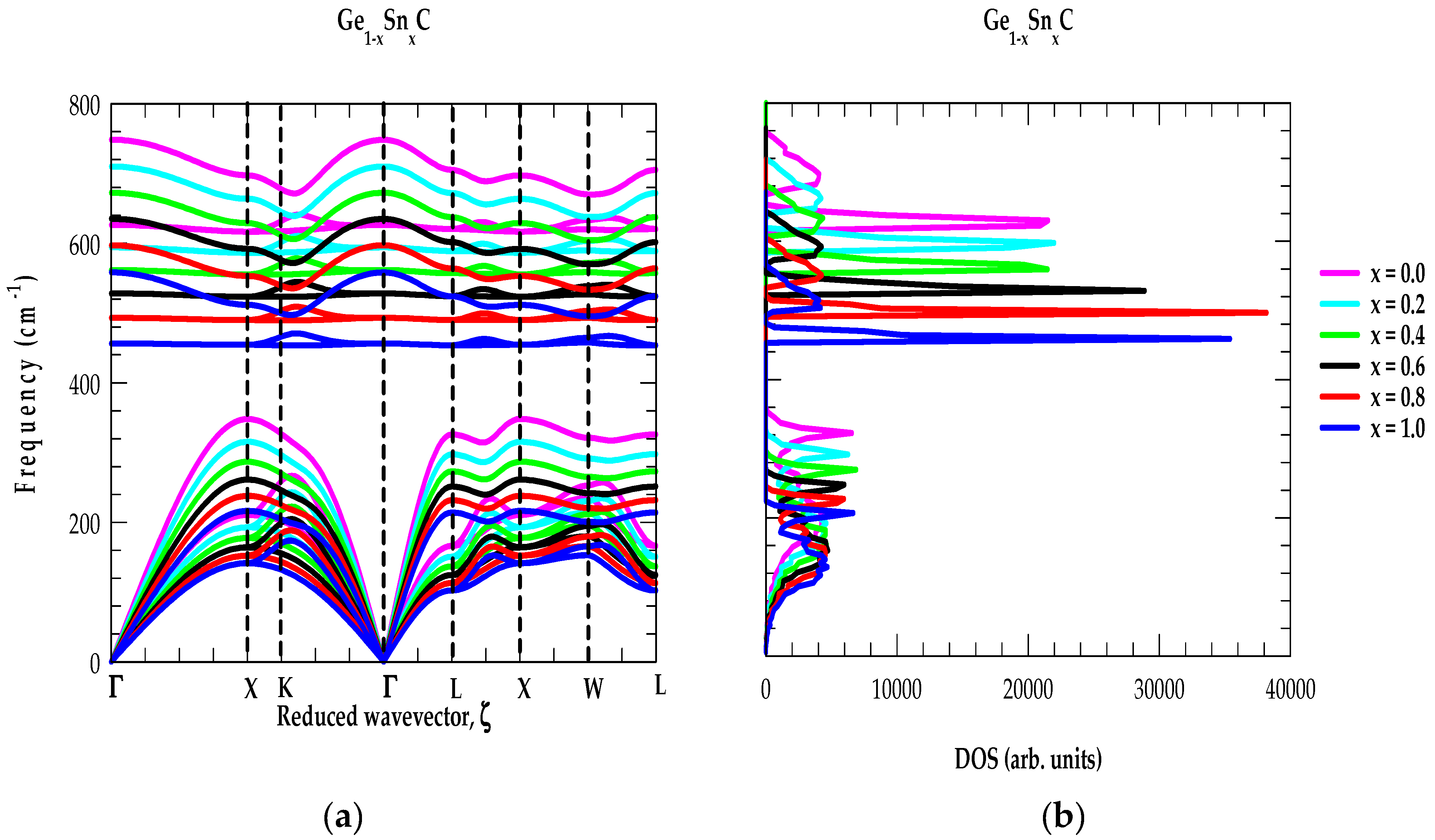
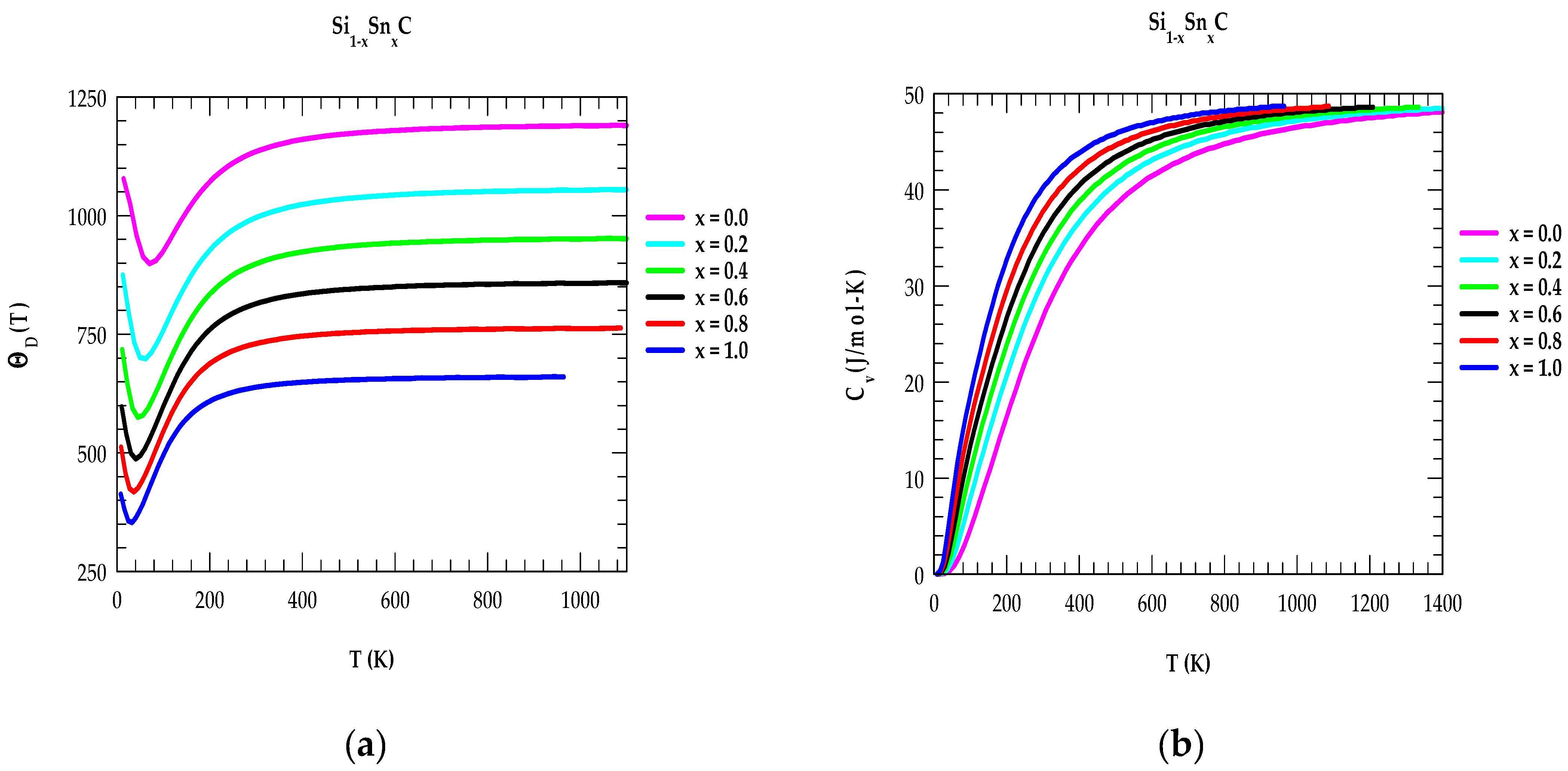
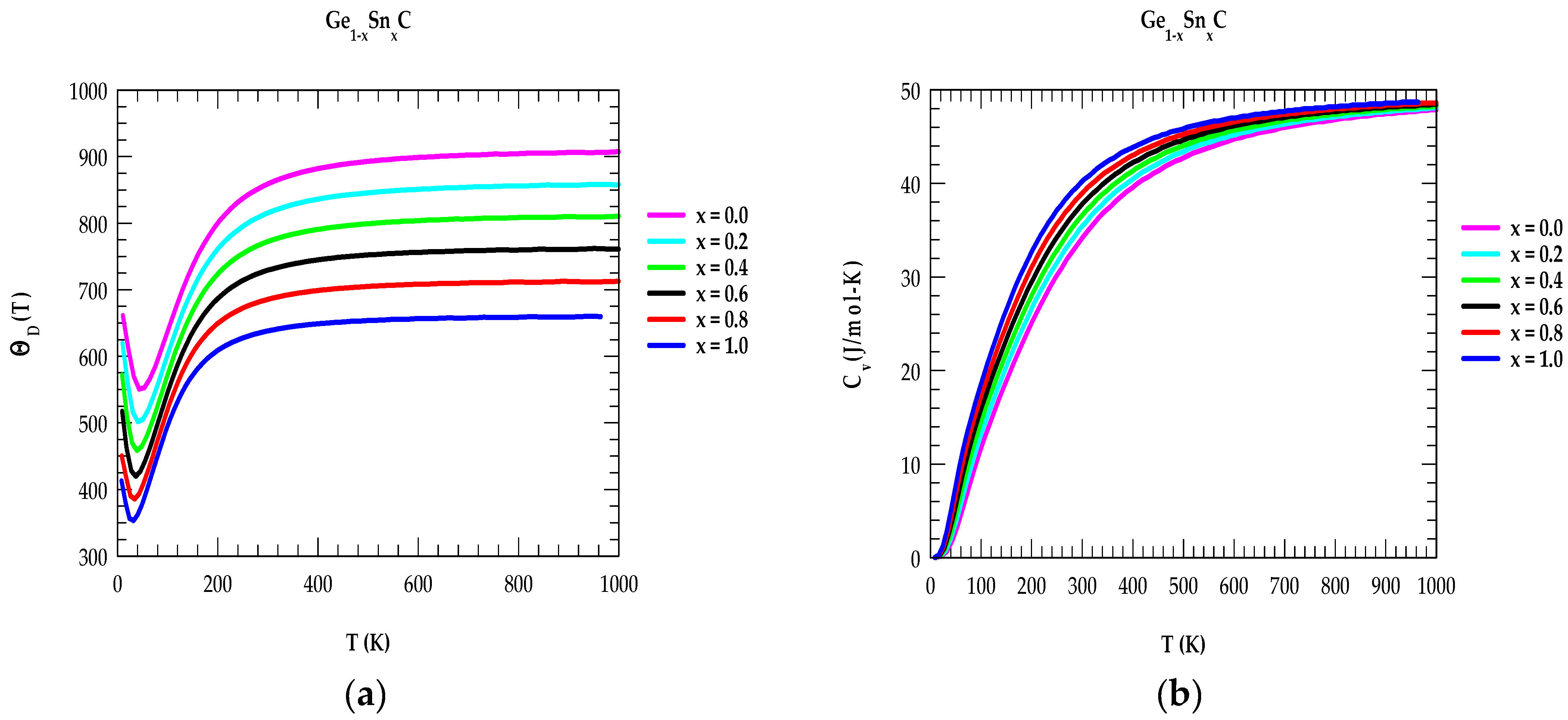
Parameters	3C-SiC	zb-GeC	zb-SnC
${\mathit{a}}_{\mathbf{0}}$ ($\mathrm{\AA }$)	4.360 (a), 4.40 (b), 4.374 (c)	4.610 (d), 4.590 (e)	5.130 (f), 5.170 (h)
${\mathbf{c}}_{\mathbf{11}}$	38.0 (a), 38.3 (b), 38.5 (c)	29.7 (f), 35.8 (g)	24.6 (g)
${\mathbf{c}}_{\mathbf{12}}$	14.2 (a), 12.5 (b), 12.2 (c)	12.4 (f), 12.2 (g)	11.3 (g)
${\mathbf{c}}_{\mathbf{44}}$	25.6 (a), 24.0 (b), 24.3 (c)	14.1 (f), 21.4 (g)	14.3 (g)
${\mathsf{\omega }}_{\mathbf{L}\mathbf{O}\left(\mathrm{\Gamma }\right)}$ ${\mathsf{\omega }}_{\mathbf{T}\mathbf{O}\left(\mathrm{\Gamma }\right)}$	974 (b) 793 (b)	748 (h) 626 (h)	558 (h) 456 (h)
${\mathsf{\omega }}_{\mathbf{L}\mathbf{O}\left(\mathbf{X}\right)}$ ${\mathsf{\omega }}_{\mathbf{T}\mathbf{O}\left(\mathbf{X}\right)}$ ${\mathsf{\omega }}_{\mathbf{L}\mathbf{A}\left(\mathbf{X}\right)}$ ${\mathsf{\omega }}_{\mathbf{T}\mathbf{A}\left(\mathbf{X}\right)}$	830 (b) 759 (b) 644 (b) 373 (b)	697 (h) 617 (h) 348 (h) 214 (h)	503 (h) 450 (h) 216 (h) 134 (h)
${\mathsf{\omega }}_{\mathbf{L}\mathbf{O}\left(\mathbf{L}\right)}$ ${\mathsf{\omega }}_{\mathbf{T}\mathbf{O}\left(\mathbf{L}\right)}$ ${\mathsf{\omega }}_{\mathbf{L}\mathbf{A}\left(\mathbf{L}\right)}$ ${\mathsf{\omega }}_{\mathbf{T}\mathbf{A}\left(\mathbf{L}\right)}$	850 (b) 770 (b) 605 (b) 260 (b)	705 (h) 612 (h) 331 (h) 162 (h)	516 (h) 440 (h) 199 (h) 109 (h)

^(a) Ref. [130]; ^(b) Ref. [129]; ^(c) Refs. [112,113]; ^(d) Ref. [115]; ^(e) Ref. [116]; ^(f) Ref. [119]; ^(g) Ref. [124]; ^(h) Ref. [125].

). For zb-SiC, the values of phonon frequencies are incorporated from inelastic X-ray scattering (IXS) measurements [129], while in GeC, SnC, we employed critical-point phonon frequencies from first-principles ABINIT results using a plane–wave pseudopotential method in density functional theory (DFT) and local-density perturbation approximation [125]. With the appropriate IFCs of binary XC materials, the dynamical matrix (cf. Section 3 and Section 3.1) is diagonalized to obtain phonon dispersions ${\omega }_j\left(\overrightarrow{\mathit{q}}\right),$ at each wave vector $\overrightarrow{q}$ point in the Brillouin zone (BZ), as well as one phonon density of states (DOS), $g\left(\omega \right)$. For mixed X_xY_1−xC alloys 0 ≤ x ≤ 1, the composition-dependent phonon frequencies (cf. Section 3.2) are simulated by using a Green’s function (GF) theory [130] in the virtual crystal approximation (VCA). Without considering additional IFCs, the RIM GF approach for the mixed alloys has offered a complete $\overrightarrow{q}$ dependent ${\omega }_j\left(\overrightarrow{\mathit{q}}\right),$ in arbitrary crystallographic directions for both the optical and acoustical phonon branches. In X_xY_1−xC materials, and using a quasi-harmonic approximation (QHA), we have systematically simulated the x-dependent ${\omega }_j\left(\overrightarrow{\mathit{q}}\right),$ and $g\left(\omega \right)$ (cf. Section 3.2) to predict their thermodynamic characteristics (e.g., Debye temperature Θ_D(T) and specific heat C_v(T)). Born’s transverse effective charges $e_T^{*}$, and Fröhlich coupling parameters ${\alpha }_F$ are also (cf. Section 3.2.3 and Section 3.2.4) calculated. Theoretical results of ${\omega }_j\left(\overrightarrow{\mathit{q}}\right)$, $g\left(\omega \right),$ and the thermo-dynamical traits for both binary XC and ternary X_xY_1−xC alloys are carefully analyzed/contrasted (cf. Section 3.1) against the existing experimental [129] and theoretical [120,121,122,123,124,125,126] data (cf. Section 3) with concluding remarks presented in Section 4.

2. Computational Details

The vibrational properties of XC materials have played a valuable role in assessing their phase transitions, transport coefficients and other physical phenomena [130,131,132,133,134,135,136,137]. Understanding the electron–phonon interactions and polaron characteristics in polar compounds is important for evaluating their optical and transport properties [138]. In X_1−xY_xC alloys, the exploration of phonons and optical and structural characteristics is crucial for correlating them to their different microscopic traits. To the best of our knowledge, no experimental and/or theoretical studies of phonons exist for X_1−xY_xC alloys, especially ones examining the contributions of lattice dynamical properties to phonon-free energy, entropy, specific heat, and Debye temperatures, etc. Accurate RIM simulations of ${\omega }_j\left(\overrightarrow{q}\right),$ and $g\left(\omega \right)$ for the binary XC materials are employed (cf. Section 2.1) to comprehend the lattice dynamics and thermodynamic properties of X_1−xY_xC alloys using a GF methodology (cf. Section 2.2).

2.1. Phonons in XC Materials

Earlier, the IXS [129] and RSS results [105] of phonon dispersions ${\omega }_j\left(\overrightarrow{q}\right),$ for the zb 3C-SiC were analyzed by exploiting the ab initio methods [120,121,122,123,124,125,126]. Recent calculations of lattice dynamics for GeC and SnC using plane–wave pseudopotential DFT approaches in the local density approximation [124,125] have provided different results. By using a realistic RIM [127], we have probed the experimental and theoretical data [105,120,121,122,123,124,125,126,129] of phonon dispersions for binary XC (3C-SiC, GeC and SnC) materials. Our choice of RIM, briefly described in Section 2.1.1, is based on the following facts: (a) a truncated RIM [139,140,141,142,143,144], where the number of IFCs is limited, a priori, failed to give accurate ${\omega }_j\left(\overrightarrow{q}\right),$ and it is not even less complicated than the more realistic scheme [127] adopted here; and (b) with regard to ${\omega }_j\left(\overrightarrow{q}\right)$, our simulated results in the high-symmetry direction are comparable (cf. Section 3) with experimental (3C-SiC) [105,129] and ab initio methods (GeC, SnC) [120,121,122,123,124,125,126].

2.1.1. Rigid-Ion Model

Here, we have briefly outlined the RIM [127] to comprehend the vibrational and thermodynamic properties of perfect binary XC materials. In this approach, the atomic displacements $u_{\alpha }\left(l\kappa |\overrightarrow{q},j\right)$ of the jth vibrational modes ${\omega }_j\left(\overrightarrow{q}\right)$, are expressed as plane waves with the wave vector $\overrightarrow{q}$ as [127]:

$$u_{\alpha }\left(l\kappa |\overrightarrow{q},j\right)=\frac{1}{\sqrt{M_{\kappa }}}{e}_{\alpha }\left(\kappa |\overrightarrow{q},j\right)e^{i[\overrightarrow{q}\overrightarrow{x}\left(l\kappa \right)-{\omega }_j\left(\overrightarrow{q}\right)t]}$$

(1)

where t is the time; the $\overrightarrow{x}\left(l\kappa \right)$ and $M_{\kappa }$ are, respectively, the position and mass of the $\left(l\kappa \right)$ atom. The term $l$ represents the position and $\kappa$ denotes the types of atoms with $\kappa$ = 1, 2 to specify the C and X, respectively, in the XC materials (see: the crystal structure, in Figure 1a, and its BZ in Figure 1b).

In the harmonic approximation, the equations of motion can be expressed either in terms of the force constant ${\Phi }_{\alpha \beta }^{sC}\left(\kappa {\kappa }^{\prime }\right|\overrightarrow{q})$ matrix elements [127]:

$$M_k{\omega }_j^2\left(\overrightarrow{q}\right)e_{\alpha }\left(\kappa |\overrightarrow{q}j\right)=\sum _{{\kappa }^{\prime }\beta }{\Phi }_{\alpha \beta }^{sC}(\kappa {\kappa }^{\prime }|\overrightarrow{q})e_{\beta }\left(\kappa \prime |\overrightarrow{q}j\right)$$

(2a)

or in terms of the dynamical $D_{\alpha \beta }^{sC}\left(\kappa {\kappa }^{\prime }|\overrightarrow{q}\right)$ matrix elements:

$${{\omega }_{ }}_j^2\left(\overrightarrow{q}\right)e_{\alpha }\left(\kappa |\overrightarrow{q},j\right)=\sum _{{\kappa }^{\prime }\beta }{D}_{\alpha \beta }^{sC}\left(\kappa {\kappa }^{\prime }|\overrightarrow{q}\right)e_{\beta }\left({\kappa }^{\prime }|\overrightarrow{q},j\right);\hspace{1em}\mathrm{w}\mathrm{i}\mathrm{t}\mathrm{h} \kappa ,{\kappa }^{\prime }=1,2$$

(2b)

where,

$$D_{\alpha \beta }^{sC}\left(\kappa {\kappa }^{\prime }|\overrightarrow{q}\right)=D_{\alpha \beta }^s\left(\kappa {\kappa }^{\prime }|\overrightarrow{q}\right)-\frac{Z_{\kappa }{Z}_{{\kappa }^{\prime }}{e}^2}{{\left(M_{\kappa }{M}_{{\kappa }^{\prime }}\right)}^{1/2}}{D}_{\alpha \beta }^C\left(\kappa {\kappa }^{\prime }|\overrightarrow{q}\right)$$

(2c)

The term ${\Phi }_{\alpha \beta }^{sC}\left(\kappa {\kappa }^{\prime }\right|\overrightarrow{q})$ [$D_{\alpha \beta }^{sC}\left(\kappa {\kappa }^{\prime }|\overrightarrow{q}\right)$] in Equations (2a)–(2c) represents the force constant [dynamical] matrix elements involving components of both the short-range ${\Phi }_{\alpha \beta }^s\left(\kappa {\kappa }^{\prime }\right|\overrightarrow{q}\left)\right[D_{\alpha \beta }^s\left(\kappa {\kappa }^{\prime }|\overrightarrow{q}\right)]$ and long-range ${\Phi }_{\alpha \beta }^C\left(\kappa {\kappa }^{\prime }|\overrightarrow{q}\right) \left[D_{\alpha \beta }^C\left(\kappa {\kappa }^{\prime }|\overrightarrow{q}\right)\right]$ Coulomb interactions [127,139,140,141,142,145,146,147]. For simulating the ${\omega }_{j }\left(\overrightarrow{q}\right)$ of binary materials, the short- (A, B, C_κ, D_κ, E_κ and F_κ) and long-range (Z_eff) interactions involved in $D_{\alpha \beta }^s\left(\kappa {\kappa }^{\prime }|\overrightarrow{q}\right)$ and $D_{\alpha \beta }^C\left(\kappa {\kappa }^{\prime }|\overrightarrow{q}\right)$ matrices are optimized (

Table 2. In the notations of Ref. [127], we have reported the optimized sets of rigid-ion model (RIM) parameters [A, B, C₁, C₂, D₁, D₂, E₁, E₂, F₁, F₂ (10⁵ dyn/cm)] at ambient pressure for novel zb XC (X = Si, Ge and Sn) materials. The term Z_eff is the effective charge (see text).

Parameters (a)	3C-SiC	GeC	SnC
A	−0.91723	−0.68066	−0.39000
B	−0.44500	−0.66000	−0.37000
C1	−0.04050	−0.02300	−0.00400
C2	−0.15900	−0.13200	−0.09300
D1	0.06440	−0.02097	−0.00135
D2	−0.33088	−0.38000	−0.07200
E1	0.06200	−0.01000	−0.01000
E2	0.10850	0.02000	0.02000
F1	−0.04100	0.03550	0.01050
F2	0.28800	0.02780	0.01480
Zeff	1.05300	0.92200	0.88030

^(a) Ref. [127].

) following successive non-linear least-square fitting procedures.

In the primitive unit cell of the zb lattice having two atoms per unit cell, the solutions of Equations (2a) and (2b) lead to an eigenvalue problem of size (6 × 6) with the values of wave vectors $\overrightarrow{q}$ restricted to the fcc lattice:

$$\left|\overrightarrow{q}\right|=\frac{\pi }{a_o}(q_1,q_2,q_3);-1 \le q_1,q_2,q_3\le 1; (q_1+q_2+q_3)\le \frac{3}{2}$$

(3)

with the triplets $(q_1,q_2,q_3)$ distributed uniformly throughout the volume of the BZ. In our calculations of phonon dispersions ${\omega }_{j }\left(\overrightarrow{\mathit{q}}\right),$ for the XC materials, we have used a mesh of 64,000 |$\overrightarrow{q}$| points in the reduced BZ. At each $\overrightarrow{q}$, there are six [127] vibrational modes—three of them are acoustic (i.e., a longitudinal ${\omega }_{LA}$ and a doubly degenerate transverse ${\omega }_{TA}$) and the remaining modes are optical, comprising a doubly degenerate transverse—${\omega }_{TO}$ and a longitudinal ${\omega }_{LO}$ optical phonons (cf. Section 3.1).

2.2. Lattice Dynamics of Ternary X_xY_1−xC Alloys

Composition-dependent phonon dispersions ${\omega }_j\left(\overrightarrow{q}\right)$ for mixed zb X_xY_1−xC crystals are simulated as a function of x (0 ≤ x ≤ 1) (cf. Section 3.2) by adopting a generalized GF theory in the VCA. For one dimensional mixed X_xY_1−xC alloy with two interpenetrating sublattices, Kutty [131] has developed a GF approach and derived the dynamical matrix equation:

$$\left|\left({\omega }^2{M}_{\alpha }^{\mu }-{\Phi }_{\alpha \alpha }^{\mu \mu }\right){\delta }_{\alpha \beta }{\delta }_{\mu \nu }-C_{\alpha }^{\mu }{\Phi }_{\alpha \beta }^{\mu \nu }\left(\overrightarrow{q}\right)\right|=0$$

(4)

by postulating that the sublattice 1 occupies C atoms while the sublattice 2 is randomly acquired by atoms of type X and Y having concentrations (x) and (1 − x), respectively. In Equation (4), $C_1^C=1$, $C_2^Y=(1-\mathrm{x})$, $C_2^X=\mathrm{x}$, $M_1^C=m_C$, $M_2^Y=m_Y$, $M_2^X=m_X$ and the term ${\Phi }_{\alpha \beta }^{\mu \nu }$ represents the IFCs. By substituting these parameters in Equation (4), we obtain the following [131]:

$$\left|\begin{array}{ccc}{\omega }^2{m}_C-{\Phi }_{11}^{11}& -{\Phi }_{12}^{12}\left(\overrightarrow{q}\right)& -{\Phi }_{12}^{12}\left(\overrightarrow{q}\right)\\ -{(1-x)\Phi }_{21}^{21}\left(\overrightarrow{q}\right)& {\omega }^2{m}_Y-{\Phi }_{22}^{22}& -{(1-x)\Phi }_{22}^{23}\left(\overrightarrow{q}\right)\\ -x{\Phi }_{21}^{31}\left(\overrightarrow{q}\right)& -x{\Phi }_{22}^{32}\left(\overrightarrow{q}\right)& {\omega }^2{m}_X-{\Phi }_{22}^{33}\end{array}\right|=0$$

(5)

with the solution of Equation (5) leading to the vibrational mode frequencies.

Extension of the above one-dimensional approach to simulate the lattice dynamics of three-dimensional ideal zb random X_xY_1−xC ternary alloys using GF theory [131] in the framework of a RIM [127] is trivial. Here, we have assumed that the mixed X_xY_1−xC crystal lattice achieves the following: (a) forms the ideal pseudo-binary alloys in the entire composition range x, (probably in contrast to reality), (b) the cation sublattice 2 is structurally close to the virtual crystal lattice where the X and Y atoms are randomly distributed having the concentration of Y (1 − x) and of X as x, (c) the anion sublattice 1 with C atoms remains undistorted, and (d) the characteristic NN atomic distances follow Vegard’s law. It is to be noted that in the RIM GF methodology, no additional IFCs are required for the descriptions of wave-vector dependent phonon dispersions ${\omega }_j\left(\overrightarrow{\mathit{q}}\right)$ of the X_xY_1−xC alloys in arbitrary crystallographic directions. To simulate the dynamical characteristics of the pseudo-binary alloys using RIM, we have deliberated the IFCs of binary XC materials (cf. Table 2) in a three-body framework. While this approach has allowed for the calculations of phonon spectra for the optical, acoustical as well as disorder-induced modes, we have neglected here the disorder-related broadening of the spectral lines.

2.3. Thermodynamic Properties

To comprehend the effects of temperature on the structural stability of XC materials, we have calculated the thermodynamic properties (up to 1500 K) in the QHA by adopting a RIM and including appropriate values of their $g\left(\omega \right)$ and ${\omega }_{j }\left(\overrightarrow{q}\right)$ [132,133,134,135,136,137,138]. In the numerical computation of T-dependent lattice heat capacity (${\mathrm{C}}_{\mathrm{V}}\left(\mathrm{T}\right)$), we have used the following:

$$E\left(T\right)=E_{tot}+E_{zp}+\int \frac{\mathrm{ħ}\omega }{\exp \left(\frac{\mathrm{ħ}\omega }{k_{B}T}\right)-1}g\left(\omega \right)d\omega$$

(6)

where $E_{tot}$ is the total static energy at 0 K which can be calculated by first-principles methods, $E_{zp}$ is the zero-point vibrational energy, $k_B$ is Boltzmann’s constant, ħ is Planck’s constant. The term $E_{zp}$ can be expressed as follows:

$$E_{zp}=\frac{1}{2}\int g\left(\omega \right)\mathrm{ħ}\omega d\omega$$

(7)

The lattice contribution to ${\mathrm{C}}_{\mathrm{V}}\left(\mathrm{T}\right)$ is obtained by using [138] the following:

$${\mathrm{C}}_{\mathrm{V}}\left(T\right)=k_B\int \frac{{\left(\frac{\mathrm{ħ}\omega }{k_{B}T}\right)}^2\mathrm{e}\mathrm{x}\mathrm{p}\left(\frac{\mathrm{ħ}\omega }{k_{B}T}\right)}{{\left[\exp \left(\frac{\mathrm{ħ}\omega }{k_{B}T}\right)-1\right]}^2}g\left(\omega \right)d\omega$$

(8)

From Debye’s equation,

$${\mathrm{C}}_{\mathrm{V}}\left(\mathrm{T}\right)=9r{k}_B{\left(\frac{\mathrm{T}}{{\mathrm{\Theta }}_{\mathrm{D}}\left(\mathrm{T}\right)}\right)}^3{\int }_0^{{\mathrm{\Theta }}_{\mathrm{D}}\left(\mathrm{T}\right)}\frac{{\left(\frac{\mathrm{ħ}\omega }{k_{B}T}\right)}^4{e}^{\left(\frac{\mathrm{ħ}\omega }{k_{B}T}\right)}}{{\left[\exp \left(\frac{\mathrm{ħ}\omega }{k_{B}T}\right)-1\right]}^2}d\omega$$

(9)

it is possible to calculate ${\mathrm{\Theta }}_D\left(\mathrm{T}\right)$. In Equation (9), the term r signifies the number of atoms per unit cell.

2.4. Interaction of Photons with Solids

In polar materials, the electrical polarization induced by the displacement of atoms offers the key quantities to understand how the deformations and electric fields are coupled, insulating them. The interaction of photons with solids comprises both ionic and electronic oscillations where the dielectric polarization is linked to their atomic polarizability [130]. The dynamic response of dielectric function on electromagnetic radiation is expressed in terms of elementary oscillators, where the strong interaction of photons with ${\omega }_{TO}$ phonons cause a large Reststrahlen absorption in the IR region. In polar crystals, the ${\omega }_{LO}$ modes produce a macroscopic electric field that interacts with electrons, resulting in a quasi-particle known as ‘polaron’ [130]. This long-range coupling instigates the Fröhlich interaction ${\alpha }_F$(cf. Section 2.4.2). Born’s transverse effective charge $e_T^{*}$ (cf. Section 2.4.1) is an equally important quantity for studying the lattice dynamics of polar XC materials.

2.4.1. Born’s Effective Charge ${\mathrm{e}}_{\mathrm{T}}^{\mathrm{*}}$

In binary crystals, Born’s transverse effective charge $e_T^{*}$ is linked to the splitting of their optical phonon frequencies $∆\omega [\equiv {\omega }_{LO\left(\Gamma \right)}-{\omega }_{TO\left(\Gamma \right)}]$. The screening of the Coulomb interaction depends on the electronic part of the dielectric function and volume of the unit cell [130]. In many compound semiconductors, the phonon splitting $∆\omega$ is assessed by measuring the pressure (P)-dependent optical modes [148] by Raman scattering. This optical phonon splitting is then related to the bonding characteristics by being scaled to the lattice constants $a_o$. The P-dependent Raman studies are difficult, and, in some cases, the results have been contentious [149]. Except for 3C-SiC [107], no P-dependent measurements are available for GeC and SnC. In Section 3.2, we have reported our RIM calculations of x-dependent phonon splitting for X_xY_1−xC alloys and predicted the qualitative behavior of $e_T^{*}$ by using [149] the following:

$$e_T^{\mathrm{*}2}=\frac{{{\epsilon }_{\mathrm{\infty }}\mu a}_0^3}{16\pi }\left({\omega }_{LO\left(\mathrm{\Gamma }\right)}^2-{\omega }_{TO\left(\mathrm{\Gamma }\right)}^2\right)$$

(10)

where ${\epsilon }_{\infty }$ is the high-frequency dielectric constant and $\mu$ is the reduced mass of the anion–cation pair.

2.4.2. Fröhlich Coefficients ${\mathsf{\alpha }}_{\mathrm{F}}$

The theory of the Fröhlich interaction in ternary alloys is very complex. In binary materials, the strength of electron–phonon interaction is expressed by a dimensionless Fröhlich coupling constant ${\alpha }_F$ $\left[130\right]$:

$${\alpha }_F=\frac{1}{2}\frac{e^2/\sqrt{\mathrm{ħ}/2m_e^{\mathrm{*}}{\omega }_{LO}}}{\mathrm{ħ}{\omega }_{LO}}\left(\frac{1}{{\epsilon }_{\mathrm{\infty }}}-\frac{1}{{\epsilon }_o}\right)$$

(11)

where $e$ is the electron charge, $m_e^{*}$ the effective electron mass and ${\epsilon }_o$ is the static dielectric constant. Except for 3C-SiC, no systematic calculations of band structures or the effective electron masses $m_e^{*}$ are known for GeC and SnC.

3. Numerical Computations and Results

Following standard practices, we have computed the lattice dynamical, as well as the thermodynamic properties of both zb binary XC and ideal ternary X_xY_1−xC alloys. For the alloys, we have adopted (cf. Section 2.2) a generalized GF method in the framework of a RIM by incorporating the necessary IFCs (see Table 2) of the binary materials. The RIM results are analyzed by comparing/contrasting them with the existing experimental [105,129] and theoretical data [120,121,122,123,124,125,126].

3.1. Lattice Dynamics of Binary XC Materials

3.1.1. Phonon Characteristics

For binary XC materials, the simulated RIM phonon dispersions ${\omega }_j\left(\overrightarrow{\mathit{q}}\right),$ and one phonon DOS $g\left(\omega \right),$ are displayed in Figure 2a and Figure 2b, respectively. At ambient pressure, the acoustic phonon branches of the three (3C-SiC, GeC and SnC) compounds exhibited positive values—demonstrating their stability in the zb phases. From the DOS $g\left(\omega \right)$, one can clearly see two features, each with noticeable intensities in the low- (acoustic) as well as the high- (optical) frequency regions. Obviously, these characteristics are linked to the average ${\omega }_{TA,}$ ${\omega }_{LA}$ and ${\omega }_{TO,}$ ${\omega }_{LO}$ modes caused by the vibrations of heavier X and lighter C atomic masses, respectively.

For 3C-SiC, GeC and SnC materials, the simulated results of phonon frequencies at high critical points Γ, X, L reported in

Table 3. Comparison of the RIM calculated phonon frequencies (cm⁻¹) at Γ, X, L critical points with the experimental and theoretical calculations (A) 3C-SiC, (B) GeC and (C) SnC, respectively (see text).

(A)
3C-SiC	${\mathit{\omega }}_{\mathit{L}\mathit{O}\left(\Gamma \right)}$	${\mathit{\omega }}_{\mathit{T}\mathit{O}\left(\Gamma \right)}$	${\mathit{\omega }}_{\mathit{L}\mathit{O}\left(\mathit{X}\right)}$	${\mathit{\omega }}_{\mathit{T}\mathit{O}\left(\mathit{X}\right)}$	${\mathit{\omega }}_{\mathit{L}\mathit{A}\left(\mathit{X}\right)}$	${\mathit{\omega }}_{\mathit{T}\mathit{A}\left(\mathit{X}\right)}$	${\mathit{\omega }}_{\mathit{L}\mathit{O}\left(\mathit{L}\right)}$	${\mathit{\omega }}_{\mathit{T}\mathit{O}\left(\mathit{L}\right)}$	${\mathit{\omega }}_{\mathit{L}\mathit{A}\left(\mathit{L}\right)}$	${\mathit{\omega }}_{\mathit{T}\mathit{A}\left(\mathit{L}\right)}$	$∆\mathit{\omega }$
Our (a)	974	797	828	760	639	373	857	787	591	250	177
Expt. (b)	974	793	830	759	644	373	850	770	605	260	181
Expt. (c)	972	796	829	761	640	373	838	766	610	266	176
Calc. (d)	953	783	811	749	623	364	832	755	608	260	170
Calc. (e)	956	783	829	755	629	366	838	766	610	261	173
Calc. (f)	945	774	807	741	622	361	817	747	601	257	171
(a) Our; (b) Ref. [105]; (c) Ref. [129]; (d) Ref. [125]; (e) Ref. [122]; (f) Ref. [123].
(B)
GeC	${\mathit{\omega }}_{\mathit{L}\mathit{O}\left(\Gamma \right)}$	${\mathit{\omega }}_{\mathit{T}\mathit{O}\left(\Gamma \right)}$	${\mathit{\omega }}_{\mathit{L}\mathit{O}\left(\mathit{X}\right)}$	${\mathit{\omega }}_{\mathit{T}\mathit{O}\left(\mathit{X}\right)}$	${\mathit{\omega }}_{\mathit{L}\mathit{A}\left(\mathit{X}\right)}$	${\mathit{\omega }}_{\mathit{T}\mathit{A}\left(\mathit{X}\right)}$	${\mathit{\omega }}_{\mathit{L}\mathit{O}\left(\mathit{L}\right)}$	${\mathit{\omega }}_{\mathit{T}\mathit{O}\left(\mathit{L}\right)}$	${\mathit{\omega }}_{\mathit{L}\mathit{A}\left(\mathit{L}\right)}$	${\mathit{\omega }}_{\mathit{T}\mathit{A}\left(\mathit{L}\right)}$	$∆\mathit{\omega }$
Our (a)	749	626	697	617	348	211	705	621	326	166	123
Calc. (b)	812	682	785	695	378	222	789	683	366	161	130
Calc. (c)	748	626	697	617	348	214	705	612	331	162	122
(a) Our; (b) Ref. [124], (c) Ref. [125].
(C)
SnC	${\mathit{\omega }}_{\mathit{L}\mathit{O}\left(\Gamma \right)}$	${\mathit{\omega }}_{\mathit{T}\mathit{O}\left(\Gamma \right)}$	${\mathit{\omega }}_{\mathit{L}\mathit{O}\left(\mathit{X}\right)}$	${\mathit{\omega }}_{\mathit{T}\mathit{O}\left(\mathit{X}\right)}$	${\mathit{\omega }}_{\mathit{L}\mathit{A}\left(\mathit{X}\right)}$	${\mathit{\omega }}_{\mathit{T}\mathit{A}\left(\mathit{X}\right)}$	${\mathit{\omega }}_{\mathit{L}\mathit{O}\left(\mathit{L}\right)}$	${\mathit{\omega }}_{\mathit{T}\mathit{O}\left(\mathit{L}\right)}$	${\mathit{\omega }}_{\mathit{L}\mathit{A}\left(\mathit{L}\right)}$	${\mathit{\omega }}_{\mathit{T}\mathit{A}\left(\mathit{L}\right)}$	$∆\mathit{\omega }$
Our (a)	558	456	512	454	216	141	524	454	214	102	102
Calc. (b)	711	590	689	621	268	150	694	600	262	106	121
Calc. (c)	558	456	503	450	216	134	516	440	199	109	102
(a) Our; (b) Ref. [124], (c) Ref. [125].

A–C are compared against the existing experimental [105,129] and/or theoretical [120,121,122,123,124,125,126] data. Our lattice dynamical results for 3C-SiC contrasted reasonably well with the available phonon data from both the experimental (RSS [105], IXS [129]) and first-principles calculations [120,121,122,123,124,125,126]. For GeC and SnC, the results are also seen in very good accord with the phonon simulations reported by Zhang et al. [125] who used a plane–wave pseudopotential method in the density functional perturbation theory within the local density approximation.

3.1.2. Thermodynamic Characteristics

To test the accuracy of our RIM ${\omega }_j\left(\overrightarrow{\mathit{q}}\right),$ and $g\left(\omega \right),$ we have followed the methodology outlined in Section 2.3 and simulated the T-dependent Debye temperature and specific heat for the binary XC materials. The results of our calculations for ${\mathrm{\Theta }}_{\mathrm{D}}\left(\mathrm{T}\right)$ and ${\mathrm{C}}_{\mathrm{V}}\left(\mathrm{T}\right)$ displayed in Figure 3a,b are compared with the experimental (3C-SiC) [149,150,151] and/or theoretical data [152,153]. While the shapes of these thermodynamic quantities for the XC materials are typical to those of the group IV elemental and III–V, II–VI, I–VII compound semiconductors, the values of C-based materials, however, differ significantly [120,124,125,152].

At a single temperature T, the outcomes of our results (cf. Figure 3a,b) for ${\mathrm{\Theta }}_{\mathrm{D}}\left(\mathrm{T}\right)$ [${\mathrm{C}}_{\mathrm{V}}\left(\mathrm{T}\right)$] have shown considerable decrease [increase] in the simulated values with the increase of cation X atomic masses (i.e., from Si → Ge → Sn). Again, at low T, our results of ${\mathrm{C}}_{\mathrm{v}}\left(\mathrm{T}\right)$ for the binary materials have not only confirmed the correct trends with appropriate shapes replicating the T³ law but also provided a reasonably good match with the existing experimental/theoretical data [149,150,151,152,153]. For 3C-SiC, the experimental values of specific heat at constant volume (${\mathrm{C}}_{\mathrm{V}}$) [149] and constant pressure (${\mathrm{C}}_{\mathrm{P}})$ [150] are included in Figure 3b using different colored symbols. As expected, the results of ${\mathrm{C}}_{\mathrm{v}}\left(\mathrm{T}\right)$ for XC binary materials at highest temperatures approached ~50 (J/mol-K) [see (Figure 3b)], in excellent agreement with the Dulong–Petit rule. Clearly, these results are justified because at higher T, one would anticipate all the excited phonon modes contributing to the thermodynamic characteristics.

3.2. Phonon and Thermodynamic Properties of X_xY_1−xC Ternary Alloys

Recent developments in the crystal growth of ultrathin X_xY_1−xC films on different substrates have given excellent opportunities to many researchers to prepare novel C-based semiconducting alloys with the expected crystal structures and compositions [42,43,44,45,46]. For future device designs and their applications using zb X_xY_1−xC alloys, we have reported our comprehensive calculations and predicted their x-dependent phonon ${\omega }_j\left(\overrightarrow{\mathit{q}}\right),g\left(\omega \right),$ and thermodynamic ${\mathrm{\Theta }}_{\mathrm{D}}\left(\mathrm{T}\right),$ ${\mathrm{C}}_{\mathrm{V}}\left(\mathrm{T}\right)$ traits by using a RIM GF methodology in the VCA (see

Table 4. (A) Comparison of the temperature-dependent RIM calculations for the Debye temperature ${\mathrm{\Theta }}_D$(T), and (B) the specific heat ${\mathrm{C}}_v$(T) in (J/mol-K) for the zb SiC, GeC and SnC materials with the existing experimental Refs. [149,150] and/or theoretical Refs. [118,119,120,123,125,152] data. Please note that the reported results in Ref. [150] are for ${\mathrm{C}}_P$(T).

(A)
$\mathbf{Debye} \mathbf{Temperature}{\mathbf{\Theta }}_{\mathit{D}}$(T)
T	3C-SiC RIM (a)	Others	GeC RIM (a)	Others	SnC RIM (a)	Others
0	1090 (a)		673 (a)		419 (a)
100	923 (a)		635 (a)		490 (a)
300	1134 (a)	960.61 (b), 1130 (c), 611.6 (d), 1151 (e), 1080 (f)	857 (a)	759.6 (g), 616 (e), 831 (f)	619 (a)	506.7 (g), 472 (e)
600	1180 (a)	951.54 (b)	899 (a)		651 (a)
900	1188 (a)	942.52 (b)	906 (a)		657 (a)
1200	1192 (a)	934.94 (b)	908 (a)		659 (a)
1500	1194 (a)	930.02 (b)	910 (a)		660 (a)
(a) Our; (b) Refs. [149,150]; (c) Ref. [152]; (d) Ref. [120]; (e) Ref. [118]; (f) Ref. [119]; (g) Ref. [123].
(B)
$\mathbf{Specific} \mathbf{heat} {\mathbf{C}}_{\mathit{v}}$(T)
T	3C-SiC RIM (a)	Others	GeC RIM (a)	Others	SnC RIM (a)	Others
300	26.24	27.09 (b), 31.39 (c), 26.8 (d)	34.31	32.35 (e)	40.01	35.70 (e)
600	41.49	42.09 (b), 44.14 (c), 41.3 (d)	44.58	44.6 (e*)	46.96	46.10 (e*)
900	45.77	47.60 (b), 47.26 (c), 46.7 (d)	47.41	47.1 (e*)	48.56	48.32 (e*)
1200	47.51	50.19 (b), 48.41 (c), 49.6 (d)	48.46	48.2 (e*)	-	-
1500	48.34	51,77 (b), 48.94 (c), 51.2 (d)	-	-	-	-
(a) Our; (b) Refs. [149,150]; (c) Ref. [152]; (d) Ref. [120]; (e) Ref. [125] (e*) estimated from the graph.

and employing the results of binary XC materials).

3.2.1. Phonon Characteristics of X_1−xY_xC Alloys

In the framework of a generalized RIM GF formalism (cf. Section 2.2), systematic simulations are performed for predicting the results of x-dependent phonon dispersions ${\omega }_j\left(\overrightarrow{\mathit{q}}\right),$ and DOS, $g\left(\omega \right)$ for the ideal mixed X_1−xY_xC ternary alloys. For instance, the calculated results of Si_1−xGe_xC alloys displayed in Figure 4a,b have clearly validated that the values of ${\omega }_j\left(\overrightarrow{\mathit{q}}\right),$ and $g\left(\omega \right)$ steadily shifting towards the lower frequency regions as x increases (i.e., from ≡0.0, 0.2, 0.4, 0.6, 0.8 and 1.0). Moreover, in the extreme situations x → 0.0, and x → 1.0, the results are seen transforming to those of the binary SiC and GeC materials.

From the phonon characteristics of Si_1−xGe_xC alloys (cf. Figure 4a,b), we have also noticed a few interesting features: (a) the x-dependent curves of ${\omega }_j\left(\overrightarrow{\mathit{q}}\right),\mathrm{a}\mathrm{n}\mathrm{d} g\left(\omega \right),$ exhibit very similar traits, (b) the ${\omega }_{TO}$ modes show nearly flat dispersions in the X → Γ → L directions which are responsible for triggering appropriate strong peaks in the $g\left(\omega \right)$, (c) the ${\omega }_{LO}$ modes have demonstrated almost flat dispersions in the L → X → W directions which initiated the correct weak peaks in the $g\left(\omega \right)$, and (d) the splitting of optical phonon modes $∆\omega$ (${\equiv \omega }_{LO\left(\mathrm{\Gamma }\right)}$ − ${\omega }_{TO\left(\mathrm{\Gamma }\right)}$) decreased from 177 cm⁻¹ → 161 cm⁻¹ → 149 cm⁻¹ → 139 cm⁻¹ → 130 cm⁻¹ → 123 cm⁻¹ with the increase of composition, x ($\equiv$0.0 → 0.2 → 0.4 → 0.6 → 0.8 → 1.0). Very similar phonon characteristics are also noticed in the Si_1−xSn_xC (cf. Figure 5a,b) and Ge_1−xSn_xC (cf. Figure 6a,b) ternary alloys.

3.2.2. Thermodynamic Characteristics of X_1−xY_xC Alloys

By using appropriate phonon dispersions and DOS, the x-dependent thermodynamic characteristics are simulated in the QHA for X_1−xY_xC ternary alloys between 0 < T < 1500 K. As an example, we have reported our results of Θ_D(T) and C_v(T) for the Si_1−xGe_xC alloys in Figure 7a and Figure 7b, respectively. Similar calculations have also been performed for other Si_1−xSn_xC (cf. Figure 8a,b) and Ge_1−xSn_xC (cf. Figure 9a,b) ternary alloys. From Figure 7a,b, some important noticeable facts can be justified. As x increases (from ≡0, 0.2, 0.4, 0.6, 0.8 and 1.0), the values of Θ_D(T) [C_v(T)] decrease [increase] and in the limiting situations x → 0.0, and x →1.0, the results transform to those of SiC and GeC materials. Based on our simulations, the binary 3C-SiC has exhibited the highest Θ_D(T) and lowest ${\mathrm{C}}_{\mathrm{v}}\left(\mathrm{T}\right),\mathsf{\alpha }\left(\mathrm{T}\right)$ values. These results, related to its shorter bond-length and larger bond strength (see Table 2), can exhibit strong radiation tolerance with excellent resistance. Obviously, these characteristics have led to 3C-SiC being quite robust at higher T with less likelihood of breakdown in extreme conditions. We, therefore, feel that 3C-SiC is an ideal compound to be employed as a fuel-cladding material in nuclear reactors and high-temperature environments.

On the other hand, both GeC and SnC revealed significantly weaker bonding [149,150,151,152,153] (see Table 2) which instigated lower Θ_D(T) and higher ${\mathrm{C}}_{\mathrm{v}}$(T), $\mathsf{\alpha }\left(\mathrm{T}\right)$ values. Thus, we anticipate that these materials may not be suitable for fuel-cladding layers in nuclear reactors and/or in higher temperature environments. However, with a very small composition (x), the ternary Si_1−xGe_xC alloys can still be deliberated. Both XC and X_1−xY_xC alloys have already been used to grow multilayer (viz., SiC/SiGe(Sn)C, GeC/Si(Ge)SnC) heterostructures. Thus, we feel that these structures may help engineers to design MQW/SL-based micro-/nanodevices for different strategic and civilian application needs.

3.2.3. Born Effective Charge for X_1−xY_xC Alloys

In polar materials, Born’s effective charge $e_T^{*}$ (also known as transverse or dynamic effective charge) manifests the coupling between lattice displacements and electrostatic fields. It is found that $e_T^{*}$ remains insensitive to the isotropic volume change but strongly affected by changes in the atomic positions associated with phase transitions (P_t). From a theoretical standpoint, $e_T^{*}$ in binary XC and ternary X_1−xY_xC alloys is important as P_t takes place due to the competition between long-range coulomb interactions and short-range forces. The long-range coulomb interactions are responsible for the observed splitting $∆\omega [\equiv {\omega }_{LO\left(\Gamma \right)}-{\omega }_{TO\left(\Gamma \right)}]$ between ${\omega }_{LO\left(\Gamma \right)}$ and ${\omega }_{TO\left(\Gamma \right)}$ phonon frequencies. In

Table 5. Simulated composition-dependent Born’s effective charges for zinc-blende ternary alloys: (A) Si_1−xGe_xC, (B) Si_1−xSn_xC, and (C) Ge_1−xSn_xC.

(A)
Si1−xGexC
x	${\mathit{\omega }}_{\mathit{L}\mathit{O}\mathbf{\left(}\mathit{\Gamma }\mathbf{\right)}}$	${\mathit{\omega }}_{\mathit{L}\mathit{O}\mathbf{\left(}\mathit{\Gamma }\mathbf{\right)}}$	$∆\mathit{\omega }$	${\mathit{e}}_{\mathit{T}}^{\mathit{*}}$ (a)	${\mathit{e}}_{\mathit{T}}^{\mathit{*}}$  others
0.0	974	797	177	2.683	2.697 (b), 2.72 (c)
0.2	911	750	161	2.675
0.4	862	713	149	2.659
0.6	821	682	139	2.629
0.8	783	653	130	2.611
1.0	749	626	123	2.581	2.62 (d)
(a) Our; (b) Ref. [107]; (c) Refs. [122,123]; (d) Ref. [124].
(B)
Si1−xSnxC
x	${\mathit{\omega }}_{\mathit{L}\mathit{O}\mathbf{\left(}\mathit{\Gamma }\mathbf{\right)}}$	${\mathit{\omega }}_{\mathit{L}\mathit{O}\mathbf{\left(}\mathit{\Gamma }\mathbf{\right)}}$	$∆\mathit{\omega }$	${\mathit{e}}_{\mathit{T}}^{\mathit{*}}$  (a)	${\mathit{e}}_{\mathit{T}}^{\mathit{*}}$  others
0.0	974	797	177	2.683	2.697 (b), 2.72 (c)
0.2	856	707	149	2.697
0.4	773	642	131	2.695
0.6	700	582	118	2.684
0.8	630	522	108	2.658
1.0	558	456	102	2.629	2.95 (d)
(a) Our; (b) Ref. [107]; (c) Refs. [122,123]; (d) Ref. [124].
(C)
Ge1−xSnxC
x	${\mathit{\omega }}_{\mathit{L}\mathit{O}\mathbf{\left(}\mathit{\Gamma }\mathbf{\right)}}$	${\mathit{\omega }}_{\mathit{L}\mathit{O}\mathbf{\left(}\mathit{\Gamma }\mathbf{\right)}}$	$∆\mathit{\omega }$	${\mathit{e}}_{\mathit{T}}^{\mathit{*}}$  (a)	${\mathit{e}}_{\mathit{T}}^{\mathit{*}}$  others
0.0	749	626	177	2.581	2.62 (d)
0.2	710	594	116	2.595
0.4	673	562	111	2.600
0.6	635	528	107	2.615
0.8	597	493	104	2.623
1.0	558	456	102	2.629	2.95 (d)
(a) Our; (d) Ref. [124].

, we have reported our simulated results of x-dependent $∆\omega$ and $e_T^{*}$ for X_1−xY_xC alloys. In the absence of $e_T^{*}$ data for ternary alloys, our theoretical results for the binary materials agree reasonably well with the experimental (for 3C-SiC) [107] and theoretical [123] results (for GeC and SnC) [122,124].

3.2.4. Fröhlich Coefficients

In semiconductors, the strength of the Fröhlich interaction is directly linked to the polar nature of its crystal lattice. In a highly polar material, the Coulomb field of a carrier (or exciton) couples more easily to the polar vibrations (i.e., ${\omega }_{LO}$ phonons) of the crystal lattice, resulting in a strong Fröhlich coupling coefficient, ${\alpha }_{F }$. By using ${\alpha }_{F }$ (cf. Equation (11)), the polaron effective mass ${ m}_p^{*}$ can be calculated [154] in terms of the bare electron mass $m_{e }$ using the following expression:

$${ m}_p^{*}=m_e·\frac{1-0.0008{{ \alpha }_{F }}^2}{1-\left(\frac{{\alpha }_{F }}{6}\right)+0.003 {{\alpha }_{F }}^2}$$

(12)

where, the static ${\epsilon }_o$ and high-frequency ${\epsilon }_{\infty }$ values of the dielectric functions provide the means of quantifying the polar nature of materials, so that further insight into the Fröhlich interaction (see Equation (11)) can be gained via the dielectric characterizations.

It is to be noted that, except for 3C-SiC, no systematic calculations of the band structures and effective electron masses $m_e^{*}$ are known for the GeC and SnC materials. With Equation (11), and using the existing parameters (${\epsilon }_o$, ${\epsilon }_{\infty }$, ${\omega }_{LO}$, $m_e^{*}$) for 3C-SiC from the literature [105,106,107], our calculation of ${\alpha }_{F }$(=0.576) has provided a value much higher than that reported by Adachi [130]. Interestingly, however, the calculation of polaron mass (${ m}_p^{*}/m_{e }\equiv$ 0.243) using Equation (12) agrees very well with the theoretical result reported by Persson and Lindefelt [154]. Obviously, more experimental and theoretical efforts are needed for assessing the accurate values of the Fröhlich interaction coefficients for both the binary XC and ternary X_1−xY_xC alloys.

4. Discussions and Conclusions

In summary, we have used a realistic RIM and reported the results of our methodical simulations for comprehending the lattice dynamical and thermodynamic characteristics of zb (SiC, GeC, SnC) binary and (S_1−xGe_xC, S_1−xSn_xC, Ge_−xSn_xC) ternary alloys. From a basic chemistry standpoint, one expects a gradual increase in the bond lengths (i.e., from Si-C → Ge-C → Sn-C) due to the differences in the sizes of the cations (Si, Ge, Sn) and (C) anion atoms. Accordingly, the increase in bond lengths will cause a decrease in their bond strengths. These facts are clearly revealed in our calculated IFCs of the RIM for XC materials (see Table 2), where the nearest-neighbor force constants [127] have shown steady decrease in their strength as one proceeds from SiC → GeC → SnC. Obviously, the atomic- and composition (x)-dependent variations in the bond strengths of mixed X_1−xY_xC alloys have instigated dramatic variations in the simulated RIM phonon dispersions ${\omega }_j\left(\overrightarrow{\mathit{q}}\right),\mathrm{D}\mathrm{O}\mathrm{S}g\left(\omega \right),$ and thermodynamic [e.g., Θ_D(T), ${\mathrm{C}}_{\mathrm{v}}$(T), $\mathsf{\alpha }\left(T\right)]$ traits.

In recent years, consistent efforts have been made using group-IV carbides and III-nitrides to develop devices for achieving efficient operations in challenging environments (viz., radiation, high-power, extreme temperature) where the electronic systems based on Si material have indicated weaknesses of survival. In this quest, it is necessary to assess the electronic and thermodynamic characteristics of XC materials to see if they satisfy the necessary requirements for their use in the high temperature/high power settings. One must note that the devices based on wide bandgap GaN and SiC have recently emerged in the commercial market for slowly replacing the traditional Si-built electronic parts. Both GaN and SiC materials with wide bandgaps, high critical electric fields, and low dielectric constants have reflected on the lower on-state resistance for a given blocking voltage. In addition, these materials have exhibited high Debye temperatures Θ_D(T), low specific heats ${\mathrm{C}}_{\mathrm{v}}$(T) and low thermal expansion $\mathsf{\alpha }\left(T\right)$ coefficients. As compared to SiC, there are a few disadvantages for the selection of GaN material. The main problems have been identified as follows: (a) the manufacturing complexity, cost, intrinsic defects, and reliability concerns about the integration of GaN into the existing processes with limited availability of substrates, and (b) the relatively lower [higher] values of Θ_D(T) [${\mathrm{C}}_{\mathrm{v}}$(T), $\mathsf{\alpha }\left(T\right)]$ [108,136]. Again, with respect to Si ($E_g$≡ 1.12 eV), the reported theoretical bandgap energies of GeC and SnC materials are 1.51 eV [118], and 0.75 eV [117], respectively. Obviously, compared to SiC, the binary GeC, SnC and/or ternary Ge_1−xSn_xC alloys with lower $E_g$ and weaker bonding have exhibited different lattice dynamical and thermodynamic properties. Therefore, we strongly feel that devices based on binary GeC, SnC and/or Ge_1−xSn_xC materials may not be suitable for radiation detection in nuclear reactors or high-temperature, high-power settings. However, from recent successful efforts in the growth of ultrathin zb XC binary and X_1−xY_xC ternary alloys, along with their predicted results of phonon, structural, and thermodynamic traits, the materials can still be credible for the preparations of heterostructures in designing MQW and SL-based micro-/nanodevices for different strategic and civilian application needs.