Efficient 2D Neck Model for Simulation of the Whiplash Injury Mechanism
Abstract
:1. Introduction
2. Numerical Modeling of the ENM-2D
2.1. Geometry and Inertia
2.2. Stiffness and Damping
2.3. Reference Configuration and Simulation Results
3. Identification Process
4. Modification of the Model and Application of the Optimization Process
5. Injury Criteria Assessment
6. Discussion
7. Limitations
8. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Administration, N.H.T.S. Traffic Safety Facts 2020: A Compilation of Motor Vehicle Crash Data. 2022. Available online: https://crashstats.nhtsa.dot.gov/ (accessed on 25 January 2023).
- Ambrósio, J. Crash analysis and dynamical behaviour of light road and rail vehicles. Veh. Syst. Dyn. 2005, 43, 385–411. [Google Scholar] [CrossRef]
- DeHaven, H. Mechanical analysis of survival in falls from heights of fifty to one hundred and fifty feet. War Med. 1942, 2, 586–596. [Google Scholar]
- Stapp, J. Human exposure to linear acceleration. Aero. Med. Lab. Air Force Rep. 1951, 5912, 2. [Google Scholar]
- Mertz, H.J.; Irwin, A.L. Anthropomorphic Test Devices and Injury Risk Assessments. In Accidental Injury: Biomechanics and Prevention; Springer: New York, NY, USA, 2015; pp. 83–112. [Google Scholar]
- Schmitt, K.U.; Niederer, P.F.; Cronin, D.S.; Morrison, B.; Muser, M.H.; Walz, F. Trauma Biomechanics: An Introduction to Injury Biomechanics, 5th ed.; Springer: Cham, Switzerland, 2019. [Google Scholar]
- Van Ratingen, M.; Williams, A.; Anders, L.; Seeck, A.; Castaing, P.; Kolke, R.; Adriaenssens, G.; and Miller, A. The European New Car Assessment Programme: A historical review. Chin. J. Traumatol. 2016, 19, 63–69. [Google Scholar] [CrossRef]
- Tameem, A.; Kapur, S.; Mutagi, H. Whiplash injury. Contin. Educ. Anaesth. Crit. Care Pain 2014, 14, 167–170. [Google Scholar] [CrossRef]
- Bogduk, N. On cervical zygapophysial joint pain after whiplash. Spine 2011, 36, 194–199. [Google Scholar] [CrossRef]
- Panjabi, M.; Jonathan, G.; Jacek, C.; Kimio, N.; Jiri, D. Whiplash produces an S-Shaped curvature of the neck with hyperextension at lower levels. Spine 1997, 22, 2489–2494. [Google Scholar]
- Carvalho, M.; Ambrosio, J. Development of generic road vehicle multibody models for crash analysis using an optimisation approach. Int. J. Crashworthiness 2011, 16, 537–556. [Google Scholar] [CrossRef]
- Ambrosio, J.; Carvalho, M.; Milho, J.; Escalante, S.; Martin, R. A validated railway vehicle interior layout with multibody dummies and finite element seats models for crash analysis. Multibody Syst. Dyn. 2022, 54, 179–212. [Google Scholar] [CrossRef]
- Roupa, I.; da Silva, M.R.; Marques, F.; Gonçalves, S.B.; Flores, P.; da Silva, M.T. On the modeling of biomechanical systems for human movement analysis: A narrative review. Arch. Comput. Methods Eng. 2022, 29, 4915–4958. [Google Scholar] [CrossRef]
- Kan, C.D.; Marzougui, D.; Bahouth, G.T.; Bedewi, N.E. Crashworthiness evaluation using integrated vehicle and occupant finite element models. Int. J. Crashworthiness 2001, 6, 387–398. [Google Scholar] [CrossRef]
- Carvalho, M.; Martins, A.; Milho, J. Validation of a railway inline seating model for occupants injury biomechanics. Int. J. Crashworthiness 2018, 23, 328–335. [Google Scholar] [CrossRef]
- Wu, W.; Han, Z.; Hu, B.; Du, C.; Xing, Z.; Zhang, C.; Gao, J.; Shan, B.; Chen, C. A graphical guide for constructing a finite element model of the cervical spine with digital orthopedic software. Ann. Transl. Med. 2021, 9, 169. [Google Scholar] [CrossRef]
- Meyer, F.; Humm, J.; Yoganandan, N.; Leszczynski, A.; Bourdet, N.; Deck, C.; Willinger, R. Development of a detailed human neck finite element model and injury risk curves under lateral impact. J. Mech. Behav. Biomed. Mater. 2021, 116, 104318. [Google Scholar] [CrossRef]
- Johnson, D.; Koya, B.; Gayzik, F.S. Comparison of Neck Injury Criteria Values Across Human Body Models of Varying Complexity. Front. Bioeng. Biotechnol. 2020, 8, 985. [Google Scholar] [CrossRef] [PubMed]
- Putra, I.; Iraeus, J.; Sato, F.; Svensson, M.Y.; Thomson, R. Finite element human body models with active reflexive muscles suitable for sex based whiplash injury prediction. Front. Bioeng. Biotechnol. 2022, 10, 968939. [Google Scholar] [CrossRef] [PubMed]
- Yang, S.; Qu, L.; Yuan, L.; Niu, J.; Song, D.; Yang, H.; Zou, J. Finite element analysis of spinal cord stress in a single segment cervical spondylotic myelopathy. Front. Surg. 2022, 9, 849096. [Google Scholar] [CrossRef]
- Schwartz, D.; Guleyupoglu, B.; Koya, B.; Stitzel, J.D.; Gayzik, F.S. Development of a computationally efficient full human body finite element model. Traffic Inj. Prev. 2015, 16, S49–S56. [Google Scholar] [CrossRef]
- Happee, R.; Hoofman, M.; Kroonenberg, A.J.V.D.; Morsink, P. A Mathematical Human Body Model for Frontal and Rearward Seated Automotive Impact Loading. J. Passeng. Cars 1998, 107, 2720–2734. [Google Scholar]
- Van der Horst, M. Human Head Neck Response in Frontal, Lateral and Rear end Impact Loading: Modelling and Validation. Ph.D. Thesis, Technische Universiteit Eindhoven, Eindhoven, The Netherlands, 2002. [Google Scholar]
- Bortenschlager, K.; Kramberger, D.; Barnsteiner, K.; Hartlieb, M.; Ferdinand, L.; Leyer, H.; Muser, M.; Schmitt, K.U. Comparison Tests of BioRID II and RID 2 with Regard to Repeatability, Reproducibility and Sensitivity for Assessment of Car Seat Protection Potential in Rear-End Impacts. Stapp Car Crash J. 2003, 47, 473–488. [Google Scholar] [CrossRef]
- Panjabi, M.; Cholewicki, J.; Nibu, K.; Babat, L.; Dvorak, J. Simulation of whiplash trauma using whole cervical spine speciments. Spine 1998, 23, 17–24. [Google Scholar] [CrossRef]
- Camacho, D.L.; Nightingale, R.W.; Robinette, J.J.; Vanguri, S.K.; Coates, D.J.; Myers, B.S. Experimental Flexibility Measurements for the Development of a Computational Head-Neck Model Validated for Near-Vertex Head Impact. J. Passeng. Cars 1997, 106, 3989–4002. [Google Scholar]
- Himmetoglu, S. Car Seat Design and Human-Body Modelling for Rear Impact Whiplash Mitigation. Ph.D. Thesis, Loughborough University, Loughborough, UK, 2008. [Google Scholar]
- Kostreva, M.M.; Chen, X. A superlinearly convergent method of feasible directions. Appl. Math. Comput. 2000, 116, 231–244. [Google Scholar] [CrossRef]
- Arora, J.S. Introduction to Optimum Design, 4th ed.; Elsevier: Amsterdam, The Netherlands, 2017. [Google Scholar]
- Carvalho, M.; Ambrósio, J.; Eberhard, P. Identification of validated multibody vehicle models for crash analysis using a hybrid optimization procedure. Struct. Multidiscip. Optim. 2011, 44, 85–97. [Google Scholar] [CrossRef]
- Yoganandan, N.; Nahum, A.M.; Melvin, J.W. Accidental Injury: Biomechanics and Prevention, 3rd ed.; Springer: Berlin/Heidelberg, Germany, 2014. [Google Scholar]
- Schmitt, K.U.; Muser, M.H.; Niederer, P. A New Neck Injury Criterion Candidate for Rear-End Collisions Taking into Account Shear Forces and Bending Moments. In Proceedings of the ESV Conference, Amsterdam, The Netherlands, 4–7 June 2001. [Google Scholar]
- Carvalho, M.; Milho, J.; Ambrosio, J.; Ramos, N. Railway occupant passive safety improvement by optimal design. Int. J. Crashworthiness 2017, 22, 624–634. [Google Scholar] [CrossRef]
- Carvalho, M.; Martins, A.P.; Milho, J. Railway seat design for injury mitigation in crash scenario. Int. J. Rail Transp. 2020, 8, 215–233. [Google Scholar] [CrossRef]
- Wang, Y.; Jiang, H.; Teo, E.C.; Gu, Y. Finite Element Analysis of Head–Neck Kinematics in Rear-End Impact Conditions with Headrest. Bioengineering 2023, 10, 1059. [Google Scholar]

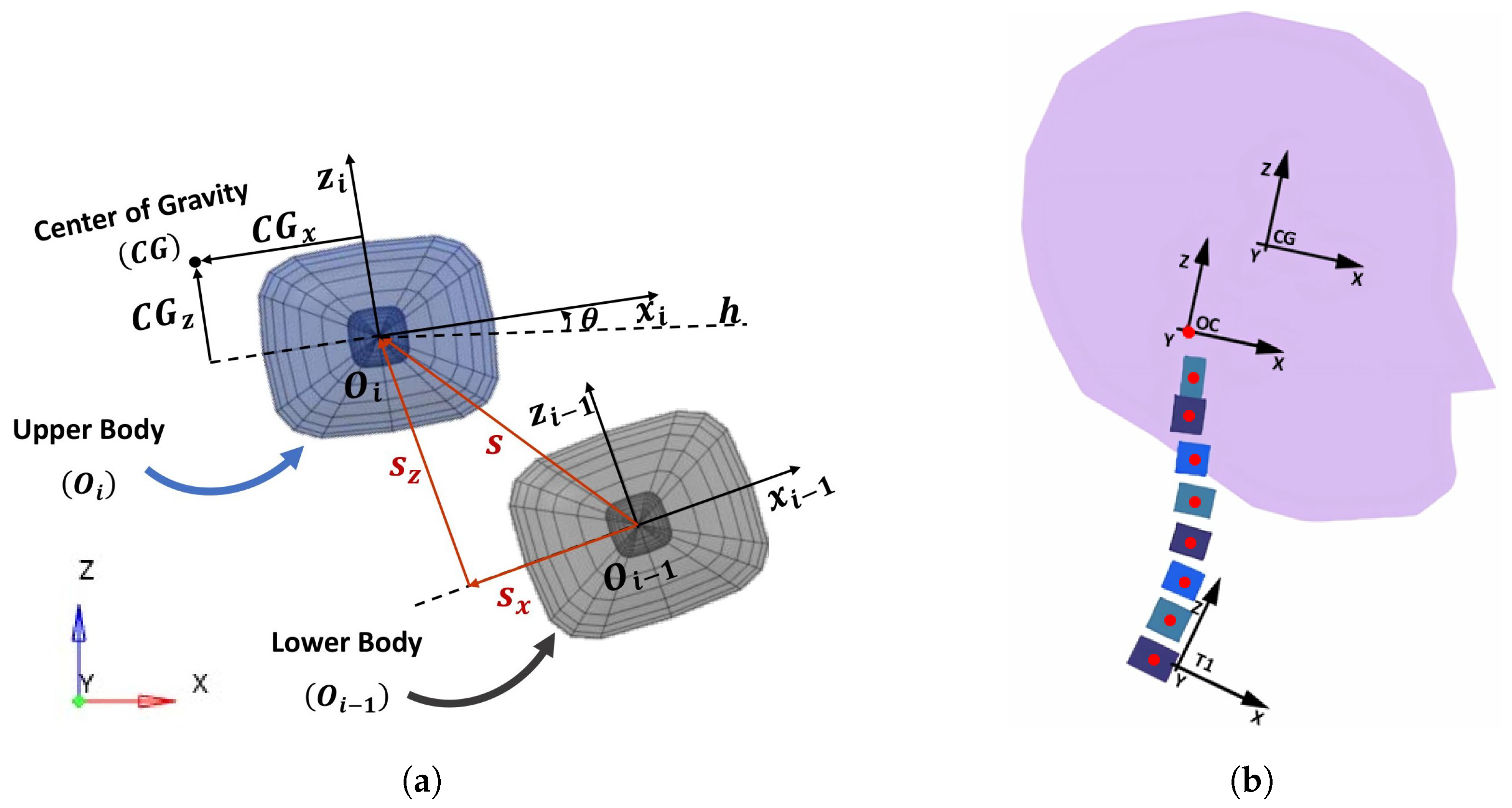


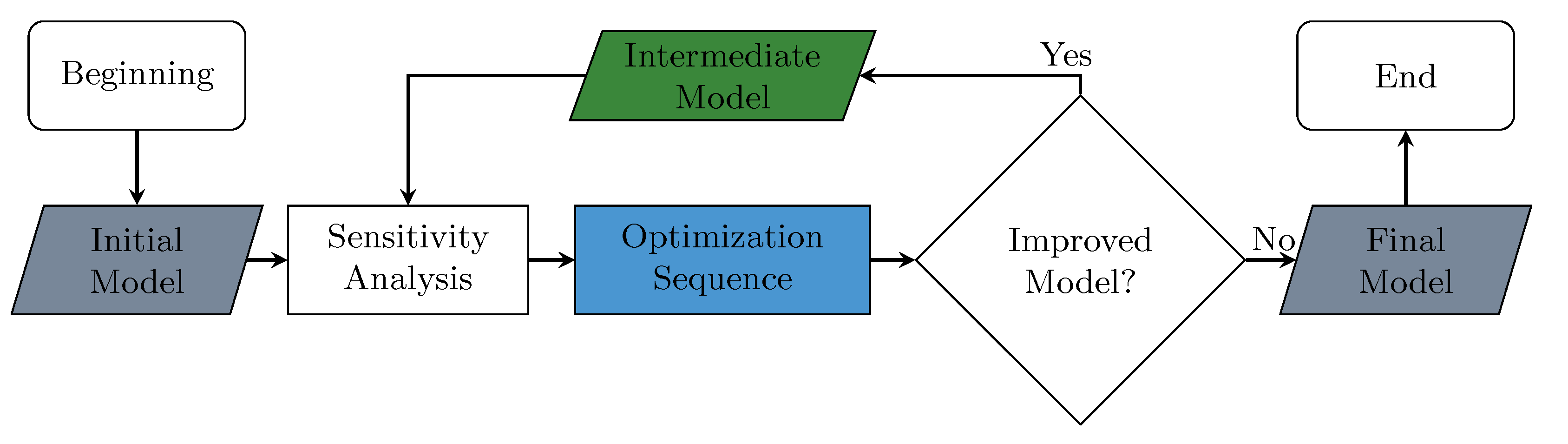

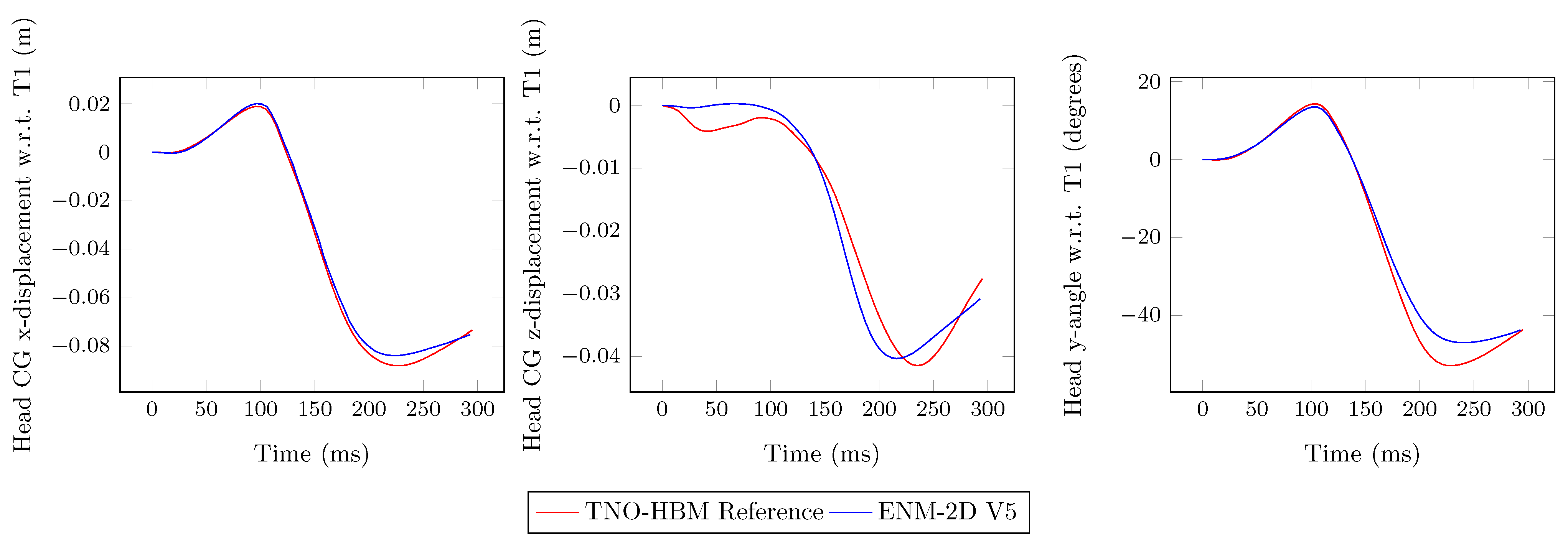
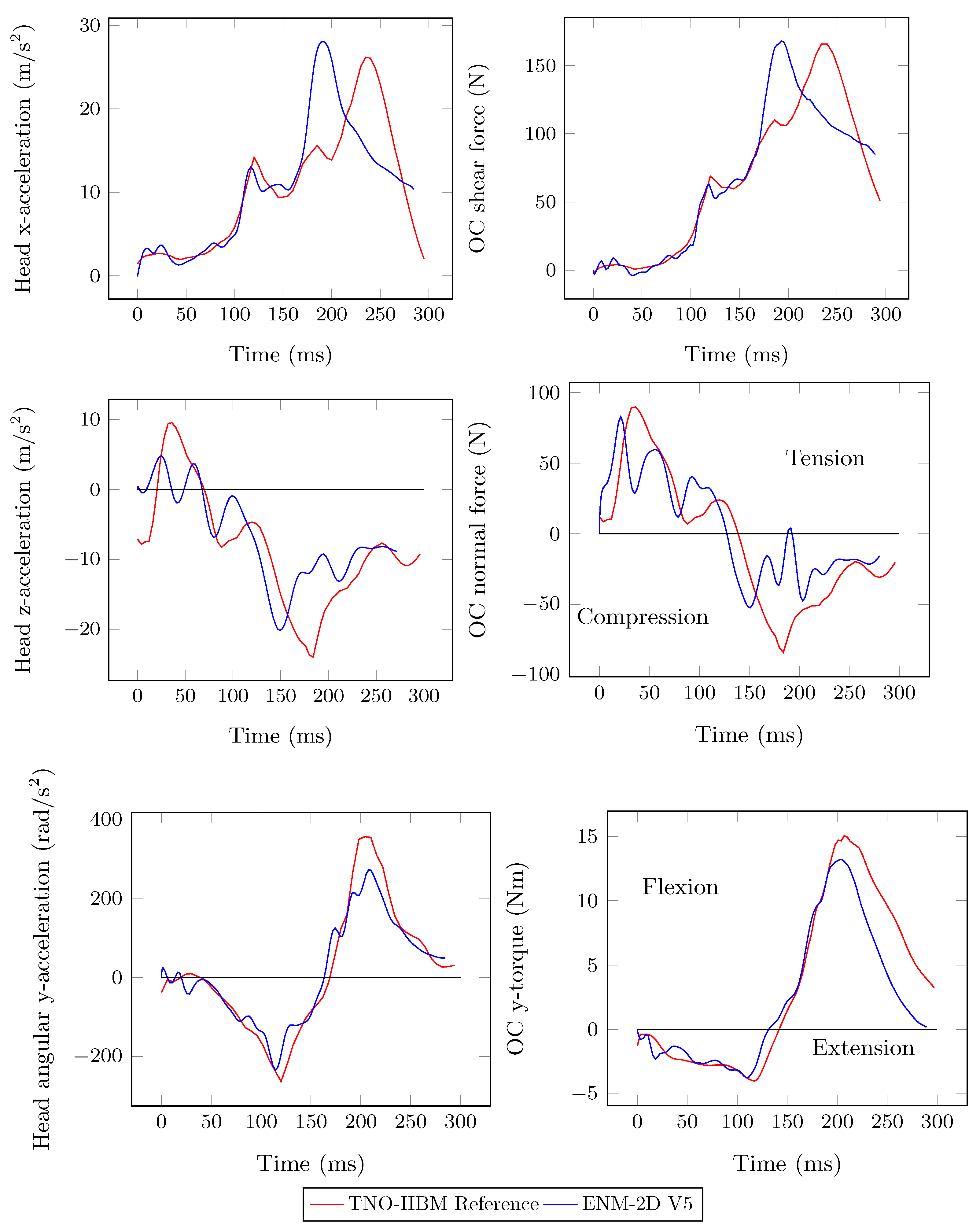


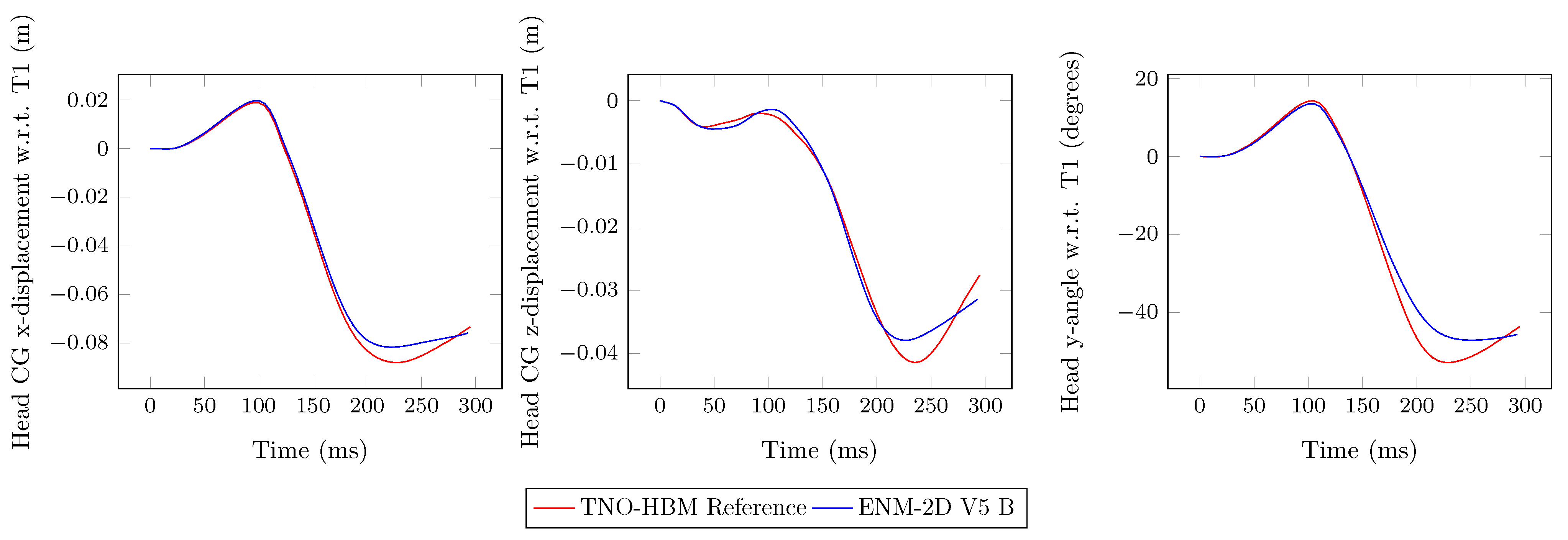
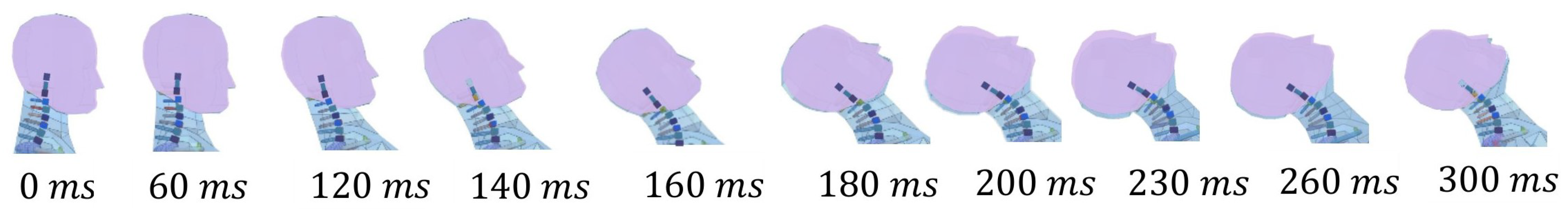
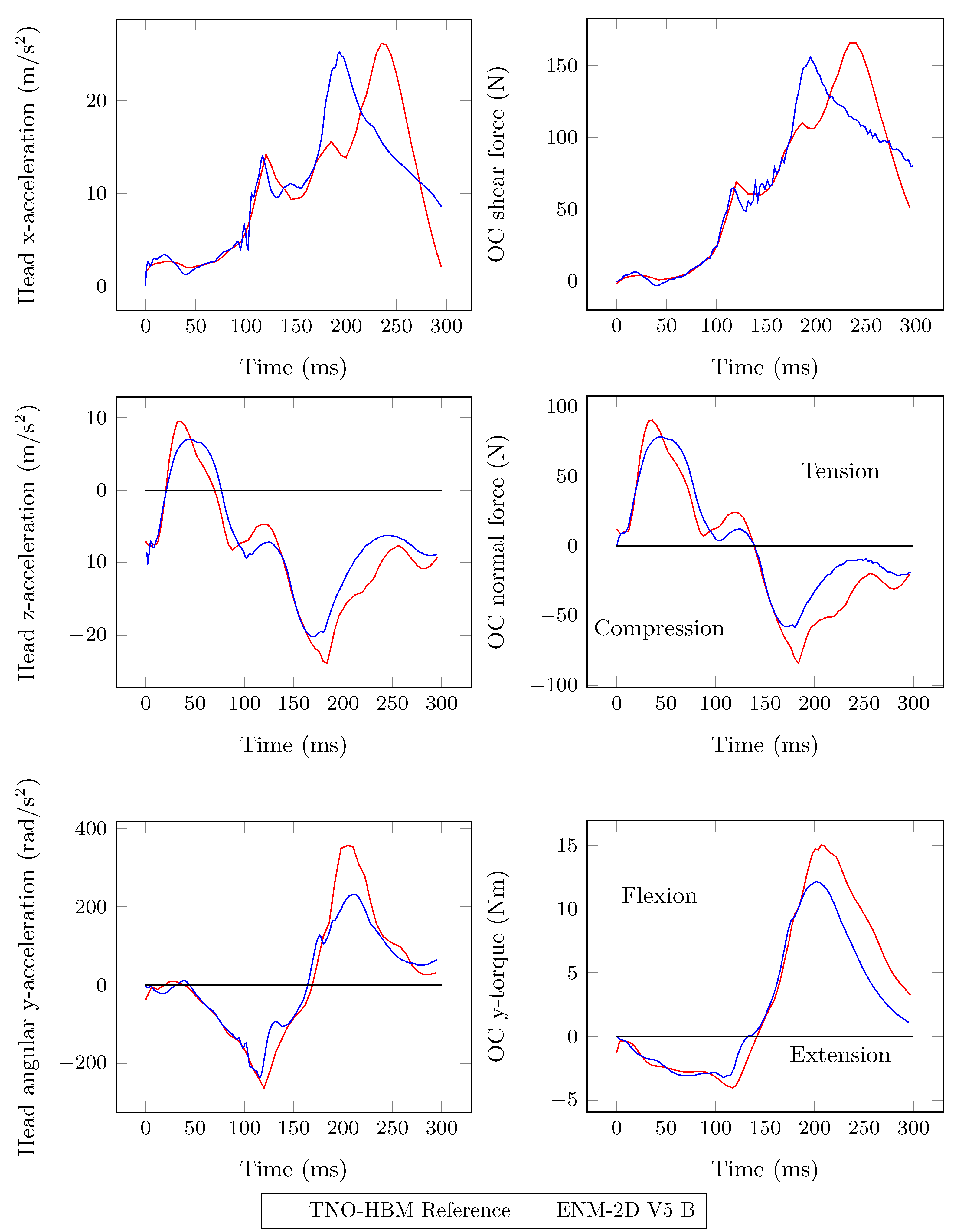
| Body | Mass (kg) | Moment of Inertia (kg m2) | Position 1 rel. to (mm) | Position 2 CG (mm) | Angle with the Horizontal (°) | ||||
|---|---|---|---|---|---|---|---|---|---|
| Ixx | Iyy | Izz | Sx | Sz | Gx | Gz | |||
| Head (C0) | 4.69 | 0.018 | 0.024 | 0.017 | −2 | 21 | 27 | 0.043 | −13 |
| C1 | 0.22 | 0.001 | 0.001 | 0.002 | 2 | 16 | −7.7 | 0 | −7 |
| C2 | 0.25 | 0.001 | 0.001 | 0.002 | −2 | 19 | −7.7 | 0 | −7 |
| C3 | 0.24 | 0.001 | 0.001 | 0.002 | −1 | 18 | −7.8 | 0 | −7 |
| C4 | 0.23 | 0.001 | 0.001 | 0.002 | 2 | 18 | −7.9 | 0 | −12 |
| C5 | 0.23 | 0.001 | 0.001 | 0.002 | 3 | 17 | −8.1 | 0 | −17 |
| C6 | 0.24 | 0.001 | 0.001 | 0.002 | 6 | 17 | −8.3 | 0 | −23 |
| C7 | 0.22 | 0.001 | 0.001 | 0.002 | 7 | 17 | −8.2 | 0 | −26 |
| T1 | 0.86 | 0.010 | 0.010 | 0.020 | 0 | 0 | 0 | 0 | −26 |
| C0/C1 | C1/C2 | C2/C3 | C3/C4 | C4/C5 | C5/C6 | C6/C7 | C7/T1 | ||
|---|---|---|---|---|---|---|---|---|---|
| Extension | (Nm) | −0.0095 | −0.0095 | −0.0037 | −0.0068 | −0.0027 | −0.0126 | −0.0125 | −0.3105 |
| (−) | −0.3937 | −0.3937 | −1.0137 | −1.1416 | −1.6410 | −0.9581 | −1.2366 | −0.6489 | |
| Flexion | (Nm) | 0.0135 | 0.0135 | 0.1029 | 0.0218 | 0.1130 | 0.0618 | 0.1406 | 0.6084 |
| (−) | 0.3052 | 0.3052 | 0.4714 | 0.7503 | 0.3929 | 0.5587 | 0.5607 | 0.3949 |
| Model 1 | Objective Functions 2 of the Optimization Sequence | |||||||
|---|---|---|---|---|---|---|---|---|
| RMS C7 | RMS C6 | RMS C5 | RMS C4 | RMS C3 | RMS C2 | RMS C1 | RMS C0 | |
| V1 (Initial model) | A2_C7/T1 B2_C7/T1 C_C7/T1 | A1_C6/C7 B1_C6/C7 C_C6/C7 | A2_C5/C6 B2_C5/C6 C_C5/C6 | A2_C4/C5 B2_C4/C5 C_C4/C5 | A2_C3/C4 B2_C3/C4 C_C3/C4 | A2_C2/C3 B2_C2/C3 C_C2/C3 | A2_C1/C2 B2_C1/C2 C_C1/C2 | A1_C0/C1 B1_C0/C1 B2_C0/C1 C_C0/C1 |
| V2 | A2_C7/T1 B2_C7/T1 C_C7/T1 | A2_C6/C7 B2_C6/C7 C_C6/C7 | A2_C5/C6 B2_C5/C6 C_C5/C6 | A2_C4/C5 B2_C4/C5 C_C4/C5 | A2_C3/C4 B2_C3/C4 C_C3/C4 | A2_C2/C3 B2_C2/C3 C_C2/C3 | A2_C1/C2 B2_C1/C2 C_C1/C2 | B1_C0/C1 A2_C0/C1 B2_C0/C1 C_C0/C1 |
| V3 | A2_C7/T1 B2_C7/T1 C_C7/T1 | A2_C6/C7 B2_C6/C7 C_C6/C7 | A1_C5/C6 A2_C5/C6 B2_C5/C6 C_C5/C6 | A2_C4/C5 B2_C4/C5 C_C4/C5 | A2_C3/C4 B2_C3/C4 | A2_C2/C3 B2_C2/C3 C_C2/C3 | A2_C1/C2 B2_C1/C2 C_C1/C2 | A2_C0/C1 B2_C0/C1 C_C0/C1 |
| V4 | A2_C7/T1 B2_C7/T1 | A2_C6/C7 B2_C6/C7 C_C6/C7 | A2_C5/C6 B2_C5/C6 C_C5/C6 | A2_C4/C5 B2_C4/C5 | A2_C3/C4 B2_C3/C4 C_C3/C4 | A2_C2/C3 B2_C2/C3 C_C2/C3 | A2_C1/C2 B2_C1/C2 C_C1/C2 | A2_C0/C1 B2_C0/C1 C_C0/C1 |
| Model | V1 B | V2 B | V3 B | V4 B |
|---|---|---|---|---|
| Objective Functions | RMS OC | RMS OC | RMS OC | RMS C0 |
| Design Variables | B2_C7/T1 B2_C6/C7 B2_C5/C6 B2_C4/C5 B2_C3/C4 | B2_C7/T1 B2_C6/C7 B2_C5/C6 B2_C4/C5 B2_C3/C4 B2_C2/C3 B2_C1/C2 | A2_C0/C1 B2_C0/C1 |
| Variables | Joints | |||||||
|---|---|---|---|---|---|---|---|---|
| C0/C1 | C1/C2 | C2/C3 | C3/C4 | C4/C5 | C5/C6 | C6/C7 | C7/T1 | |
| (Nm) | −0.0071 | −0.0095 | −0.0037 | −0.0068 | −0.0027 | −0.01225 | −0.0125 | −0.3105 |
| (−) | −0.2215 | −0.3937 | −1.014 | −1.142 | −1.641 | −0.9581 | −1.237 | −0.6489 |
| (Nm) | 0.0159 | 0.0330 | 0.2512 | 0.0433 | 0.2335 | 0.1037 | 0.2057 | 1.322 |
| (−) | 0.4131 | 0.7029 | 0.8907 | 1.173 | 0.7685 | 1.027 | 0.8521 | 1.240 |
| C (Nms/rad) | 2.954 | 4.395 | 1.582 | 1.758 | 3.516 | 4.395 | 3.279 | 3.516 |
| Interval | Models | Injury Criteria | ||||
|---|---|---|---|---|---|---|
| Head | Neck | |||||
| HIC36 | Nep | Nfp | Nea | Nfa | ||
| [0; 300] ms | TNO-HBM | 127.1 | 0.0291 | 0 | 0.1596 | 0.3284 |
| ENM-2D | 137.7 | 0.0509 | 0 | 0.1403 | 0.3159 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Henriques, D.; Martins, A.P.; Carvalho, M.S. Efficient 2D Neck Model for Simulation of the Whiplash Injury Mechanism. Bioengineering 2024, 11, 129. https://doi.org/10.3390/bioengineering11020129
Henriques D, Martins AP, Carvalho MS. Efficient 2D Neck Model for Simulation of the Whiplash Injury Mechanism. Bioengineering. 2024; 11(2):129. https://doi.org/10.3390/bioengineering11020129
Chicago/Turabian StyleHenriques, Diamantino, Ana P. Martins, and Marta S. Carvalho. 2024. "Efficient 2D Neck Model for Simulation of the Whiplash Injury Mechanism" Bioengineering 11, no. 2: 129. https://doi.org/10.3390/bioengineering11020129
APA StyleHenriques, D., Martins, A. P., & Carvalho, M. S. (2024). Efficient 2D Neck Model for Simulation of the Whiplash Injury Mechanism. Bioengineering, 11(2), 129. https://doi.org/10.3390/bioengineering11020129









