Engineering Auxetic Cylinders and Intestine to Improve Longitudinal Intestinal Lengthening and Tailoring Procedure
Abstract
1. Introduction
2. Materials and Methods
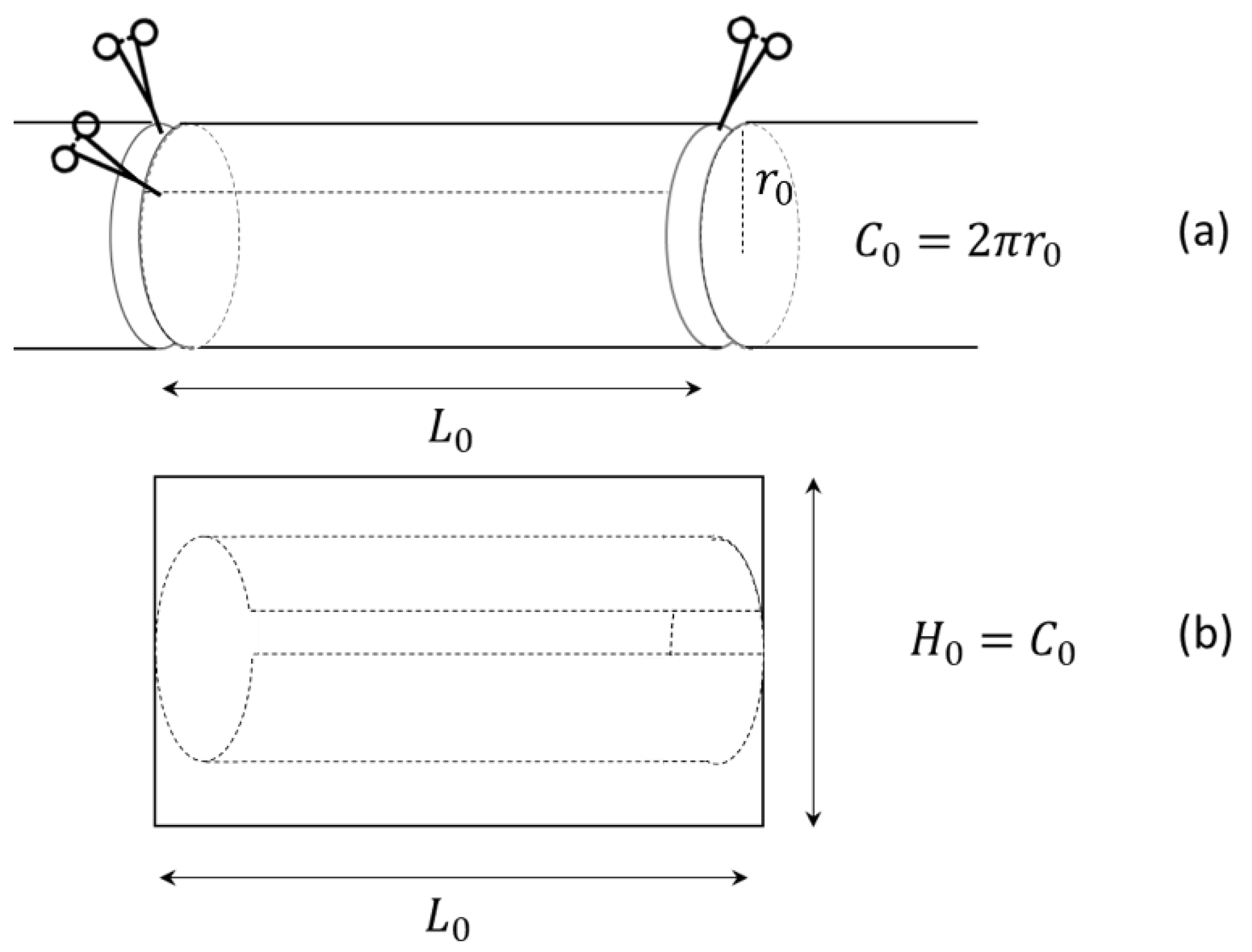
2.1. Fabrication of Auxetic Cylinders
2.2. Characterization
2.3. Finite Element Modelling
2.4. Mathematical Analysis of the LILT Procedure
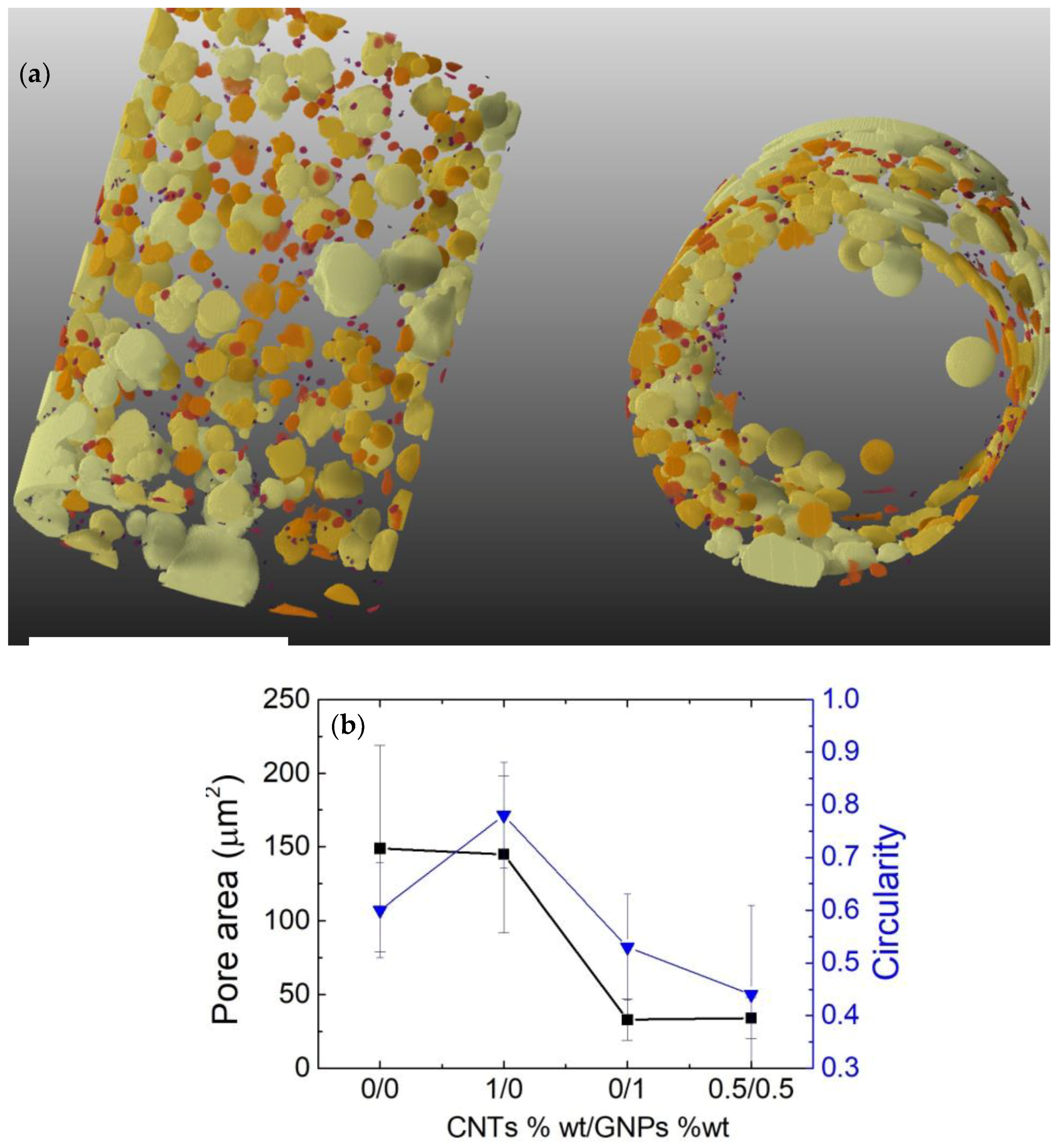
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Evans, K.E.; Alderson, A. Auxetic Materials: Functional Materials and Structures from Lateral Thinking! Adv. Mater. 2000, 12, 617. [Google Scholar] [CrossRef]
- Gibson, L.J.; Ashby, M.F.; Schajer, G.S.; Robertson, C.I. The mechanics of two-dimensional cellular materials. Proc. R. Soc. A 1982, 382, 25. [Google Scholar]
- Greaves, G.N.; Greer, A.; Lakes, R.S.; Rouxel, T. Poisson’s ratio and modern materials. Nat. Mater. 2011, 10, 823. [Google Scholar] [CrossRef] [PubMed]
- Chun, K.Y.; Rho, Y.; Oh, J.; Ahn, J.H.; Kim, Y.Y.; Choi, H.R.; Baik, S. Highly conductive, printable and stretchable composite films of carbon nanotubes and silver. Nat. Nanotechnol. 2010, 5, 853. [Google Scholar] [CrossRef] [PubMed]
- Suhr, J.; Koratkar, N.; Keblinski, P.; Ajayan, P. Viscoelasticity in carbon nanotube composites. Nat. Mater. 2005, 4, 134. [Google Scholar] [CrossRef] [PubMed]
- Lipomi, D.J.; Vosgueritchian, M.; Tee, B.C.K.; Hellstrom, S.L.; Lee, J.A.; Fox, C.H.; Bao, Z.N. Skin-like pressure and strain sensors based on transparent elastic films of carbon nanotubes. Nat. Nanotechnol. 2011, 6, 788. [Google Scholar] [CrossRef]
- Miura, K. Method of packaging and deployment of large membranes in space. Inst. Space Astronaut. Sci. Rep. 1985, 618, 1–9. [Google Scholar]
- Isobe, M.; Okumura, K. Initial rigid response and softening transition of highly stretchable kirigami sheet materials. Sci. Rep. 2016, 6, 24758. [Google Scholar] [CrossRef]
- Cheng, Y.C.; Lu, H.C.; Lee, X.; Zeng, H.; Priimagi, A. Kirigami-Based Light-Induced Shape-Morphing and Locomotion. Adv. Mater. 2020, 32, 1906233. [Google Scholar] [CrossRef]
- Gatt, R.; Attard, D.; Farrugia, P.S.; Azzopardi, K.M.; Mizzi, L.; Brincat, J.P.; Grima, J.N. A realistic generic model for anti-tetrachiral systems. Phys. Status Solidi B 2013, 250, 2012–2019. [Google Scholar] [CrossRef]
- Valentini, L.; Bittolo Bon, S.; Pugno, N.M. Graphene and carbon nanotube auxetic rubber bionic composites with negative variation of the electrical resistance and comparison with their nonbionic counterparts. Adv. Funct. Mater. 2017, 27, 1606526. [Google Scholar] [CrossRef]
- Vilarino, F.; Spyridonos, P.; De Iorio, F.; Vitria, J.; Azpiroz, F.; Radeva, P. Intestinal motility assessment with video capsule endoscopy: Automatic annotation of phasic intestinal contractions. IEEE Trans. Med. Imaging 2010, 29, 246–259. [Google Scholar] [CrossRef] [PubMed]
- Vu, H.; Echigo, T.; Sagawa, R.; Yagi, K.; Shiba, M.; Higuchi, K.; Arakawa, T.; Yagi, Y. Detection of contractions in adaptive transit time of the small bowel from wireless capsule endoscopy videos. Comput. Biol. Med. 2009, 39, 16–26. [Google Scholar] [CrossRef] [PubMed]
- Ganousse-Mazeron, S.; Lacaille, F.; Colomb-Jung, V.; Talbotec, C.; Ruemmele, F.; Sauvat, F.; Chardot, C.; Canioni, D.; Jan, D.; Revillon, Y.; et al. Assessment and outcome of children with intestinal failure referred for intestinal transplantation. Clin. Nutr. 2015, 34, 428–435. [Google Scholar] [CrossRef] [PubMed]
- Abu-Elmagd, K. The concept of gut rehabilitation and the future of visceral transplantation. Nat. Rev. Gastroenterol. Hepatol. 2015, 12, 108–120. [Google Scholar] [CrossRef]
- Coletta, R.; Khalil, B.A.; Morabito, A. Short bowel syndrome in children: Surgical and medical perspectives. Semin. Pediatr. Surg. 2014, 23, 291–297. [Google Scholar] [CrossRef]
- Morabito, A.; Ugolini, S.; Cianci, M.C.; Coletta, R. Current surgical concepts and indications in the management of the short bowel state: A call for the use of multidisciplinary intestinal rehabilitation programs. Children 2021, 29, 654. [Google Scholar] [CrossRef]
- Thompson, J.S.; Langnas, A.N.; Pinch, L.W.; Kaufman, S.; Quigley, E.M.; Vanderhoof, J.A. Surgical Approach to Short-Bowel Syndrome. Experience in a Population of 160 Patients. Ann. Surg. 1995, 222, 600–605. [Google Scholar] [CrossRef]
- Kim, H.B.; Fauza, D.; Garza, J.; Oh, J.T.; Nurko, S.; Jaksic, T. Serial transverse enteroplasty (STEP): A novel bowel lengthening procedure. J. Pediatr. Surg. 2003, 38, 425–429. [Google Scholar] [CrossRef]
- Lauro, A.; Coletta, R.; Morabito, A. Restoring gut physiology in short bowel patients: From bench to clinical application of autologous intestinal reconstructive procedures. Expert Rev. Gastroenterol. Hepatol. 2019, 13, 785–796. [Google Scholar] [CrossRef]
- Matsuoka, M.; Akasaka, T.; Hashimoto, T.; Totsuka, Y.; Watari, F. Improvement in cell proliferation on silicone rubber by carbon nanotube coating. Biomed. Mater. Eng. 2009, 19, 155. [Google Scholar] [CrossRef] [PubMed]
- Barducci, L.; Norton, J.C.; Sarker, S.; Mohammed, S.; Jones, R.; Valdastri, P.; Terry, B.S. Fundamentals of the gut for capsule engineers. Prog. Biomed. Eng. 2020, 2, 042002. [Google Scholar] [CrossRef]
- Strek, T.; Jopek, H. Effective mechanical properties of concentric cylindrical composites with auxetic phase. Phys. Status Solidi B 2012, 249, 1359–1365. [Google Scholar] [CrossRef]
- Garcea, S.C.; Wang, Y.; Withers, P.J. X-ray computed tomography of polymer composites. Compos. Sci. Technol. 2018, 156, 305–319. [Google Scholar] [CrossRef]
- Gapinski, B.; Wieczorowski, M.; Grzelka, M.; Arroyo Alonso, P.; Bermudez Tome, A. The application of micro computed tomography to assess quality of parts manufactured by means of rapid prototyping. Polimery 2017, 62, 53–59. [Google Scholar] [CrossRef]
- Thompson, A.; Leach, R. Introduction to Industrial X-ray Computed Tomography. In Industrial X-ray Computed Tomography; Springer International Publishing: New York, NY, USA, 2018; pp. 1–23. [Google Scholar]
- Valentini, L.; Bittolo Bon, S.; Pugno, N.M. Microorganism nutrition processes as a general route for the preparation of bionic nanocomposites based on intractable polymers. ACS Appl. Mater. Interfaces 2016, 8, 22714–22720. [Google Scholar] [CrossRef]
- Brown, G.O. The history of the Darcy-Weisbach equation for pipe flow resistance. Environ. Water Resour. Hist. 2022, 38, 34–43. [Google Scholar]
- Cecarini, V.; Gogoi, O.; Bonfili, L.; Veneruso, I.; Pacinelli, G.; De Carlo, S.; Benvenuti, F.; D’Argenio, V.; Angeletti, M.; Cannella, N.; et al. Modulation of Gut Microbiota and Neuroprotective Effect of a Yeast-Enriched Beer. Nutrients 2022, 14, 2380. [Google Scholar] [CrossRef]




| Silicone Cylinder | Intestine | |
|---|---|---|
| Young’s modulus [MPa] | 1.8 | 0.1 |
| Density [Kg m−3] | 1000 | 1000 |
| Poisson Coefficient (ν) [-] | From −0.6 to 0.4 with 0.1 step (Poisson Coefficient equal to 0 was not considered) | 0.49 [21] |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Valentini, L.; Chiesa, I.; De Maria, C.; Ugolini, S.; Volpe, Y.; Mussi, E.; Pappalardo, L.; Coletta, R.; Morabito, A. Engineering Auxetic Cylinders and Intestine to Improve Longitudinal Intestinal Lengthening and Tailoring Procedure. Bioengineering 2022, 9, 658. https://doi.org/10.3390/bioengineering9110658
Valentini L, Chiesa I, De Maria C, Ugolini S, Volpe Y, Mussi E, Pappalardo L, Coletta R, Morabito A. Engineering Auxetic Cylinders and Intestine to Improve Longitudinal Intestinal Lengthening and Tailoring Procedure. Bioengineering. 2022; 9(11):658. https://doi.org/10.3390/bioengineering9110658
Chicago/Turabian StyleValentini, Luca, Irene Chiesa, Carmelo De Maria, Sara Ugolini, Yary Volpe, Elisa Mussi, Lucia Pappalardo, Riccardo Coletta, and Antonino Morabito. 2022. "Engineering Auxetic Cylinders and Intestine to Improve Longitudinal Intestinal Lengthening and Tailoring Procedure" Bioengineering 9, no. 11: 658. https://doi.org/10.3390/bioengineering9110658
APA StyleValentini, L., Chiesa, I., De Maria, C., Ugolini, S., Volpe, Y., Mussi, E., Pappalardo, L., Coletta, R., & Morabito, A. (2022). Engineering Auxetic Cylinders and Intestine to Improve Longitudinal Intestinal Lengthening and Tailoring Procedure. Bioengineering, 9(11), 658. https://doi.org/10.3390/bioengineering9110658











