Mass Transport and Turbulent Statistics within Two Branching Coral Colonies
Abstract
:1. Introduction
2. Materials and Methods
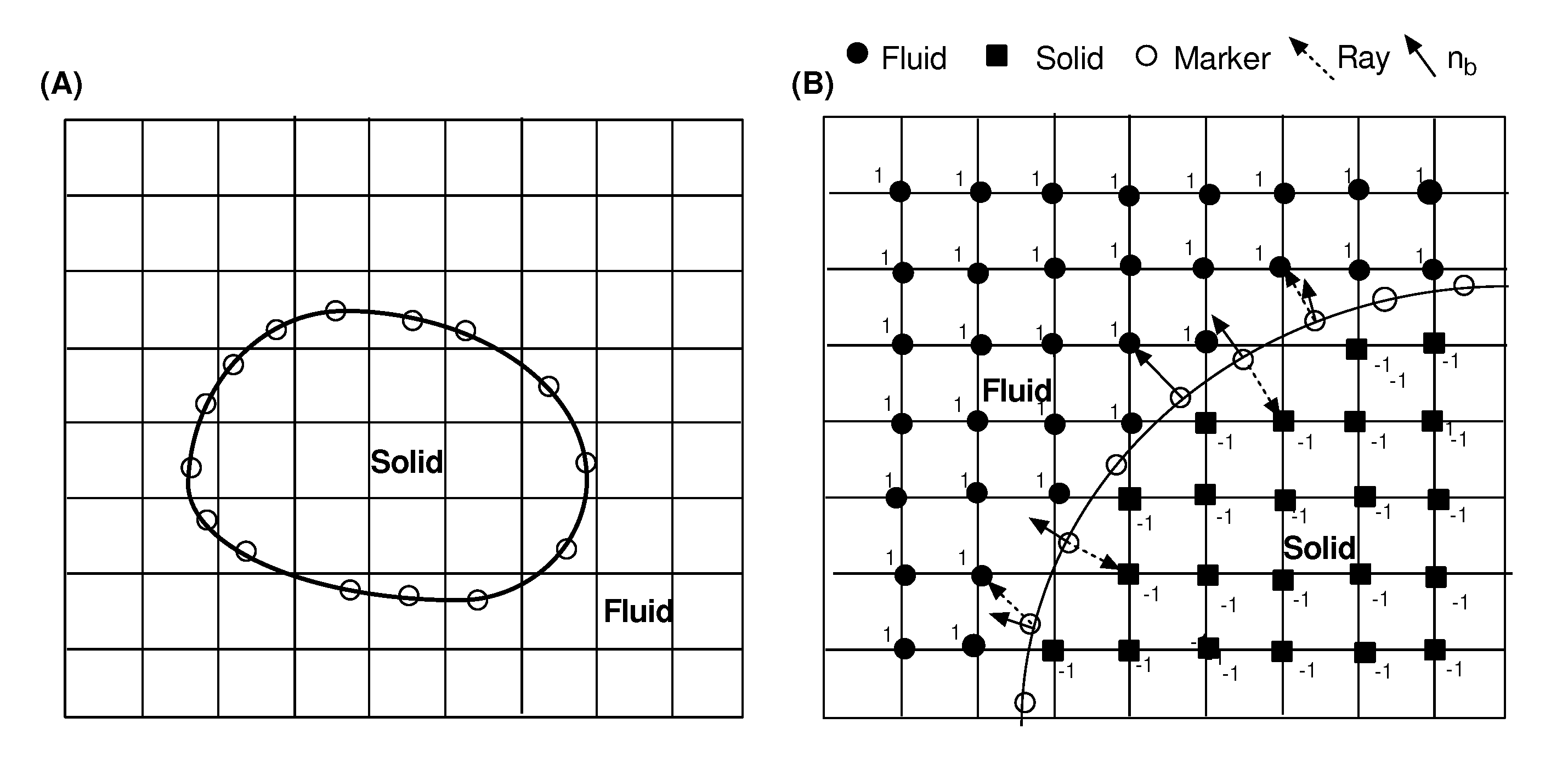
2.1. Immersed Boundary Method with Large Eddy Simulation
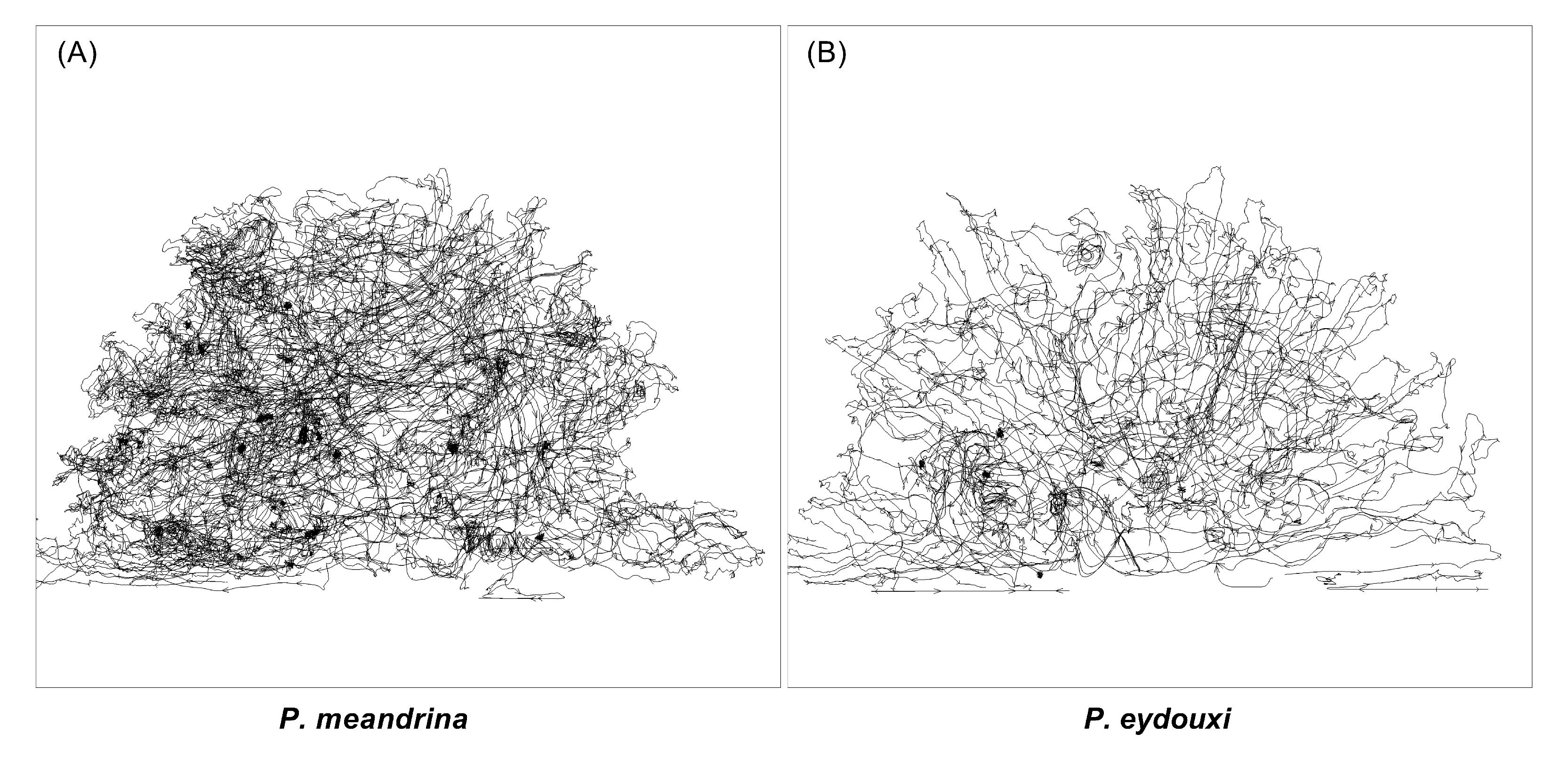
2.2. Coral Geometries
3. Results
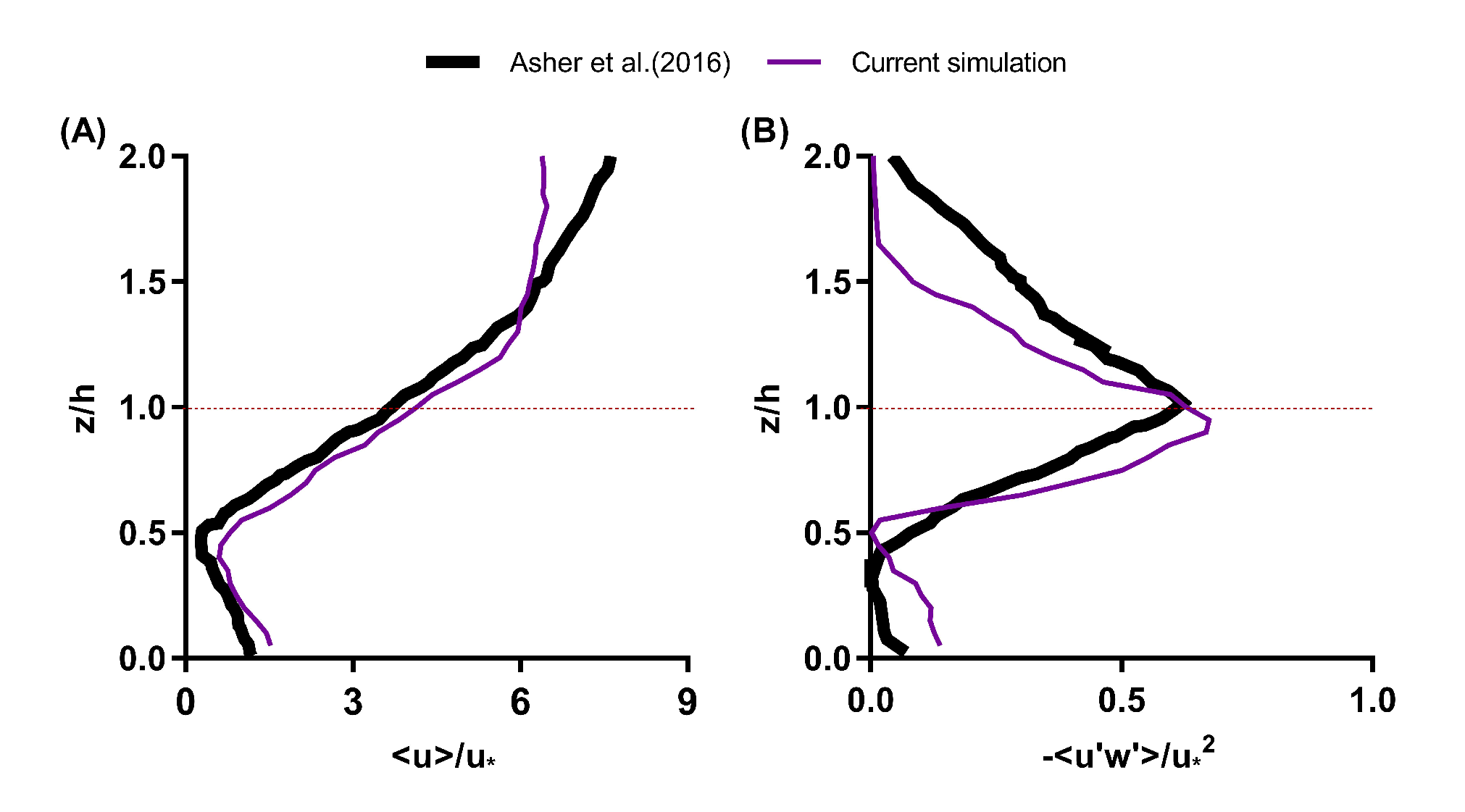
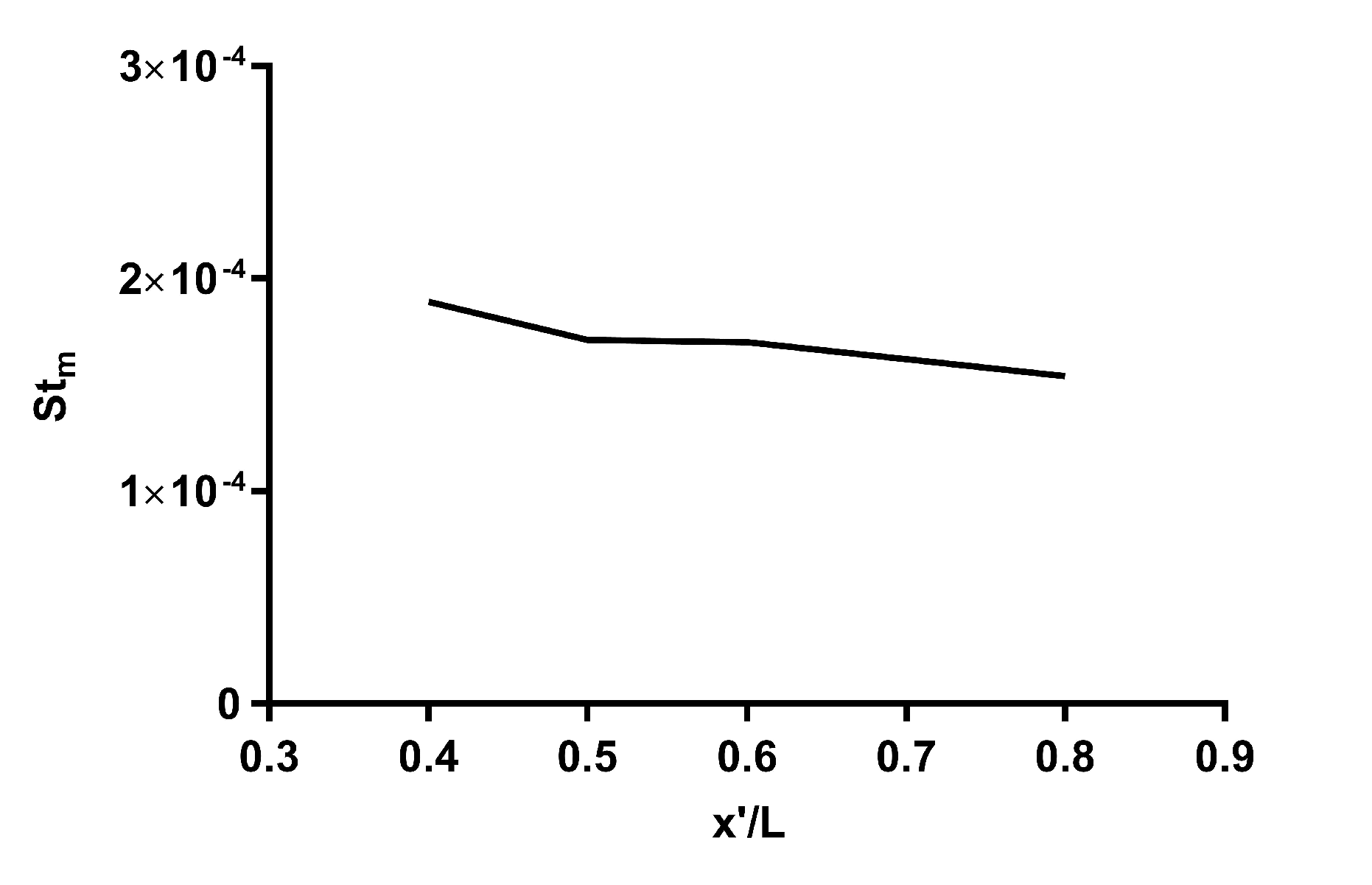
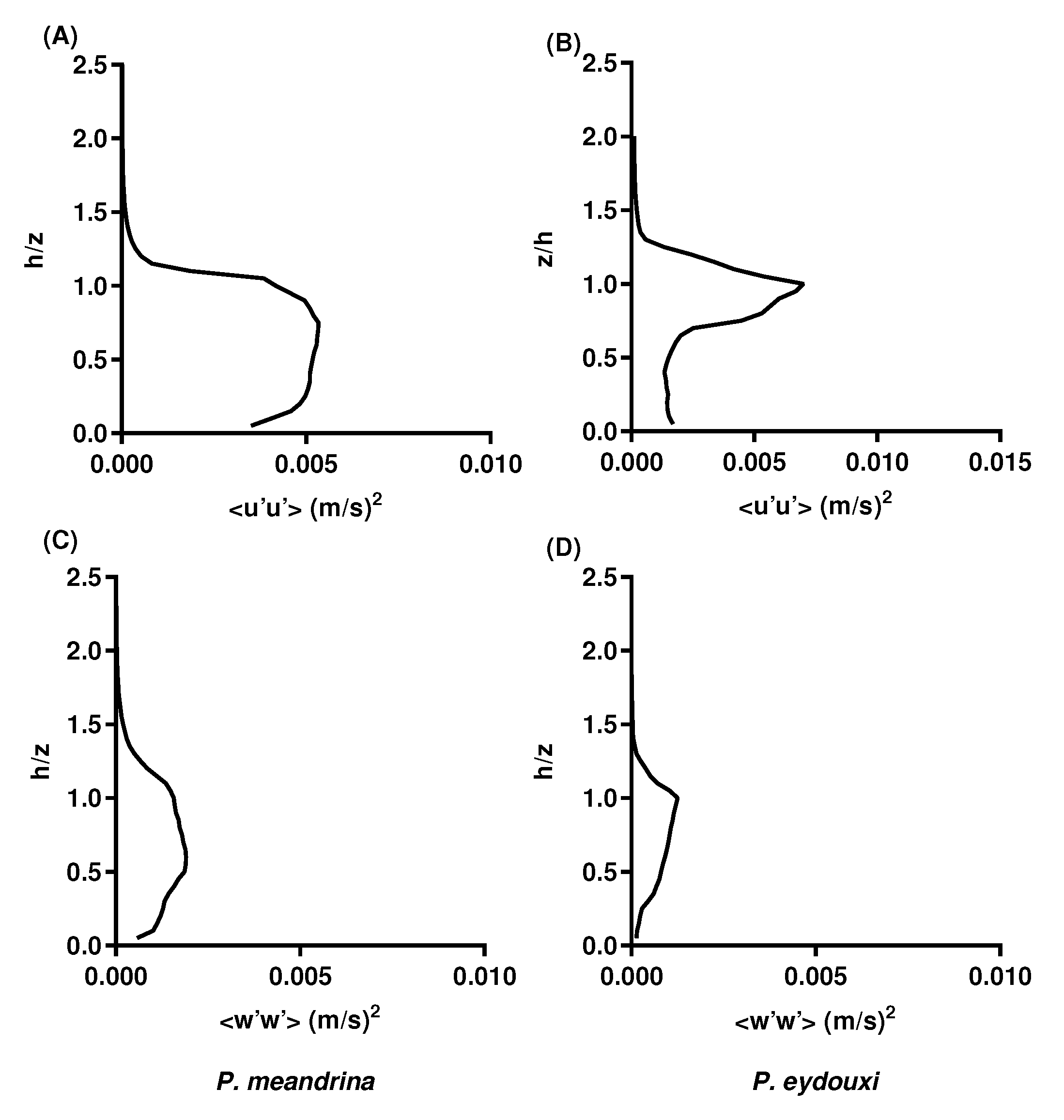
3.1. Validation of the Simulation Results
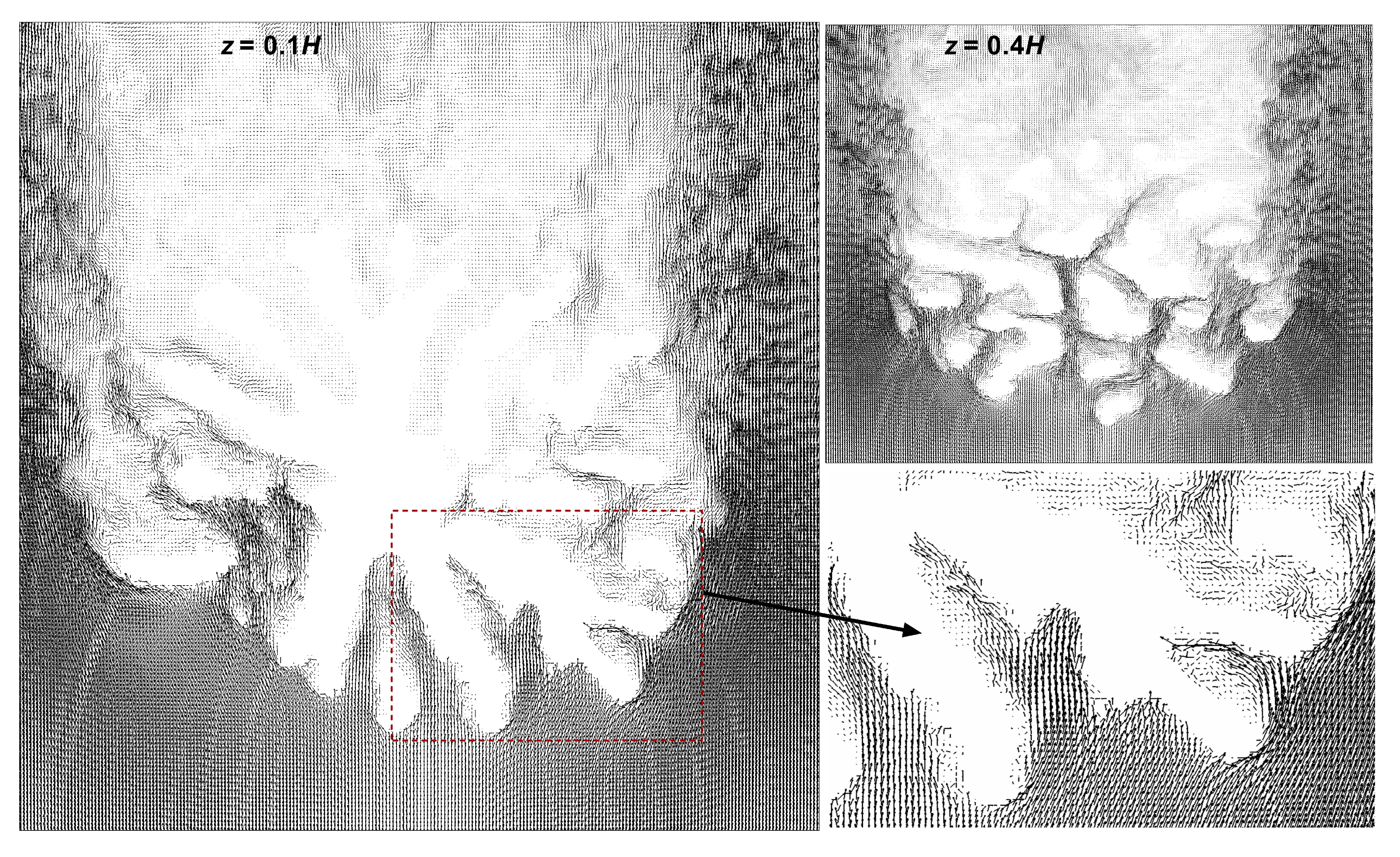
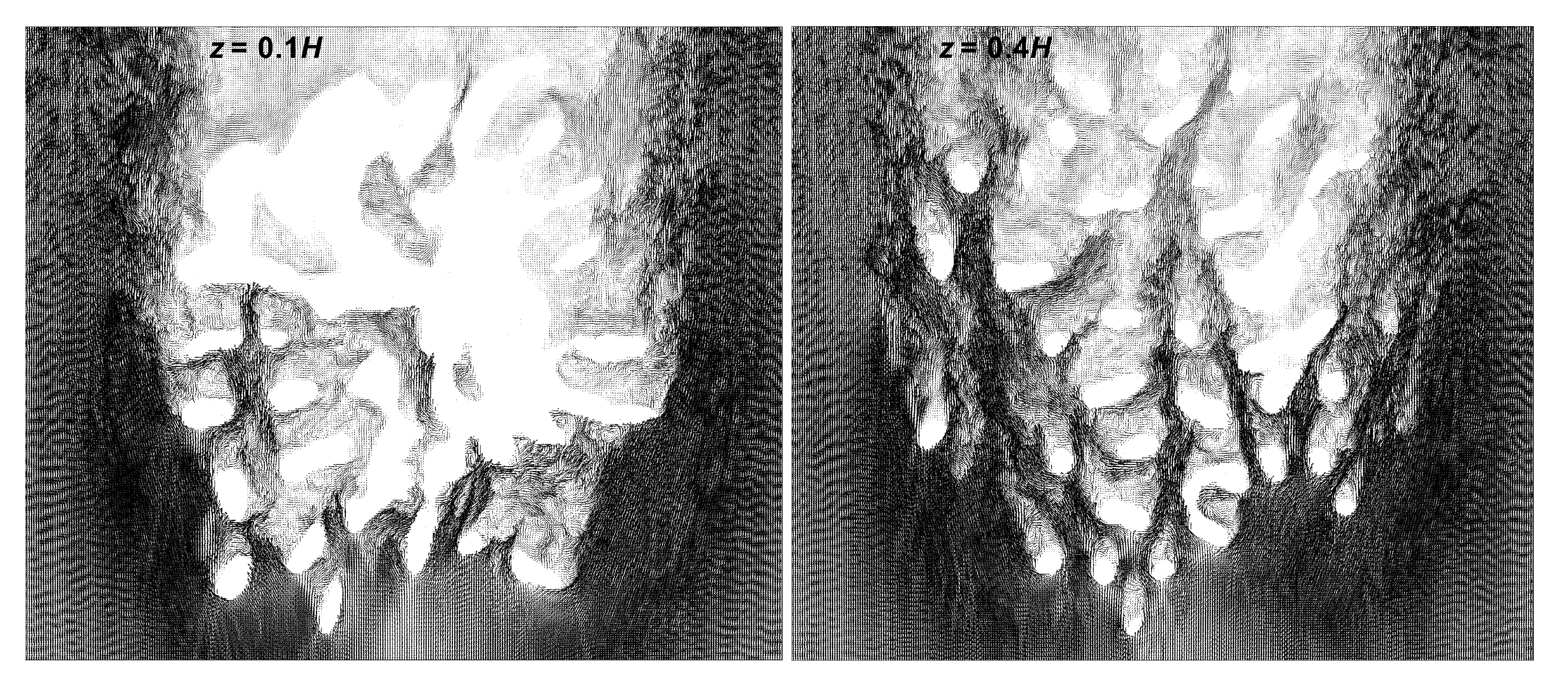
3.2. Comparison of Flow Profiles and Transport Mechanism between Pocillopra Geometries
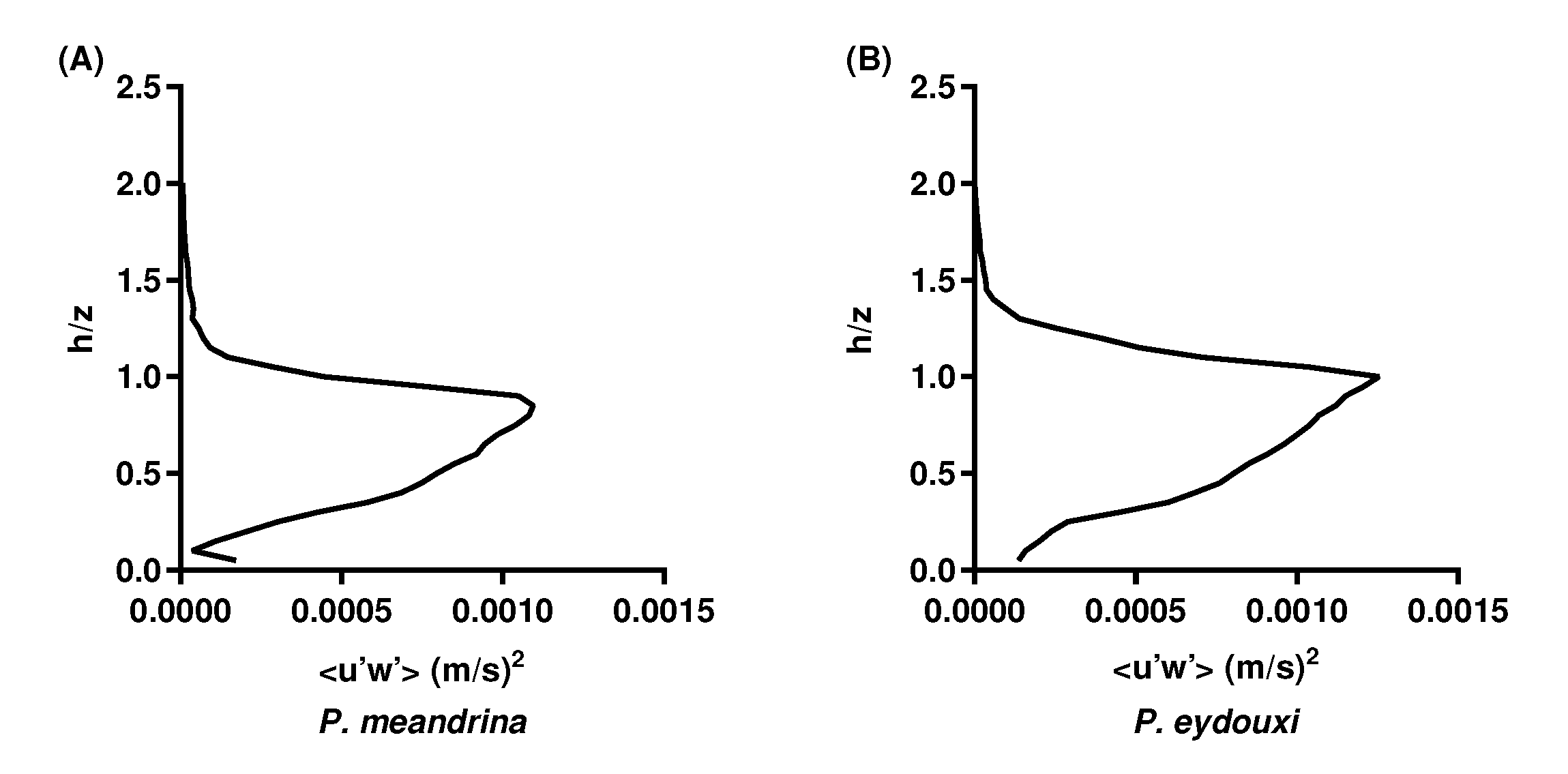
3.3. Turbulent Momentum Flux
4. Discussion
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Fabricius, K.E.; Genin, A.; Benayahu, Y. Flow-dependent herbivory and growth in zooxanthellae-free soft corals. Limnol. Oceanogr. 1995, 40, 1290–1301. [Google Scholar] [CrossRef]
- Mass, T.; Genin, A.; Shavit, U.; Grinstein, M.; Tchernov, D. Flow enhances photosynthesis in marine benthic autotrophs by increasing the efflux of oxygen from the organism to the water. Proc. Natl. Acad. Sci. USA 2010, 107, 2527–2531. [Google Scholar] [CrossRef] [Green Version]
- Hondzo, M. Dissolved oxygen transfer at the sediment-water interface in a turbulent flow. Water Resour. Res. 1998, 34, 3525–3533. [Google Scholar] [CrossRef]
- Chamberlain, J.A., Jr.; Graus, R.R. Water flow and hydromechanical adaptations of branched reef corals. Bull. Mar. Sci. 1975, 25, 112–125. [Google Scholar]
- Sebens, K.P.; Witting, J.; Helmuth, B. Effects of water flow and branch spacing on particle capture by the reef coral Madracis mirabilis (Duchassaing and Michelotti). J. Exp. Mar. Biol. Ecol. 1997, 211, 1–28. [Google Scholar] [CrossRef]
- Hunter, T. Suspension feeding in oscillating flow: The effect of colony morphology and flow regime on plankton capture by the hydroid Obelia longissima. Biol. Bull. 1989, 176, 41–49. [Google Scholar] [CrossRef]
- Mass, T.; Genin, A. Environmental versus intrinsic determination of colony symmetry in the coral Pocillopora verrucosa. Mar. Ecol. Prog. Ser. 2008, 369, 131–137. [Google Scholar] [CrossRef]
- Kaandorp, J.A.; Kübler, J.E. The Algorithmic Beauty of Seaweeds, Sponges and Corals; Springer: Berlin, Germany, 2001. [Google Scholar]
- Patterson, M.R.; Sebens, K.P.; Olson, R.R. In situ measurements of flow effects on primary production and dark respiration in reef corals. Limnol. Oceanogr. 1991, 36, 936–948. [Google Scholar] [CrossRef]
- Shashar, N.; Kinane, S.; Jokiel, P.; Patterson, M. Hydromechanical boundary layers over a coral reef. J. Exp. Mar. Biol. Ecol. 1996, 199, 17–28. [Google Scholar] [CrossRef]
- Larkum, A.W.; Koch, E.M.; Kühl, M. Diffusive boundary layers and photosynthesis of the epilithic algal community of coral reefs. Mar. Biol. 2003, 142, 1073–1082. [Google Scholar] [CrossRef]
- Bruno, J.F.; Edmunds, P.J. Metabolic consequences of phenotypic plasticity in the coral Madracis mirabilis (Duchassaing and Michelotti): The effect of morphology and water flow on aggregate respiration. J. Exp. Mar. Biol. Ecol. 1998, 229, 187–195. [Google Scholar] [CrossRef]
- Jimenez, I.M.; Kühl, M.; Larkum, A.W.; Ralph, P.J. Heat budget and thermal microenvironment of shallow-water corals: Do massive corals get warmer than branching corals? Limnol. Oceanogr. 2008, 53, 1548–1561. [Google Scholar] [CrossRef] [Green Version]
- Chan, N.; Wangpraseurt, D.; Kühl, M.; Connolly, S.R. Flow and coral morphology control coral surface pH: Implications for the effects of ocean acidification. Front. Mar. Sci. 2016, 3, 10. [Google Scholar] [CrossRef]
- Reidenbach, M.A.; Koseff, J.R.; Monismith, S.G. Laboratory experiments of fine-scale mixing and mass transport within a coral canopy. Phys. Fluids 2007, 19, 075107. [Google Scholar] [CrossRef]
- Stocking, J.B.; Rippe, J.P.; Reidenbach, M.A. Structure and dynamics of turbulent boundary layer flow over healthy and algae-covered corals. Coral Reefs 2016, 35, 1047–1059. [Google Scholar] [CrossRef]
- Hench, J.L.; Rosman, J.H. Observations of spatial flow patterns at the coral colony scale on a shallow reef flat. J. Geophys. Res. Ocean. 2013, 118, 1142–1156. [Google Scholar] [CrossRef] [Green Version]
- Pomeroy, A.W.; Lowe, R.J.; Ghisalberti, M.; Storlazzi, C.; Symonds, G.; Roelvink, D. Sediment transport in the presence of large reef bottom roughness. J. Geophys. Res. Ocean. 2017, 122, 1347–1368. [Google Scholar] [CrossRef]
- Sous, D.; Bouchette, F.; Doerflinger, E.; Meulé, S.; Certain, R.; Toulemonde, G.; Dubarbier, B.; Salvat, B. On the small-scale fractal geometrical structure of a living coral reef barrier. Earth Surf. Process. Landf. 2020. [Google Scholar] [CrossRef]
- Asher, S.; Niewerth, S.; Koll, K.; Shavit, U. Vertical variations of coral reef drag forces. J. Geophys. Res. Ocean. 2016, 121, 3549–3563. [Google Scholar] [CrossRef] [Green Version]
- Chang, S.; Elkins, C.; Alley, M.; Eaton, J.; Monismitha, S. Flow inside a coral colony measured using magnetic resonance velocimetry. Limnol. Oceanogr. 2009, 54, 1819–1827. [Google Scholar] [CrossRef]
- Chang, S.; Iaccarino, G.; Ham, F.; Elkins, C.; Monismith, S. Local shear and mass transfer on individual coral colonies: Computations in unidirectional and wave-driven flows. J. Geophys. Res. Ocean. 2014, 119, 2599–2619. [Google Scholar] [CrossRef]
- Shapiro, O.H.; Fernandez, V.I.; Garren, M.; Guasto, J.S.; Debaillon-Vesque, F.P.; Kramarsky-Winter, E.; Vardi, A.; Stocker, R. Vortical ciliary flows actively enhance mass transport in reef corals. Proc. Natl. Acad. Sci. USA 2014, 111, 13391–13396. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Hossain, M.M.; Staples, A.E. Passive vortical flows enhance mass transport in the interior of a coral colony. Phys. Fluids 2019, 31, 061701. [Google Scholar] [CrossRef]
- Atkinson, M.; Bilger, R. Effects of water velocity on phosphate uptake in coral reef-hat communities. Limnol. Oceanogr. 1992, 37, 273–279. [Google Scholar] [CrossRef]
- Bilger, R.; Atkinson, M. Anomalous mass transfer of phosphate on coral reef flats. Limnol. Oceanogr. 1992, 37, 261–272. [Google Scholar] [CrossRef]
- Chang, S.; Elkins, C.; Eaton, J.K.; Monismith, S. Local mass transfer measurements for corals and other complex geometries using gypsum dissolution. Exp. Fluids 2013, 54, 1563. [Google Scholar] [CrossRef]
- Reidenbach, M.A.; Koseff, J.R.; Monismith, S.G.; Steinbuckc, J.V.; Genin, A. The effects of waves and morphology on mass transfer within branched reef corals. Limnol. Oceanogr. 2006, 51, 1134–1141. [Google Scholar] [CrossRef]
- Reidenbach, M.A.; Monismith, S.G.; Koseff, J.R.; Yahel, G.; Genin, A. Boundary layer turbulence and flow structure over a fringing coral reef. Limnol. Oceanogr. 2006, 51, 1956–1968. [Google Scholar] [CrossRef]
- Asher, S.; Shavit, U. The effect of water depth and internal geometry on the turbulent flow inside a coral reef. J. Geophys. Res. Ocean. 2019, 124, 3508–3522. [Google Scholar] [CrossRef]
- Nepf, H.; Vivoni, E. Flow structure in depth-limited, vegetated flow. J. Geophys. Res. Ocean. 2000, 105, 28547–28557. [Google Scholar] [CrossRef]
- Finnigan, J. Turbulence in plant canopies. Annu. Rev. Fluid Mech. 2000, 32, 519–571. [Google Scholar] [CrossRef]
- Moltchanov, S.; Bohbot-Raviv, Y.; Shavit, U. Dispersive stresses at the canopy upstream edge. Bound.-Layer Meteorol. 2011, 139, 333–351. [Google Scholar] [CrossRef]
- Rosman, J.H.; Hench, J.L. A framework for understanding drag parameterizations for coral reefs. J. Geophys. Res. Ocean. 2011, 116. [Google Scholar] [CrossRef] [Green Version]
- Stocking, J.B.; Laforsch, C.; Sigl, R.; Reidenbach, M.A. The role of turbulent hydrodynamics and surface morphology on heat and mass transfer in corals. J. R. Soc. Interface 2018, 15, 20180448. [Google Scholar] [CrossRef] [Green Version]
- Osorio-Cano, J.D.; Osorio, A.F.; Alcérreca-Huerta, J.C.; Oumeraci, H. Drag and inertia forces on a branched coral colony of Acropora palmata. J. Fluids Struct. 2019, 88, 31–47. [Google Scholar] [CrossRef]
- Kaandorp, J.A.; Koopman, E.A.; Sloot, P.M.; Bak, R.P.; Vermeij, M.J.; Lampmann, L.E. Simulation and analysis of flow patterns around the scleractinian coral Madracis mirabilis (Duchassaing and Michelotti). Philos. Trans. R. Soc. Lond. Ser. B Biol. Sci. 2003, 358, 1551–1557. [Google Scholar] [CrossRef] [Green Version]
- Kaandorp, J.A.; Sloot, P.M.; Merks, R.M.; Bak, R.P.; Vermeij, M.J.; Maier, C. Morphogenesis of the branching reef coral Madracis mirabilis. Proc. R. Soc. B Biol. Sci. 2005, 272, 127–133. [Google Scholar] [CrossRef] [Green Version]
- Chindapol, N.; Kaandorp, J.A.; Cronemberger, C.; Mass, T.; Genin, A. Modelling growth and form of the scleractinian coral Pocillopora verrucosa and the influence of hydrodynamics. PLoS Comput. Biol. 2013, 9, e1002849. [Google Scholar] [CrossRef] [Green Version]
- Meneveau, C.; Lund, T.S.; Cabot, W.H. A Lagrangian dynamic subgrid-scale model of turbulence. J. Fluid Mech. 1996, 319, 353–385. [Google Scholar] [CrossRef] [Green Version]
- Bailey, B.N.; Stoll, R. Turbulence in sparse, organized vegetative canopies: A large-eddy simulation study. Bound.-Layer Meteorol. 2013, 147, 369–400. [Google Scholar] [CrossRef]
- Peskin, C.S. The immersed boundary method. Acta Numer. 2002, 11, 479–517. [Google Scholar] [CrossRef] [Green Version]
- Mohd-Yusof, J. methods for complex geometries. Annu. Res. Briefs 1998, 325. [Google Scholar]
- Fadlun, E.; Verzicco, R.; Orlandi, P.; Mohd-Yusof, J. Combined immersed-boundary finite-difference methods for three-dimensional complex flow simulations. J. Comput. Phys. 2000, 161, 35–60. [Google Scholar] [CrossRef]
- Balaras, E. Modeling complex boundaries using an external force field on fixed Cartesian grids in large-eddy simulations. Comput. Fluids 2004, 33, 375–404. [Google Scholar] [CrossRef]
- Yang, J.; Balaras, E. An embedded-boundary formulation for large-eddy simulation of turbulent flows interacting with moving boundaries. J. Comput. Phys. 2006, 215, 12–40. [Google Scholar] [CrossRef] [Green Version]
- Lowe, R.J.; Koseff, J.R.; Monismith, S.G.; Falter, J.L. Oscillatory flow through submerged canopies: 2. Canopy mass transfer. J. Geophys. Res. Ocean. 2005, 110. [Google Scholar] [CrossRef] [Green Version]
- Jørgensen, B.B.; Revsbech, N.P. Diffusive boundary layers and the oxygen uptake of sediments and detritus 1. Limnol. Oceanogr. 1985, 30, 111–122. [Google Scholar] [CrossRef]
- Hossain, M.M.; Staples, A.E. Effects of coral colony morphology on turbulent flow dynamics. bioRxiv 2019, 839902. [Google Scholar] [CrossRef]
- Lowe, R.J.; Shavit, U.; Falter, J.L.; Koseff, J.R.; Monismith, S.G. Modeling flow in coral communities with and without waves: A synthesis of porous media and canopy flow approaches. Limnol. Oceanogr. 2008, 53, 2668–2680. [Google Scholar] [CrossRef]
- Pokrajac, D.; Finnigan, J.; Manes, C.; McEwan, I.; Nikora, V. On the definition of the shear velocity in rough bed open channel flows. River Flow 2006, 1, 89–98. [Google Scholar]
- Jiro. GRABIT. Available online: https://www.mathworks.com/matlabcentral/fileexchange/7173-grabit (accessed on 3 September 2020).
- Langdon, C.; Takahashi, T.; Sweeney, C.; Chipman, D.; Goddard, J.; Marubini, F.; Aceves, H.; Barnett, H.; Atkinson, M.J. Effect of calcium carbonate saturation state on the calcification rate of an experimental coral reef. Glob. Biogeochem. Cycles 2000, 14, 639–654. [Google Scholar] [CrossRef]
- Poggi, D.; Katul, G. The ejection-sweep cycle over bare and forested gentle hills: A laboratory experiment. Bound.-Layer Meteorol. 2007, 122, 493–515. [Google Scholar] [CrossRef]
- Finnigan, J.J.; Shaw, R.H.; Patton, E.G. Turbulence structure above a vegetation canopy. J. Fluid Mech. 2009, 637, 387–424. [Google Scholar] [CrossRef] [Green Version]
- Nepf, H.M. Flow and transport in regions with aquatic vegetation. Annu. Rev. Fluid Mech. 2012, 44, 123–142. [Google Scholar] [CrossRef] [Green Version]
- Pacherres, C.O.; Ahmerkamp, S.; Schmidt-Grieb, G.M.; Holtappels, M.; Richter, C. Ciliary vortex flows and oxygen dynamics in the coral boundary layer. Sci. Rep. 2020, 10, 1–10. [Google Scholar] [CrossRef]

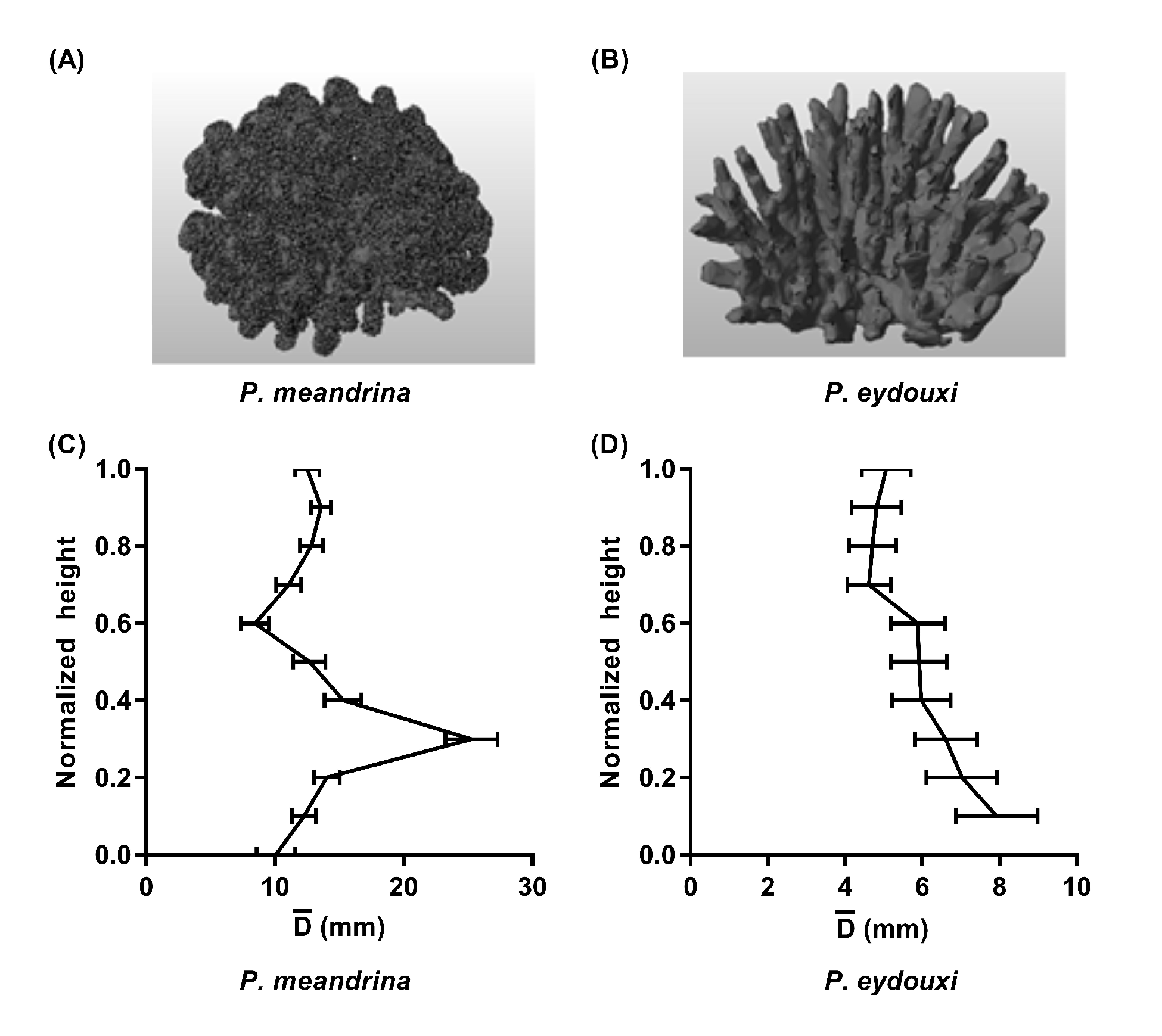


| Colony | Length (m) | Width (m) | Height (m) | Volume/Surface Area | Interbranch Distance/Diameter |
|---|---|---|---|---|---|
| P. meandrina | to | ||||
| P. eydouxi | 8 |
| Colony | (m) | (m/s) | Diameter (m) | (s) | St | Increase (%) |
|---|---|---|---|---|---|---|
| P. meandrina (*) | L | |||||
| P. meandrina (†) | L | |||||
| L | ||||||
| L | ||||||
| L | ||||||
| P. eydouxi (*) | L |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hossain, M.M.; Staples, A.E. Mass Transport and Turbulent Statistics within Two Branching Coral Colonies. Fluids 2020, 5, 153. https://doi.org/10.3390/fluids5030153
Hossain MM, Staples AE. Mass Transport and Turbulent Statistics within Two Branching Coral Colonies. Fluids. 2020; 5(3):153. https://doi.org/10.3390/fluids5030153
Chicago/Turabian StyleHossain, Md Monir, and Anne E. Staples. 2020. "Mass Transport and Turbulent Statistics within Two Branching Coral Colonies" Fluids 5, no. 3: 153. https://doi.org/10.3390/fluids5030153
APA StyleHossain, M. M., & Staples, A. E. (2020). Mass Transport and Turbulent Statistics within Two Branching Coral Colonies. Fluids, 5(3), 153. https://doi.org/10.3390/fluids5030153






