The Effect of Magnetically Induced Local Structure and Volume Fraction on the Electromagnetic Properties of Elastomer Samples with Ferrofluid Droplet Inserts
Abstract
:1. Introduction
2. Obtaining and Characterizing Samples
- -
- For samples A0 and Ah: MFM = 0.05 g and MSR = 4 g (2 g of each component of the silicon rubber, A and B);
- -
- For samples B0 and Bh: MFM = 0.10 g and MSR = 4 g (2 g of each component of the silicon rubber, A and B);
- -
- For samples C0 and Ch: MFM = 0.15 g and MSR = 4 g (2 g of each component of the silicon rubber, A and B).
3. Results and Discussion
3.1. Investigation of Complex Effective Magnetic Permeability
3.2. Investigation of Complex Dielectric Permittivity
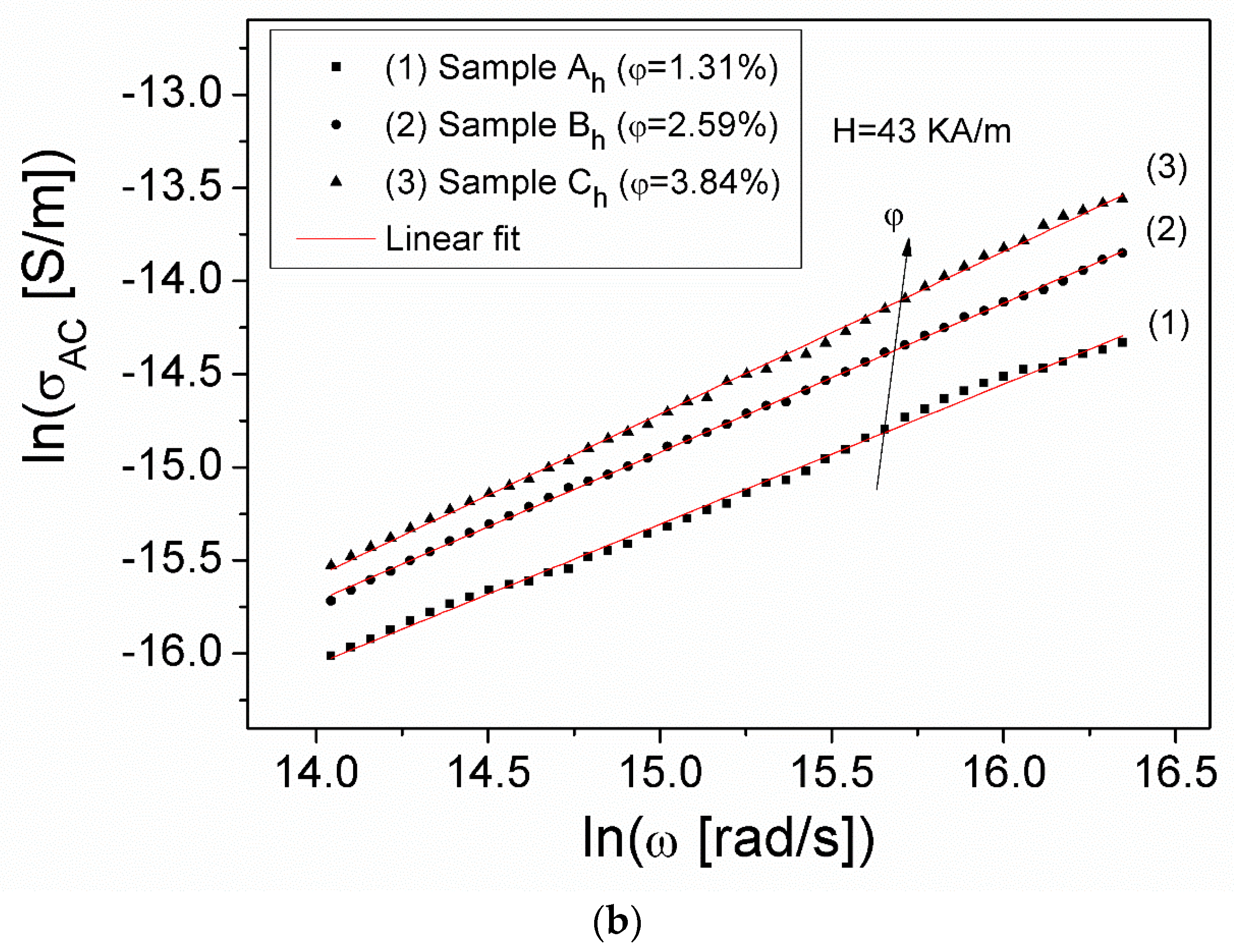
3.3. DC and AC Conductivity
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Choi, S.; Han, S.I.; Kim, D.; Hyeon, T.; Kim, D.H. High-performance stretchable conductive nanocomposites: Materials, processes, and device applications. Chem. Soc. Rev. 2019, 48, 1566–1595. [Google Scholar] [CrossRef] [PubMed]
- Wang, B.; Thukral, A.; Xie, Z.; Liu, L.; Zhang, X.; Huang, W.; Yu, X.; Yu, C.; Marks, T.J.; Facchetti, A. Flexible and stretchable metal oxide nanofiber networks for multimodal and monolithically integrated wearable electronics. Nat. Commun. 2020, 11, 2405–2420. [Google Scholar] [CrossRef] [PubMed]
- Cao, J.; Ling, Z.; Lin, X.; Wu, Y.; Fang, X.; Zhang, Z. Flexible composite phase change material with enhanced thermophysical, dielectric, and mechanical properties for battery thermal management. J. Energy Storage 2022, 52 Pt A, 104796. [Google Scholar] [CrossRef]
- Namkoong, M.; Guo, H.; Rahman, M.S.; Wang, D.; Pfeil, C.J.; Hager, S.; Tian, L. Moldable and transferable conductive nanocomposites for epidermal electronics. NPJ Flex Electron. 2022, 6, 41. [Google Scholar] [CrossRef] [PubMed]
- Zhang, C.; Liu, S.; Wei, F.; Dong, L.; Zhao, D.; Ou, Y. Magnetodielectric Properties of Ordered Microstructured Polydimethylsiloxane-Based Magnetorheological Elastomer with Fe3O4@rGO Nanoparticles. Polymers 2023, 15, 941. [Google Scholar] [CrossRef] [PubMed]
- Kurahatti, R.V.; Surendranathan, A.O.; Kori, S.A.; Singh, N.; Ramesh Kumar, A.V.; Srivastava, S. Defence applications of polymer nanocomposites. Def. Sci. J. 2010, 60, 551–563. [Google Scholar] [CrossRef]
- Hammami, H.; Arous, M.; Lagache Mand Kallel, A. Study of the interfacial MWS relaxation by dielectric spectroscopy in unidirectional PZT fibres/epoxy resin composites. J. Alloys Compd. 2007, 430, 1–8. [Google Scholar] [CrossRef]
- Jamal, E.M.A.; Joy, P.A.; Kurian, P.; Anantharaman, M.R. Synthesis of nickel-rubber nanocomposites and evaluation of their dielectric properties. Mat. Sci. Eng. B 2009, 156, 24–31. [Google Scholar] [CrossRef]
- Yakovenko, O.S.; Yu, L.; Matzui, L.; Vovchenko, L.; Lozitsky, O.V.; Prokopov, O.I.; Lazarenko, O.A.; Zhuravkov, A.V.; Oliynyk, V.V.; Launets, V.L.; et al. Electrophysical properties of epoxy-based composites with graphite nanoplatelets and magnetically aligned magnetite. Mol. Cryst. Liq. Cryst. 2018, 661, 68–80. [Google Scholar] [CrossRef]
- Yakovenko, O.S.; Yu, L.; Matzui, L.; Vovchenko, L.; Oliynyk, V.V.; Trukhanov, A.V.; Trukhanov, S.V.; Borovoy, M.O.; Tesel’ko, P.O.; Launets, V.L.; et al. Effect of magnetic fillers and their orientation on the electrodynamic properties of BaFe12−xGaxO19 (x = 0.1–1.2)—Epoxy composites with carbon nanotubes within GHz range. Appl. Nanosci. 2020, 10, 4747–4752. [Google Scholar] [CrossRef]
- Mohseni, F.; Pullar, C.R.; Vieira, J.M.; Joao, S.; Amaral, J.S. Bonded ferrite-based exchange-coupled nanocomposite magnet produced by Warm compaction. J. Phys. D Appl. Phys. 2020, 53, 494003. [Google Scholar] [CrossRef]
- Bica, I. The obtaining of magneto-rheological suspensions based on silicon oil and iron particles. Mat. Sci. Eng. B 2003, 98, 89–93. [Google Scholar] [CrossRef]
- Bunoiu, M.; Bica, I. Magnetorheological elastomer based on silicone rubber, carbonyl iron and Rochelle salt: Effects of alternating electric and static magnetic fields intensities. J. Ind. Eng. Chem. 2016, 37, 312–318. [Google Scholar] [CrossRef]
- Bica, I.; Anitas, E.M.; Averis, L.M.E.; Bunoiu, M. Magnetodielectric effects in composite materials based on paraffin, carbonyl iron and graphene. J. Ind. Eng. Chem. 2015, 21, 1323–1327. [Google Scholar] [CrossRef]
- Vinnik, D.A.; Podgornov, F.V.; Zabeivorota, N.S.; Trofimov, E.A.; Zhivulin, V.E.; Chernukha, A.S.; Gavrilyak, M.V.; Gudkova, S.A.; Zherebtsov, D.A.; Ryabov, A.V.; et al. Effect of treatment conditions on structure and magnetodielectric properties of barium. J. Magn. Magn. Mater. 2020, 498, 166190. [Google Scholar] [CrossRef]
- Kozlovskiy, A.L.; Kenzhina, I.E.; Zdorovets, M.V. FeCo–Fe2CoO4/Co3O4 nanocomposites: Phase transformations as a result of thermal annealing and practical application in catalysis. Ceram. Int. 2020, 46 Pt A, 10262–10269. [Google Scholar] [CrossRef]
- Rosensweig, R.E. Ferrohydrodynamics; Cambridge University Press: Cambridge, UK, 1985. [Google Scholar]
- Rajnak, M.; Timko, M.; Kopcansky, P.; Paulovicova, K.; Tothova, J.; Kurimsky, J.; Dolnik, B.; Cimbala, R.; Avdeev, M.V.; Petrenko, V.I.; et al. Structure and viscosity of a transformer oil-based ferrofluid under an external electric field. J. Magn. Magn. Mater. 2017, 431, 99–102. [Google Scholar] [CrossRef]
- Timko, M.; Marton, K.; Tomco, L.; Kiraly, J.; Molcan, M.; Rajnak, M.; Kopcansky, P.; Cimbala, R.; Stoian, F.; Holotescu, S.; et al. Magneto-dielectric properties of transformer oil based magnetic fluids in the frequency range up to 2 MHz. Magnetohydrodynamics 2012, 48, 427–434. [Google Scholar]
- Prochima. Rtv-530 Silicon Rubber Moudable. Available online: http://www.prochima.com/ENG/product.asp?id=7 (accessed on 21 August 2023).
- FerroTec. EFH Series Educational Ferrofluid Type: EFH1. Available online: https://ferrofluid.ferrotec.com/products/ferrofluid-educational-fluid/efh/efh1/ (accessed on 21 August 2023).
- Al-Ghamdi, H.; Hemily, H.M.; Saleh, I.H.; Ghataas, Z.F.; Abdel-Halim, A.A.; Sayyed, M.I.; Yasmin, S.; Almuqrin, A.H.; Elsafi, M. Impact of WO3-Nanoparticles on Silicone Rubber for Radiation Protection Efficiency. Materials 2022, 15, 5706. [Google Scholar] [CrossRef]
- Ercuta, A. Sensitive AC hysteresigraph of extended driving field capability. IEEE Trans. Instrum. Measur. 2020, 69, 1643–1651. [Google Scholar] [CrossRef]
- Chantrell, R.W.; Popplewell, J.; Charles, S.W. Measurements of particle size distribution parameters in ferrofluids. IEEE Trans. Magn. 1978, 14, 975. [Google Scholar] [CrossRef]
- Barsoukov, E.; Macdonald, J.R. Impedance Spectroscopy: Theory, Experiments and Applications; Wiley: New York, NY, USA, 2005. [Google Scholar]
- Debye, P. Polar Molecules; The Chemical Catalog Company: New York, NY, USA, 1929. [Google Scholar]
- Odenbach, S. (Ed.) Ferrofluids; Lecture Notes in Physics Series; Springer: Berlin/Heidelberg, Germany, 2002; Volume 594. [Google Scholar]
- Fannin, P.C.; Charles, S.W. The study of a ferrofluid exhibiting both Brownian and Neel relaxation. J. Phys. D Appl. Phys. 1989, 22, 187–191. [Google Scholar] [CrossRef]
- Bickford, L.R. Ferromagnetic Resonance Absorption in Magnetite Single Crystals. Phys. Rev. 1950, 78, 449. [Google Scholar] [CrossRef]
- Malaescu, I.; Marin, C.N. Study of magnetic fluids by means of magnetic spectroscopy. Phys. B Cond. Matter. 2005, 365, 134–140. [Google Scholar] [CrossRef]
- Malaescu, I.; Bunoiu, M.O.; Teusdea, A.; Marin, C.N. Investigations on the electrical conductivity and complex dielectric permittivity of a ferrofluid subjected to the action of a polarizing magnetic field. Mat. Res. Bull. 2023, 164, 112281. [Google Scholar] [CrossRef]
- ASTM D150-98; Standard Test Methods for AC Loss Characteristics and permittivity (Dielectric Constant) of Solid Electrical Insulation. ANSI: Washington, DC, USA, 2004.
- Scaife, B.K.P. Principles of Dielectrics; Oxford Clarendon Press: Oxford, UK, 1998. [Google Scholar]
- Marin, C.N.; Fannin, P.C.; Malaescu, I.; Matu, G. Macroscopic and microscopic electrical properties of a ferrofluid in a low frequency field. Phys. Lett. A 2020, 384, 126786. [Google Scholar] [CrossRef]
- Trukhanov, A.V.; Algarou, N.A.; Slimani, Y.; Almessiere, M.A.; Baykal, A.; Tishkevich, D.I.; Vinnik, D.A.; Vakhitov, M.G.; Klygach, D.S.; Silibin, M.V.; et al. Peculiarities of the microwave properties of hard–soft functional composites SrTb0.01Tm0.01Fe11.98O19–AFe2O4 (A = Co, Ni, Zn, Cu, or Mn). RSC Adv. 2020, 10, 32638. [Google Scholar] [CrossRef] [PubMed]
- Jonscher, A.K. Universal Relaxation Law, 1st ed.; Chelsea Dielectrics Press: London, UK, 1996. [Google Scholar]
- Funke, K. Jump relaxation in solid electrolytes. Prog. Solid State Chem. 1993, 22, 111–195. [Google Scholar] [CrossRef]
- Okuda, T.; Jufuku, N.; Hidaka, S.; Terada, N. Magnetic, transport, and thermoelectric properties of the delafossite oxides CuCr1−xMgxO2 (0 ≤ x ≤ 0.04). Phys. Rev. B 2005, 72, 144403. [Google Scholar] [CrossRef]
- Pike, G.E. AC conductivity of scandium oxide and a new hopping model for conductivity. Phys. Rev. B 1972, 6, 1572–1580. [Google Scholar] [CrossRef]
- Elliott, S.R. A theory of ac conduction in chalcogenide glasses. Philos. Mag. B 1977, 36, 1291–1304. [Google Scholar] [CrossRef]
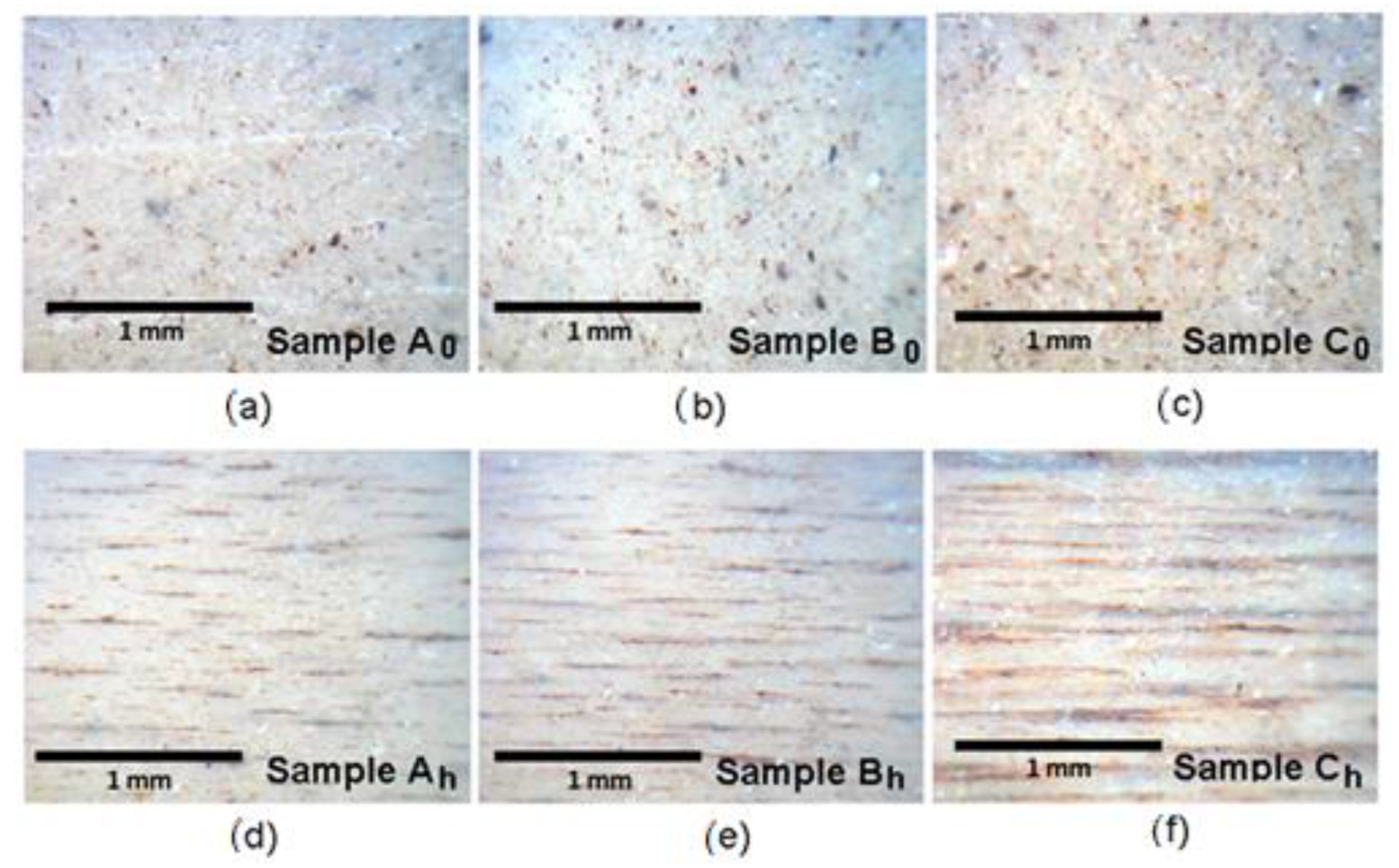
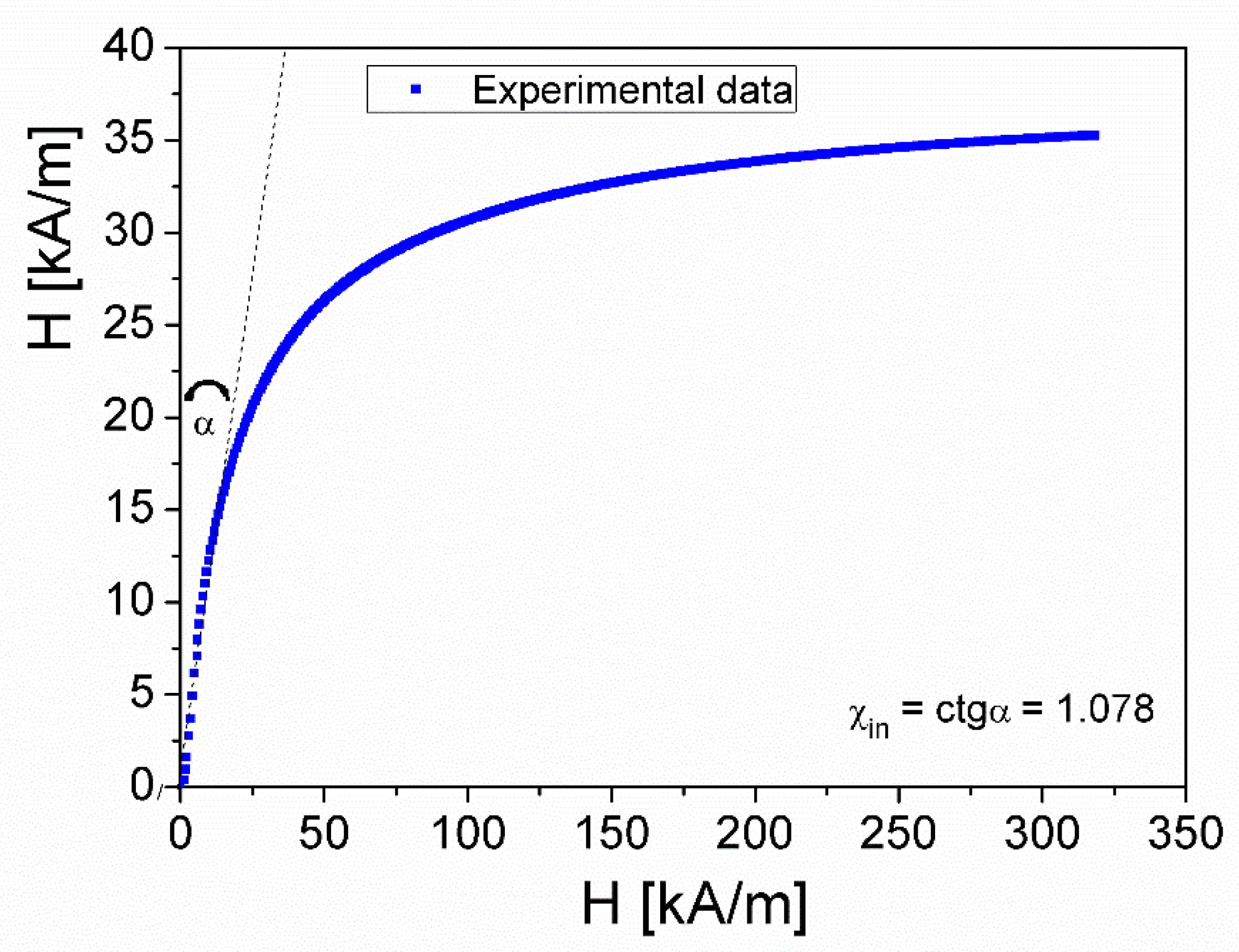


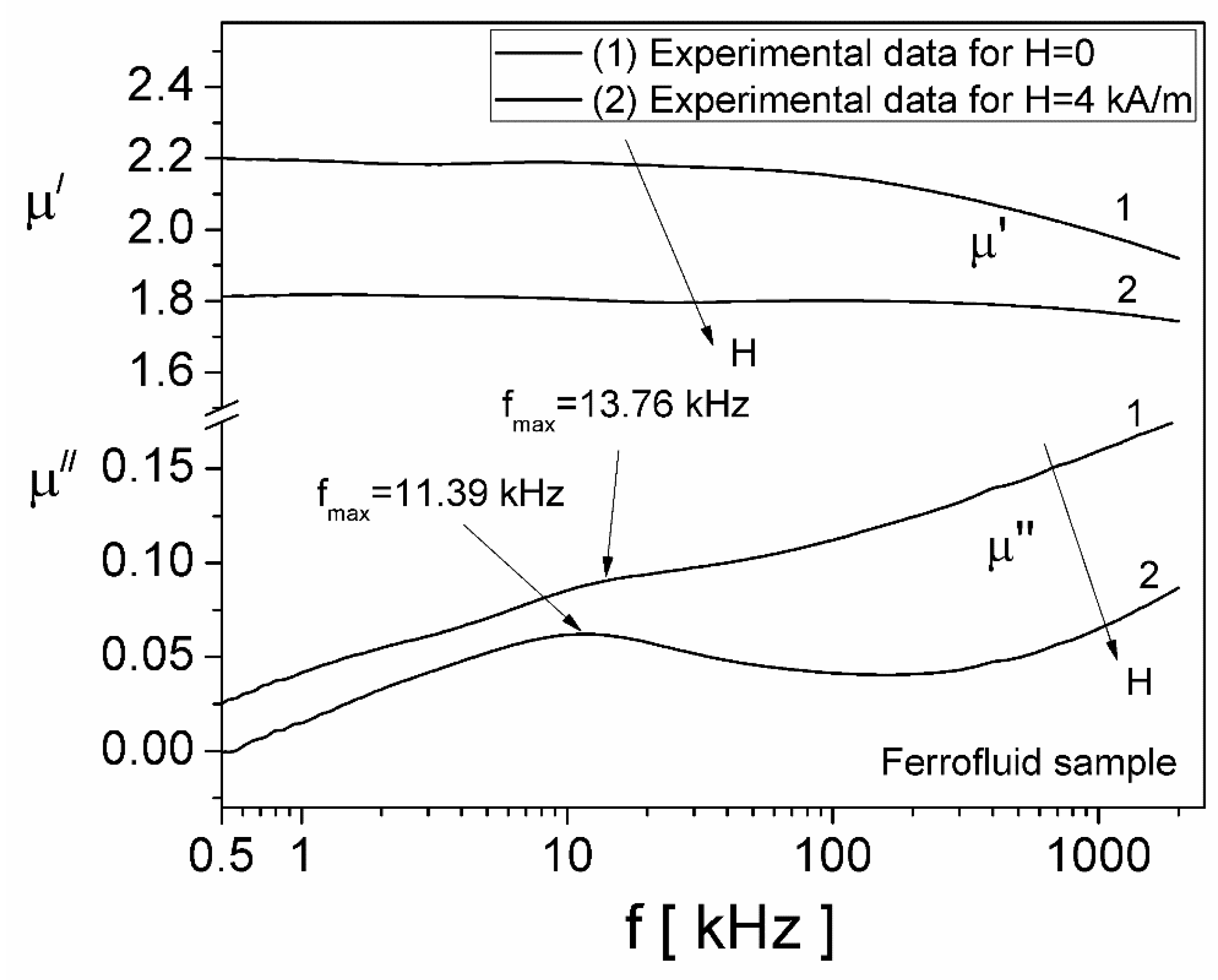



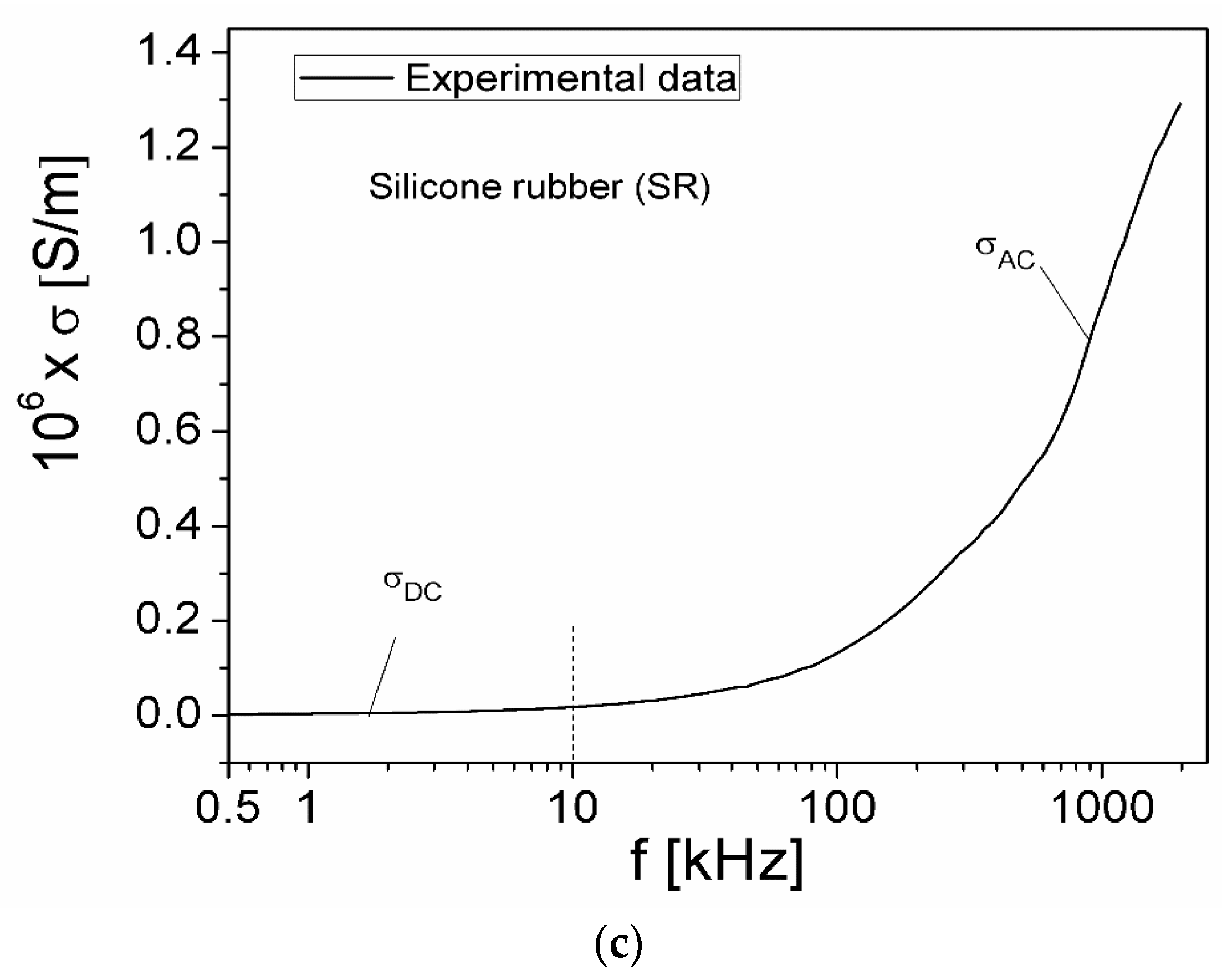

| Samples | A0 | B0 | C0 | Ah | Bh | Ch |
|---|---|---|---|---|---|---|
| φ = 1.31% | φ = 2.59% | φ = 3.84% | φ = 1.31% | φ = 2.59% | φ = 3.84% | |
| Parameters | H = 0 | H = 43 kA/m | ||||
| σDC (S/m) | 4.26∙10−9 | 9.40∙10−9 | 1.03∙10−8 | 4.93∙10−9 | 1.73∙10−8 | 1.86∙10−8 |
| n | 0.897 | 0.915 | 0.938 | 0.751 | 0.807 | 0.872 |
| A (S/m) | 5.42∙10−13 | 5.72∙10−13 | 4.73∙10−13 | 28.7∙10−13 | 20.4∙10−13 | 8.56∙10−13 |
| Wm (eV) | 1.51 | 1.83 | 2.51 | 0.62 | 0.81 | 1.22 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Marin, C.N.; Malaescu, I. The Effect of Magnetically Induced Local Structure and Volume Fraction on the Electromagnetic Properties of Elastomer Samples with Ferrofluid Droplet Inserts. Magnetochemistry 2024, 10, 4. https://doi.org/10.3390/magnetochemistry10010004
Marin CN, Malaescu I. The Effect of Magnetically Induced Local Structure and Volume Fraction on the Electromagnetic Properties of Elastomer Samples with Ferrofluid Droplet Inserts. Magnetochemistry. 2024; 10(1):4. https://doi.org/10.3390/magnetochemistry10010004
Chicago/Turabian StyleMarin, Catalin N., and Iosif Malaescu. 2024. "The Effect of Magnetically Induced Local Structure and Volume Fraction on the Electromagnetic Properties of Elastomer Samples with Ferrofluid Droplet Inserts" Magnetochemistry 10, no. 1: 4. https://doi.org/10.3390/magnetochemistry10010004
APA StyleMarin, C. N., & Malaescu, I. (2024). The Effect of Magnetically Induced Local Structure and Volume Fraction on the Electromagnetic Properties of Elastomer Samples with Ferrofluid Droplet Inserts. Magnetochemistry, 10(1), 4. https://doi.org/10.3390/magnetochemistry10010004






