Cationic Mismatch Effect Induced by Double Substitution on the Structural and Magnetic Properties of La0.5Ca0.5MnO3
Abstract
1. Introduction
2. Materials and Methods
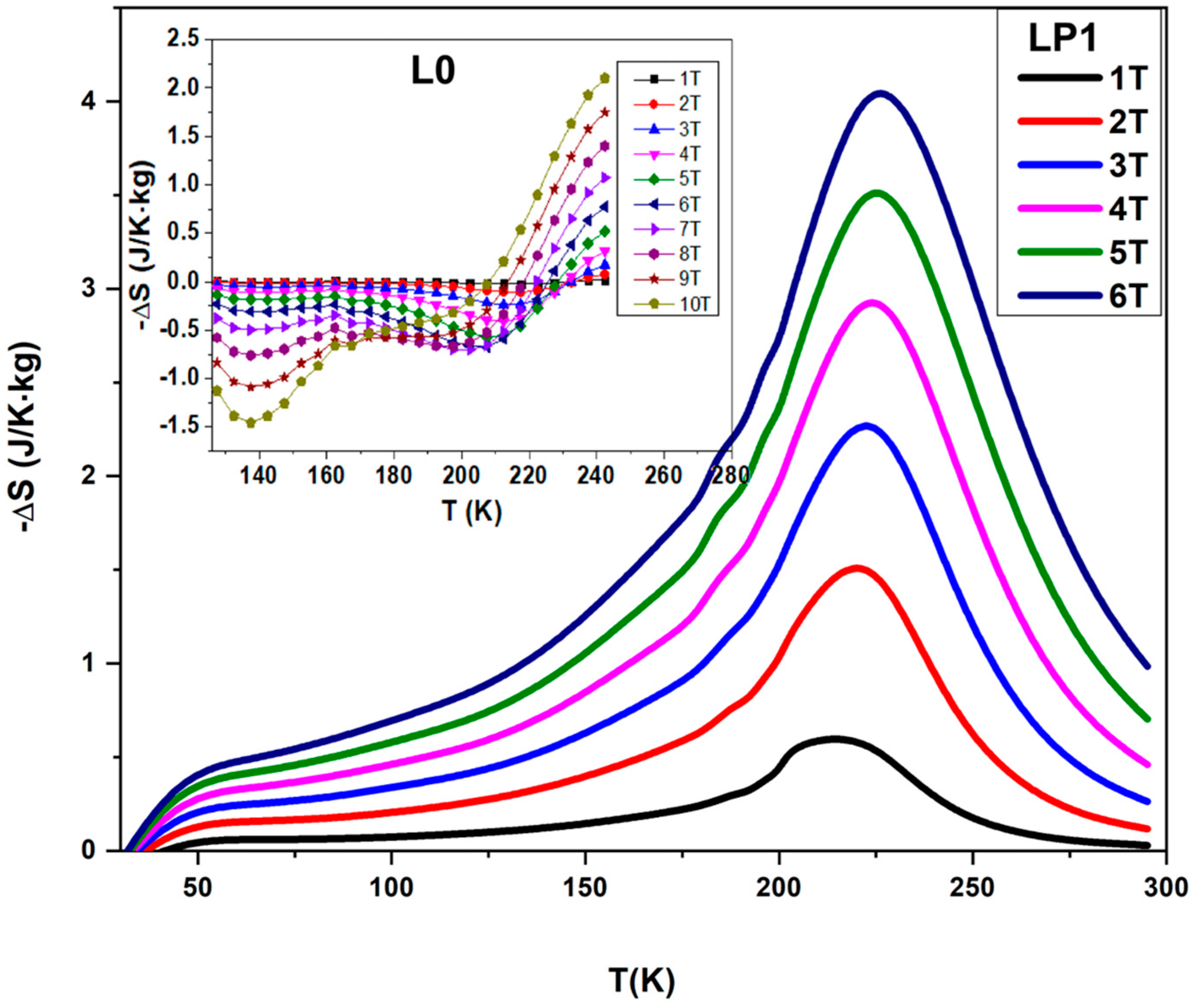
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| FM | Ferromagnetic |
| AFM | Antiferromagnetic |
| CO | Charge ordering |
| EDX | Energy-dispersive X-ray |
| PM | Paramagnetic |
| MPS | Magnetic phase separation |
| RCP | Relative cooling power |
References
- Daoud-Aladine, A.; Rodriguez-Carvajal, J.; Pinsard-Gaudart, L.; Fernandez-Diaz, M.T.; Revcolevschi, A. Zener polaron orderingin half-doped manganites. Phys. Rev. Lett. 2002, 89, 097205. [Google Scholar] [CrossRef] [PubMed]
- Nagaev, E.L.; Podel’shchikov, A.I.; Zil’berwarg, V.E. Re-entrant electronic phase separation in magnetic semiconductors and materials exhibiting colossal magnetoresistance. J. Exp. Theor. Phys. 1998, 78, 9823–9832. [Google Scholar] [CrossRef]
- Damay, F.; Martin, C.; Hervieu, M.; Maignan, A.; Raveau, B.; André, G.; Bourée, F. Structural transitions in the manganite Pr0.5Sr0.5MnO3. J. Magn. Magn. Mater. 1998, 184, 71–82. [Google Scholar] [CrossRef]
- Sundaresan, A.; Maignan, A.; Raveau, B. Effect of A-site cation size mismatch on charge ordering and colossal magnetoresistance properties of perovskite manganites. Phys. Rev. B 1997, 56, 5092–5095. [Google Scholar] [CrossRef]
- Schiffer, P.E.; Ramirez, A.P.; Bao, W.; Cheong, S.W. Low temperature magnetoresistance and the magnetic phase diagram of La1−xCaxMnO3. Phys. Rev. Lett. 1995, 75, 3336–3339. [Google Scholar] [CrossRef] [PubMed]
- Salakhova, R.T.; Pyatakov, A.P.; Zverev, V.I.; Pimentel, B.; Caraballo Vivas, R.J.; Makarova, L.A.; Perov, N.S.; Tishin, A.M.; Shtil, A.A.; Reis, M.S. The frequency dependence of magnetic heating for La0.75Sr0.25MnO3 nanoparticles. J. Magn. Magn. Mater. 2019, 470, 38–40. [Google Scholar] [CrossRef]
- Iqbal, M.; Khan, M.N.; Khan, A.A.; Zaka, I.; Mehmood, A.; Ahmad, I. Investigation of magnetic, magnetocaloric, and critical properties of La0.5Ba0.5MnO3 manganite. J. Supercond. Nov. Magn. 2018, 31, 3535–3544. [Google Scholar] [CrossRef]
- Serrao, C.R.; Sundaresan, A.; Rao, C.N.R. Multiferroic nature of charge-ordered rare earth manganites. J. Phys. Condens. Matter 2007, 19, 496217. [Google Scholar] [CrossRef]
- Krichene, A.; Boujelben, W.; Rathod, K.N.; Gadani, K.; Shah, N.A.; Solanki, P.S. Room-temperature colossal magnetodielectric effect in La0.4Eu0.1Ca0.5MnO3 manganite. J. Electron. Mater. 2020, 49, 5244–5247. [Google Scholar] [CrossRef]
- Krichene, A.; Boujelben, W. Multifunctionality of phase-separated manganites. J. Supercond. Nov. Magn. 2022, 35, 2609–2613. [Google Scholar] [CrossRef]
- Dhieb, S.; Krichene, A.; Chniba Boudjada, N.; Boujelben, W. Structural and magnetic properties of charge-ordered La0.5-xHoxCa0.5MnO3 (0 ≤ x ≤ 0.15). J. Alloys Compd. 2020, 832, 153728. [Google Scholar] [CrossRef]
- Mansuri, I.; Varshney, D.; Kaurav, N.; Lu, C.L.; Kuo, Y.K. Effects of A-site disorder on magnetic, electrical and thermal properties of La0.5−xLnxCa0.5−ySryMnO3 manganites. J. Magn. Magn Mater. 2011, 323, 316–323. [Google Scholar] [CrossRef]
- Salamon, M.B.; Lin, P.; Chun, S.H. Colossal magnetoresistance is a Griffiths singularity. Phys. Rev. Lett. 2002, 97, 197203. [Google Scholar] [CrossRef] [PubMed]
- Pramanik, A.K.; Banerjee, A. Phase separation and the effect of quenched disorder in Pr0.5Sr0.5MnO3. J. Phys. Condens. Matter 2008, 20, 275207. [Google Scholar]
- Abdelhedi, W.; Krichene, A.; Chniba Boudjada, N.; Boujelben, W. Cationic mismatch effect on structural and magnetic behavior of La0.5−xEuxCa0.5−yBayMnO3 charge–ordred manganites. Ceram. Int. 2024, 50, 11528–11538. [Google Scholar] [CrossRef]
- Abdelhedi, W.; Krichene, A.; Boujelben, W.; Chniba Boudjada, C. The impact of quenched disorder on the structural and magnetic properties and the low temperature inverse magnetocaloric effect in phase-separated La0.4Re0.1Ca0.5−yBayMnO3 manganites (Re = Nd, Eu, Gd). Mater. Chem. Phys. 2025, 332, 130272. [Google Scholar] [CrossRef]
- Soylu Koc, N.; Altintas, S.P.; Mahamdioua, N.; Terzioglu, C. Cation size mismatch effect in (La1-yREy)1.4Ca1.6Mn2O7 perovskite manganites. J. Alloys Compd. 2019, 797, 471–476. [Google Scholar] [CrossRef]
- Rodriguez-Martinez, L.M.; Attfield, J.P. Cation disorder and size effects in magnetoresistive manganese oxide perovskites. Phys. Rev. B 1996, 54, R15622(R). [Google Scholar] [CrossRef]
- Rodriguez-Martinez, L.M.; Attfield, J.P. Cation disorder and the metal-insulator transition temperature in manganese oxide perovskites. Phys. Rev. B 1998, 58, 2426. [Google Scholar] [CrossRef]
- Shannon, R.D. Revised effective ionic radii and systematic studies of interatomic distances in halides and chalcogenides. Acta Crystallogr. A 1976, 32, 751–767. [Google Scholar] [CrossRef]
- Gauswami, N.M.; Jadav, G.D.; Kanjariya, P.V.; Dadhania, D.A.; Bhalodia, J.A. Structural and microstructural properties of Al-doped NSMO manganites prepared through modified sol–gel method. J. Mater. Sci. Mater. Electron. 2024, 35, 1059. [Google Scholar] [CrossRef]
- Jatmika, J.; Winarsih, S.; Imaduddin, A.; Risdiana, R. Enhancing low field magnetoresistance in La0.7Ca0.25Sr0.05MnO3/Mn3O4 composite nanoparticles: Unveiling its transport mechanism. J. Sol-Gel Sci. Technol. 2025, 113, 399–412. [Google Scholar] [CrossRef]
- Mabrouki, W.; Krichene, A.; Chniba Boudjada, N.; Boujelben, A. Microstructural analysis by X-ray powder diffraction of nanosized Pr0.67Sr0.33MnO3 manganite. Appl. Phys. A 2024, 130, 230. [Google Scholar] [CrossRef]
- Noorasid, N.S.; Arith, F.; Aliyaselvam, O.V.; Salehuddin, F.; Mustafa, A.N.; Chelvanathan, P.; Azam, M.A.; Amin, N. Low-temperature sol-gel synthesized TiO2 with different titanium tetraisopropoxide (TTIP) molarity for flexible emerging solar cell. J. Sol-Gel Sci. Technol. 2024, 109, 826–834. [Google Scholar] [CrossRef]
- Kolat, V.S.; Izgi, T.; Kaya, A.O.; Bayri, N.; Gencer, H.; Atalay, S. Metamagnetic transition and magnetocaloric effect in charge ordered Pr0.68Ca0.32−xSrxMnO3 (x = 0, 0.1, 0.18, 0.26 and 0.32) compounds. J. Magn. Magn Mater. 2010, 322, 427–433. [Google Scholar]
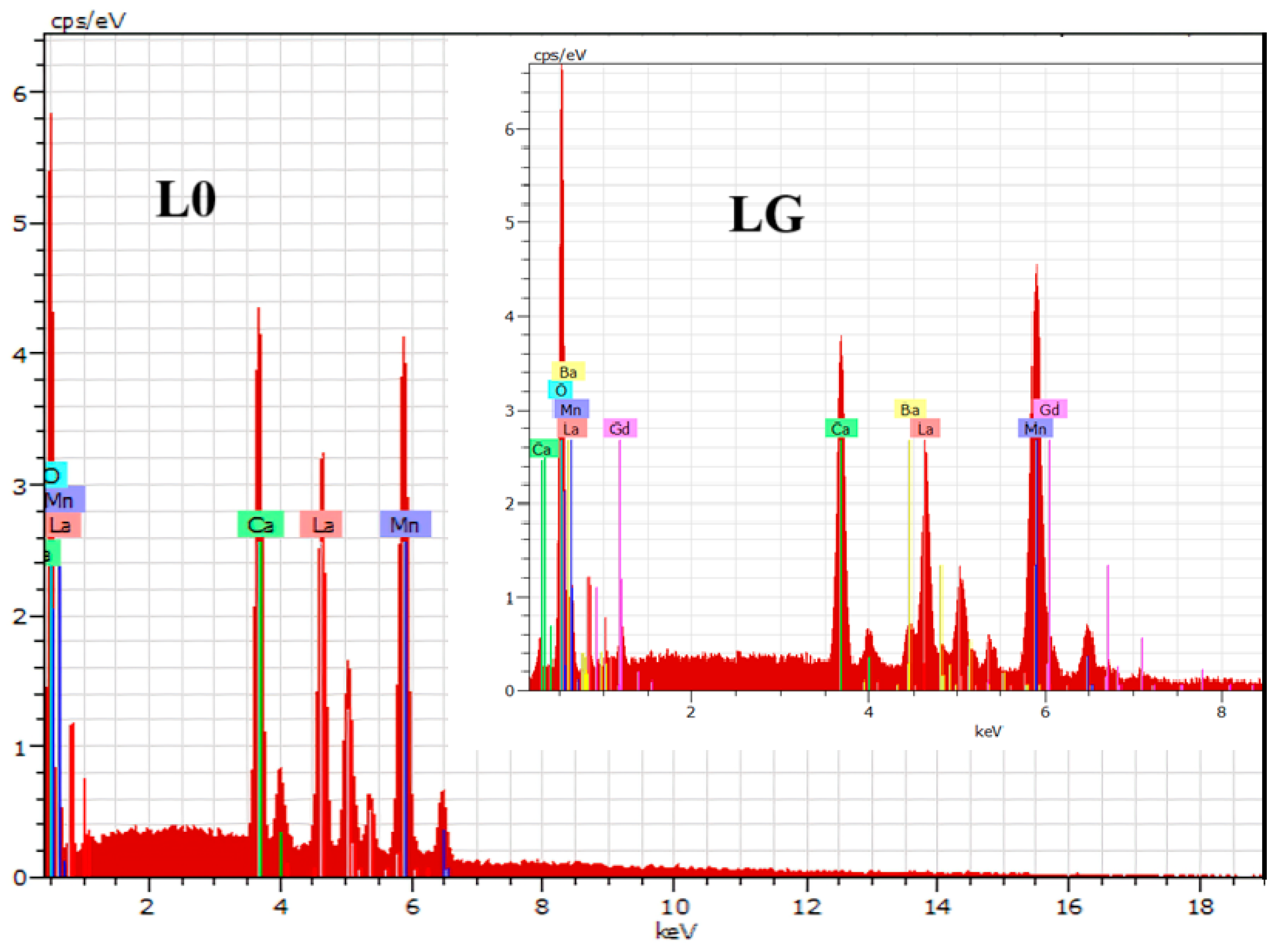
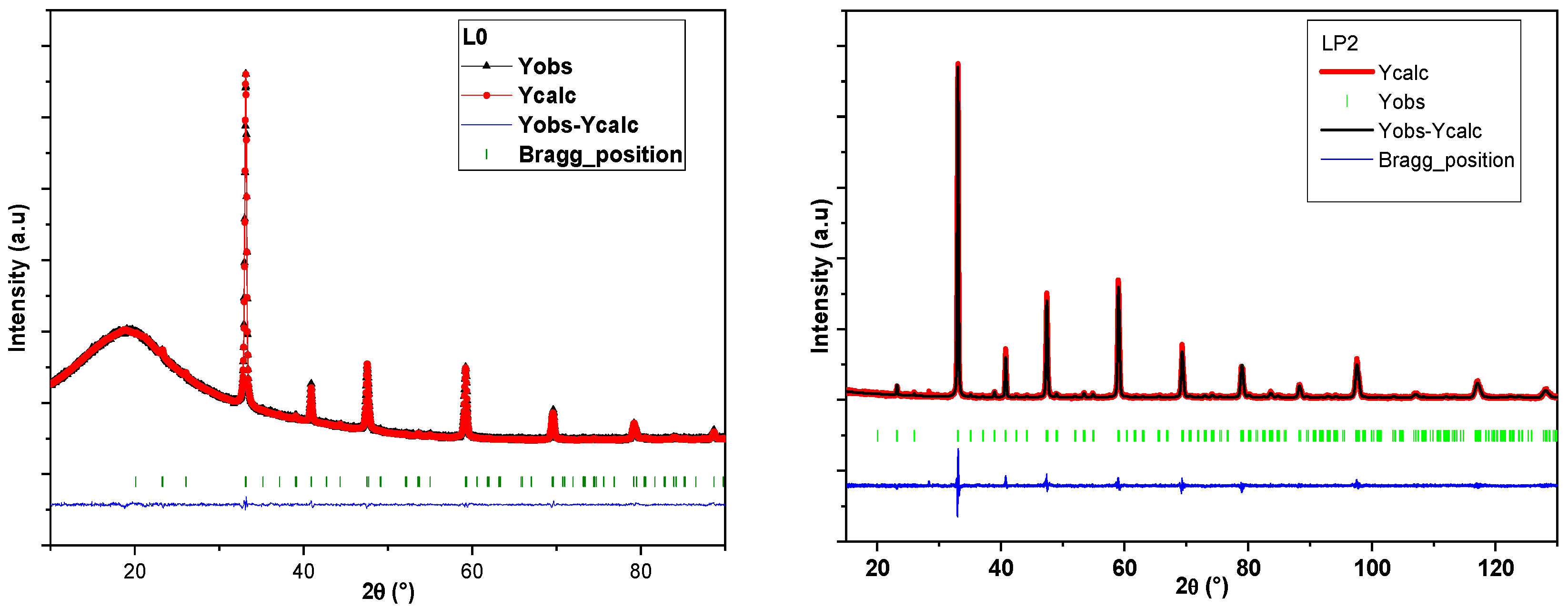


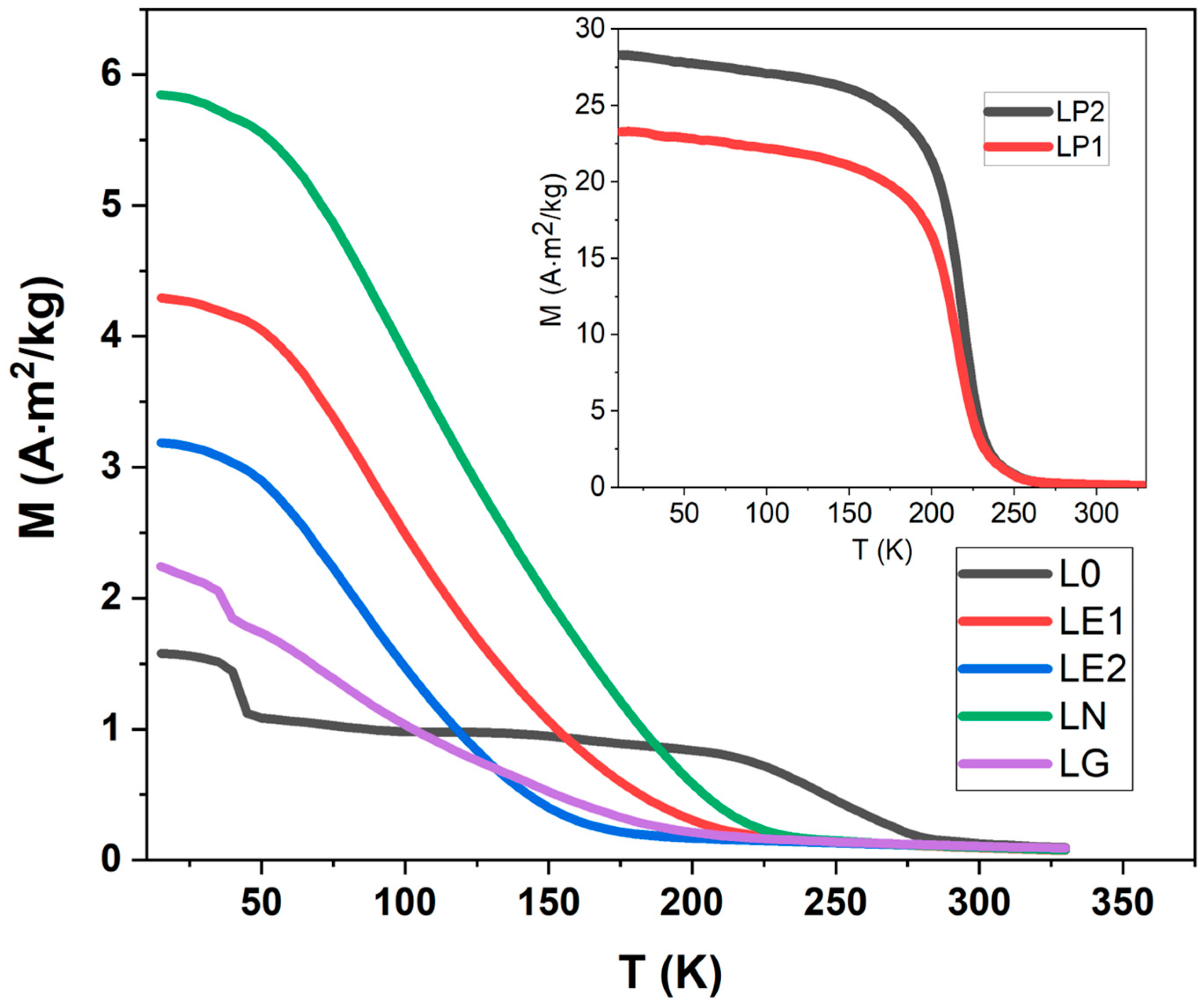



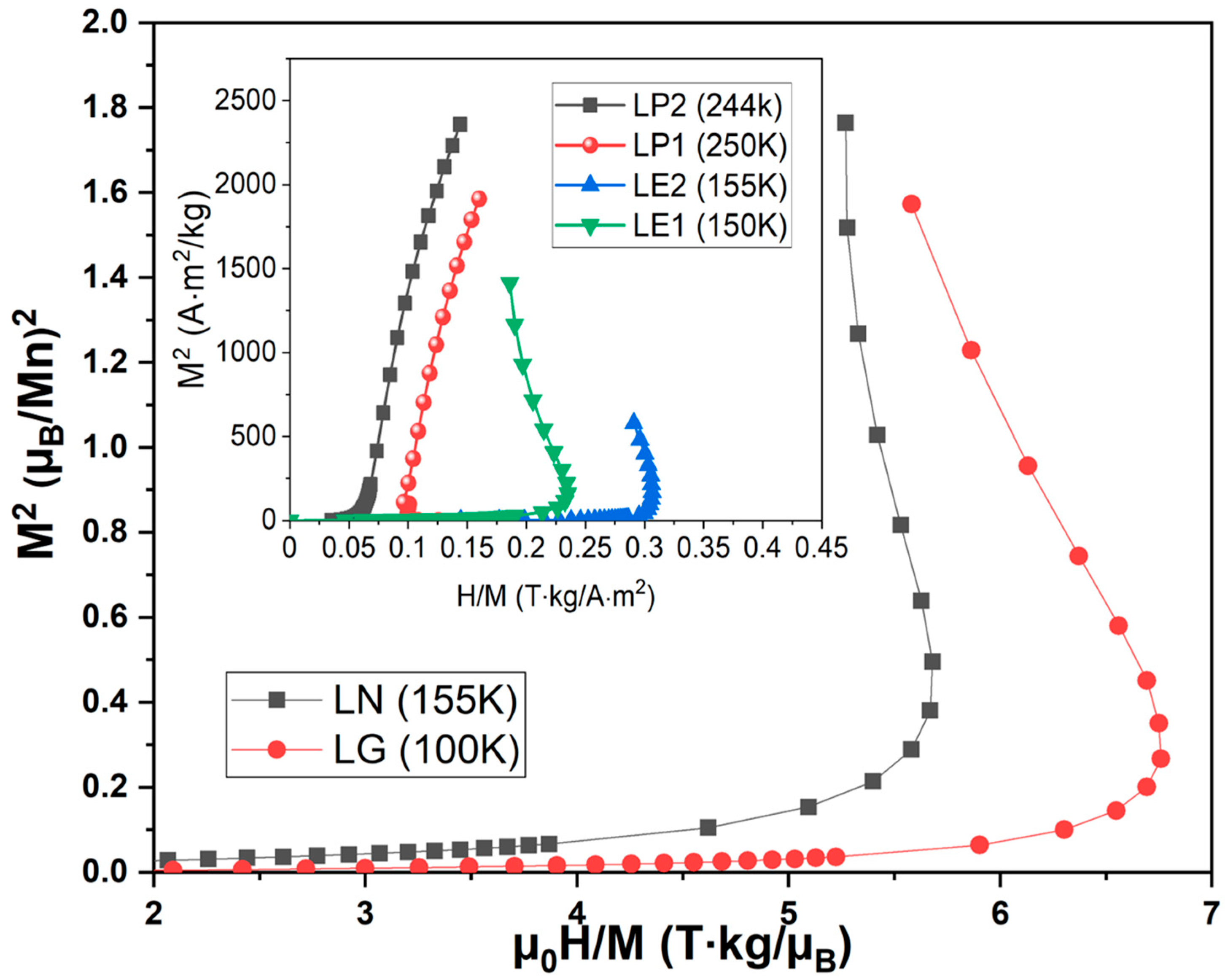

| Sample | a (Å) | b (Å) | c (Å) | σ2 (10−4 Å2) |
|---|---|---|---|---|
| L0 | 5.3954(4) | 7.6593(2) | 5.3983(4) | 3.2 |
| LP1 | 5.4144(3) | 7.6714(2) | 5.4147(2) | 6.2 |
| LP2 | 5.4161(3) | 7.6771(2) | 5.4154(3) | 6.9 |
| LE1 | 5.4008(6) | 7.6664(3) | 5.4010(6) | 21.8 |
| LE2 | 5.4218(3) | 7.6254(3) | 5.4088(3) | 40.2 |
| LN | 5.3973(10) | 7.6650(4) | 5.3973(9) | 17.0 |
| LG | 5.3996(7) | 7.6633(3) | 5.3989(7) | 51.0 |
| Sample | TC (K) | θp (K) | CP/µ0 (µB K T−1) | (µB) | (µB) |
|---|---|---|---|---|---|
| L0 | 250 | 218 | 7.82 | 5.81 | 4.41 |
| LP1 | 215 | 227 | 9.37 | 4.49 | 4.56 |
| LP2 | 220 | 225 | 7.97 | 6.47 | 5.98 |
| LN | 97 | 156 | 5.63 | 4.92 | 4.56 |
| LE1 | 90 | 179 | 8.55 | 6.17 | 5.98 |
| LE2 | 85 | 210 | 10.11 | 7.40 | 4.41 |
| LG | 72 | 100 | 7.00 | 5.49 | 5.07 |
| Sample | LP1 | LP2 | ||||
|---|---|---|---|---|---|---|
| µ0H (T) | (J K−1 kg−1) | (K) | RCP (J kg−1) | (J K−1 kg−1) | (K) | RCP (J kg−1) |
| 1 | 1.48 | 10.05 | 14.87 | 0.88 | 33 | 29.04 |
| 2 | 2.24 | 33.09 | 74.12 | 1.75 | 48.37 | 84.65 |
| 3 | 2.84 | 48.82 | 138.64 | 2.48 | 50.94 | 126.33 |
| 4 | 3.29 | 62.04 | 204.11 | 3.1 | 58.94 | 182.71 |
| 5 | 3.68 | 74.26 | 273.28 | 3.63 | 69.52 | 252.36 |
| 6 | 4.12 | 85.04 | 350.38 | 4.16 | 73.79 | 306.97 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Abdelhedi, W.; Krichene, A.; Boujelben, W.; Chniba-Boudjada, N. Cationic Mismatch Effect Induced by Double Substitution on the Structural and Magnetic Properties of La0.5Ca0.5MnO3. Magnetochemistry 2025, 11, 36. https://doi.org/10.3390/magnetochemistry11050036
Abdelhedi W, Krichene A, Boujelben W, Chniba-Boudjada N. Cationic Mismatch Effect Induced by Double Substitution on the Structural and Magnetic Properties of La0.5Ca0.5MnO3. Magnetochemistry. 2025; 11(5):36. https://doi.org/10.3390/magnetochemistry11050036
Chicago/Turabian StyleAbdelhedi, Wadie, Akram Krichene, Wahiba Boujelben, and Nassira Chniba-Boudjada. 2025. "Cationic Mismatch Effect Induced by Double Substitution on the Structural and Magnetic Properties of La0.5Ca0.5MnO3" Magnetochemistry 11, no. 5: 36. https://doi.org/10.3390/magnetochemistry11050036
APA StyleAbdelhedi, W., Krichene, A., Boujelben, W., & Chniba-Boudjada, N. (2025). Cationic Mismatch Effect Induced by Double Substitution on the Structural and Magnetic Properties of La0.5Ca0.5MnO3. Magnetochemistry, 11(5), 36. https://doi.org/10.3390/magnetochemistry11050036






