A Modular Cell Balancing Circuit and Strategy Based on Bidirectional Flyback Converter †
Abstract
1. Introduction
- (1)
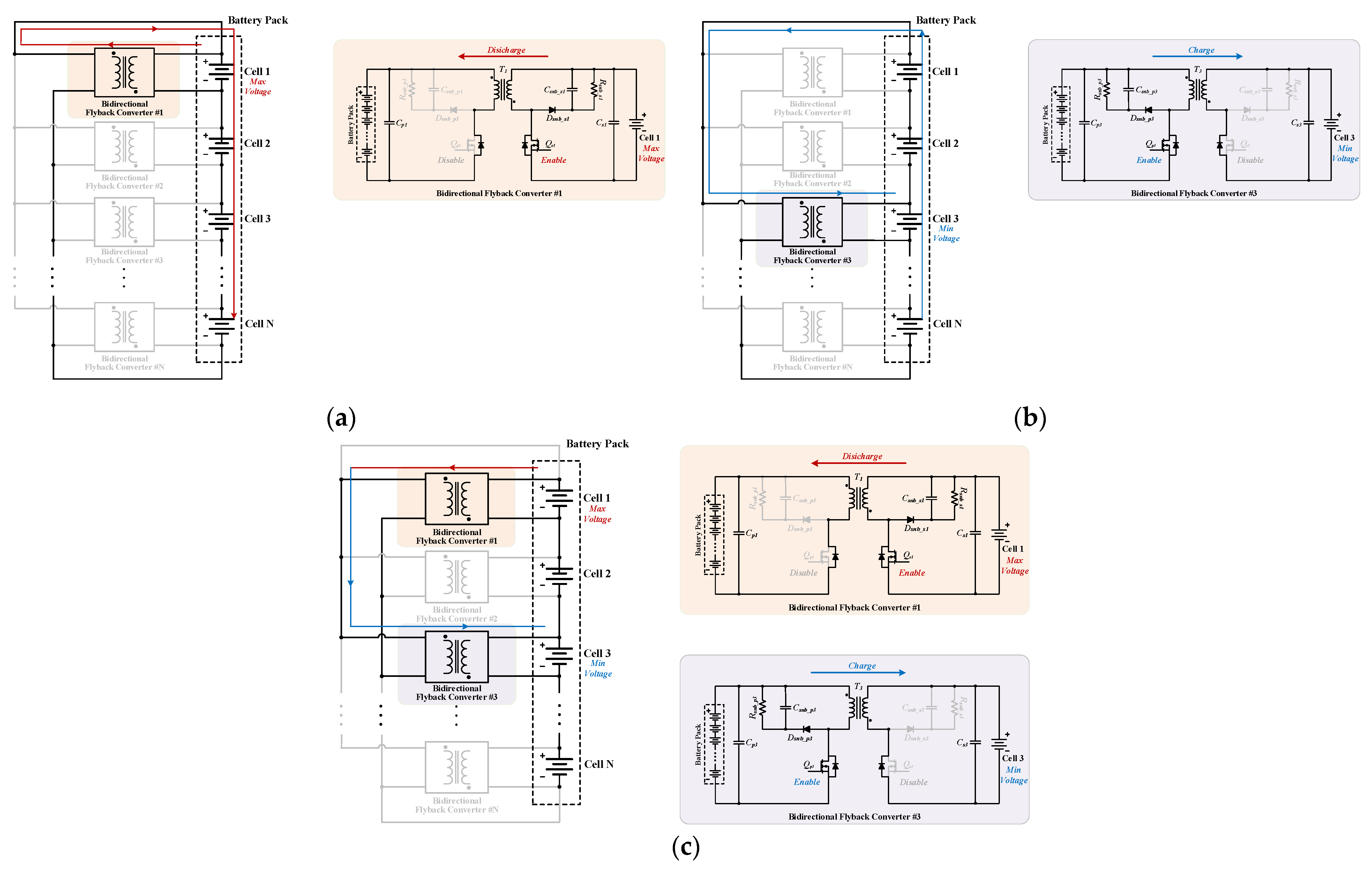
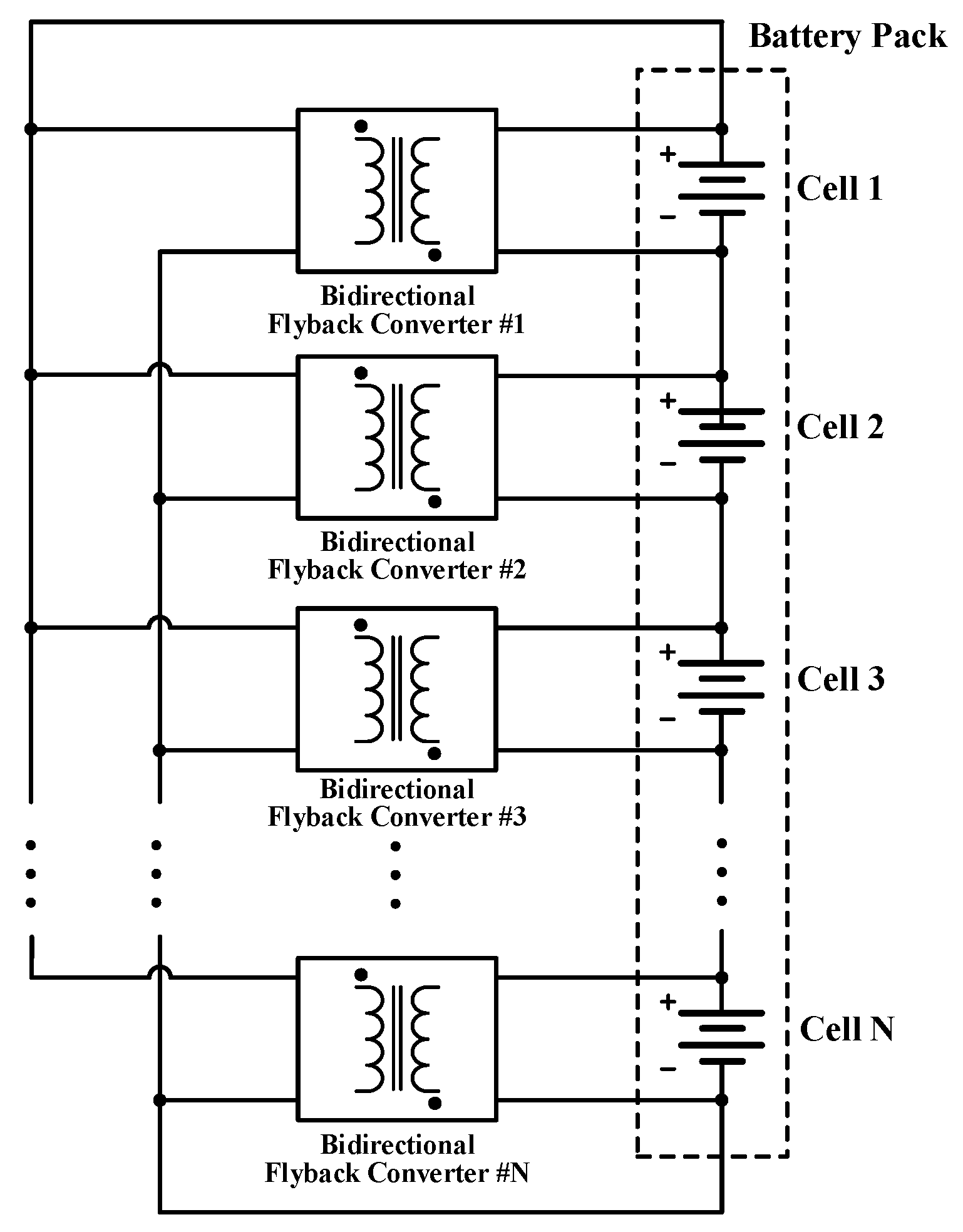
- The proposed configuration is equipped with a symmetrical structure BFC for each cell, where all BFCs are connected in an input-parallel output-series (IPOS) configuration, which allows for efficient energy transfer in either direction between any two cells. Due to the modular design, it is easy to expand the system without redesigning the whole circuit.
- (2)
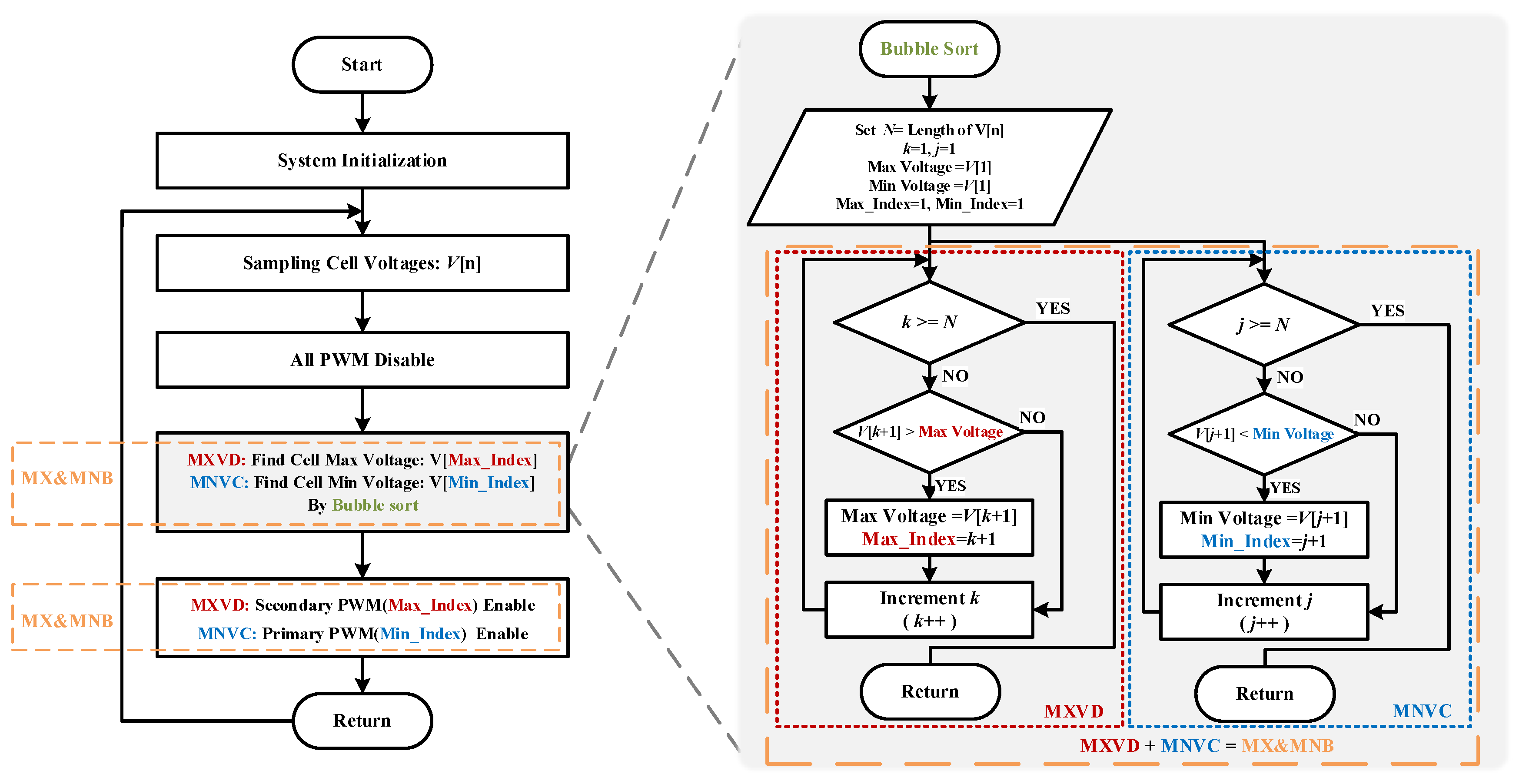
- The charging/discharging mode of a particular cell could be controlled by adjusting the PWM signal on the primary or secondary side. The process of discharging the maximum voltage cell in the battery pack is called the maximum voltage discharge method (MXVD). Charging the minimum voltage cell with the minimum voltage is the minimum voltage charging method (MNVC). Combining the two methods to simultaneously control the discharging of the maximum voltage cell and charging of the minimum voltage cell is further proposed as the peer-to-peer energy transfer maximum and minimum voltage balancing method (MX&MNB). The algorithm and flowchart of the balancing strategies are described, which can be simply implemented in a low-cost microcontroller.
- (3)
- To validate the feasibility and balancing performance of the proposed configuration and methods, a cell balancing hardware prototype is designed and built. The experimental results show that all three proposed methods are effective in achieving cell balancing. Compared with MXVD and MNVC, the MX&MNB method enables energy transfer directly between the cells with the highest voltage difference, which effectively reduces the balancing time.
2. The Cell Balancing Circuit Based on Bidirectional Flyback Converter
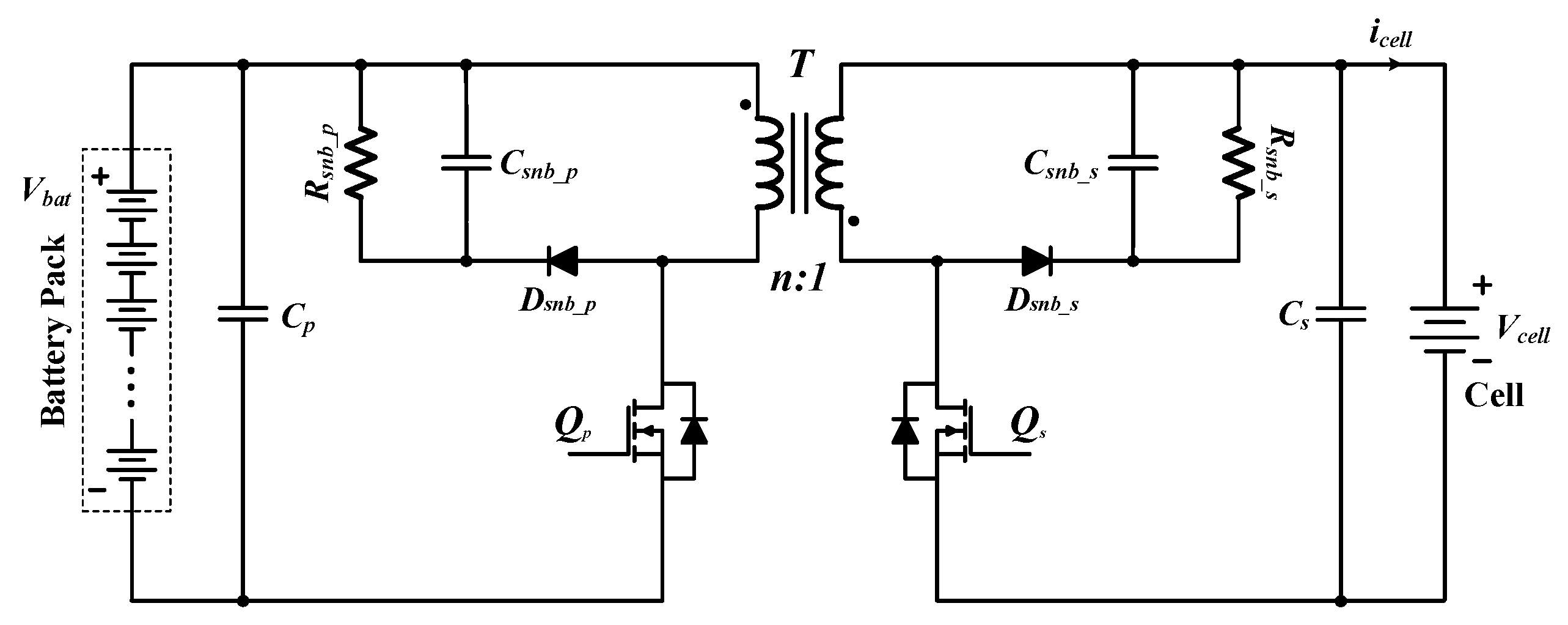
2.1. The Configuration of the Proposed Cell Balancing Circuit
2.2. Operation Principle of Bidirectional Flyback Converter
3. The Proposed Cell Balancing Strategy
3.1. The Working Principle of the Balancing Strategy
- (1)
- MXVD
- (2)
- MNVC
- (3)
- MX&MNB
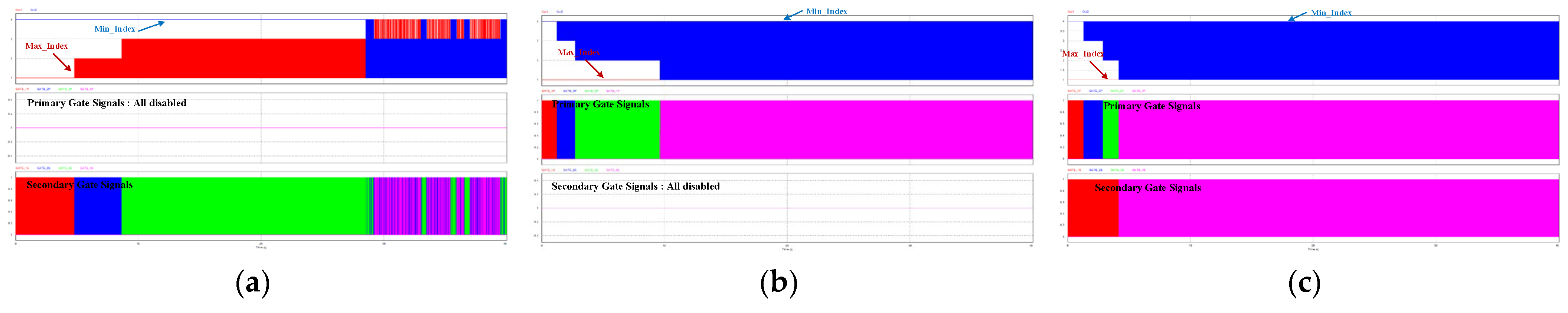
3.2. Cell Balancing Algorithm
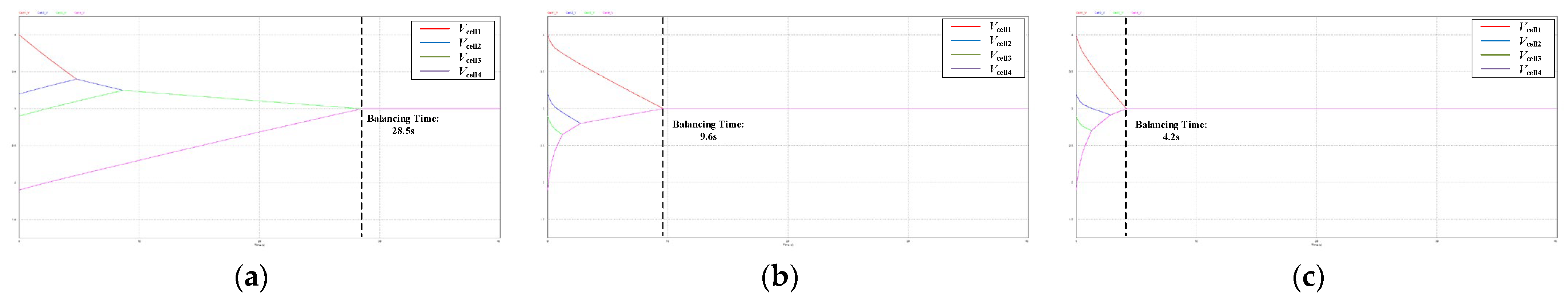
4. Simulation and Experimental Verification
4.1. Simulation
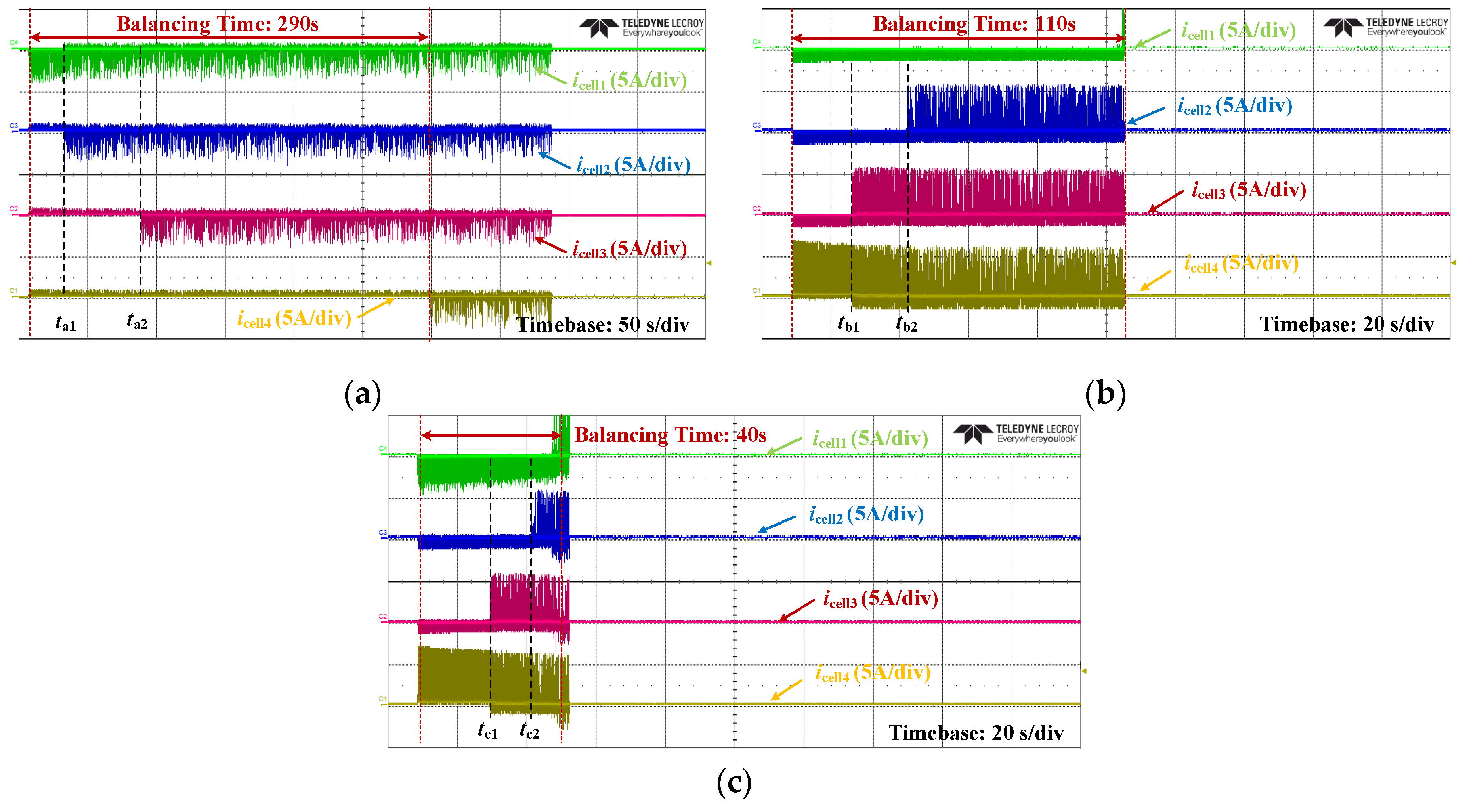
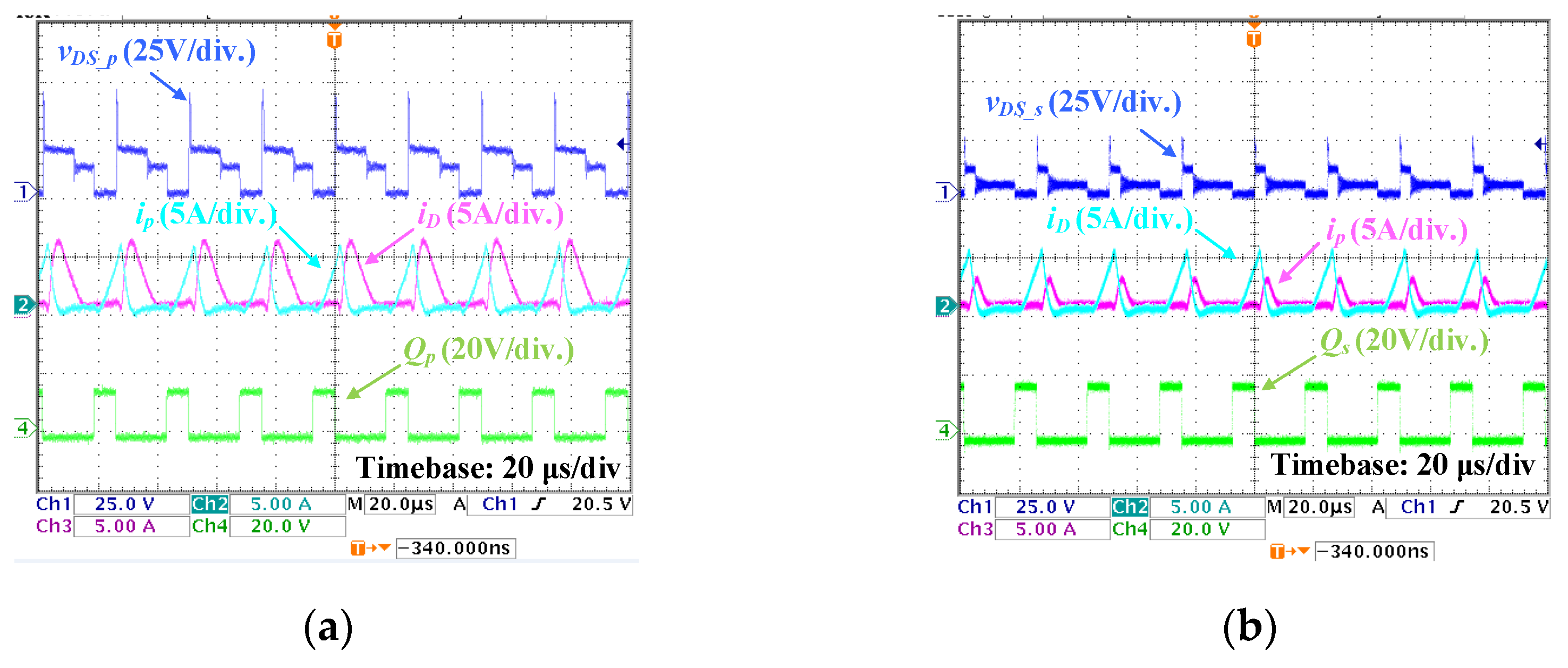
4.2. Experimental Verification
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| BFC | Bidirectional flyback converter |
| MXVD | Maximum voltage discharge |
| MNVC | Minimum voltage charge |
| MX&MNB | Maximum and minimum voltage balancing |
| IPOS | Input-parallel output-series |
| C2C | Cell-to-cell |
| C2P | Cell-to-pack |
| CCM | Current continuous mode |
| DCM | Current discontinuous mode |
References
- Lin, T.; Chen, Z.; Zheng, C.; Huang, D.; Zhou, S. Fault Diagnosis of Lithium-Ion Battery Pack Based on Hybrid System and Dual Extended Kalman Filter Algorithm. IEEE Trans. Transp. Electrif. 2021, 7, 26–36. [Google Scholar] [CrossRef]
- Lim, C.-S.; Lee, K.-J.; Ku, N.-J.; Hyun, D.-S.; Kim, R.-Y. A Modularized Equalization Method Based on Magnetizing Energy for a Series-Connected Lithium-Ion Battery String. IEEE Trans. Power Electron. 2014, 29, 1791–1799. [Google Scholar] [CrossRef]
- Chen, M.; Rincon-Mora, G.A. Accurate electrical battery model capable of predicting runtime and I-V performance. IEEE Trans. Energy Convers. 2006, 21, 504–511. [Google Scholar] [CrossRef]
- Kwon, J.-H.; Choi, S.-C.; Zhou, G.; Park, S.-M.; Park, S.-J.; Wang, Y. A Cell-to-cell Voltage Balancing Strategy with Bidirectional Flyback Converter. In Proceedings of the 2024 IEEE 10th International Power Electronics and Motion Control Conference (IPEMC2024-ECCE Asia), Chengdu, China, 17–20 May 2024; pp. 4088–4093. [Google Scholar] [CrossRef]
- El Ghossein, N.; Sari, A.; Venet, P. Effects of the Hybrid Composition of Commercial Lithium-Ion Capacitors on Their Floating Aging. IEEE Trans. Power Electron. 2019, 34, 2292–2299. [Google Scholar] [CrossRef]
- Milas, N.T.; Tatakis, E.C. Fast Battery Cell Voltage Equalizer Based on the Bidirectional Flyback Converter. IEEE Trans. Transp. Electrif. 2023, 9, 4922–4940. [Google Scholar] [CrossRef]
- Zhang, Z.; Gui, H.; Gu, D.-J.; Yang, Y.; Ren, X. A Hierarchical Active Balancing Architecture for Lithium-Ion Batteries. IEEE Trans. Power Electron. 2017, 32, 2757–2768. [Google Scholar] [CrossRef]
- Wang, Y.; Kim, S.-Y.; Chen, Y.; Zhang, H.; Park, S.-J. An SMPS-Based Lithium-Ion Battery Test System for Internal Resistance Measurement. IEEE Trans. Transp. Electrif. 2023, 9, 934–944. [Google Scholar] [CrossRef]
- Shang, Y.; Zhu, C.; Fu, Y.; Mi, C.C. An Integrated Heater Equalizer for Lithium-Ion Batteries of Electric Vehicles. IEEE Trans. Ind. Electron. 2019, 66, 4398–4405. [Google Scholar] [CrossRef]
- Naguib, M.; Kollmeyer, P.; Emadi, A. Lithium-Ion Battery Pack Robust State of Charge Estimation, Cell Inconsistency, and Balancing: Review. IEEE Access 2021, 9, 50570–50582. [Google Scholar] [CrossRef]
- Izadi, Y.; Beiranvand, R. A Comprehensive Review of Battery and Supercapacitor Cells Voltage-Equalizer Circuits. IEEE Trans. Power Electron. 2023, 38, 15671–15692. [Google Scholar] [CrossRef]
- Raeber, M.; Heinzelmann, A.; Abdeslam, D.O. Analysis of an Active Charge Balancing Method Based on a Single Nonisolated DC/DC Converter. IEEE Trans. Ind. Electron. 2021, 68, 2257–2265. [Google Scholar] [CrossRef]
- Qi, X.; Wang, Y.; Fang, M.; Liu, W. A Reduced-Component-Count Centralized Equalization System for Series-Connected Battery Packs Based on a Novel Integrated Cascade Topology. IEEE Trans. Ind. Appl. 2021, 57, 6105–6116. [Google Scholar] [CrossRef]
- Qi, X.; Wang, Y.; Wang, Y.; Chen, Z. Optimization of Centralized Equalization Systems Based on an Integrated Cascade Bidirectional DC–DC Converter. IEEE Trans. Ind. Electron. 2022, 69, 249–259. [Google Scholar] [CrossRef]
- Xiong, H.; Song, D.; Shi, F.; Wei, Y.; Jinzhen, L. Novel voltage equalisation circuit of the lithium battery pack based on bidirectional flyback converter. IET Power Electron. 2020, 13, 2194–2200. [Google Scholar] [CrossRef]
- Zhou, G.; Zhang, X.; Gao, K.; Tian, Q.; Xu, S. Two-Mode Active Balancing Circuit Based on Switched-Capacitor and Three-Resonant-State LC Units for Series-Connected Cell Strings. IEEE Trans. Ind. Electron. 2022, 69, 4845–4858. [Google Scholar] [CrossRef]
- Nazi, H.; Babaei, E. A Modularized Bidirectional Charge Equalizer for Series-Connected Cell Strings. IEEE Trans. Ind. Electron. 2021, 68, 6739–6749. [Google Scholar] [CrossRef]
- Qi, X.; Wang, Y.; Fang, M.; Wang, Y.; Chen, Z. Multiport DC–DC Converter with Integrated Cascaded Structure for Optimizing Centralized Battery Equalization System. IEEE Trans. Power Electron. 2022, 37, 15111–15126. [Google Scholar] [CrossRef]
- Avila, A.; Garcia-Bediaga, A.; Alzuguren, I.; Vasić, M.; Rujas, A. A Modular Multifunction Power Converter Based on a Multiwinding Flyback Transformer for EV Application. IEEE Trans. Transp. Electrif. 2022, 8, 168–179. [Google Scholar] [CrossRef]
- Lee, J.-H.; Park, S.-J.; Lim, S.-K. Improvement of Multilevel DC/DC Converter for E-Mobility Charging Station. Electronics 2020, 9, 2037. [Google Scholar] [CrossRef]
- Jaguemont, J.; Boulon, L.; Dubé, Y. A comprehensive review of lithium-ion batteries used in hybrid and electric vehicles at cold temperatures. Appl. Energy 2016, 164, 99–114. [Google Scholar] [CrossRef]
- Ding, X.; Zhang, D.; Cheng, J.; Wang, B.; Chai, Y.; Zhao, Z.; Xiong, R.; Luk, P.C.K. A Novel Active Equalization Topology for Series-Connected Lithium-ion Battery Packs. IEEE Trans. Ind. Appl. 2020, 56, 6892–6903. [Google Scholar] [CrossRef]
- Tashakor, N.; Farjah, E.; Ghanbari, T. A Bidirectional Battery Charger with Modular Integrated Charge Equalization Circuit. IEEE Trans. Power Electron. 2017, 32, 2133–2145. [Google Scholar] [CrossRef]
- Kim, M.-Y.; Kim, J.-H.; Moon, G.-W. Center-Cell Concentration Structure of a Cell-to-Cell Balancing Circuit with a Reduced Number of Switches. IEEE Trans. Power Electron. 2014, 29, 5285–5297. [Google Scholar] [CrossRef]
- Shang, Y.; Zhang, C.; Cui, N.; Guerrero, J.M. A Cell-to-Cell Battery Equalizer with Zero-Current Switching and Zero-Voltage Gap Based on Quasi-Resonant LC Converter and Boost Converter. IEEE Trans. Power Electron. 2015, 30, 3731–3747. [Google Scholar] [CrossRef]
- Lee, K.-M.; Lee, S.-W.; Choi, Y.-G.; Kang, B. Active Balancing of Li-Ion Battery Cells Using Transformer as Energy Carrier. IEEE Trans. Ind. Electron. 2017, 64, 1251–1257. [Google Scholar] [CrossRef]
- Wang, Y.; Kim, S.-Y.; Zhang, H.; Chen, Y.; Park, S.-J. Design and analysis of an input-series quasi-resonant flyback high-voltage SMPS based on an integrated transformer. IET Power Electron. 2022, 15, 1058–1074. [Google Scholar] [CrossRef]
- Mahafzah, K.A.; Obeidat, M.A.; Al-Shetwi, A.Q.; Ustun, T.S. A Novel Synchronized Multiple Output DC-DC Converter Based on Hybrid Flyback-Cuk Topologies. Batteries 2022, 8, 93. [Google Scholar] [CrossRef]
- Hannan, M.A.; Hoque, M.M.; Peng, S.E.; Uddin, M.N. Lithium-Ion Battery Charge Equalization Algorithm for Electric Vehicle Applications. IEEE Trans. Ind. Appl. 2017, 53, 2541–2549. [Google Scholar] [CrossRef]
- Chen, Y.; Liu, X.; Cui, Y.; Zou, J.; Yang, S. A MultiWinding Transformer Cell-to-Cell Active Equalization Method for Lithium-Ion Batteries with Reduced Number of Driving Circuits. IEEE Trans. Power Electron. 2016, 31, 4916–4929. [Google Scholar]
- SPark, H.; Park, K.-B.; Kim, H.-S.; Moon, G.-W.; Youn, M.-J. Single-Magnetic Cell-to-Cell Charge Equalization Converter with Reduced Number of Transformer Windings. IEEE Trans. Power Electron. 2012, 27, 2900–2911. [Google Scholar]

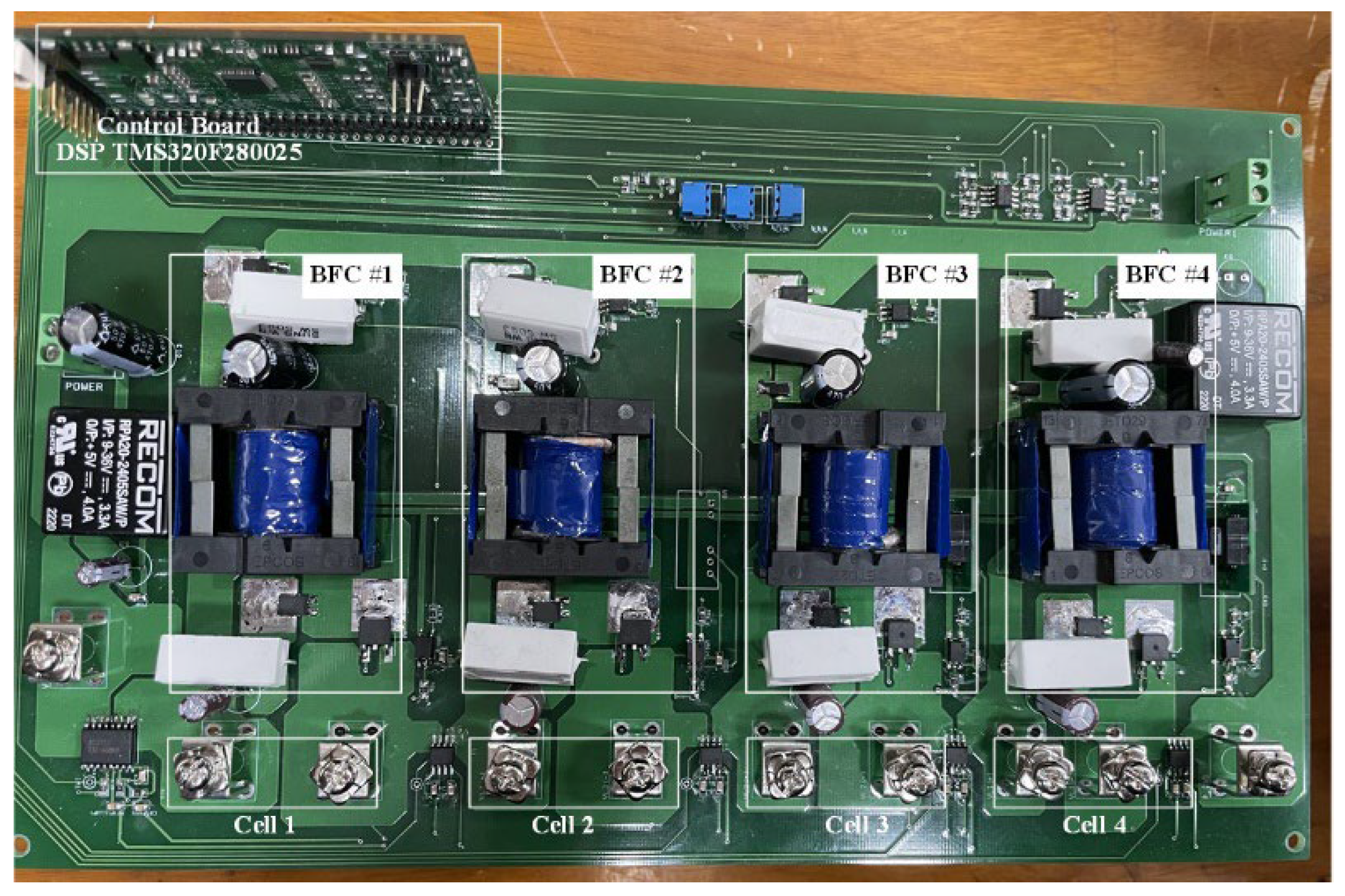


| Parameters | Specifications |
|---|---|
| N | 4 |
| Vcell1 | 4.0 V |
| Vcell2 | 3.2 V |
| Vcell3 | 2.9 V |
| Vcell4 | 1.9 V |
| Csub | 100 μF |
| Rsub | 470 Ω |
| Switching frequency | 10 kHz |
| Primary switching | DMN6068LK3 |
| Secondary switching | AOD424 |
| Core | ETD29/16/10-3C90 |
| Transformer turns ratio | 10:5 |
| Method | Balancing Time/s |
|---|---|
| MXVD | 28.5 |
| MNVC | 9.6 |
| MX&MNB | 4.2 |
| Method | Balancing Time/s |
|---|---|
| MXVD | 290 |
| MNVC | 110 |
| MX&MNB | 40 |
| Method | Topology | Switching Devices | Inductor | Transformer | Control Strategy | Modular Design |
|---|---|---|---|---|---|---|
| Proposed | Flyback | 2N | - | N | Simple | Y |
| [12] | Buck-boost + Switch matrix | 4 + 2(N + 1) | 1 | - | Complex | N |
| [13] | Buck-boost + Flyback + Switch matrix | 6 + 2(N + 1) | 1 | 1 | Complex | N |
| [15] | Flyback + Switch array | N + 2 | - | 1 | Moderate | Y |
| [17] | Full-bridge + Switch array | 7 + 2N + 1 | - | 1 (multioutput) | Moderate | Y |
| [18] | Buck-boost + Flyback + Switch matrix | 2 + 2(N + 1) + 4 | 2 | 1 (multioutput) | Complex | Y |
| [29] | Flyback + Switch matrix | 4 + 2(N + 1) | - | 2 | Simple | Y |
| [30] | Flyback + Bidirectional switch | 2N | - | 1 (multioutput) | Simple | N |
| [31] | Bidirectional switch + Multioutput transformer | 2N | N | 1 (multioutput) | Complex | N |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Y.; Kwon, J.-H.; Choi, S.-C.; Zhou, G.; Park, S.-J. A Modular Cell Balancing Circuit and Strategy Based on Bidirectional Flyback Converter. Batteries 2025, 11, 168. https://doi.org/10.3390/batteries11050168
Wang Y, Kwon J-H, Choi S-C, Zhou G, Park S-J. A Modular Cell Balancing Circuit and Strategy Based on Bidirectional Flyback Converter. Batteries. 2025; 11(5):168. https://doi.org/10.3390/batteries11050168
Chicago/Turabian StyleWang, Yipei, Jun-Hyeong Kwon, Seong-Cheol Choi, Guangxu Zhou, and Sung-Jun Park. 2025. "A Modular Cell Balancing Circuit and Strategy Based on Bidirectional Flyback Converter" Batteries 11, no. 5: 168. https://doi.org/10.3390/batteries11050168
APA StyleWang, Y., Kwon, J.-H., Choi, S.-C., Zhou, G., & Park, S.-J. (2025). A Modular Cell Balancing Circuit and Strategy Based on Bidirectional Flyback Converter. Batteries, 11(5), 168. https://doi.org/10.3390/batteries11050168







