Modeling Anisotropic Transport in Polycrystalline Battery Materials
Abstract
:1. Introduction
2. Materials and Methods
2.1. Multi-Phase Diffusion
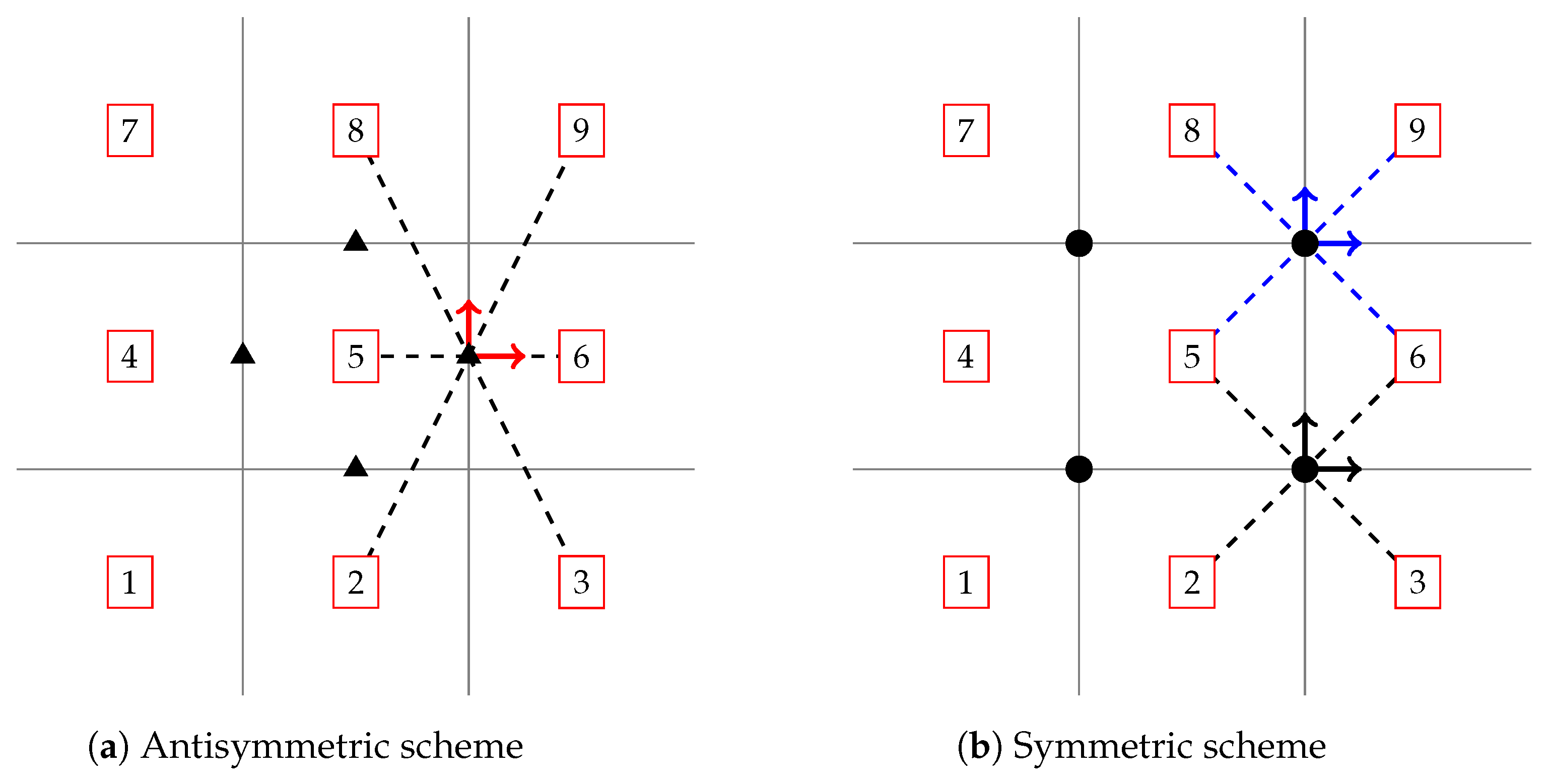
2.2. Anisotropic Diffusion Tensor
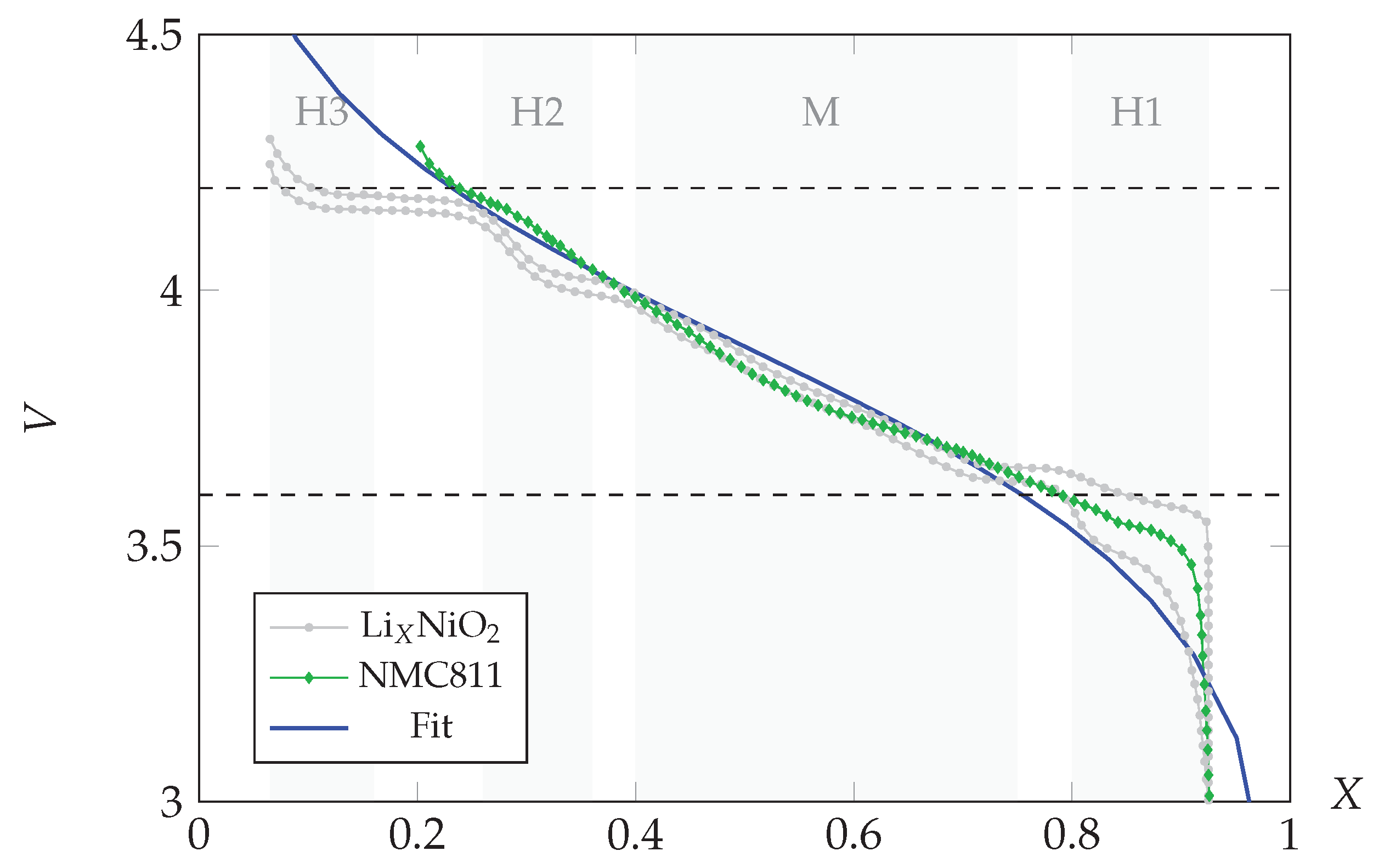
2.3. Simulation Parameters for High-Nickel NMC
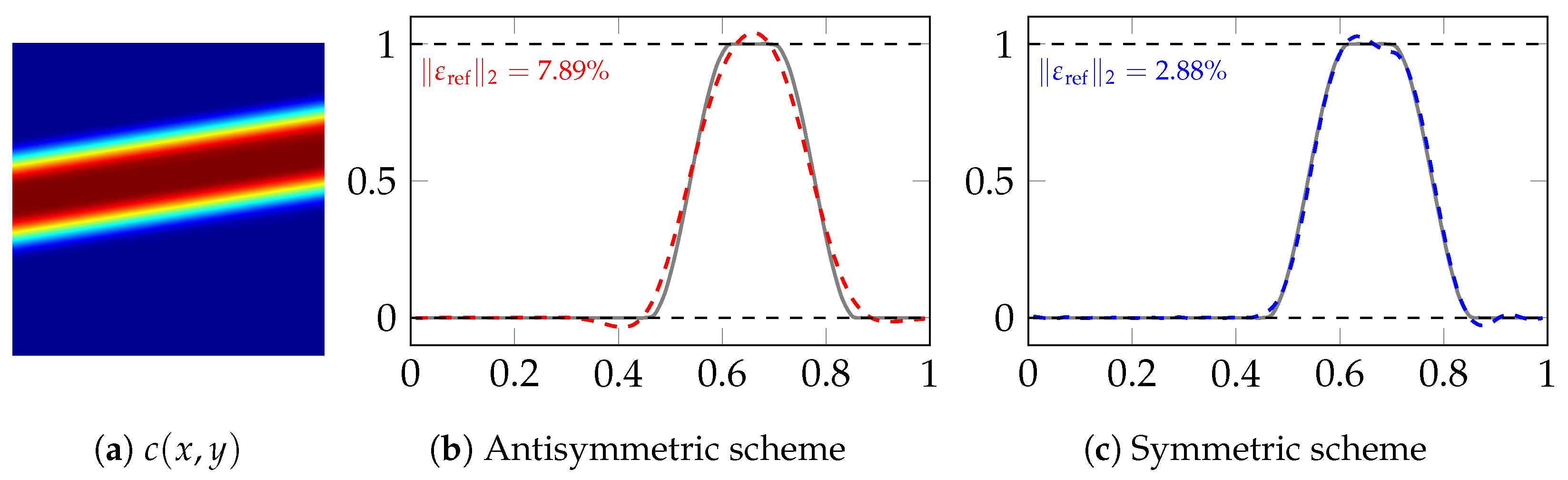
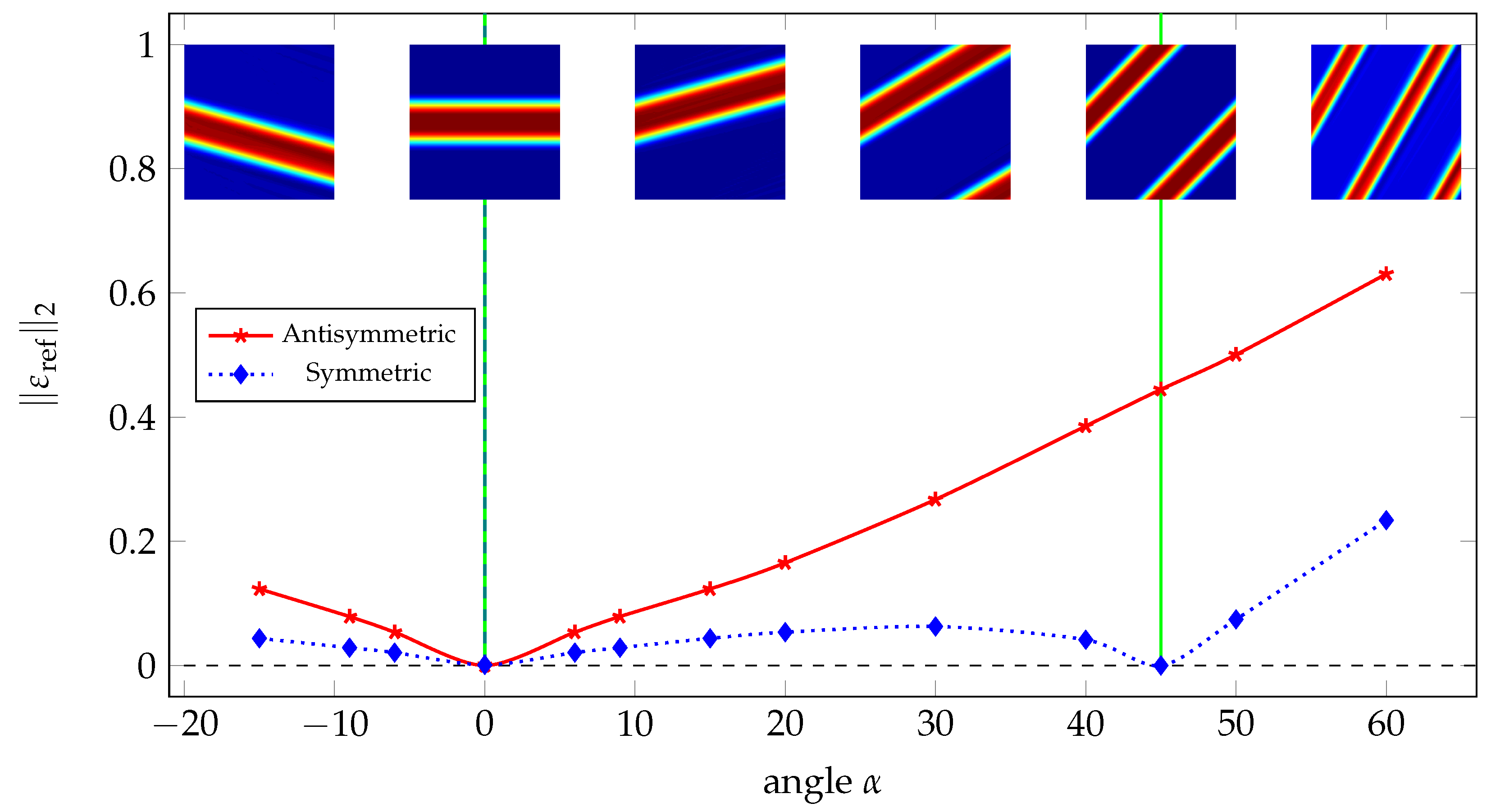
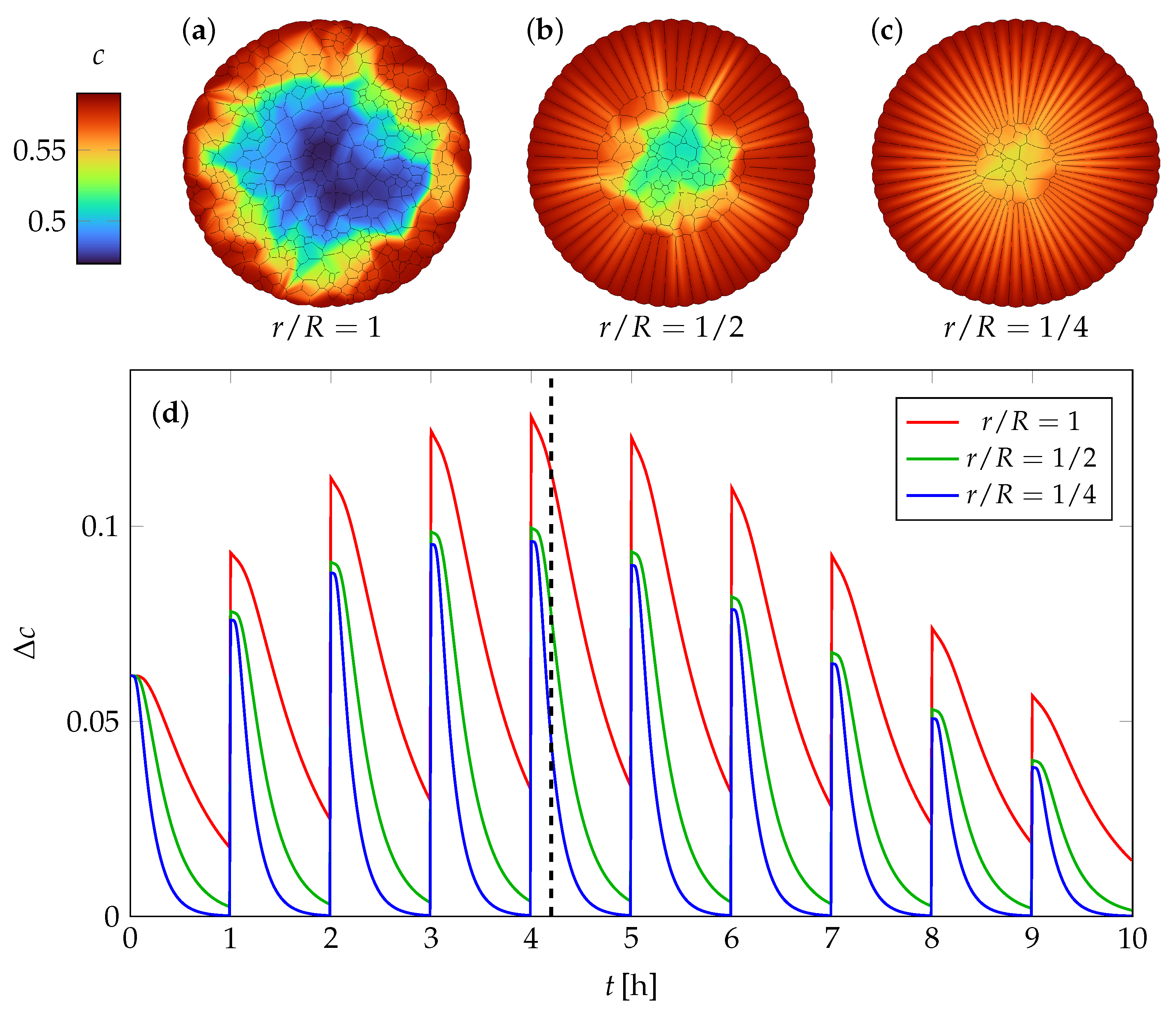
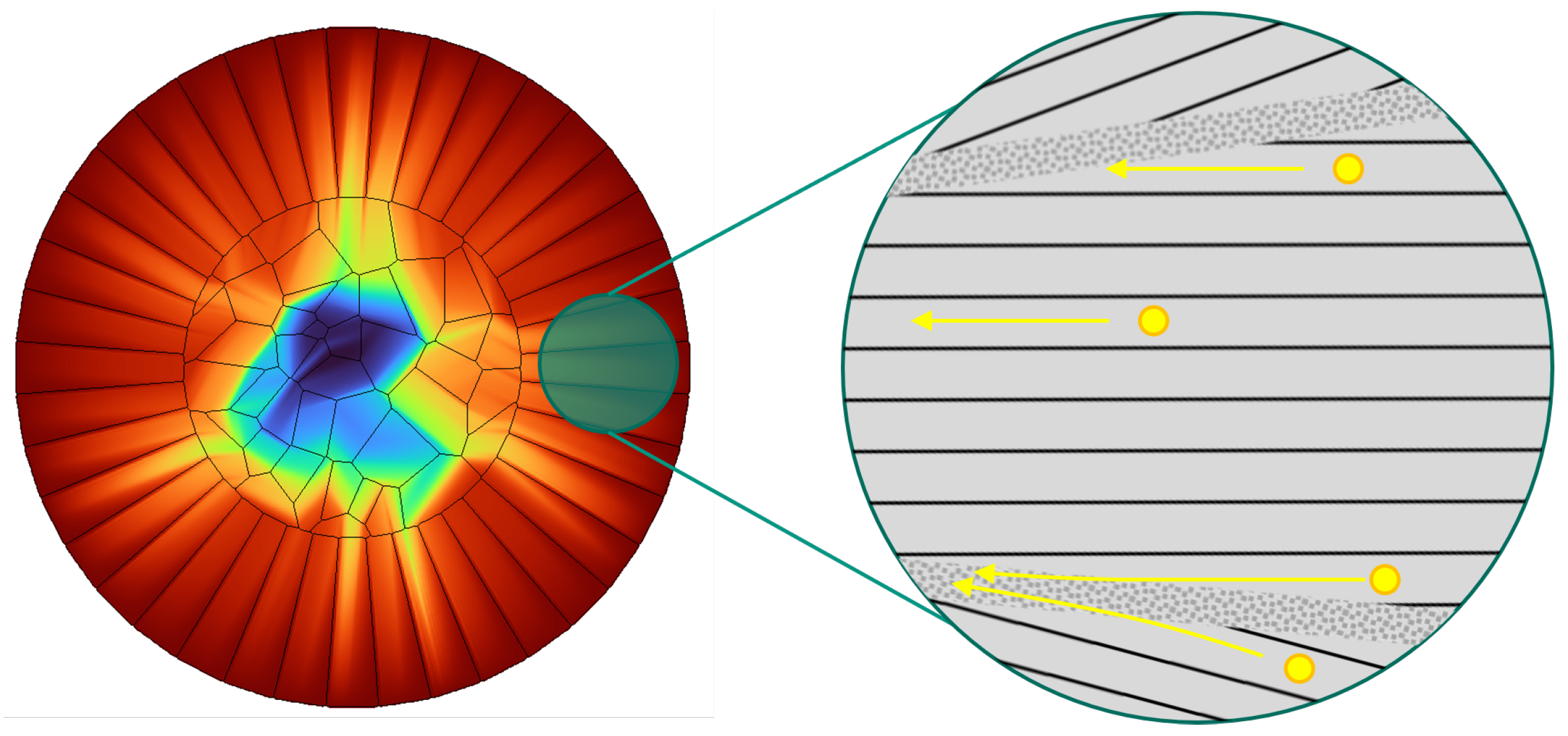
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
References
- Radin, M.D.; Hy, S.; Sina, M.; Fang, C.; Liu, H.; Vinckeviciute, J.; Zhang, M.; Whittingham, M.S.; Meng, Y.S.; Van der Ven, A. Narrowing the Gap between Theoretical and Practical Capacities in Li-Ion Layered Oxide Cathode Materials. Adv. Energy Mater. 2017, 7, 1602888. [Google Scholar] [CrossRef] [Green Version]
- Delmas, C.; Fouassier, C.; Hagenmuller, P. Structural classification and properties of the layered oxides. Physica B+C 1980, 99, 81–85. [Google Scholar] [CrossRef]
- Bouwman, P.J.; Boukamp, B.A.; Bouwmeester, H.J.M.; Notten, P.H.L. Influence of Diffusion Plane Orientation on Electrochemical Properties of Thin Film LiCoO2 Electrodes. J. Electrochem. Soc. 2002, 149, A699. [Google Scholar] [CrossRef]
- Noh, H.J.; Youn, S.; Yoon, C.S.; Sun, Y.K. Comparison of the structural and electrochemical properties of layered Li[NixCoyMnz]O2 (x = 1/3, 0.5, 0.6, 0.7, 0.8 and 0.85) cathode material for lithium-ion batteries. J. Power Sources 2013, 233, 121–130. [Google Scholar] [CrossRef]
- Kondrakov, A.O.; Schmidt, A.; Xu, J.; Geßwein, H.; Mönig, R.; Hartmann, P.; Sommer, H.; Brezesinski, T.; Janek, J. Anisotropic Lattice Strain and Mechanical Degradation of High- and Low-Nickel NCM Cathode Materials for Li-Ion Batteries. J. Phys. Chem. C 2017, 121, 3286–3294. [Google Scholar] [CrossRef]
- Xu, R.; de Vasconcelos, L.S.; Shi, J.; Li, J.; Zhao, K. Disintegration of Meatball Electrodes for LiNixMnyCozO2 Cathode Materials. Exp. Mech. 2018, 58, 549–559. [Google Scholar] [CrossRef]
- Kim, U.H.; Park, G.T.; Son, B.K.; Nam, G.W.; Liu, J.; Kuo, L.Y.; Kaghazchi, P.; Yoon, C.S.; Sun, Y.K. Heuristic solution for achieving long-term cycle stability for Ni-rich layered cathodes at full depth of discharge. Nat. Energy 2020, 5, 860–869. [Google Scholar] [CrossRef]
- Xu, Z.; Jiang, Z.; Kuai, C.; Xu, R.; Qin, C.; Zhang, Y.; Rahman, M.M.; Wei, C.; Nordlund, D.; Sun, C.J.; et al. Charge distribution guided by grain crystallographic orientations in polycrystalline battery materials. Nat. Commun. 2020, 11, 83. [Google Scholar] [CrossRef] [Green Version]
- Pfeiffer, L.F.; Jobst, N.; Gauckler, C.; Lindén, M.; Marinaro, M.; Passerini, S.; Wohlfahrt-Mehrens, M.; Axmann, P. Layered P2-NaxMn3/4Ni1/4O2 Cathode Materials For Sodium-Ion Batteries: Synthesis, Electrochemistry and Influence of Ambient Storage. Front. Energy Res. 2022, 10, 1–17. [Google Scholar] [CrossRef]
- Müller, M.; Schneider, L.; Bohn, N.; Binder, J.R.; Bauer, W. Effect of Nanostructured and Open-Porous Particle Morphology on Electrode Processing and Electrochemical Performance of Li-Ion Batteries. ACS Appl. Energy Mater. 2021, 4, 1993–2003. [Google Scholar] [CrossRef]
- Daubner, S.; Weichel, M.; Schneider, D.; Nestler, B. Modeling intercalation in cathode materials with phase-field methods: Assumptions and implications using the example of LiFePO4. Electrochim. Acta 2022, 421, 140516. [Google Scholar] [CrossRef]
- Lim, J.M.; Kim, H.; Cho, K.; Cho, M. Fundamental mechanisms of fracture and its suppression in Ni-rich layered cathodes: Mechanics-based multiscale approaches. Extrem. Mech. Lett. 2018, 22, 98–105. [Google Scholar] [CrossRef]
- Taghikhani, K.; Weddle, P.J.; Berger, J.R.; Kee, R.J. Modeling Coupled Chemo-Mechanical Behavior of Randomly Oriented NMC811 Polycrystalline Li-Ion Battery Cathodes. J. Electrochem. Soc. 2021, 168, 080511. [Google Scholar] [CrossRef]
- Cogswell, D.; Bazant, M. Coherency strain and the kinetics of phase separation in LiFePO4 nanoparticles. ACS Nano 2012, 6, 2215–2225. [Google Scholar] [CrossRef]
- Tang, M.; Belak, J.; Dorr, M. Anisotropic phase boundary morphology in nanoscale olivine electrode particles. J. Phys. Chem. C 2011, 115, 4922–4926. [Google Scholar] [CrossRef]
- Abdellahi, A.; Akyildiz, O.; Malik, R.; Thornton, K.; Ceder, G. Particle-size and morphology dependence of the preferred interface orientation in LiFePO4 nano-particles. J. Mater. Chem. A 2014, 2, 15437–15447. [Google Scholar] [CrossRef]
- Li, Y.; Zhang, K.; Pan, Y.; Yang, F. Anisotropic behavior in the lithiation of a silicon nanopillar. J. Energy Storage 2023, 57, 106271. [Google Scholar] [CrossRef]
- Kobayashi, R. Modeling and numerical simulations of dendritic crystal growth. Phys. D Nonlinear Phenom. 1993, 63, 410–423. [Google Scholar] [CrossRef]
- Schoof, E.; Schneider, D.; Streichhan, N.; Mittnacht, T.; Selzer, M.; Nestler, B. Multiphase-field modeling of martensitic phase transformation in a dual-phase microstructure. Int. J. Solids Struct. 2018, 134, 181–194. [Google Scholar] [CrossRef]
- Kim, S.G.; Kim, D.I.; Kim, W.T.; Park, Y.B. Computer simulations of two-dimensional and three-dimensional ideal grain growth. Phys. Rev. E 2006, 74, 061605. [Google Scholar] [CrossRef] [Green Version]
- Hötzer, J.; Reiter, A.; Hierl, H.; Steinmetz, P.; Selzer, M.; Nestler, B. The parallel multi-physics phase-field framework PACE3D. J. Comput. Sci. 2018, 26, 1–12. [Google Scholar] [CrossRef]
- Günter, S.; Yu, Q.; Krüger, J.; Lackner, K. Modelling of heat transport in magnetised plasmas using non-aligned coordinates. J. Comput. Phys. 2005, 209, 354–370. [Google Scholar] [CrossRef]
- Sharma, P.; Hammett, G.W. Preserving monotonicity in anisotropic diffusion. J. Comput. Phys. 2007, 227, 123–142. [Google Scholar] [CrossRef] [Green Version]
- Dubois, Y.; Commerçon, B. An implicit scheme for solving the anisotropic diffusion of heat and cosmic rays in the RAMSES code. Astron. Astrophys. 2016, 585, 1–10. [Google Scholar] [CrossRef] [Green Version]
- Van Es, B.; Koren, B.; de Blank, H.J. Finite-difference schemes for anisotropic diffusion. J. Comput. Phys. 2014, 272, 526–549. [Google Scholar] [CrossRef] [Green Version]
- Eiken, J.; Böttger, B.; Steinbach, I. Multiphase-field approach for multicomponent alloys with extrapolation scheme for numerical application. Phys. Rev. E 2006, 73, 066122. [Google Scholar] [CrossRef]
- Steinbach, I. Phase-field models in materials science. Model. Simul. Mater. Sci. Eng. 2009, 17, 073001. [Google Scholar] [CrossRef]
- De Biasi, L.; Schiele, A.; Roca-Ayats, M.; Garcia, G.; Brezesinski, T.; Hartmann, P.; Janek, J. Phase Transformation Behavior and Stability of LiNiO2 Cathode Material for Li-Ion Batteries Obtained from In Situ Gas Analysis and Operando X-Ray Diffraction. ChemSusChem 2019, 12, 2240–2250. [Google Scholar] [CrossRef]
- Choudhury, A.; Nestler, B. Grand-potential formulation for multicomponent phase transformations combined with thin-interface asymptotics of the double-obstacle potential. Phys. Rev. E 2012, 85, 021602. [Google Scholar] [CrossRef]
- Spring, K.W. Euler parameters and the use of quaternion algebra in the manipulation of finite rotations: A review. Mech. Mach. Theory 1986, 21, 365–373. [Google Scholar] [CrossRef]
- Heinz, A.; Neumann, P. Representation of orientation and disorientation data for cubic, hexagonal, tetragonal and orthorhombic crystals. Acta Crystallogr. Sect. Found. Crystallogr. 1991, 47, 780–789. [Google Scholar] [CrossRef]
- Li, W.; Reimers, J.; Dahn, J. In situ x-diffraction and electrochemical studies of Li1−xNiO2. Solid State Ionics 1993, 67, 123–130. [Google Scholar] [CrossRef]
- Van Der Ven, A.; Bhattacharya, J.; Belak, A.A. Understanding Li diffusion in Li-intercalation compounds. Accounts Chem. Res. 2013, 46, 1216–1225. [Google Scholar] [CrossRef]
- Trevisanello, E.; Ruess, R.; Conforto, G.; Richter, F.H.; Janek, J. Polycrystalline and Single Crystalline NCM Cathode Materials—Quantifying Particle Cracking, Active Surface Area, and Lithium Diffusion. Adv. Energy Mater. 2021, 11, 2003400. [Google Scholar] [CrossRef]
- Hoffrogge, P.; Mukherjee, A.; Nani, E.; Amos, P.K.; Wang, F.; Schneider, D.; Nestler, B. Multiphase-field model for surface diffusion and attachment kinetics in the grand-potential framework. Phys. Rev. E 2021, 103, 033307. [Google Scholar] [CrossRef]
- Yoon, C.S.; Park, K.J.; Kim, U.H.; Kang, K.H.; Ryu, H.H.; Sun, Y.K. High-Energy Ni-Rich Li[NixCoyMn1−x−y]O2 Cathodes via Compositional Partitioning for Next-Generation Electric Vehicles. Chem. Mater. 2017, 29, 10436–10445. [Google Scholar] [CrossRef]
- Mao, J.; Liu, X.; Liu, J.; Jiang, H.; Zhang, T.; Shao, G.; Ai, G.; Mao, W.; Feng, Y.; Yang, W.; et al. P2-type Na2/3Ni1/3Mn2/3O2 Cathode Material with Excellent Rate and Cycling Performance for Sodium-Ion Batteries. J. Electrochem. Soc. 2019, 166, A3980–A3986. [Google Scholar] [CrossRef] [Green Version]
- Van der Ven, A.; Ceder, G. Lithium diffusion mechanisms in layered intercalation compounds. J. Power Sources 2001, 97–98, 529–531. [Google Scholar] [CrossRef]
- Malik, R.; Burch, D.; Bazant, M.; Ceder, G. Particle size dependence of the ionic diffusivity. Nano Lett. 2010, 10, 4123–4127. [Google Scholar] [CrossRef]
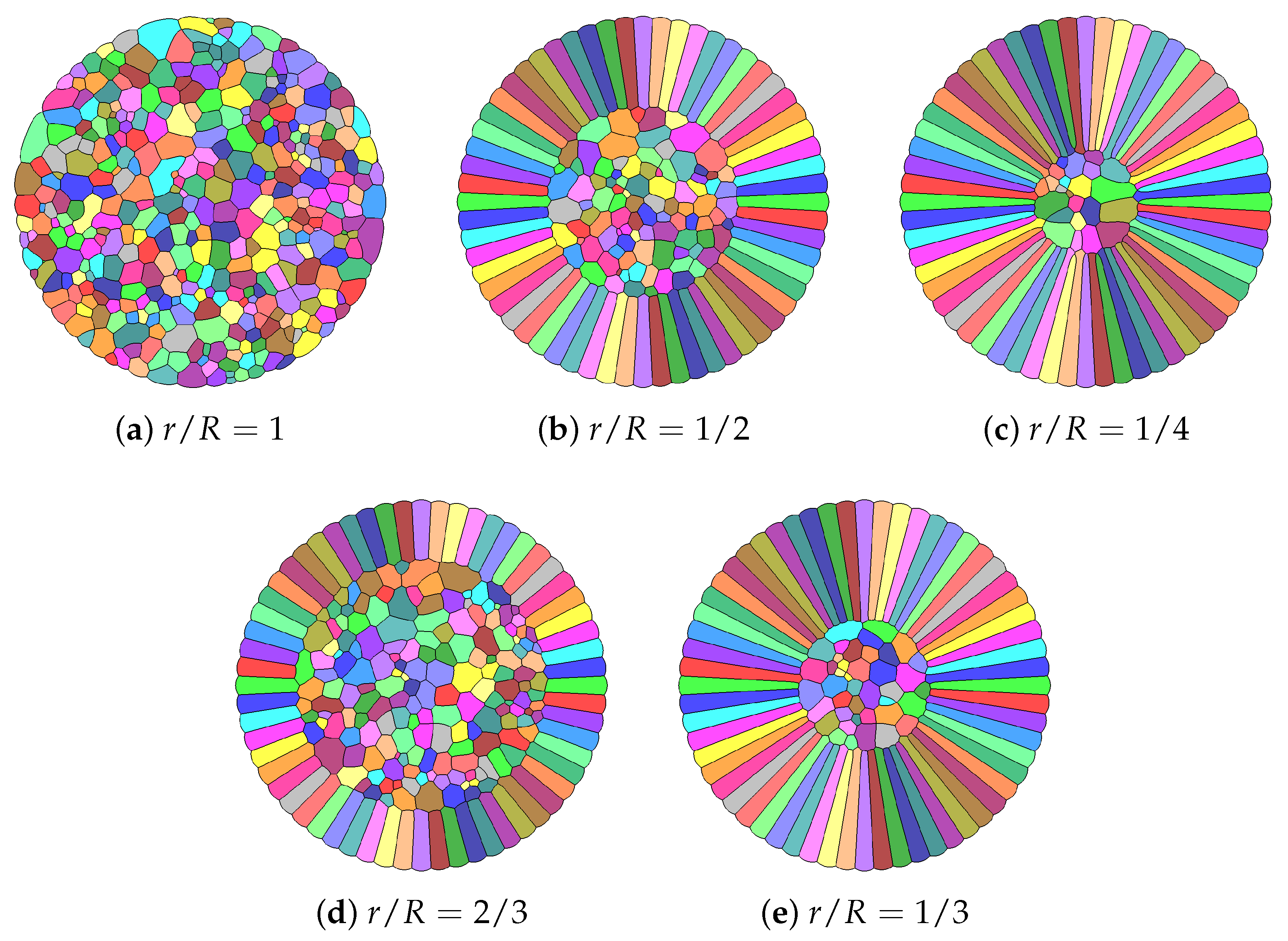


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Daubner, S.; Weichel, M.; Hoffrogge, P.W.; Schneider, D.; Nestler, B. Modeling Anisotropic Transport in Polycrystalline Battery Materials. Batteries 2023, 9, 310. https://doi.org/10.3390/batteries9060310
Daubner S, Weichel M, Hoffrogge PW, Schneider D, Nestler B. Modeling Anisotropic Transport in Polycrystalline Battery Materials. Batteries. 2023; 9(6):310. https://doi.org/10.3390/batteries9060310
Chicago/Turabian StyleDaubner, Simon, Marcel Weichel, Paul W. Hoffrogge, Daniel Schneider, and Britta Nestler. 2023. "Modeling Anisotropic Transport in Polycrystalline Battery Materials" Batteries 9, no. 6: 310. https://doi.org/10.3390/batteries9060310
APA StyleDaubner, S., Weichel, M., Hoffrogge, P. W., Schneider, D., & Nestler, B. (2023). Modeling Anisotropic Transport in Polycrystalline Battery Materials. Batteries, 9(6), 310. https://doi.org/10.3390/batteries9060310


.png)



