Figure 1.
A prosthesis operated by means of a neural headset: neurally actuated prosthetic prosthesis (NAPP).
Figure 1.
A prosthesis operated by means of a neural headset: neurally actuated prosthetic prosthesis (NAPP).
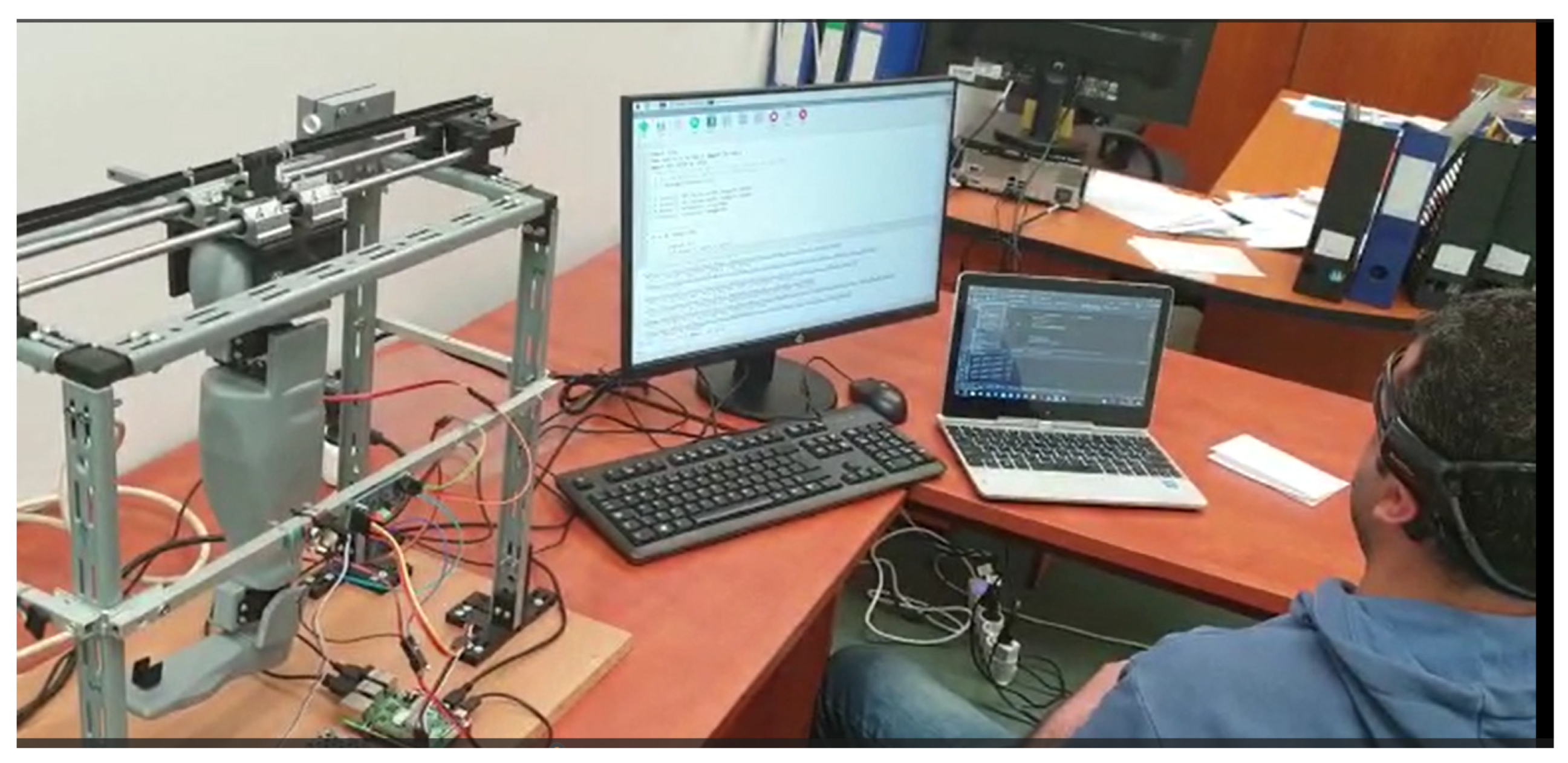
Figure 2.
The bionic leg experimental setup: 1. HBM’s WA200-series displacement sensor #260510079; 2. QuantumX CX22B-W computer S/N F0F9578297C8; 3. strain gauge MX840B S/N 0009E520A43; 4. Servomotor K-Power HBL090 ball joint; 5. K-Power HBL090 ankle actuator; 6. Accelerometer PCB Piezotronics 356A43 S/N LW348378; 7. Raspberry Pi4 model B 4GB RAM; and 8. power supply; 9. Motor JGB37-520 (12 V, 1:90, 107 RPM).
Figure 2.
The bionic leg experimental setup: 1. HBM’s WA200-series displacement sensor #260510079; 2. QuantumX CX22B-W computer S/N F0F9578297C8; 3. strain gauge MX840B S/N 0009E520A43; 4. Servomotor K-Power HBL090 ball joint; 5. K-Power HBL090 ankle actuator; 6. Accelerometer PCB Piezotronics 356A43 S/N LW348378; 7. Raspberry Pi4 model B 4GB RAM; and 8. power supply; 9. Motor JGB37-520 (12 V, 1:90, 107 RPM).
Figure 3.
Specimens for determining the physical/mechanical characteristics of the PLA used in the construction of the bionic leg: 1. compression test specimen; 2. tensile test specimen; 3. test specimen for bending stress.
Figure 3.
Specimens for determining the physical/mechanical characteristics of the PLA used in the construction of the bionic leg: 1. compression test specimen; 2. tensile test specimen; 3. test specimen for bending stress.
Figure 4.
The 3D printing of the three component elements of the bionic leg.
Figure 4.
The 3D printing of the three component elements of the bionic leg.
Figure 5.
An example of the 3D printing of the calf, with characteristics according to
Table 2.
Figure 5.
An example of the 3D printing of the calf, with characteristics according to
Table 2.
Figure 6.
The component elements of the bionic foot: a. sole; b. calf; c. knee.
Figure 6.
The component elements of the bionic foot: a. sole; b. calf; c. knee.
Figure 7.
The calf geometry, with a separately molded interior.
Figure 7.
The calf geometry, with a separately molded interior.
Figure 8.
Schematic of the foot in the initial position, and at one point in time, t.
Figure 8.
Schematic of the foot in the initial position, and at one point in time, t.
Figure 9.
The position of the local coordinate systems.
Figure 9.
The position of the local coordinate systems.
Figure 10.
The coordinates of the positions where the accelerometers were attached.
Figure 10.
The coordinates of the positions where the accelerometers were attached.
Figure 11.
The calf and sole insulation.
Figure 11.
The calf and sole insulation.
Figure 12.
INSTRON 8872 universal testing machine [
30].
Figure 12.
INSTRON 8872 universal testing machine [
30].
Figure 13.
Image correlation system (a—Camera 1, b—Camera 2, c—light source).
Figure 13.
Image correlation system (a—Camera 1, b—Camera 2, c—light source).
Figure 14.
Tested specimens: (a) traction, (b) bending, (c) compression.
Figure 14.
Tested specimens: (a) traction, (b) bending, (c) compression.
Figure 15.
Finite element discretization.
Figure 15.
Finite element discretization.
Figure 16.
“Revolved” connections with the imposition of angular displacements.
Figure 16.
“Revolved” connections with the imposition of angular displacements.
Figure 17.
The boundary conditions, gravitational acceleration, and rotations imposed in the joints on the geometric configuration from the initial time instant.
Figure 17.
The boundary conditions, gravitational acceleration, and rotations imposed in the joints on the geometric configuration from the initial time instant.
Figure 18.
The time variation in the experimentally and analytically determined acceleration components for sensor 1.
Figure 18.
The time variation in the experimentally and analytically determined acceleration components for sensor 1.
Figure 19.
The time variation in experimentally and analytically determined acceleration components for sensor 2.
Figure 19.
The time variation in experimentally and analytically determined acceleration components for sensor 2.
Figure 20.
The stress–strain curves of the ten specimens subjected to traction.
Figure 20.
The stress–strain curves of the ten specimens subjected to traction.
Figure 21.
The stress–strain curves of the ten specimens subjected to compression.
Figure 21.
The stress–strain curves of the ten specimens subjected to compression.
Figure 22.
The stress–strain curves of the ten specimens subjected to bending.
Figure 22.
The stress–strain curves of the ten specimens subjected to bending.
Figure 23.
Total displacement at 0.833 s.
Figure 23.
Total displacement at 0.833 s.
Figure 24.
Total displacement at 1.667 s.
Figure 24.
Total displacement at 1.667 s.
Figure 25.
Total displacement at 2.5 s.
Figure 25.
Total displacement at 2.5 s.
Figure 26.
Total displacement at 3.333 s.
Figure 26.
Total displacement at 3.333 s.
Figure 27.
Total displacement at 4.167 s.
Figure 27.
Total displacement at 4.167 s.
Figure 28.
Total displacement at 5 s.
Figure 28.
Total displacement at 5 s.
Figure 29.
Von Mises stress at 0.833 s.
Figure 29.
Von Mises stress at 0.833 s.
Figure 30.
Von Mises stress at 1.667 s.
Figure 30.
Von Mises stress at 1.667 s.
Figure 31.
Von Mises stress at 2.5 s.
Figure 31.
Von Mises stress at 2.5 s.
Figure 32.
Von Mises stress at 3.333 s.
Figure 32.
Von Mises stress at 3.333 s.
Figure 33.
Von Mises stress at 4.167 s.
Figure 33.
Von Mises stress at 4.167 s.
Figure 34.
Von Mises stress at 5 s.
Figure 34.
Von Mises stress at 5 s.
Figure 35.
A detail of the maximum equivalent voltage area.
Figure 35.
A detail of the maximum equivalent voltage area.
Figure 36.
The time variation in the knee–joint bond force components.
Figure 36.
The time variation in the knee–joint bond force components.
Figure 37.
The time variation in the link moment components in the knee joint.
Figure 37.
The time variation in the link moment components in the knee joint.
Figure 38.
The resultant force.
Figure 38.
The resultant force.
Figure 39.
The resulting moment.
Figure 39.
The resulting moment.
Figure 40.
The simplified geometry of the knee joint.
Figure 40.
The simplified geometry of the knee joint.
Figure 41.
The discretization of the knee joint in the contact area between the actuator bushing and the knee joint.
Figure 41.
The discretization of the knee joint in the contact area between the actuator bushing and the knee joint.
Figure 42.
The equivalent von Mises stresses in PLA.
Figure 42.
The equivalent von Mises stresses in PLA.
Figure 43.
The von Mises equivalent stresses from the bushing with the servomotor.
Figure 43.
The von Mises equivalent stresses from the bushing with the servomotor.
Figure 44.
The von Mises equivalent stresses from the fastening screws.
Figure 44.
The von Mises equivalent stresses from the fastening screws.
Figure 45.
The characteristic curves of the three tests performed for PLA.
Figure 45.
The characteristic curves of the three tests performed for PLA.
Figure 46.
The values of the transverse shrinkage coefficient of the studied PLA.
Figure 46.
The values of the transverse shrinkage coefficient of the studied PLA.
Figure 47.
The values of the longitudinal modulus of elasticity of the PLA.
Figure 47.
The values of the longitudinal modulus of elasticity of the PLA.
Figure 48.
The yield strength values of the PLA.
Figure 48.
The yield strength values of the PLA.
Figure 49.
The breaking point values of the PLA.
Figure 49.
The breaking point values of the PLA.
Table 1.
Physical and mechanical properties of PLA
1 [
33].
Table 1.
Physical and mechanical properties of PLA
1 [
33].
| Properties | Unit | PLA |
|---|
| | g/cm3 | 1.21–1.25 |
| σ | MPA | 21–60 |
| E | GPA | 0.35–3.5 |
| ε | % | 2.5–6 |
| Σ * | Nm/g | 16.8–48.0 |
| E * | kNm/g | 0.28–2.80 |
| Tg | °C | 45–60 |
| Tm | °C | 150–162 |
Table 2.
The printing characteristics of the leg specimens and components are as follows.
Table 2.
The printing characteristics of the leg specimens and components are as follows.
| Characteristics | Unit |
|---|
| Layer height | | 0.15 mm |
| First layer height | | 0.2 mm |
| Perimeters | | 2 lines |
| Solid layers | Top | 8 layers |
| Bottom | 5 layers |
| Temperature | Nozzle | 210 °C |
| Bed | 60 °C |
| Infill | Fill density | 30% |
| Fill pattern | Gyroid |
| Max volumetric speed | | 15 mm3/s |
Table 3.
The specimen dimensions required for compression.
Table 3.
The specimen dimensions required for compression.
| Properties | Diameter, mm | Height, mm |
|---|
| PLA 1 | 19.93 | 19.98 |
| PLA 2 | 19.87 | 19.97 |
| PLA 3 | 19.92 | 20.01 |
| PLA 4 | 19.89 | 19.98 |
| PLA 5 | 19.85 | 19.99 |
| PLA 6 | 19.86 | 20.00 |
| PLA 7 | 19.86 | 19.98 |
| PLA 8 | 19.87 | 20.00 |
| PLA 9 | 19.91 | 19.98 |
| PLA 10 | 19.89 | 19.99 |
| Average, mm | 19.885 | 19.998 |
| Coefficient of variation, % | 0.139 | 0.062 |
Table 4.
The specimen dimensions required for traction.
Table 4.
The specimen dimensions required for traction.
| Properties | Width, mm | Thickness, mm |
|---|
| PLA 1 | 10.02 | 4.94 |
| PLA 2 | 10.02 | 4.96 |
| PLA 3 | 9.99 | 4.90 |
| PLA 4 | 9.99 | 4.92 |
| PLA 5 | 9.99 | 4.91 |
| PLA 6 | 10.02 | 4.93 |
| PLA 7 | 10.02 | 4.92 |
| PLA 8 | 10.02 | 4.91 |
| PLA 9 | 10.01 | 4.95 |
| PLA 10 | 10.02 | 4.91 |
| Average, mm | 10.010 | 4.925 |
| Coefficient of variation, % | 0.141 | 0.398 |
Table 5.
The specimen dimensions required for bending.
Table 5.
The specimen dimensions required for bending.
| Properties | Width, mm | Thickness, mm |
|---|
| PLA 1 | 19.90 | 4.96 |
| PLA 2 | 19.94 | 4.91 |
| PLA 3 | 19.86 | 4.99 |
| PLA 4 | 19.90 | 4.96 |
| PLA 5 | 19.86 | 4.97 |
| PLA 6 | 19.96 | 4.97 |
| PLA 7 | 19.88 | 4.95 |
| PLA 8 | 20.01 | 4.91 |
| PLA 9 | 19.93 | 4.98 |
| PLA 10 | 19.89 | 4.94 |
| Average, mm | 19.913 | 4.954 |
| Coefficient of variation, % | 0.238 | 0.548 |
Table 6.
The calf and sole mass characteristics.
Table 6.
The calf and sole mass characteristics.
| Leg Component | The X-Coordinate of the Center of Mass Relative to the Local Frame (mm) | The Y-Coordinate of the Center of Mass Relative to the Local Frame (mm) | Mass (kg) |
|---|
| Calf | 0.72 | 100.45 | 0.757 |
| Sole | 36.39 | 33.60 | 0.167 |
Table 7.
The accelerometer positions relative to the sole-bound system.
Table 7.
The accelerometer positions relative to the sole-bound system.
| Position | Coordinate x2 (mm) | Coordinate y2 (mm) |
|---|
| Position 1—on the axis of the joint | 0 | 0 |
| Position 2—in the front part of the sole | 105 | 35.51 |
Table 8.
Specimen dimensions required for bending.
Table 8.
Specimen dimensions required for bending.
| Characteristic | Unit | Value |
|---|
| Distance between columns | mm | 455 |
| Distance between the lower and upper tank | mm | maxim 820 |
| Force cell | kN | ±25 |
| Piston stroke | mm | ±50 (100) |
| Sample holder system | | hydraulic |
Table 9.
Specimen dimensions required for bending.
Table 9.
Specimen dimensions required for bending.
| Characteristic | Unit | Value |
|---|
| Rooms | buc | 2 |
| Resolution | Mpx | 5 |
| Sensor size | | 2/3″ |
| Frame rate | Hz | Up to 560 |
| Communication | | USB 3.0 |
Table 10.
The mechanical characteristics of the tensile specimens.
Table 10.
The mechanical characteristics of the tensile specimens.
| Specimen | ν | Longitudinal Modulus of Elasticity, MPa | Drip Limit, MPa | Breaking Limit, MPa |
|---|
| PLA 1 | 0.304 | 1818.763 | 28.706 | 29.246 |
| PLA 2 | 0.298 | 1677.115 | 22.027 | 22.425 |
| PLA 3 | 0.322 | 1674.872 | 22.822 | 22.936 |
| PLA 4 | 0.324 | 1766.250 | 22.910 | 23.044 |
| PLA 5 | 0.303 | 1672.996 | 21.326 | 21.565 |
| PLA 6 | 0.288 | 2317.956 | 22.678 | 24.460 |
| PLA 7 | 0.370 | 1750.915 | 22.740 | 23.075 |
| PLA 8 | 0.316 | 1687.123 | 22.895 | 22.988 |
| PLA 9 | 0.316 | 1662.154 | 22.452 | 22.543 |
| PLA 10 | 0.316 | 1671.644 | 23.414 | 23.607 |
| Median | 0.316 | 1769.979 | 23.197 | 23.589 |
| Coefficient of variation, % | 7.031 | 11.272 | 8.695 | 9.010 |
Table 11.
The mechanical characteristics of the samples subjected to compression.
Table 11.
The mechanical characteristics of the samples subjected to compression.
| Specimen | ν | Longitudinal Modulus of Elasticity, MPa | Drip Limit, MPa | Breaking Limit, MPa |
|---|
| PLA 1 | 0.293 | 700.948 | 13.685 | 14.466 |
| PLA 2 | 0.273 | 733.401 | 12.845 | 14.634 |
| PLA 3 | 0.360 | 692.464 | 13.831 | 14.645 |
| PLA 4 | 0.279 | 710.637 | 12.513 | 14.488 |
| PLA 5 | 0.293 | 705.894 | 13.683 | 14.583 |
| PLA 6 | 0.306 | 700.088 | 13.390 | 14.385 |
| PLA 7 | 0.287 | 701.966 | 13.525 | 14.537 |
| PLA 8 | 0.311 | 716.933 | 13.552 | 14.440 |
| PLA 9 | 0.290 | 715.457 | 13.134 | 14.315 |
| PLA 10 | 0.307 | 730.164 | 13.555 | 14.546 |
| Median | 0.300 | 710.795 | 13.371 | 14.504 |
| Coefficient of variation, % | 8.141 | 1.873 | 3.121 | 0.731 |
Table 12.
The mechanical characteristics of the samples subjected to compression.
Table 12.
The mechanical characteristics of the samples subjected to compression.
| Specimen | Longitudinal Modulus of Elasticity, MPa | Drip Limit, MPa | Breaking Limit, MPa |
|---|
| PLA 1 | 2181.526 | 41.781 | 48.424 |
| PLA 2 | 2212.134 | 43.496 | 46.498 |
| PLA 3 | 2112.032 | 40.056 | 47.331 |
| PLA 4 | 2161.289 | 41.159 | 48.424 |
| PLA 5 | 2234.892 | 43.913 | 50.014 |
| PLA 6 | 2182.306 | 39.152 | 46.252 |
| PLA 7 | 2170.113 | 44.762 | 49.596 |
| PLA 8 | 2204.104 | 43.626 | 48.519 |
| PLA 9 | 2163.171 | 38.326 | 42.939 |
| PLA 10 | 2229.581 | 37.838 | 47.601 |
| Median | 2182.415 | 41.411 | 47.560 |
| Coefficient of variation, % | 1.809 | 6.033 | 4.253 |