Topology Optimization of an Automotive Seatbelt Bracket Considering Fatigue
Abstract
1. Introduction
2. Problem Formulation and Research Methodology
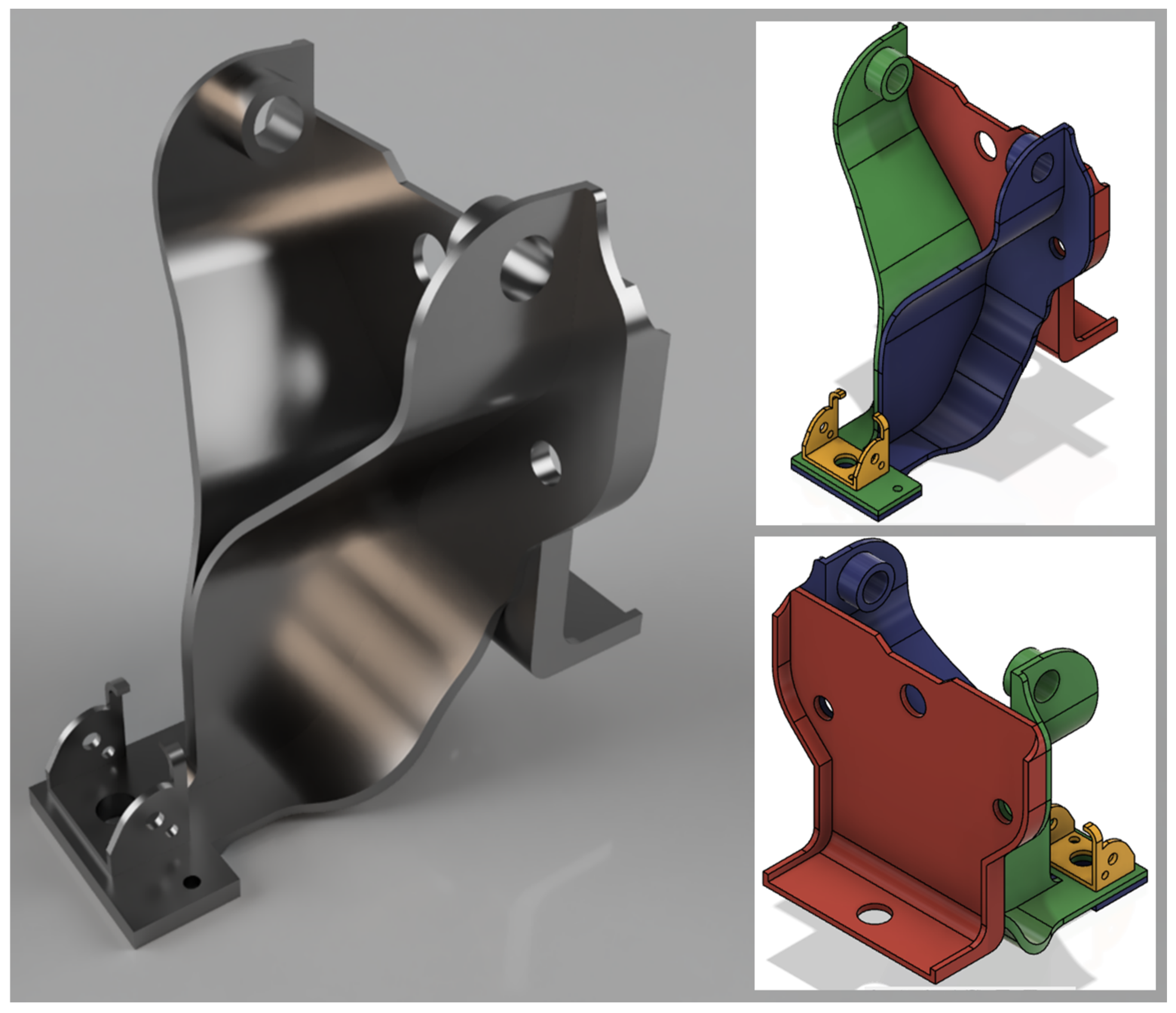
2.1. Assembly Geometry, Forces, and Material Properties
2.2. Finite Element Analysis and Topology Optimization
3. Results and Discussion
4. Conclusions
- The suggested methodology was effective to optimize the bracket assembly for the extreme loading and fatigue life required.
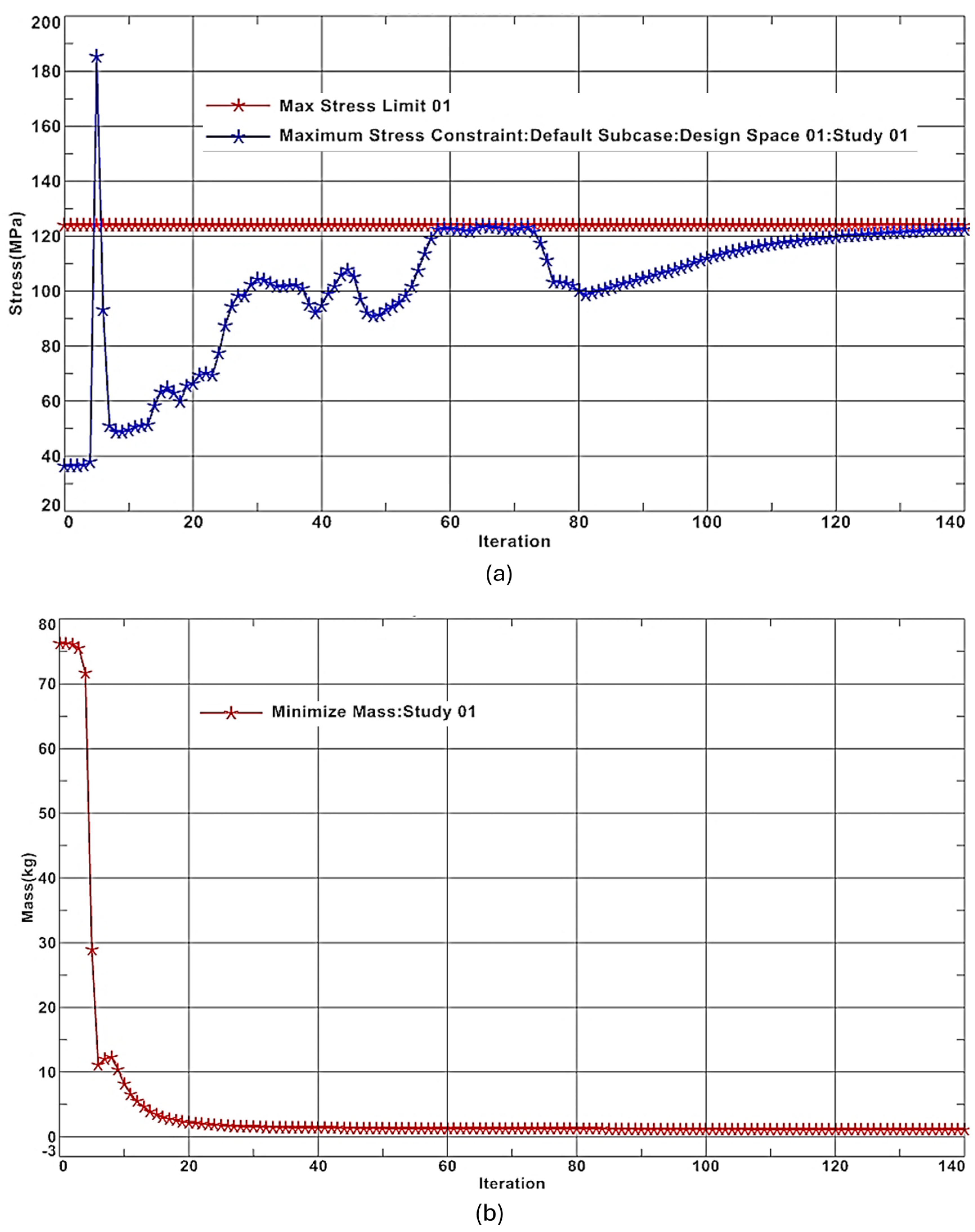
- The optimization process took 140 iterations and showed convergence stability after 80 iterations. The optimized part mass was 77% lower compared to original bracket assembly.
- The optimized geometry achieved 21% lower maximum stress compared to bracket assembly.
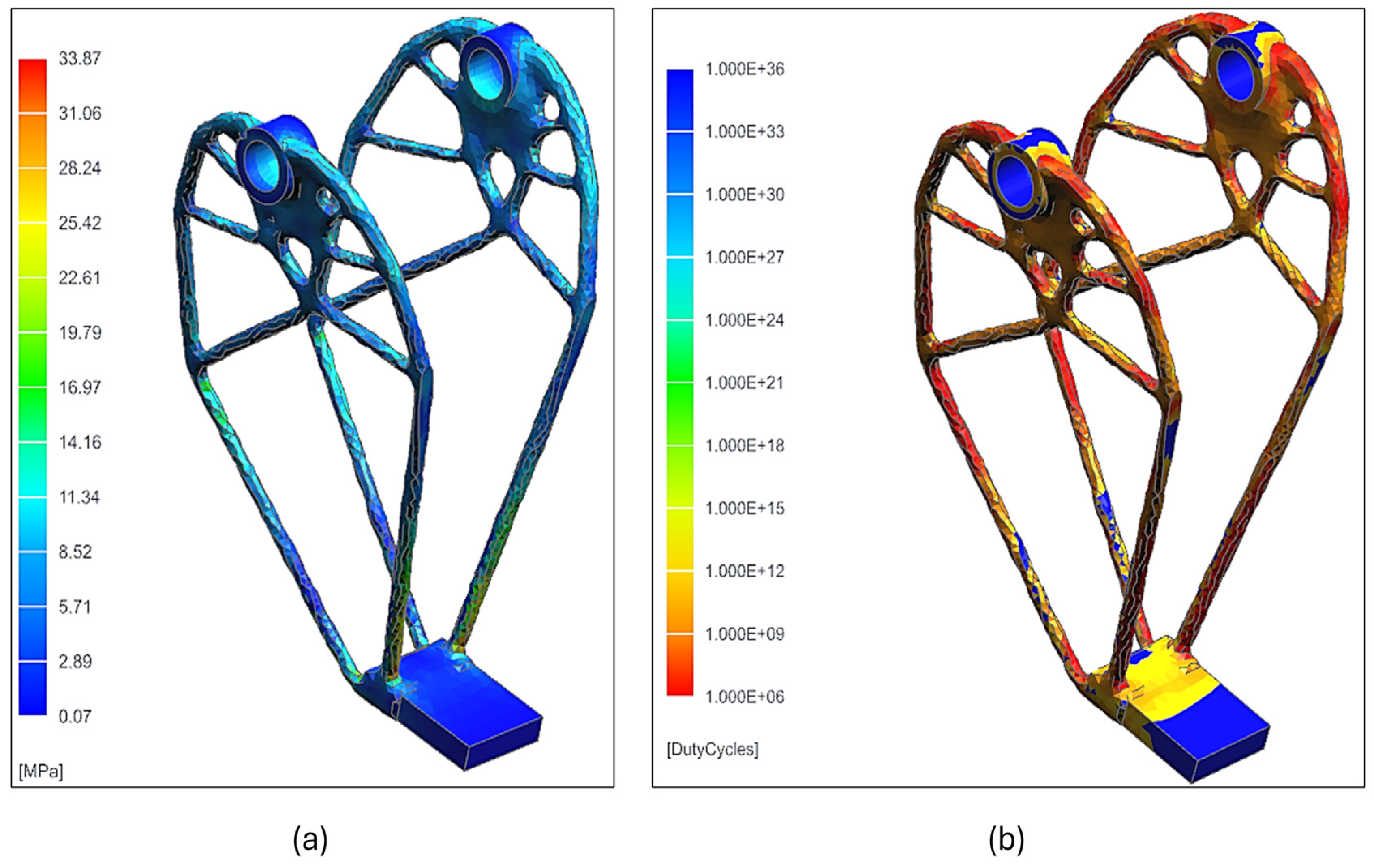
- Maximum stress during durability analysis was 33 MPa, and the minimum number of duty cycles was estimated to be one million cycles.
- The proposed design optimization scheme has proven computational efficiency due to its simplicity and use of standard commercially available solvers.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Abdelhafeez, A.M.; Soo, S.L.; Aspinwall, D.K.; Dowson, A.; Arnold, D. The Influence of Burr Formation and Feed Rate on the Fatigue Life of Drilled Titanium and Aluminium Alloys Used in Aircraft Manufacture. CIRP Ann. 2018, 67, 103–108. [Google Scholar] [CrossRef]
- Holmberg, E.; Torstenfelt, B.; Klarbring, A. Fatigue Constrained Topology Optimization. Struct. Multidisc. Optim. 2014, 50, 207–219. [Google Scholar] [CrossRef]
- Oest, J.; Lund, E. Topology Optimization with Finite-Life Fatigue Constraints. Struct. Multidisc. Optim. 2017, 56, 1045–1059. [Google Scholar] [CrossRef]
- Guo, X.; Zhang, W.; Zhong, W. Doing Topology Optimization Explicitly and Geometrically—A New Moving Morphable Components Based Framework. J. Appl. Mech. 2014, 81, 081009. [Google Scholar] [CrossRef]
- Brighenti, R. Patch Repair Design Optimisation for Fracture and Fatigue Improvements of Cracked Plates. Int. J. Solids Struct. 2007, 44, 1115–1131. [Google Scholar] [CrossRef]
- Li, Y.; Zhou, G.; Chang, T.; Yang, L.; Wu, F. Topology Optimization with Aperiodic Load Fatigue Constraints Based on Bidirectional Evolutionary Structural Optimization. CMES 2021, 130, 499–511. [Google Scholar] [CrossRef]
- Boursier Niutta, C.; Tridello, A.; Paolino, D.S. Fatigue Design of Additive Manufacturing Components through Topology Optimization: Comparison of Methodologies Based on the Defect Distribution and on the Stress Gradient. Fatigue Fract. Eng. Mater. Struct. 2023, 46, 3429–3445. [Google Scholar] [CrossRef]
- Hou, J.; Zhu, J.; Wang, J.; Zhang, W. Topology Optimization of the Multi-Fasteners Jointed Structure Considering Fatigue Constraints. Int. J. Simul. Multidisci. Des. Optim. 2018, 9, A4. [Google Scholar] [CrossRef]
- Lee, Y.-S.; González, J.A.; Lee, J.H.; Kim, Y.I.; Park, K.C.; Han, S. Structural Topology Optimization of the Transition Piece for an Offshore Wind Turbine with Jacket Foundation. Renew. Energy 2016, 85, 1214–1225. [Google Scholar] [CrossRef]
- Azad, M.M.; Kim, D.; Khalid, S.; Kim, H.S. Topology Optimization and Fatigue Life Estimation of Sustainable Medical Waste Shredder Blade. Mathematics 2022, 10, 1863. [Google Scholar] [CrossRef]
- Ye, H.; Zhang, Y.; Wei, N.; Wang, W.; Xiao, Y. The Fatigue Topology Optimization of Orthotropic Materials. J. Phys. Conf. Ser. 2023, 2535, 012027. [Google Scholar] [CrossRef]
- Desmorat, B.; Desmorat, R. Topology optimization in damage governed low cycle fatigue. Comptes Rendus Mécanique 2008, 336, 448–453. [Google Scholar] [CrossRef]
- Nvss, S.; Esakki, B.; Yang, L.-J.; Udayagiri, C.; Vepa, K.S. Design and Development of Unibody Quadcopter Structure Using Optimization and Additive Manufacturing Techniques. Designs 2022, 6, 8. [Google Scholar] [CrossRef]
- Zhao, T.; Zhang, Y.; Ou, Y.; Ding, W.; Cheng, F. Fail-Safe Topology Optimization Considering Fatigue. Struct. Multidisc. Optim. 2023, 66, 132. [Google Scholar] [CrossRef]
- Jeong, S.H.; Lee, J.W.; Yoon, G.H.; Choi, D.H. Topology Optimization Considering the Fatigue Constraint of Variable Amplitude Load Based on the Equivalent Static Load Approach. Appl. Math. Model. 2018, 56, 626–647. [Google Scholar] [CrossRef]
- Kudarauskas, N. Analysis of Emergency Braking of a Vehicle. Transport 2007, 22, 154–159. [Google Scholar] [CrossRef]
- Xiao, S.; Yang, J.; Crandall, J.R. Investigation of Chest Injury Mechanism Caused by Different Seatbelt Loads in Frontal Impact. Acta Bioeng. Biomech. 2017, 19, 53–62. [Google Scholar] [CrossRef]
- Múčka, P. International Roughness Index Thresholds Based on Whole-Body Vibration in Passenger Cars. Transp. Res. Rec. 2021, 2675, 305–320. [Google Scholar] [CrossRef]
- Kumaresh, S.A.; Aladdin, M.F. A Study of Vibration Transmission on Seated Person in Passenger Vehicle. AIP Conf. Proc. 2019, 2137, 040001. [Google Scholar]
- Pan-Zagorski, W.; Johnson, P.W.; Pereny, M.; Kim, J.H. Automotive Seat Comfort and Vibration Performance Evaluation in Dynamic Settings. Appl. Sci. 2022, 12, 4033. [Google Scholar] [CrossRef]
- ISO 2631-1:1997; Mechanical Vibration and Shock—Evaluation of Human Exposure to Whole-Body Vibration. Part 1: General Requirements. International Organization for Standardization: Geneva, Switzerland, 1997. Available online: https://www.iso.org/standard/7612.html (accessed on 28 August 2024).
- Kaščak, J.; Gašpár, Š.; Paško, J.; Husár, J.; Knapčíková, L. Polylactic Acid and Its Cellulose Based Composite as a Significant Tool for the Production of Optimized Models Modified for Additive Manufacturing. Sustainability 2021, 13, 1256. [Google Scholar] [CrossRef]





| Property | Value |
|---|---|
| Density (kg/m3) | 7870 |
| Modulus of elasticity (GPa) | 200 |
| Poisson’s ratio | 0.27 |
| Yield stress (MPa) | 200 |
| Tensile strength (MPa) | 500 |
| Endurance limit (MPa) | 260 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hassan, A.A.; Biswas, B. Topology Optimization of an Automotive Seatbelt Bracket Considering Fatigue. Designs 2024, 8, 99. https://doi.org/10.3390/designs8050099
Hassan AA, Biswas B. Topology Optimization of an Automotive Seatbelt Bracket Considering Fatigue. Designs. 2024; 8(5):99. https://doi.org/10.3390/designs8050099
Chicago/Turabian StyleHassan, Ali Abdelhafeez, and Bikram Biswas. 2024. "Topology Optimization of an Automotive Seatbelt Bracket Considering Fatigue" Designs 8, no. 5: 99. https://doi.org/10.3390/designs8050099
APA StyleHassan, A. A., & Biswas, B. (2024). Topology Optimization of an Automotive Seatbelt Bracket Considering Fatigue. Designs, 8(5), 99. https://doi.org/10.3390/designs8050099







