Incremental Viscoelastic Damage Contact Models for Asphalt Mixture Fracture Assessment
Abstract
:1. Introduction
2. Objectives and Scope
3. Three-Dimensional Numerical Formulation
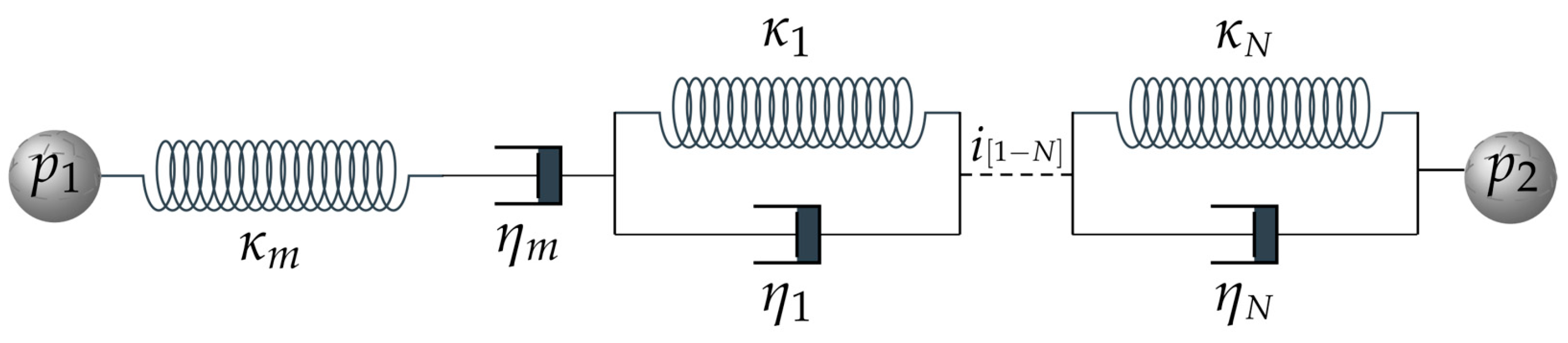
3.1. Viscoelastic Contact Model
3.2. Damage Numerical Modelling
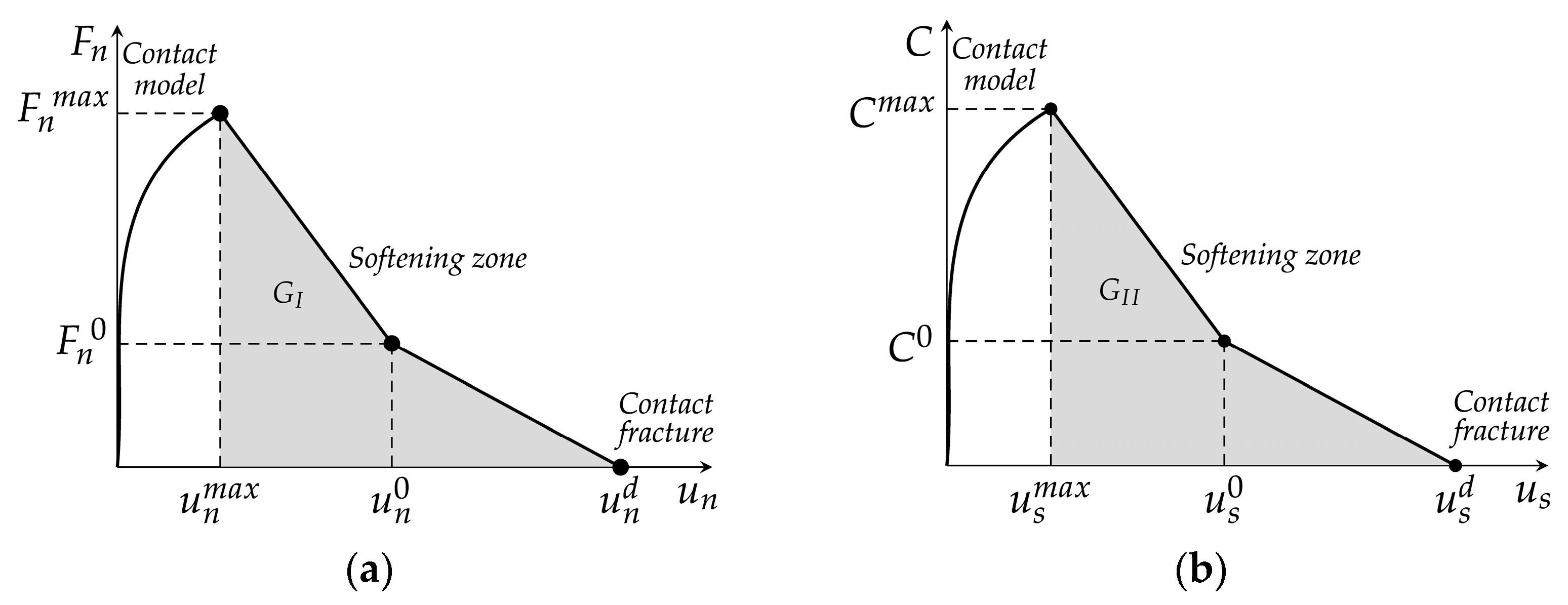
3.2.1. GK-Brittle Damage Model
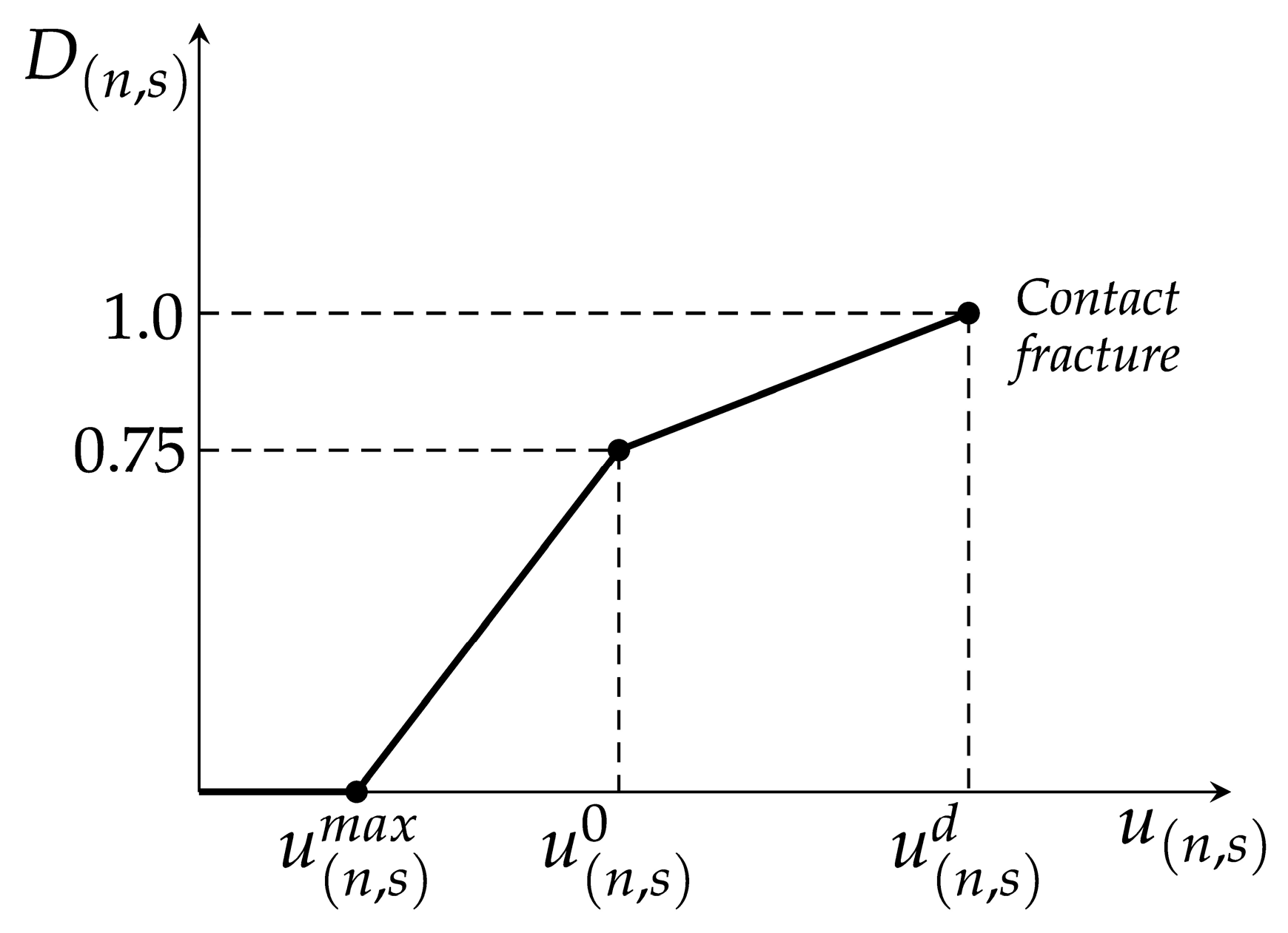
3.2.2. GK-Bilinear Softening Damage Model
4. Parametric Study of the Proposed GK-Damage Contact Models
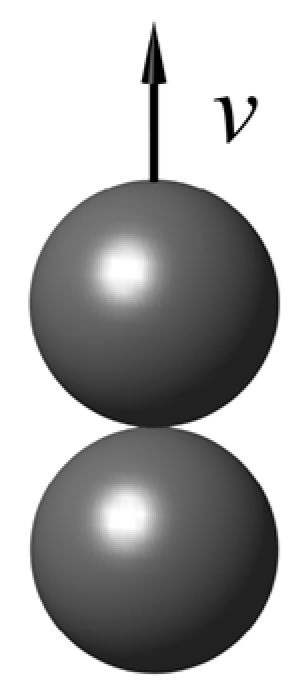
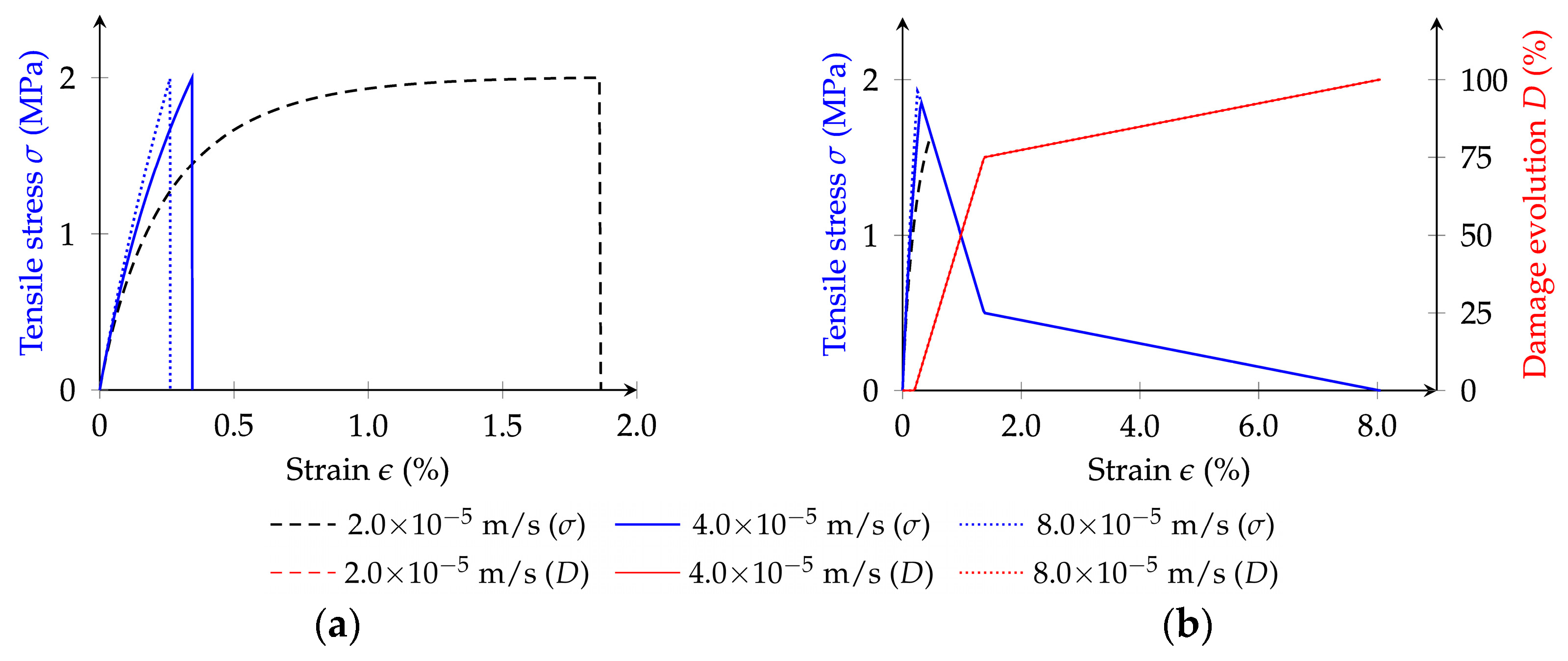
4.1. Influence of the Loading Velocity
4.2. Influence of the Maximum Contact Tensile Strength
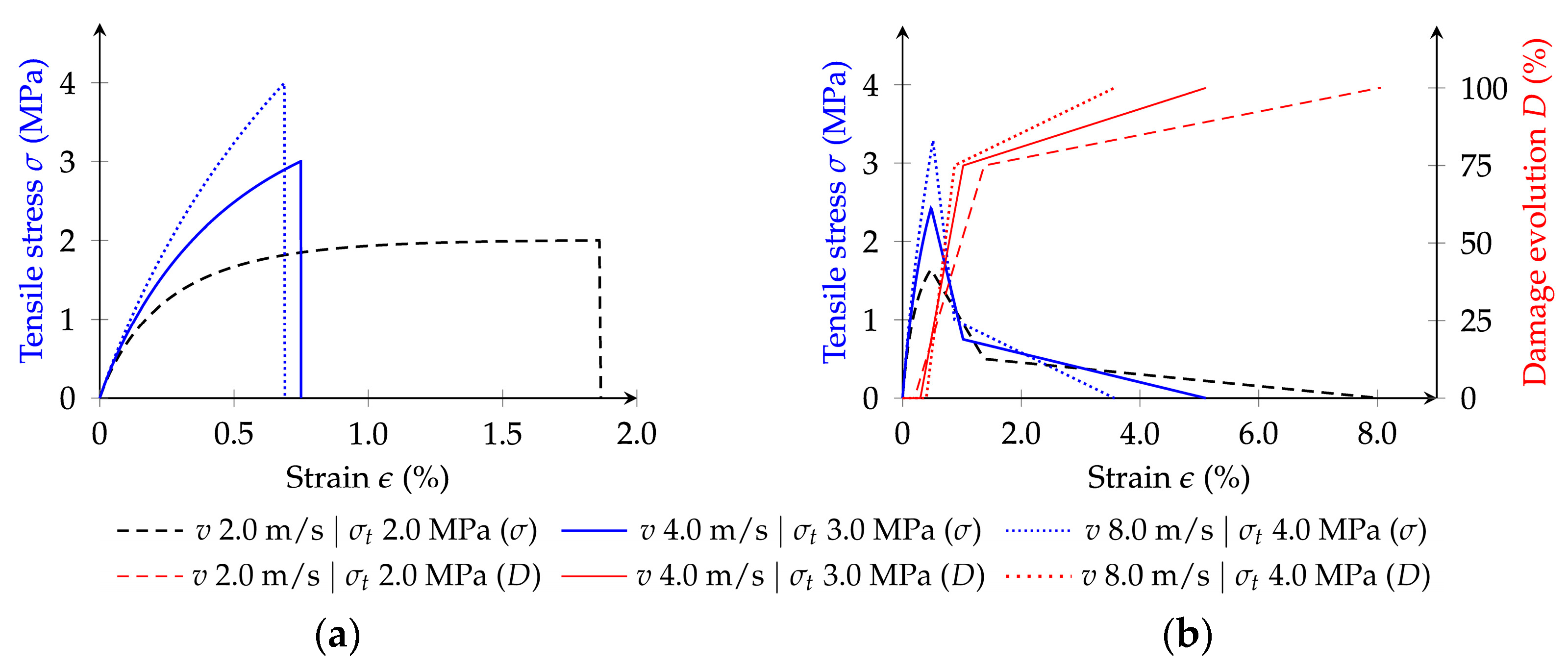
4.3. Influence of Both the Loading Velocity and Maximum Contact Tensile Strength
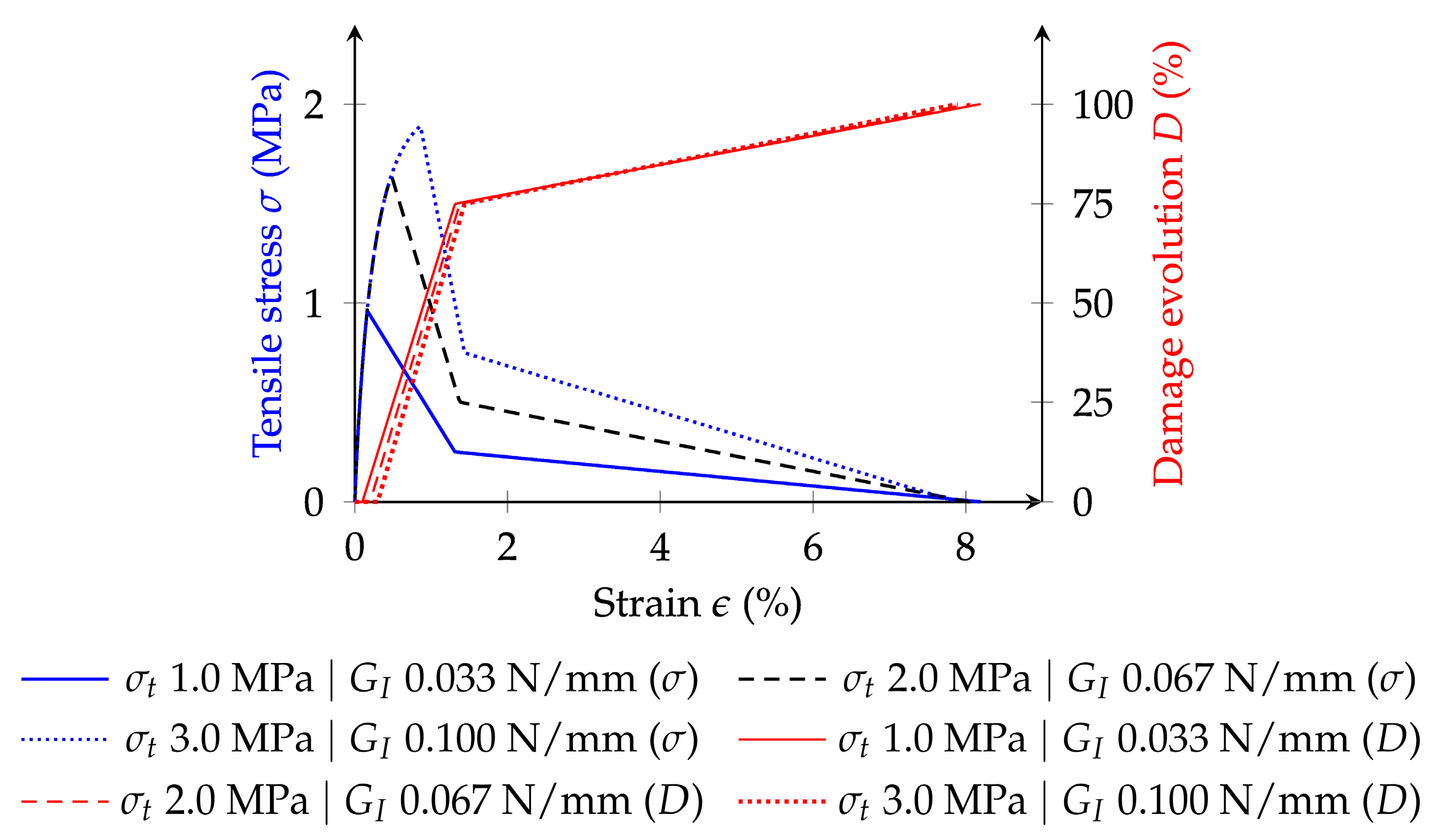
4.4. Influence of the Contact Fracture Energy in Mode I
4.5. Influence of Both the Fracture Energy in Mode I and Maximum Contact Tensile Strength
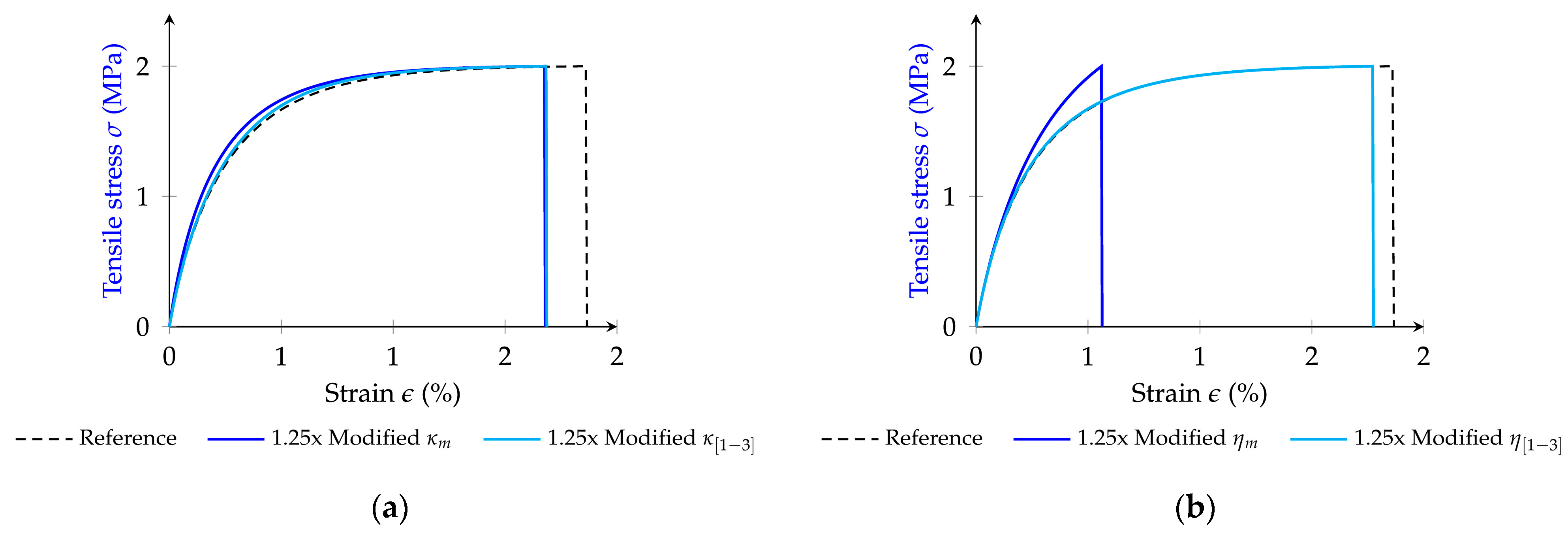
4.6. Influence of the GK Contact Viscoelastic Model Parameters
5. Numerical Simulations
5.1. Experimental and Numerical Tests
5.2. Numerical Specimen Calibration
5.2.1. Asphalt Mastic Model
5.2.2. Asphalt Mixture Model
5.3. GK Viscoelastic Contact Parameters for DEM Numerical Simulations
5.4. Brittle and Bilinear Strength Parameters
6. Results
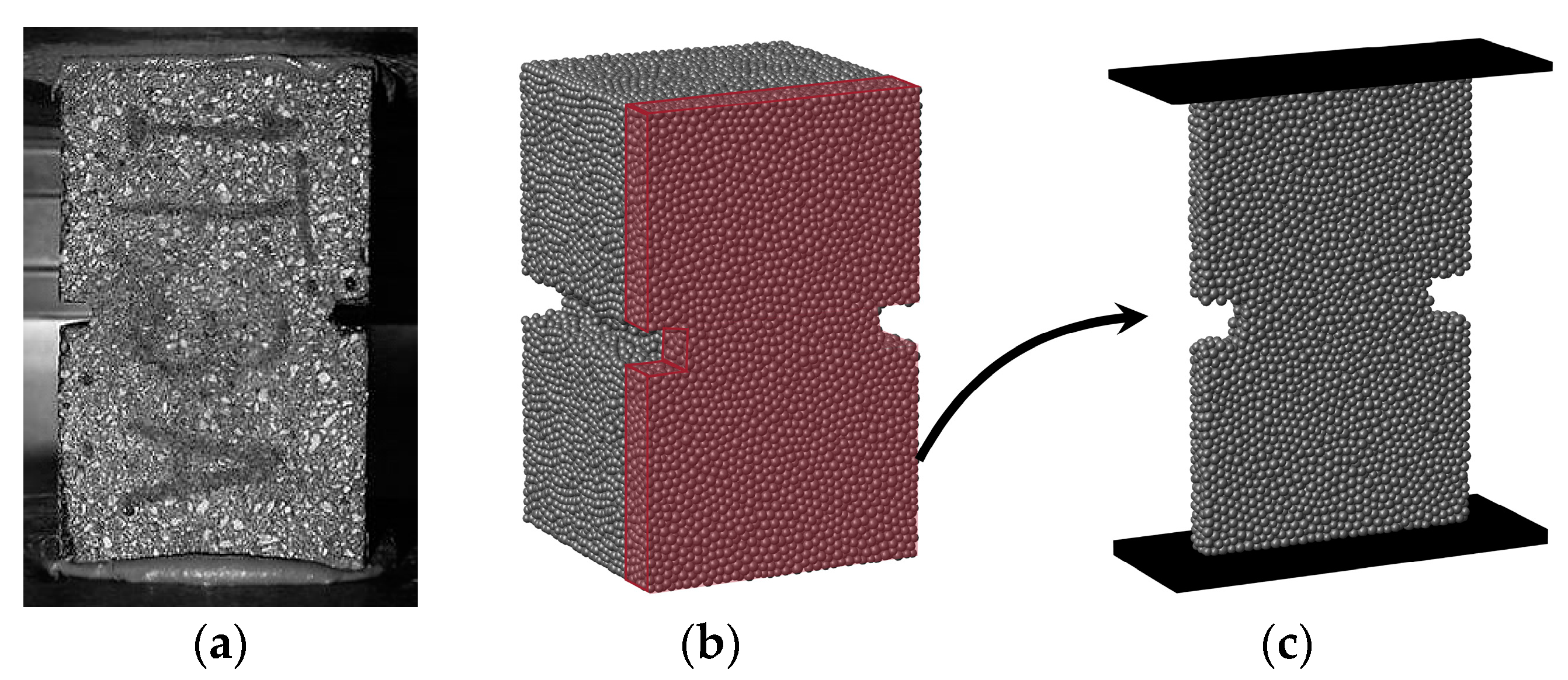
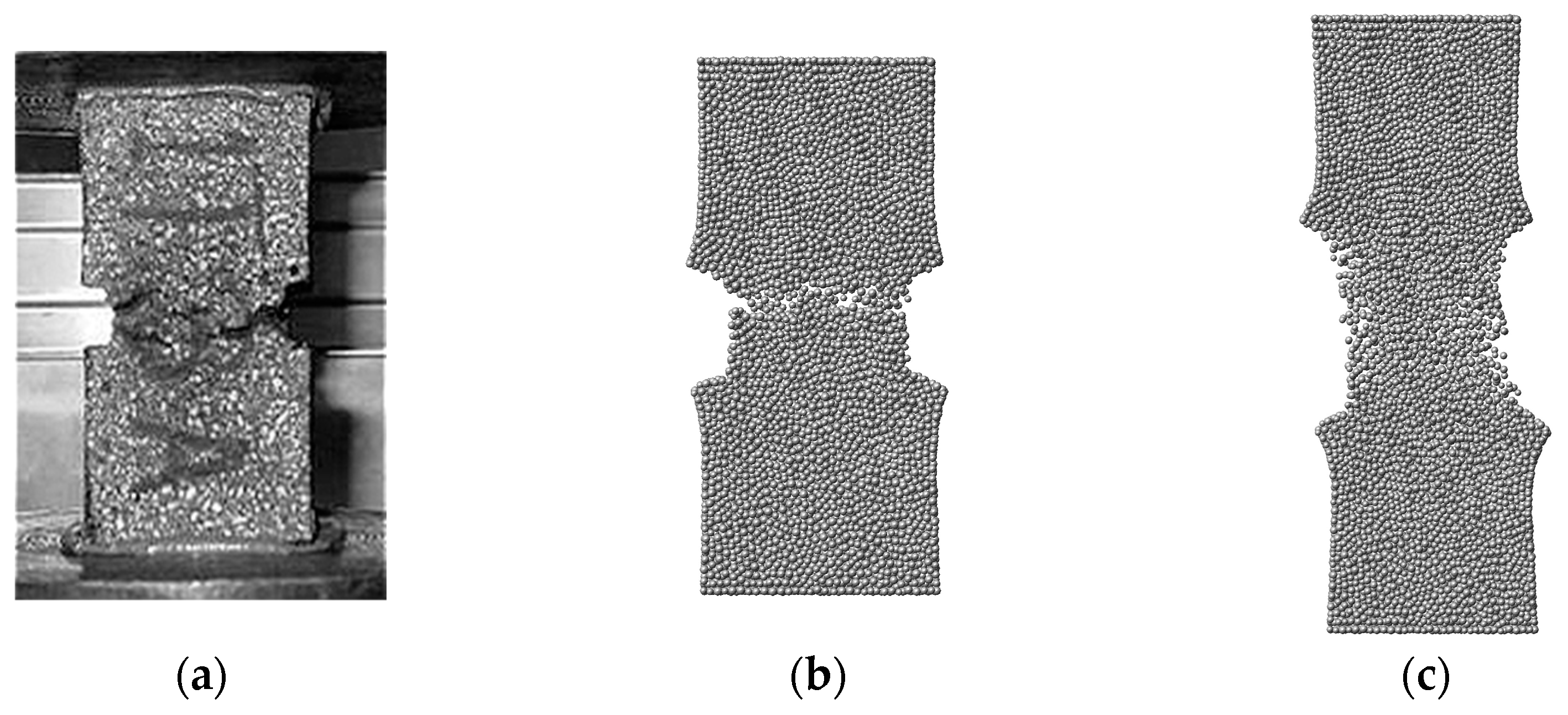
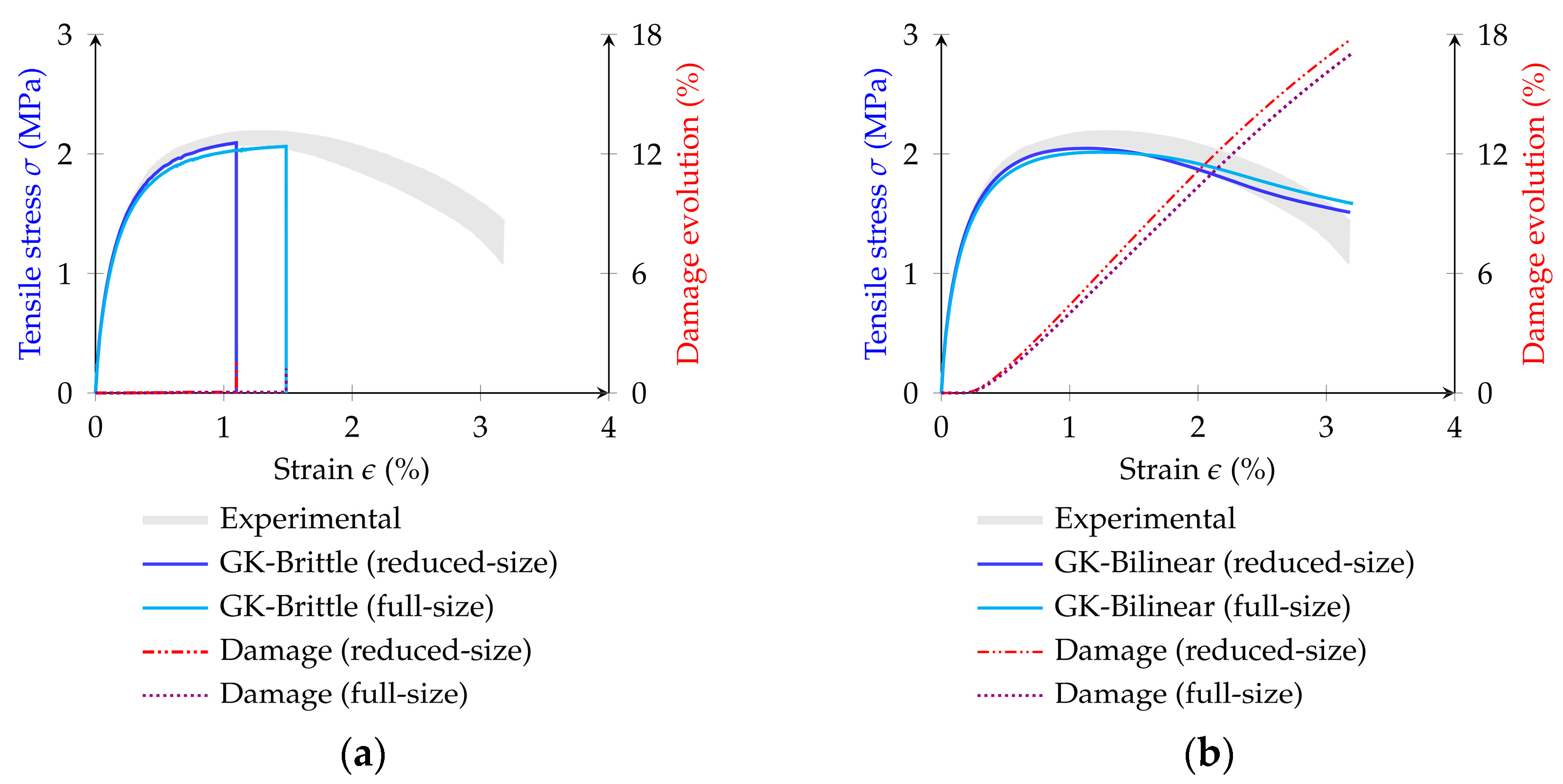
6.1. Asphalt Mastic Numerical Modelling—Reduced-Size Specimen
6.2. Asphalt Mastic Numerical Modelling—Full-Size Specimen
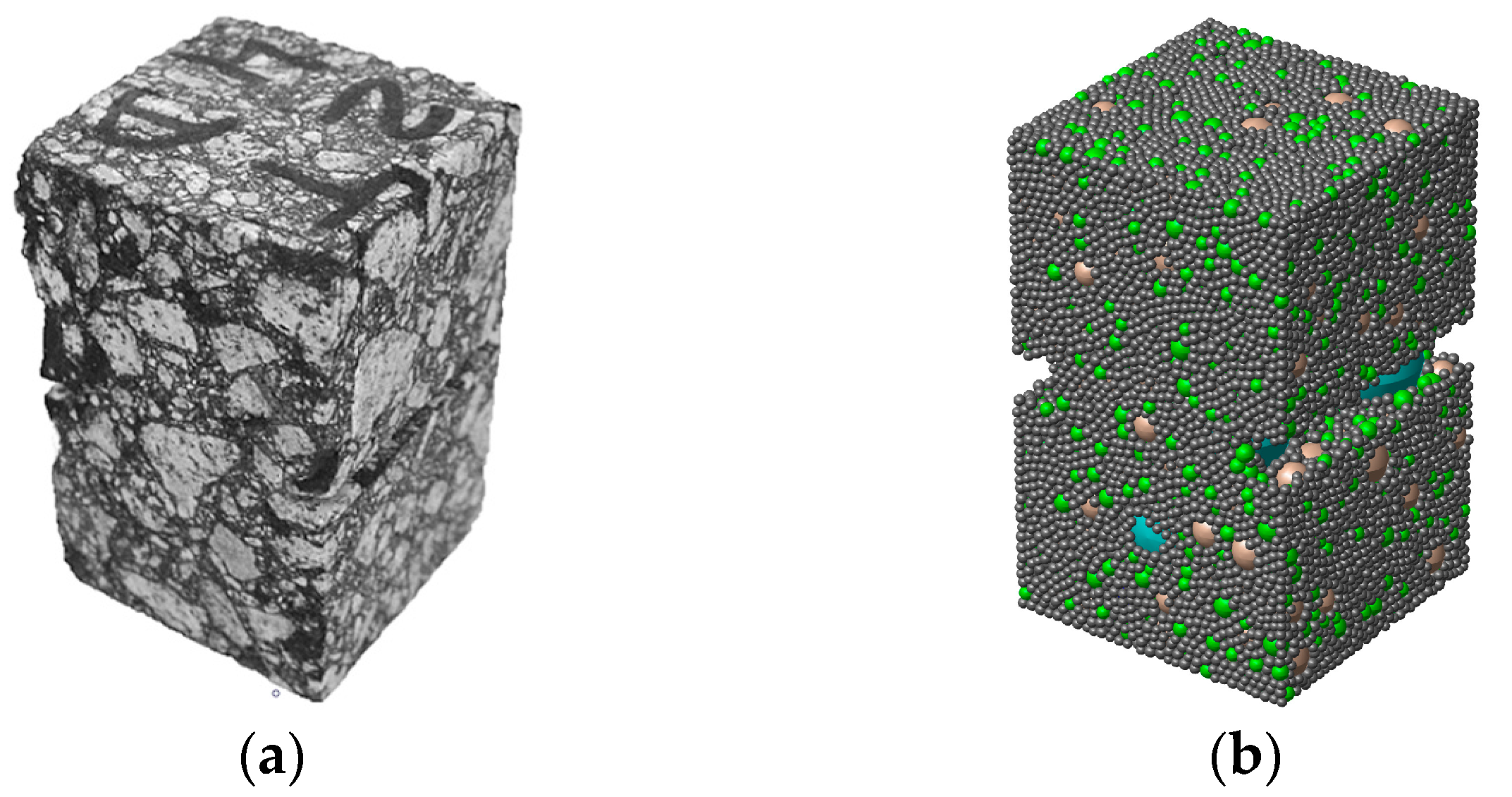
6.3. Asphalt Mixture Numerical Modelling
6.3.1. Stress–Strain Response
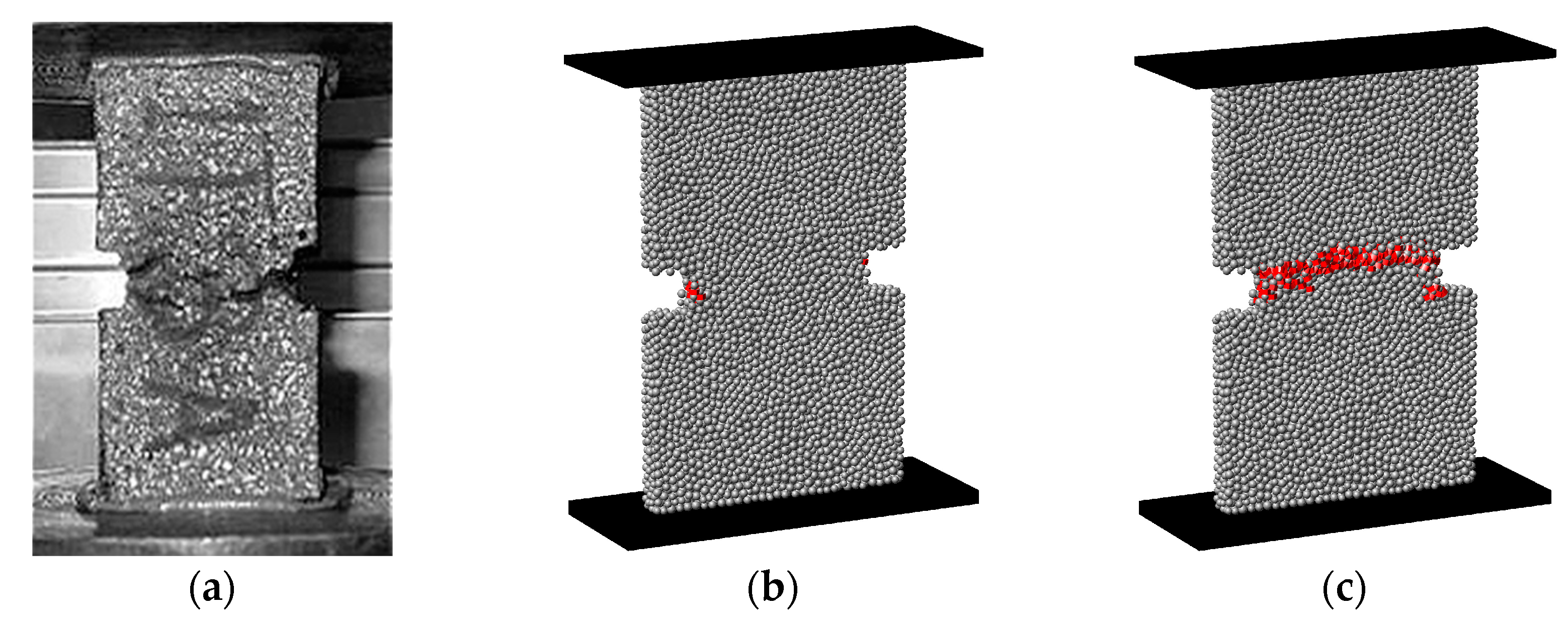
6.3.2. Contact Damage Evolution Assessment
6.3.3. Contact Property Fine-Tuning
7. Summary and Conclusions
- -
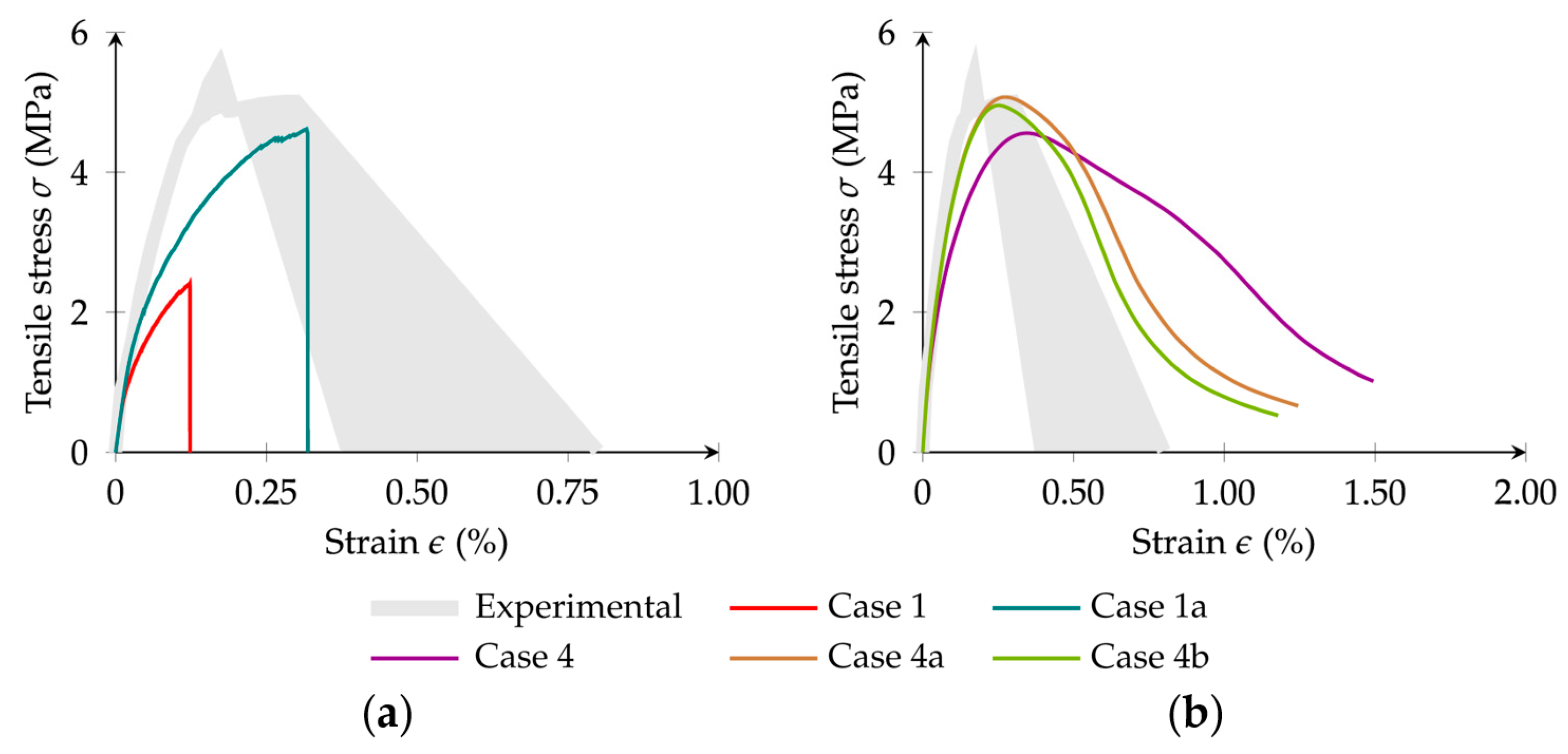
- The impact of the GK-Damage parameters and loading velocity was evaluated for both the proposed GK-Damage models for a single contact. For the GK-Bilinear model, adjustments in the contact fracture energy in mode I and the maximum contact tensile strength can be applied to control the predicted peak stress and post-peak ductility. The viscosity element affects both GK-Damage contact models, while the stiffness parameters ( and ) have a more significant influence on the GK-Brittle model response. The effect of the viscosity of the Kelvin chain () is negligible on both GK-Damage models. The loading velocity directly influences the response of both GK-Damage models.
- -
- The proposed GK-Bilinear model improves the numerical representation of asphalt mixtures, especially when adopted for aggregate-to-mastic and mastic-to-mastic contacts, and aggregate-to-aggregate contacts follow a softening damage approach. A fine-tuning of the GK-Damage contact properties is shown to improve numerical predictions for both GK-Damage models.
- -
- Within a GK-Brittle model, the model adopted for the aggregate-to-aggregate contacts, with or without cohesion, does not significantly influence the predicted macroscopic responses. Its influence is more pronounced in particle models that adopt a GK-Bilinear model.
- -
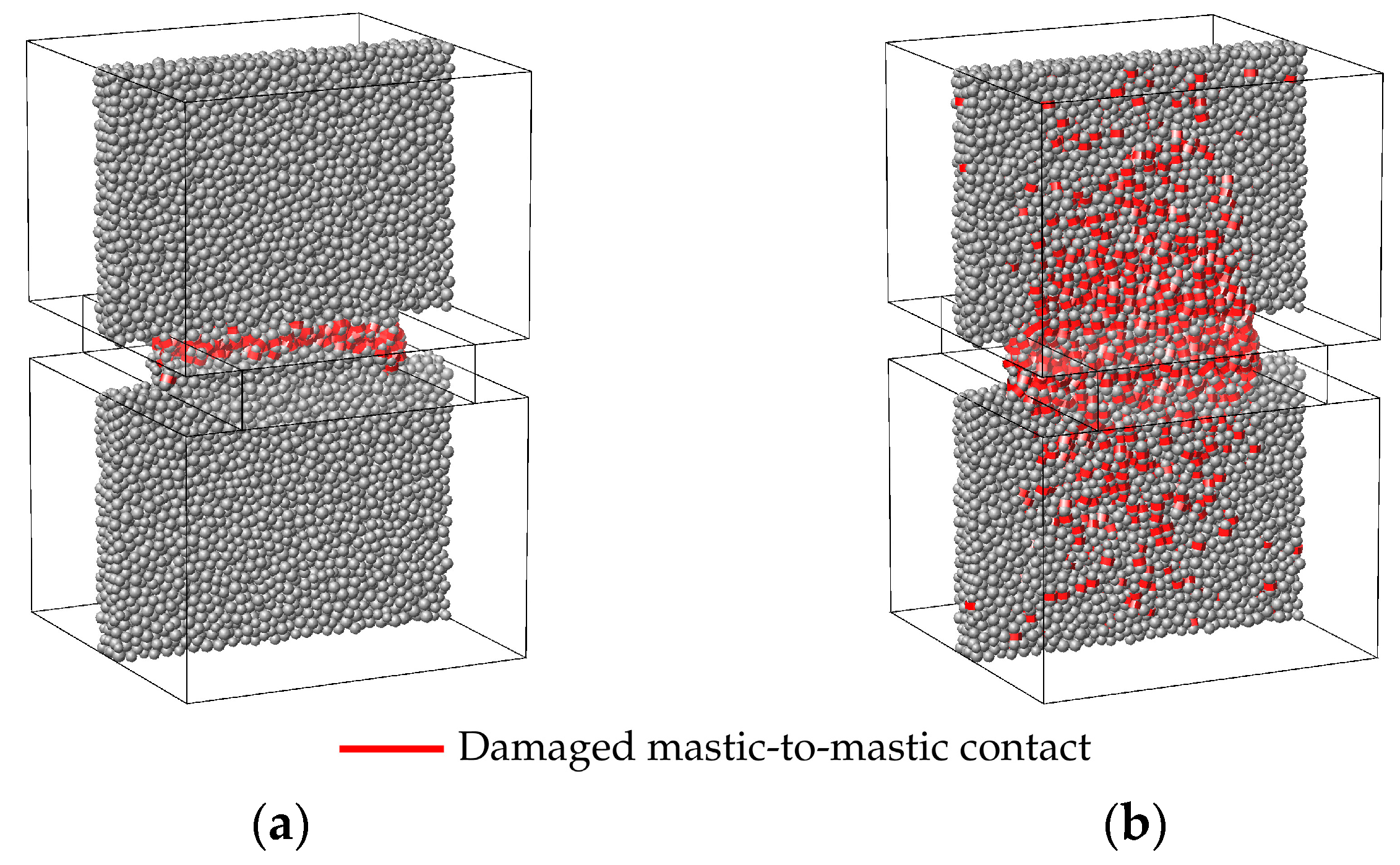
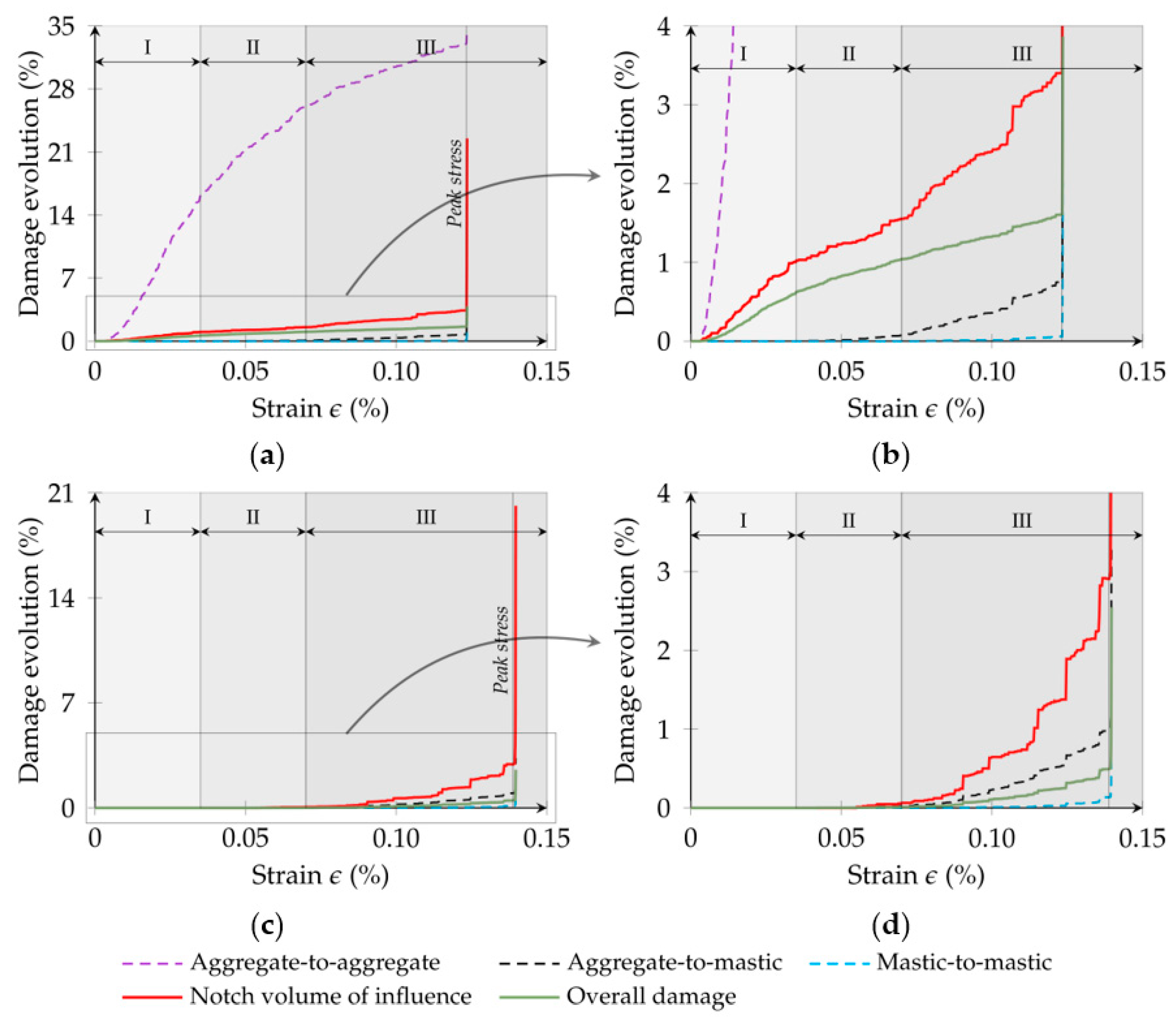
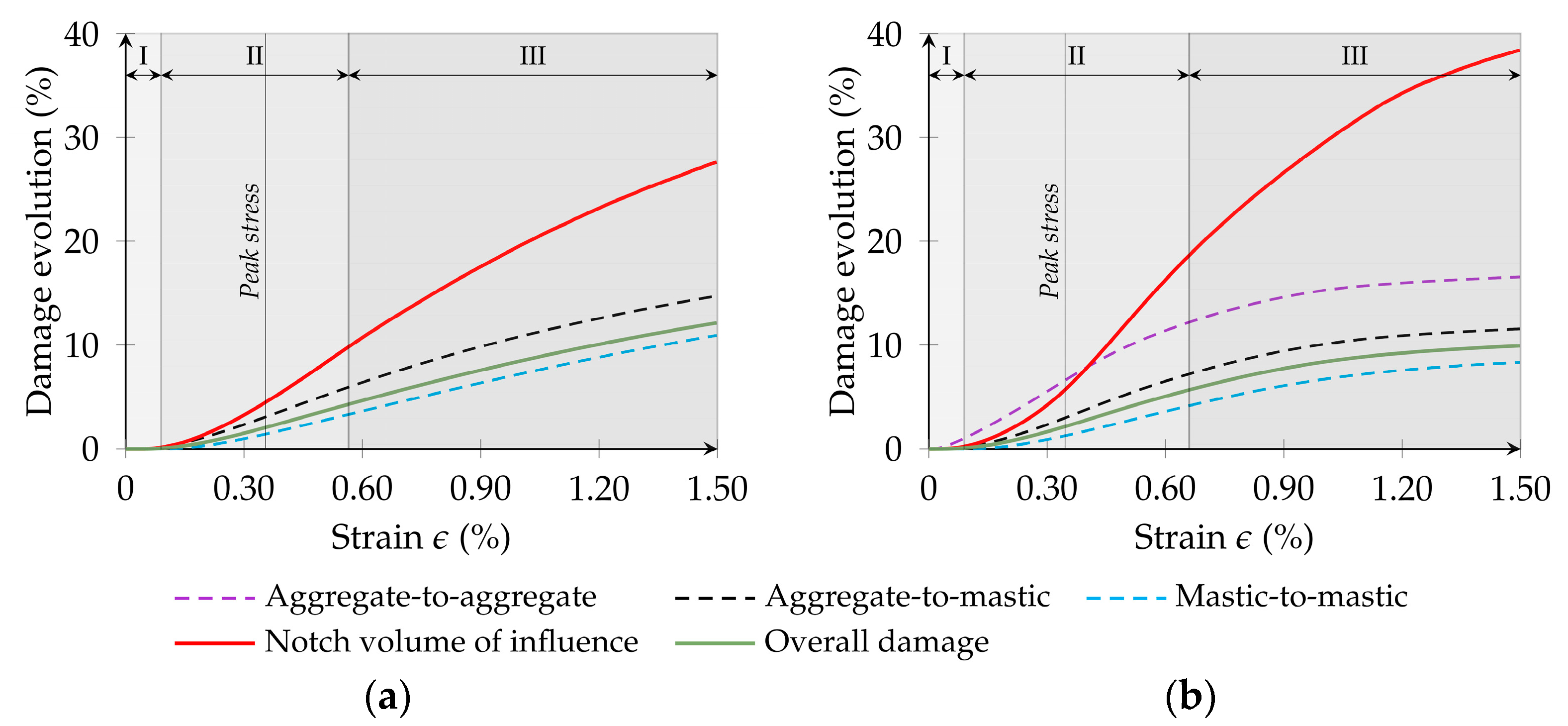
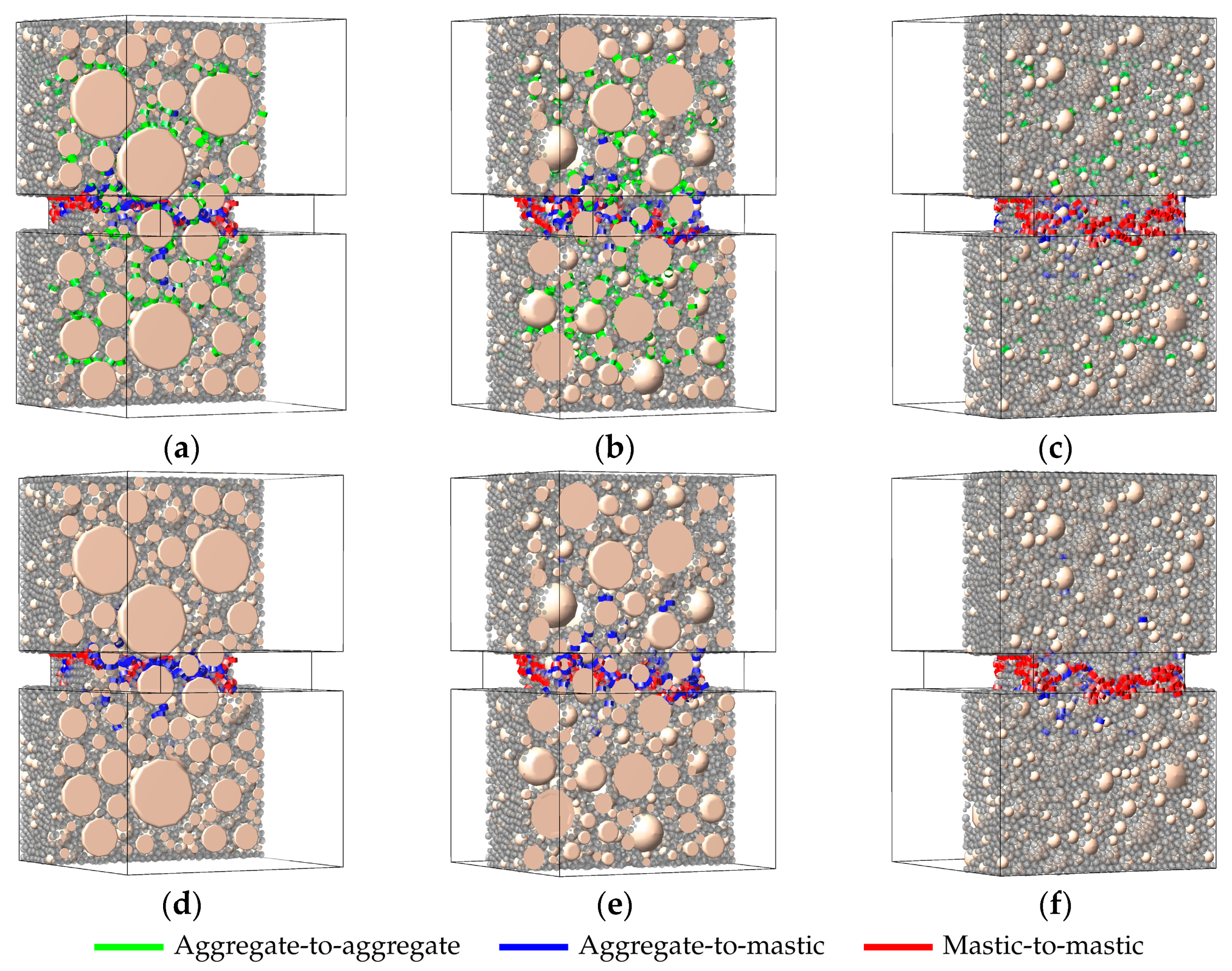
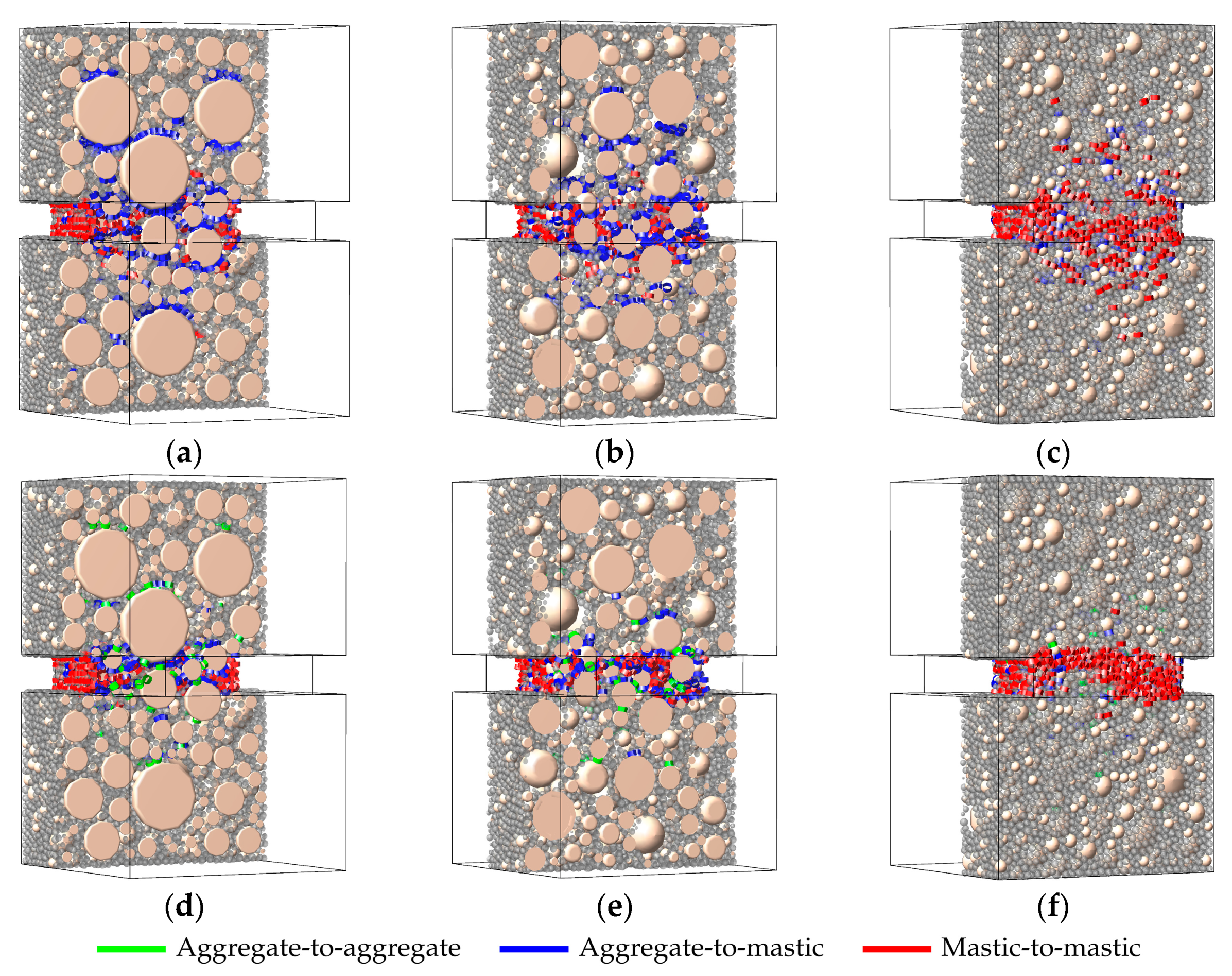
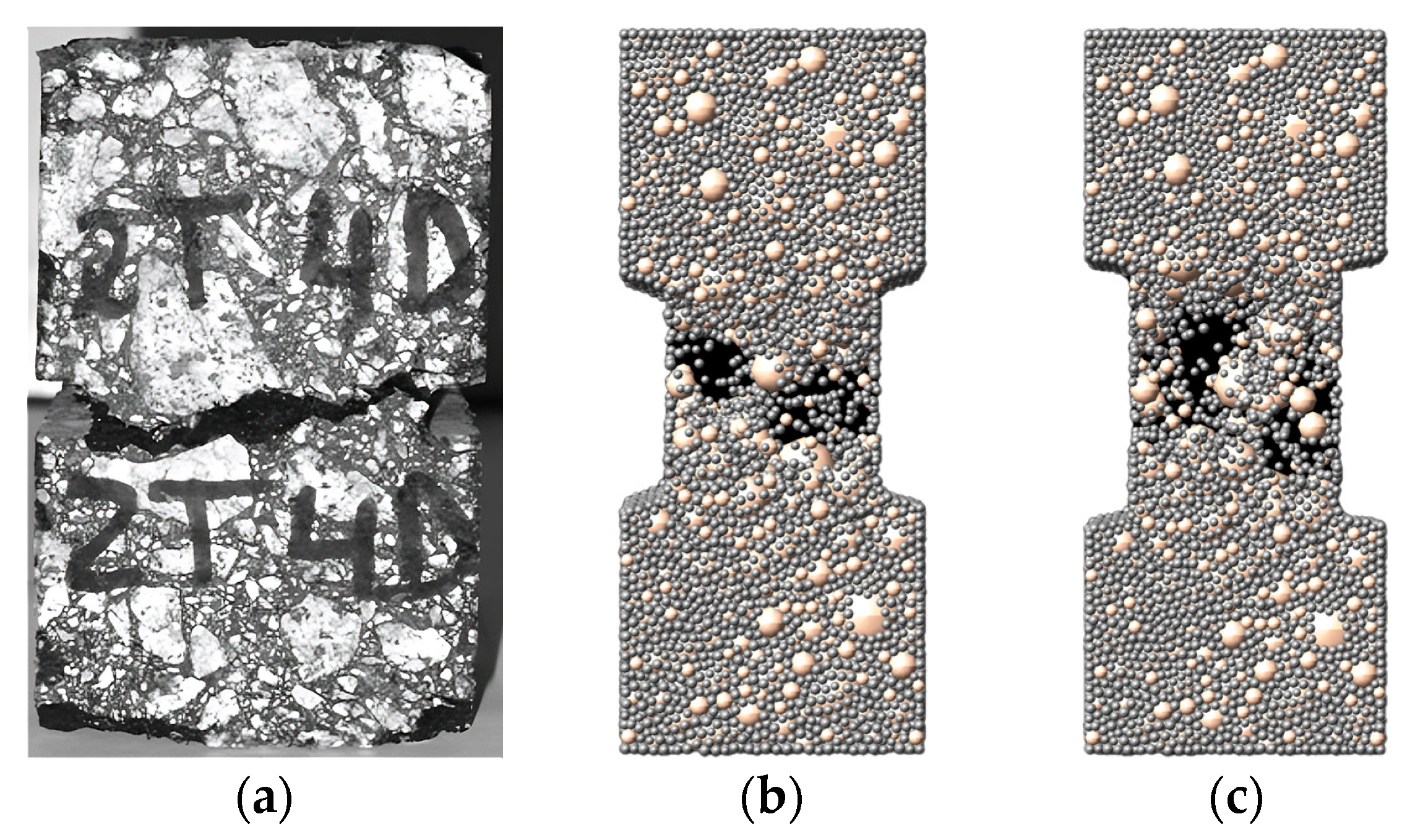
- In asphalt mixtures, aggregate-to-mastic contacts are the primary points of failure. The percentage of damaged contacts at these interactions is more pronounced than that within the mastic phase. Under a tensile loading test of a notched specimen, contact damage between the notch tips is significantly higher than in other parts of the asphalt mixture, leading to the formation of a macro-crack that results in the specimen’s failure.
- -
- The evolution of contact damage can be divided into three different stages. Stage I involves mainly damage evolution located between adjacent aggregates (when allowed) as a result of the rearrangement of these elements in the specimen, predominantly occurring outside the notch tips. In Stage II, damage develops in the viscoelastic damage contacts as stress increases, especially around the notch tips. In Stage III, damage may follow a rapid increase (GK-Brittle) or a stabilisation tendency (GK-Bilinear).
- -
- The proposed GK-Damage models have close computational runtimes for the level of deformation, with an increase of nearly 10% for the GK-Bilinear model due to additional checks performed. Also, by adopting a reduced-size mastic specimen to calibrate the GK-Damage contact parameters, simulation runtimes decreased by eight times. For asphalt mixtures, simulation runtimes varied from 2 days for the GK-Brittle model to 11 days for the GK-Bilinear model.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Gómez-Meijide, B.; Ajam, H.; Garcia, A.; Vansteenkiste, S. Effect of Bitumen Properties in the Induction Healing Capacity of Asphalt Mixes. Constr. Build. Mater. 2018, 190, 131–139. [Google Scholar] [CrossRef]
- Norambuena-Contreras, J.; Yalcin, E.; Garcia, A.; Al-Mansoori, T.; Yilmaz, M.; Hudson-Griffiths, R. Effect of Mixing and Ageing on the Mechanical and Self-Healing Properties of Asphalt Mixtures Containing Polymeric Capsules. Constr. Build. Mater. 2018, 175, 254–266. [Google Scholar] [CrossRef]
- Guerrieri, M.; Parla, G.; Khanmohamadi, M.; Neduzha, L. Asphalt Pavement Damage Detection through Deep Learning Technique and Cost-Effective Equipment: A Case Study in Urban Roads Crossed by Tramway Lines. Infrastructures 2024, 9, 34. [Google Scholar] [CrossRef]
- Su, J.-F.; Qiu, J.; Schlangen, E.; Wang, Y.-Y. Experimental Investigation of Self-Healing Behavior of Bitumen/Microcapsule Composites by a Modified Beam on Elastic Foundation Method. Mater. Struct. 2015, 48, 4067–4076. [Google Scholar] [CrossRef]
- Raffaniello, A.; Bauer, M.; Safiuddin, M.; El-Hakim, M. Traffic and Climate Impacts on Rutting and Thermal Cracking in Flexible and Composite Pavements. Infrastructures 2022, 7, 100. [Google Scholar] [CrossRef]
- Micaelo, R.; Freire, A.C.; Pereira, G. Asphalt Self-Healing with Encapsulated Rejuvenators: Effect of Calcium-Alginate Capsules on Stiffness, Fatigue and Rutting Properties. Mater. Struct. 2020, 53, 20. [Google Scholar] [CrossRef]
- Gao, L.; Li, H.; Xie, J.; Yang, X. Mixed-Mode Fracture Modeling of Cold Recycled Mixture Using Discrete Element Method. Constr. Build. Mater. 2017, 151, 625–635. [Google Scholar] [CrossRef]
- Peng, Y.; Bao, J. Micromechanical Analysis of Asphalt-Mixture Shear Strength Using the Three-Dimensional Discrete Element Method. J. Mater. Civ. Eng. 2018, 30, 04018302. [Google Scholar] [CrossRef]
- Li, J.; Zhang, J.; Qian, G.; Zheng, J.; Zhang, Y. Three-Dimensional Simulation of Aggregate and Asphalt Mixture Using Parameterized Shape and Size Gradation. J. Mater. Civ. Eng. 2019, 31, 04019004. [Google Scholar] [CrossRef]
- Xue, B.; Pei, J.; Zhou, B.; Zhang, J.; Li, R.; Guo, F. Using Random Heterogeneous DEM Model to Simulate the SCB Fracture Behavior of Asphalt Concrete. Constr. Build. Mater. 2020, 236, 117580. [Google Scholar] [CrossRef]
- Wu, J.; Li, D.; Zhu, B.; Wu, C. Milling Process Simulation of Old Asphalt Mixture by Discrete Element. Constr. Build. Mater. 2018, 186, 996–1004. [Google Scholar] [CrossRef]
- Zhu, X.; Yu, H.; Qian, G.; Yao, D.; Dai, W.; Zhang, H.; Li, J.; Zhong, H. Evaluation of Asphalt Mixture Micromechanical Behavior Evolution in the Failure Process Based on Discrete Element Method. Case Stud. Constr. Mater. 2023, 18, e01773. [Google Scholar] [CrossRef]
- Peng, Y.; Sun, L. Aggregate Distribution Influence on the Indirect Tensile Test of Asphalt Mixtures Using the Discrete Element Method. Int. J. Pavement Eng. 2017, 18, 668–681. [Google Scholar] [CrossRef]
- Kim, H.; Wagoner, M.P.; Buttlar, W.G. Micromechanical Fracture Modeling of Asphalt Concrete Using a Single-Edge Notched Beam Test. Mater. Struct. 2009, 42, 677–689. [Google Scholar] [CrossRef]
- Hill, B.C.; Giraldo-Londoño, O.; Paulino, G.H.; Buttlar, W.G. Inverse Estimation of Cohesive Fracture Properties of Asphalt Mixtures Using an Optimization Approach. Exp. Mech. 2017, 57, 637–648. [Google Scholar] [CrossRef]
- Meza-Lopez, J.; Noreña, N.; Meza, C.; Romanel, C. Modeling of Asphalt Concrete Fracture Tests with the Discrete-Element Method. J. Mater. Civ. Eng. 2020, 32, 04020228. [Google Scholar] [CrossRef]
- Liang, H.; Shi, L.; Wang, D.; Xiao, X.; Deng, K. Influence of Graded Coarse Aggregate Content and Specific Surface Area on the Fracture Properties of Asphalt Mixtures Based on Discrete Element Simulations and Indoor Tests. Constr. Build. Mater. 2021, 299, 123942. [Google Scholar] [CrossRef]
- Peng, Y.; Gao, H. Comparative Modelling of Indirect Tensile Strength of Asphalt Mixtures with and without Considering Air Void Characteristics. Int. J. Pavement Eng. 2021, 22, 1601–1610. [Google Scholar] [CrossRef]
- Sheng, Y.; Jia, H.; Lv, H.; Chen, H.; Zhao, X.; Wang, R.; Meng, J. Study on Mesoscopic Mechanics of Recycled Asphalt Mixture in the Indirect Tensile Test. Math. Probl. Eng. 2020, 2020, 6621275. [Google Scholar] [CrossRef]
- Nian, T.; Ge, J.; Li, P.; Wang, M.; Mao, Y. Improved Discrete Element Numerical Simulation and Experiment on Low-Temperature Anti-Cracking Performance of Asphalt Mixture Based on PFC2D. Constr. Build. Mater. 2021, 283, 122792. [Google Scholar] [CrossRef]
- Xie, S.; Yi, J.; Wang, H.; Yang, S.-H.; Xu, M.; Feng, D. Mechanical Response Analysis of Transverse Crack Treatment of Asphalt Pavement Based on DEM. Int. J. Pavement Eng. 2022, 23, 2206–2226. [Google Scholar] [CrossRef]
- Wang, H.; Buttlar, W.G. Three-Dimensional Micromechanical Pavement Model Development for the Study of Block Cracking. Constr. Build. Mater. 2019, 206, 35–45. [Google Scholar] [CrossRef]
- Wang, H.; Behnia, B.; Buttlar, W.G.; Reis, H. Development of Two-Dimensional Micromechanical, Viscoelastic, and Heterogeneous-Based Models for the Study of Block Cracking in Asphalt Pavements. Constr. Build. Mater. 2020, 244, 118146. [Google Scholar] [CrossRef]
- Zhou, M.; Cao, W. Mesoscopic Analysis of Fatigue Damage Development in Asphalt Mixture Based on Modified Burgers Contact Algorithm in Discrete Element Modeling. Materials 2024, 17, 2025. [Google Scholar] [CrossRef] [PubMed]
- Dai, Q.; You, Z. Prediction of Creep Stiffness of Asphalt Mixture with Micromechanical Finite-Element and Discrete-Element Models. J. Eng. Mech. 2007, 133, 163–173. [Google Scholar] [CrossRef]
- Câmara, G.; Azevedo, N.M.; Micaelo, R.; Silva, H. Generalised Kelvin Contact Models for DEM Modelling of Asphalt Mixtures. Int. J. Pavement Eng. 2023, 24, 2179625. [Google Scholar] [CrossRef]
- Ren, J.; Sun, L. Characterizing Air Void Effect on Fracture of Asphalt Concrete at Low-Temperature Using Discrete Element Method. Eng. Fract. Mech. 2017, 170, 23–43. [Google Scholar] [CrossRef]
- Sun, L.; Ren, J.; Zhang, S. Fracture Characteristics of Asphalt Concrete in Mixed-Loading Mode at Low-Temperature Based on Discrete-Element Method. J. Mater. Civ. Eng. 2018, 30, 04018321. [Google Scholar] [CrossRef]
- Xu, Y.; Jiang, Y.; Xue, J.; Ren, J. Investigating the Effect of Aggregate Characteristics on the Macroscopic and Microscopic Fracture Mechanisms of Asphalt Concrete at Low-Temperature. Materials 2019, 12, 2675. [Google Scholar] [CrossRef]
- Lu, D.X.; Nguyen, N.H.T.; Bui, H.H. A Cohesive Viscoelastic-Elastoplastic-Damage Model for DEM and Its Applications to Predict the Rate- and Time-Dependent Behaviour of Asphalt Concretes. Int. J. Plast. 2022, 157, 103391. [Google Scholar] [CrossRef]
- Caballero, A.; Willam, K.J.; Carol, I. Consistent Tangent Formulation for 3D Interface Modeling of Cracking/Fracture in Quasi-Brittle Materials. Comput. Methods Appl. Mech. Eng. 2008, 197, 2804–2822. [Google Scholar] [CrossRef]
- Lu, D.X.; Bui, H.H.; Saleh, M. Predicting the Rutting Behaviour of Asphalt Concrete in the Modified Wheel Tracking Test Using DEM and a Cohesive Viscoelastic–Elastoplastic-Damage Contact Model. Comput. Part. Mech. 2024. [Google Scholar] [CrossRef]
- Azevedo, N.M.; Farinha, M.L.B.; Oliveira, S. Assessment of Contact Laws Accounting for Softening in 3D Rigid Concrete Particle Models. Buildings 2024, 14, 801. [Google Scholar] [CrossRef]
- Câmara, G.; Micaelo, R.; Monteiro Azevedo, N. 3D DEM Model Simulation of Asphalt Mastics with Sunflower Oil. Comput. Part. Mech. 2023, 10, 1569–1586. [Google Scholar] [CrossRef]
- Dan, H.-C.; Zhang, Z.; Chen, J.; Cao, W. Low-Temperature Fracture Characteristics of Asphalt Mixtures Using the Eccentric Single-Edge Notched Bend Test: A 3D Discrete Element Study. Constr. Build. Mater. 2022, 344, 128182. [Google Scholar] [CrossRef]
- Jin, D.; Boateng, K.A.; Chen, S.; Xin, K.; You, Z. Comparison of Rubber Asphalt with Polymer Asphalt under Long-Term Aging Conditions in Michigan. Sustainability 2022, 14, 10987. [Google Scholar] [CrossRef]
- Jin, D.; Yin, L.; Malburg, L.; You, Z. Laboratory Evaluation and Field Demonstration of Cold In-Place Recycling Asphalt Mixture in Michigan Low-Volume Road. Case Stud. Constr. Mater. 2024, 20, e02923. [Google Scholar] [CrossRef]
- Kim, H.; Wagoner, M.P.; Buttlar, W.G. Simulation of Fracture Behavior in Asphalt Concrete Using a Heterogeneous Cohesive Zone Discrete Element Model. J. Mater. Civ. Eng. 2008, 20, 552–563. [Google Scholar] [CrossRef]
- Monteiro Azevedo, N.; Lemos, J.V.; Almeida, J. A Discrete Particle Model for Reinforced Concrete Fracture Analysis. Struct. Eng. Mech. 2010, 36, 343–361. [Google Scholar] [CrossRef]
- Kim, H.; Buttlar, W.G. Discrete Fracture Modeling of Asphalt Concrete. Int. J. Solids Struct. 2009, 46, 2593–2604. [Google Scholar] [CrossRef]
- Dan, H.-C.; Zhang, Z.; Chen, J.-Q.; Wang, H. Numerical Simulation of an Indirect Tensile Test for Asphalt Mixtures Using Discrete Element Method Software. J. Mater. Civ. Eng. 2018, 30, 04018067. [Google Scholar] [CrossRef]
- Silva, H. Caracterização do Mastique Betuminoso e da Ligação Agregado-Mastique. Ph.D. Thesis, Universidade do Minho, Guimarães, Portugal, 2005. [Google Scholar]
- Câmara, G.; Azevedo, N.M.; Micaelo, R. Impact of Rejuvenator-Modified Mastic on Asphalt Mixture Stiffness: Meso-Scale Discrete Element Method Approach. Buildings 2023, 13, 3023. [Google Scholar] [CrossRef]
- Micaelo, R.; Monteiro Azevedo, N.; Câmara, G. Improving Asphalt Discrete Numerical Modelling with Realistic Particle Shapes. In Proceedings of the VIII International Conference on Particle-Based Methods PARTICLES 2023, Milan, Italy, 9–11 October 2023. [Google Scholar]
- Li, Y.; Jiang, W.; Xiao, J.; Zhao, F.; Zhang, S.; Xing, C.; Yuan, D. Effects of Kneading and Impact Action on the Movement of Aggregates in Asphalt Mixtures during Compaction. Constr. Build. Mater. 2023, 366, 130210. [Google Scholar] [CrossRef]
- Nian, T.; Ge, J.; Li, P.; Guo, R.; Li, J.; Wang, M. Improved Three-Dimensional Discrete Modeling Method and Anti-Cracking Properties of Asphalt Mixture. Constr. Build. Mater. 2022, 321, 126405. [Google Scholar] [CrossRef]
- Michot-Roberto, S. 3D-Simulation of Packing of Aggregates with Realistic Morphology and Size Distribution by Using a Real-Time Physic’s Engine. Ph.D. Thesis, University of Nottingham, Nottingham, UK, 2022. [Google Scholar]
- Ling, M.; Zhang, Y.; Kaseer, F.; Martin, A.E.; Lytton, R.L. Investigation of Fracture Behavior of Asphalt Mixture Composite Using Energy-Based Approach. Compos. Part B Eng. 2020, 181, 107324. [Google Scholar] [CrossRef]
- Peng, Y.; Harvey, J.T.; Sun, L. Three-Dimensional Discrete-Element Modeling of Aggregate Homogeneity Influence on Indirect Tensile Strength of Asphalt Mixtures. J. Mater. Civ. Eng. 2017, 29, 04017211. [Google Scholar] [CrossRef]
- Peng, Y.; Wan, L.; Sun, L.-J. Three-Dimensional Discrete Element Modelling of Influence Factors of Indirect Tensile Strength of Asphalt Mixtures. Int. J. Pavement Eng. 2019, 20, 724–733. [Google Scholar] [CrossRef]
- Kim, H.; Buttlar, W.G. Multi-Scale Fracture Modeling of Asphalt Composite Structures. Compos. Sci. Technol. 2009, 69, 2716–2723. [Google Scholar] [CrossRef]
- Gao, H.; Yang, X.; Zhang, C. Experimental and Numerical Analysis of Three-Point Bending Fracture of Pre-Notched Asphalt Mixture Beam. Constr. Build. Mater. 2015, 90, 1–10. [Google Scholar] [CrossRef]







| Parameters | Values |
|---|---|
| (kPa) | |
| (kPa·s) | |
| (kPa) | |
| (kPa·s) |
| Contact Model | Maximum Contact Tensile Strength (MPa)— | Contact Fracture Energy in Mode I (N/mm)— |
|---|---|---|
| GK-Brittle | 2.0 | - |
| GK-Bilinear | 0.067 |
| Case Study | Loading Velocity (m/s) | Maximum Contact Tensile Strength (MPa)— |
|---|---|---|
| 2.0 m/s 2.0 MPa (reference) | 2.0 | |
| .0 m/s 3.0 MPa | 3.0 | |
| 8.0 m/s 4.0 MPa | 4.0 |
| Case Study | Maximum Contact Tensile Strength (MPa)— | Contact Fracture Energy in Mode I (N/mm)— |
|---|---|---|
| 1.0 MPa 0.033 N/mm | 1.0 | 0.033 |
| 2.0 MPa 0.067 N/mm | 2.0 | 0.067 |
| 3.0 MPa 0.100 N/mm | 3.0 | 0.100 |
| Sieve Size (mm) | Aggregates | Mastic | ||||
|---|---|---|---|---|---|---|
| 19.0 | 12.5 | 9.5 | 4.75 | 2.0 | <2.0 | |
| Number of particles | - | 13 | 28 | 298 | 2315 | 24,096 |
| Volume (mm3) | - | 27,432.4 | 21,033.3 | 39,397.5 | 25,786.4 | 61,305.9 |
| Contact Type | Number of Interactions |
|---|---|
| Aggregate-to-aggregate | 5414 |
| Aggregate-to-mastic | 57,723 |
| Mastic-to-mastic | 86,813 |
| Aggregate-to-wall | 97 |
| Mastic-to-wall | 1794 |
| Case Study | Contact Type | Contact Model |
|---|---|---|
| Case 1 | Aggregate-to-aggregate | BRM |
| Aggregate-to-mastic | GK-Brittle | |
| Mastic-to-mastic | GK-Brittle | |
| Aggregate-to-aggregate | BRM-NT | |
| Case 2 | Aggregate-to-mastic | GK-Brittle |
| Mastic-to-mastic | GK-Brittle | |
| Aggregate-to-aggregate | BRM-NT | |
| Case 3 | Aggregate-to-mastic | GK-Bilinear |
| Mastic-to-mastic | GK-Bilinear | |
| Aggregate-to-aggregate | BSM | |
| Case 4 | Aggregate-to-mastic | GK-Bilinear |
| Mastic-to-mastic | GK-Bilinear |
| Parameters | Values |
|---|---|
| (kPa) | |
| (kPa∙s) | |
| (kPa) | |
| (kPa∙s) |
| Contact Model | GK-Brittle | GK-Bilinear |
|---|---|---|
| Maximum contact tensile stress (MPa) | 6.12 | |
| Maximum contact cohesion stress (MPa) | 24.48 | |
| Contact fracture energy in mode I (N/mm) | - | 0.35 |
| Contact fracture energy in mode II (N/mm) | - | 56.44 |
| Case Study | Aggregate-to-Aggregate Contact Model | Number of Damaged Contacts: Notch (Total Specimen) | ||
|---|---|---|---|---|
| Aggregate-to-Aggregate | Aggregate-to-Mastic | Mastic-to-Mastic | ||
| 1 | BRM | 454 (1559) | 1422 (1534) | 1291 (1293) |
| 2 | BRM-NT | - | 1386 (1498) | 1234 (1234) |
| 3 | BRM-NT | - | 1890 (3161) | 1721 (2037) |
| 4 | BSM | 265 (390) | 1933 (2169) | 2297 (2317) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Câmara, G.; Micaelo, R.; Azevedo, N.M.; Silva, H. Incremental Viscoelastic Damage Contact Models for Asphalt Mixture Fracture Assessment. Infrastructures 2024, 9, 118. https://doi.org/10.3390/infrastructures9070118
Câmara G, Micaelo R, Azevedo NM, Silva H. Incremental Viscoelastic Damage Contact Models for Asphalt Mixture Fracture Assessment. Infrastructures. 2024; 9(7):118. https://doi.org/10.3390/infrastructures9070118
Chicago/Turabian StyleCâmara, Gustavo, Rui Micaelo, Nuno Monteiro Azevedo, and Hugo Silva. 2024. "Incremental Viscoelastic Damage Contact Models for Asphalt Mixture Fracture Assessment" Infrastructures 9, no. 7: 118. https://doi.org/10.3390/infrastructures9070118
APA StyleCâmara, G., Micaelo, R., Azevedo, N. M., & Silva, H. (2024). Incremental Viscoelastic Damage Contact Models for Asphalt Mixture Fracture Assessment. Infrastructures, 9(7), 118. https://doi.org/10.3390/infrastructures9070118









