Phenomenon of Scattering of Zeros of the (p,q)-Cosine Sigmoid Polynomials and (p,q)-Sine Sigmoid Polynomials
Abstract
:1. Introduction
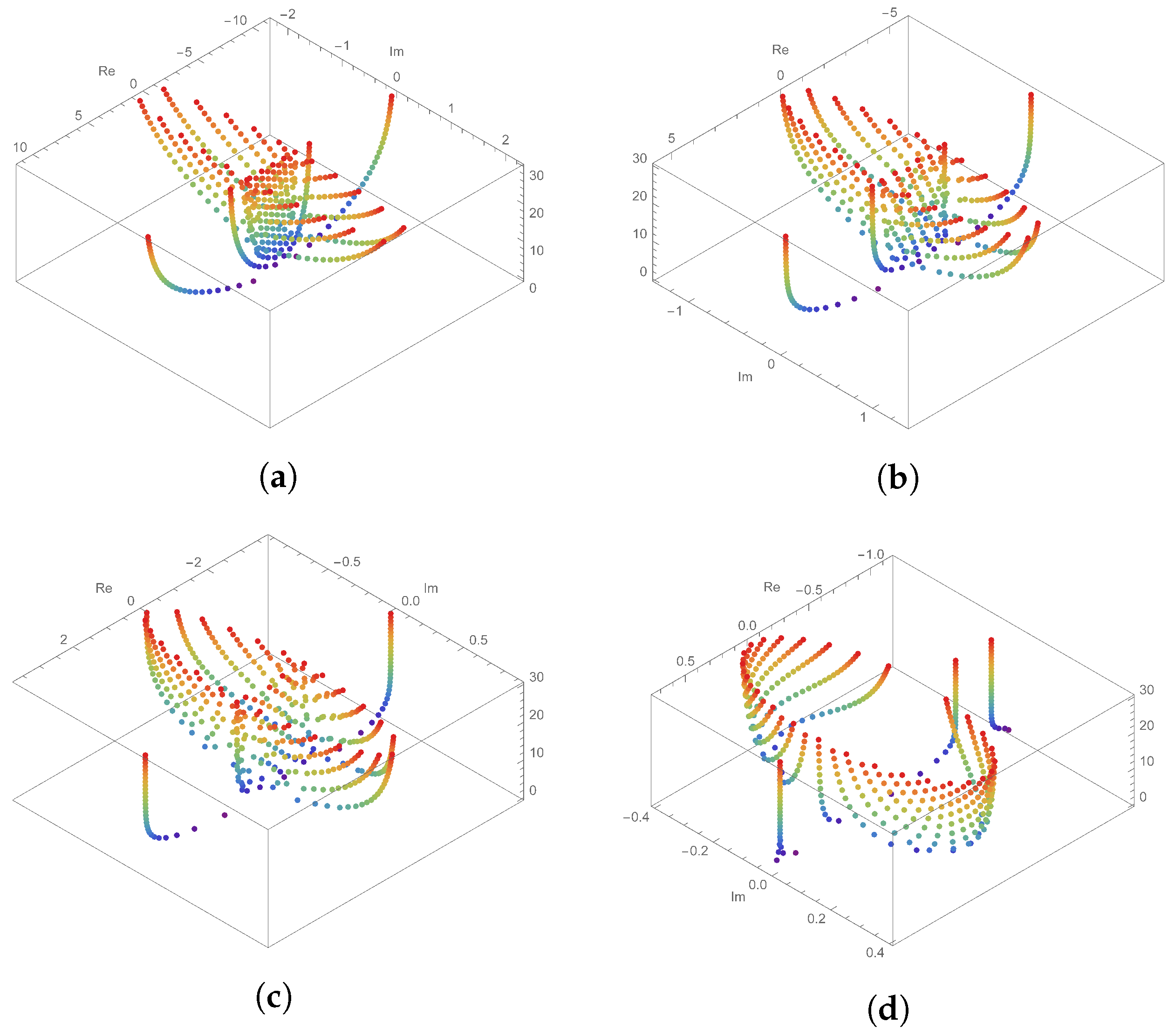
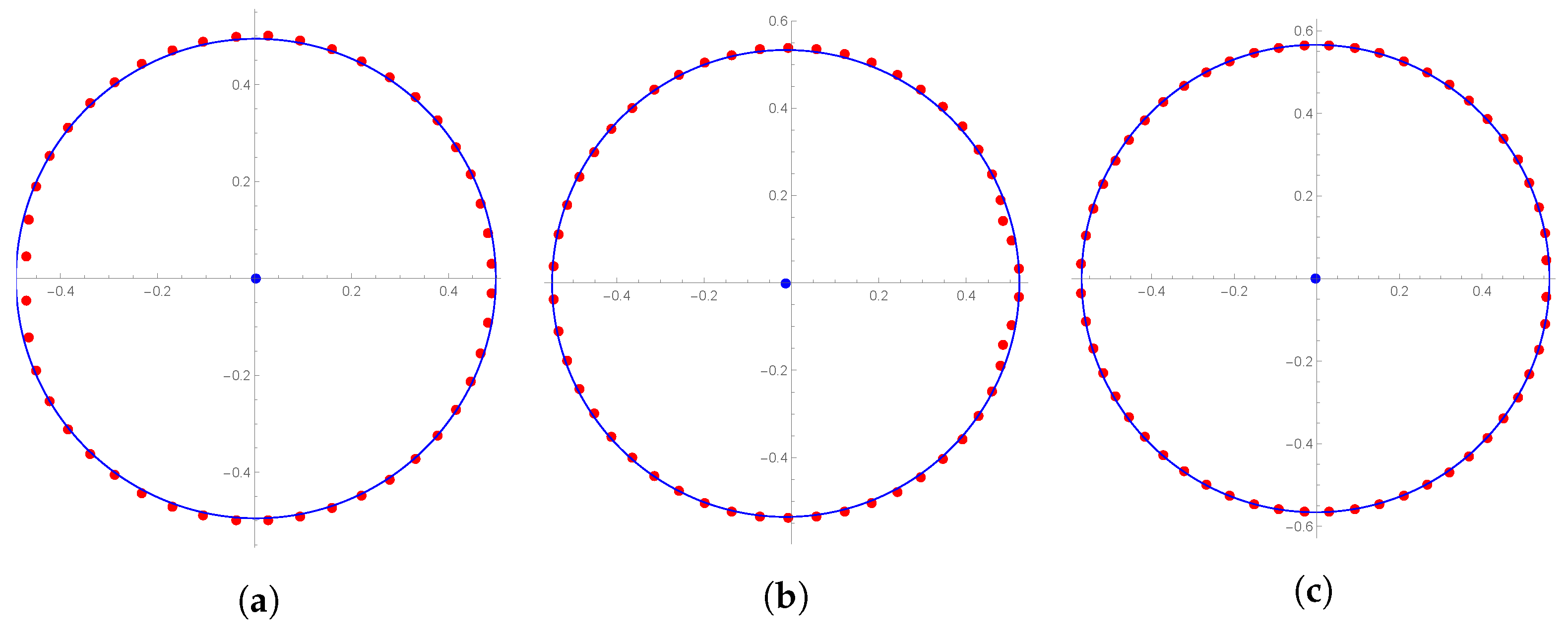
2. Some Properties and Approximate Roots of -Cosine Sigmoid Polynomials
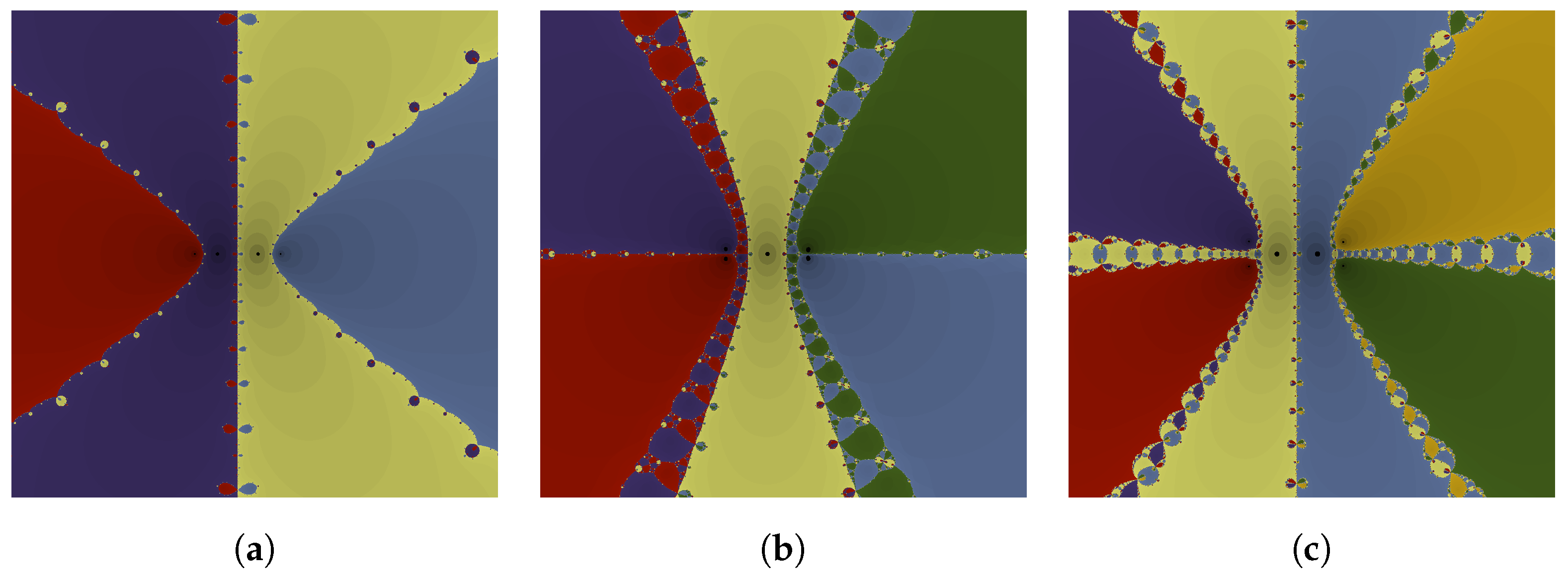
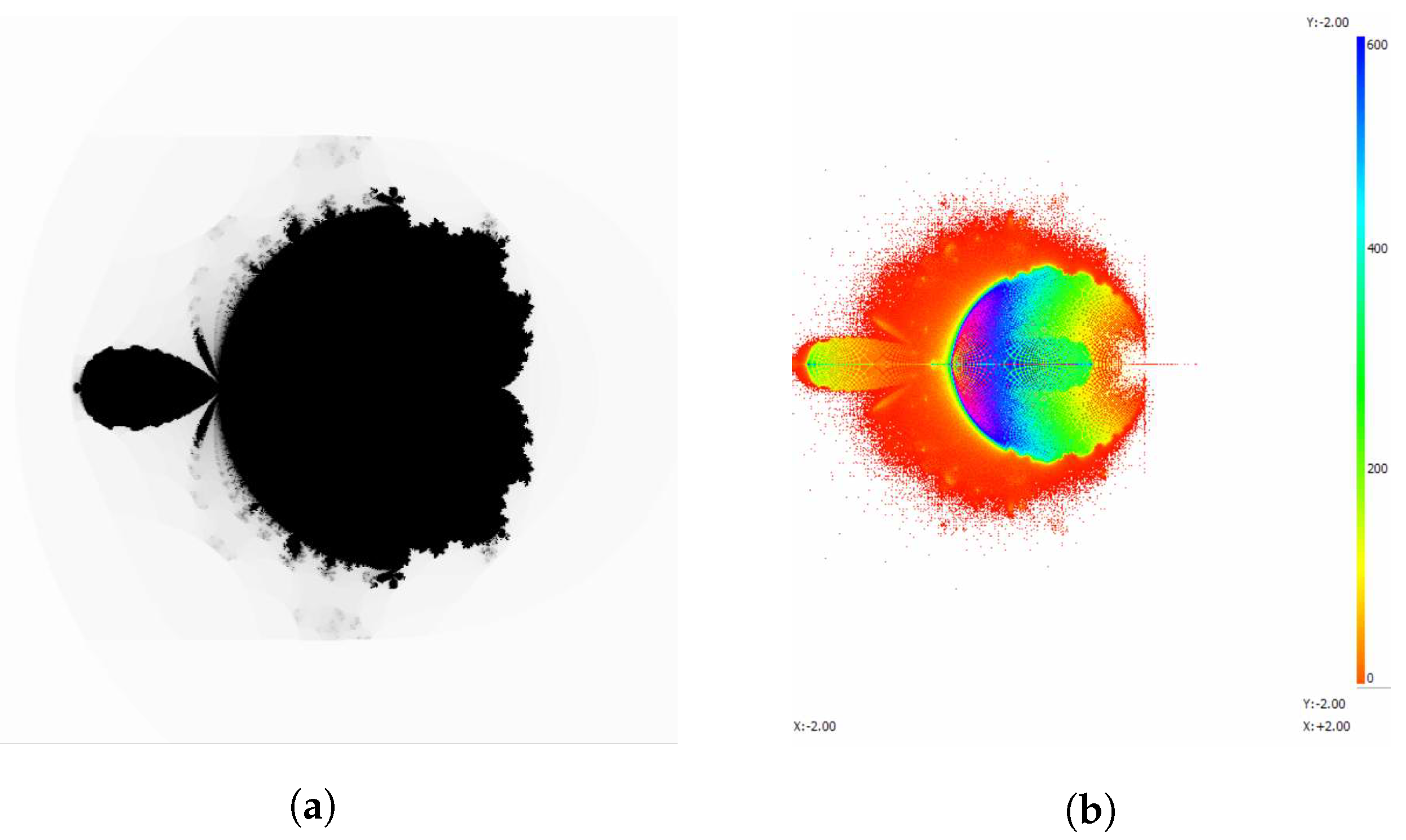
3. Some Fractal Figures and Properties of -Sine Sigmoid Polynomials
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Chakrabarti, R.; Jagannathan, R. A (p,q)-oscillator realization of two-parameter quantum algebras. J. Phys. A Math. Gen. 1991, 24, L711. [Google Scholar] [CrossRef]
- Araci, S.; Duran, U.; Acikgoz, M.; Srivastava, H.M. A certain (p,q)-derivative operator and associated divided differences. J. Comput. Theor. Nanosci. 2016, 301. [Google Scholar] [CrossRef] [Green Version]
- Brodimas, G.; Jannussis, A.; Mignani, R. Two-Parameter Quantum Groups; Preprint; Universita di Roma: Rome, Italy, 1991; Nr. 820. [Google Scholar]
- Burban, M.; Klimyk, A.U. (P,Q)-differentiation, (P,Q)-integration and (P,Q)-hypergeometric functions related to quantum groups. Integral Transform. Spec. Funct. 1994, 2, 15–36. [Google Scholar] [CrossRef]
- Corcino, R.B. On (P,Q)-Binomial coefficients. Electron. J. Comb. Number Theory 2008, 8, A29. [Google Scholar]
- Jagannathan, R.; Rao, K.S. Two-parameter quantum algebras, twin-basic numbers, and associated generalized hypergeometric series. Proceeding of the International Conference on Number Theory and Mathematical Physics, Srinivasa Ramanujan Centre, Kumbakonam, India, 20–21 December 2005. [Google Scholar]
- Jagannathan, R. (P,Q)-Special functions. arXiv 1998, arXiv:math/9803142v1. [Google Scholar]
- Kang, J.Y.; Ryoo, C.S. A numerical Investigation on the structure of the zeros of the q-tangent polynomials. In Polynomials-Theory and Application; IntechOpen: London, UK, 2019; pp. 1–18. [Google Scholar]
- Ryoo, C.S. A numerical investigation on the zeros of the tangent polynomials. J. Appl. Math. Inform. 2014, 32, 315–322. [Google Scholar] [CrossRef]
- Barnsley, M.F. Fractals Everywhere, 3rd ed.; Academic Press: Boston, MA, USA; San Diego, CA, USA; New York, NY, USA, 1988. [Google Scholar]
- Han, J.; Moraga, C. The influence of the sigmoid function parameters on the speed of backpropagation learning. Int. Workshop Artif. Neural Netw. 2005, 930, 195–201. [Google Scholar]
- Han, J.; Wilson, R.S.; Leurgans, S.E. Sigmoidal mixed models for longitudinal data. Stat. Methods Med. Res. 2018, 27, 863–875. [Google Scholar] [CrossRef]
- Kwan, H.K. Simple sigmoid-like activation function suitable for digital hardware implementation. Stat. Methods Med. Res. 1992, 28, 1379–1380. [Google Scholar] [CrossRef]
- Kang, J.Y. Some relationships between sigmoid polynomials and other polynomials. J. Appl. Pure. Math. 2019, 1, 57–67. [Google Scholar]
- Kang, J.Y. Explicit properties of q-sigmoid polynomials combining q-cosine function. J. Appl. Math. Inform. 2021, 39, 541–552. [Google Scholar]
- Rodrigues, P.S.; Lopes, G.W.; Santos, R.M.; Coltri, E.; Giraldi, G.A. A q-extension of sigmoid functions and the application for enhancement of ultrasound images. Entropy 2019, 21, 430. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kang, J.Y. Some Properties and Distribution of the Zeros of the q-Sigmoid Polynomials. Discret. Dyn. Nat. Soc. 2020, 2020, 4169840. [Google Scholar] [CrossRef]
- Kang, J.Y. Determination of the properties of (p,q)-sigmoid polynomials and the structure of their roots. In Number Theory and Its Applications; IntechOpen: London, UK, 2020; pp. 1–19. [Google Scholar]
- Ryoo, C.S.; Kang, J.Y. Structure of approximate roots based on symmetric properties of (p,q)-cosine and (p,q)-sine Bernoulli polynomials. Symmetry 2020, 12, 885. [Google Scholar] [CrossRef]
- Garcia, F.; Fernandez, A.; Barrallo, J.; Martin, L. Coloring Dynamical Systems in the Complex Plane. Retrieved 21 January 2008. Available online: http://math.unipa.it/grim/Jbarrallo.PDF (accessed on 30 August 2021).

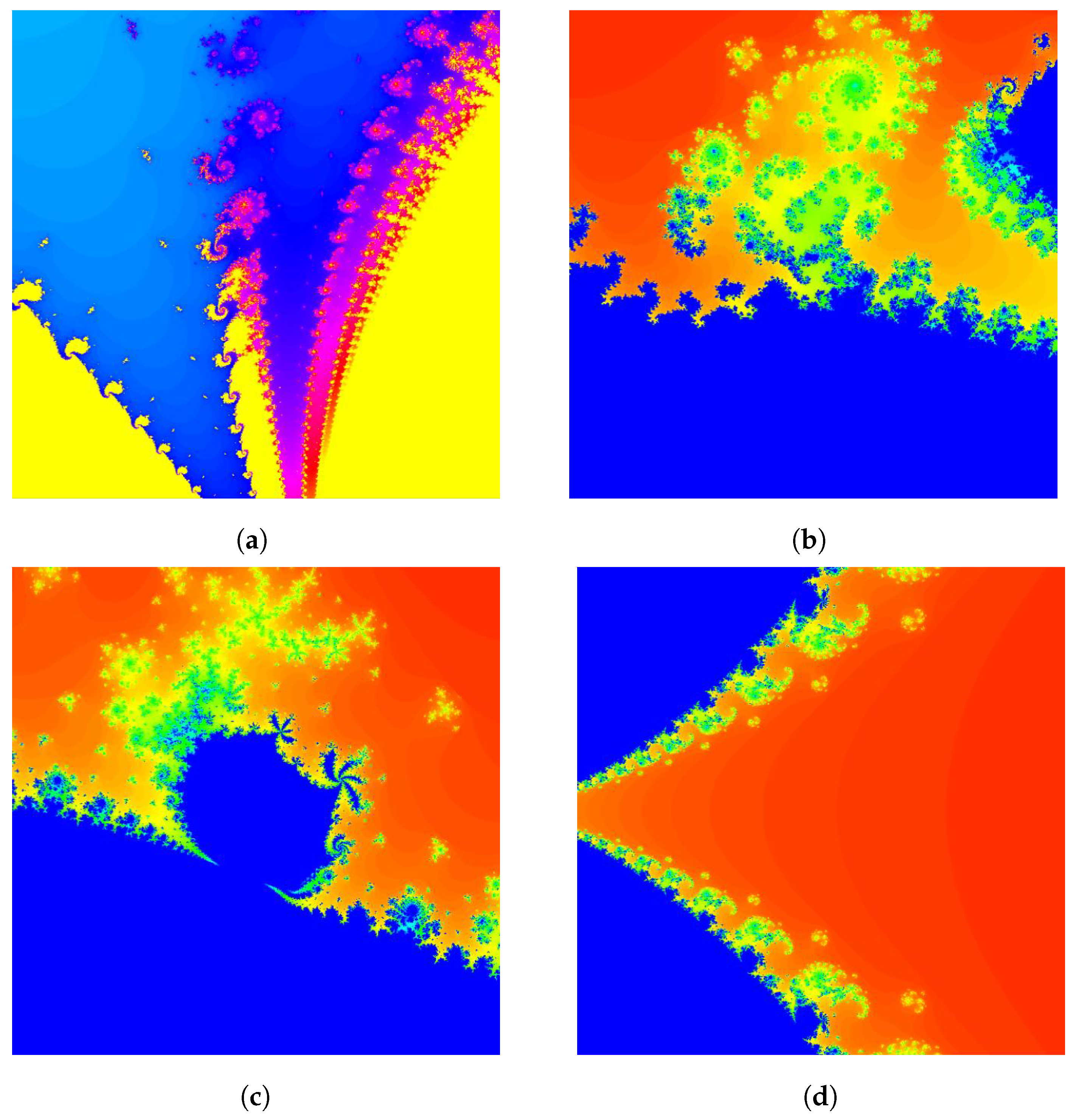
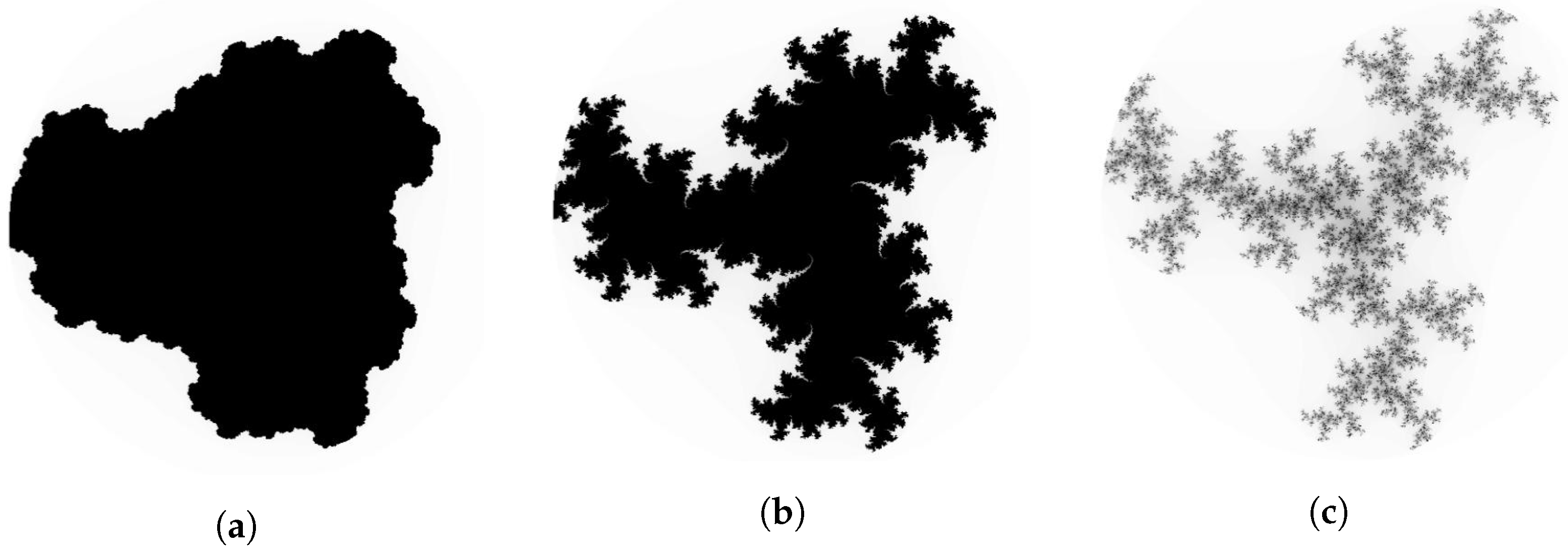
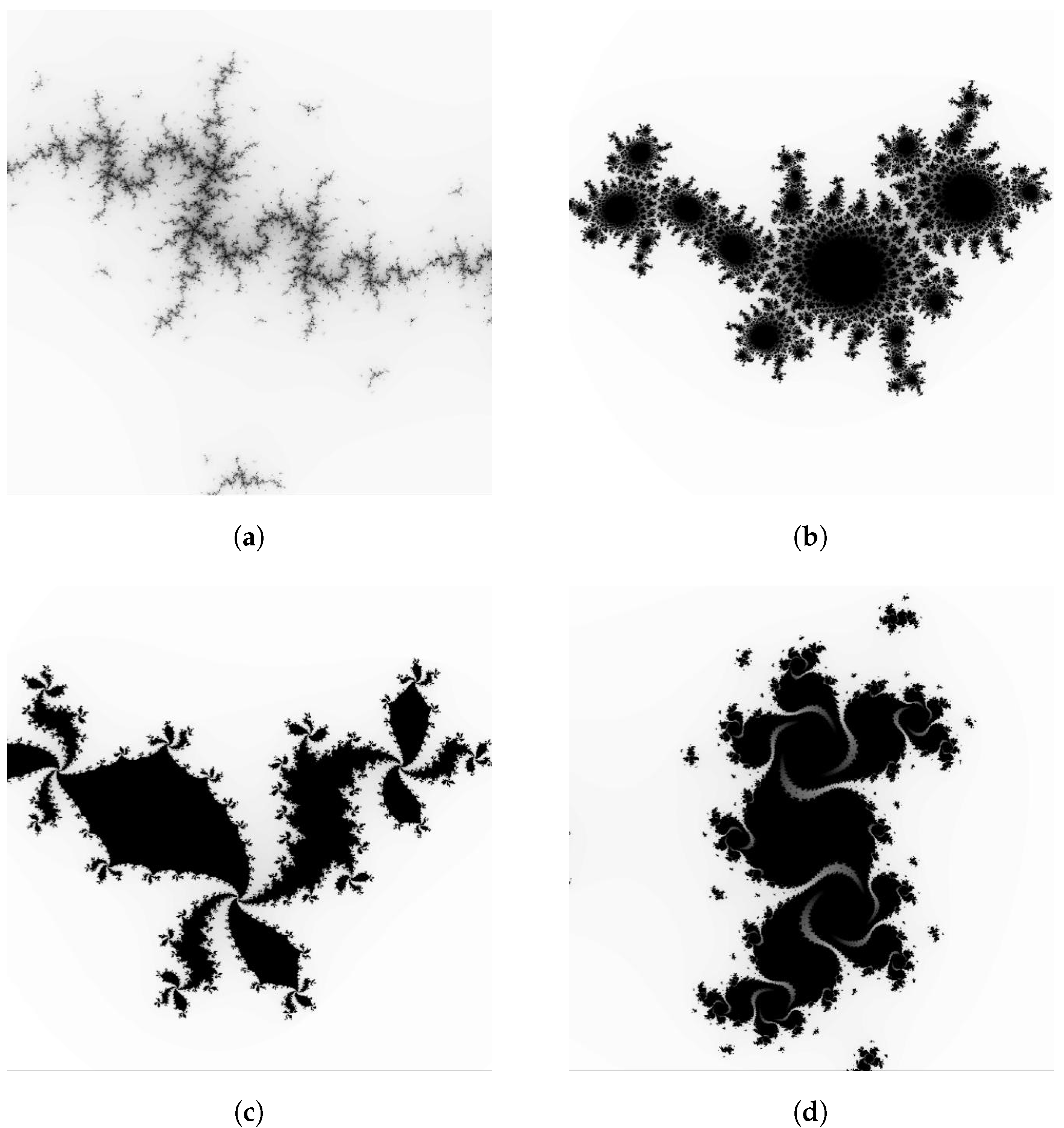
| n | 30 | 40 | 50 | 60 |
|---|---|---|---|---|
| 0.729155 | 0.00704653 | 0.303158 | 0.604624 | |
| 1.82395 | 1.04214 | 1.36616 | 1.70358 | |
| 2.06903 | 2.146 | 3.68302 | 2.38741 | |
| 3.57276 | 3.65711 | 11.383 | 3.691 | |
| 11.0688 | 11.3091 | 11.4058 |
| n | 30 | 40 | 50 | 60 |
|---|---|---|---|---|
| 0.399349 | 0.405326 | 0.401946 | 0.532724 | |
| 0.711465 | 0.711465 | 0.711465 | 0.711465 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ryoo, C.S.; Kang, J.Y. Phenomenon of Scattering of Zeros of the (p,q)-Cosine Sigmoid Polynomials and (p,q)-Sine Sigmoid Polynomials. Fractal Fract. 2021, 5, 245. https://doi.org/10.3390/fractalfract5040245
Ryoo CS, Kang JY. Phenomenon of Scattering of Zeros of the (p,q)-Cosine Sigmoid Polynomials and (p,q)-Sine Sigmoid Polynomials. Fractal and Fractional. 2021; 5(4):245. https://doi.org/10.3390/fractalfract5040245
Chicago/Turabian StyleRyoo, Cheon Seoung, and Jung Yoog Kang. 2021. "Phenomenon of Scattering of Zeros of the (p,q)-Cosine Sigmoid Polynomials and (p,q)-Sine Sigmoid Polynomials" Fractal and Fractional 5, no. 4: 245. https://doi.org/10.3390/fractalfract5040245





