A Stochastic Bayesian Regularization Approach for the Fractional Food Chain Supply System with Allee Effects
Abstract
:1. Introduction
2. Mathematical FFSCS-AE
- The design of the FDs based on the dynamical FFSCS-AE is provided to scrutinize the accurate and real performances.
- The stochastic computing performances have not been executed to solve the dynamical FFSCS-AE.
- The ANNs computing procedures along with the Bayesian regularization scheme have been used to present the dynamical FFSCS-AE using the FDs in 0 and 1.
- The precision of the ANNs computing procedures along with the Bayesian regularization scheme is provided based on the comparative measures of the achieved and reference results.
- The absolute error measures in good performances are reported, which show the exactness and capability of the ANNs computing procedures along with the Bayesian regularization scheme.
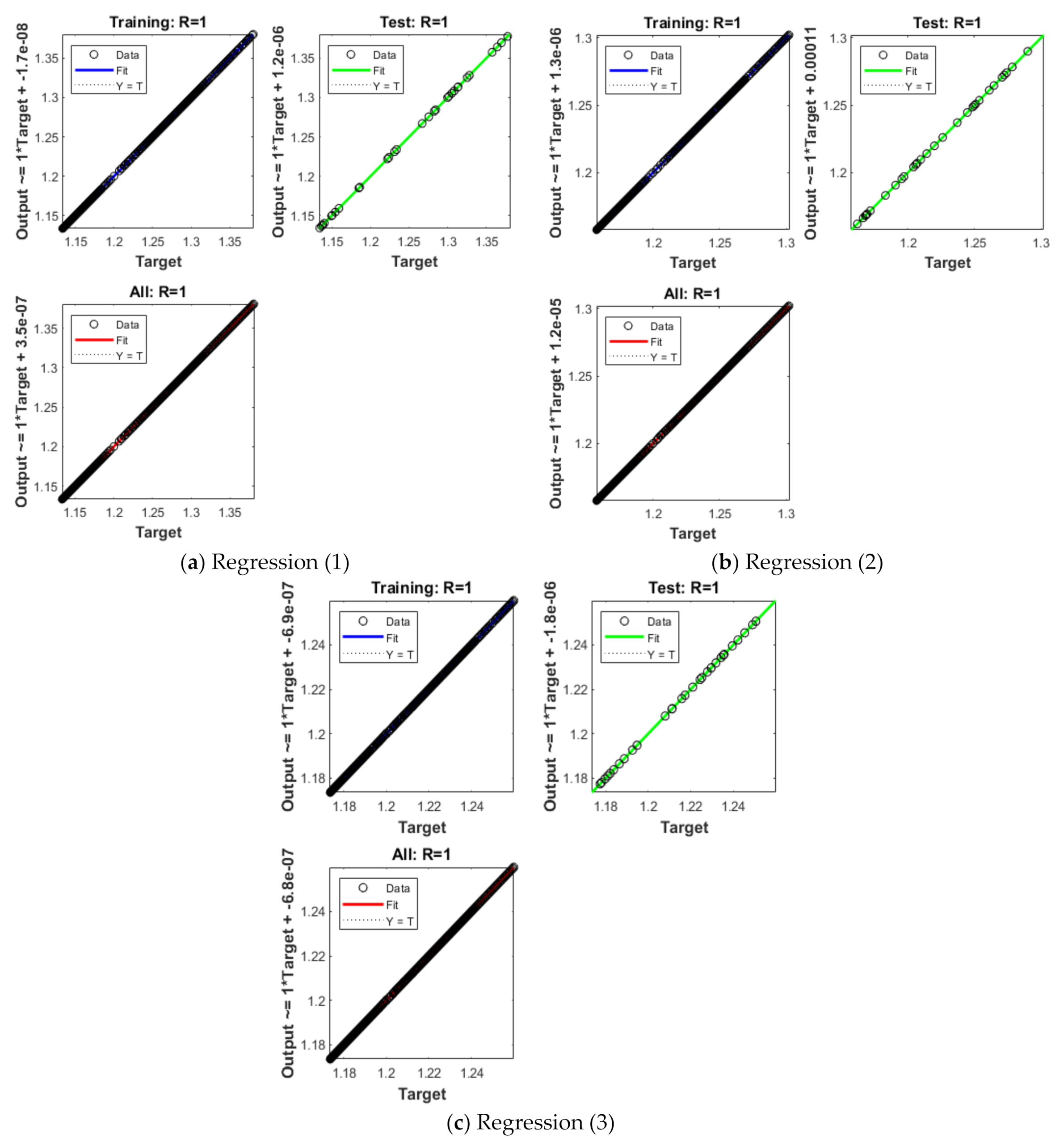
- The regression measures, correlation values, error histograms (EHs), and state transition (STs) performances have been provided using the dynamical FFSCS-AE.
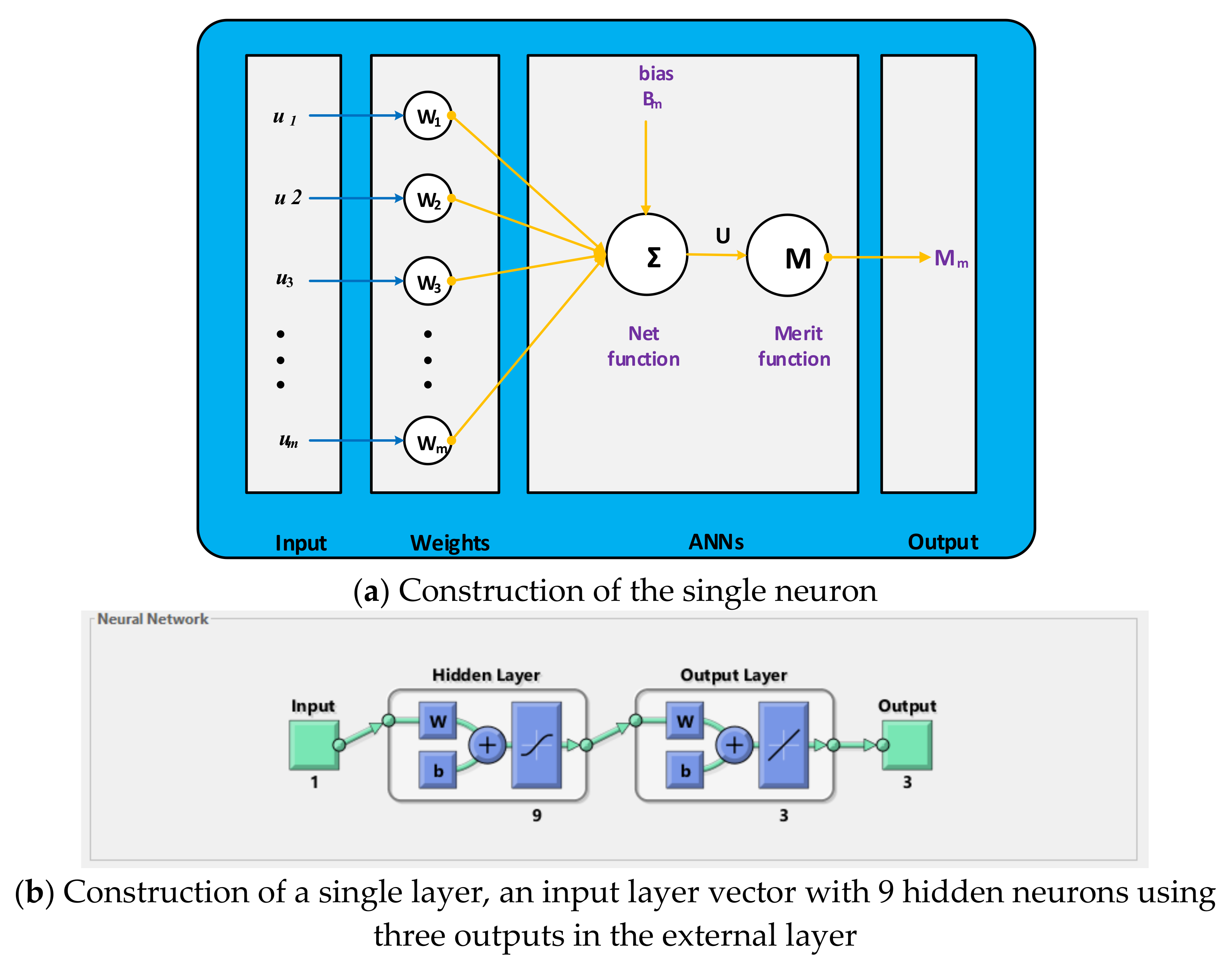
3. Proposed ANNs along with the Bayesian Regularization Approach
- (i)
- The noteworthy ANNs procedures along with the Bayesian regularization method are presented.
- (ii)
- The execution performances using the computing ANNs procedures along with the Bayesian regularization method for the dynamical FFSCS.
4. Results Based on the Dynamical FFSCS
5. Concluding Remarks
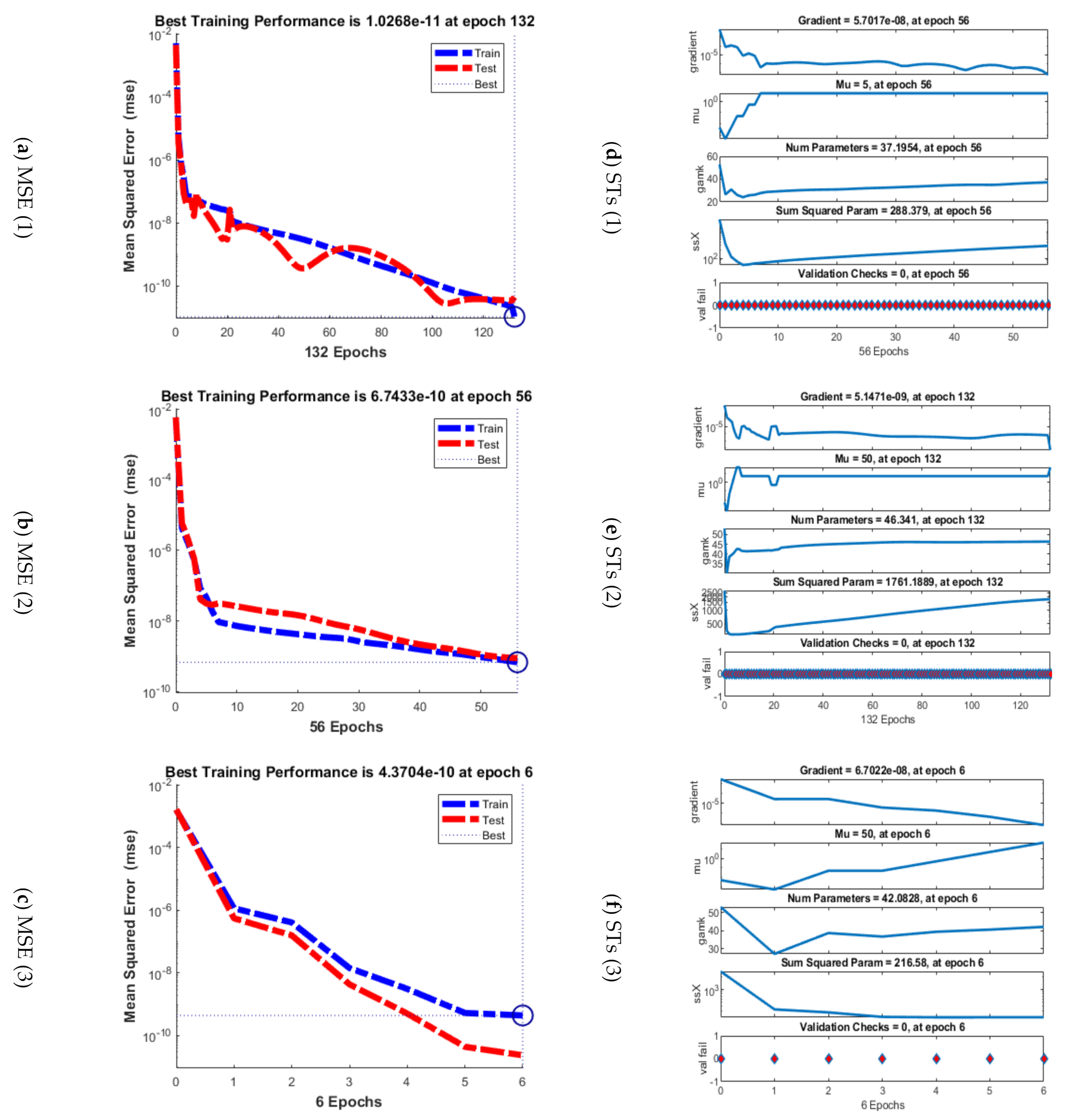
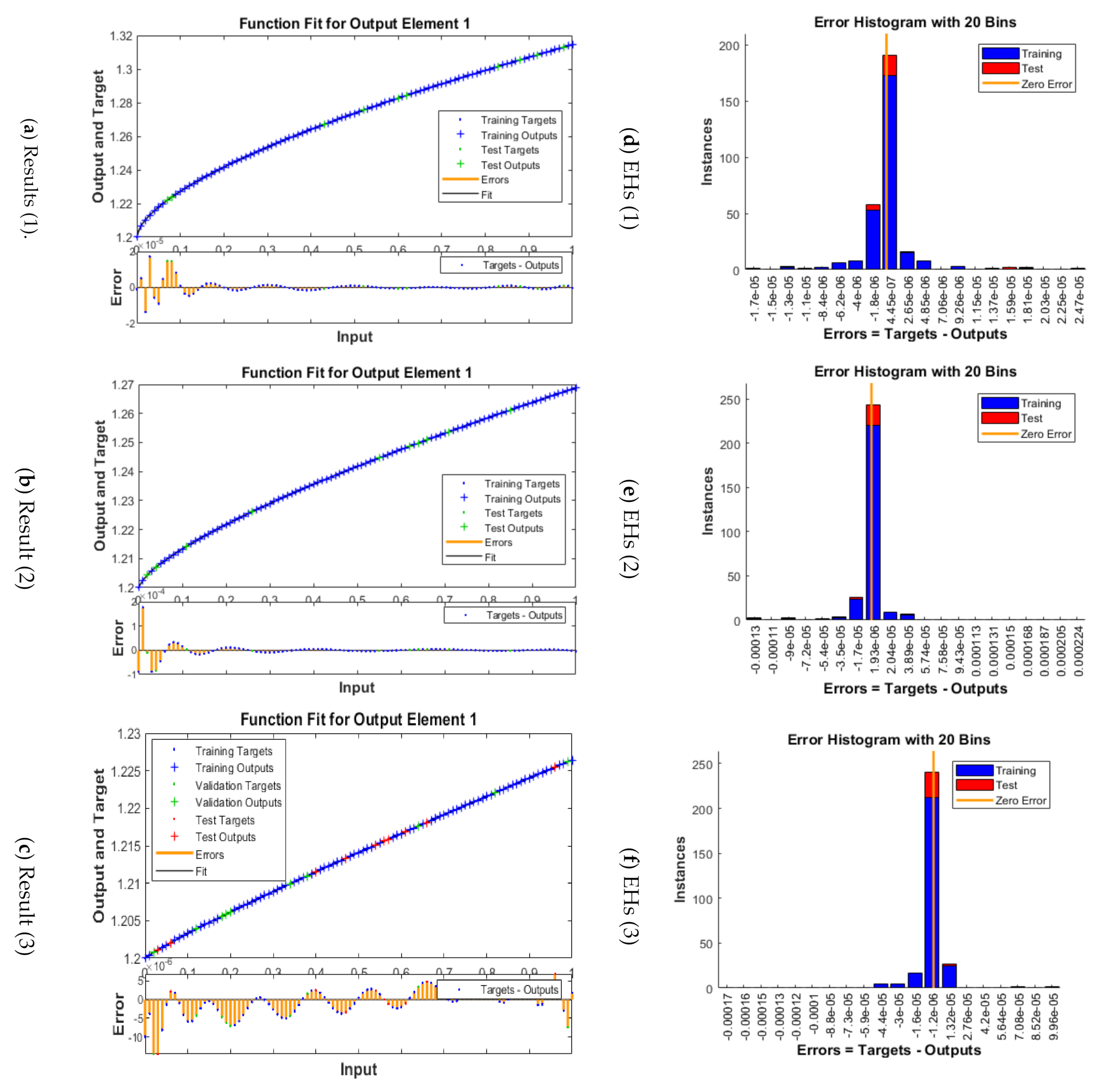
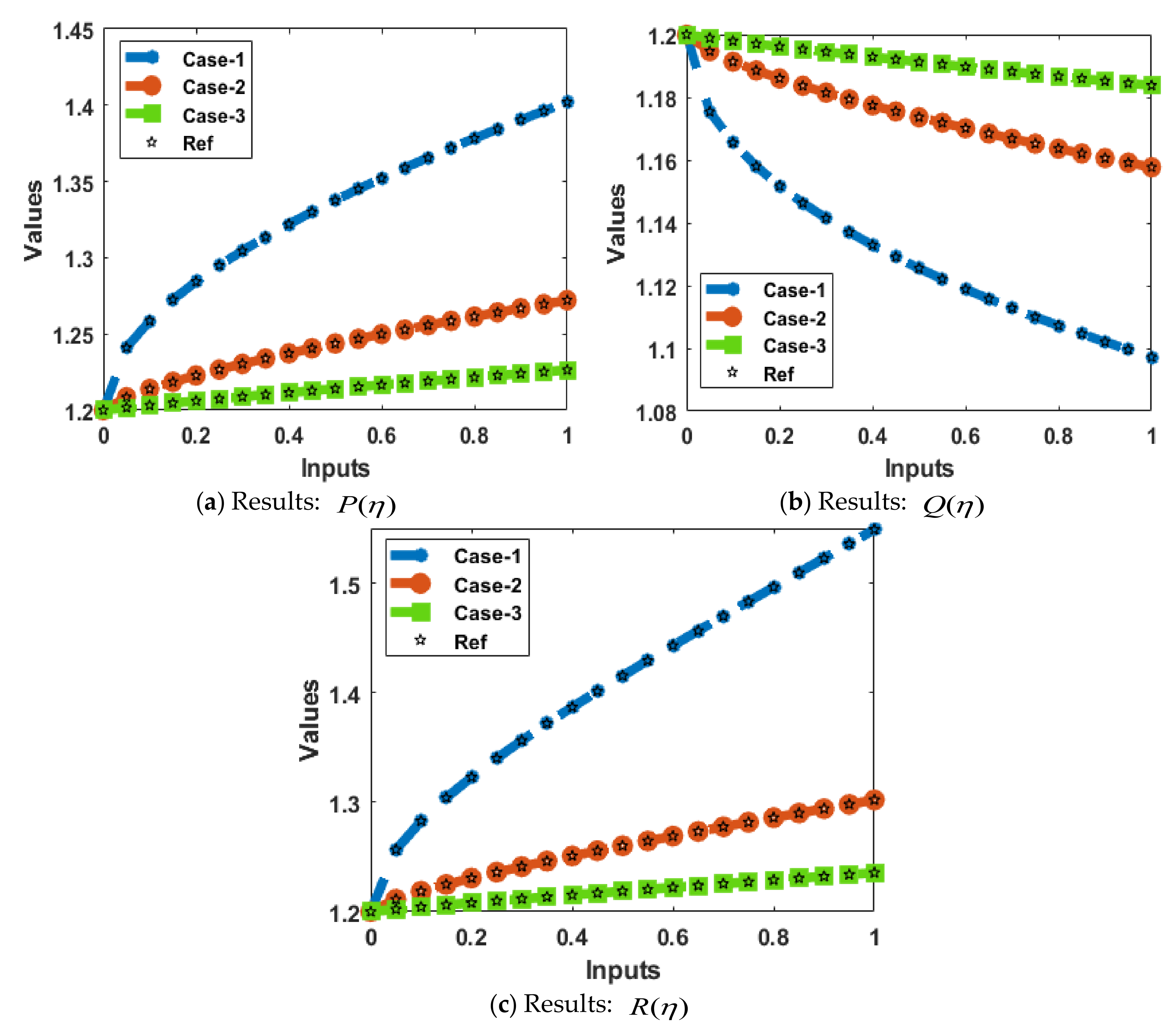
- The computing numerical performances for three different variations of the dynamical FFSCS have been provided by using the ANNs along with the Bayesian regularization technique.
- The data selection for the dynamical FFSCS is selected for train as 78% and 11% for both test and endorsement.
- The accuracy of the designed ANNs along with the Bayesian regularization approach has been approved by using the comparison of obtained and reference solutions.
- The AE for each variation of the mathematical dynamical FFSCS are performed in good measures, which presents the exactness of the scheme.
- For the rationality, ability, reliability, and exactness are authenticated by using the ANNs procedure enhanced by the Bayesian regularization method through the statistical performances.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Berryman, A.A. The orgins and evolution of predator-prey theory. Ecology 1992, 73, 1530–1535. [Google Scholar] [CrossRef]
- Damien, M.; Tougeron, K. Prey–predator phenological mismatch under climate change. Curr. Opin. Insect Sci. 2019, 35, 60–68. [Google Scholar] [CrossRef] [PubMed]
- Holling, C.S. The components of predation as revealed by a study of small-mammal predation of the European pine sawfly. Can. Entomol. 1959, 91, 293–320. [Google Scholar] [CrossRef]
- Holling, C.S. Some characteristics of simple types of predation and parasitism. Can. Entomol. 1959, 91, 385–398. [Google Scholar] [CrossRef]
- Sivasamy, R.; Sivakumar, M.; Balachandran, K.; Sathiyanathan, K. Spatial pattern of ratiodependent predator–prey model with prey harvesting and cross-diffusion. Intern. J. Bifurc. Chaos 2019, 29, 1950036. [Google Scholar] [CrossRef]
- Misra, A.K.; Dubey, B. A ratio-dependent predator–prey model with delay and harvesting. J. Biol. Syst. 2010, 18, 437–453. [Google Scholar] [CrossRef]
- Arditi, R.; Ginzburg, L.R. Coupling in predator–prey dynamics: Ratio-dependence. J. Theoret. Biol. 1989, 139, 311–326. [Google Scholar] [CrossRef]
- Beddington, J.R. Mutual interference between parasites or predators and its effect on searching efficiency. J. Anim. Ecol. 1975, 44, 331–340. [Google Scholar] [CrossRef]
- Pal, P.J.; Mandal, P.K. Bifurcation analysis of a modified Leslie–Gower predator–prey model with Beddington–DeAngelis functional response and strong Allee effect. Math. Comput. Simul. 2014, 97, 123–146. [Google Scholar] [CrossRef]
- DeAngelis, D.L.; Goldstein, R.A.; O’Neill, R.V. A model for tropic interaction. Ecology 1975, 56, 881–892. [Google Scholar] [CrossRef]
- Hastings, A.; Powell, T. Chaos in a three-species food chain. Ecology 1991, 72, 896–903. [Google Scholar] [CrossRef]
- Jana, D.; Agrawal, R.; Upadhyay, R.K. Toppredator interference and gestation delay as determinants of the dynamics of a realistic model food chain. Chaos Solitons Fractals 2014, 69, 50–63. [Google Scholar] [CrossRef]
- Upadhyay, R.K.; Naji, R.K. Dynamics of a three species food chain model with Crowley–Martin type functional response. Chaos Solitons Fractals 2009, 42, 1337–1346. [Google Scholar] [CrossRef]
- Umar, M.; Sabir, Z.; Raja, M.A.Z.; Sánchez, Y.G. A stochastic numerical computing heuristic of SIR nonlinear model based on dengue fever. Results Phys. 2020, 19, 103585. [Google Scholar] [CrossRef]
- Umar, M.; Sabir, Z.; Raja, M.A.Z.; Amin, F.; Saeed, T.; Guerrero-Sanchez, Y. Integrated neuro-swarm heuristic with interior-point for nonlinear SITR model for dynamics of novel COVID-19. Alex. Eng. J. 2021, 60, 2811–2824. [Google Scholar] [CrossRef]
- Sánchez, Y.G.; Sabir, Z.; Günerhan, H.; Baskonus, H.M. Analytical and approximate solutions of a novel nervous stomach mathematical model. Discret. Dyn. Nat. Soc. 2020, 2020, 5063271. [Google Scholar]
- Brassil, C.E. Mean time to extinction of a metapopulation with an Allee effect. Ecol. Model. 2001, 143, 9–16. [Google Scholar] [CrossRef]
- Dennis, B. Allee effects: Population growth, critical density, and the chance of extinction. Natur. Resour. Modeling 1989, 3, 481–538. [Google Scholar] [CrossRef]
- Cai, Y.; Zhao, C.; Wang, W.; Wang, J. Dynamics of a Leslie–Gower predator–prey model with additive Allee effect. Appl. Math. Model 2015, 39, 2092–2106. [Google Scholar] [CrossRef]
- Indrajaya, D.; Suryanto, A.; Alghofari, A.R. Dynamics of modified Leslie–Gower predator–prey model with Beddington–DeAngelis functional response and additive Allee effect. Int. J. Ecol. Dev. 2016, 31, 60–71. [Google Scholar]
- Vinoth, S.; Sivasamy, R.; Sathiyanathan, K.; Rajchakit, G.; Hammachukiattikul, P.; Vadivel, R.; Gunasekaran, N. Dynamical analysis of a delayed food chain model with additive Allee effect. Adv. Differ. Equ. 2021, 2021, 54. [Google Scholar] [CrossRef]
- Goyal, M.; Baskonus, H.M.; Prakash, A. An efficient technique for a time fractional model of lassa hemorrhagic fever spreading in pregnant women. Eur. Phys. J. Plus 2019, 134, 482. [Google Scholar] [CrossRef]
- Guirao, J.L.G.; Sabir, Z.; Saeed, T. Design and numerical solutions of a novel third-order nonlinear Emden–Fowler delay differential model. Math. Probl. Eng. 2020, 2020, 1–9. [Google Scholar] [CrossRef]
- Umar, M.; Sabir, Z.; Raja, M.A.Z.; Shoaib, M.; Gupta, M.; Sánchez, Y.G. A stochastic intelligent computing with neuro-evolution heuristics for nonlinear SITR system of novel COVID-19 dynamics. Symmetry 2020, 12, 1628. [Google Scholar] [CrossRef]
- Umar, M.; Sabir, Z.; Raja, M.A.Z.; Aguilar, J.G.; Amin, F.; Shoaib, M. Neuro-swarm intelligent computing paradigm for nonlinear HIV infection model with CD4+ T-cells. Math. Comput. Simul. 2021, 188, 241–253. [Google Scholar] [CrossRef]
- Sabir, Z. Stochastic numerical investigations for nonlinear three-species food chain system. Int. J. Biomath. 2022, 15, 2250005. [Google Scholar] [CrossRef]
- Sabir, Z.; Guirao, J.L.; Saeed, T. Solving a novel designed second order nonlinear Lane–Emden delay differential model using the heuristic techniques. Appl. Soft Comput. 2021, 102, 107105. [Google Scholar] [CrossRef]
- Yokuş, A.; Gülbahar, S. Numerical solutions with linearization techniques of the fractional Harry Dym equation. Appl. Math. Nonlinear Sci. 2019, 4, 35–42. [Google Scholar] [CrossRef]
- Ilhan, E.; Kıymaz, I.O. A generalization of truncated M-fractional derivative and applications to fractional differential equations. Appl. Math. Nonlinear Sci. 2020, 5, 171–188. [Google Scholar] [CrossRef]
- Bonilla, B.; Rivero, M.; Trujillo, J.J. On systems of linear fractional differential equations with constant coefficients. Appl. Math. Comput. 2007, 187, 68–78. [Google Scholar] [CrossRef]
- Ibrahim, R.W.; Momani, S. On the existence and uniqueness of solutions of a class of fractional differential equations. J. Math. Anal. Appl. 2007, 334, 1–10. [Google Scholar] [CrossRef]
- Yu, F. Integrable coupling system of fractional soliton equation hierarchy. Phys. Lett. A 2009, 373, 3730–3733. [Google Scholar] [CrossRef]
- Momani, S.; Ibrahim, R.W. On a fractional integral equation of periodic functions involving Weyl–Riesz operator in Banach algebras. J. Math. Anal. Appl. 2008, 339, 1210–1219. [Google Scholar] [CrossRef]
- Diethelm, K.; Ford, N.J. Analysis of fractional differential equations. J. Math. Anal. Appl. 2002, 265, 229–248. [Google Scholar] [CrossRef]
- Kuang, Y.; Beretta, E. Global quantities analysis of a ratio-dependent predator–prey system. J. Math. Biol. 1998, 36, 389–406. [Google Scholar] [CrossRef]
- Hassell, M.P. The Dynamics of Arthropod Predatorprey Systems; Princeton University Press: Princeton, NJ, USA, 1978. [Google Scholar]
- Ma, Z.; Li, W.; Yan, X. Stability and Hopf bifurcation for a three species food chain model with time delay and spatial diffusion. Appl. Math. Comput. 2012, 219, 2713–2731. [Google Scholar] [CrossRef]
- Freedman, H.I.; So, J.H. Global stability and persistence of simple food chains. Math. Biosci. 1985, 76, 69–86. [Google Scholar] [CrossRef]
- El-Owaidy, H.; Ragab, A.; Ismail, M. Mathematical analysis of a food-web model. Appl. Math. Comput. 2001, 121, 155–167. [Google Scholar] [CrossRef]
- Kuznetsov, Y.; Rinaldi, S. Remarks on food chain dynamics. Math. Biosci. 1996, 134, 1–33. [Google Scholar] [CrossRef]
- Freedman, H.; Waltman, P. Mathematical analysis of some three-species food-chain models. Math. Biosci. 1977, 33, 257–276. [Google Scholar] [CrossRef]
- Rinaldi, S.; Bo, S.D.; De Nittis, E. On the role of body size in a tri-trophic metapopulation model. J. Math. Biol. 1996, 35, 158–176. [Google Scholar] [CrossRef]
- Muratori, S.; Rinaldi, S. Low-and high-frequency oscillations in three-dimensional food chain systems. SIAM J. Appl. Math. 1992, 52, 1688–1706. [Google Scholar] [CrossRef]
- Aziz-Alaoui, M.A. Study of a Leslie–Gower-type tritrophic population model. Chaos Solitons Fractals 2002, 14, 1275–1293. [Google Scholar] [CrossRef]
- Upadhyay, R.K.; Iyengar, S.R.K.; Rai, V. Chaos: An ecological reality? Int. J. Bifurc. Chaos 1998, 8, 1325–1333. [Google Scholar] [CrossRef]
- Leslie, P.H.; Gower, J.C. The properties of a stochastic model for the predator-prey type of interaction between two species. Biometrika 1960, 47, 219–234. [Google Scholar] [CrossRef]
- Shah, K.; Alqudah, M.A.; Jarad, F.; Abdeljawad, T. Semi-analytical study of Kuang Pine Wilt Disease model with convex rate under Caputo–Febrizio fractional order derivative. Chaos Solitons Fractals 2020, 135, 109754. [Google Scholar] [CrossRef]
- Yang, X.J.; Ragulskis, M.; Tana, T. A new general fractional-order derivataive with Rabotnov fractional-exponential kernel applied to model the anomalous heat transfer. Therm. Sci. 2019, 23, 1677–1681. [Google Scholar] [CrossRef]
- Owolabi, K.M.; Hammouch, Z. Spatiotemporal patterns in the Belousov–Zhabotinskii reaction systems with Atangana–Baleanu fractional order derivative. Phys. A Stat. Mech. Its Appl. 2019, 523, 1072–1090. [Google Scholar] [CrossRef]
- Ghanbari, B.; Djilali, S. Mathematical and numerical analysis of a three-species predator-prey model with herd behavior and time fractional-order derivative. Math. Methods Appl. Sci. 2020, 43, 1736–1752. [Google Scholar] [CrossRef]
- Din, A.; Li, Y.; Khan, F.M.; Khan, Z.U.; Liu, P. On Analysis of fractional order mathematical model of Hepatitis B using Atangana–Baleanu Caputo (ABC) derivative. Fractals 2022, 30, 2240017. [Google Scholar] [CrossRef]
- Srivastava, H.M.; Dubey, V.P.; Kumar, R.; Singh, J.; Kumar, J.; Baleanu, D. An efficient computational approach for a fractional-order biological population model with carrying capacity. Chaos Solitons Fractals 2020, 138, 109880. [Google Scholar] [CrossRef]
- Hong, Y.; Liu, Y.; Chen, Y.; Liu, Y.; Yu, L.; Liu, Y.; Cheng, H. Application of fractional-order derivative in the quantitative estimation of soil organic matter content through visible and near-infrared spectroscopy. Geoderma 2019, 337, 758–769. [Google Scholar] [CrossRef]



| Parameter | Settings |
|---|---|
| Maximum mu performances | 1010 |
| Fitness measures (MSE) | 0 |
| Hidden neurons | 9 |
| Decreeing mu performances | 0.2 |
| Increasing mu measures | 12 |
| Adaptive parameter (mu) | 6 × 10−0.4 |
| Substantiation fail amount | 6 |
| Maximum Epochs | 720 |
| Minimum gradient | 10−0.6 |
| Training data | 78% |
| Validation data | 11% |
| Testing data | 11% |
| Sample selection | Random |
| Hidden/output/input | Single |
| Dataset generation | Adam |
| Implementation and stoppage standards | Default |
| Case | MSE | Epoch | Gradient | Performance | Mu | Time | |
|---|---|---|---|---|---|---|---|
| Test | Train | ||||||
| 1 | 4.35× 10−11 | 1.02 × 10−11 | 132 | 5.15 × 10−09 | 1.03 × 10−11 | 50 | 4 |
| 2 | 8.812 × 10−10 | 6.743 × 10−10 | 56 | 5.70 × 10−09 | 6.74 × 10−10 | 5 | 2 |
| 3 | 2.331× 10−11 | 4.37 × 10−10 | 6 | 6.67 × 10−08 | 4.37 × 10−10 | 50 | 2 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Souayeh, B.; Sabir, Z.; Hdhiri, N.; Al-Kouz, W.; Alam, M.W.; Alsheddi, T. A Stochastic Bayesian Regularization Approach for the Fractional Food Chain Supply System with Allee Effects. Fractal Fract. 2022, 6, 553. https://doi.org/10.3390/fractalfract6100553
Souayeh B, Sabir Z, Hdhiri N, Al-Kouz W, Alam MW, Alsheddi T. A Stochastic Bayesian Regularization Approach for the Fractional Food Chain Supply System with Allee Effects. Fractal and Fractional. 2022; 6(10):553. https://doi.org/10.3390/fractalfract6100553
Chicago/Turabian StyleSouayeh, Basma, Zulqurnain Sabir, Najib Hdhiri, Wael Al-Kouz, Mir Waqas Alam, and Tarfa Alsheddi. 2022. "A Stochastic Bayesian Regularization Approach for the Fractional Food Chain Supply System with Allee Effects" Fractal and Fractional 6, no. 10: 553. https://doi.org/10.3390/fractalfract6100553
APA StyleSouayeh, B., Sabir, Z., Hdhiri, N., Al-Kouz, W., Alam, M. W., & Alsheddi, T. (2022). A Stochastic Bayesian Regularization Approach for the Fractional Food Chain Supply System with Allee Effects. Fractal and Fractional, 6(10), 553. https://doi.org/10.3390/fractalfract6100553









