Front Propagation of Exponentially Truncated Fractional-Order Epidemics
Abstract
:1. Introduction
2. Preliminaries for Fractional-Order Operators
3. Spatial Propagation of an Epidemic
3.1. Brownian Motion
3.2. Pure (Untruncated) Lévy Flights
3.3. Truncated Lévy Flights
3.3.1. Theoretical Analysis (Right-Propagating Front)
3.3.2. Theoretical Analysis (Left-Propagating Front)
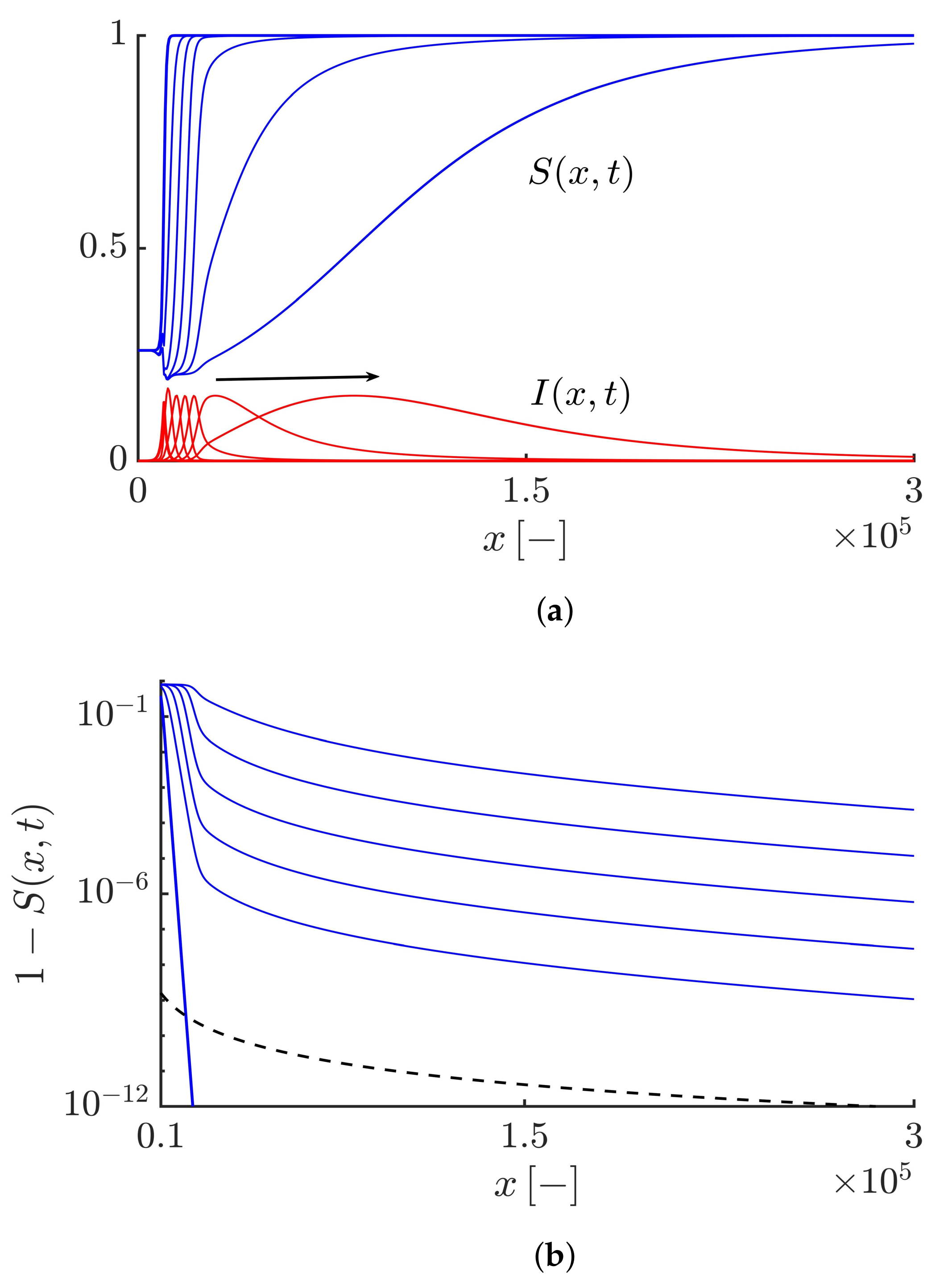
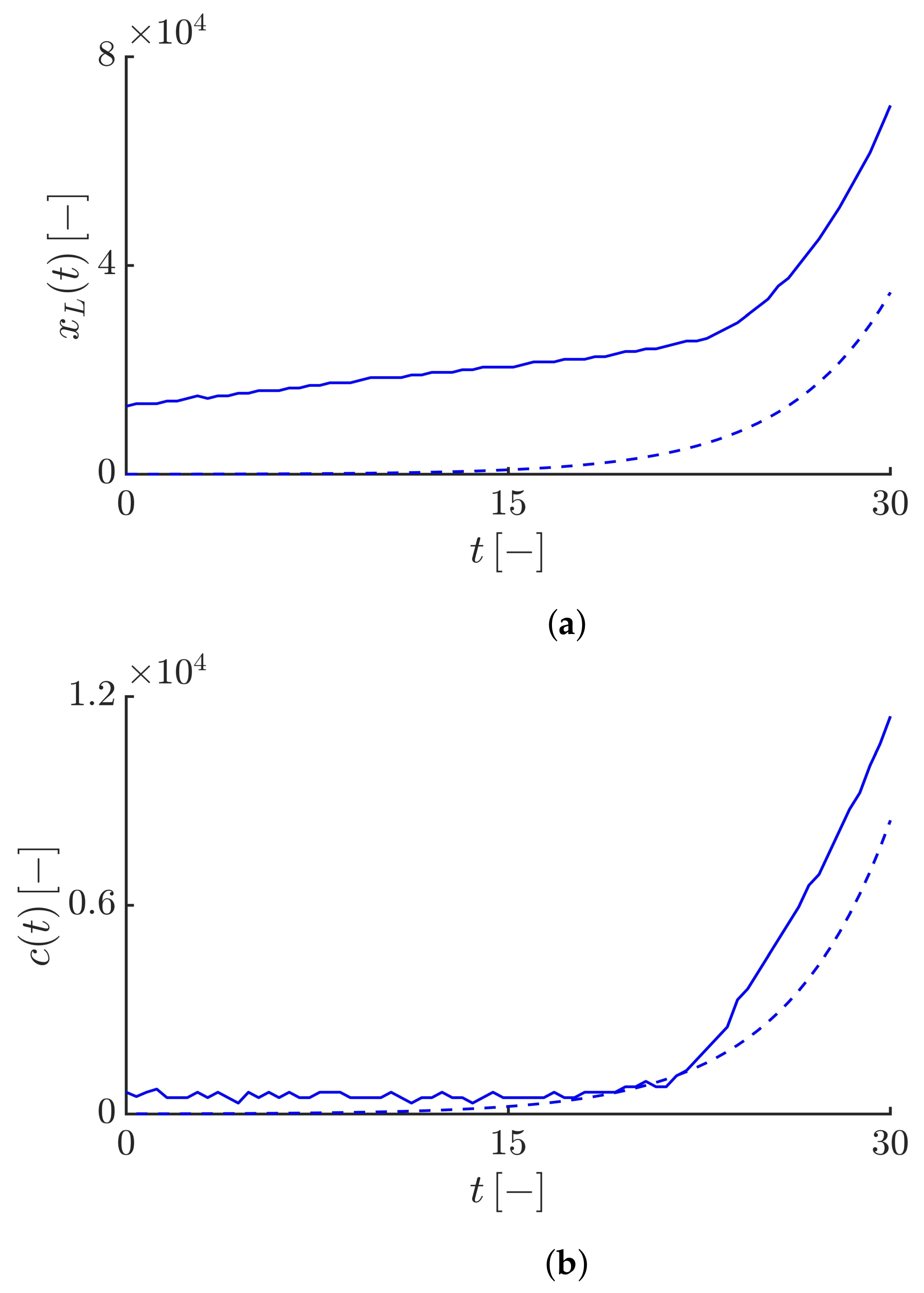
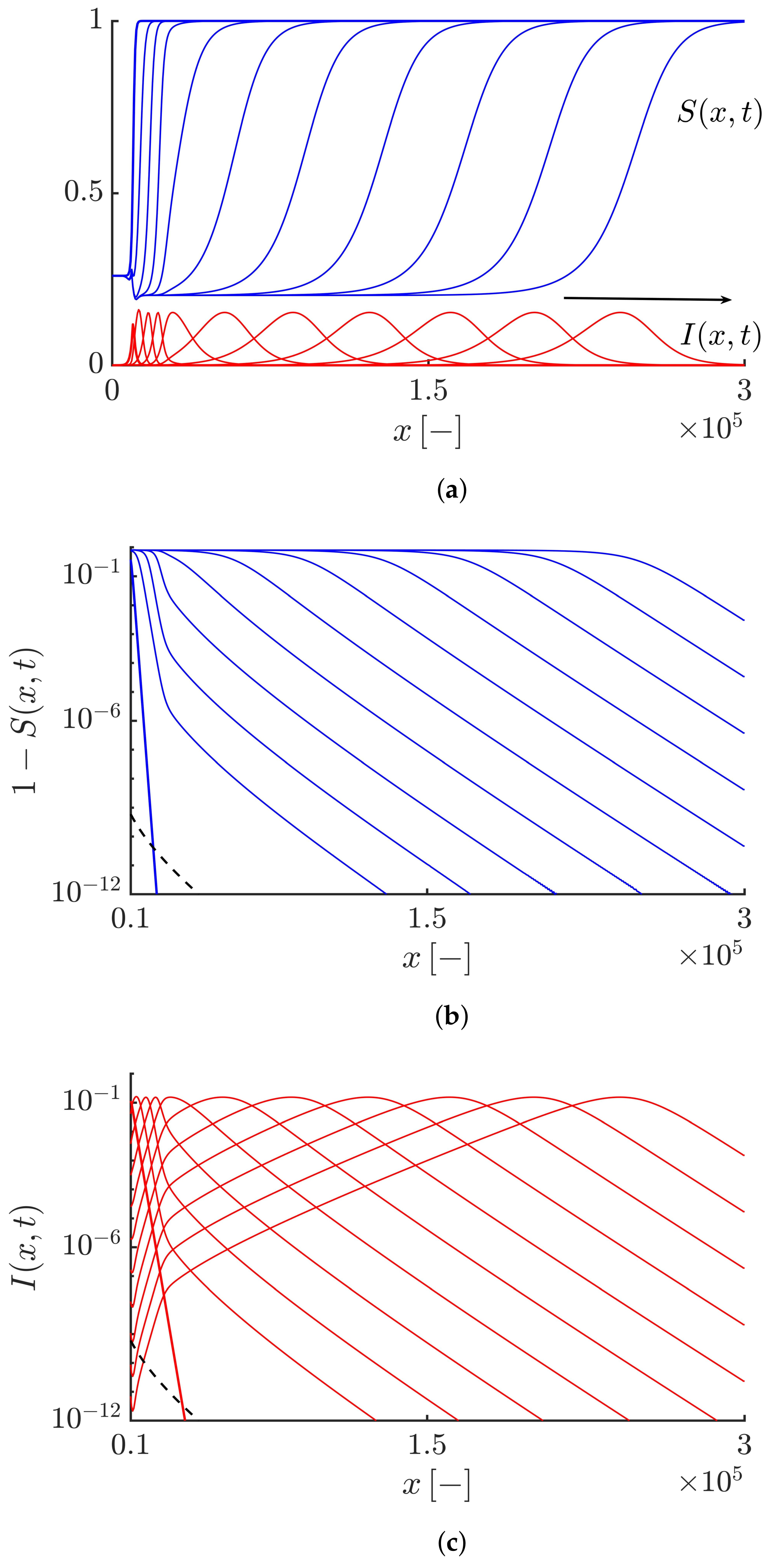
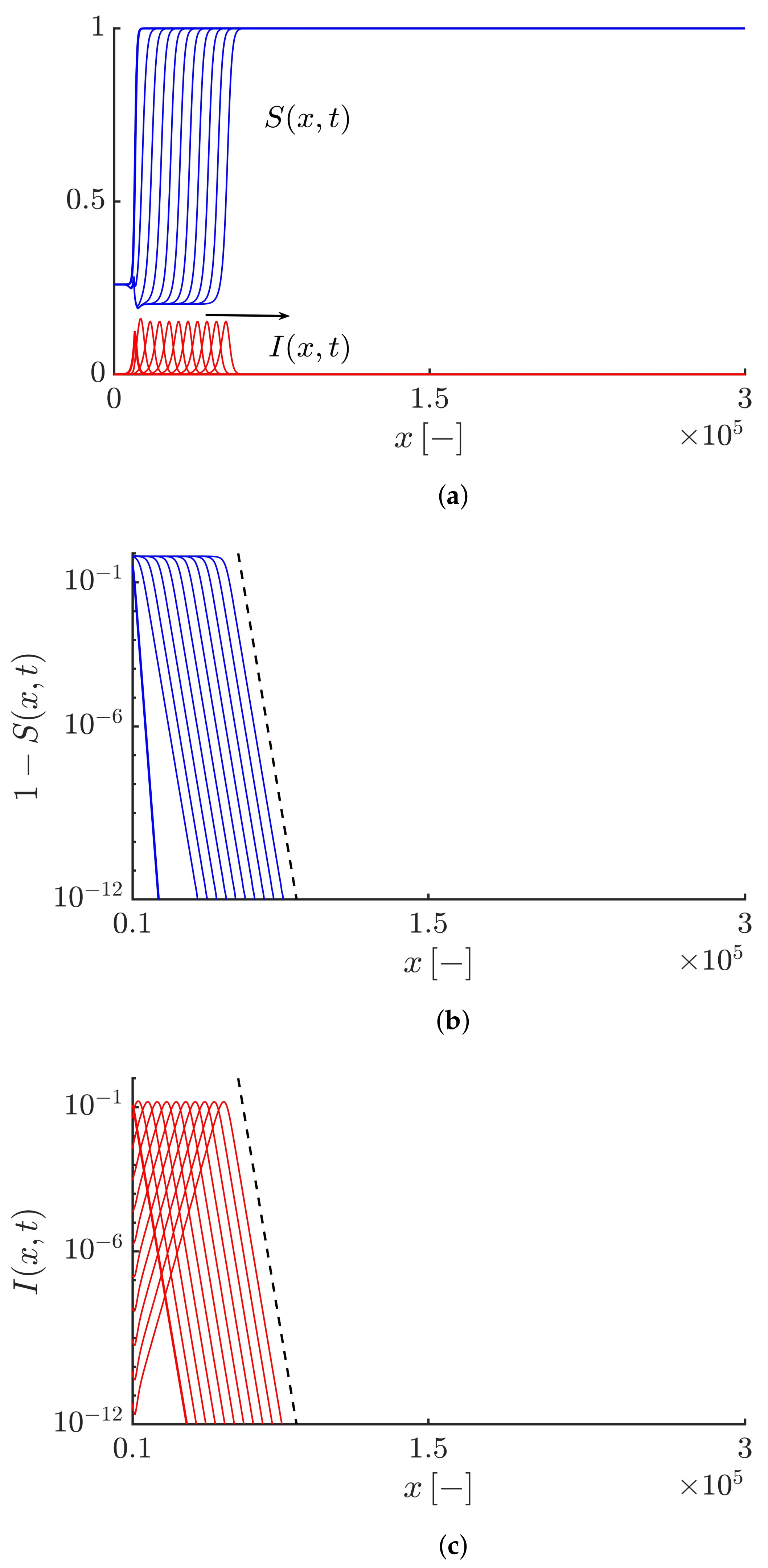
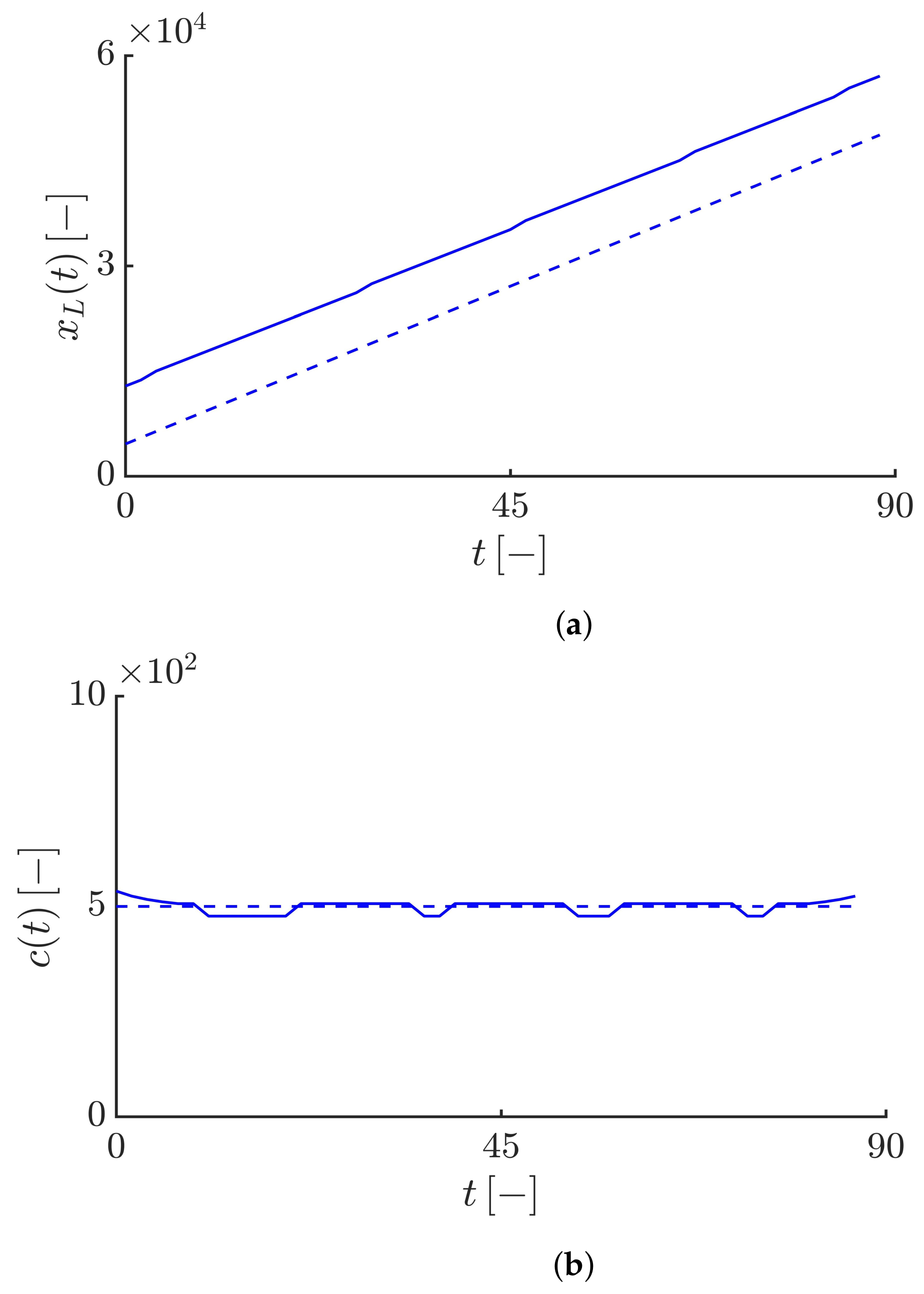
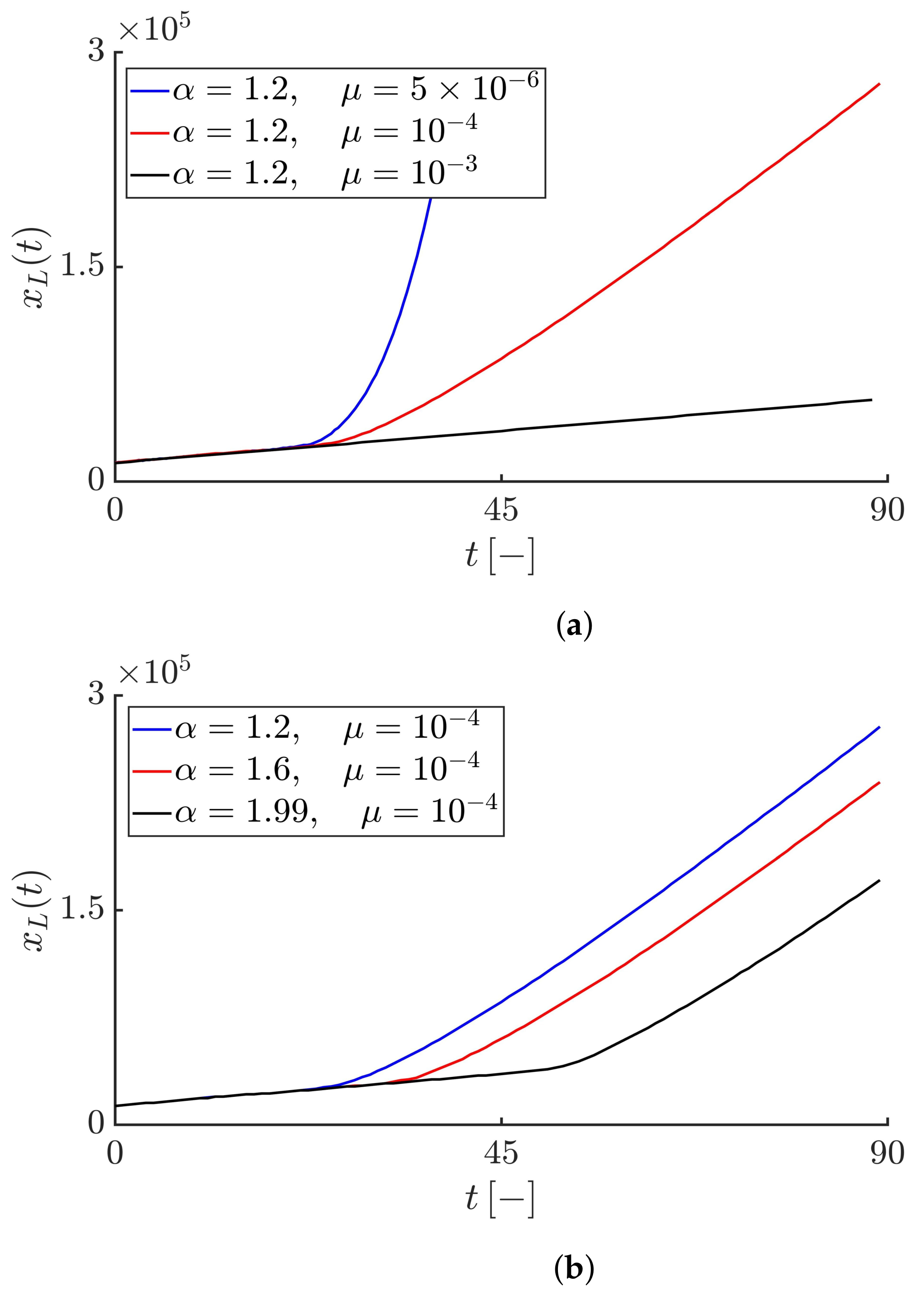
4. Numerical Examples
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Cleaveland, S.; Laurenson, M.K.; Taylor, L.H. Diseases of humans and their domestic mammals: Pathogen characteristics, host range and the risk of emergence. Philos. Trans. R. Soc. London. Ser. B Biol. Sci. 2001, 356, 991–999. [Google Scholar] [CrossRef] [PubMed]
- Nelson, K.E.; Williams, C.M. Infectious Disease Epidemiology: Theory and Practice; Jones & Bartlett Publishers: Burlington, MA, USA, 2014. [Google Scholar]
- Ciotti, M.; Ciccozzi, M.; Terrinoni, A.; Jiang, W.C.; Wang, C.B.; Bernardini, S. The COVID-19 pandemic. Crit. Rev. Clin. Lab. Sci. 2020, 57, 365–388. [Google Scholar] [CrossRef] [PubMed]
- Brauer, F. Modeling influenza: Pandemics and seasonal epidemics. In Mathematical Epidemiology; Springer: Berlin/Heidelberg, Germany, 2008; pp. 321–347. [Google Scholar]
- Cruz-Pacheco, G.; Esteva, L.; Montaño-Hirose, J.A.; Vargas, C. Modelling the dynamics of West Nile virus. Bull. Math. Biol. 2005, 67, 1157–1172. [Google Scholar] [CrossRef] [PubMed]
- Esteva, L.; Vargas, C. Analysis of a dengue disease transmission model. Math. Biosci. 1998, 150, 131–151. [Google Scholar] [CrossRef]
- Chen, Y.C.; Lu, P.E.; Chang, C.S.; Liu, T.H. A time-dependent SIR model for COVID-19 with undetectable infected persons. IEEE Trans. Netw. Sci. Eng. 2020, 7, 3279–3294. [Google Scholar] [CrossRef]
- Keeling, M.J.; Rohani, P. Modeling Infectious Diseases in Humans and Animals; Princeton University Press: Princeton, NJ, USA, 2011. [Google Scholar]
- Van den Driessche, P.; Watmough, J. Further notes on the basic reproduction number. In Mathematical Epidemiology; Springer: Berlin/Heidelberg, Germany, 2008; pp. 159–178. [Google Scholar]
- Bowman, C.; Gumel, A.; Van den Driessche, P.; Wu, J.; Zhu, H. A mathematical model for assessing control strategies against West Nile virus. Bull. Math. Biol. 2005, 67, 1107–1133. [Google Scholar] [CrossRef] [PubMed]
- Wu, J. Spatial structure: Partial differential equations models. In Mathematical Epidemiology; Springer: Berlin/Heidelberg, Germany, 2008; pp. 191–203. [Google Scholar]
- Maidana, N.A.; Yang, H.M. Spatial spreading of West Nile Virus described by traveling waves. J. Theor. Biol. 2009, 258, 403–417. [Google Scholar] [CrossRef]
- Maidana, N.A.; Yang, H.M. Describing the geographic spread of dengue disease by traveling waves. Math. Biosci. 2008, 215, 64–77. [Google Scholar] [CrossRef]
- Källén, A.; Arcuri, P.; Murray, J. A simple model for the spatial spread and control of rabies. J. Theor. Biol. 1985, 116, 377–393. [Google Scholar] [CrossRef]
- Murray, J.D. Mathematical Biology II: Spatial Models and Biomedical Applications; Springer: New York, NY, USA, 2001; Volume 3. [Google Scholar]
- Okubo, A.; Levin, S.A. Diffusion and Ecological Problems: Modern Perspectives; Springer: New York, NY, USA, 2001; Volume 14. [Google Scholar]
- Shigesada, N.; Kawasaki, K. Biological Invasions: Theory and Practice; Oxford University Press: Oxford, UK, 1997. [Google Scholar]
- Metzler, R.; Klafter, J. The random walk’s guide to anomalous diffusion: A fractional dynamics approach. Phys. Rep. 2000, 339, 1–77. [Google Scholar] [CrossRef]
- Humphries, N.E.; Queiroz, N.; Dyer, J.R.; Pade, N.G.; Musyl, M.K.; Schaefer, K.M.; Fuller, D.W.; Brunnschweiler, J.M.; Doyle, T.K.; Houghton, J.D.; et al. Environmental context explains Lévy and Brownian movement patterns of marine predators. Nature 2010, 465, 1066–1069. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Sims, D.W.; Southall, E.J.; Humphries, N.E.; Hays, G.C.; Bradshaw, C.J.; Pitchford, J.W.; James, A.; Ahmed, M.Z.; Brierley, A.S.; Hindell, M.A.; et al. Scaling laws of marine predator search behaviour. Nature 2008, 451, 1098–1102. [Google Scholar] [CrossRef] [PubMed]
- Reynolds, A.M.; Frye, M.A. Free-flight odor tracking in Drosophila is consistent with an optimal intermittent scale-free search. PLoS ONE 2007, 2, e354. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Lihoreau, M.; Ings, T.C.; Chittka, L.; Reynolds, A.M. Signatures of a globally optimal searching strategy in the three-dimensional foraging flights of bumblebees. Sci. Rep. 2016, 6, 30401. [Google Scholar] [CrossRef] [Green Version]
- Reynolds, A.M.; Smith, A.D.; Menzel, R.; Greggers, U.; Reynolds, D.R.; Riley, J.R. Displaced honey bees perform optimal scale-free search flights. Ecology 2007, 88, 1955–1961. [Google Scholar] [CrossRef] [PubMed]
- Reynolds, A.M.; Smith, A.D.; Reynolds, D.R.; Carreck, N.L.; Osborne, J.L. Honeybees perform optimal scale-free searching flights when attempting to locate a food source. J. Exp. Biol. 2007, 210, 3763–3770. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Humphries, N.E.; Weimerskirch, H.; Sims, D.W. A new approach for objective identification of turns and steps in organism movement data relevant to random walk modelling. Methods Ecol. Evol. 2013, 4, 930–938. [Google Scholar] [CrossRef]
- Brockmann, D.; Hufnagel, L.; Geisel, T. The scaling laws of human travel. Nature 2006, 439, 462–465. [Google Scholar] [CrossRef]
- Chaves, A. A fractional diffusion equation to describe Lévy flights. Phys. Lett. A 1998, 239, 13–16. [Google Scholar] [CrossRef]
- Hanert, E. Front dynamics in a two-species competition model driven by Lévy flights. J. Theor. Biol. 2012, 300, 134–142. [Google Scholar] [CrossRef]
- Vallaeys, V.; Tyson, R.C.; Lane, W.D.; Deleersnijder, E.; Hanert, E. A Lévy-flight diffusion model to predict transgenic pollen dispersal. J. R. Soc. Interface 2017, 14, 20160889. [Google Scholar] [CrossRef] [PubMed]
- Raghib, M.; Levin, S.A.; Kevrekidis, I.G. Multiscale analysis of collective motion and decision-making in swarms: An advection–diffusion equation with memory approach. J. Theor. Biol. 2010, 264, 893–913. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- del Castillo-Negrete, D.; Carreras, B.; Lynch, V. Fractional diffusion in plasma turbulence. Phys. Plasmas 2004, 11, 3854–3864. [Google Scholar] [CrossRef]
- del Castillo-Negrete, D.; Carreras, B.; Lynch, V. Nondiffusive transport in plasma turbulence: A fractional diffusion approach. Phys. Rev. Lett. 2005, 94, 065003. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Cartea, A.; del Castillo-Negrete, D. Fractional diffusion models of option prices in markets with jumps. Phys. A Stat. Mech. Its Appl. 2007, 374, 749–763. [Google Scholar] [CrossRef] [Green Version]
- Yuan, J.; Zhao, L.; Huang, C.; Xiao, M. Stability and bifurcation analysis of a fractional predator–prey model involving two nonidentical delays. Math. Comput. Simul. 2021, 181, 562–580. [Google Scholar] [CrossRef]
- Huang, C.; Liu, H.; Chen, X.; Zhang, M.; Ding, L.; Cao, J.; Alsaedi, A. Dynamic optimal control of enhancing feedback treatment for a delayed fractional order predator–prey model. Phys. A Stat. Mech. Its Appl. 2020, 554, 124136. [Google Scholar] [CrossRef]
- Djilali, S.; Ghanbari, B.; Bentout, S.; Mezouaghi, A. Turing-Hopf bifurcation in a diffusive mussel-algae model with time-fractional-order derivative. Chaos Solitons Fractals 2020, 138, 109954. [Google Scholar] [CrossRef]
- Xu, C.; Liao, M.; Li, P.; Guo, Y.; Liu, Z. Bifurcation properties for fractional order delayed BAM neural networks. Cogn. Comput. 2021, 13, 322–356. [Google Scholar] [CrossRef]
- Huang, C.; Nie, X.; Zhao, X.; Song, Q.; Tu, Z.; Xiao, M.; Cao, J. Novel bifurcation results for a delayed fractional-order quaternion-valued neural network. Neural Netw. 2019, 117, 67–93. [Google Scholar] [CrossRef] [PubMed]
- Xu, C.; Zhang, W.; Aouiti, C.; Liu, Z.; Liao, M.; Li, P. Further investigation on bifurcation and their control of fractional-order bidirectional associative memory neural networks involving four neurons and multiple delays. Math. Methods Appl. Sci. 2021, 1–24. [Google Scholar] [CrossRef]
- Hanert, E.; Schumacher, E.; Deleersnijder, E. Front dynamics in fractional-order epidemic models. J. Theor. Biol. 2011, 279, 9–16. [Google Scholar] [CrossRef] [Green Version]
- Mantegna, R.N.; Stanley, H.E. Stochastic process with ultraslow convergence to a Gaussian: The truncated Lévy flight. Phys. Rev. Lett. 1994, 73, 2946. [Google Scholar] [CrossRef]
- Koponen, I. Analytic approach to the problem of convergence of truncated Lévy flights towards the Gaussian stochastic process. Phys. Rev. E 1995, 52, 1197. [Google Scholar] [CrossRef]
- Rosiński, J. Tempering stable processes. Stoch. Process. Their Appl. 2007, 117, 677–707. [Google Scholar] [CrossRef] [Green Version]
- Cartea, Á.; del Castillo-Negrete, D. Fluid limit of the continuous-time random walk with general Lévy jump distribution functions. Phys. Rev. E 2007, 76, 041105. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Baeumer, B.; Meerschaert, M.M. Tempered stable Lévy motion and transient super-diffusion. J. Comput. Appl. Math. 2010, 233, 2438–2448. [Google Scholar] [CrossRef] [Green Version]
- del Castillo-Negrete, D. Truncation effects in superdiffusive front propagation with Lévy flights. Phys. Rev. E 2009, 79, 031120. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Small, M.; Walker, D.M.; Tse, C.K. Scale-free distribution of avian influenza outbreaks. Phys. Rev. Lett. 2007, 99, 188702. [Google Scholar] [CrossRef] [Green Version]
- Mundt, C.C.; Sackett, K.E.; Wallace, L.D.; Cowger, C.; Dudley, J.P. Long-distance dispersal and accelerating waves of disease: Empirical relationships. Am. Nat. 2009, 173, 456–466. [Google Scholar] [CrossRef] [PubMed]
- Samko, S.G.; Kilbas, A.A.; Marichev, O.I. Fractional Integrals and Derivatives: Theory and Applications; Gordon and Breach: New York, NY, USA, 1993. [Google Scholar]
- Podlubny, I. Fractional Differential Equations: An Introduction to Fractional Derivatives, Fractional Differential Equations, to Methods of Their Solution and Some of Their Applications; Elsevier: Amsterdam, The Netherlands, 1998. [Google Scholar]
- Mainardi, F.; Luchko, Y.; Pagnini, G. The fundamental solution of the space-time fractional diffusion equation. arXiv 2007, arXiv:cond-mat/0702419. [Google Scholar]
- Hanert, E. On the numerical solution of space-time fractional diffusion models. Comput. Fluids 2011, 46, 33–39. [Google Scholar] [CrossRef]
- Piret, C.; Hanert, E. A radial basis functions method for fractional diffusion equations. J. Comput. Phys. 2013, 238, 71–81. [Google Scholar] [CrossRef]
- Hanert, E.; Piret, C. A Chebyshev pseudospectral method to solve the space-time tempered fractional diffusion equation. SIAM J. Sci. Comput. 2014, 36, A1797–A1812. [Google Scholar] [CrossRef]
- Hanert, E. A comparison of three Eulerian numerical methods for fractional-order transport models. Environ. Fluid Mech. 2010, 10, 7–20. [Google Scholar] [CrossRef]
- Zhang, X.; Lv, M.; Crawford, J.W.; Young, I.M. The impact of boundary on the fractional advection–dispersion equation for solute transport in soil: Defining the fractional dispersive flux with the Caputo derivatives. Adv. Water Resour. 2007, 30, 1205–1217. [Google Scholar] [CrossRef]
- Viswanathan, G.M.; Raposo, E.; Da Luz, M. Lévy flights and superdiffusion in the context of biological encounters and random searches. Phys. Life Rev. 2008, 5, 133–150. [Google Scholar] [CrossRef]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Farhadi, A.; Hanert, E. Front Propagation of Exponentially Truncated Fractional-Order Epidemics. Fractal Fract. 2022, 6, 53. https://doi.org/10.3390/fractalfract6020053
Farhadi A, Hanert E. Front Propagation of Exponentially Truncated Fractional-Order Epidemics. Fractal and Fractional. 2022; 6(2):53. https://doi.org/10.3390/fractalfract6020053
Chicago/Turabian StyleFarhadi, Afshin, and Emmanuel Hanert. 2022. "Front Propagation of Exponentially Truncated Fractional-Order Epidemics" Fractal and Fractional 6, no. 2: 53. https://doi.org/10.3390/fractalfract6020053
APA StyleFarhadi, A., & Hanert, E. (2022). Front Propagation of Exponentially Truncated Fractional-Order Epidemics. Fractal and Fractional, 6(2), 53. https://doi.org/10.3390/fractalfract6020053





