Analytical Solutions of the Predator–Prey Model with Fractional Derivative Order via Applications of Three Modified Mathematical Methods
Abstract
:1. Introduction
2. Formation of Methods
2.1. Modified Extended Auxiliary Equation Mapping Method
2.2. Extended Simple Equation Method
2.3. Modified F-Expansion Method
3. Applications
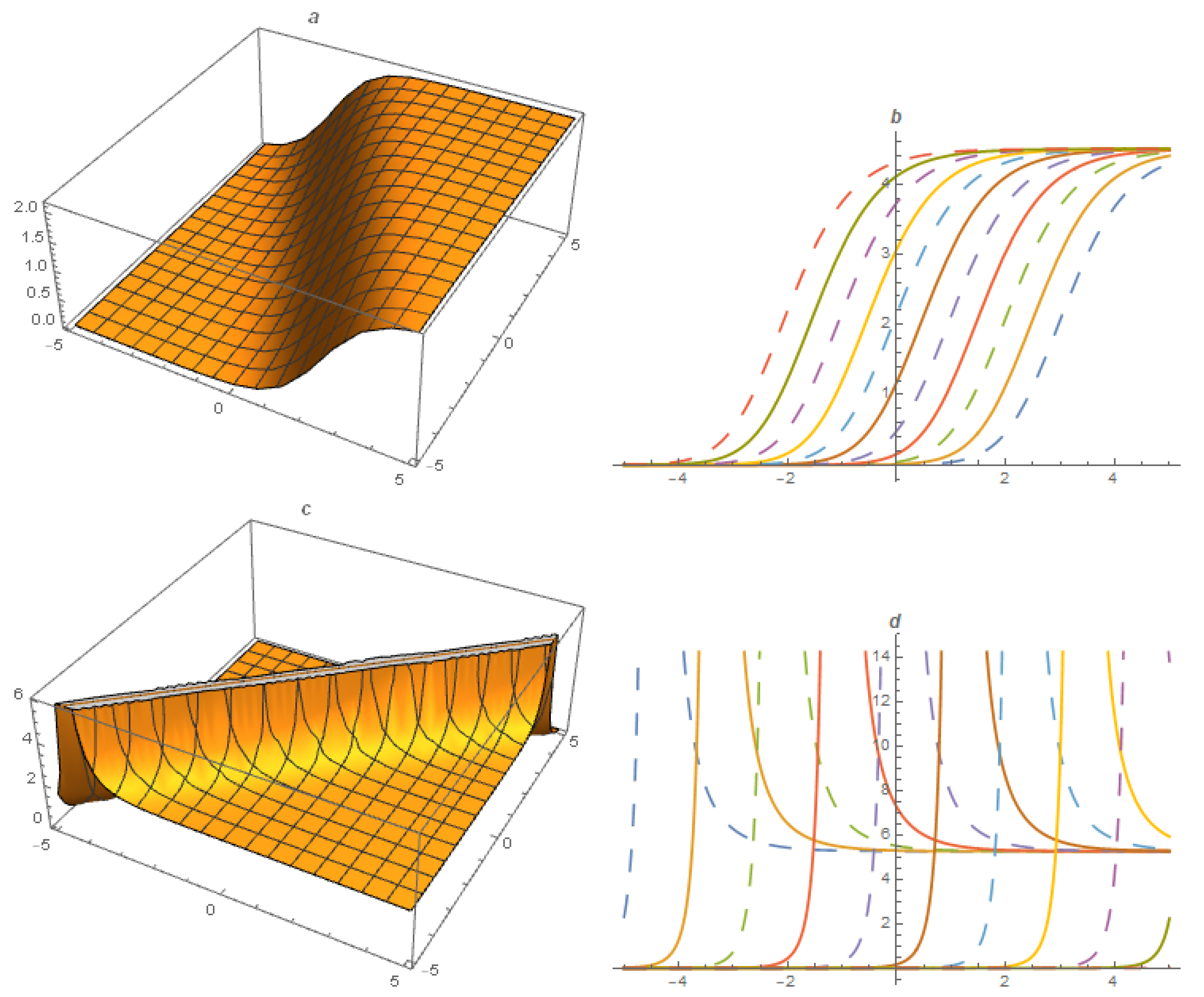
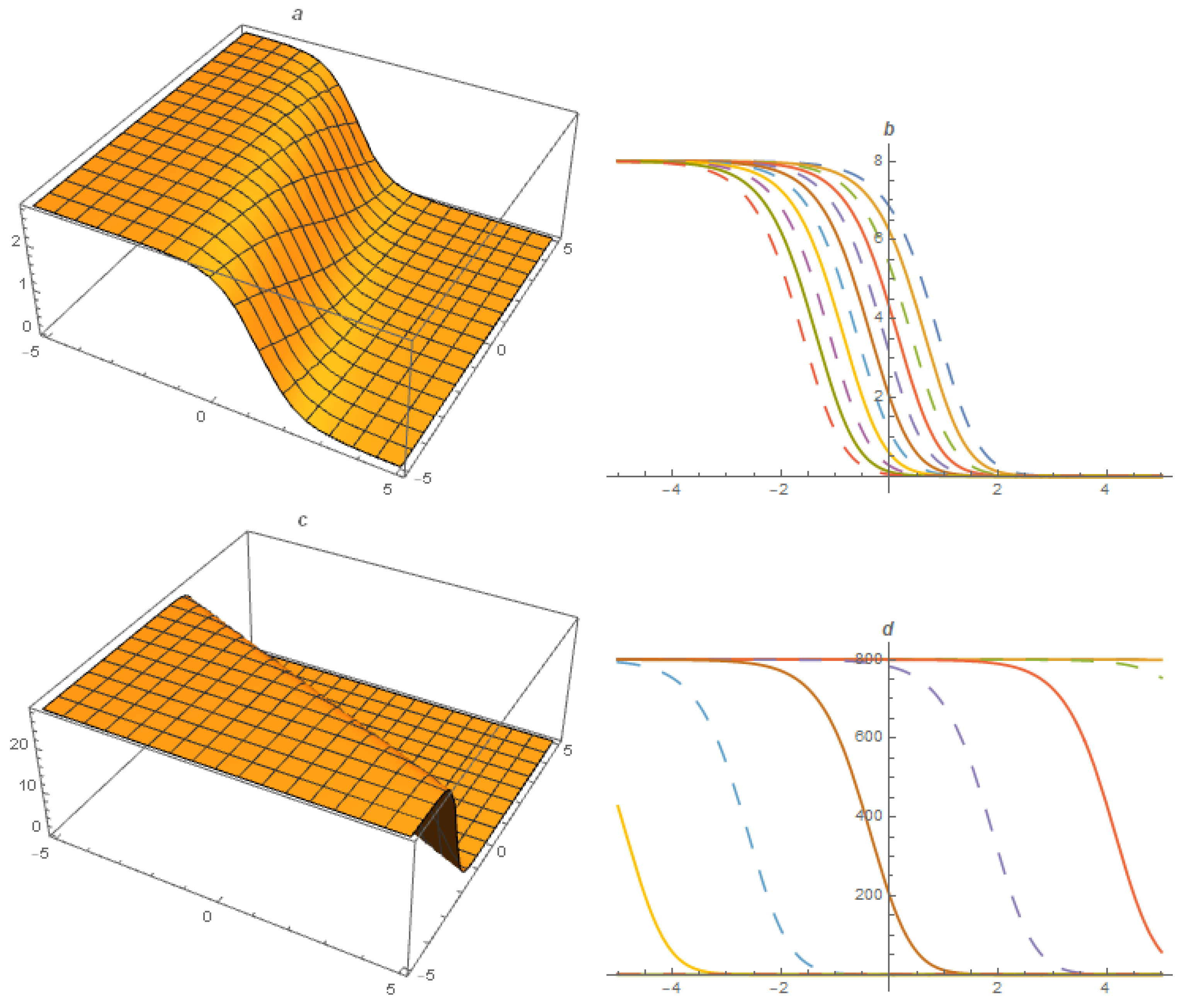
3.1. Application of Modified Extended Auxiliary Equation Mapping Method
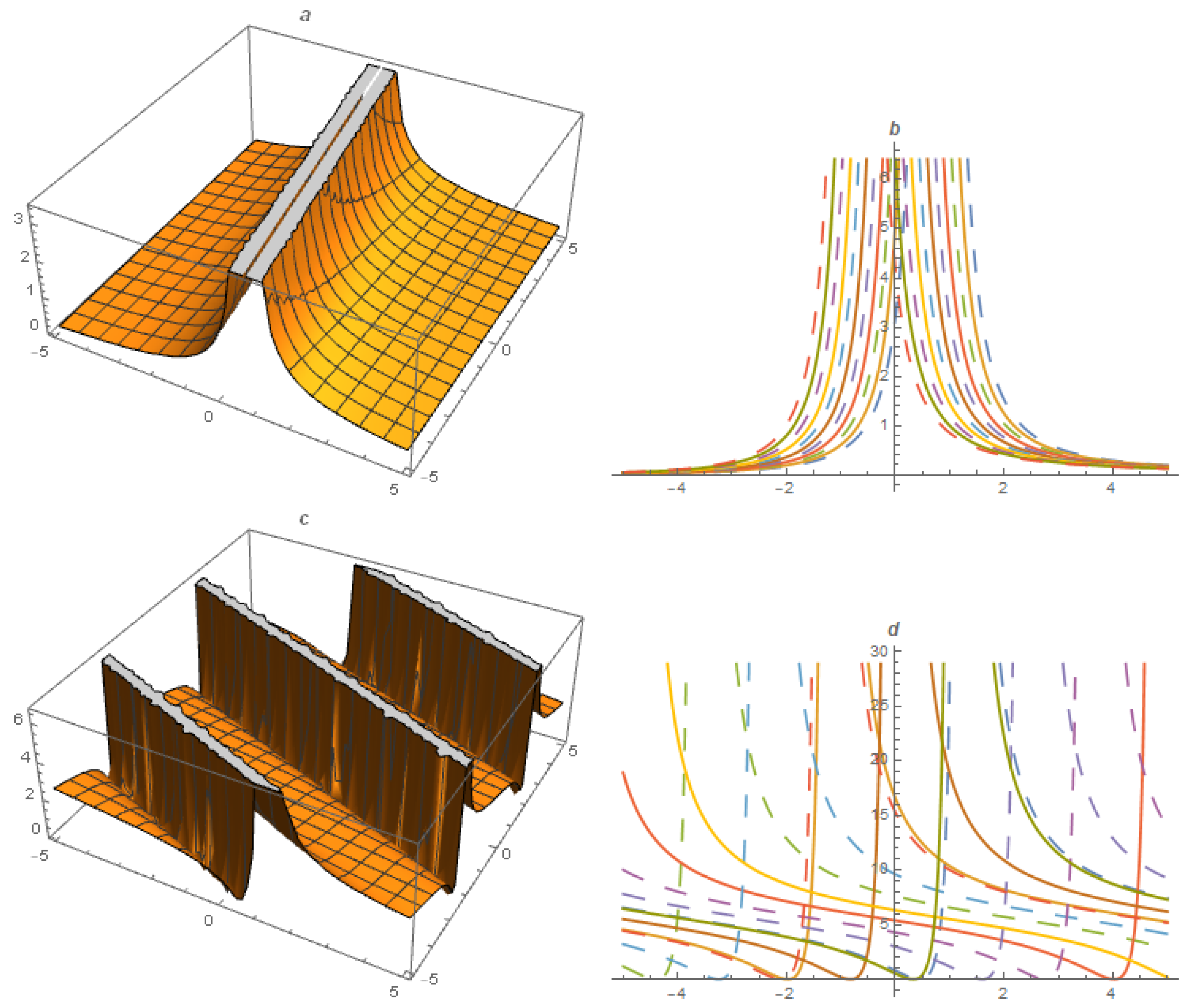
3.2. Application of Extended Simple Equation Method
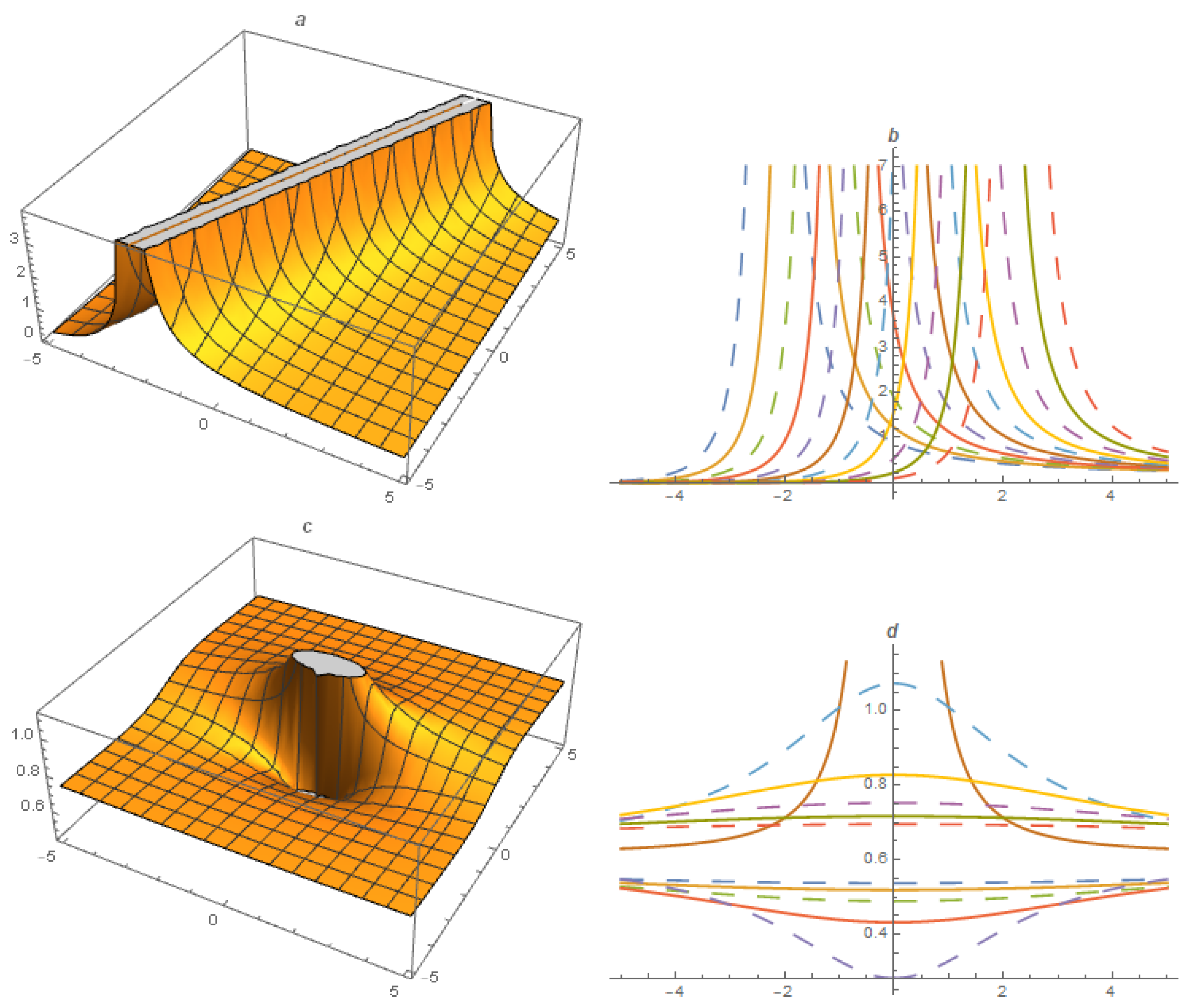
3.3. Application of Modified F-Expansion Method
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Wagner, J.P.; Schreiner, P.R. London dispersion in molecular chemistry-reconsidering steric effects. Angew. Chem. Int. Ed. 2015, 54, 12274. [Google Scholar] [CrossRef] [PubMed]
- Kaplan, G.; Menzio, G. The morphology of price dispersion. Int. Econ. Rev. 2015, 56, 1165. [Google Scholar] [CrossRef] [Green Version]
- Abbagari, S.; Houwe, A.; Saliou, Y.; Douvagaï; Chu, Y.M.; Inc, M.; Doka, S.Y. Analytical survey of the Predator-Prey model with fractional derivative order. AIP Adv. 2021, 11, 035127. [Google Scholar] [CrossRef]
- Dubey, B. A Prey-Predator Model with a Reserved Area. Nonlinear Anal. Model. Control 2007, 12, 479. [Google Scholar] [CrossRef] [Green Version]
- Nikan, O.; Avazzadeh, Z. Numerical simulation of fractional evolution model arising in viscoelastic mechanics. Appl. Numer. Math. 2021, 169, 303–320. [Google Scholar] [CrossRef]
- Oderinu, R.A.; Owolabi, J.A.; Taiwo, M. Approximate solutions of linear time-fractional differential equations. J. Math. Comput. Sci. 2023, 29, 60–72. [Google Scholar] [CrossRef]
- AlAhmad, R.; AlAhmad, Q.; Abdelhadi, A. Solution of fractional autonomous ordinary differential equations. J. Math. Comput. Sci. 2022, 27, 59–64. [Google Scholar] [CrossRef]
- Akram, T.; Abbas, M.; Ali, A. A numerical study on time fractional Fisher equation using an extended cubic B-spline approximation. J. Math. Comput. Sci. 2021, 22, 85–96. [Google Scholar] [CrossRef]
- Alphonse, H.; Jamilu, S.; Zakia, H.; Doka, S.Y. Solitary pulses of the conformable derivative nonlinear differential equation governing wave propagation in low-pass electrical transmission line. Phys. Scr. 2020, 95, 045203. [Google Scholar]
- Rizvi, S.T.; Seadawy, A.R.; Batool, T.; Ashraf, M.A. Homoclinic breaters, mulitwave, periodic cross-kink and periodic cross-rational solutions for improved perturbed nonlinear Schrödinger’s with quadratic-cubic nonlinearity. Chaos Solitons Fractals 2022, 161, 112353. [Google Scholar] [CrossRef]
- Shihua, C.; Fabio, B.; Jose, M.S.; Yi, L.; Philippe, G. Chirped Peregrine solitons in a class of cubic-quintic nonlinear Schrödinger equations. Phys. Rev. E 2016, 93, 062202. [Google Scholar]
- Sharma, V.K. Chirped soliton-like solutions of generalized nonlinear Schrödinger equation for pulse propagation in negative index material embedded into a Kerr medium. J. Phys. 2016, 90, 1271. [Google Scholar] [CrossRef]
- Goyal, A.; Gupta, R.; Kumar, C.N.; Raju, T.S. Chirped femtosecond solitons and double-kink solitons in the cubic-quintic nonlinear Schrödinger equation with self-steepening and self-frequency shift. Phys. Rev. A 2011, 84, 063830. [Google Scholar]
- Elsayed, M.E.; Mohamed, E.M.A. Application of newly proposed Sub-ODE method to locate chirped optical solutions to Triki-Biswas equation. Optik 2020, 207, 164360. [Google Scholar]
- He, J.; Xu, S.; Porsezian, K. Rogue waves of the FOKAS-LENELLS equation. J. Phys. Soc. Jpn. 2012, 81, 124007. [Google Scholar] [CrossRef] [Green Version]
- Alphonse, H.; Malwe, B.H.; Nestor, S.; Dikwa, J.; Mibaile, J.; Gambo, B.; Doka, S.Y.; Kofane, T.C.; Salam, K.; Biwas, A.; et al. Optical solitons for higher-order nonlinear Schrödingers equation with three exotic integration architectures. Optik 2019, 179, 861. [Google Scholar] [CrossRef]
- Min, X.; Yang, R.; Tian, J.; Xue, W.; Christian, J.M. Exact dipole solitary wave solution in metamaterials with higher-order dispersion. J. Mod. Opt. 2016, 63, S44. [Google Scholar] [CrossRef] [Green Version]
- Michael, S.; Maxim, S.S.; Neset, A.; Evgeni, Y.P.; Giuseppe, D.; Nadia, M.; Mark, J.B.; Aleksei, M.Z. Generalized Nonlinear Schrödinger Equation for Dispersive Susceptibility and Permeability: Application to Negative Index Materials. Phys. Rev. Lett. 2005, 95, 013902. [Google Scholar]
- Korkmaz, A.; Hepson, E.; Hosseini, K.O.; Rezazadeh, H.; Eslami, M. Sine-Gordon expansion method for exact solutions to conformable time fractional equations in RLW-class. J. King Saud-Univ.-Sci. 2020, 32, 567. [Google Scholar] [CrossRef]
- Li, P.; Yang, R.; Xu, Z. Gray solitary-wave solutions in nonlinear negative-index materials. Phys. Rev. E 2010, 82, 046603. [Google Scholar] [CrossRef] [Green Version]
- Yakada, S.; Depelair, B.; Gambo, B.; Serge, Y.D. Miscellaneous new traveling waves in metamaterials by means of the new extended direct algebraic method. Optik 2019, 197, 163108. [Google Scholar] [CrossRef]
- Abbagari, S.; Alper, K.; Hadi, R.; Paulin, T.M.S.; Ahmet, B. Soliton solutions in different classes for the Kaup-Newell model equation. Mod. Phys. Lett. B 2020, 34, 2050038. [Google Scholar]
- Houwe, A.; Inc, M.; Doka, S.Y.; Akinlar, M.A.; Baleanu, D. Chirped solitons in negative index materials generated by Kerr nonlinearity. Results Phys. 2020, 17, 103097. [Google Scholar] [CrossRef]
- Kudryashov, N.A. One method for finding exact solutions of nonlinear differential equations. Commun. Nonlinear Sci. Numer. Simulat. 2012, 17, 2248. [Google Scholar] [CrossRef] [Green Version]
- Zayed, E.M.E.; Arnous, A.H. DNA Dynamics Studied Using the Homogenous Balance Method. Chin. Phys. Lett. 2012, 29, 080203. [Google Scholar] [CrossRef]
- Houwe, A.; Abbagari, S.; Salathiel, Y.; Inc, M.; Doka, S.Y.; Crepin, K.T.; Baleanu, D. Complex traveling-wave and solitons solutions to the Klein-Gordon-Zakharov equations. Results Phys. 2020, 17, 103127. [Google Scholar] [CrossRef]
- Kudryashov, N.A.; Ryabov, P.N.; Fedyanin, T.E.; Kutukov, A.A. Analytical and numerical solutions of the generalized dispersive Swift-Hohenberg equation. Phys. Lett. A 2013, 377, 753. [Google Scholar] [CrossRef]
- Kudryashov, N.A. Quasi-exact solutions of the dissipative Kuramoto-Sivashinsky equation. Appl. Math. Comput. 2013, 219, 9245. [Google Scholar] [CrossRef]
- Ryabov, P.N.; Sinelshchikov, D.I.; Kochanov, M.B. Exact solutions of the Kudryashov-Sinelshchikov equation using the multiple (G′/G)-expansion method. Appl. Math. Comput. 2011, 218, 3965. [Google Scholar]
- Houwe, A.; Abbagari, S.; Inc, M.; Betchewe, G.; Doka, S.Y.; Crépin, K.T.; Nisar, K.S. Chirped solitons in discrete electrical transmission line. Results Phys. 2020, 18, 103188. [Google Scholar] [CrossRef]
- Wang, M.; Li, X.; Zhang, J. The (G′/G)-expansion method and travelling wave solutions of nonlinear evolution equations in mathematical physics. Phys. Lett. A 2008, 372, 417. [Google Scholar] [CrossRef]
- Ismail, A.; Turgut, O. Analytic study on two nonlinear evolution equations by using the (G′/G′)-expansion method. Appl. Math. Comput. 2009, 209, 425. [Google Scholar]
- Nestor, S.; Nestor, G.B.; Inc, M.; Doka, S.Y. Exact traveling wave solutions to the higher-order nonlinear Schrödinger equation having Kerr nonlinearity form using two strategic integrations. Eur. Phys. J. Plus 2020, 135, 380. [Google Scholar] [CrossRef]
- Canabarro, A.; Santos, B.; Bernardo, B.D.; Moura, A.L.; Soares, W.C.; Lima, E.D.; Gléria, I.; Lyra, M.L. Modulation instability in noninstantaneous Kerr media with walk-off and cross-phase modulation for mixed group velocity dispersion regimes. Phys. Rev. A 2016, 93, 023834. [Google Scholar] [CrossRef]
- Nestor, S.; Abbagari, S.; Houwe, A.; Betchewe, G.; Doka, S.Y. Diverse chirped optical solitons and new complex traveling waves in nonlinear optical fibers. Commun. Theor. Phys. 2020, 72, 065501. [Google Scholar] [CrossRef]
- Nestor, S.; Houwe, A.; Rezazadeh, H.; Bekir, A.; Betchewe, G.; Doka, S.Y. New solitary waves for the Klein-Gordon-Zakharov equations. Mod. Phys. Lett. B 2020, 34, 2050246. [Google Scholar] [CrossRef]
- Nestor, S.; Houwe, A.; Betchewe, G.; Inc, M.; Doka, S.Y. A series of abundant new optical solitons to the conformable space-time fractional perturbed nonlinear Schrodinger equation. Phys. Scr. 2020, 95, 085108. [Google Scholar] [CrossRef]
- Nestor, S.; Betchewe, G.; Rezazadeh, H.; Bekir, A.; Doka, S.Y. Exact optical solitons to the perturbed nonlinear equation with Schrödinerh dual-power law of nonlinearity. Opt. Quantum Electron. 2020, 52, 318. [Google Scholar]
- Rezazadeh, H.; Souleymanou, A.; Korkmaz, A.; Khater, M.M.A.; Mukam, S.P.T.; Kuetche, V.K. New exact solitary waves solutions to the fractional Fokas-Lenells equation via Atangana-Baleanu derivative operator. Mod. Phys. Lett. B 2020, 34, 2050309. [Google Scholar] [CrossRef]
- Souleymanou, A.; Ali, K.K.; Rezazadeh, H.; Eslami, M.; Mirzazadeh, M.; Korkmaz, A. The propagation of waves in thin-film ferroelectric materials. Pramana-J. Phys. 2019, 93, 27. [Google Scholar] [CrossRef]
- Souleymanou, A.; Kuetche, V.K.; Bouetou, T.B.; Kofane, T.C. Scattering Behavior of Waveguide Channels of a New Coupled Integrable Dispersionless System. Chin. Phys. Lett. 2011, 28, 120501–120504. [Google Scholar] [CrossRef]
- Souleymanou, A.; Kuetche, V.K.; Bouetou, T.B.; Kofane, T.C. Traveling Wave-Guide Channels of a New Coupled Integrable Dispersionless System. Commun. Theor. Phys. 2012, 57, 10. [Google Scholar] [CrossRef]
- Souleymanou, A.; Youssoufa, S.; Tchokouansi, H.T.; Kuetche, V.K.; Bouetou, T.B.; Kofane, T.C. N-Rotating Loop-Soliton Solution of the Coupled Integrable Dispersionless Equation. J. Appl. Math. Phys. 2017, 5, 1370. [Google Scholar]
- Souleymanou, A.; Mukam, S.P.T.; Houwe, A.; Kuetche, V.K.; Inc, M.; Doka, S.Y.; Almohsen, B.; Bouetou, T.B. Controllable rational solutions in nonlinear optics fibers. Eur. Phys. J. Plus 2020, 135, 633. [Google Scholar]
- Yepez-Martinez, H.; Rezazadeh, H.; Souleymanou, A.; Mukam, S.P.T.; Eslami, M.; Kuetche, V.K.; Bekir, A. The Extended Modified Method Appliedto Optical Solitons solutions in Birefringent Fibers with weak nonlocal nonlinearity and four wave mixing. Chin. J. Phys. 2019, 58, 137. [Google Scholar] [CrossRef]
- Mukam, S.P.T.; Souleymanou, A.; Kuetche, V.K.; Bouetou, T.B. Rogue wave dynamics in barotropic relaxing media. Pramana J. Phys. 2018, 91, 56. [Google Scholar] [CrossRef]
- Inc, M.; Akgül, A.; Geng, F. Reproducing Kernel Hilbert Space Method for Solving Bratu’s Problem. Bull. Malays. Math. Sci. Soc. 2015, 38, 271. [Google Scholar] [CrossRef]
- Akgül, A.; Kilicman, A.; Inc, M. Improved (G′/G)-Expansion Method for the Space and Time Fractional Foam Drainage and KdV Equations. Abstr. Appl. Anal. 2013, 2013, 414353. [Google Scholar] [CrossRef] [Green Version]
- Akgül, A.; Inc, M.; Karatas, E.; Baleanu, D. Numerical solutions of fractional differential equations of Lane-Emden type by an accurate technique. Adv. Differ. Equ. 2015, 2015, 220. [Google Scholar] [CrossRef] [Green Version]
- Akgül, A. A novel method for a fractional derivative with non-local and non-singular kernel. Chaos Solitons Fractals 2018, 2014, 478. [Google Scholar] [CrossRef]
- Baleanu, D.; Fernandez, A.; Akgül, A. On a Fractional Operator Combining Proportional and Classical Differintegrals. Mathematics 2020, 8, 360. [Google Scholar] [CrossRef]
- Seadawy, A.R.; Ali, A.; Helal, M.A. Analytical wave solutions of the (2+1)-dimensional Boiti-Leon-Pempinelli and Boiti-Leon-Manna-Pempinelli equations by mathematical methods. Math. Methods Appl. Sci. 2021, 44, 14292–14315. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Areshi, M.; Seadawy, A.R.; Ali, A.; Alharbi, A.F.; Aljohani, A.F. Analytical Solutions of the Predator–Prey Model with Fractional Derivative Order via Applications of Three Modified Mathematical Methods. Fractal Fract. 2023, 7, 128. https://doi.org/10.3390/fractalfract7020128
Areshi M, Seadawy AR, Ali A, Alharbi AF, Aljohani AF. Analytical Solutions of the Predator–Prey Model with Fractional Derivative Order via Applications of Three Modified Mathematical Methods. Fractal and Fractional. 2023; 7(2):128. https://doi.org/10.3390/fractalfract7020128
Chicago/Turabian StyleAreshi, Mounirah, Aly R. Seadawy, Asghar Ali, Amal F. Alharbi, and Abdulrahman F. Aljohani. 2023. "Analytical Solutions of the Predator–Prey Model with Fractional Derivative Order via Applications of Three Modified Mathematical Methods" Fractal and Fractional 7, no. 2: 128. https://doi.org/10.3390/fractalfract7020128
APA StyleAreshi, M., Seadawy, A. R., Ali, A., Alharbi, A. F., & Aljohani, A. F. (2023). Analytical Solutions of the Predator–Prey Model with Fractional Derivative Order via Applications of Three Modified Mathematical Methods. Fractal and Fractional, 7(2), 128. https://doi.org/10.3390/fractalfract7020128