RBF-Based Local Meshless Method for Fractional Diffusion Equations
Abstract
:1. Introduction
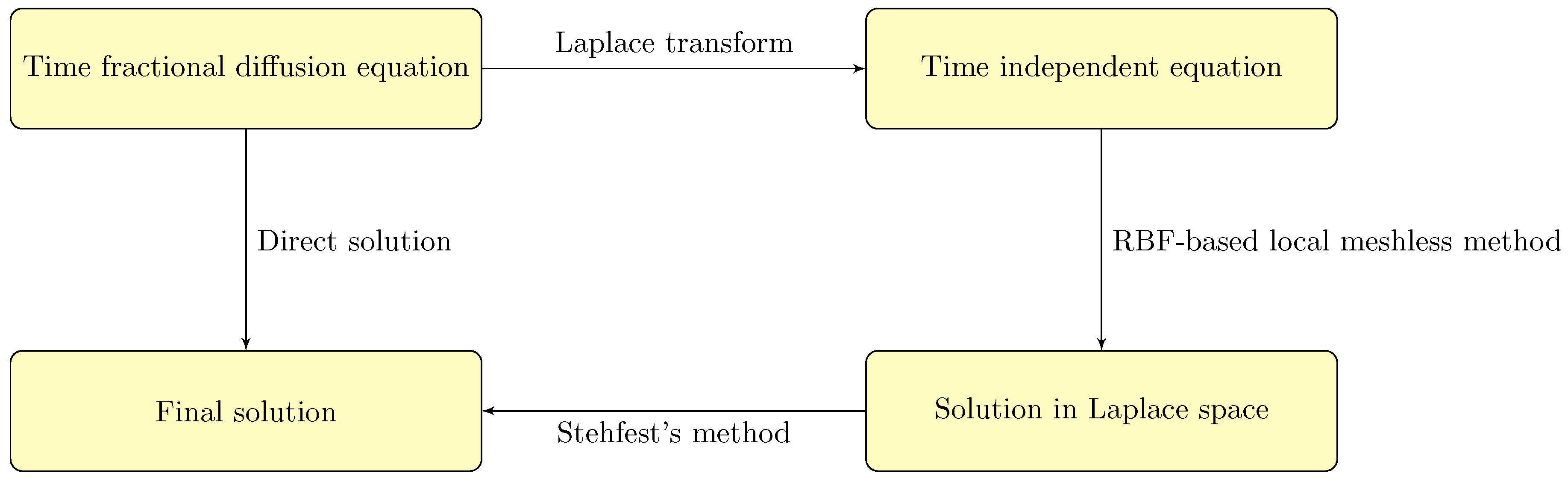
2. Proposed Method
2.1. Time-Fractional Diffusion Equation
2.2. Implementation of the Proposed Method
2.2.1. (i) Laplace Transform
2.2.2. (ii) RBF-Based Local Meshless Method
2.2.3. Selection of Best Shape Parameter
| Algorithm 1 Optimal Shape Parameter. |
| Step 1: set Step 2: select Step 3: while and Step 4: Construct the matrix Step 4: Step 5: Step 6: if Step 7: if (optimal) = . |
2.2.4. (iii) Numerical Approximation of Inverse Laplace Transform via Stehfest’s Method
2.3. Error Analysis
- 1.
- The convergence of depends only on the values of in the neighborhood of t.
- 2.
- Assume that for some and someThen as
- 3.
- Assume that the function has bounded variation in the neighborhood of Then
3. Stability
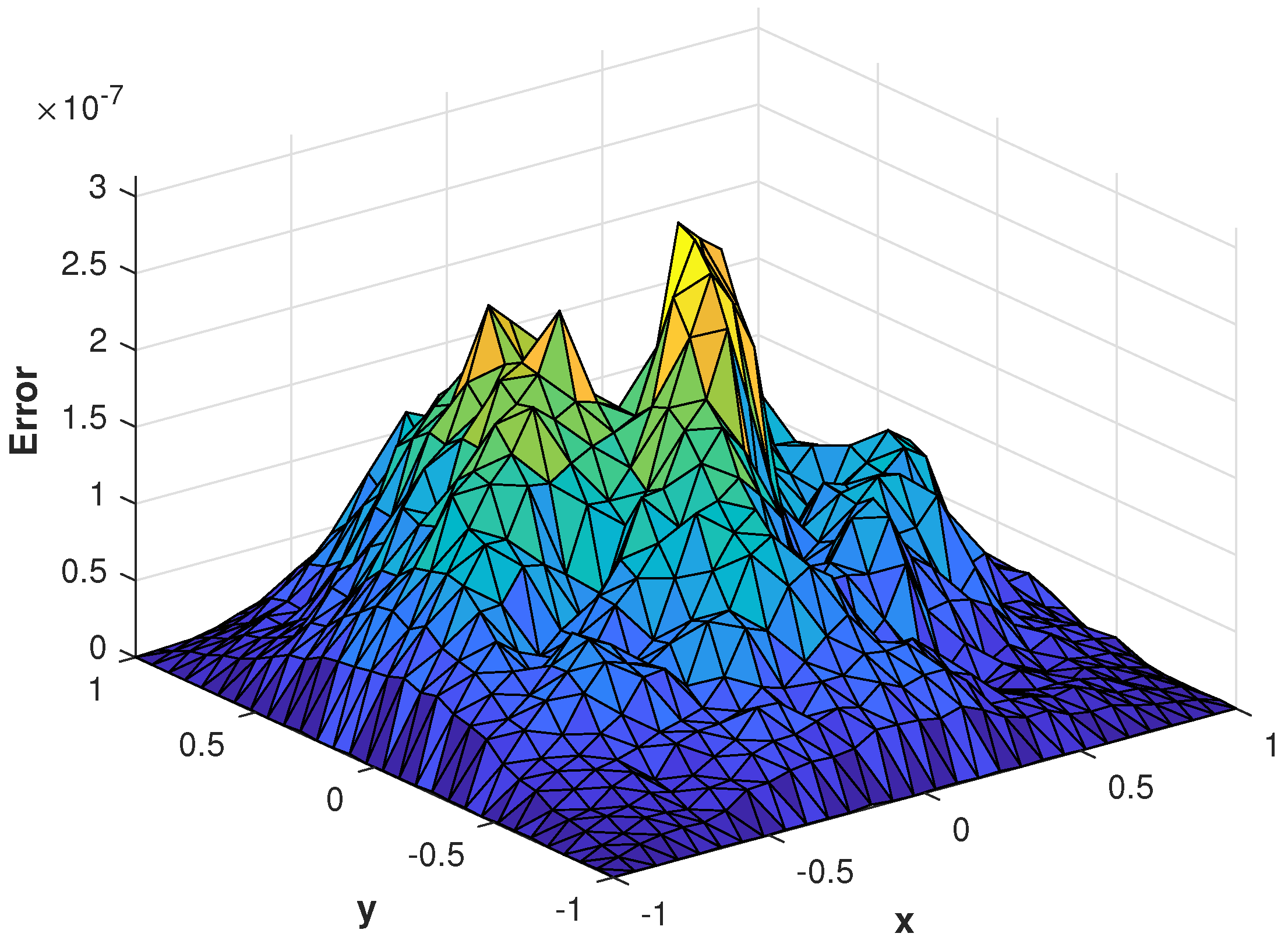
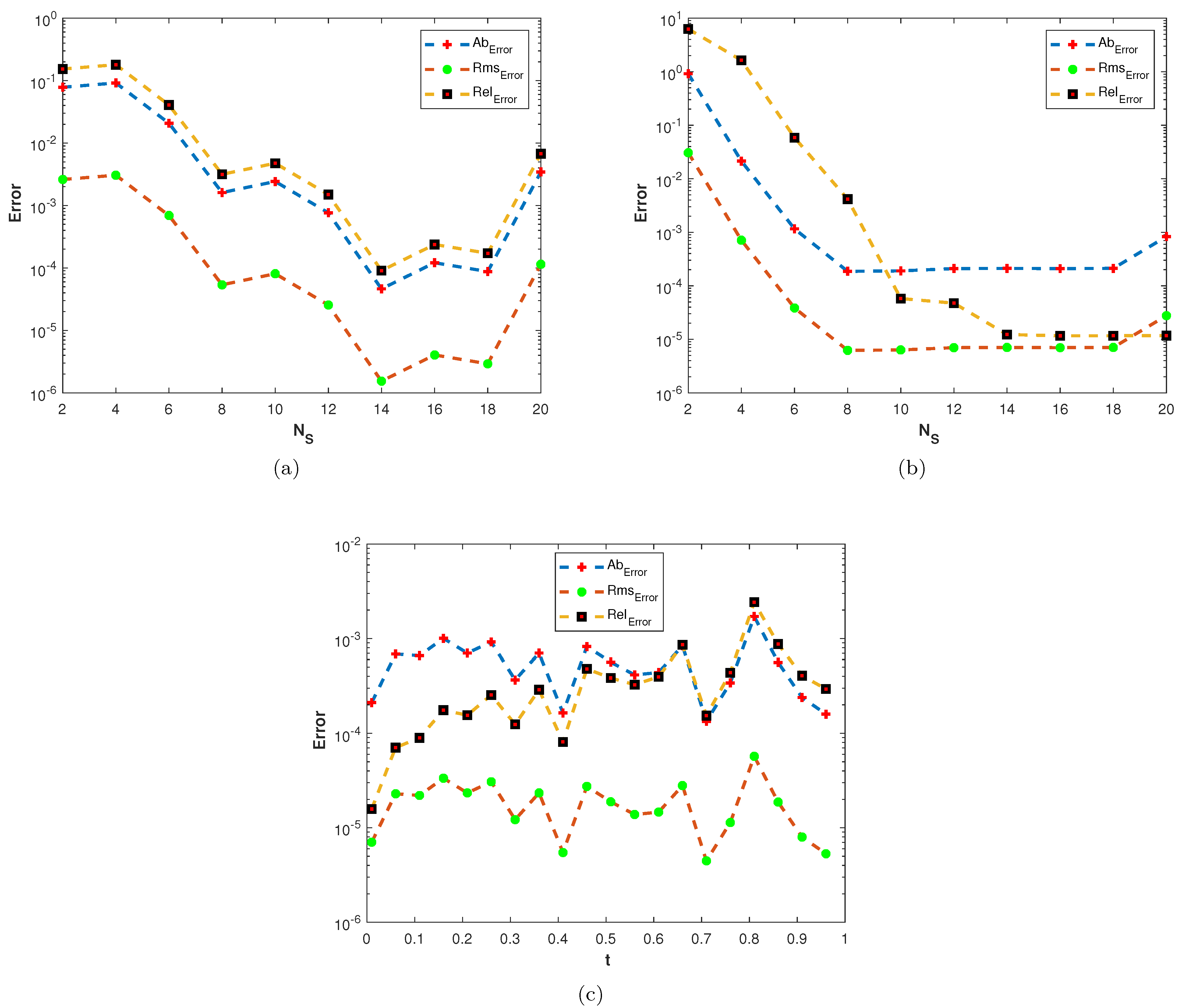
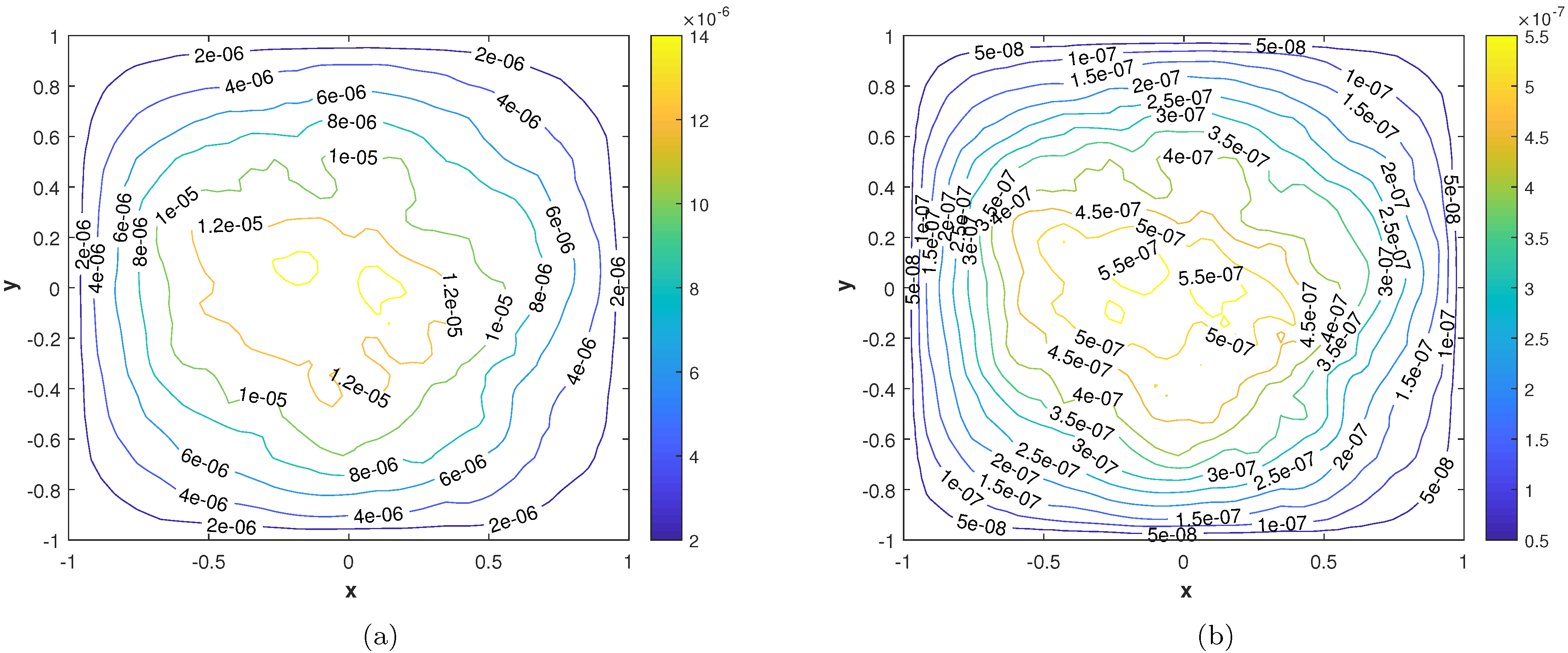
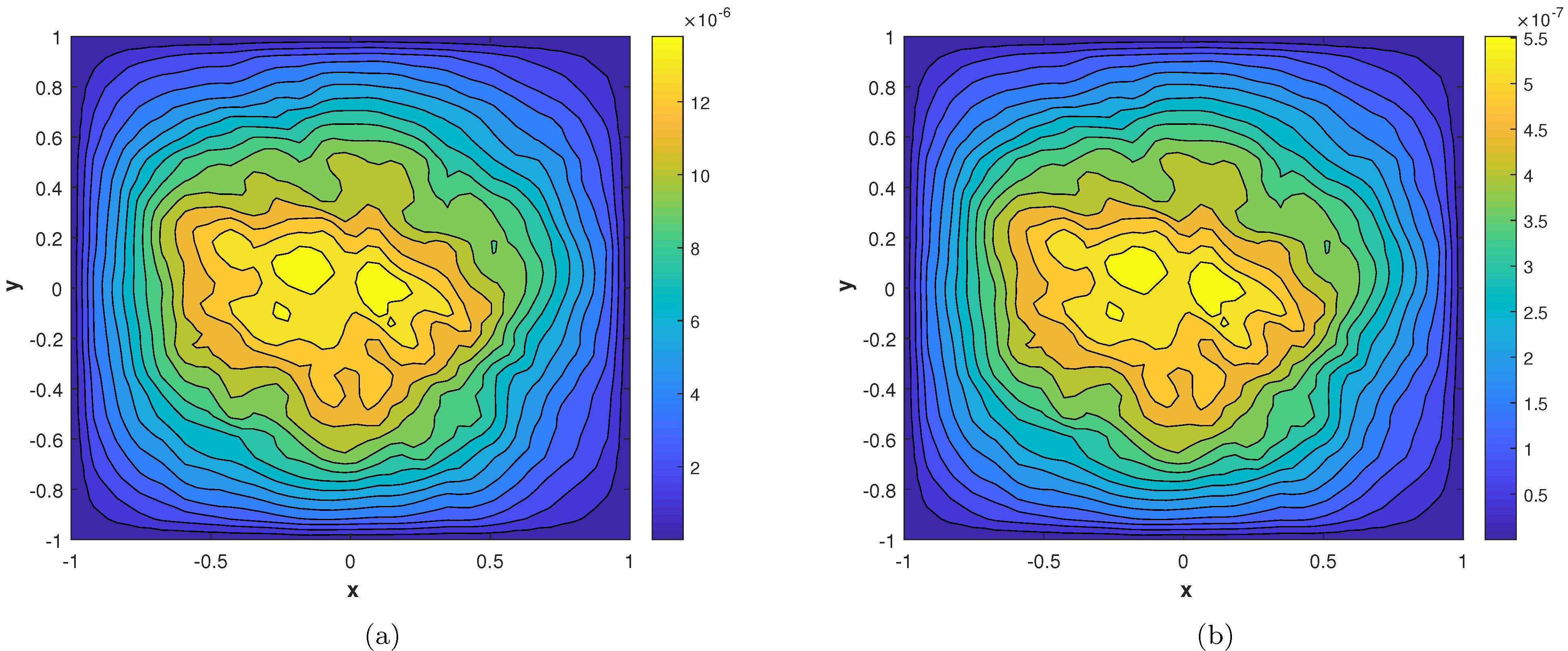
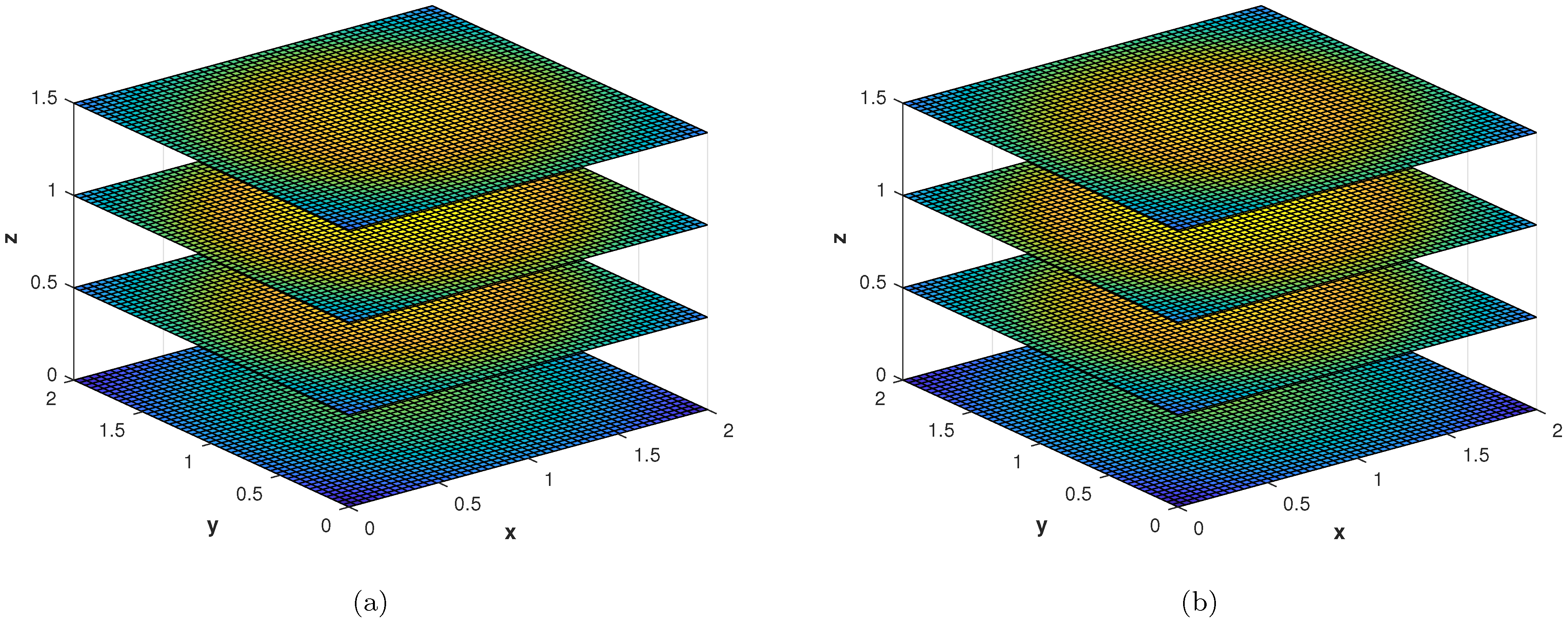
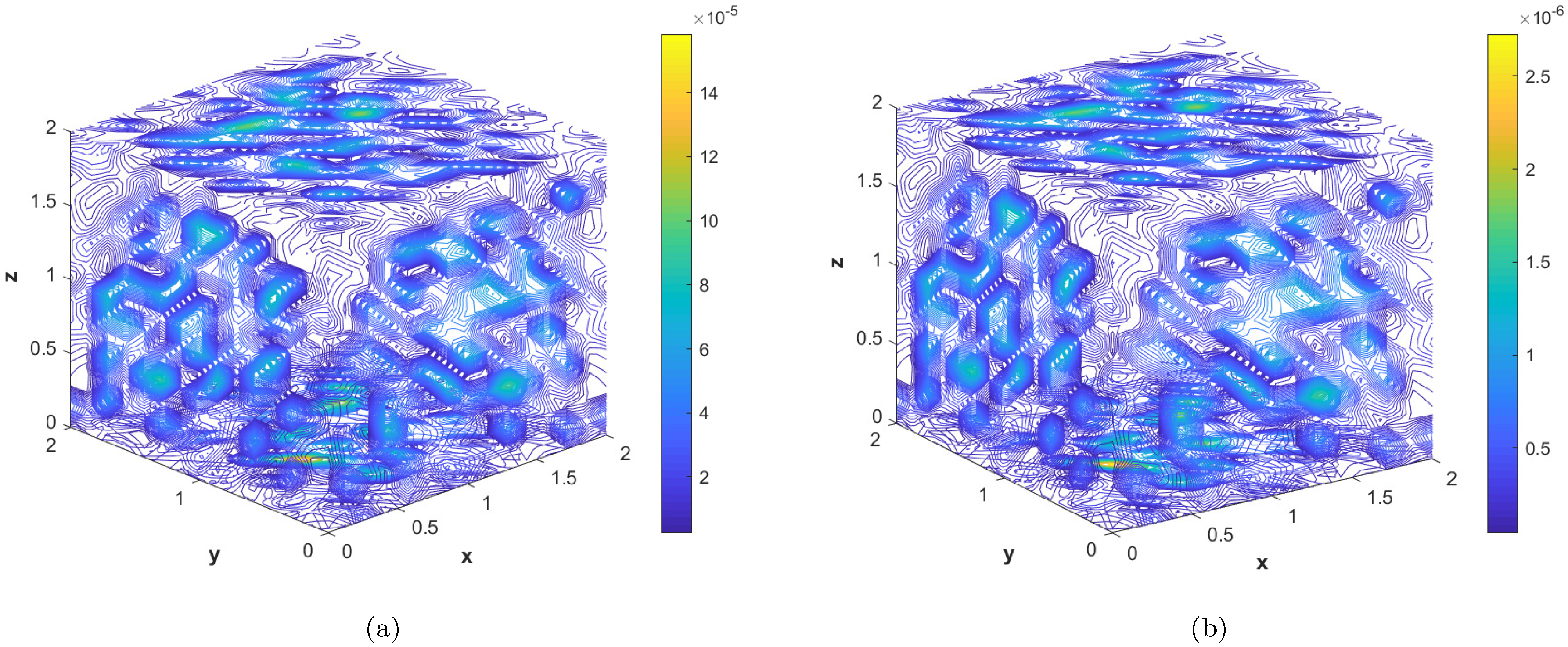
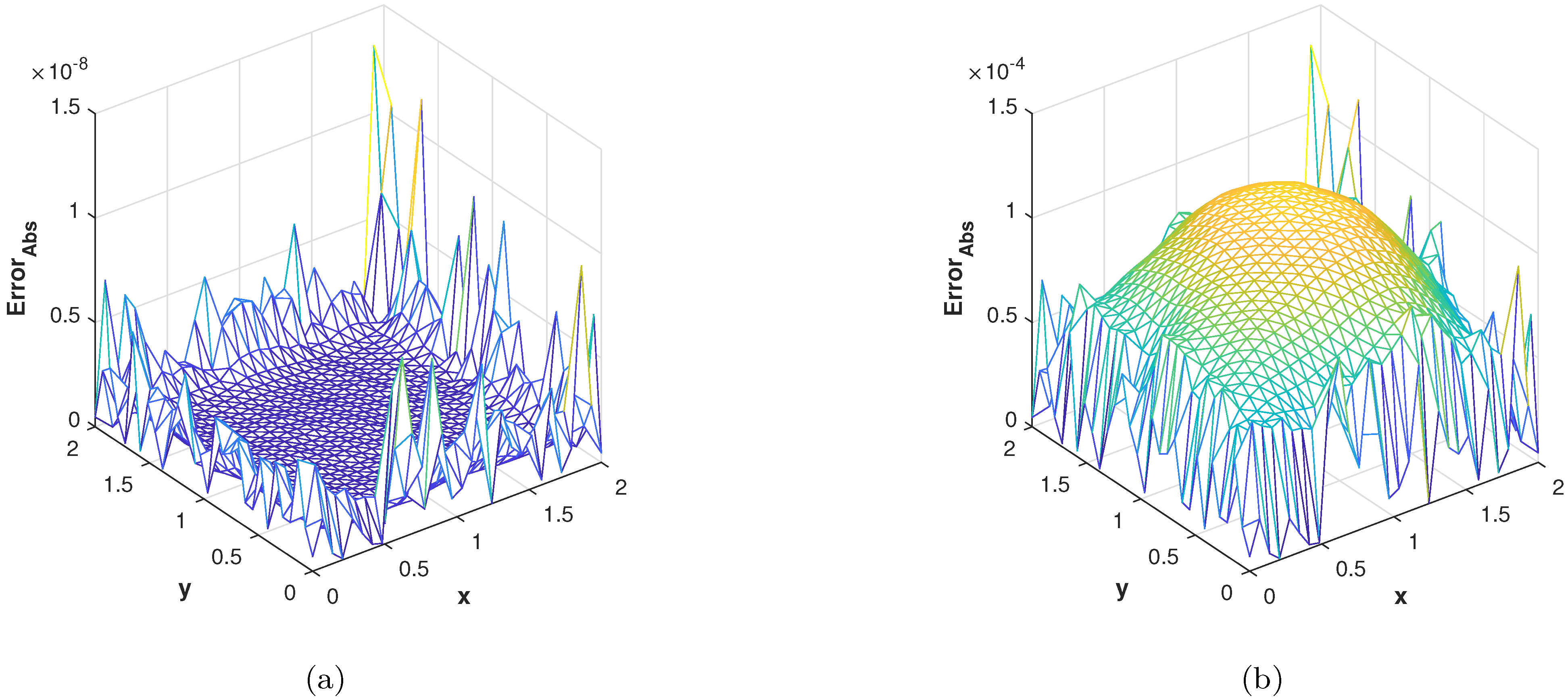
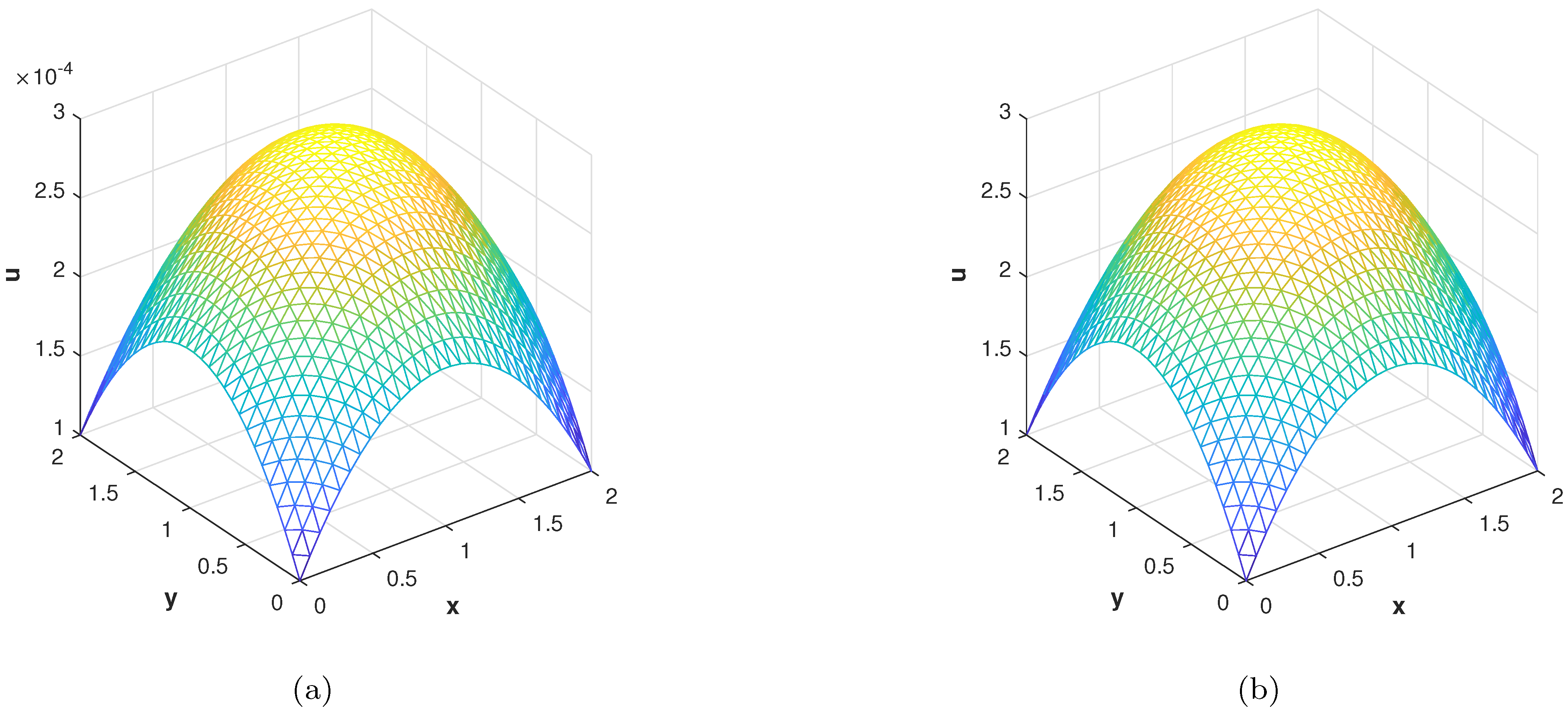
4. Numerical Results and Discussion
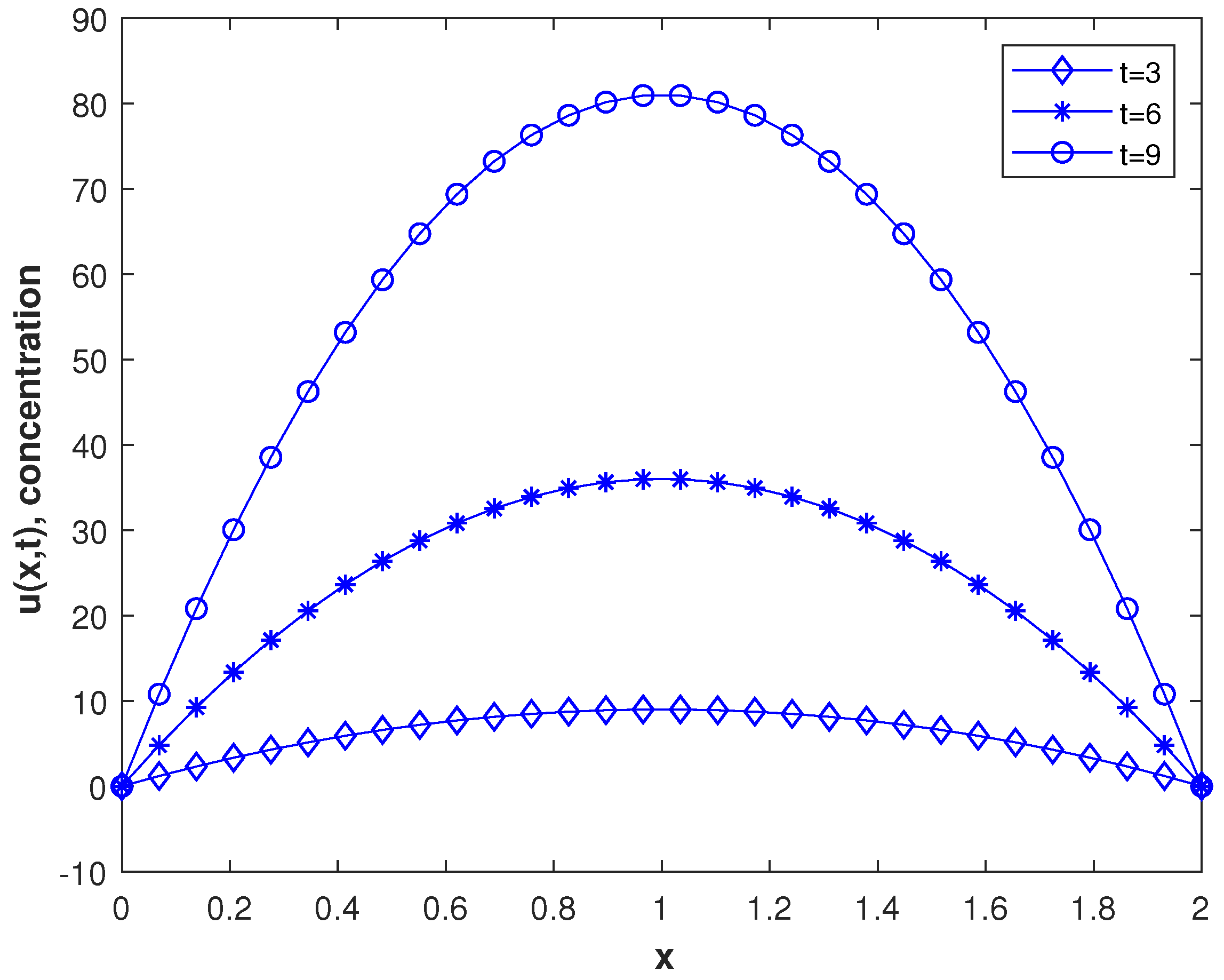
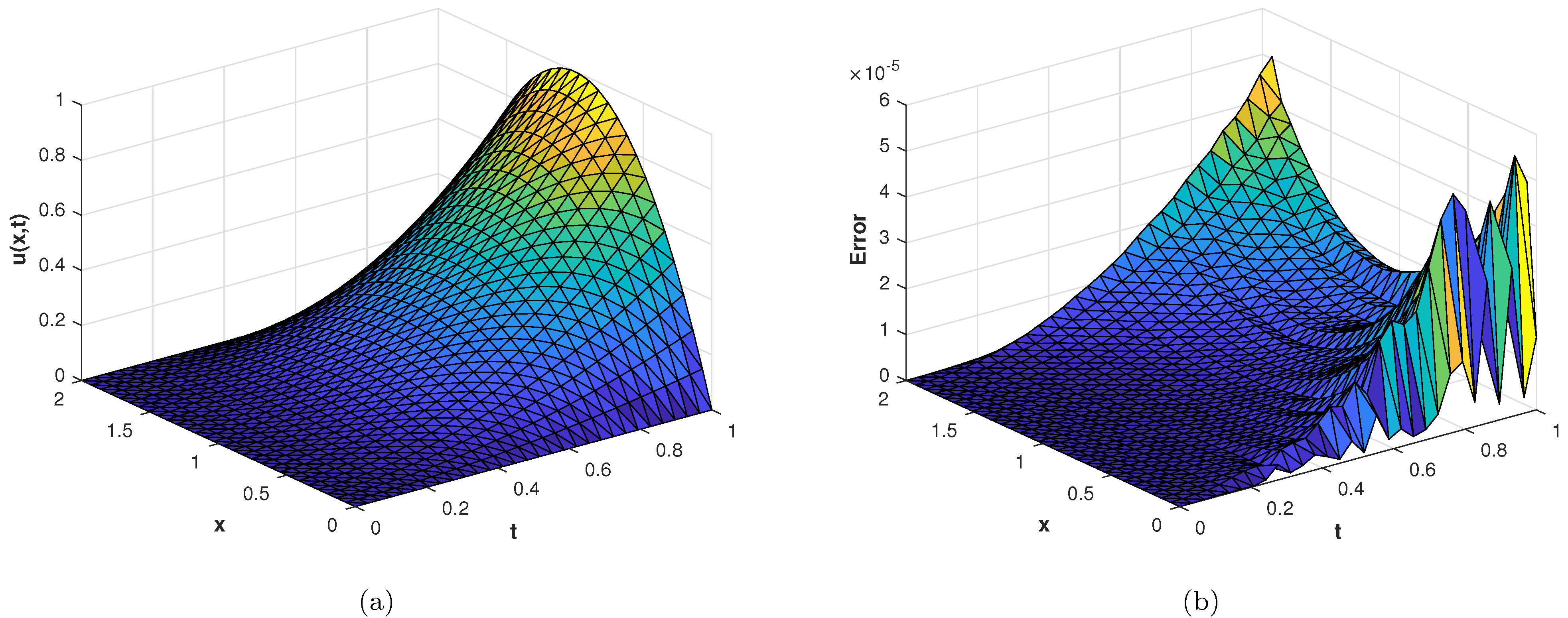
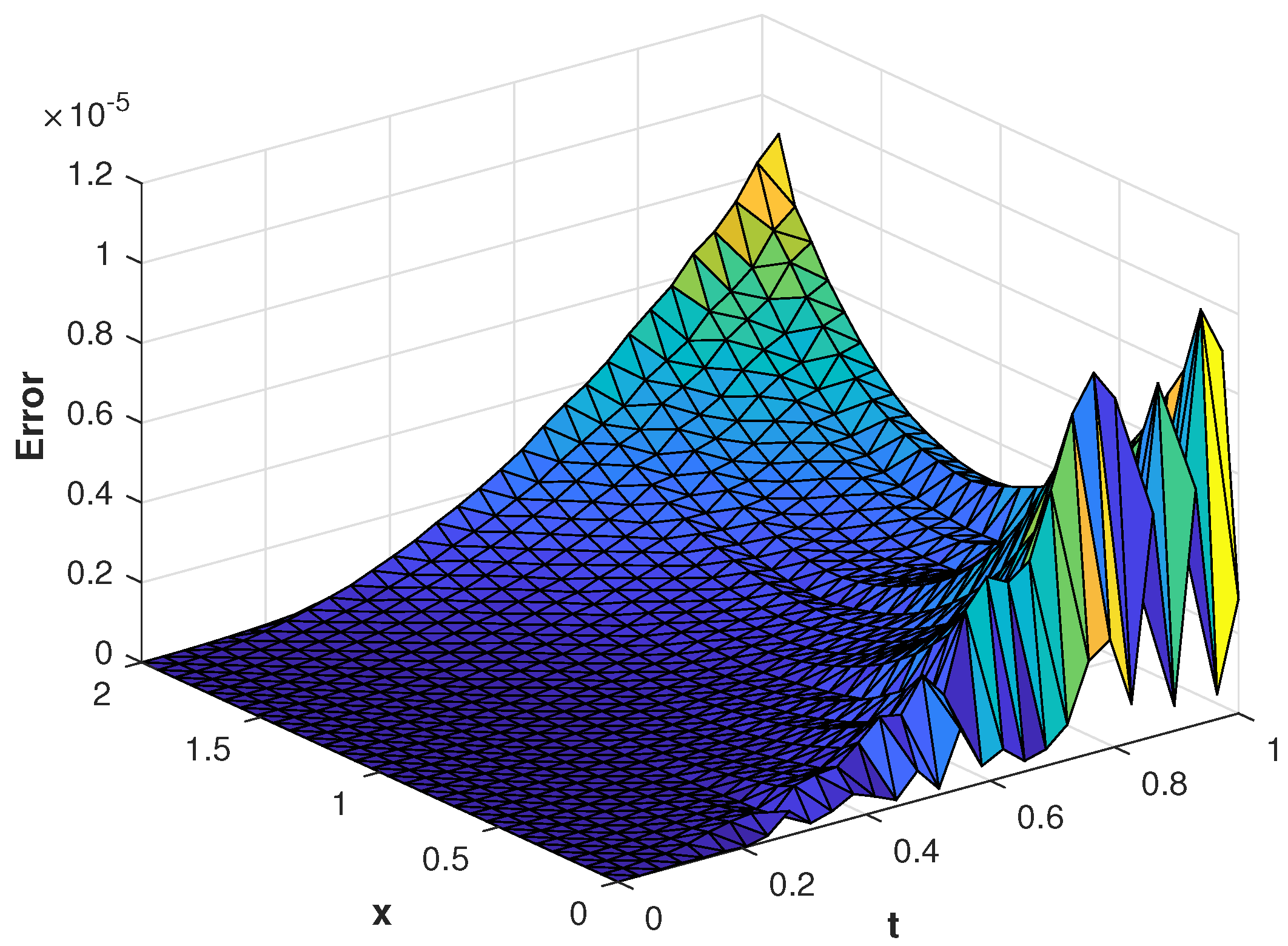
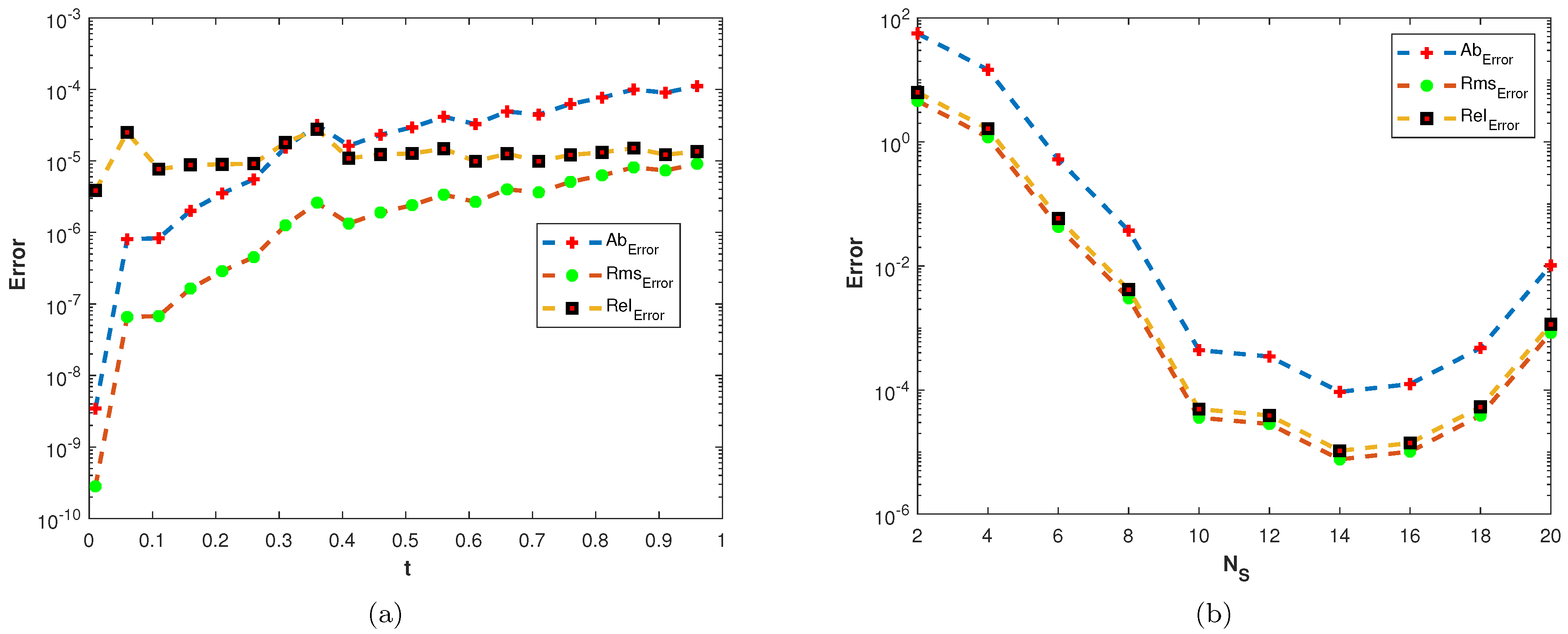
4.1. Problem 1
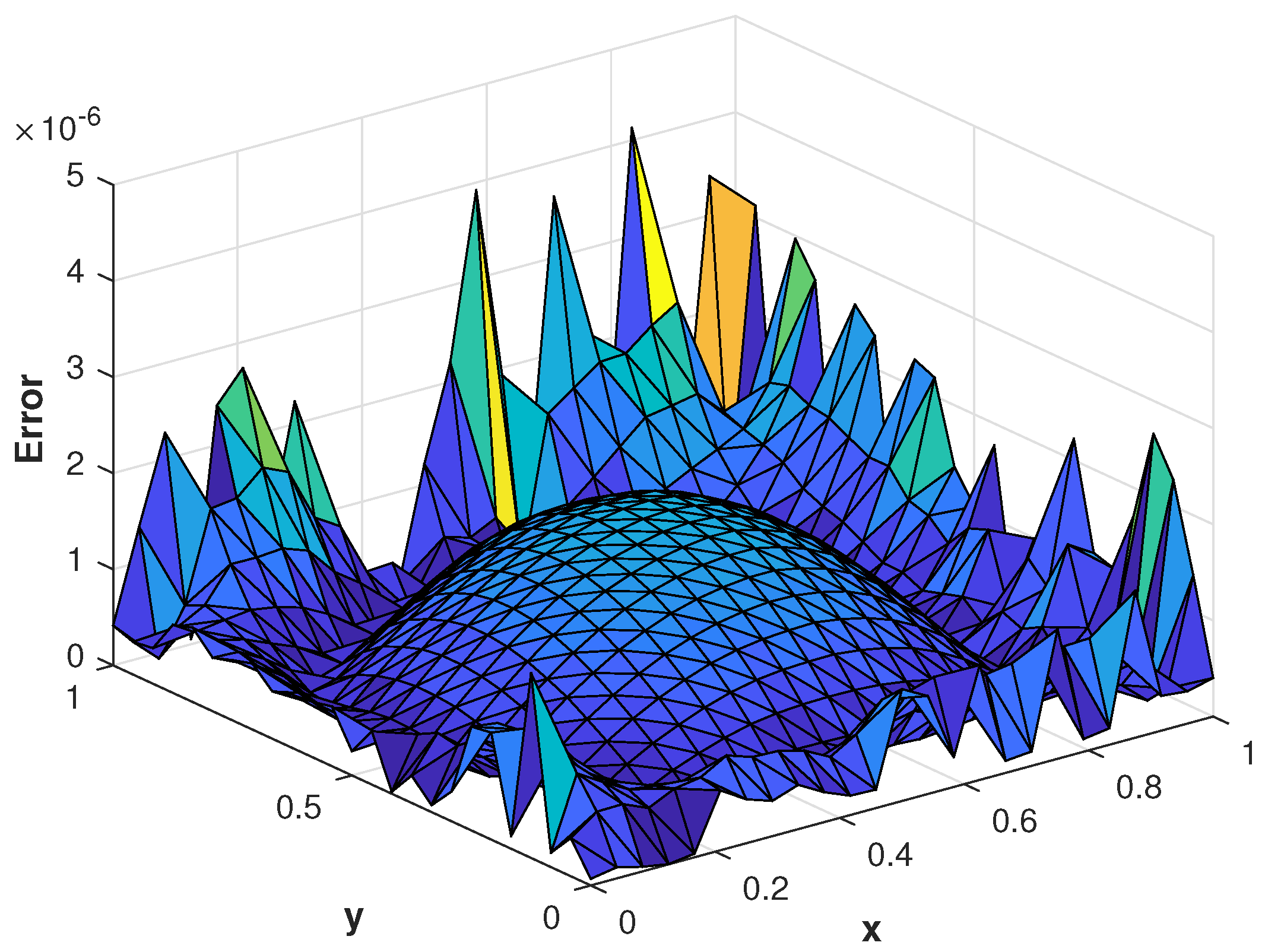
4.2. Problem 2
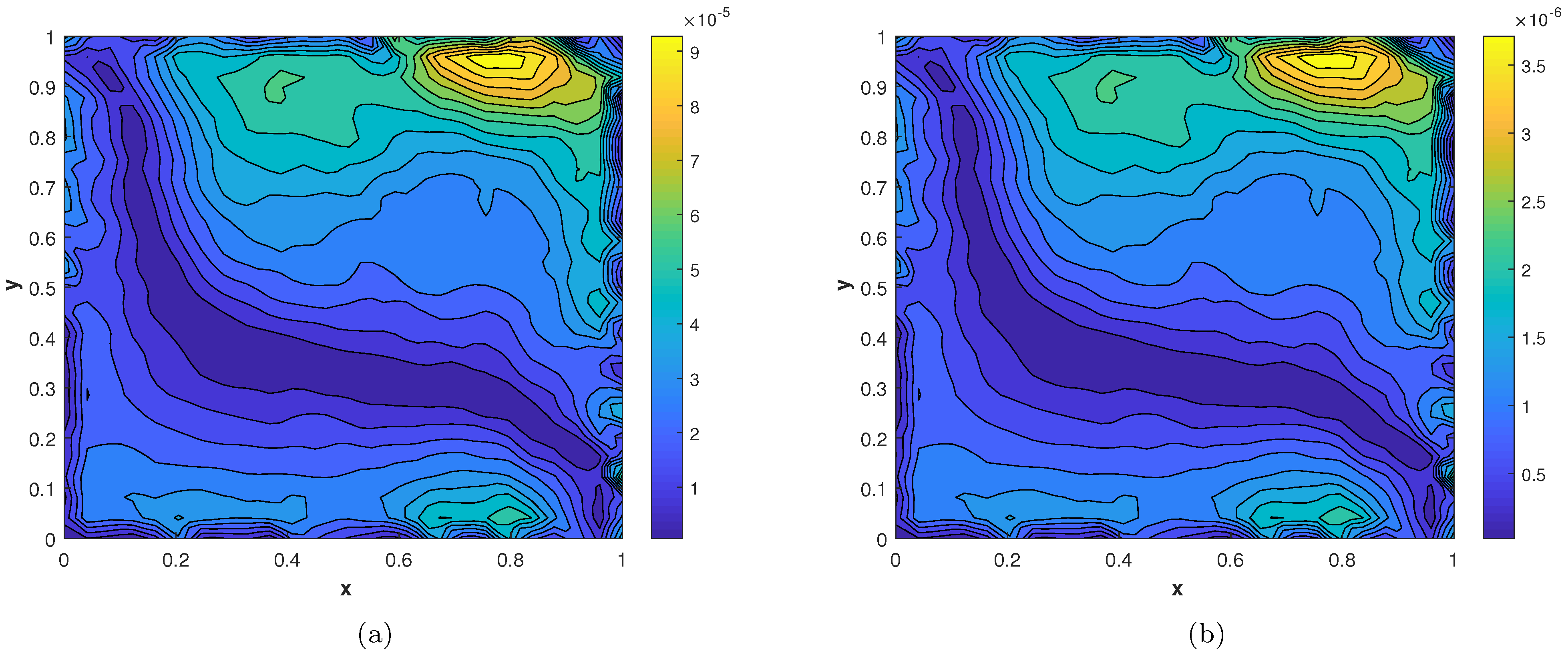
4.3. Problem 3
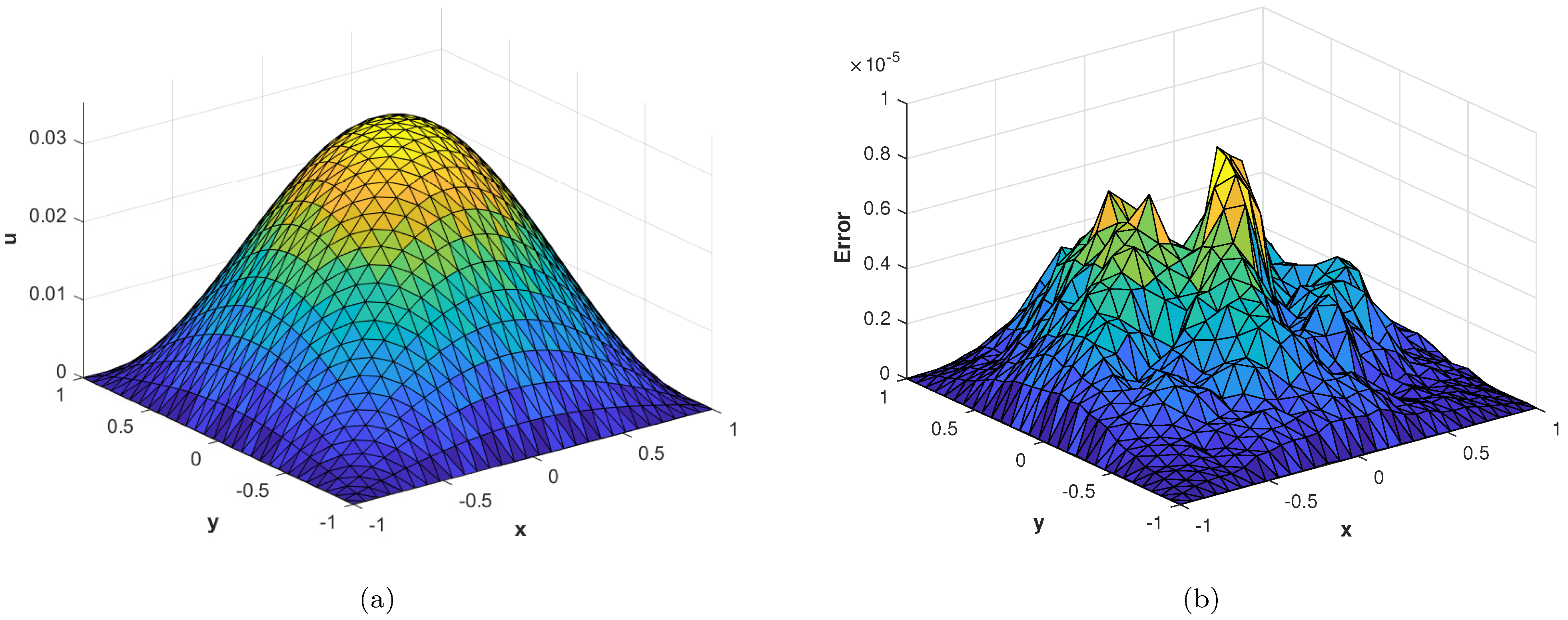
4.4. Problem 4
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Podlubny, I. Fractional Differential Equations; Academic Press: San Diego, CA, USA, 1999. [Google Scholar]
- Miller, K.S.; Ross, B. An Introduction to the Fractional Calculus and Fractional Differential Equations; Wiley: Hoboken, NJ, USA, 1993. [Google Scholar]
- Samko, S.G.; Kilbas, A.A.; Marichev, O.I. Fractional Integrals and Derivatives (Theory and Applications); Gordon and Breach Science Publishers: Basel, Switzerland, 1993. [Google Scholar]
- Metzler, R.; Klafter, J. The random walk’s guide to anomalous diffusion: A fractional dynamics approach. Phys. Rep. 2000, 339, 1–77. [Google Scholar] [CrossRef]
- Hilfer, R. (Ed.) Applications of Fractional Calculus in Physics; World Scientific: Singapore, 2000. [Google Scholar]
- Gorenflo, R.; Mainardi, F.; Moretti, D.; Pagnini, G.; Paradisi, P. Discrete random walk models for space–time fractional diffusion. Chem. Phys. 2002, 284, 521–541. [Google Scholar] [CrossRef]
- Scher, H.; Montroll, E.W. Anomalous transit–time dispersion in amorphous solids. Phys. Rev. B 1975, 12, 2455. [Google Scholar] [CrossRef]
- Hristov, J. Magnetic field diffusion in ferromagnetic materials: Fractional calculus approaches. Int. J. Optim. Control. Theor. Appl. (IJOCTA) 2021, 11, 1–15. [Google Scholar] [CrossRef]
- Macías-Díaz, J.E. On a discrete model that dissipates the free energy of a time-space fractional generalized nonlinear parabolic equation. Appl. Numer. Math. 2022, 172, 215–223. [Google Scholar] [CrossRef]
- Shao, X.H.; Kang, C.B. A preconditioner based on sine transform for space fractional diffusion equations. Appl. Numer. Math. 2022, 178, 248–261. [Google Scholar] [CrossRef]
- Witten, T.A. Insights from soft condensed matter. Rev. Mod. Phys. 1999, 71, 367–373. [Google Scholar] [CrossRef]
- Chang, F.X.; Chen, J.; Huang, W. Anomalous diffusion and fractional advection-diffusion equation. Acta Phys. Sin. 2005, 54, 1113–1117. [Google Scholar] [CrossRef]
- Wyss, W. The fractional diffusion equation. J. Math. Phys. 1986, 27, 2782–2785. [Google Scholar] [CrossRef]
- Meerschaert, M.M.; Tadjeran, C. Finite difference approximations for fractional advection-dispersion flow equations. J. Comput. Appl. Math. 2004, 172, 65–77. [Google Scholar] [CrossRef] [Green Version]
- Greenenko, A.A.; Chechkin, A.V.; Shul’ga, N.F. Anomalous diffusion and Lévy flights in channeling. Phys. Lett. A 2004, 324, 82–85. [Google Scholar] [CrossRef]
- Chen, W. Time-space fabric underlying anomalous diffusion. Chaos Solitons Fractals 2006, 28, 923–929. [Google Scholar] [CrossRef]
- Chen, W.; Sun, H.; Zhang, X.; Korošak, D. Anomalous diffusion modeling by fractal and fractional derivatives. Comput. Math. Appl. 2010, 59, 1754–1758. [Google Scholar] [CrossRef]
- Agrawal, O.P. Solution for a fractional diffusion-wave equation defined in a bounded domain. Nonlinear Dyn. 2002, 29, 145–155. [Google Scholar] [CrossRef]
- Bayrak, M.A.; Demir, A.; Ozbilge, E. Numerical solution of fractional diffusion equation by Chebyshev collocation method and residual power series method. Alex. Eng. J. 2020, 59, 4709–4717. [Google Scholar] [CrossRef]
- Sousa, E. Numerical approximations for fractional diffusion equations via splines. Comput. Math. Appl. 2011, 62, 938–944. [Google Scholar] [CrossRef]
- Li, C.; Zhao, Z.; Chen, Y. Numerical approximation of nonlinear fractional differential equations with subdiffusion and superdiffusion. Comput. Math. Appl. 2011, 62, 855–875. [Google Scholar] [CrossRef]
- Zheng, X.; Wang, H. Analysis and numerical approximation to time-fractional diffusion equation with a general time-dependent variable order. Nonlinear Dyn. 2021, 104, 4203–4219. [Google Scholar] [CrossRef]
- Bouchama, K.; Arioua, Y.; Merzougui, A. The numerical solution of the space-time fractional diffusion equation involving the Caputo-Katugampola fractional derivative. Numer. Algebra Control Optim. 2022, 12, 621. [Google Scholar] [CrossRef]
- Tadjeran, C.; Meerschaert, M.M.; Scheffler, H.P. A second-order accurate numerical approximation for the fractional diffusion equation. J. Comput. Phys. 2006, 213, 205–213. [Google Scholar] [CrossRef]
- Liu, F.; Anh, V.; Turner, I.; Zhuang, P. Time fractional advection dispersion equation. J. Appl. Math. Comput. 2003, 13, 233–245. [Google Scholar] [CrossRef]
- Lin, Y.; Xu, C. Finite difference/spectral approximations for the time-fractional diffusion equation. J. Comput. Phys. 2005, 225, 1533–1552. [Google Scholar] [CrossRef]
- Assari, P.; Dehghan, M. Application of dual-Chebyshev wavelets for the numerical solution of boundary integral equations with logarithmic singular kernels. Eng. Comput. 2019, 35, 175–190. [Google Scholar] [CrossRef]
- Assari, P.; Adibi, H.; Dehghan, M. A Meshless Discrete Galerkin (mdg) Method for the Numerical Solution of Integral Equations with Logarithmic Kernels. J. Comput. Appl. Math. 2014, 267, 160–181. [Google Scholar] [CrossRef]
- Dehghan, M.; Salehi, R. A meshless local Petrov-Galerkin method for the time-dependent Maxwell equations. J. Comput. Appl. Math. 2014, 268, 93–110. [Google Scholar] [CrossRef]
- Dehghan, M.; Salehi, R. The numerical solution of the non-linear integro-differential equations based on the meshless method. J. Comput. Appl. Math. 2012, 236, 2367–2377. [Google Scholar] [CrossRef]
- Sarra, S.A.; Kansa, E.J. Multiquadric radial basis function approximation methods for the numerical solution of partial differential equations. Adv. Comput. Mech. 2009, 2, 220. [Google Scholar]
- Lee, C.K.; Liu, X.; Fan, S.C. Local multiquadric approximation for solving boundary value problems. Comput. Mech. 2003, 30, 396–409. [Google Scholar] [CrossRef]
- Jackson, S.J.; Stevens, D.; Giddings, D.; Power, H. An adaptive RBF finite collocation approach to track transport processes across moving fronts. Comput. Math. Appl. 2016, 71, 278–300. [Google Scholar] [CrossRef]
- Naffa, M.; Al-Gahtani, H.J. RBF-based meshless method for large deflection of thin plates. Eng. Anal. Bound. Elem. 2007, 31, 311–317. [Google Scholar] [CrossRef]
- Leitao, V.M.A. RBF-based meshless methods for 2D elastostatic problems. Eng. Anal. Bound. Elem. 2004, 28, 1271–1281. [Google Scholar] [CrossRef]
- Dehghan, M.; Shirzadi, M. Meshless simulation of stochastic advection-diffusion equations based on radial basis functions. Eng. Anal. Bound. Elem. 2015, 53, 18–26. [Google Scholar] [CrossRef]
- Šarler, B.; Vertnik, R. Meshfree explicit local radial basis function collocation method for diffusion problems. Comput. Math. Appl. 2006, 51, 1269–1282. [Google Scholar] [CrossRef]
- Dehghan, M.; Abbaszadeh, M. Numerical investigation based on direct meshless local Petrov Galerkin (direct MLPG) method for solving generalized Zakharov system in one and two dimensions and generalized Gross-Pitaevskii equation. Eng. Comput. 2017, 33, 983–996. [Google Scholar] [CrossRef]
- Vertnik, R.; Šarler, B. Meshless local radial basis function collocation method for convective-diffusive solid-liquid phase change problems. Int. J. Numer. Methods Heat Fluid Flow 2006, 16, 617–640. [Google Scholar] [CrossRef]
- Jiwari, R.; Singh, S.; Singh, P. Local RBF-FD-Based Mesh-free Scheme for Singularly Perturbed Convection-Diffusion-Reaction Models with Variable Coefficients. J. Math. 2022, 2022, 3119482. [Google Scholar] [CrossRef]
- Kosec, G.; Šarler, B. Local RBF collocation method for Darcy flow. Comput. Model. Eng. Sci. 2008, 25, 197. [Google Scholar]
- Yao, G.; Islam, S.; Šarler, B. Assessment of global and local meshless methods based on collocation with radial basis functions for parabolic partial differential equations in three dimensions. Eng. Anal. Bound. Elem. 2012, 36, 1640–1648. [Google Scholar] [CrossRef]
- Kamran; Khan, S.; Alhazmi, S.E.; Alotaibi, F.M.; Ferrara, M.; Ahmadian, A. On the Numerical Approximation of Mobile-Immobile Advection-Dispersion Model of Fractional Order Arising from Solute Transport in Porous Media. Fractal Fract. 2022, 6, 445. [Google Scholar] [CrossRef]
- Davies, A.J.; Crann, D.; Kane, S.J.; Lai, C.H. A hybrid Laplace transform/finite difference boundary element method for diffusion problems. Comput. Model. Eng. Sci. 2007, 18, 79–86. [Google Scholar]
- Rizzo, F.J.; Shippy, D.J. A method of solution for certain problems of transient heat conduction. AIAA J. 1970, 8, 2004–2009. [Google Scholar] [CrossRef]
- Dubner, H.; Abate, J. Numerical inversion of Laplace transforms by relating them to the finite Fourier cosine transform. J. ACM (JACM) 1968, 15, 115–123. [Google Scholar] [CrossRef]
- Durbin, F. Numerical inversion of Laplace transforms: An efficient improvement to Dubner and Abate’s method. Comput. J. 1974, 17, 371–376. [Google Scholar] [CrossRef] [Green Version]
- Crump, K.S. Numerical inversion of Laplace transforms using a Fourier series approximation. J. ACM (JACM) 1976, 23, 89–96. [Google Scholar] [CrossRef]
- De Hoog, F.R.; Knight, J.H.; Stokes, A.N. An improved method for numerical inversion of Laplace transforms. SIAM J. Sci. Stat. Comput. 1982, 3, 357–366. [Google Scholar] [CrossRef]
- Weeks, W.T. Numerical inversion of Laplace transforms using Laguerre functions. J. ACM (JACM) 1966, 13, 419–429. [Google Scholar] [CrossRef]
- Talbot, A. The accurate numerical inversion of Laplace transforms. IMA J. Appl. Math. 1979, 23, 97–120. [Google Scholar] [CrossRef]
- Dingfelder, B.; Weideman, J.A.C. An improved Talbot method for numerical Laplace transform inversion. Numer. Algorithms 2015, 68, 167–183. [Google Scholar] [CrossRef]
- Thomée, V. A high order parallel method for time discretization of parabolic type equations based on Laplace transformation and quadrature. Int. J. Numer. Anal. Anal. Model. 2005, 2, 85–96. [Google Scholar]
- Lpez-Fernández, M.; Palencia, C. On the numerical inversion of the Laplace transform of certain holomorphic mappings. Appl. Numer. Math. 2004, 51, 289–303. [Google Scholar] [CrossRef]
- Kamran; Kamal, R.; Rahmat, G.; Shah, K. On the Numerical Approximation of Three-Dimensional Time Fractional Convection-Diffusion Equations. Math. Probl. Eng. 2021, 2021, 4640467. [Google Scholar] [CrossRef]
- Kamal, R.; Kamran; Rahmat, G.; Ahmadian, A.; Arshad, N.I.; Salahshour, S. Approximation of linear one dimensional partial differential equations including fractional derivative with non-singular kernel. Adv. Differ. Equ. 2021, 2021, 317. [Google Scholar] [CrossRef]
- Stehfest, H. Algorithm 368: Numerical inversion of Laplace transforms [D5]. Commun. ACM 1970, 13, 47–49. [Google Scholar] [CrossRef]
- Stehfest, H. Remark on algorithm 368: Numerical inversion of Laplace transforms. Commun. ACM 1970, 13, 624. [Google Scholar] [CrossRef]
- Davies, B.; Martin, B. Numerical inversion of the Laplace transform: A survey and comparison of methods. J. Comput. Phys. 1979, 33, 1–32. [Google Scholar] [CrossRef]
- Schaback, R. Error estimates and condition numbers for radial basis function interpolation. Adv. Comput. Math. 1995, 3, 251–264. [Google Scholar] [CrossRef]
- Trefethen, L.N.; Bau, I.I.I.D. Numerical Linear Algebra; Siam: Philadelphia, PA, USA, 1997; Volume 50. [Google Scholar]
- Kuznetsov, A. On the Convergence of the Gaver–Stehfest Algorithm. SIAM J. Numer. Anal. 2013, 51, 2984–2998. [Google Scholar] [CrossRef]
- Abate, J.; Valkó, P.P. Multi-precision Laplace transform inversion. Int. J. Numer. Methods Eng. 2004, 60, 979–993. [Google Scholar] [CrossRef]
- Abate, J.; Whitt, W. A unified framework for numerically inverting Laplace transforms. Informs J. Comput. 2006, 18, 408–421. [Google Scholar] [CrossRef]
- Fu, Z.J.; Chen, W.; Yang, H.T. Boundary particle method for Laplace transformed time fractional diffusion equations. J. Comput. Phys. 2013, 235, 52–66. [Google Scholar] [CrossRef]
- Chen, W.; Ye, L.; Sun, H. Fractional diffusion equations by the Kansa method. Comput. Math. Appl. 2010, 59, 1614–1620. [Google Scholar] [CrossRef]
- Liu, Q.X.; Gu, Y.; Zhuang, P.; Liu, F.; Nie, Y. An implicit RBF meshless approach for time fractional diffusion equations. Comput. Mech. 2011, 48, 1–12. [Google Scholar] [CrossRef] [Green Version]





| CPU(s) | |||||||
|---|---|---|---|---|---|---|---|
| 140 | 20 | 18 | 4.4420 | 3.7542 | 5.1591 | 0.101494 | |
| 145 | 2.0215 | 1.6787 | 2.3067 | 0.087209 | |||
| 150 | 1.6953 | 1.3842 | 1.9018 | 0.102077 | |||
| 150 | 25 | 16 | 1.6953 | 1.3842 | 1.9018 | 0.083023 | |
| 26 | 1.5566 | 1.2710 | 1.7462 | 0.092403 | |||
| 29 | 1.2755 | 1.0414 | 1.4308 | 0.095714 | |||
| 100 | 6 | 8 | 3.0429 | 3.0429 | 4.1876 | 0.072115 | |
| 10 | 1.9506 | 1.9506 | 2.6845 | 0.069145 | |||
| 12 | 1.1745 | 1.1745 | 1.6163 | 0.083798 | |||
| [66] | 1.7 |
| CPU(s) | |||||
|---|---|---|---|---|---|
| 15 | 4.88 | 2.44 | 7.50 | 4.069811 | |
| 16 | 1.34 | 6.68 | 2.05 | 0.937441 | |
| 17 | 7.80 | 3.87 | 1.19 | 0.785719 | |
| 18 | 5.60 | 2.81 | 8.68 | 0.741108 | |
| 10 | 2.70 | 1.09 | 3.39 | 1.635850 | |
| 12 | 2.22 | 8.68 | 2.68 | 1.221204 | |
| 14 | 2.28 | 1.13 | 3.49 | 1.309271 | |
| 16 | 3.00 | 1.21 | 3.76 | 1.231612 | |
| 18 | 3.20 | 1.27 | 3.94 | 1.309295 | |
| 20 | 8.20 | 3.28 | 1.01 | 1.418298 | |
| [67] | 1.140 |
| CPU(s) | |||||
|---|---|---|---|---|---|
| 20 | 1.5787 | 6.3150 | 3.7390 | 0.887272 | |
| 21 | 1.4402 | 5.7610 | 3.4110 | 0.832852 | |
| 22 | 9.2418 | 3.6967 | 2.1888 | 0.870290 | |
| 23 | 2.8984 | 1.1594 | 6.8645 | 0.969934 | |
| 24 | 3.4135 | 1.3654 | 8.0845 | 0.912345 | |
| 25 | 3.8871 | 1.5548 | 9.2061 | 1.039839 | |
| 26 | 3.0696 | 1.2278 | 7.2699 | 1.000615 | |
| CPU(s) | |||||
| 484 | 2.0243 | 9.2016 | 5.4793 | 0.754501 | |
| 529 | 2.8677 | 1.2468 | 7.4092 | 0.787635 | |
| 576 | 5.3314 | 2.2214 | 1.3176 | 0.777492 | |
| 625 | 3.8871 | 1.5548 | 9.2061 | 0.877768 | |
| 676 | 4.0459 | 1.5561 | 9.1988 | 1.037267 | |
| 729 | 3.4507 | 1.2780 | 7.5438 | 0.965576 | |
| CPU(s) | |||||
| 10 | 2.4250 | 8.0833 | 4.7530 | 0.976971 | |
| 12 | 7.6536 | 2.5512 | 1.5001 | 1.074337 | |
| 14 | 4.6386 | 1.5462 | 9.0918 | 1.101967 | |
| 16 | 1.2244 | 4.0813 | 2.3998 | 1.095336 | |
| 18 | 1.0368 | 3.4560 | 2.0322 | 1.120181 |
| CPU(s) | |||||
|---|---|---|---|---|---|
| 729 | 2.4631 | 1.0781 | 5.7929 | 5.831264 | |
| 1000 | 1.8778 | 9.6171 | 5.1065 | 8.208304 | |
| 1331 | 3.7038 | 8.0097 | 4.2130 | 12.144358 | |
| 1728 | 1.6588 | 7.3857 | 3.8551 | 17.086863 | |
| 2197 | 2.6392 | 8.7479 | 4.5370 | 25.868403 | |
| CPU(s) | |||||
| 16 | 2.0937 | 6.9537 | 3.6576 | 11.743146 | |
| 18 | 2.0961 | 6.9627 | 3.6623 | 13.808768 | |
| 20 | 2.0930 | 7.0879 | 3.7282 | 11.128933 | |
| 22 | 3.0057 | 8.5166 | 4.4797 | 11.274943 | |
| CPU(s) | |||||
| 71 | 2.0501 | 8.2774 | 4.3539 | 14.044730 | |
| 72 | 2.0674 | 8.0409 | 4.2295 | 14.006118 | |
| 73 | 2.5473 | 8.2782 | 4.3543 | 16.449350 | |
| 74 | 2.0207 | 8.2277 | 4.3277 | 16.104030 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kamran; Irfan, M.; Alotaibi, F.M.; Haque, S.; Mlaiki, N.; Shah, K. RBF-Based Local Meshless Method for Fractional Diffusion Equations. Fractal Fract. 2023, 7, 143. https://doi.org/10.3390/fractalfract7020143
Kamran, Irfan M, Alotaibi FM, Haque S, Mlaiki N, Shah K. RBF-Based Local Meshless Method for Fractional Diffusion Equations. Fractal and Fractional. 2023; 7(2):143. https://doi.org/10.3390/fractalfract7020143
Chicago/Turabian StyleKamran, Muhammad Irfan, Fahad M. Alotaibi, Salma Haque, Nabil Mlaiki, and Kamal Shah. 2023. "RBF-Based Local Meshless Method for Fractional Diffusion Equations" Fractal and Fractional 7, no. 2: 143. https://doi.org/10.3390/fractalfract7020143
APA StyleKamran, Irfan, M., Alotaibi, F. M., Haque, S., Mlaiki, N., & Shah, K. (2023). RBF-Based Local Meshless Method for Fractional Diffusion Equations. Fractal and Fractional, 7(2), 143. https://doi.org/10.3390/fractalfract7020143








