Scaling Analysis of Time-Reversal Asymmetries in Fully Developed Turbulence
Abstract
:1. Introduction
2. Material and Methods
2.1. Methods: Tiple Correlations as Indicators of Time-Reversal Asymmetries
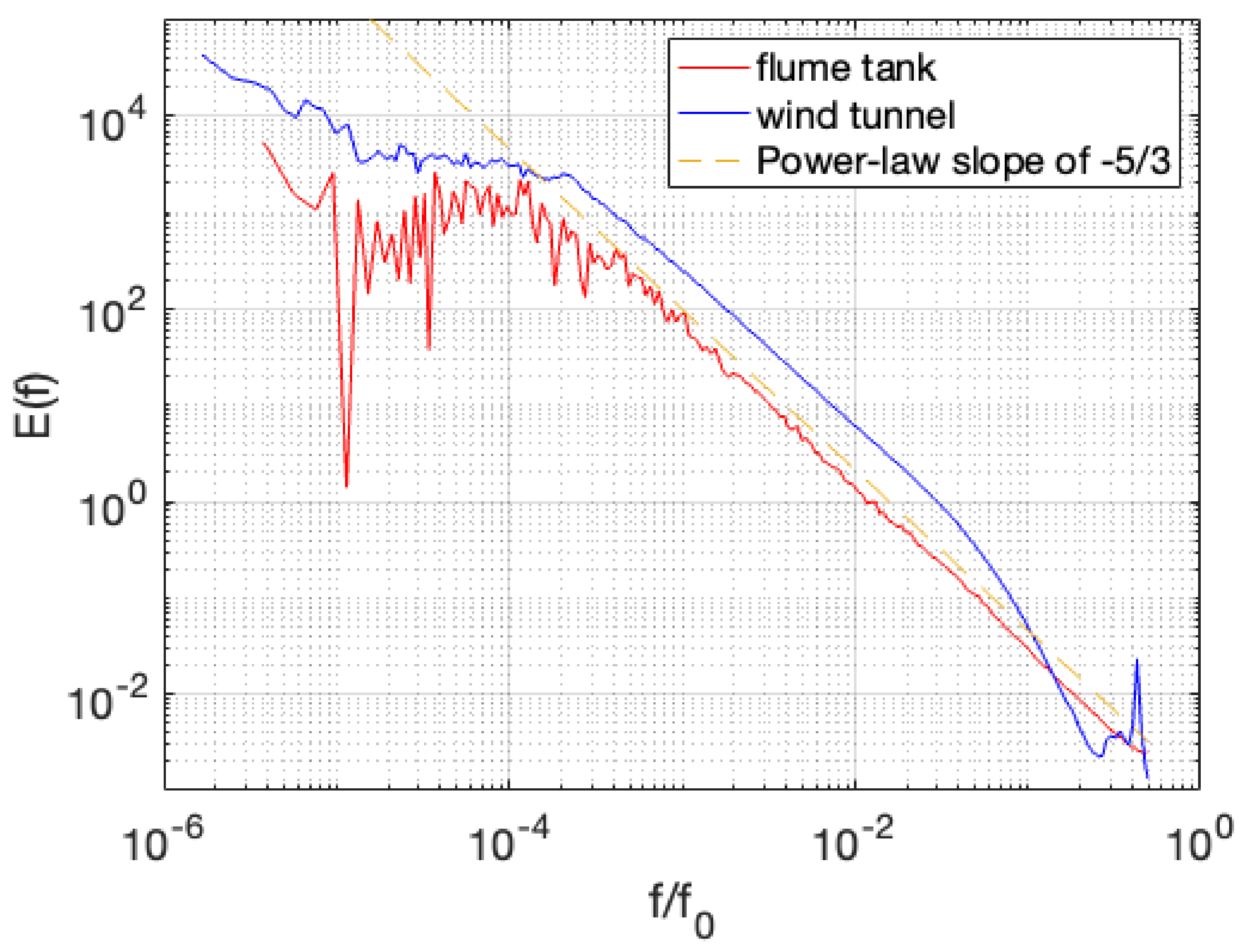
2.2. Presentation of the Databases
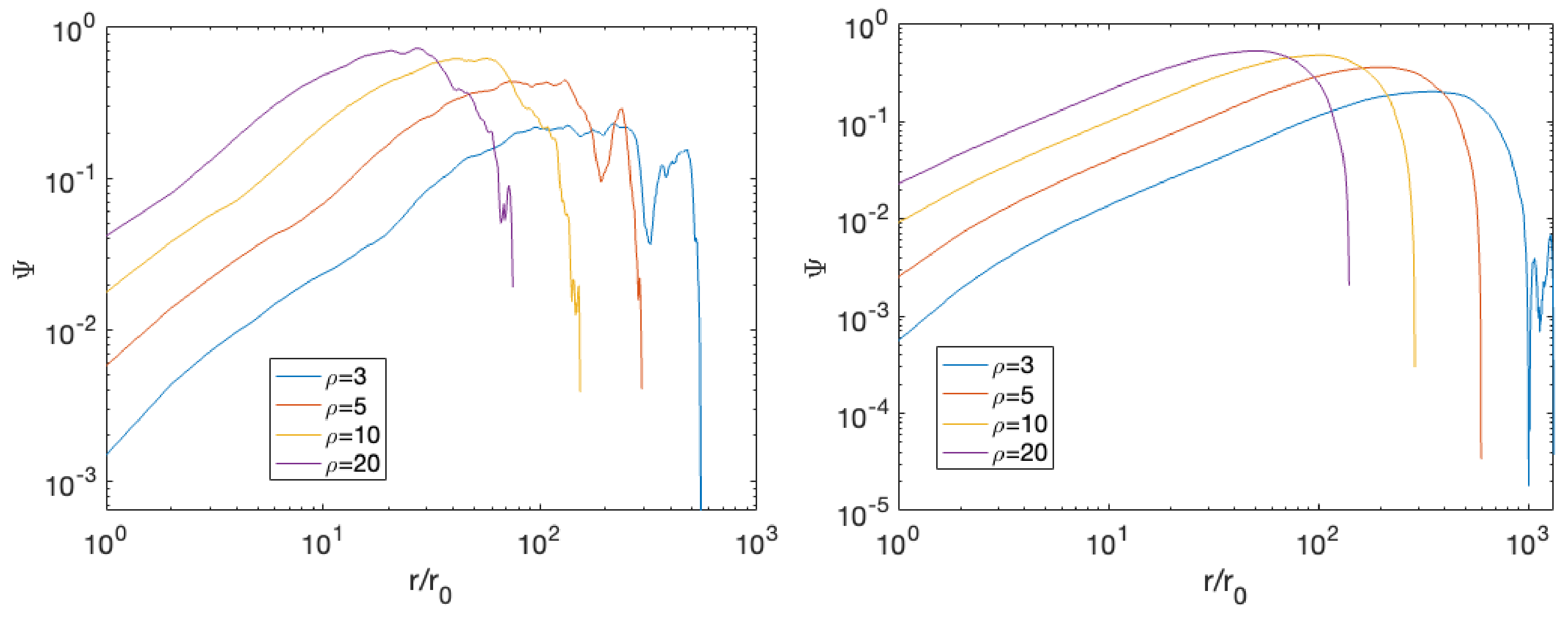
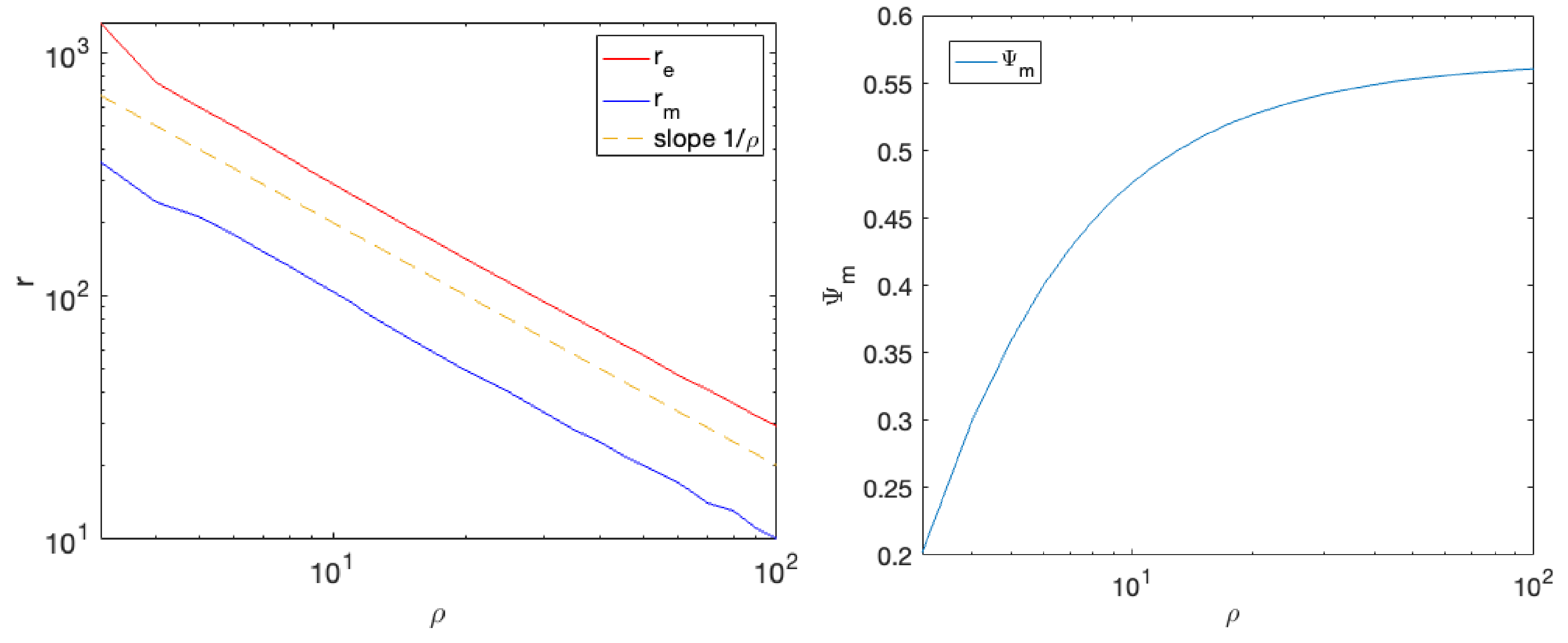
3. Results: Triple Correlation Analyses and Time Reversal Symmetries
4. Discussion and Conclusions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Frisch, U. Turbulence: The Legacy of A.N. Kolmogorov; Cambridge University Press: Cambridge, UK, 1995. [Google Scholar]
- Pope, S.B. Turbulent Flows; Cambridge University Press: Cambridge, UK, 2000. [Google Scholar]
- Kolmogorov, A.N. Local structure of turbulence in an incompressible fluid at very high Reynolds numbers. Dokl. Akad. Nauk SSSR 1941, 30, 299–303. [Google Scholar] [CrossRef]
- Obukhov, A.M. Spectral energy distribution in a turbulent flow. Dokl. Akad. Nauk SSSR 1941, 32, 22–24. [Google Scholar]
- Schmitt, F.G.; Huang, Y. Stochastic Analysis of Scaling Time Series: From Turbulence Theory to Applications; Cambridge University Press: Cambridge, UK, 2016. [Google Scholar]
- Fang, L.; Bos, W.J.T.; Shao, L.; Bertoglio, J.-P. Time reversibility of Navier-Stokes turbulence and its implication for subgrid scale models. J. Turbul. 2012, 13, 1–14. [Google Scholar] [CrossRef]
- Iacobello, G.; Chowdhuri, S.; Ridolfi, L.; Rondoni, L.; Scarsoglio, S. Coherent structures at the origin of time irreversibility in wall turbulence. Comm. Phys. 2023, 6, 91. [Google Scholar]
- Jucha, J.; Xu, H.; Pumir, A.; Bodenschatz, E. Time-reversal-symmetry breaking in turbulence. Phys. Rev. Lett. 2014, 113, 054501. [Google Scholar] [CrossRef]
- Xu, H.; Pumir, A.; Falkovich, G.; Bodenschatz, E.; Shats, M.; Xia, H.; Francois, N.; Boffetta, G. Flight-crash events in turbulence. Proc. Natl. Acad. Sci. USA 2014, 111, 7558–7563. [Google Scholar] [CrossRef]
- Xu, H.; Pumir, A.; Bodenschatz, E. Lagrangian view of time irreversibility of fluid turbulence. Sci. China-Phys. Mech. Astron. 2016, 59, 614702. [Google Scholar] [CrossRef]
- Pumir, A.; Xu, H.; Bodenschatz, E.; Grauer, R. Single-particle motion and vortex stretching in three-dimensional turbulent flows. Phys. Rev. Lett. 2016, 116, 124502. [Google Scholar] [CrossRef]
- Vencini, M.; Biferale, L.; Boffetta, G.; De Pietro, M. Time irreversibility and multifractality of power along single particle trajectories in turbulence. Phys. Rev. Fluids 2017, 2, 104604. [Google Scholar] [CrossRef]
- Bhatnagar, A.; Gupta, A.; Mitra, D.; Pandit, R. Heavy inertial particles in turbulent flows gain energy slowly but lose it rapidly. Phys. Rev. E 2018, 97, 033102. [Google Scholar] [CrossRef]
- Picardo, J.R.; Bhatnagar, A.; Ray, S.S. Lagrangian irreversibility and Eulerian dissipation in fully developed turbulence. Phys. Rev. Fluids 2020, 5, 042601. [Google Scholar] [CrossRef]
- Josserand, C.; Le Berre, M.; Lehner, T.; Pomeau, Y. Turbulence: Does energy cascade exist? J. Stat. Phys. 2017, 167, 596–625. [Google Scholar] [CrossRef]
- Le Berre, M.; Pomeau, Y. Singularities in turbulent flows: How to observe them? Phys. D 2023, 443, 133563. [Google Scholar] [CrossRef]
- Pomeau, Y. Symétrie des fluctuations dans le renversement du temps. J. Phys. 1982, 43, 859–867. [Google Scholar] [CrossRef]
- Pomeau, Y. Time in science: Reversibility vs. irreversibility. Lect. Notes Phys. 2004, 644, 425–436. [Google Scholar]
- Ramsey, J.B.; Rothman, P. Time irreversibility and business cycle asymmetry. J. Money Credit Bank. 1996, 28, 1–21. [Google Scholar] [CrossRef]
- Cheng, Q. On time-reversibility of linear processes. Biometrika 1999, 86, 483–486. [Google Scholar] [CrossRef]
- Chen, Y.T.; Chou, R.Y.; Kuan, C.M. Testing time reversibility without moment restrictions. J. Econom. 2000, 95, 199–218. [Google Scholar] [CrossRef]
- Psaradakis, Z. Assessing time-reversibility under minimal assumptions. J. Time Ser. Anal. 2008, 29, 881–905. [Google Scholar] [CrossRef]
- Telesca, L.; Flores-Marquez, E.L.; Ramirez-Rojas, A. Time-reversibility in seismic sequences: Application to the seismicity of Mexican subduction zone. Physica A 2018, 492, 1373–1381. [Google Scholar] [CrossRef]
- Duran Medina, O.; Schmitt, F.G.; Calif, R.; Germain, G.; Gaurier, B. Turbulence analysis and multiscale correlations between synchronized flow velocity and marine turbine power production. Renew. Energy 2017, 112, 314–327. [Google Scholar] [CrossRef]
- Gaurier, B.; Germain, G.; Facq, J.-V.; Bacchetti, T.; Duran-Medina, O. Experimental measurements of a synchronized flow velocity and a marine current turbine power production. SEANOE 2018. [Google Scholar] [CrossRef]
- Kang, H.; Chester, S.; Meneveau, C. Decaying turbulence in an active-grid-generated flow and comparisons with large-eddy simulation. J. Fluid Mech. 2003, 480, 129–160. [Google Scholar] [CrossRef]
- Huang, Y.X.; Schmitt, F.G.; Lu, Z.M.; Liu, Y.L. An amplitude-frequency study of turbulent scaling intermittency using Empirical Mode Decomposition and Hibert Spectral Analysis. Europhys. Lett. 2008, 84, 40010. [Google Scholar] [CrossRef]
- Huang, Y.X.; Schmitt, F.G.; Lu, Z.M.; Liu, Y.L. Autocorrelation function of velocity increments time series in fully developed turbulence. Europhys. Lett. 2009, 86, 40010. [Google Scholar] [CrossRef]
- Kolmogorov, A.N. Dissipation of energy in locally isotropic turbulence. Dokl. Akad. Nauk SSSR 1941, 32, 19–21. [Google Scholar]
- Falkovich, G. Symmetries of the turbulent state. J. Phys. A Math. Theor. 2009, 42, 123001. [Google Scholar] [CrossRef]
- Verma, M.K. Asymmetric energy transfers in driven nonequilibrium systems and arrow of time. Eur. Phys. J. B 2019, 92, 190. [Google Scholar] [CrossRef]
- Vela-Martin, A.; Jimenez, J. Entropy, irreversibility and cascades in the inertial range of isotropic turbulence. J. Fluid Mech. 2021, 915, A36. [Google Scholar] [CrossRef]
- Davidson, P.A. Turbulence, an Introduction for Scientists and Engineers; Oxford University Press: Oxford, UK, 2015. [Google Scholar]





Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Schmitt, F.G. Scaling Analysis of Time-Reversal Asymmetries in Fully Developed Turbulence. Fractal Fract. 2023, 7, 630. https://doi.org/10.3390/fractalfract7080630
Schmitt FG. Scaling Analysis of Time-Reversal Asymmetries in Fully Developed Turbulence. Fractal and Fractional. 2023; 7(8):630. https://doi.org/10.3390/fractalfract7080630
Chicago/Turabian StyleSchmitt, François G. 2023. "Scaling Analysis of Time-Reversal Asymmetries in Fully Developed Turbulence" Fractal and Fractional 7, no. 8: 630. https://doi.org/10.3390/fractalfract7080630
APA StyleSchmitt, F. G. (2023). Scaling Analysis of Time-Reversal Asymmetries in Fully Developed Turbulence. Fractal and Fractional, 7(8), 630. https://doi.org/10.3390/fractalfract7080630





