Numerical Investigation of the Fractional Diffusion Wave Equation with the Mittag–Leffler Function
Abstract
:1. Introduction
2. Preliminaries
Basis Functions
3. Description of Numerical Scheme
4. The Stability of the Presented Scheme
5. The Convergence of the Presented Scheme
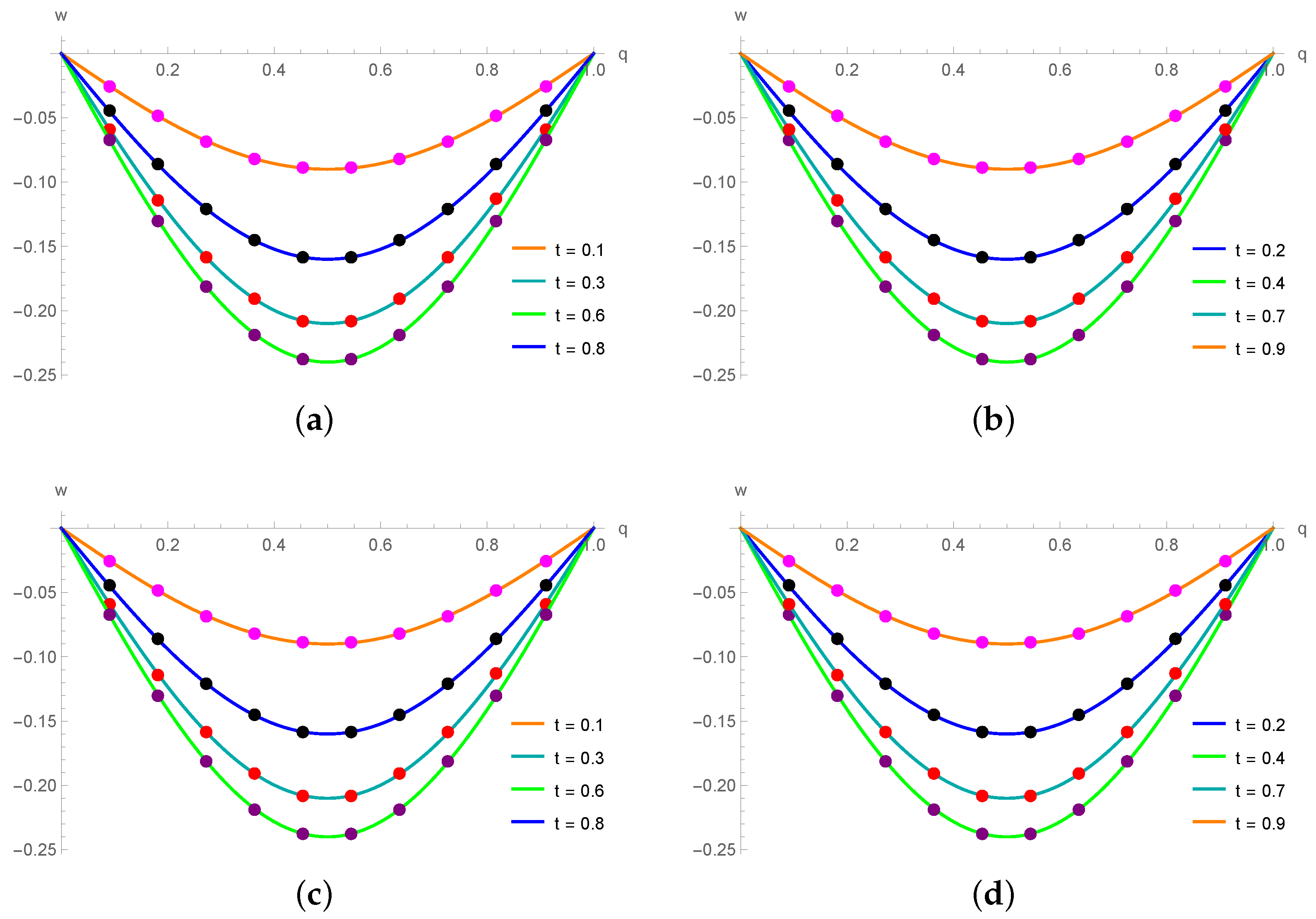
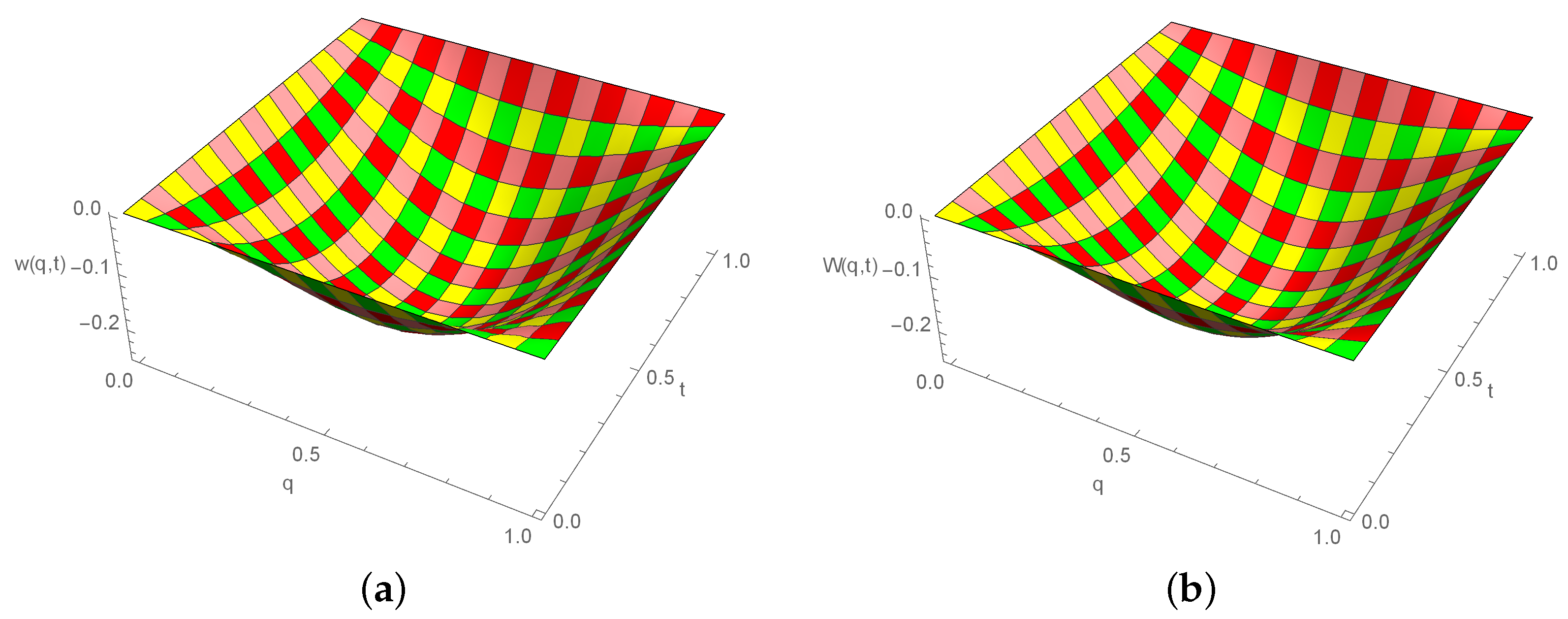
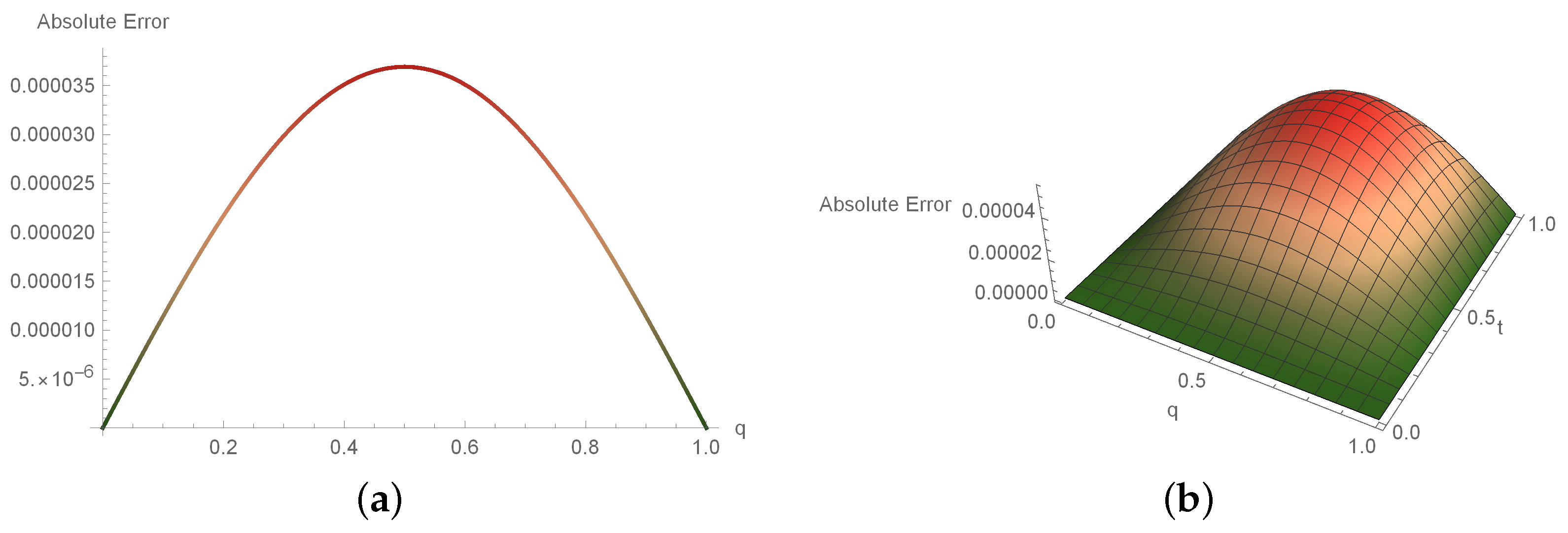
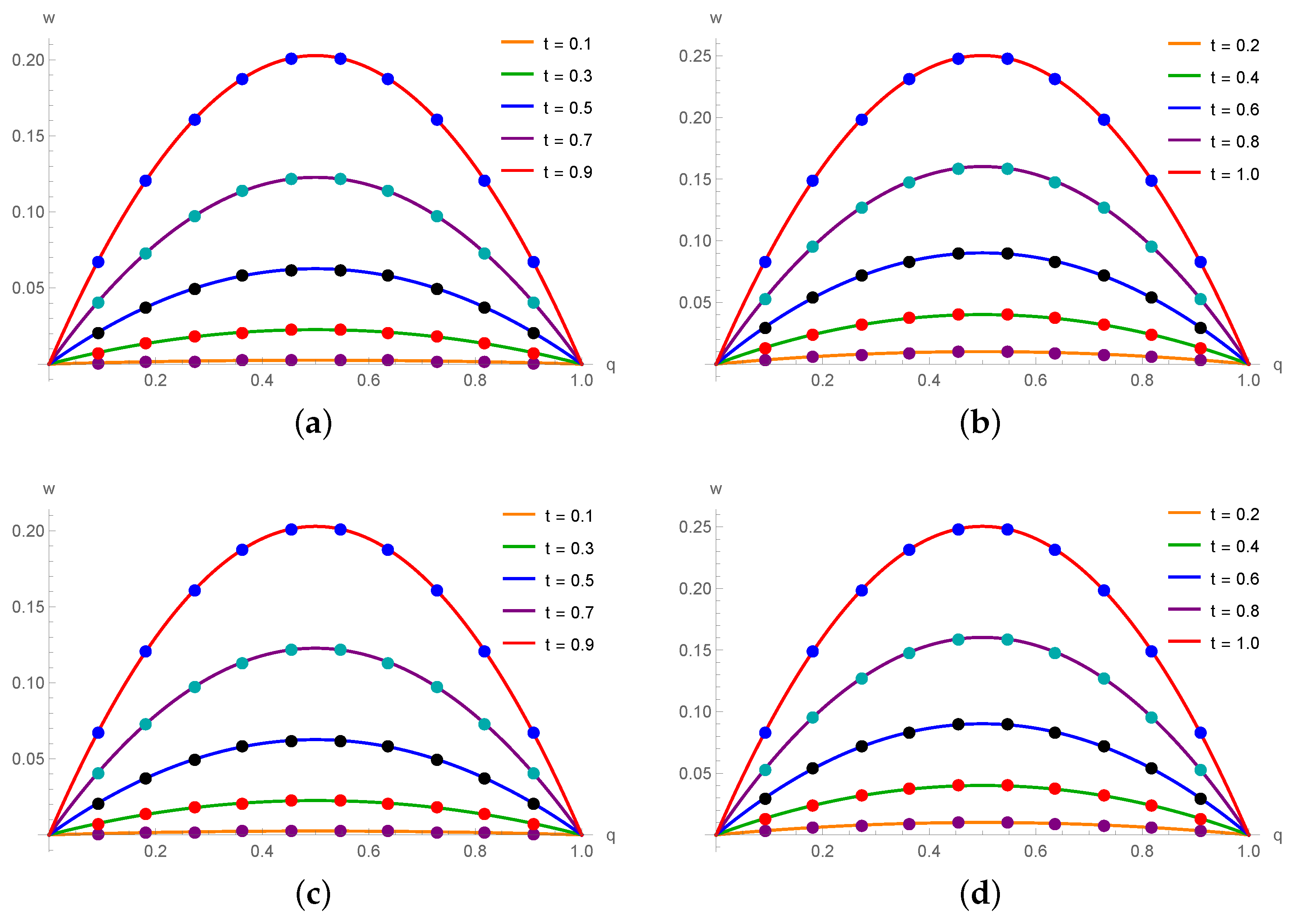
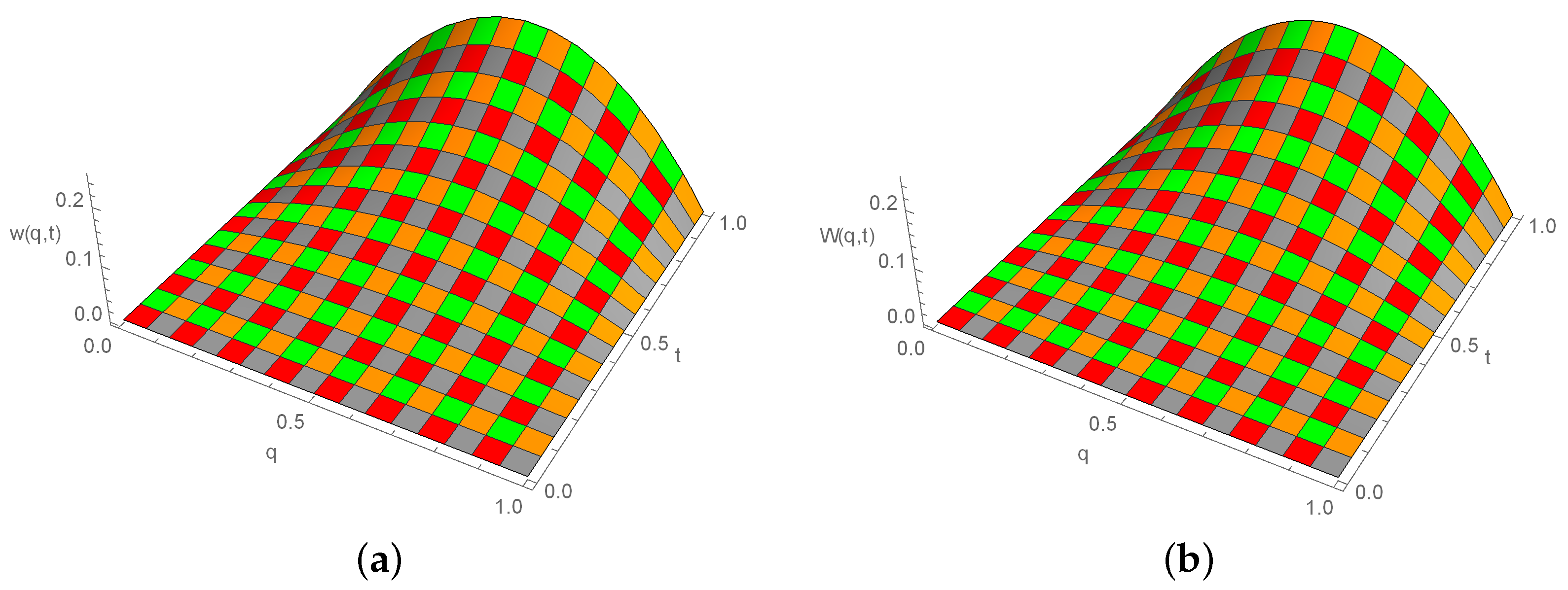
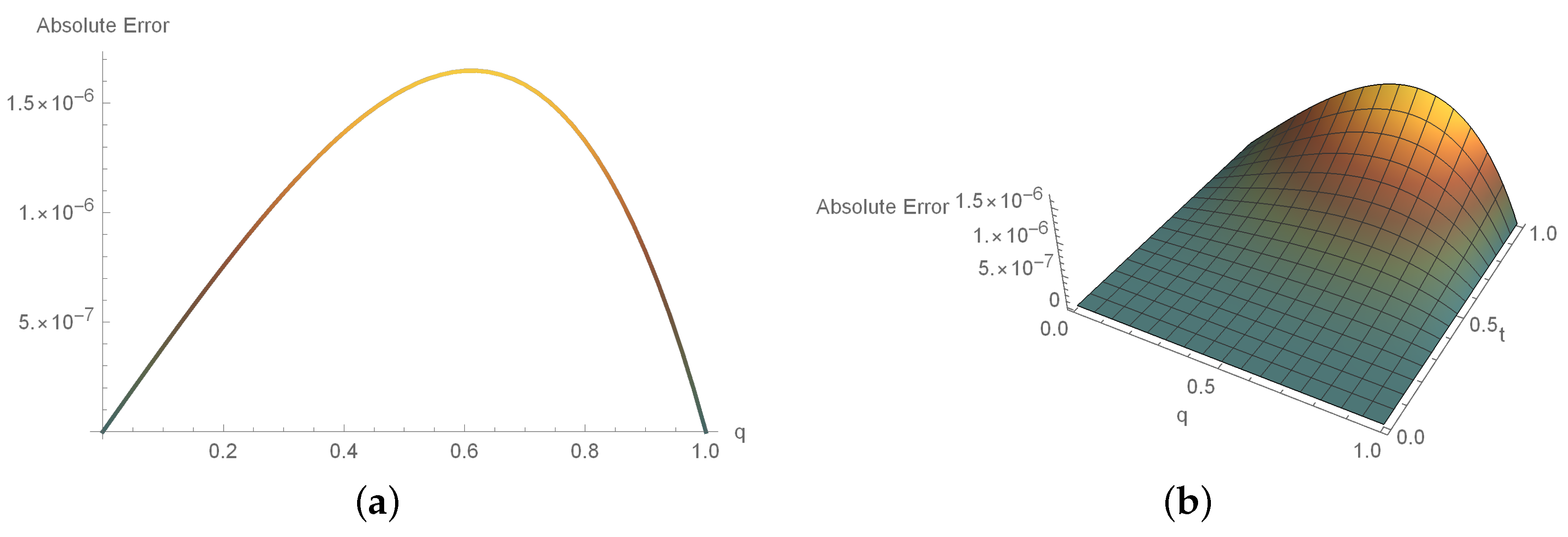
6. Numerical Results and Discussion
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Leibniz, G.W. Letter from Hanover, Germany to G. F. A. L’Hospital, 30 September 1695; Mathematische Schriften 1849; Olms: Hidesheim, Germany, Reprinted 1962; pp. 301–302. [Google Scholar]
- Miller, K.S.; Ross, B. An Introduction to the Fractional Calculus and Fractional Differential Equations; Wiley: New York, NY, USA, 1993. [Google Scholar]
- Podlubny, I. Fractional Differential Equations: An Introduction to Fractional Derivatives, Fractional Differential Equations, to Methods of Their Solution and Some of Their Applications; Elsevier: Amsterdam, The Netherlands; Academic Press: San Diego, CA, USA, 1998; pp. 115–122. [Google Scholar]
- Magin, R.L. Fractional Calculus in Bioengineering; Begell House: Redding, CA, USA, 2006. [Google Scholar]
- Iftikhar, N.; Baleanu, D.; Riaz, M.B.; Husnine, S.M. Heat and mass transfer of natural convective flow with slanted magnetic field via fractional operators. J. Appl. Comput. Mech. 2021, 7, 189–212. [Google Scholar]
- Sokolov, I.M.; Klafter, J.; Blumen, A. Fractional kinetics. Phys. Today 2002, 55, 48–54. [Google Scholar] [CrossRef]
- Caputo, M.; Fabrizio, M. Damage and fatigue described by a fractional derivative model. J. Comput. Phys. 2015, 293, 400–408. [Google Scholar] [CrossRef]
- Atangana, A.; Bildik, N. The use of fractional order derivative to predict the groundwater flow. Math. Probl. Eng. 2013, 2013, 543026. [Google Scholar] [CrossRef]
- Atangana, A.; Vermeulen, P.D. Analytical solutions of a space-time fractional derivative of groundwater flow equation. Abstr. Appl. Anal. 2014, 2014, 381753. [Google Scholar] [CrossRef]
- Tenreiro Machado, J.A.; Silva, M.F.; Barbosa, R.S.; Jesus, I.S.; Reis, C.M.; Marcos, M.G.; Galhano, A.F. Some applications of fractional calculus in engineering. Math. Probl. Eng. 2010, 2010, 639801. [Google Scholar] [CrossRef]
- Metzler, R.; Jeon, J.H.; Cherstvy, A.G.; Barkai, E. Anomalous diffusion models and their properties: Non-stationarity, non-ergodicity, and ageing at the centenary of single particle tracking. Phys. Chem. Chem. Phys. 2014, 16, 24128–24164. [Google Scholar] [CrossRef]
- Karaman, M.M.; Sui, Y.; Wang, H.; Magin, R.L.; Li, Y.; Zhou, X.J. Differentiating low- and high-grade pediatric brain tumors using a continuous-time random-walk diffusion model at high b-values. Magn. Reson. Med. 2015, 76, 1149–1157. [Google Scholar] [CrossRef]
- Zhang, Y.; Baeumer, B.; Chen, L.; Reeves, D.M.; Sun, H. A fully subordinated linear flow model for hillslope subsurface stormflow. Water Resour. Res. 2017, 53, 3491–3504. [Google Scholar] [CrossRef]
- Tarasova, V.V.; Tarasov, V.E. Concept of dynamic memory in economics. Commun. Nonlinear Sci. Numer. Simul. 2018, 55, 127–145. [Google Scholar] [CrossRef]
- Boulaaras, S.; Rehman, Z.U.; Abdullah, F.A.; Jan, R.; Abdalla, M.; Jan, A. Coronavirus dynamics, infections and preventive interventions using fractional-calculus analysis. AIMS Math. 2023, 8, 8680–8701. [Google Scholar] [CrossRef]
- Bas, E.; Ozarslan, R. Real world applications of fractional models by Atangana-Baleanu fractional derivative. Chaos Solit. Fractals 2018, 116, 121–125. [Google Scholar] [CrossRef]
- Gómez-Aguilar, J.F.; Escobar-Jiménez, R.F.; López-López, M.G.; Alvarado-Martínez, V.M. Atangana-Baleanu fractional derivative applied to electromagnetic waves in dielectric media. J. Electromagn. Waves Appl. 2016, 30, 1937–1952. [Google Scholar]
- Ghanbari, B.; Atangana, A. A new application of fractional Atangana-Baleanu derivatives: Designing ABC-fractional masks in image processing. Phys. A Stat. Mech. Appl. 2020, 542, 123516. [Google Scholar] [CrossRef]
- Gao, W.; Ghanbari, B.; Baskonus, H.M. New numerical simulations for some real world problems with Atangana-Baleanu fractional derivative. Chaos Solitons Fractals 2019, 128, 34–43. [Google Scholar] [CrossRef]
- Ravichandran, C.; Logeswari, K.; Khan, A.; Abdeljawad, T.; Gómez-Aguilar, J.F. An epidemiological model for computer virus with Atangana-Baleanu fractional derivative. Results Phys. 2023, 51, 106601. [Google Scholar] [CrossRef]
- Hanif, A.; Butt, A.I.K. Atangana-Baleanu fractional dynamics of dengue fever with optimal control strategies. AIMS Math. 2023, 8, 15499–15535. [Google Scholar] [CrossRef]
- Goyal, M.; Saraswat, A.K.; Prakash, A. Numerical analysis of fractional coronavirus model with Atangana-Baleanu derivative in Liouville-Caputo sense. Ind. J. Phys. 2023, 97, 147–164. [Google Scholar] [CrossRef]
- Liu, Z.; Cheng, A.; Li, X. A novel finite difference discrete scheme for the time fractional diffusion-wave equation. Appl. Numer. Math. 2018, 134, 17–30. [Google Scholar] [CrossRef]
- Huang, J.; Tang, Y.; Vázquez, L.; Yang, J. Two finite difference schemes for time fractional diffusion-wave equation. Numer. Algorithms 2013, 64, 707–720. [Google Scholar] [CrossRef]
- Dehghan, M.; Abbaszadeh, M.; Mohebbi, A. Analysis of a meshless method for the time fractional diffusion-wave equation. Numer. Algorithms 2016, 73, 445–476. [Google Scholar] [CrossRef]
- Ali, U.; Iqbal, A.; Sohail, M.; Abdullah, F.A.; Khan, Z. Compact implicit difference approximation for time-fractional diffusion-wave equation. Alex. Eng. J. 2022, 61, 4119–4126. [Google Scholar] [CrossRef]
- Wei, L. Analysis of a new finite difference/local discontinuous Galerkin method for the fractional diffusion-wave equation. Appl. Math. Comput. 2017, 304, 180–189. [Google Scholar] [CrossRef]
- Soltani Sarvestani, F.; Heydari, M.H.; Niknam, A.; Avazzadeh, Z. A wavelet approach for the multi-term time fractional diffusion-wave equation. Int. J. Comput. Math. 2019, 96, 640–661. [Google Scholar] [CrossRef]
- Huang, J.; Yang, D.; Jay, L.O. Efficient methods for nonlinear time fractional diffusion-wave equations and their fast implementations. Numer. Algorithms 2020, 85, 375–397. [Google Scholar] [CrossRef]
- Fardi, M.; Al-Omari, S.K.Q.; Araci, S. A pseudo-spectral method based on reproducing kernel for solving the time-fractional diffusion-wave equation. Adv. Cont. Disc. Mod. 2022, 2022, 54. [Google Scholar] [CrossRef]
- Chen, J.; Liu, F.; Anh, V.; Shen, S.; Liu, Q.; Liao, C. The analytical solution and numerical solution of the fractional diffusion-wave equation with damping. Appl. Math. Comput. 2012, 219, 1737–1748. [Google Scholar] [CrossRef]
- Yang, J.Y.; Huang, J.F.; Liang, D.M.; Tang, Y.F. Numerical solution of fractional diffusion-wave equation based on fractional multistep method. Appl. Math. Model. 2014, 38, 3652–3661. [Google Scholar] [CrossRef]
- Chen, A.; Li, C. Numerical solution of fractional diffusion-wave equation. Numer. Funct. Anal. Optim. 2016, 37, 19–39. [Google Scholar] [CrossRef]
- Hashemi, M.S.; Inc, M.; Parto-Haghighi, M.; Bayram, M. On numerical solution of the time-fractional diffusion-wave equation with the fictitious time integration method. Eur. Phys. J. Plus 2019, 134, 488. [Google Scholar] [CrossRef]
- Shafiq, M.; Abbas, M.; Abualnaja, K.M.; Huntul, M.J.; Majeed, A.; Nazir, T. An efficient technique based on cubic B-spline functions for solving time-fractional advection diffusion equation involving Atangana-Baleanu derivative. Eng. Comput. 2022, 38, 901–917. [Google Scholar] [CrossRef] [PubMed]
- Abbas, M.; Bibi, A.; Alzaidi, A.S.M.; Nazir, T.; Majeed, A.; Akram, G. Numerical solutions of third-order time-fractional differential equations using cubic B-spline functions. Fractal Fract. 2022, 6, 528. [Google Scholar] [CrossRef]
- Dhiman, N.; Huntul, M.J.; Tamsir, M. A modified trigonometric cubic B-spline collocation technique for solving the time-fractional diffusion equation. Eng. Comput. 2021, 38, 2921–2936. [Google Scholar] [CrossRef]
- Shafiq, M.; Abbas, M.; Abdullah, F.A.; Majeed, A.; Abdeljawad, T.; Alqudah, M.A. Numerical solutions of time fractional Burgers’ equation involving Atangana-Baleanu derivative via cubic B-spline functions. Results Phys. 2022, 34, 105244. [Google Scholar] [CrossRef]
- Majeed, A.; Kamran, M.; Rafique, M. An approximation to the solution of time fractional modified Burgers’ equation using extended cubic B-spline method. Comput. Appl. Math. 2020, 39, 257. [Google Scholar] [CrossRef]
- Khader, M.M.; Abualnaja, K.M. Galerkin-FEM for obtaining the numerical solution of the linear fractional Klein-Gordon equation. J. Appl. Anal. Comput. 2019, 9, 261–270. [Google Scholar] [CrossRef]
- Majeed, A.; Kamran, M.; Iqbal, M.K.; Baleanu, D. Solving time fractional Burgers’ and Fisher’s equations using cubic B-spline approximation method. Adv. Differ. Equ. 2020, 2020, 175. [Google Scholar] [CrossRef]
- Shafiq, M.; Abdullah, F.A.; Abbas, M.; Alzaidi, A.S.M.; Riaz, M.B. Memory effect analysis using piecewise cubic B-spline of time fractional diffusion equation. Fractals 2022, 30, 2240270. [Google Scholar] [CrossRef]
- Poulin, J.R. Calculating Infinite Series Using Parseval’s Identity. Master’s Thesis, The University of Maine, Orono, ME, USA, 2020. [Google Scholar]
- Boyce, W.E.; Diprima, R.C.; Meade, D.B. Elementary Differential Equations and Boundary Value Problems; Wiley: New York, NY, USA, 1992. [Google Scholar]
- Kadalbajoo, M.K.; Arora, P. B-spline collocation method for the singular-perturbation problem using artificial viscosity. Comput. Math. Appl. 2009, 57, 650–663. [Google Scholar] [CrossRef]
- Hall, C.A. On error bounds for spline interpolation. J. Approx. Theory 1968, 1, 209–218. [Google Scholar] [CrossRef]
- De Boor, C. On the convergence of odd-degree spline interpolation. J. Approx. Theory 1968, 1, 452–463. [Google Scholar] [CrossRef]
- Khader, M.M.; Adel, M.H. Numerical solutions of fractional wave equations using an efficient class of FDM based on the Hermite formula. Adv. Differ. Equ. 2016, 2016, 34. [Google Scholar] [CrossRef]
- Avazzadeh, Z.; Hosseini, V.R.; Chen, W. Radial basis functions and FDM for solving fractional diffusion-wave equation. Iran. J. Sci. Technol. 2014, 38, 205–212. [Google Scholar]



| Problem | B-Spline Function | Fractional Derivative | Finite Difference Scheme | |
|---|---|---|---|---|
| Reference [35] | Time fractional advection diffusion equation | Cubic B-spline functions | First-order Atangana–Baleanu fractional derivative | First-order implicit finite difference scheme |
| Reference [36] | Third-order time fractional differential equation | Cubic B-spline functions with new approximations | Caputo fractional derivative | Crank–Nicholson finite difference scheme |
| Proposed Method | Time fractional diffusion wave equation | Cubic B-spline functions | Second-order Atangana–Baleanu fractional derivative | Second-order implicit finite difference scheme |
| q | Exact Result | Approximate Result | Absolute Error |
|---|---|---|---|
| 0.1 | |||
| 0.2 | |||
| 0.3 | |||
| 0.4 | |||
| 0.5 | |||
| 0.6 | |||
| 0.7 | |||
| 0.8 | |||
| 0.9 |
| 0.2 | ||||
| 0.4 | ||||
| 0.6 | ||||
| 0.8 | ||||
| 1.0 | ||||
| N | Order | CPU Time (s) | ||
|---|---|---|---|---|
| 10 | ⋯ | 0.89063 | ||
| 20 | 2.26563 | |||
| 40 | 4.01563 | |||
| 80 | 11.4375 | |||
| 160 | 39.3594 |
| HF [48] | Proposed Method | |||
|---|---|---|---|---|
| 0.01149 | ||||
| 0.00361 | ||||
| 0.00120 | ||||
| 0.00115 | ||||
| 0.00021 | ||||
| 0.00019 | ||||
| 0.00006 | ||||
| 0.00004 | ||||
| HF [48] | Proposed Method | |||
|---|---|---|---|---|
| 0.01396 | ||||
| 0.01064 | ||||
| 0.00736 | ||||
| 0.00653 | ||||
| 0.00586 | ||||
| 0.00494 | ||||
| 0.00460 | ||||
| 0.00443 | ||||
| Approximate Result | Absolute Error | ||||
|---|---|---|---|---|---|
| Exact Result | |||||
| 0.1 | 0.000900146277 | ||||
| 0.2 | 0.001600273755 | ||||
| 0.3 | 0.002100370496 | ||||
| 0.4 | 0.002400430339 | ||||
| 0.5 | 0.002500450539 | ||||
| 0.6 | 0.002400430339 | ||||
| 0.7 | 0.002100370496 | ||||
| 0.8 | 0.001600273755 | ||||
| 0.9 | 0.000900146277 | ||||
| h | Order | CPU Time (s) | |||
|---|---|---|---|---|---|
| ⋯ | 0.04688 | ||||
| 0.29688 | |||||
| 4.23438 | |||||
| 187.781 |
| t | ||
|---|---|---|
| 0.2 | ||
| 0.4 | ||
| 0.6 | ||
| 0.8 | ||
| 1.0 |
| M | ||
|---|---|---|
| 10 | ||
| 20 | ||
| 40 | ||
| 80 | ||
| 160 |
| Approximate Solution | Absolute Error | ||||
|---|---|---|---|---|---|
| Exact Solution | |||||
| 0.1 | |||||
| 0.2 | |||||
| 0.3 | |||||
| 0.4 | |||||
| 0.5 | |||||
| 0.6 | |||||
| 0.7 | |||||
| 0.8 | |||||
| 0.9 | |||||
| M = 10 | M = 50 | ||||
|---|---|---|---|---|---|
| Exact Solution | RBF [49] | Proposed Method | RBF [49] | Proposed Method | |
| 0.1 | |||||
| 0.2 | |||||
| 0.3 | |||||
| 0.4 | |||||
| 0.5 | |||||
| 0.6 | |||||
| 0.7 | |||||
| 0.8 | |||||
| 0.9 | |||||
| 1.0 | |||||
| q | ||||
|---|---|---|---|---|
| 0.1 | ||||
| 0.2 | ||||
| 0.3 | ||||
| 0.4 | ||||
| 0.5 | ||||
| 0.6 | ||||
| 0.7 | ||||
| 0.8 | ||||
| 0.9 |
| h | Order | CPU Time (s) | |||
|---|---|---|---|---|---|
| ⋯ | 0.07813 | ||||
| 0.34375 | |||||
| 6.48438 | |||||
| 192.672 |
| t | ||
|---|---|---|
| 0.2 | ||
| 0.4 | ||
| 0.6 | ||
| 0.8 | ||
| 1.0 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shafiq, M.; Abbas, M.; El-Shewy, E.K.; Abdelrahman, M.A.E.; Abdo, N.F.; El-Rahman, A.A. Numerical Investigation of the Fractional Diffusion Wave Equation with the Mittag–Leffler Function. Fractal Fract. 2024, 8, 18. https://doi.org/10.3390/fractalfract8010018
Shafiq M, Abbas M, El-Shewy EK, Abdelrahman MAE, Abdo NF, El-Rahman AA. Numerical Investigation of the Fractional Diffusion Wave Equation with the Mittag–Leffler Function. Fractal and Fractional. 2024; 8(1):18. https://doi.org/10.3390/fractalfract8010018
Chicago/Turabian StyleShafiq, Madiha, Muhammad Abbas, Emad K. El-Shewy, Mahmoud A. E. Abdelrahman, Noura F. Abdo, and Ali A. El-Rahman. 2024. "Numerical Investigation of the Fractional Diffusion Wave Equation with the Mittag–Leffler Function" Fractal and Fractional 8, no. 1: 18. https://doi.org/10.3390/fractalfract8010018
APA StyleShafiq, M., Abbas, M., El-Shewy, E. K., Abdelrahman, M. A. E., Abdo, N. F., & El-Rahman, A. A. (2024). Numerical Investigation of the Fractional Diffusion Wave Equation with the Mittag–Leffler Function. Fractal and Fractional, 8(1), 18. https://doi.org/10.3390/fractalfract8010018









