Some Insights into the Sierpiński Triangle Paradox
Abstract
:1. Introduction
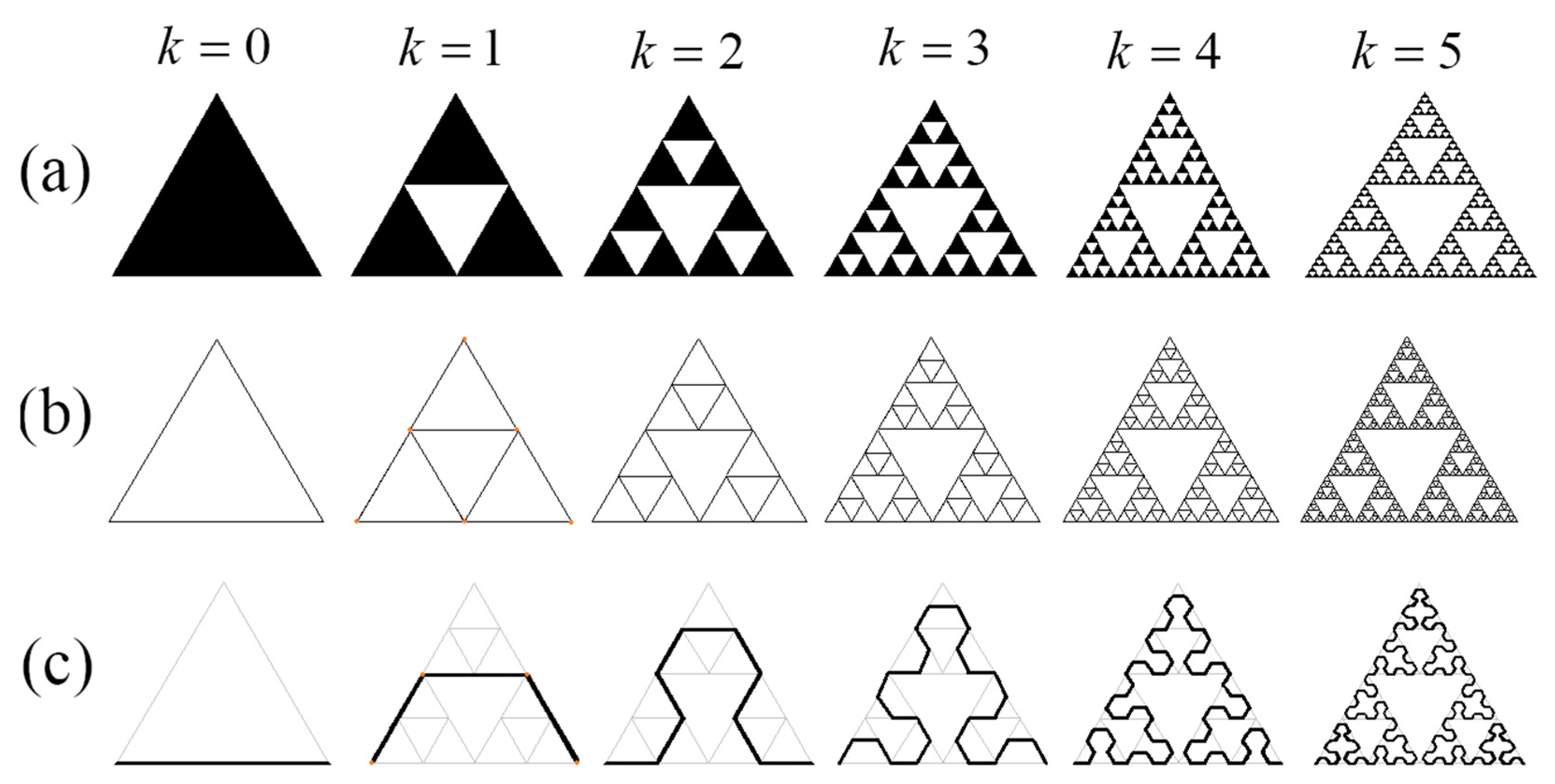
2. Topological Features of the SG
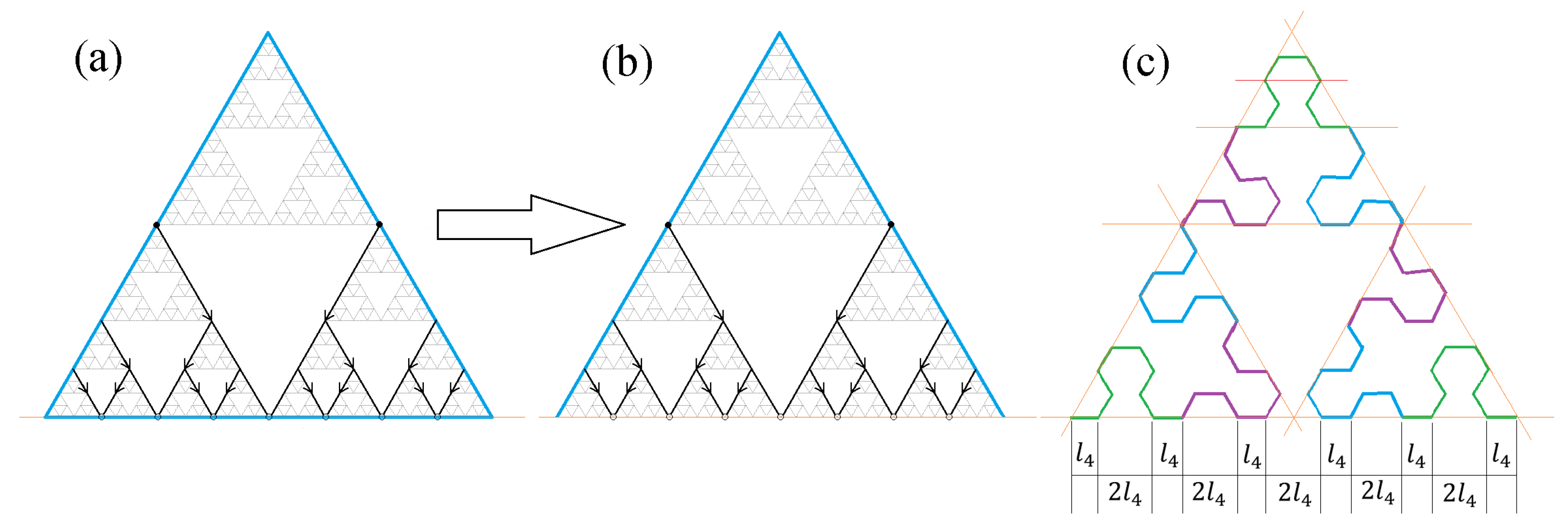
3. The Topological Difference Between SG and SAC
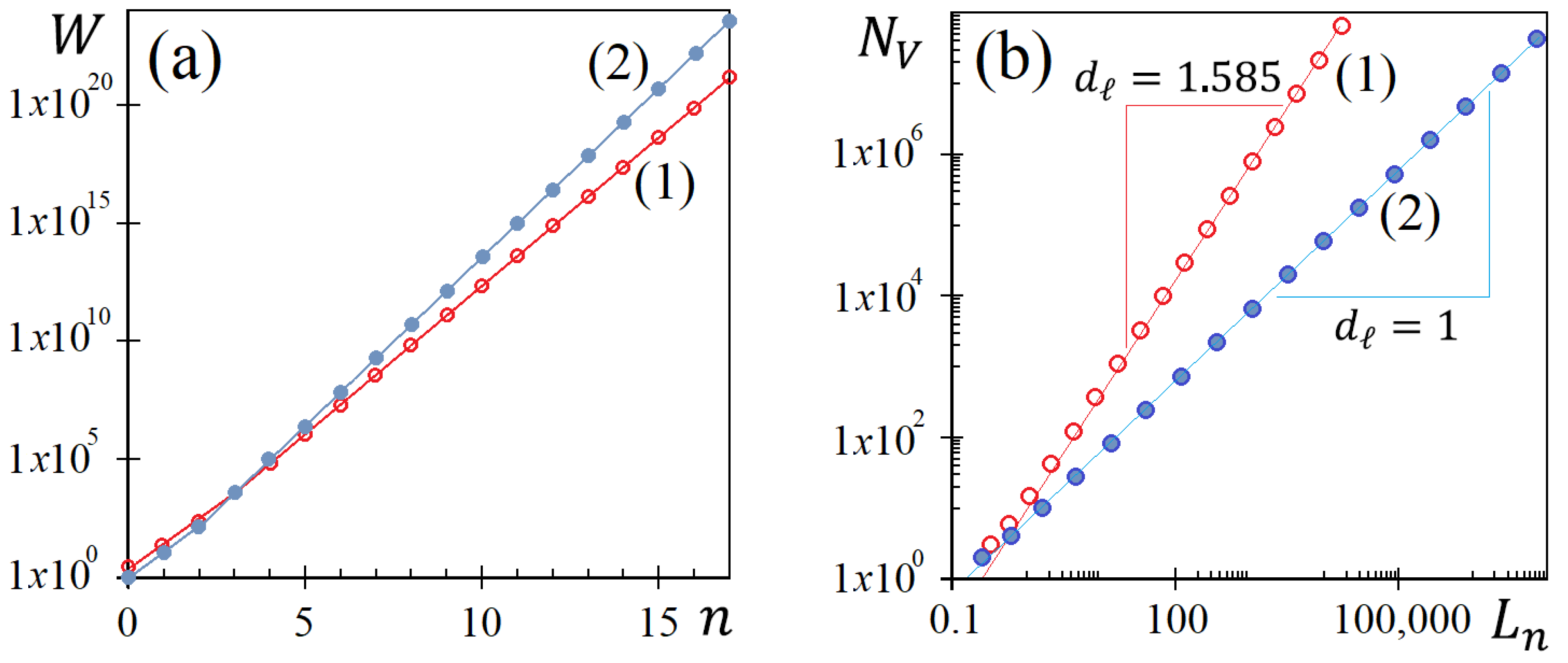
4. Dynamic Properties of SG and SAC
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Mandelbrot, B.B. The Fractal Geometry of Nature; Freeman: New York, NY, USA, 1982. [Google Scholar]
- Saxe, G.B.; Farid, A.M. The Interplay between Individual and Collective Activity: An Analysis of Classroom Discussions about the Sierpiński Triangle. Int. J. Res. Undergrad. Math. Educ. 2023, 9, 632–665. [Google Scholar] [CrossRef]
- Patiño-Ortiz, J.; Patiño-Ortiz, M.; Martínez-Cruz, M.A.; Balankin, A.S. A Brief Survey of Paradigmatic Fractals from a Topological Perspective. Fractal Fract. 2023, 7, 597. [Google Scholar] [CrossRef]
- Sierpiński, W. Sur une courbe dont tout point est un point de ramification. C. R. Acad. Paris 1915, 160, 302–305. [Google Scholar]
- Conversano, E.; Lalli, T.L. Sierpiński Triangles in Stone, on Medieval Floors in Rome. J. Appl. Math. 2011, 4, 113–122. [Google Scholar]
- Magrone, P. Sierpiński’s curve: A (beautiful) paradigm of recursion. Slov. Časopis Geom. Graf. 2020, 17, 17–28. [Google Scholar]
- Khovanova, T.; Nie, E.; Puranik, A. The Pioneering Role of the Sierpiński Gasket. Math Horizons 2015, 23, 5–9. [Google Scholar] [CrossRef]
- Claus, N. La tour d’Hanoi jeu de calcul. Sci. Nat. 1884, 1, 127–128. [Google Scholar]
- Lu, X. Towers of Hanoi graphs. Int. J. Comput. Math. 1986, 19, 23–38. [Google Scholar] [CrossRef]
- Stewart, I. Four Encounters with Sierpiński’s Gasket. Math. Intell. 1995, 17, 52–64. [Google Scholar] [CrossRef]
- Reiter, C.; With, J. 101 ways to build a Sierpiński triangle. ACM SIGAPL APL Quote Quad. 1997, 27, 8–16. Available online: http://hdl.handle.net/10385/1842 (accessed on 21 April 2015). [CrossRef]
- Balankin, A.S.; Patiño, J.; Patiño, M. Inherent features of fractal sets and key attributes of fractal models. Fractals 2022, 30, 2250082. [Google Scholar] [CrossRef]
- Yu, B.; Li, J. Some fractal characters of porous media. Fractals 2001, 9, 365–372. [Google Scholar] [CrossRef]
- Xu, P. A discussion on fractal models for transport physics of porous media. Fractals 2015, 23, 1530001. [Google Scholar] [CrossRef]
- Krawczuk, A.; Genoni, A. Current developments and trends in quantum crystallography. Acta Cryst. B 2024, 80, 249–274. [Google Scholar] [CrossRef]
- Biesenthal, T.; Maczewsky, L.J.; Yang, Z.; Kremer, M.; Segev, M.; Szameit, A.; Heinrich, M. Fractal photonic topological insulators. Science 2022, 376, 1114–1119. [Google Scholar] [CrossRef]
- Bicket, I.C.; Bellido, E.P.; McRae, D.M.; Lagugné-Labarthet, F.; Botton, G.A. Hierarchical plasmon resonances in fractal structures. ACS Photonics 2020, 7, 1246–1254. [Google Scholar] [CrossRef]
- Rojo-Francàs, A.; Pansari, P.; Bhattacharya, U.; Juliá-Díaz, B.; Grass, T. Anomalous quantum transport in fractal lattices. Commun. Phys. 2024, 7, 259. [Google Scholar] [CrossRef]
- Oftadeh, R.; Haghpanah, B.; Vella, D.; Boudaoud, A.; Vaziri, A. Optimal fractal-like hierarchical honeycombs. Phys. Rev. Lett. 2014, 113, 104301. [Google Scholar] [CrossRef]
- Cheng, Q.; Yin, J.; Wen, J.; Yu, D. Mechanical properties of 3D-printed hierarchical structures based on Sierpinski triangles. Int. J. Mech. Sci. 2023, 247, 108172. [Google Scholar] [CrossRef]
- Yazid, M.Y.I.; Baharuddin, M.H.; Islam, M.S.; Islam, M.T.; Almutairi, A.F. A Sierpinski arrowhead curve slot vivaldi antenna for microwave head imaging system. IEEE Access 2023, 11, 32335–32347. [Google Scholar] [CrossRef]
- Husain, A.; Nanda, M.N.; Chowdary, M.S.; Sajid, M. Fractals: An Eclectic Survey, Part-II. Fractal Fract. 2022, 6, 379. [Google Scholar] [CrossRef]
- Sierpiński, W. Sur une courbe cantorienne qui contient une image biunivoque et continue de toute courbe donnee. C. R. Acad. Paris 1916, 162, 629–632. [Google Scholar]
- Koudela, L. The Sierpiński triangle and its coordinate functions. In Scientific Papers of the University of Pardubice. Series D. Faculty of Economics and Administration; Vista previa de los detalles de la publicación; Univerzita Pardubice: Pardubice, Czech Republic, 2010; Volume 17, pp. 108–112. [Google Scholar]
- Sokolov, I.M.; Schnörer, H.; Blumen, A. Diffusion-controlled reaction A+B→0 on Peano curves. Phys. Rev. A 1991, 43, 5698. [Google Scholar] [CrossRef] [PubMed]
- Lind, J.; Rohde, S. Space-filling curves and phases of the Loewner equation. Indiana Univ. Math. J. 2012, 61, 2231–2249. Available online: https://www.jstor.org/stable/24904124 (accessed on 17 March 2010). [CrossRef]
- Luna-Elizarrarás, M.E.; Shapiro, M.; Balankin, A.S. Fractal-type sets in the four-dimensional space using bicomplex and hyperbolic numbers. Anal. Math. Phys. 2020, 10, 13. [Google Scholar] [CrossRef]
- Falconer, K.S. Fractal Geometry: Mathematical Foundations and Applications; Wiley: New York, NY, USA, 1990. [Google Scholar]
- Riane, N.; David, C. Sierpiński Gasket versus Arrowhead curve. Commun. Nonlinear Sci. Numer. Simulat. 2020, 89, 105311. [Google Scholar] [CrossRef]
- Arenas-Díaz, G.; Sabogal-Pedraza, S.M. Acerca del triángulo de Sierpiñski. Rev. Acad. Colomb. Cienc. 2009, 33, 395–405. [Google Scholar] [CrossRef]
- Ettestad, D.; Carbonara, J. Distinguishing between Sierpinski triangle constructions. Fractals 2019, 27, 1950091. [Google Scholar] [CrossRef]
- Jakovac, M.; Klavzar, S. Vertex-, edge-, and total-colorings of Sierpinski-like graphs. Discret. Math. 2009, 309, 1548–1556. [Google Scholar] [CrossRef]
- Vejnar, B. A topological characterization of the Sierpiński triangle. Topol. Appl. 2012, 159, 1404–1408. [Google Scholar] [CrossRef]
- Hinz, A.M.; Klavžar, S.; Zemljič, S.S. A survey and classification of Sierpiński-type graphs. Discret. Appl. Math. 2017, 217, 565–600. [Google Scholar] [CrossRef]
- Ettestad, D.; Carbonara, J. The Sierpinski triangle plane. Fractals 2018, 26, 1850003. [Google Scholar] [CrossRef]
- Fang, J.; Rafiullah, M.; Siddiqui, H. Topological properties of Sierpiński network and its application. Comb. Chem. High Throughput Screen. 2022, 25, 568–578. [Google Scholar] [CrossRef] [PubMed]
- Rammal, R.; d’Auriac, J.A.; Benoit, A. Metric properties of fractal lattices. J. Phys. A 1984, 17, L491–L494. [Google Scholar] [CrossRef]
- Schmutz, M. The Hausdorff dimension as an intrinsic metric property of fractals. Europhys. Lett. 1986, 2, 897–899. [Google Scholar] [CrossRef]
- Grassberger, P. Spreading of epidemic processes leading to fractal structures. In Fractals in Physics; Elsevier: New York, NY, USA, 1986; pp. 273–278. [Google Scholar]
- Hong, D.C.; Havlin, S.; Stanley, H.E. Family of growth fractals with continuously tunable chemical dimension. J. Phys. A 1985, 18, L1103. [Google Scholar] [CrossRef]
- Ori, O.; Cataldo, F.; Vukicevic, D.; Graovac, A. Wiener Way to Dimensionality. Iranian J. Math. Chem. 2010, 1, 5–15. [Google Scholar] [CrossRef]
- Luo, C.; Zuo, L.; Zhang, P.B. The Wiener index of Sierpiński-like graphs. J. Comb. Optim. 2018, 35, 814–841. [Google Scholar] [CrossRef]
- Chen, J.; He, L.; Wang, Q. Eccentric distance sum of Sierpiński gasket and Sierpiński network. Fractals 2019, 27, 1950016. [Google Scholar] [CrossRef]
- Imran, M.; Jamil, M.K. Sharp bounds on certain degree based topological indices for generalized Sierpiński graphs. Chaos Solitons Fractals 2020, 132, 109608. [Google Scholar] [CrossRef]
- Ali, A. Remarks on certain degree-based topological indices of generalized Sierpiński graphs. Chaos Solitons Fractals 2020, 138, 109956. [Google Scholar] [CrossRef]
- Divya, A.; Manimaran, A. Topological indices for the iterations of Sierpiński rhombus and Koch snowflake. Eur. Phys. J. Spec. Top. 2021, 230, 3971–3980. [Google Scholar] [CrossRef]
- Padmapriya, P.; Mathad, V. Topological indices of Sierpiński gasket and Sierpiński gasket rhombus graphs. TWMS J. Appl. Eng. Math. 2022, 12, 136–148. [Google Scholar]
- Lu, Y.; Xu, J.; Xi, L. Fractal version of hyper-Wiener index. Chaos Solitons Fractals 2023, 166, 112973. [Google Scholar] [CrossRef]
- Gayathiri, V.; Manimaran, A. Computing Certain Topological Indices of Silicate Triangle Fractal Network Modeled by the Sierpiński Triangle Network. Contemp. Math. 2024, 5, 2150–2164. [Google Scholar] [CrossRef]
- Wiener, H. Structural determination of paraffin boiling points. J. Am. Chem. Soc. 1947, 69, 17–20. [Google Scholar] [CrossRef] [PubMed]
- Balankin, A.S. A survey of fractal features of Bernoulli percolation. Chaos Solitons Fractals 2024, 184, 115044. [Google Scholar] [CrossRef]
- Hinz, A.M.; Schief, A. The average distance on the Sierpiński gasket. Probab. Theory Relat. Fields 1990, 87, 129–138. [Google Scholar] [CrossRef]
- Sato, H.; Denker, M. Sierpiński Gasket as a Martin Boundary II—(The Intrinsic Metric). Publ. Res. Inst. Math. Sci. 1999, 35, 769–794. Available online: https://ems.press/journals/prims/articles/2733 (accessed on 31 October 1999).
- Cristea, L.L.; Steinsky, B. Distances in Sierpiński graphs and on the Sierpiński gasket. Aequat. Math. 2013, 85, 201–219. [Google Scholar] [CrossRef]
- Saltan, M.; Özdemir, Y.; Demir, B. Geodesics of the Sierpiński gasket. Fractals 2018, 26, 1850024. [Google Scholar] [CrossRef]
- Saltan, M.; Özdemir, Y.; Demir, B. An explicit formula of the intrinsic metric on the Sierpiński gasket via code representation. Turk. J. Math. 2018, 42, 716–725. [Google Scholar] [CrossRef]
- Saltan, M. Intrinsic Metrics on Sierpiński -Like Triangles and Their Geometric Properties. Symmetry 2018, 10, 204. [Google Scholar] [CrossRef]
- Gu, J.; Fan, J.; Ye, Q.; Xi, L. Mean geodesic distance of the level-n Sierpiński gasket. J. Math. Anal. Appl. 2022, 508, 125853. [Google Scholar] [CrossRef]
- Hinz, A.M.; auf der Heide, C.H.; Zemljič, S.S. Metric properties of Sierpiński triangle graphs. Discret. Appl. Math. 2022, 319, 439–453. [Google Scholar] [CrossRef]
- Kigami, J.; Takahashi, K. “The Sierpiński gasket minus its bottom line” as a tree of Sierpiński gaskets. Math. Z. 2024, 306, 28. [Google Scholar] [CrossRef]
- Buczolich, Z. Category of density points of fat Cantor sets. Real Anal. Exch. 2003, 29, 497–502. [Google Scholar] [CrossRef]
- Dellwo, D.R. Fat Cantor sets and their skinny companions. Heliyon 2023, 9, e14862. [Google Scholar] [CrossRef]
- Liu, J.G.; Pego, R.L. A Simple Construction of Fat Cantor Sets. Am. Math. Mon. 2024, 131, 525. [Google Scholar] [CrossRef]
- Stanley, H.E. Application of fractal concepts to polymer statistics and to anomalous transport in randomly porous media. J. Stat. Phys. 1984, 36, 843–860. [Google Scholar] [CrossRef]
- Nakayama, T.; Yakubo, K.; Orbach, R. Dynamical properties of fractal networks: Scaling, numerical simulations, and physical realizations. Rev. Mod. Phys. 1994, 66, 381–443. [Google Scholar] [CrossRef]
- Havlin, S.; Ben-Avraham, D. Diffusion in disordered media. Adv. Phys. 1987, 36, 695–798. [Google Scholar] [CrossRef]
- Alexander, S.; Orbach, R. Density of states on fractals: Fractions. J. Phys. Lett. 1982, 43, L623–L631. [Google Scholar] [CrossRef]
- Orbach, R. Dynamics of fractal networks. Science 1986, 231, 814–819. [Google Scholar] [CrossRef]
- Balankin, A.S. A continuum framework for mechanics of fractal materials I: From fractional space to continuum with fractal metric. Eur. Phys. J. B 2015, 88, 90. [Google Scholar] [CrossRef]
- Liu, S.H. Spectral dimension of elastic Sierpinski gaskets. Phys. Rev. B 1984, 30, 4045(R)–4047(R). [Google Scholar] [CrossRef]
- Liu, S.H.; Liu, A.J. Spectral dimension of elastic Sierpinski gaskets with general elastic forces. Phys. Rev. B 1985, 32, 4753–4755. [Google Scholar] [CrossRef]
- Maritan, A.; Stella, A. Spectral dimension of a fractal structure with long-range interactions. Phys. Rev. B 1986, 34, 456–459. [Google Scholar] [CrossRef]
- Burioni, R.; Cassi, D. Universal Properties of Spectral Dimension. Phys. Rev. Lett. 1996, 76, 1091–1993. [Google Scholar] [CrossRef]
- Balankin, A.S. Effective degrees of freedom of a random walk on a fractal. Phys. Rev. E 2015, 92, 062146. [Google Scholar] [CrossRef]
- Balankin, A.S. Fractional space approach to studies of physical phenomena on fractals and in confined low-dimensional systems. Chaos Solitons Fractals 2020, 132, 109572. [Google Scholar] [CrossRef]
- Haynes, C.P.; Roberts, A.P. Generalization of the fractal Einstein law relating conduction and diffusion on networks. Phys. Rev. Lett. 2009, 103, 020601. [Google Scholar] [CrossRef] [PubMed]
- Rammal, R.; Toulouse, G. Random walks on fractal structures and percolation clusters. J. Phys. Lett. 1983, 44, 13–22. [Google Scholar] [CrossRef]
- Telcs, T. The Art of Random Walks; Springer: New York, NY, USA, 2006. [Google Scholar]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Martínez-Cruz, M.-Á.; Patiño-Ortiz, J.; Patiño-Ortiz, M.; Balankin, A.S. Some Insights into the Sierpiński Triangle Paradox. Fractal Fract. 2024, 8, 655. https://doi.org/10.3390/fractalfract8110655
Martínez-Cruz M-Á, Patiño-Ortiz J, Patiño-Ortiz M, Balankin AS. Some Insights into the Sierpiński Triangle Paradox. Fractal and Fractional. 2024; 8(11):655. https://doi.org/10.3390/fractalfract8110655
Chicago/Turabian StyleMartínez-Cruz, Miguel-Ángel, Julián Patiño-Ortiz, Miguel Patiño-Ortiz, and Alexander S. Balankin. 2024. "Some Insights into the Sierpiński Triangle Paradox" Fractal and Fractional 8, no. 11: 655. https://doi.org/10.3390/fractalfract8110655
APA StyleMartínez-Cruz, M.-Á., Patiño-Ortiz, J., Patiño-Ortiz, M., & Balankin, A. S. (2024). Some Insights into the Sierpiński Triangle Paradox. Fractal and Fractional, 8(11), 655. https://doi.org/10.3390/fractalfract8110655






