Bridging Pre-Invex Mappings and Fractional Integrals: A Pathway to Iterative Schemes via Error Boundaries of Maclaurin’s Rule
Abstract
:1. Introduction
2. Auxiliary Result
3. Main Results
4. Applications
4.1. Application to Means
- .
- .
- .
4.2. Applications to Error Bounds
- Taking the quadrature scheme into consideration,
4.3. Applications to Matrices
4.4. Iterative Methods
4.5. Examples and Visual Explanation of Iterative Scheme 1
- In the first example, we investigate the topic linked to the plug flow of Casson fluids in blood using the Rheology and Fractional Nonlinear Equations Model [34]. The decline in flow rate can be calculated by the following equation:Here, we pick , and by picking the initial value , Iterative Scheme 1 with predicts the desired root in the third iteration.
- We look into the issue of porosity in biogels ([34]). The subsequent equation illustrates the dependence between pressure and velocity:where , , and are the initial values. Then, Iterative Scheme 1 with determines the root in 13 iterations.
- .
- .
- .
- .
- ;
- .
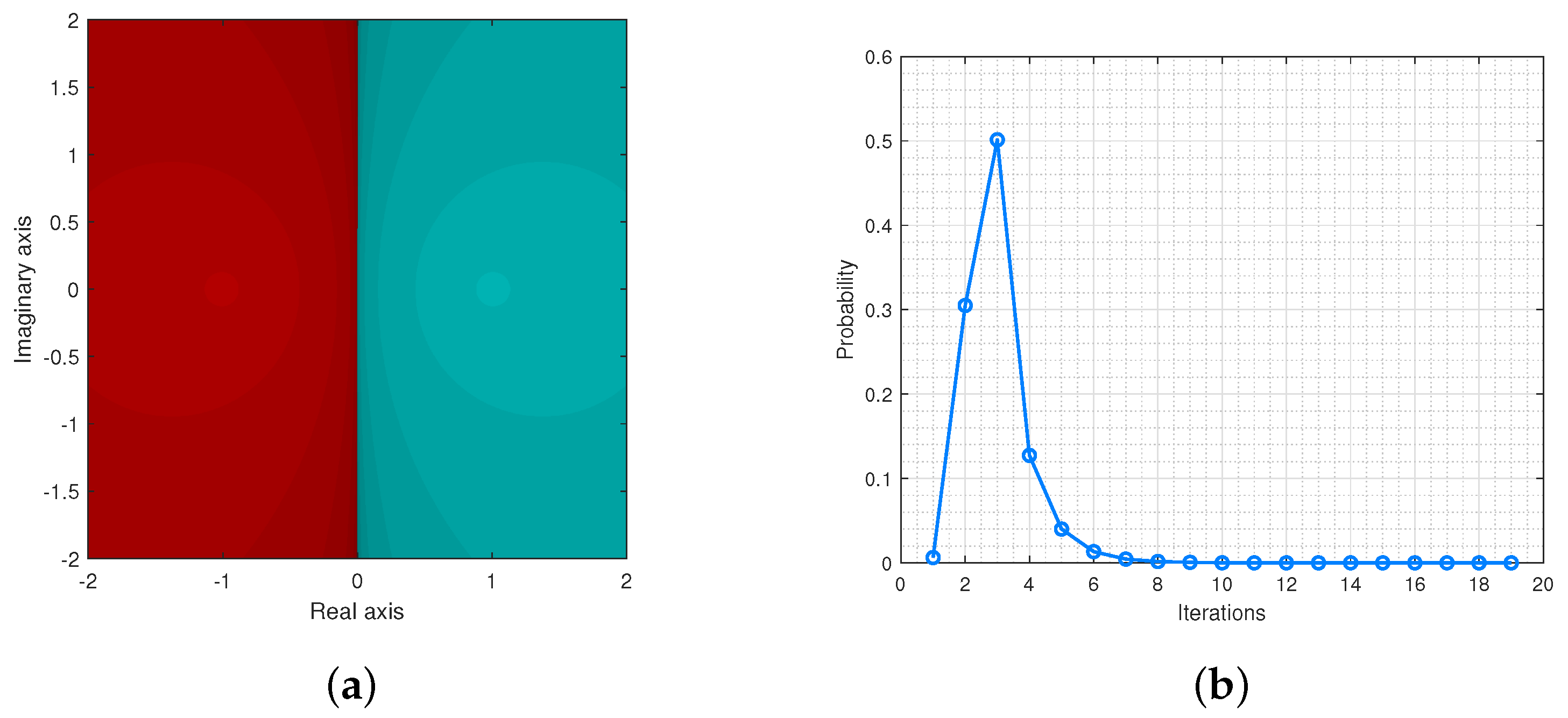
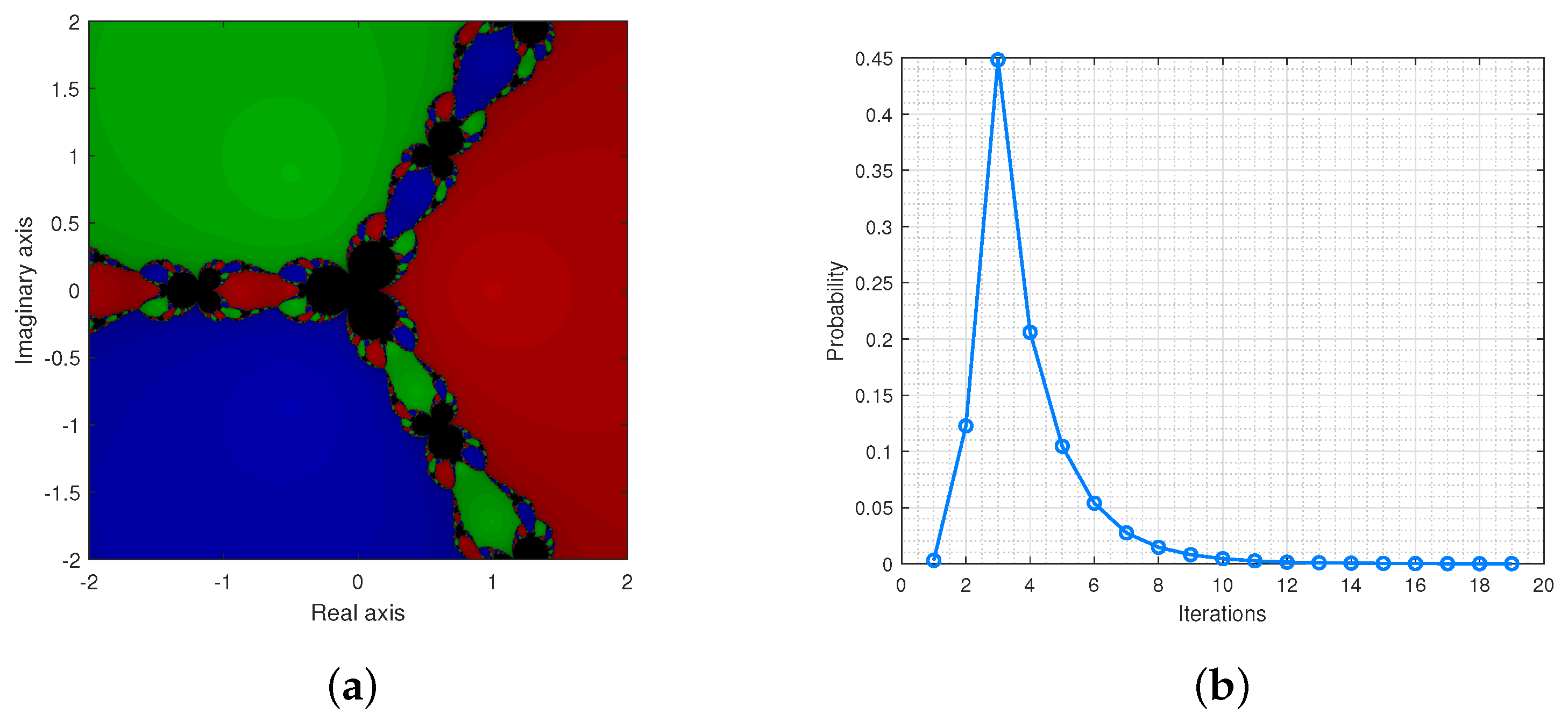
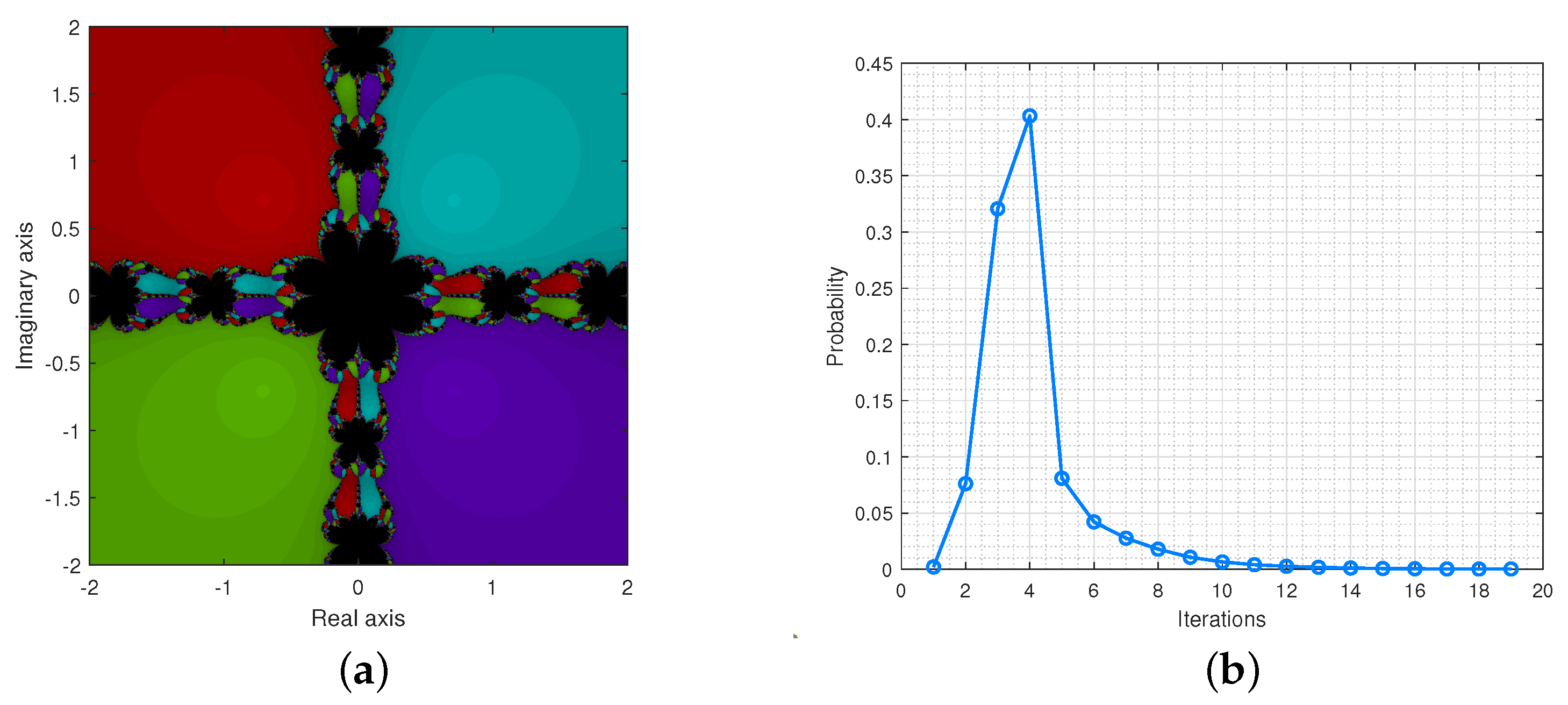
4.6. Basins of Attraction
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ben-Israel, A.; Mond, B. What is invexity? ANZIAM J. 1986, 28, 1–9. [Google Scholar] [CrossRef]
- Weir, T.; Mond, B. Pre-invex functions in multiple objective optimization. J. Math. Anal. Appl. 1988, 136, 29–38. [Google Scholar] [CrossRef]
- Mohan, S.R.; Neogy, S.K. On invex sets and preinvex functions. J. Math. Anal. Appl. 1995, 189, 901–908. [Google Scholar] [CrossRef]
- Matloka, M. Inequalities for h-preinvex functions. Appl. Math. Comput. 2014, 234, 52–57. [Google Scholar] [CrossRef]
- Noor, M.A.; Noor, K.I.; Awan, M.U.; Li, J. On Hermite-Hadamard inequalities for h-preinvex functions. Filomat 2014, 28, 1463–1474. [Google Scholar] [CrossRef]
- Ozcan, S. Some integral inequalities of Hermite-Hadamard type for multiplicatively preinvex functions. AIMS Math. 2020, 5, 1505–1518. [Google Scholar] [CrossRef]
- Lakhdari, A.; Meftah, B. Some fractional weighted trapezoid type inequalities for preinvex functions. Int. J. Nonlinear Anal. Appl. 2022, 13, 3567–3587. [Google Scholar]
- Tariq, M.; Shaikh, A.A.; Sahoo, S.K.; Ahmad, H.; Sitthiwirattham, T.; Reunsumrit, J. New integral inequalities via generalized preinvex functions. Axioms 2021, 10, 296. [Google Scholar] [CrossRef]
- Meftah, B. New integral inequalities for s-preinvex functions. Int. J. Nonlinear Anal. Appl. 2017, 8, 331–336. [Google Scholar]
- Du, T.S.; Liao, J.G.; Li, Y.J. Properties and integral inequalities of Hadamard-Simpson type for the generalized (s,m)-preinvex functions. J. Nonlinear Sci. Appl. 2016, 9, 3112–3126. [Google Scholar] [CrossRef]
- Gorenflo, R.; Mainardi, F. Fractional Calculus: Integral and Differential Equations of Fractional Order; Springer: Vienna, Austria, 1997; pp. 223–276. [Google Scholar]
- Sun, H.; Zhang, Y.; Baleanu, D.; Chen, W.; Chen, Y. A new collection of real world applications of fractional calculus in science and engineering. Commun. Nonlinear Sci. Numer. Simul. 2018, 64, 213–231. [Google Scholar] [CrossRef]
- Kilbas, A.A.; Srivastava, H.M.; Trujillo, J.J. Theory and Applications of Fractional Differential Equations; Elsevier: Amsterdam, The Netherlands, 2006; Volume 204. [Google Scholar]
- Hyder, A.A.; Barakat, M.A.; Fathallah, A. Enlarged integral inequalities through recent fractional generalized operators. J. Inequalities Appl. 2022, 2022, 95. [Google Scholar] [CrossRef]
- Hyder, A.A.; Barakat, M.A.; Soliman, A.H. A new class of fractional inequalities through the convexity concept and enlarged Riemann-Liouville integrals. J. Inequalities Appl. 2023, 2023, 137. [Google Scholar] [CrossRef]
- Sarikaya, M.Z.; Ertugral, F. On the generalized Hermite-Hadamard inequalities. Ann. Univ.-Craiova-Math. Comput. Sci. Ser. 2020, 47, 193–213. [Google Scholar]
- Dragomir, S.S.; Agarwal, R. Two inequalities for differentiable mappings and applications to special means of real numbers and to trapezoidal formula. Appl. Math. Lett. 1998, 11, 91–95. [Google Scholar] [CrossRef]
- Alomari, M.W. A companion of the generalized trapezoid inequality and applications. J. Math. Appl. 2013, 36, 5–15. [Google Scholar]
- Sarikaya, M.Z.; Yildirim, H. On Hermite-Hadamard type inequalities for Riemann-Liouville fractional integrals. Miskolc Math. Notes 2016, 17, 1049–1059. [Google Scholar] [CrossRef]
- Dragomir, S.S. On the midpoint quadrature formula for mappings with bounded variation and applications. Kragujev. J. Math. 2000, 22, 13–19. [Google Scholar]
- Set, E.; Akdemir, A.O.; Ozdemir, E.M. Simpson type integral inequalities for convex functions via Riemann-Liouville integrals. Filomat 2017, 31, 4415–4420. [Google Scholar] [CrossRef]
- Zhu, W.S.; Meftah, B.; Xu, H.; Jarad, F.; Lakhdari, A. On parameterized inequalities for fractional multiplicative integrals. Demonstr. Math. 2024, 57, 20230155. [Google Scholar] [CrossRef]
- Hussain, S.; Khalid, J.; Chu, Y.M. Some generalized fractional integral Simpson’s type inequalities with applications. AIMS Math. 2020, 5, 5859–5883. [Google Scholar] [CrossRef]
- Saleh, W.; Lakhdari, A.; Abdeljawad, T.; Meftah, B. On fractional biparameterized Newton-type inequalities. J. Inequalities Appl. 2023, 2023, 122. [Google Scholar] [CrossRef]
- Dragomir, S.S.; Agarwal, R.P.; Cerone, P. On Simpson’s inequality and applications. J. Inequal. Appl. 2000, 2000, 891030. [Google Scholar] [CrossRef]
- Ali, M.A.; Abbas, M.; Budak, H.; Agarwal, P.; Murtaza, G.; Chu, Y.M. New quantum boundaries for quantum Simpson’s and quantum Newton’s type inequalities for preinvex functions. Adv. Differ. Equations 2021, 2021, 1–21. [Google Scholar] [CrossRef]
- Bin-Mohsin, B.; Javed, M.Z.; Awan, M.U.; Khan, A.G.; Cesarano, C.; Noor, M.A. Exploration of quantum Milne-Mercer-type inequalities with applications. Symmetry 2023, 15, 1096. [Google Scholar] [CrossRef]
- You, X.; Hezenci, F.; Budak, H.; Kara, H. New Simpson type inequalities for twice differentiable functions via generalized fractional integrals. AIMS Math. 2022, 7, 3959–3971. [Google Scholar] [CrossRef]
- Davis, P.J.; Rabinowitz, P. Methods of Numerical Integration; Courier Corporation: North Chelmsford, MA, USA, 2007. [Google Scholar]
- Franjić, I.; Pećarić, J. Corrected Euler-Maclaurin’s formulae. Rend. Del Circ. Mat. Palermo 2005, 54, 259–272. [Google Scholar]
- Hezenci, F.; Budak, H. Fractional Euler-Maclaurin-type inequalities for various function classes. Comput. Appl. Math. 2024, 43, 261. [Google Scholar] [CrossRef]
- Hezenci, F.; Budak, H. Some Riemann-Liouville fractional integral inequalities of corrected Euler-Maclaurin-type. J. Anal. 2024, 32, 1309–1330. [Google Scholar] [CrossRef]
- Sababheh, M. Convex functions and means of matrices. Math. Inequal. Appl. 2017, 20, 29–47. [Google Scholar] [CrossRef]
- Fournier, R.L. Basic Transport Phenomena in Biomedical Engineering; Taylor & Francis: New York., NY, USA, 2007. [Google Scholar]
- Burden, R.K.; Faires, J.D. Numerical Analysis, 9th ed.; Brooks/Cole, Cengage Learning: Boston, MA, USA, 2011. [Google Scholar]
- Abbasbandy, S. Improving Newton–Raphson method for nonlinear equations by modified Adomian decomposition method. Appl. Math. Comput. 2003, 145, 887–893. [Google Scholar] [CrossRef]
- Chun, C. Iterative methods improving Newton’s method by the decomposition method. Comput. Math. Appl. 2005, 50, 1559–1568. [Google Scholar] [CrossRef]





| Methods | ||||||
|---|---|---|---|---|---|---|
| NM | 2 | 5 | 0 | |||
| AM | 2 | 4 | 0 | 0 | ||
| HM | 2 | 4 | 0 | 0 | ||
| CM | 2 | 4 | 0 | 0 | ||
| ALG | 2 | 4 | 0 | 0 | ||
| NM | 6 | |||||
| AM | 5 | 0 | ||||
| HM | 4 | 0 | ||||
| CM | 5 | 0 | ||||
| ALG | 5 | 0 | ||||
| NM | 5 | |||||
| AM | 4 | |||||
| HM | 4 | 0 | ||||
| CM | 4 | 0 | ||||
| ALG | 4 | 0 | ||||
| NM | 2 | 4 | ||||
| AM | 2 | 4 | ||||
| HM | 2 | 4 | ||||
| CM | 2 | 3 | ||||
| ALG | 2 | 4 |
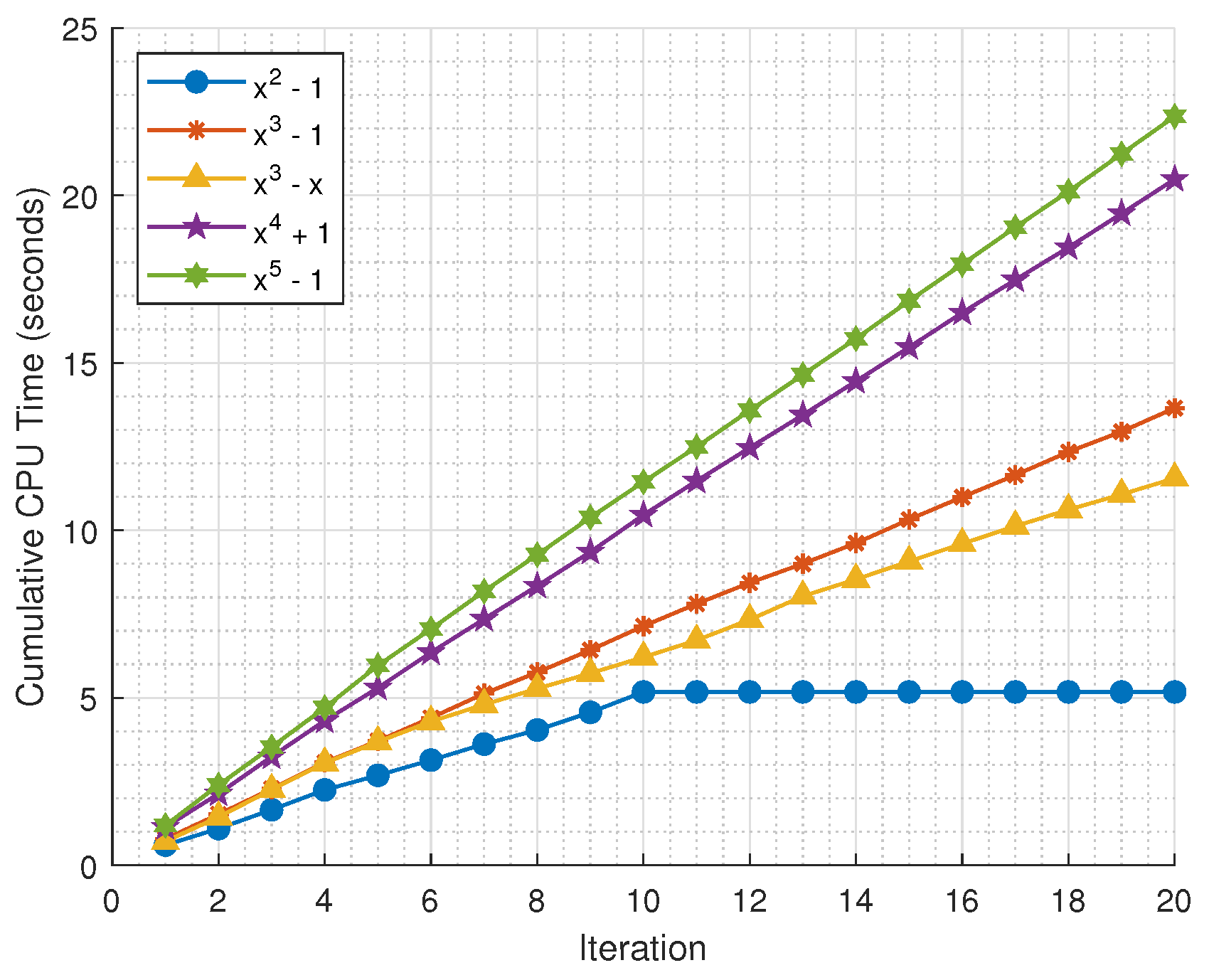
| IT | |||||
|---|---|---|---|---|---|
| 2 | 1.0959 | 1.5226 | 1.4417 | 2.1351 | 2.395 |
| 4 | 2.2509 | 3.0736 | 3.0528 | 4.3157 | 4.7046 |
| 6 | 3.1393 | 4.4026 | 4.2922 | 6.3544 | 7.0545 |
| 8 | 4.0382 | 5.7601 | 5.2743 | 8.3408 | 9.2717 |
| 10 | 5.1752 | 7.1421 | 6.2136 | 10.459 | 11.447 |
| 12 | 5.1752 | 8.4335 | 7.3323 | 12.466 | 13.579 |
| 14 | 5.1752 | 9.6182 | 8.5374 | 14.451 | 15.722 |
| 16 | 5.1752 | 10.994 | 9.6007 | 16.497 | 17.941 |
| 18 | 5.1752 | 12.343 | 10.616 | 18.445 | 20.117 |
| 20 | 5.1752 | 13.649 | 11.555 | 20.482 | 22.357 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, Q.; Rukhsar; Awan, M.U.; Bin-Mohsin, B.; Javed, M.Z.; Ciurdariu, L.; Meftah, B. Bridging Pre-Invex Mappings and Fractional Integrals: A Pathway to Iterative Schemes via Error Boundaries of Maclaurin’s Rule. Fractal Fract. 2024, 8, 734. https://doi.org/10.3390/fractalfract8120734
Liu Q, Rukhsar, Awan MU, Bin-Mohsin B, Javed MZ, Ciurdariu L, Meftah B. Bridging Pre-Invex Mappings and Fractional Integrals: A Pathway to Iterative Schemes via Error Boundaries of Maclaurin’s Rule. Fractal and Fractional. 2024; 8(12):734. https://doi.org/10.3390/fractalfract8120734
Chicago/Turabian StyleLiu, Qi, Rukhsar, Muhammad Uzair Awan, Bandar Bin-Mohsin, Muhammad Zakria Javed, Loredana Ciurdariu, and Badreddine Meftah. 2024. "Bridging Pre-Invex Mappings and Fractional Integrals: A Pathway to Iterative Schemes via Error Boundaries of Maclaurin’s Rule" Fractal and Fractional 8, no. 12: 734. https://doi.org/10.3390/fractalfract8120734
APA StyleLiu, Q., Rukhsar, Awan, M. U., Bin-Mohsin, B., Javed, M. Z., Ciurdariu, L., & Meftah, B. (2024). Bridging Pre-Invex Mappings and Fractional Integrals: A Pathway to Iterative Schemes via Error Boundaries of Maclaurin’s Rule. Fractal and Fractional, 8(12), 734. https://doi.org/10.3390/fractalfract8120734






