Conformal and Non-Minimal Couplings in Fractional Cosmology
Abstract
:1. Introduction
2. Theoretical Framework
2.1. Grünwald–Letnikov Approach
2.2. Riemann–Liouville Approach
2.2.1. Caputo’s Approach
2.2.2. Rule for Successive Fractional Derivatives
2.2.3. Fractional Derivatives as Generalizations of Integer-Order Derivatives
2.2.4. Leibniz’s Rule for the Fractional Derivative of the Product
2.3. Fractional Integral
2.3.1. Liouville Fractional Integral
2.3.2. Riemann Fractional Integral
2.4. Link between Integration and Fractional Differentiation
2.4.1. Liouville Fractional Derivative
2.4.2. Fractional Riemann Derivative
2.4.3. Liouville–Caputo Fractional Derivative
2.4.4. Caputo Fractional Derivative
2.5. Fractional Differential Equations
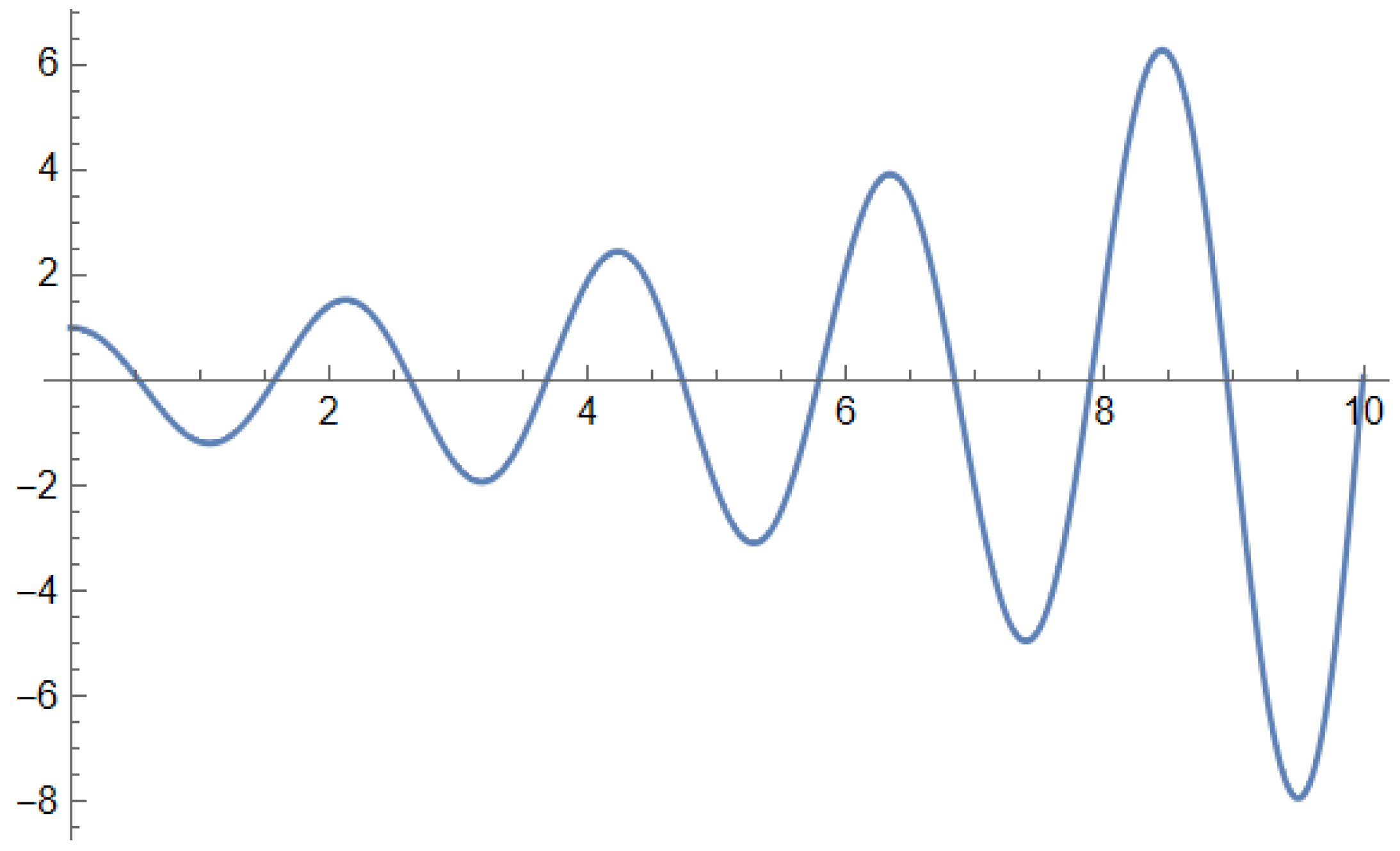
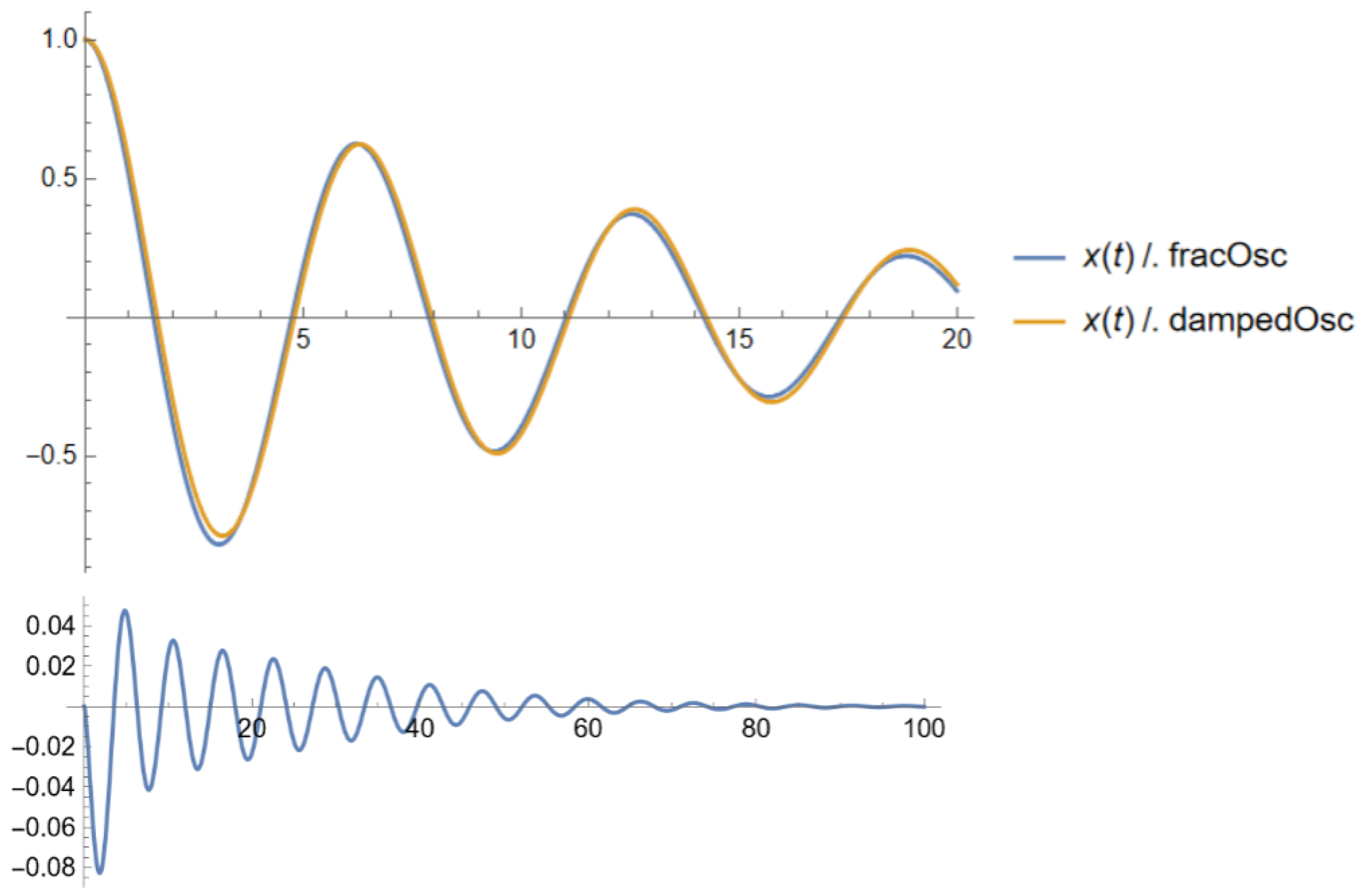
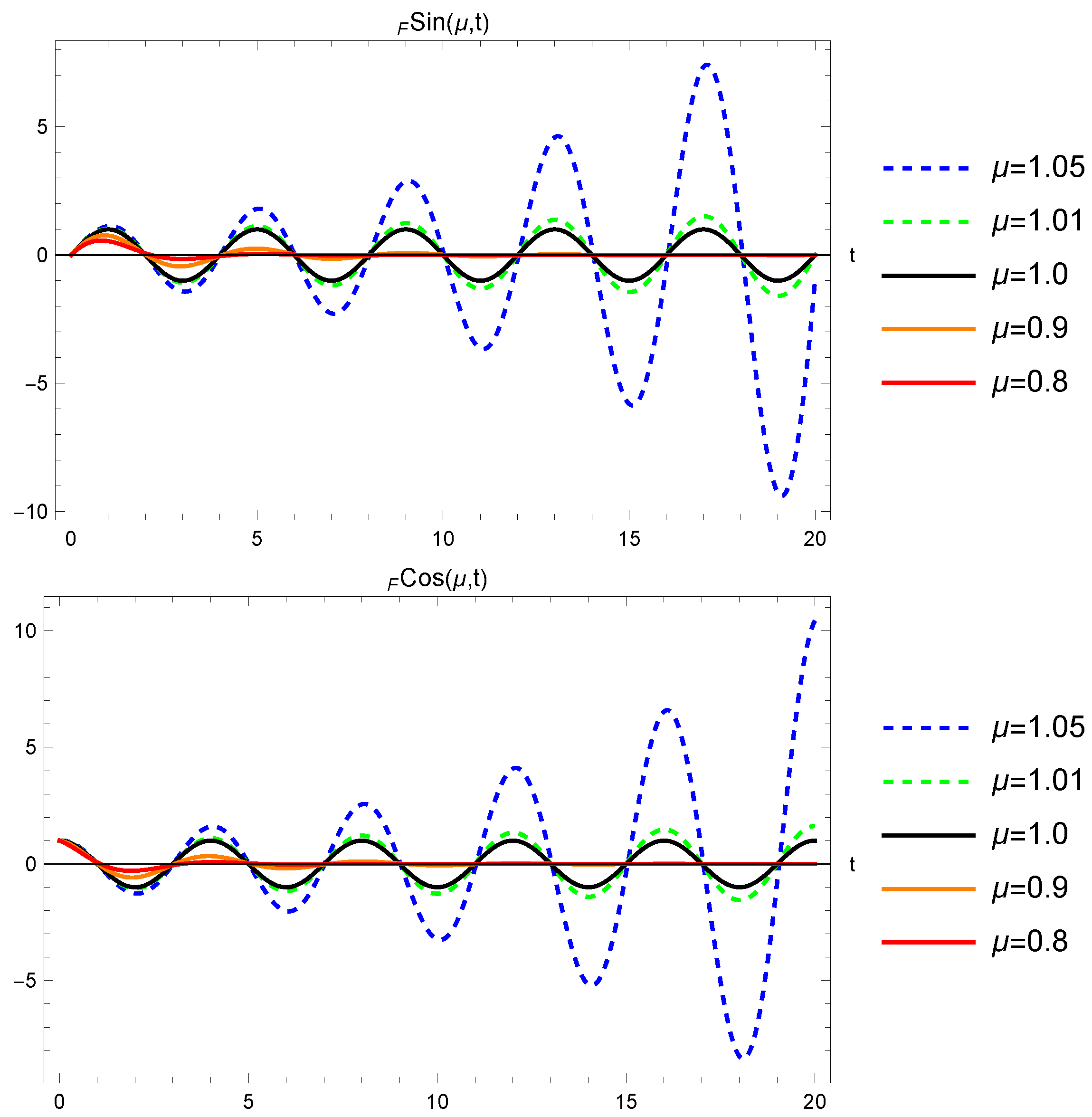
2.6. Fractional Harmonic Oscillator
2.6.1. Harmonic Oscillator According to Fourier
2.6.2. Harmonic Oscillator According to Riemann
2.6.3. Harmonic Oscillator According to Caputo
2.7. q-Deformed Lie Algebras and Fractional Calculus
2.7.1. q-Deformed Lie Algebras
2.7.2. The Fractional q-Deformed Quantum Harmonic Oscillator
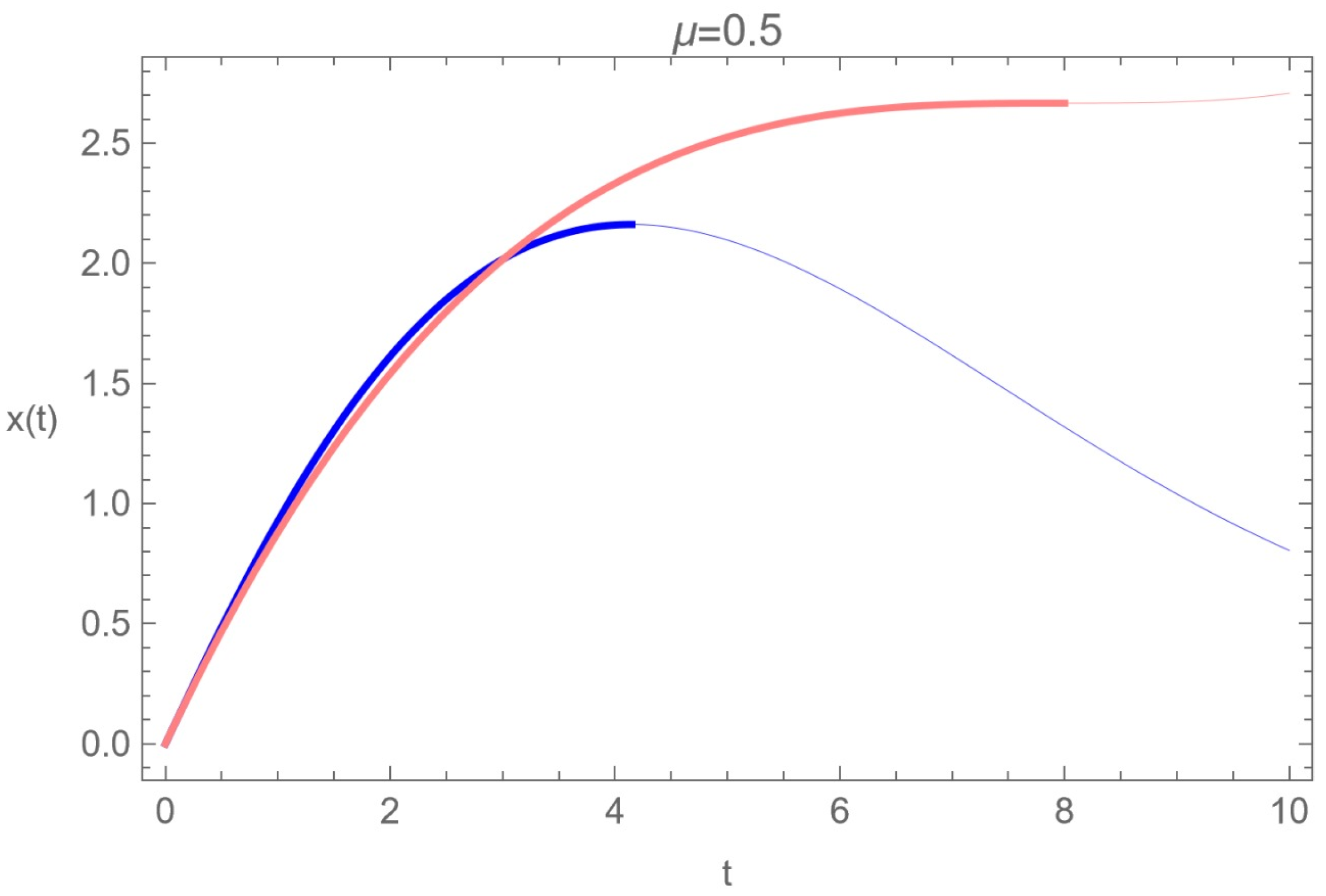
2.8. Fractional Friction
3. Gravity Models with Fractional Derivatives
3.1. Cosmological Model in the Fractional Formulation of Gravity
3.2. Minimal Coupling
3.2.1. Model with Cold Dark Matter
3.2.2. Interpretation of the Fractional Term as a Dark Energy Source
3.2.3. Tests against Cosmological Observations
3.2.4. Dynamical Systems Analysis
- . The eigenvalues are. It is normally hyperbolic with a stable 2D manifold for , an unstable 2D manifold for , or . This point is a saddle for , or , or .
- . The eigenvalues are , indicating that the system is non-hyperbolic.
- , with . The eigenvalues are The conditions are as follows:
- (a)
- Source for .
- (b)
- Sink for or .
- The eigenvalues are This point is a saddle for all and .
- The eigenvalues are. This point is a saddle for all .
- .The eigenvalues are .This point is a saddle for all and .
3.3. Non-Minimal Coupling
3.3.1. Dynamical Systems Analysis
- The set is an invariant set of (244)–(247) whenThat is, when
- (a)
- o
- (b)
- o
- (c)
- o
- (d)
- .
- Taking , we obtain the dynamical system, as follows:Systems (249)–(251) support the following equilibrium points:
- (a)
- , which is a non-hyperbolic critical point curve.
- (b)
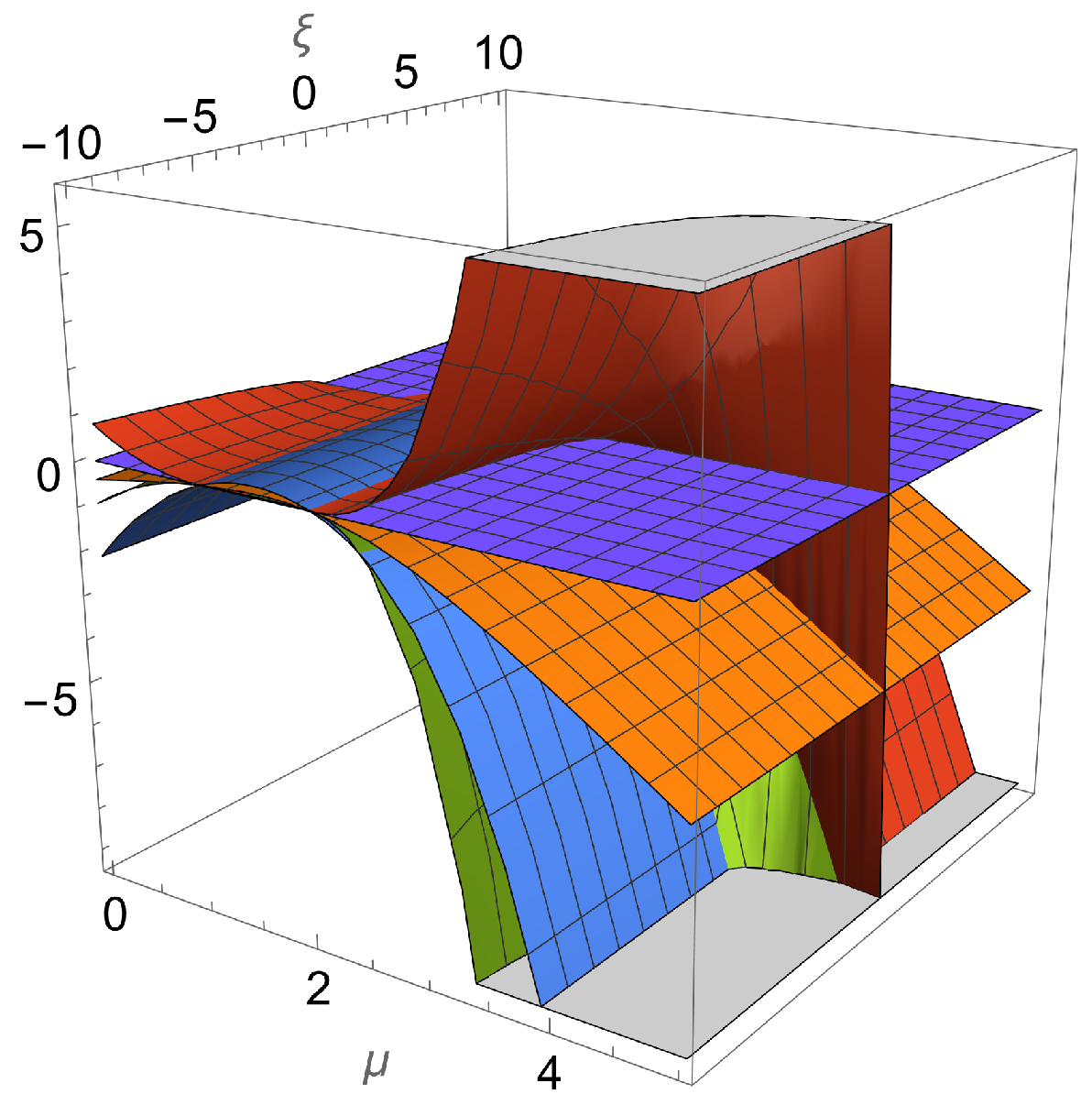
- , where with eigenvalues , where are complicated expressions that depend on and . Since , this point only exists for In Figure 8, the point can show source or saddle behavior.
- (c)
- with eigenvalues , where are complicated expressions that depend on and . In Figure 8, the point is a saddle.
- (d)
- , with eigenvalues , where, again, are complicated expressions that depend on and . Since , this point only exists for In Figure 9, the point can show source or saddle behavior.
- (e)
- , with eigenvalues , where are complicated expressions that depend on and . In Figure 9, the point is a saddle.
3.3.2. Alternative Formulation of the Dynamical System
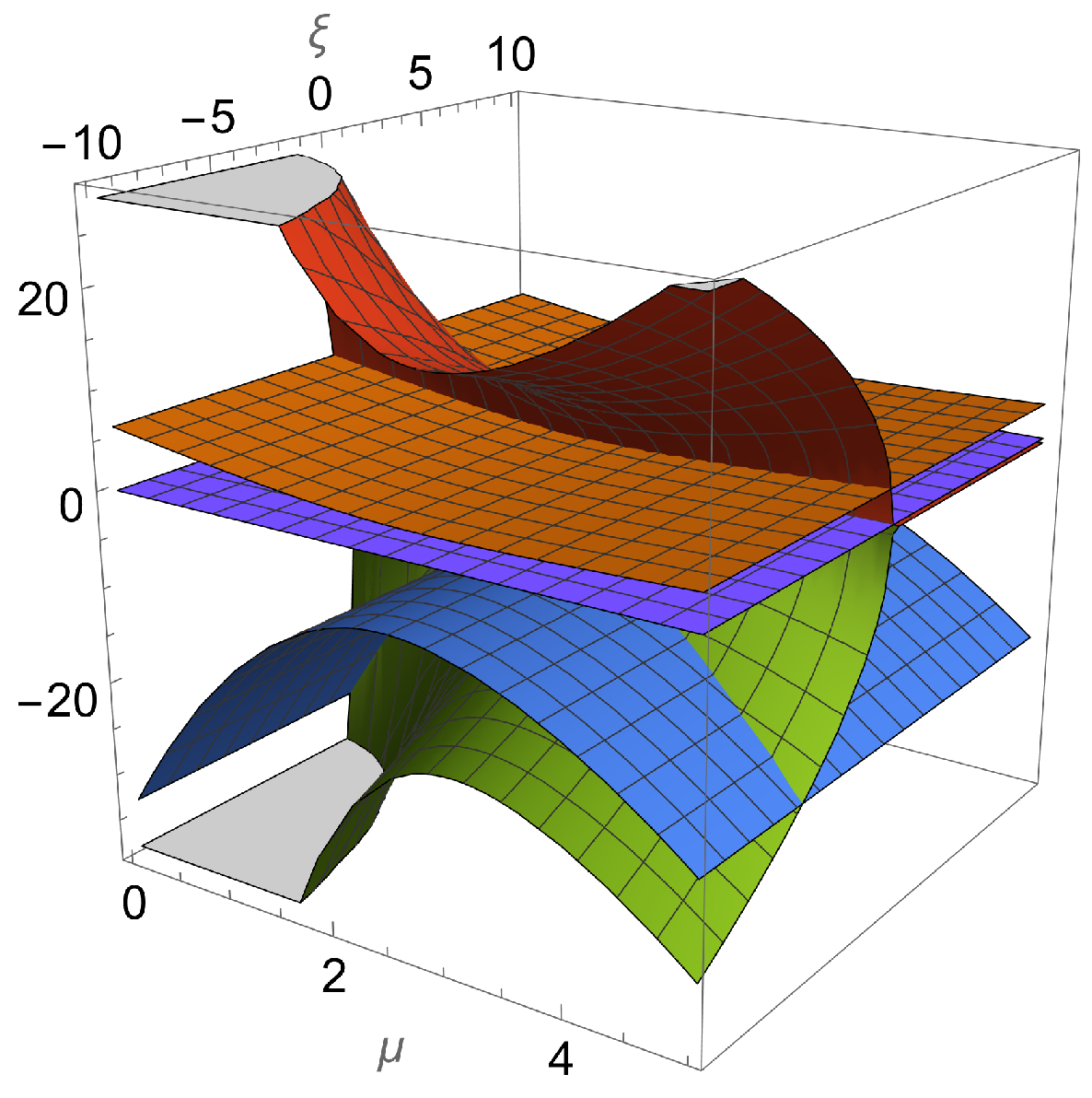
- The line of equilibrium points with coordinates parameterized by . The equation of state parameter is complex infinity. The eigenvalues are as follows: . The curve is a saddle because it has at least two eigenvalues with different signs.
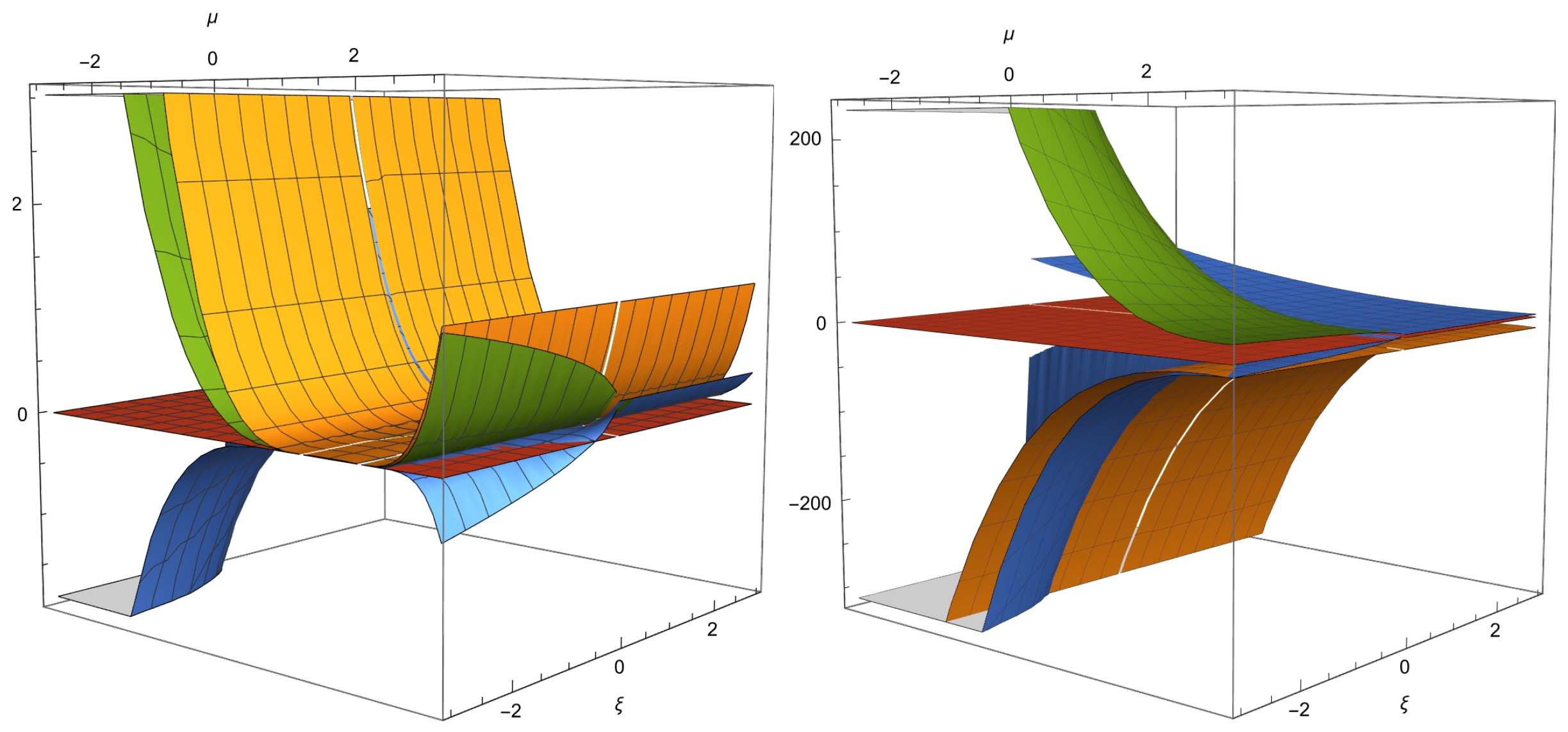
- The equilibrium point, . The equation of state parameter is . The eigenvalues are as follows:,where , and is the positive square root of 17,36114,481). This is a saddle for the following:. This is a source for the following: . This case is discarded under the assumption . Figure 12 presents the real parts of the eigenvalues associated with . This shows that the point is well for , or a saddle (assuming ).
- The equilibrium point . The equation of state parameter is . The eigenvalues are as follows:,where , and is the positive square root of . The point is always saddle, as shown in Figure 13.
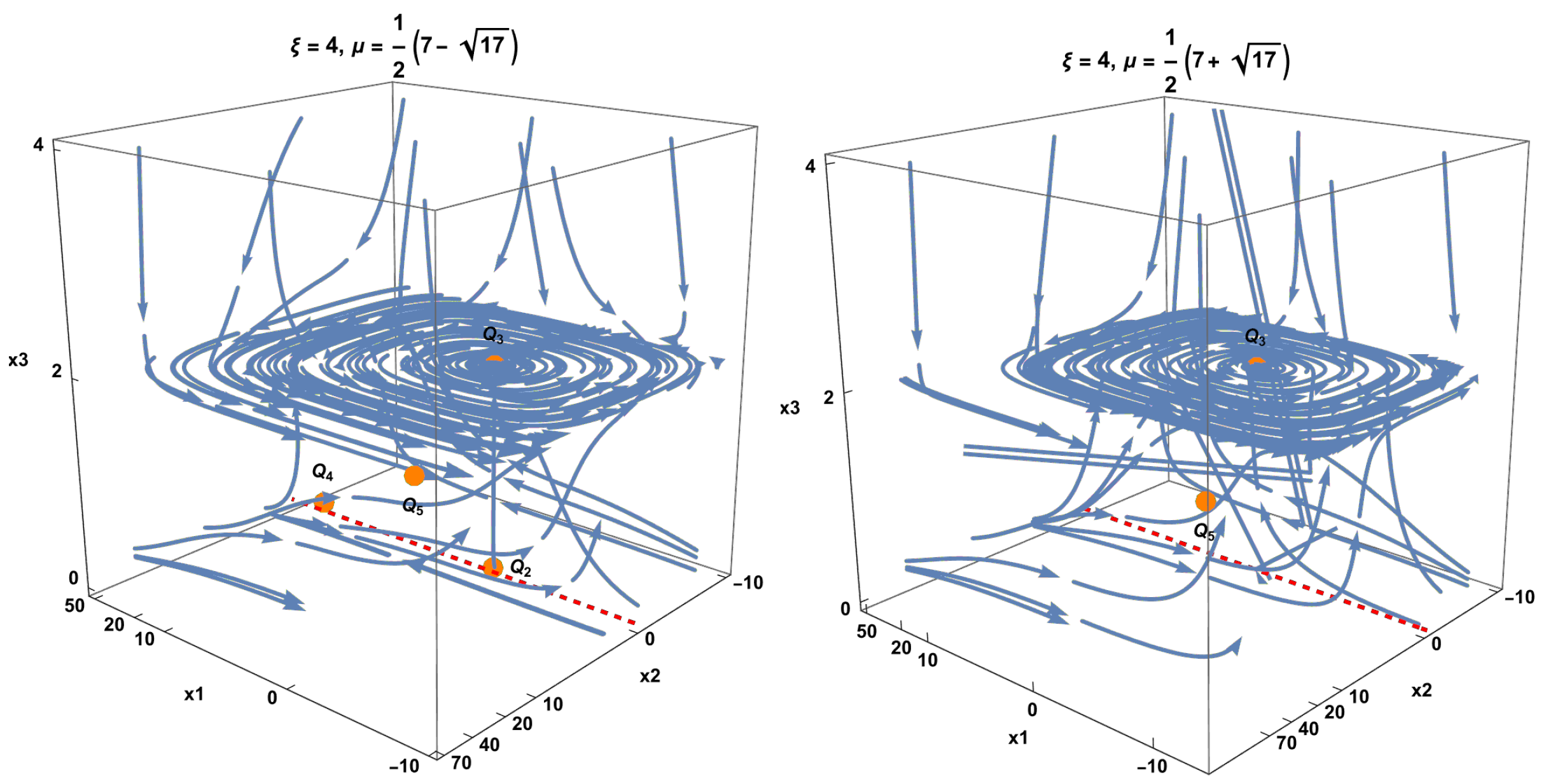
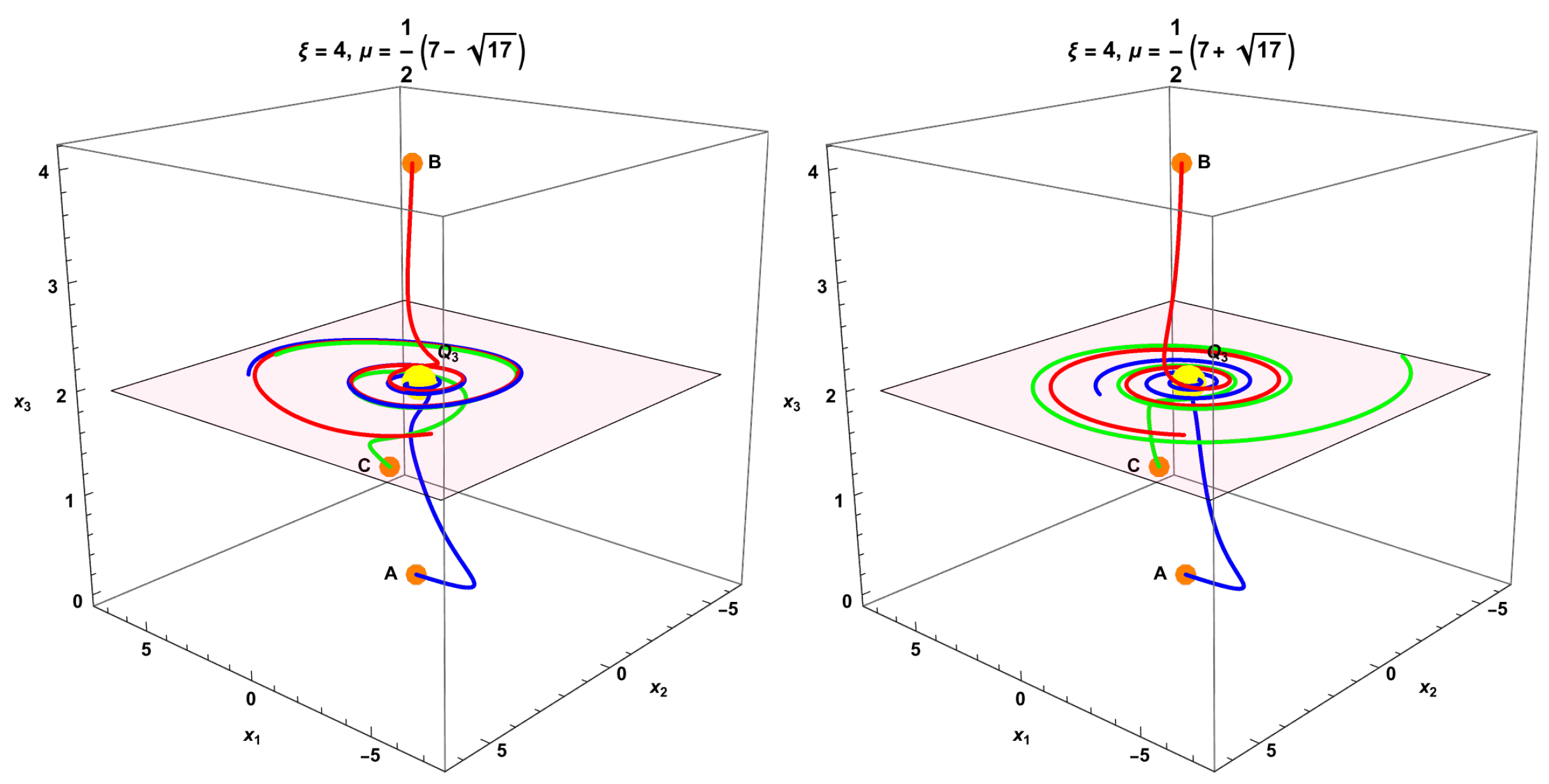
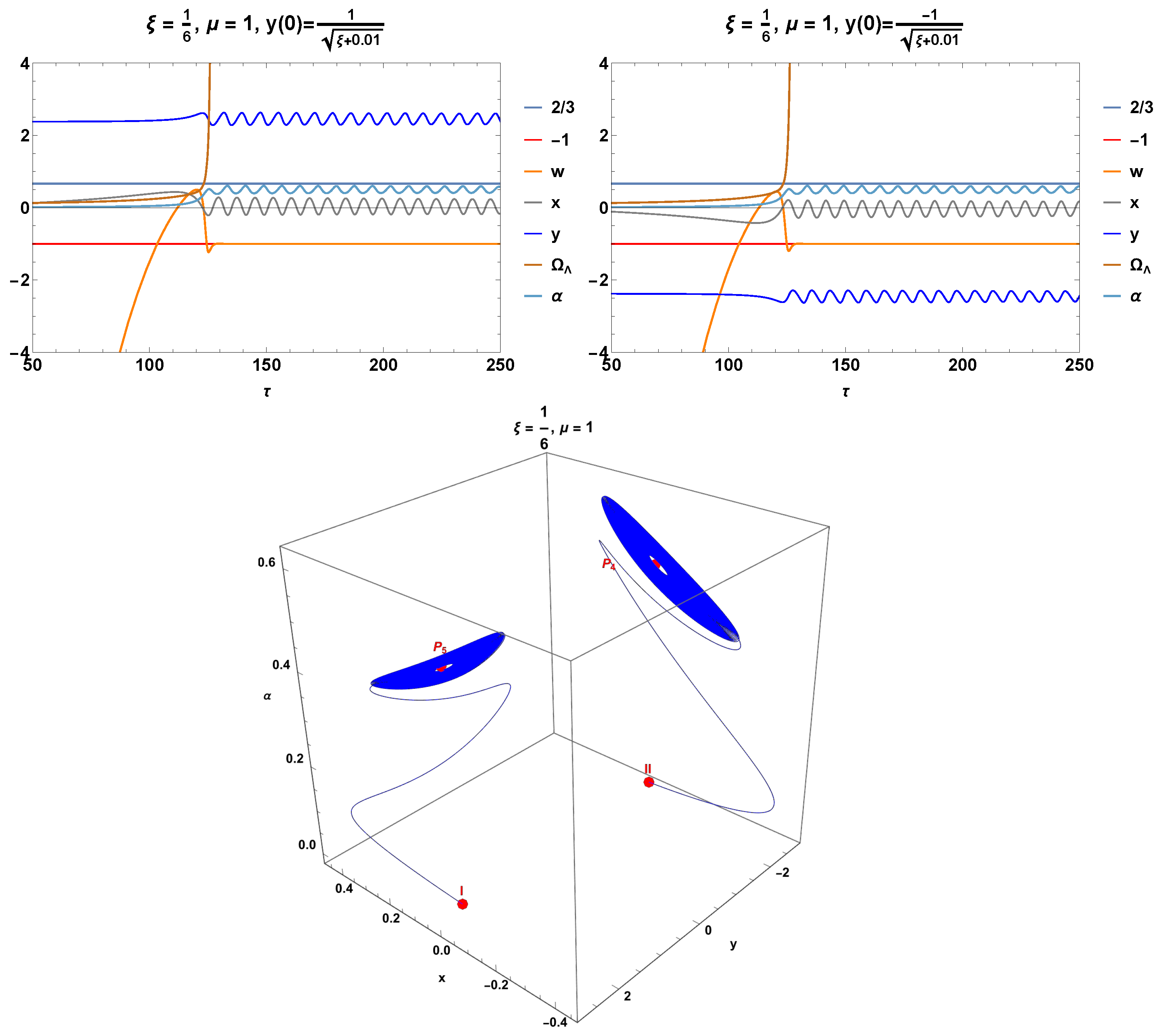
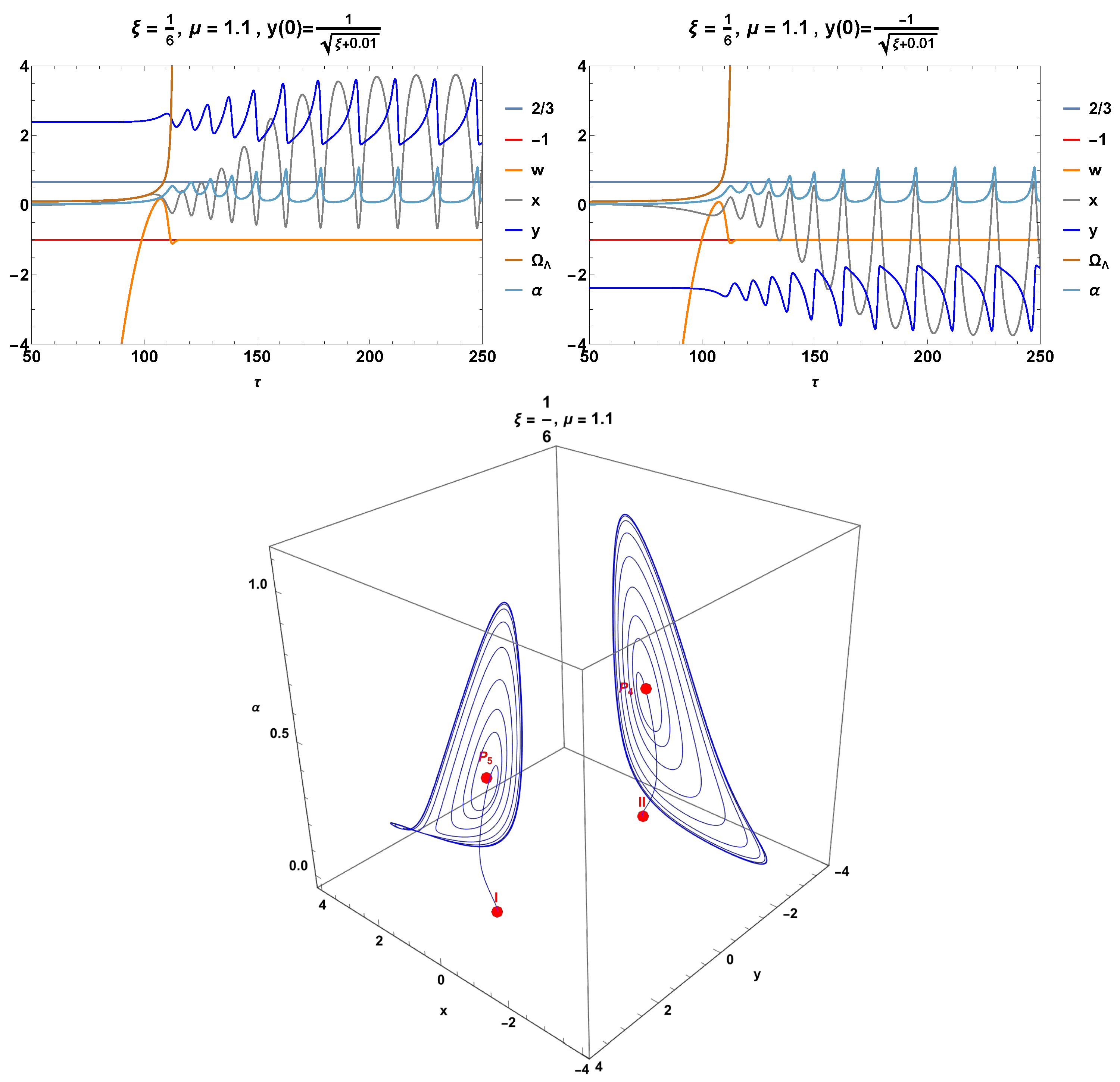
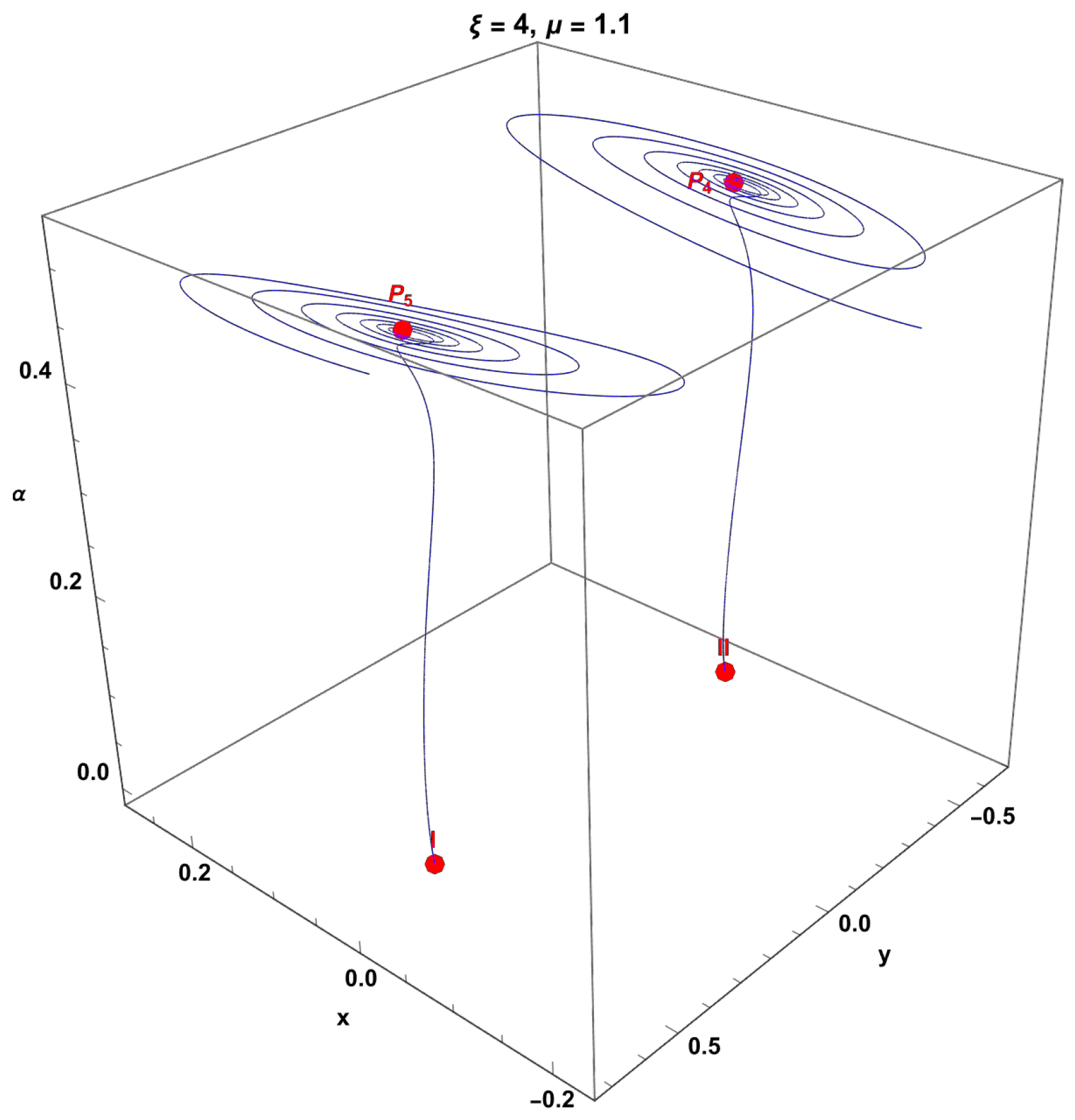
- The equilibrium point . The equation of state parameter is indeterminate. The eigenvalues are. Eigenvalue 1 corresponds to the coordinate . This point is always a saddle when . In the invariant set , they can be attractors if . For , the eigenvalues reduce to , and as shown in the lower panel of Figure 14, we see spiral attractors. The analysis of this invariant set is presented in Section 3.3.3.
- The equilibrium point . The equation of state parameter is indeterminate. The eigenvalues are. Eigenvalue 1 corresponds to the coordinate . This point is always a saddle when . In the invariant set where , they can be attractors if . For , the eigenvalues reduce to , and as shown in the lower panel in Figure 14, are spiral attractors. The analysis of this invariant set is presented in Section 3.3.3.There are additional points that cancel out the numerator and denominator, such as the following:
- The set .
- The line . In both cases, the stability analysis of these point curves will be left for future research since it cannot be implemented with the techniques developed in this paper.
- The line . The eigenvalues arewhen . Therefore, this point is a saddle.
- The point . The eigenvalues arewhen . Therefore, this point is a saddle.
- The set .
- The line . In both cases, the stability analysis of these point curves will be left for future research since it cannot be implemented with the techniques developed in this paper.
- The line . The eigenvalues arewhen . Therefore, this point is a saddle.
- The point . The eigenvalues arewhen . Therefore, this point is a saddle. All have indeterminate parameters of the equation of the state of matter.
- The line .
- The line .
- The line .
- The line . Stability can be determined numerically since the Jacobian matrix has infinite entries.
3.3.3. Invariant Set
- The equilibrium point curve parameterized by . The equation of state parameter is complex infinity. The eigenvalues are. The curve is a saddle because it has at least two eigenvalues with different signs.
- The equilibrium point . There exists () for . The eigenvalues are ,,, where and are the positive square roots of. The critical point is a saddle in its existence interval, as shown in Figure 19.The equation of state parameter is .
- The equilibrium point . The eigenvalues are,,, where and are the positive square roots of the following: . is a sink for .It is a saddle otherwise.The equation of state parameter is .
- The equilibrium point . The eigenvalues are. The equation of state parameter is . The point is a sink for and and becomes a saddle for , as shown in Figure 17.
- The equilibrium point . The eigenvalues are. The equation of state parameter is . The point is a sink for and and is a saddle for , as shown in Figure 17.
- The equilibrium point .
- The equilibrium point . The equation of state parameter is .
- The equilibrium point .
- The equilibrium point . The equation of state parameter is .Due to the complexity of the stability analysis of these four points, it will be left for future research since it cannot be implemented with the techniques developed in this paper.
- The equilibrium points .
- The equilibrium points .
- The equilibrium points .
- The equilibrium points .
3.3.4. Asymptotic Expansions for
3.3.5. Asymptotic Expansions for
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Special Functions
Appendix A.1. Gamma Function
Appendix A.2. Mittag-Leffler Functions
Appendix A.3. Hypergeometric Functions
Appendix A.4. Euler Polynomials: Incomplete Riemann or Hurwitz Zeta Function
References
- Bandyopadhyay, B.; Kamal, S. Stabilization and Control of Fractional Order Systems: A Sliding Mode Approach; Lecture Notes in Electrical Engineering; Springer International Publishing: Berlin/Heidelberg, Germany, 2014. [Google Scholar]
- Herrmann, R. Fractional Calculus: An Introduction for Physicists, 2nd ed.; World Scientific Publishing Company: Singapore, 2014. [Google Scholar]
- Klafter, J.; Lim, S.C.; Metzler, R. Fractional Dynamics: Recent Advances; World Scientific: Singapore, 2012. [Google Scholar]
- Lorenzo, C.F.; Hartley, T.T. The Fractional Trigonometry: With Applications to Fractional Differential Equations and Science; Wiley: Hoboken, NJ, USA, 2016. [Google Scholar]
- Malinowska, A.B.; Odzijewicz, T.; Torres, D.F.M. Advanced Methods in the Fractional Calculus of Variations; Springer briefs in applied sciences and technology; Springer International Publishing: Berlin/Heidelberg, Germany, 2015. [Google Scholar]
- Monje, C.A.; Chen, Y.Q.; Vinagre, B.M.; Xue, D.; Feliu-Batlle, V. Fractional-Order Systems and Controls: Fundamentals and Applications; Advances in Industrial Control; Springer: London, UK, 2010. [Google Scholar]
- Padula, F.; Visioli, A. Advances in Robust Fractional Control; Springer International Publishing: Berlin/Heidelberg, Germany, 2014. [Google Scholar]
- Tarasov, V.E. Review of some promising fractional physical models. Int. J. Mod. Phys. B 2013, 27, 1330005. [Google Scholar] [CrossRef]
- Tarasov, V.E. Applications in Physics, Part A; De Gruyter Reference; De Gruyter: Berlin, Germany, 2019. [Google Scholar]
- Kilbas, A.; Srivastava, H.; Trujillo, J. Theory and applications of fractional differential equations. In North Holland Mathematical Studies; Elsevier: Amsterdam, The Netherlands, 2006; Volume 204. [Google Scholar]
- Podlubny, I. Fractional Differential Equations; Elsevier: Amsterdam, The Netherlands, 1998; Volume 198. [Google Scholar]
- Uchaikin, V.V. Fractional Derivatives for Physicists and Engineers; Higher Education Press: Beijing, China, 2013. [Google Scholar]
- Wheatcraft, S.W.; Meerschaert, M.M. Fractional conservation of mass. Adv. Water Resour. 2008, 31, 1377–1381. [Google Scholar] [CrossRef]
- Oldham, K.B. Signal-independent electroanalytical method. Anal. Chem. 1972, 44, 196–198. [Google Scholar] [CrossRef]
- Pospíšil, L.; Hromadová, M.; Sokolová, R.; Lanza, C. Kinetics of radical dimerization. Simple evaluation of rate constant from convolution voltammetry and faradaic phase angle data. Electrochim. Acta 2019, 300, 284–289. [Google Scholar] [CrossRef]
- Atangana, A.; Bildik, N. The use of fractional order derivative to predict the groundwater flow. Math. Probl. Eng. 2013, 2013, 543026. [Google Scholar] [CrossRef]
- Atangana, A.; Vermeulen, P.D. Analytical solutions of a space-time fractional derivative of groundwater flow equation. In Abstract and Applied Analysis; Hindawi Limited: London, UK, 2014; Volume 2014, pp. 1–11. [Google Scholar]
- Benson, D.A.; Wheatcraft, S.W.; Meerschaert, M.M. Application of a fractional advection-dispersion equation. Water Resour. Res. 2000, 36, 1403–1412. [Google Scholar] [CrossRef]
- Benson, D.A.; Wheatcraft, S.W.; Meerschaert, M.M. The fractional-order governing equation of Lévy motion. Water Resour. Res. 2000, 36, 1413–1423. [Google Scholar] [CrossRef]
- Benson, D.A.; Schumer, R.; Meerschaert, M.M.; Wheatcraft, S.W. Fractional dispersion, Lévy motion, and the MADE tracer tests. Transp. Porous Media 2001, 42, 211–240. [Google Scholar] [CrossRef]
- Metzler, R.; Klafter, J. The random walk’s guide to anomalous diffusion: A fractional dynamics approach. Phys. Rep. 2000, 339, 1–77. [Google Scholar] [CrossRef]
- Mainardi, F.; Luchko, Y.; Pagnini, G. The fundamental solution of the space-time fractional diffusion equation. arXiv 2007, arXiv:cond-mat/0702419. [Google Scholar]
- Atangana, A.; Kilicman, A. On the generalized mass transport equation to the concept of variable fractional derivative. Math. Probl. Eng. 2014, 2014, 542809. [Google Scholar] [CrossRef]
- Gorenflo, R.; Mainardi, F. Fractional diffusion processes: Probability distributions and continuous time random walk. In Processes with Long-Range Correlations: Theory and Applications; Springer: Berlin/Heidelberg, Germany, 2003; pp. 148–166. [Google Scholar]
- Colbrook, M.J.; Ma, X.; Hopkins, P.F.; Squire, J. Scaling laws of passive-scalar diffusion in the interstellar medium. Mon. Not. R. Astron. Soc. 2017, 467, 2421–2429. [Google Scholar] [CrossRef]
- Mainardi, F. Fractional Calculus and Waves in Linear Viscoelasticity: An Introduction to Mathematical Models; World Scientific: Singapore, 2022. [Google Scholar]
- Laskin, N. Fractional quantum mechanics. Phys. Rev. E 2000, 62, 3135. [Google Scholar] [CrossRef]
- Laskin, N. Fractional schrödinger equation. Phys. Rev. E 2002, 66, 056108. [Google Scholar] [CrossRef] [PubMed]
- Bhrawy, A.H.; Zaky, M. An improved collocation method for multi-dimensional space–time variable-order fractional Schrödinger equations. Appl. Numer. Math. 2017, 111, 197–218. [Google Scholar] [CrossRef]
- Lim, S.C. Fractional derivative quantum fields at positive temperature. Phys. A 2006, 363, 269–281. [Google Scholar] [CrossRef]
- Lim, S.C.; Eab, C.H. Fractional Quantum Fields; De Gruyter: Berlin, Germany, 2019; pp. 237–256. [Google Scholar]
- Moniz, P.V.; Jalalzadeh, S. From Fractional Quantum Mechanics to Quantum Cosmology: An Overture. Mathematics 2020, 8, 313. [Google Scholar] [CrossRef]
- Rasouli, S.M.M.; Jalalzadeh, S.; Moniz, P.V. Broadening quantum cosmology with a fractional whirl. Mod. Phys. Lett. A 2021, 36, 2140005. [Google Scholar] [CrossRef]
- Moniz, P.V.; Jalalzadeh, S. Challenging Routes in Quantum Cosmology; World Scientific Publishing: Singapore, 2020. [Google Scholar]
- Calcagni, G.; Kuroyanagi, S. Stochastic gravitational-wave background in quantum gravity. J. Cosmol. Astropart. Phys. 2021, 3, 019. [Google Scholar] [CrossRef]
- El-Nabulsi, R.A. Fractional derivatives generalization of Einstein’s field equations. Indian J. Phys. 2013, 87, 195–200. [Google Scholar] [CrossRef]
- El-Nabulsi, R.A. Non-minimal coupling in fractional action cosmology. Indian J. Phys. 2013, 87, 835–840. [Google Scholar] [CrossRef]
- Jalalzadeh, S.; da Silva, F.R.; Moniz, P.V. Prospecting black hole thermodynamics with fractional quantum mechanics. Eur. Phys. J. C 2021, 81, 632. [Google Scholar] [CrossRef]
- Vacaru, S.I. Fractional Dynamics from Einstein Gravity, General Solutions, and Black Holes. Int. J. Theor. Phys. 2012, 51, 1338–1359. [Google Scholar] [CrossRef]
- Vacaru, S.I. Fractional Nonholonomic Ricci Flows. Chaos Solitons Fractals 2012, 45, 1266–1276. [Google Scholar] [CrossRef]
- Rami, E.N.A. Fractional dynamics, fractional weak bosons masses and physics beyond the standard model. Chaos Solitons Fractals 2009, 41, 2262–2270. [Google Scholar] [CrossRef]
- Debnath, U.; Jamil, M.; Chattopadhyay, S. Fractional Action Cosmology: Emergent, Logamediate, Intermediate, Power Law Scenarios of the Universe and Generalized Second Law of Thermodynamics. Int. J. Theor. Phys. 2012, 51, 812–837. [Google Scholar] [CrossRef]
- Debnath, U.; Chattopadhyay, S.; Jamil, M. Fractional action cosmology: Some dark energy models in emergent, logamediate, and intermediate scenarios of the universe. J. Theor. Appl. Phys. 2013, 7, 25. [Google Scholar] [CrossRef]
- El-Nabulsi, R.A. Cosmology with a fractional action principle. Rom. Rep. Phys. 2007, 59, 763–771. [Google Scholar]
- El-Nabulsi, R.A. Fractional Lagrangian Formulation of General Relativity and Emergence of Complex, Spinorial and Noncommutative Gravity. Int. J. Geom. Methods Mod. Phys. 2009, 6, 25–76. [Google Scholar] [CrossRef]
- Jamil, M.; Momeni, D.; Rashid, M.A. Fractional Action Cosmology with Power Law Weight Function. J. Phys. Conf. Ser. 2012, 354, 012008. [Google Scholar] [CrossRef]
- Shchigolev, V.K. Testing Fractional Action Cosmology. Eur. Phys. J. Plus 2016, 131, 256. [Google Scholar] [CrossRef]
- Giusti, A. MOND-like Fractional Laplacian Theory. Phys. Rev. D 2020, 101, 124029. [Google Scholar] [CrossRef]
- Rami, E.-N.A. Fractional action oscillating phantom cosmology with conformal coupling. Eur. Phys. J. Plus 2015, 130, 102. [Google Scholar] [CrossRef]
- Calcagni, G. Fractal universe and quantum gravity. Phys. Rev. Lett. 2010, 104, 251301. [Google Scholar] [CrossRef] [PubMed]
- Calcagni, G. Quantum field theory, gravity and cosmology in a fractal universe. J. High Energy Phys. 2010, 3, 120. [Google Scholar] [CrossRef]
- Calcagni, G. Multi-scale gravity and cosmology. J. Cosmol. Astropart. Phys. 2013, 12, 041. [Google Scholar] [CrossRef]
- Calcagni, G. Quantum scalar field theories with fractional operators. Class. Quantum Gravity 2021, 38, 165006. [Google Scholar] [CrossRef]
- Calcagni, G. Classical and quantum gravity with fractional operators. Class. Quantum Gravity 2021, 38, 165005, Erratum in Class. Quantum Gravity 2021, 38, 169601. [Google Scholar] [CrossRef]
- Calcagni, G.; De Felice, A. Dark energy in multifractional spacetimes. Phys. Rev. D 2020, 102, 103529. [Google Scholar] [CrossRef]
- Calcagni, G.; Kuroyanagi, S.; Marsat, S.; Sakellariadou, M.; Tamanini, N.; Tasinato, G. Quantum gravity and gravitational-wave astronomy. J. Cosmol. Astropart. Phys. 2019, 10, 012. [Google Scholar] [CrossRef]
- Calcagni, G.; Kuroyanagi, S.; Tsujikawa, S. Cosmic microwave background and inflation in multi-fractional spacetimes. J. Cosmol. Astropart. Phys. 2016, 8, 039. [Google Scholar] [CrossRef]
- Vacaru, S.I. New Classes of Off-Diagonal Cosmological Solutions in Einstein Gravity. Int. J. Theor. Phys. 2010, 49, 2753–2776. [Google Scholar] [CrossRef]
- Roberts, M.D. fractional derivative Cosmology. SOP Trans. Theor. Phys. 2014, 1, 310. [Google Scholar] [CrossRef]
- Shchigolev, V.K. Cosmological Models with fractional derivatives and Fractional Action Functional. Commun. Theor. Phys. 2011, 56, 389–396. [Google Scholar] [CrossRef]
- Shchigolev, V.K. Cosmic Evolution in Fractional Action Cosmology. Discontinuity Nonlinearity Complex. 2013, 2, 115–123. [Google Scholar] [CrossRef]
- Shchigolev, V.K. Fractional Einstein-Hilbert Action Cosmology. Mod. Phys. Lett. A 2013, 28, 1350056. [Google Scholar] [CrossRef]
- Shchigolev, V.K. Fractional-order derivatives in cosmological models of accelerated expansion. Mod. Phys. Lett. A 2021, 36, 2130014. [Google Scholar] [CrossRef]
- García-Aspeitia, M.A.; Fernandez-Anaya, G.; Hernández-Almada, A.; Leon, G.; Magaña, J. Cosmology under the fractional calculus approach. Mon. Not. R. Astron. Soc. 2022, 517, 4813–4826. [Google Scholar] [CrossRef]
- Jalalzadeh, S.; Costa, E.W.O.; Moniz, P.V. de Sitter fractional quantum cosmology. Phys. Rev. D 2022, 105, L121901. [Google Scholar] [CrossRef]
- Landim, R.G. Fractional dark energy: Phantom behavior and negative absolute temperature. Phys. Rev. D 2021, 104, 103508. [Google Scholar] [CrossRef]
- Landim, R.G. Fractional dark energy. Phys. Rev. D 2021, 103, 083511. [Google Scholar] [CrossRef]
- Micolta-Riascos, B.; Millano, A.D.; Leon, G.; Erices, C.; Paliathanasis, A. Revisiting fractional cosmology. Fractal Fract. 2023, 7, 149. [Google Scholar] [CrossRef]
- González, E.; Leon, G.; Fernandez-Anaya, G. Exact solutions and cosmological constraints in fractional cosmology. Fractal Fract. 2023, 7, 368. [Google Scholar] [CrossRef]
- Leon, G.; García-Aspeitia, M.A.; Fernandez-Anaya, G.; Hernández-Almada, A.; Magaña, J.; González, E. Cosmology under the fractional calculus approach: A possible H0 tension resolution? PoS 2023, CORFU2022, 248. [Google Scholar] [CrossRef]
- Wolfram Research. Mathematica; Version 13.3; Wolfram Research: Champaign, IL, USA, 2023. [Google Scholar]
- West, B.J. Fractional Calculus and the Future of Science. Entropy 2021, 23, 1566. [Google Scholar] [CrossRef] [PubMed]
- Stanislavsky, A. Fractional oscillator. Phys. Rev. E 2004, 70, 051103. [Google Scholar] [CrossRef]
- Arraut, I.; Segovia, C. A q-deformation of the Bogoliubov transformations. Phys. Lett. A 2018, 382, 464–466. [Google Scholar] [CrossRef]
- Hou, B.Y.; Xu, L.C. The Hopf algebraic structure of q-deformed Heisenberg algebra when q is a root of unity. Commun. Theor. Phys. 1995, 24, 481. [Google Scholar] [CrossRef]
- Bonatsos, D.; Daskaloyannis, C. Quantum groups and their applications in nuclear physics. Prog. Part. Nucl. Phys. 1999, 43, 537–618. [Google Scholar] [CrossRef]
- Barrientos, E.; Mendoza, S.; Padilla, P. Extending Friedmann equations using fractional derivatives using a Last Step Modification technique: The case of a matter dominated accelerated expanding Universe. Symmetry 2021, 13, 174. [Google Scholar] [CrossRef]
- Frederico, G.S.F.; Torres, D.F.M. Necessary optimality conditions for fractional action-like problems with intrinsic and observer times. WSEAS Trans. Math. 2008, 7, 6–11. [Google Scholar]
- Agrawal, O.P. Fractional variational calculus in terms of Riesz fractional derivatives. J. Phys. A Math. Theor. 2007, 40, 6287. [Google Scholar] [CrossRef]
- Baleanu, D.; Muslih, S. Lagrangian formulation of classical fields within Riemann-Liouville fractional derivatives. Phys. Scr. 2005, 72, 119–121. [Google Scholar] [CrossRef]
- Baleanu, D.; Trujillo, J. A new method of finding the fractional Euler-Lagrange and Hamilton equations within caputo fractional derivatives. Commun. Nonlinear Sci. Numer. Simul. 2010, 15, 1111–1115. [Google Scholar] [CrossRef]
- El-Nabulsi, R.A.; Torres, D.F. Fractional action-like variational problems. J. Math. Phys. 2008, 49, 053521. [Google Scholar] [CrossRef]
- Odzijewicz, T.; Malinowska, A.; Torres, D. Generalized fractional calculus with applications to the calculus of variations. Comput. Math. Appl. 2012, 64, 3351–3366. [Google Scholar] [CrossRef]
- Odzijewicz, T.; Malinowska, A.; Torres, D. Noether’s theorem for fractional variational problems of variable order. Open Phys. 2013, 11, 691–701. [Google Scholar] [CrossRef]
- Odzijewicz, T.; Malinowska, A.; Torres, D. Variable order fractional variational calculus for double integrals. In Proceedings of the 2012 IEEE 51st IEEE Conference on Decision and Control (CDC), Maui, HI, USA, 10–13 December 2012; IEEE: Piscataway, NJ, USA, 2013; pp. 6873–6878. [Google Scholar]
- Hernández-Almada, A.; Leon, G.; Magaña, J.; García-Aspeitia, M.A.; Motta, V. Generalized Emergent Dark Energy: Observational Hubble data constraints and stability analysis. Mon. Not. R. Astron. Soc. 2020, 497, 1590–1602. [Google Scholar] [CrossRef]
- Hernández-Almada, A.; Leon, G.; Magaña, J.; García-Aspeitia, M.A.; Motta, V.; Saridakis, E.N.; Yesmakhanova, K. Kaniadakis-holographic dark energy: Observational constraints and global dynamics. Mon. Not. R. Astron. Soc. 2022, 511, 4147–4158. [Google Scholar] [CrossRef]
- Hernández-Almada, A.; Leon, G.; Magaña, J.; García-Aspeitia, M.A.; Motta, V.; Saridakis, E.N.; Yesmakhanova, K.; Millano, A.D. Observational constraints and dynamical analysis of Kaniadakis horizon-entropy cosmology. Mon. Not. R. Astron. Soc. 2021, 512, 5122–5134. [Google Scholar] [CrossRef]
- Leon, G.; Magaña, J.; Hernández-Almada, A.; García-Aspeitia, M.A.; Verdugo, T.; Motta, V. Barrow Entropy Cosmology: An observational approach with a hint of stability analysis. J. Cosmol. Astropart. Phys. 2021, 12, 032. [Google Scholar] [CrossRef]
- Di Valentino, E.; Mena, O.; Pan, S.; Visinelli, L.; Yang, W.; Melchiorri, A.; Mota, D.F.; Riess, A.G.; Silk, J. In the realm of the Hubble tension—A review of solutions. Class. Quantum Gravity 2021, 38, 153001. [Google Scholar] [CrossRef]
- Efstathiou, G. To H0 or not to H0? Mon. Not. R. Astron. Soc. 2021, 505, 3866–3872. [Google Scholar] [CrossRef]
- Aghanim, N.; Akrami, Y.; Ashdown, M.; Aumont, J.; Baccigalupi, C.; Ballardini, M.; Banday, A.J.; Barreiro, R.B.; Bartolo, N.; Basak, S.; et al. Planck 2018 results. VI. Cosmological parameters. Astron. Astrophys. 2020, 641, A6, Erratum in Astron. Astrophys. 2021, 652, C4. [Google Scholar]
- Carroll, S. Addison-Wesley. Spacetime and Geometry: An Introduction to General Relativity; Addison Wesley: Boston, MA, USA, 2004. [Google Scholar]
- Carroll, S.M. Spacetime and Geometry; Cambridge University Press: Cambridge, UK, 2019. [Google Scholar]
- Wald, R.M. General Relativity; University of Chicago Press: Chicago, IL, USA, 2010. [Google Scholar]
- Foreman-Mackey, D.; Hogg, D.W.; Lang, D.; Goodman, J. emcee: The MCMC hammer. Publ. Astron. Soc. Pac. 2013, 125, 306–312. [Google Scholar] [CrossRef]
- Tavakol, R. Introduction to Dynamical Systems, Ch 4. Part One; Cambridge University Press: Cambridge, UK, 1997; pp. 84–98. [Google Scholar]
- Wainwright, J.; Ellis, G.F.R. (Eds.) Dynamical Systems in Cosmology; Cambridge University Press: Cambridge, UK, 1997. [Google Scholar]
- Perko, L. Differential Equations and Dynamical Systems, 3rd ed.; Springer: Berlin/Heidelberg, Germany, 2001. [Google Scholar]
- Coley, A. Dynamical Systems and Cosmology; Kluwer: Dordrecht, The Netherlands, 2003; Volume 291. [Google Scholar]
- Hirsch, M.W.; Smale, S.; Devaney, R. Differential Equations, Dynamical Systems, and An Introduction to Chaos; Academic Press: London, UK; San Diego, CA, USA, 2004. [Google Scholar]
- Wiggins, S. Introduction to Applied Nonlinear Dynamical Systems and Chaos; Texts in Applied Mathematics; Springer: Berlin/Heidelberg, Germany, 2006. [Google Scholar]
- Berglund, N.; Gentz, B.B. Noise-Induced Phenomena in Slow-Fast Dynamical Systems; Series: Probability and Applications; Springer: London, UK, 2006. [Google Scholar]
- Fenichel, N. Geometric singular perturbation theory for ordinary differential equations. J. Differ. Equ. 1979, 31, 53–98. [Google Scholar] [CrossRef]
- Fusco, G.; Hale, J.K. Slow-motion manifolds, dormant instability, and singular perturbations. J. Dyn. Differ. Equ. 1989, 1, 75–94. [Google Scholar] [CrossRef]
- Dumortier, F.; Roussarie, R. Canard Cycles and Center Manifolds. Mem. Am. Math. Soc. 1996, 121, 577. [Google Scholar] [CrossRef]
- Holmes, M.H. Introduction to Perturbation Methods; Springer Science+Business Media: New York, NY, USA, 2013; ISBN 978-1-4614-5477-9. [Google Scholar] [CrossRef]
- Kevorkian, J.; Cole, J.D. Perturbation Methods in Applied Mathematics; Applied Mathematical Sciences Series; Springer: New York, NY, USA, 1981; Volume 34, ISBN 978-1-4757-4213-8. [Google Scholar] [CrossRef]
- Verhulst, F. Methods and Applications of Singular Perturbations: Boundary Layers and Multiple Timescale Dynamics; Springer: New York, NY, USA, 2000; ISBN 978-0-387-22966-9. [Google Scholar] [CrossRef]
- Popp, K. Non-smooth mechanical systems. J. Appl. Math. Mech. 2000, 64, 765–772. [Google Scholar] [CrossRef]
- Abramowitz, M.; Stegun, I.A. Handbook of Mathematical Functions; Dover Publications: New York, NY, USA, 1965; Volume 361. [Google Scholar]





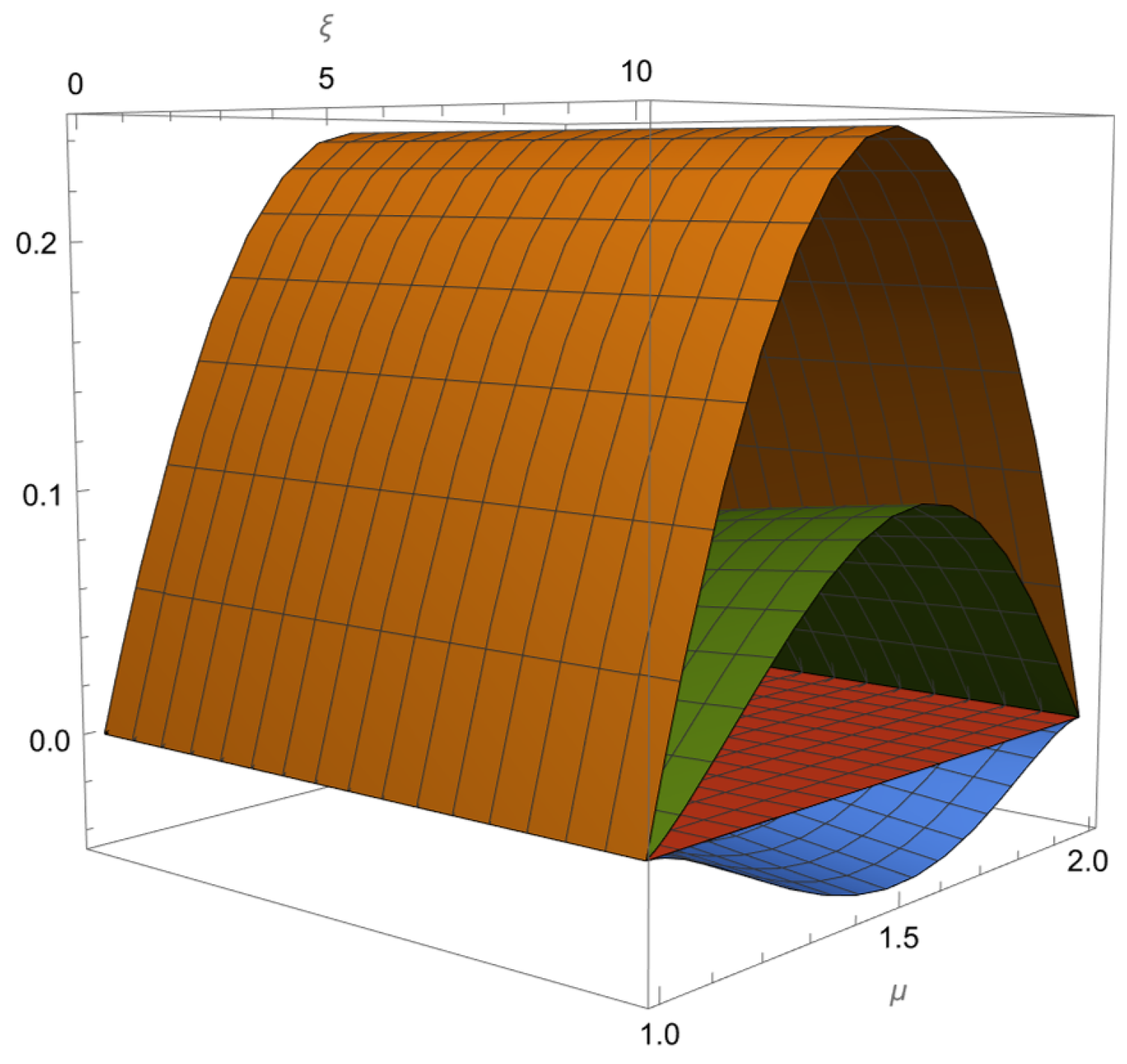
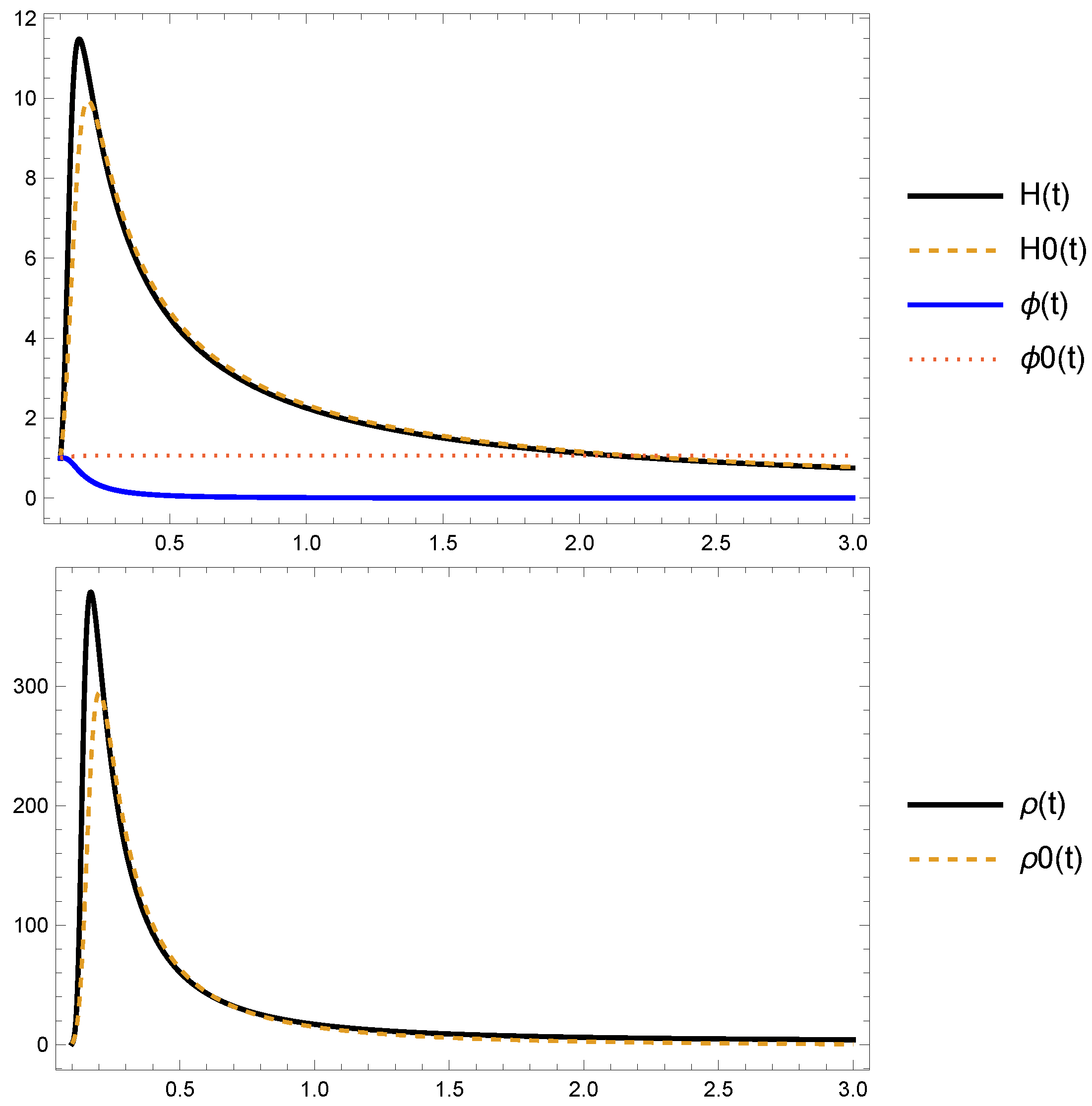
| Data | Best-Fit Values | ||||
|---|---|---|---|---|---|
| CDM Model | |||||
| SNe Ia | ⋯ | ⋯ | |||
| OHD | ⋯ | ⋯ | |||
| SNe Ia plus OHD | ⋯ | ⋯ | |||
| Fractional cosmological model (dust plus radiation) [64] (The uncertainties presented correspond to CL) | |||||
| SNe Ia | ⋯ | ||||
| CC | ⋯ | ||||
| SNe Ia plus CC | ⋯ | ||||
| Fractional cosmological model [69] (The uncertainties presented correspond to , , and CL) | |||||
| SNe Ia | ⋯ | ||||
| OHD | ⋯ | ||||
| SNe Ia plus OHD | ⋯ | ||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Marroquín, K.; Leon, G.; Millano, A.D.; Michea, C.; Paliathanasis, A. Conformal and Non-Minimal Couplings in Fractional Cosmology. Fractal Fract. 2024, 8, 253. https://doi.org/10.3390/fractalfract8050253
Marroquín K, Leon G, Millano AD, Michea C, Paliathanasis A. Conformal and Non-Minimal Couplings in Fractional Cosmology. Fractal and Fractional. 2024; 8(5):253. https://doi.org/10.3390/fractalfract8050253
Chicago/Turabian StyleMarroquín, Kevin, Genly Leon, Alfredo D. Millano, Claudio Michea, and Andronikos Paliathanasis. 2024. "Conformal and Non-Minimal Couplings in Fractional Cosmology" Fractal and Fractional 8, no. 5: 253. https://doi.org/10.3390/fractalfract8050253
APA StyleMarroquín, K., Leon, G., Millano, A. D., Michea, C., & Paliathanasis, A. (2024). Conformal and Non-Minimal Couplings in Fractional Cosmology. Fractal and Fractional, 8(5), 253. https://doi.org/10.3390/fractalfract8050253












