Discrete Fractional-Order Modeling of Recurrent Childhood Diseases Using the Caputo Difference Operator
Abstract
:1. Introduction
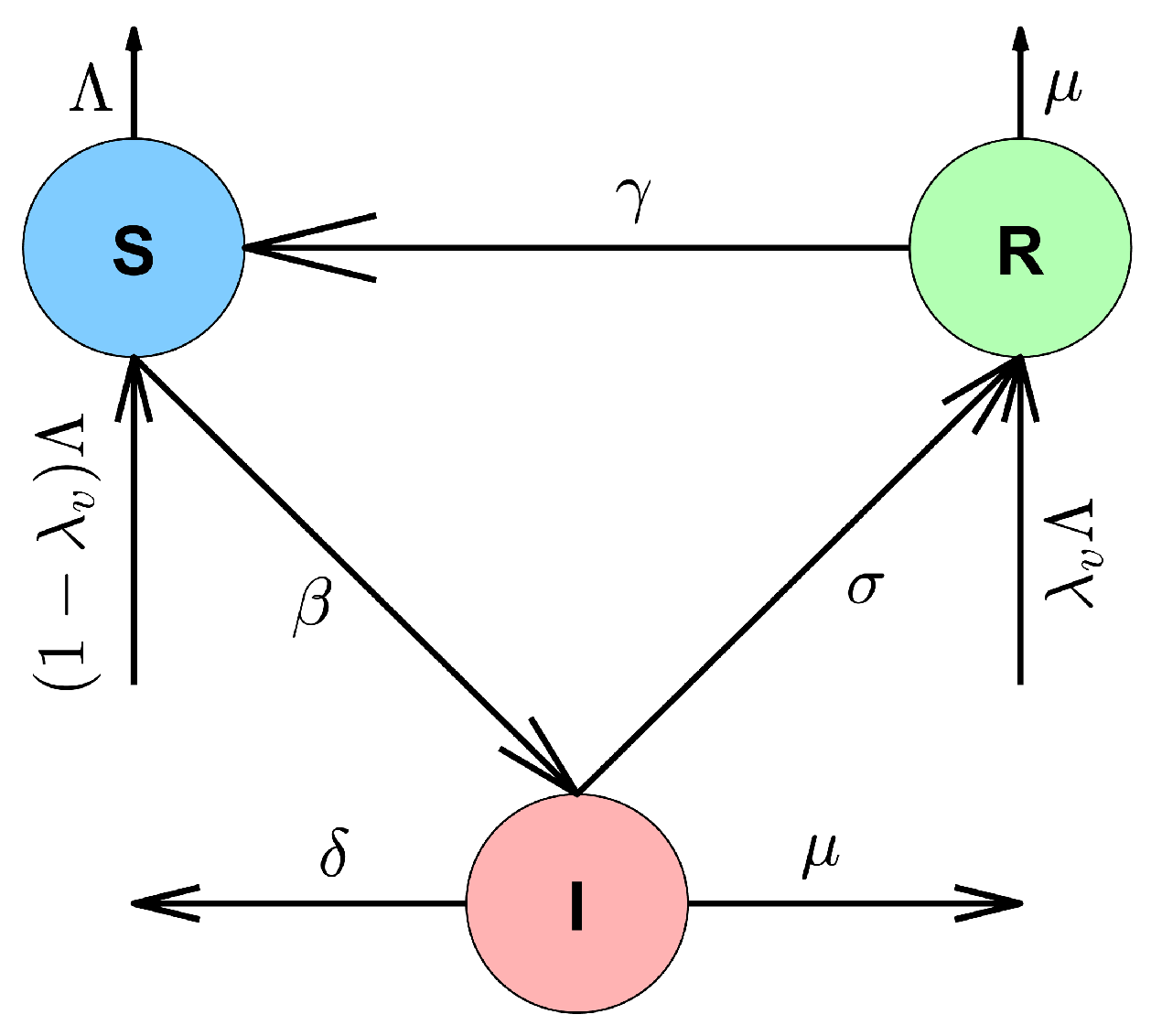
- Newborns enter the susceptible class at a constant birth rate , with a fraction vaccinated at birth.
- Individuals in the population have a constant natural death rate , which affects all compartments equally.
- The disease spreads via contact between susceptible () and infected () individuals, with a transmission rate .
- Infected individuals recover at rate and move to the recovered class () but lose immunity over time at rate , returning to the susceptible class.
- Individuals die at a natural rate , and infected individuals have an additional disease-related mortality rate .
2. Existence and Uniqueness
- There is a bounded function such that for all .
- There is a constant such that for every for all .
3. Ulam Stability
- (i):
- (ii):
4. Parameters Estimation
- Case 1. This scenario features a moderate infection rate () and recovery rate (), representing a disease that can spread within the population but lacks an exceedingly high transmission rate or fatality impact (). With a disease-induced mortality rate of , the risk of death is low but present. The moderate immunity loss rate () suggests that individuals may become susceptible again, though not immediately, pointing to diseases that have limited reinfection rates and maintain a relatively stable population effect. This case could represent diseases where reinfection is uncommon, and overall population health remains steady.
- Case 2. This case models a highly infectious disease scenario characterized by a higher infection rate () alongside a substantial recovery rate (), reflecting a rapidly spreading disease with a reasonable chance of recovery. However, the mortality rate () is elevated, underscoring the disease’s severity. The increased vaccination rate () suggests a need to control transmission more aggressively. The parameter represents the natural death rate, while . This setup mirrors diseases such as measles in low-vaccination communities or pertussis, where high transmission and mortality risks necessitate vigilant infection control measures.
- Case 3. This case represents a recurrent disease, characterized by a heightened immunity loss rate (), leading to individuals quickly losing immunity and re-entering the susceptible population. With an infection rate of , a low mortality rate (), and a moderate recovery rate (), the disease poses minimal risk of death but persists due to frequent reinfections. The low natural death rate () and vaccination rate () reflect minimal demographic impact and vaccination efforts. Diseases like RSV or rotavirus, where reinfections are common but rarely fatal, exemplify this case, highlighting the ongoing healthcare burden posed by recurrent infections.
5. Basic Analysis of the Model
5.1. Non-Negativity of the Solutions
5.2. Disease-Free Equilibrium
5.3. Endemic Equilibrium
5.4. Basic Reproduction Number
5.5. Stability Analysis of Fixed Points
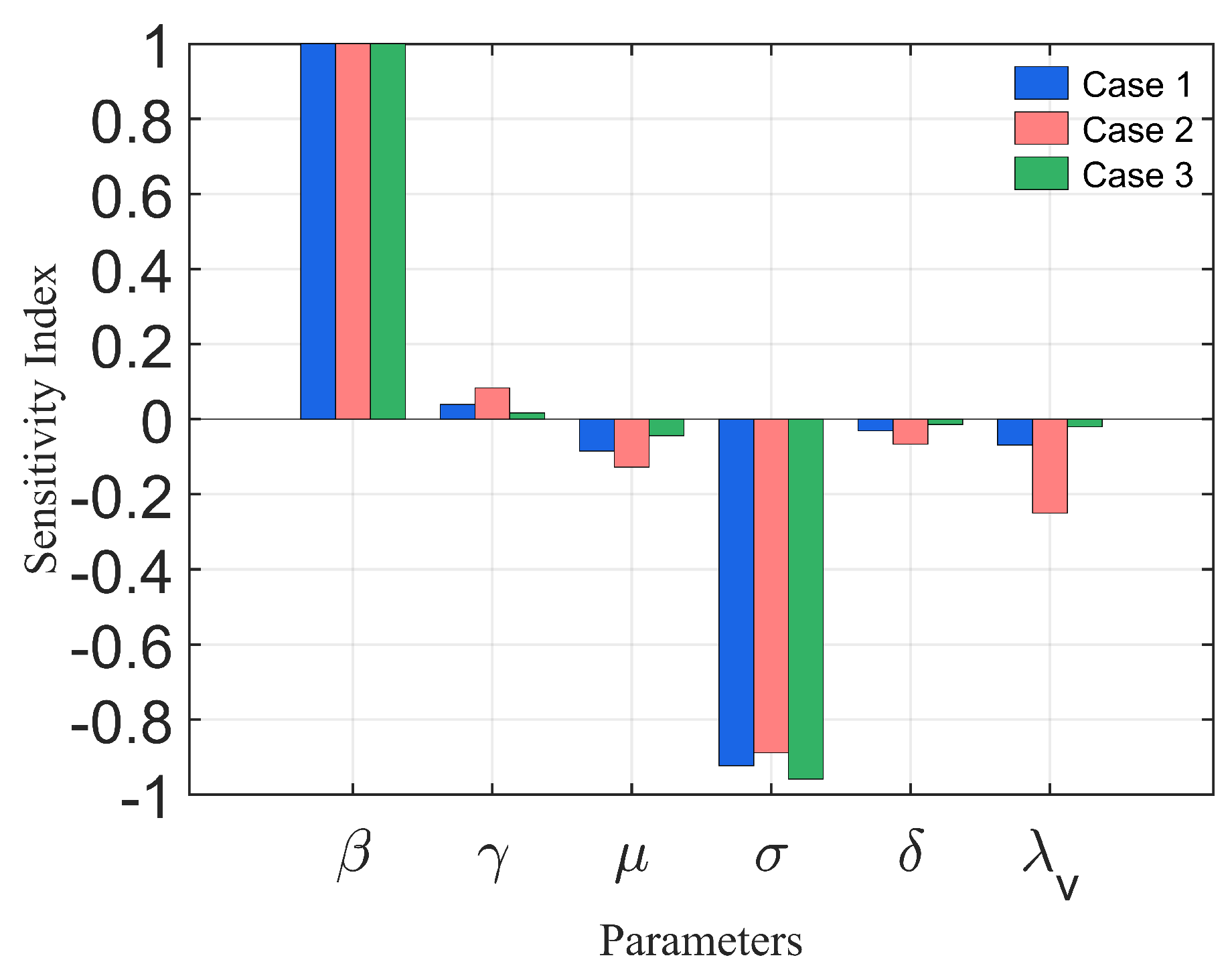
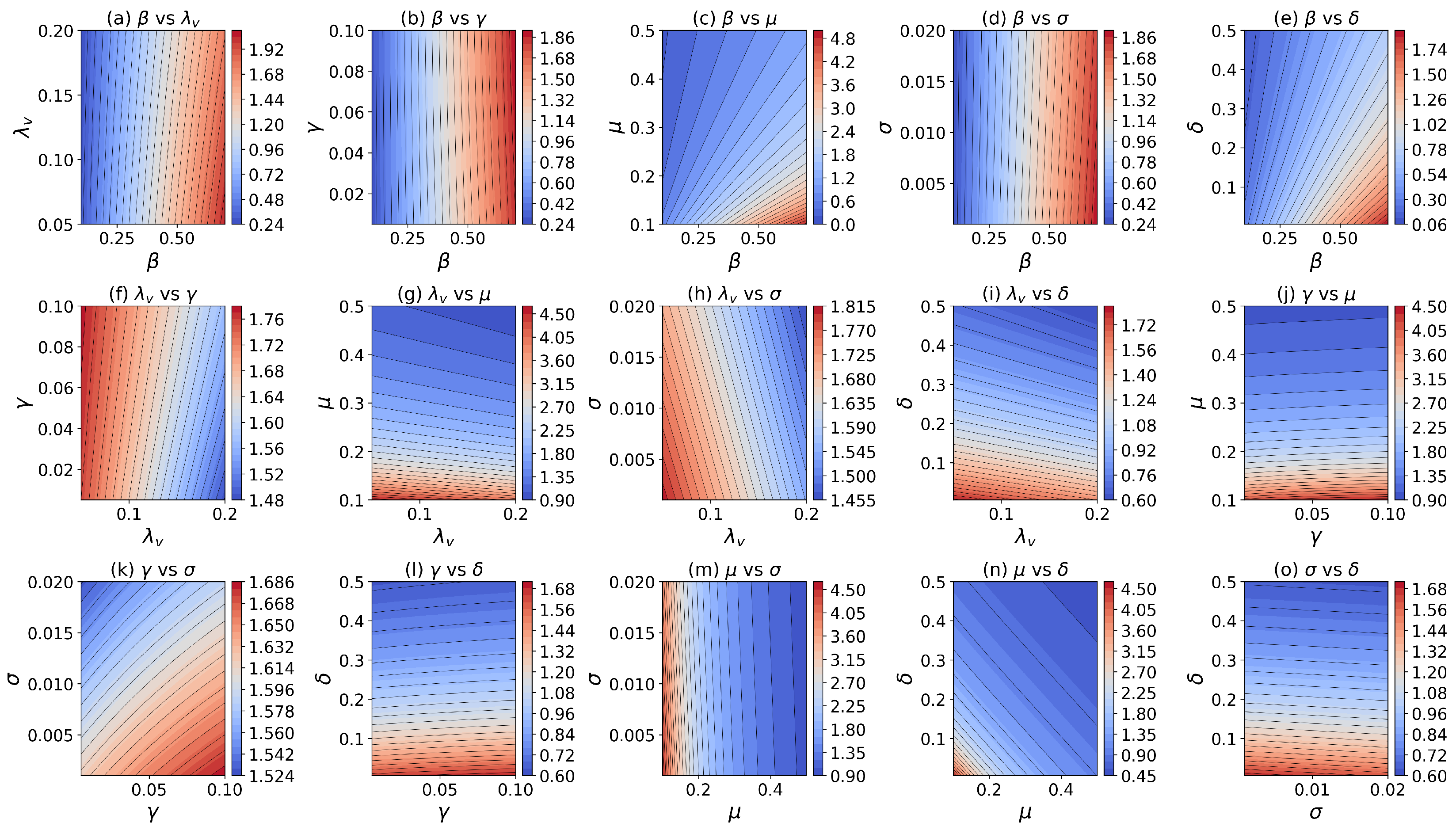
5.6. Sensitivity Analysis
5.7. Scenario Analysis
6. Numerical Solution and Discussion
- Case 1. Figure 6 illustrates the model’s dynamics for Case 1 parameter values. In Figure 6a with , the susceptible population () declines gradually as children become infected, and then rises slowly as immunity fades, bringing individuals back to susceptibility. This slower change is due to a moderate infection rate () and a moderate immunity loss rate (). The infected population () shows moderate peaks, reflecting a stable pattern influenced by the balanced shifts in the susceptible population. Meanwhile, the recovered population () increases steadily after outbreaks and gradually declines as immunity wanes, demonstrating stable recovery and immunity loss dynamics.

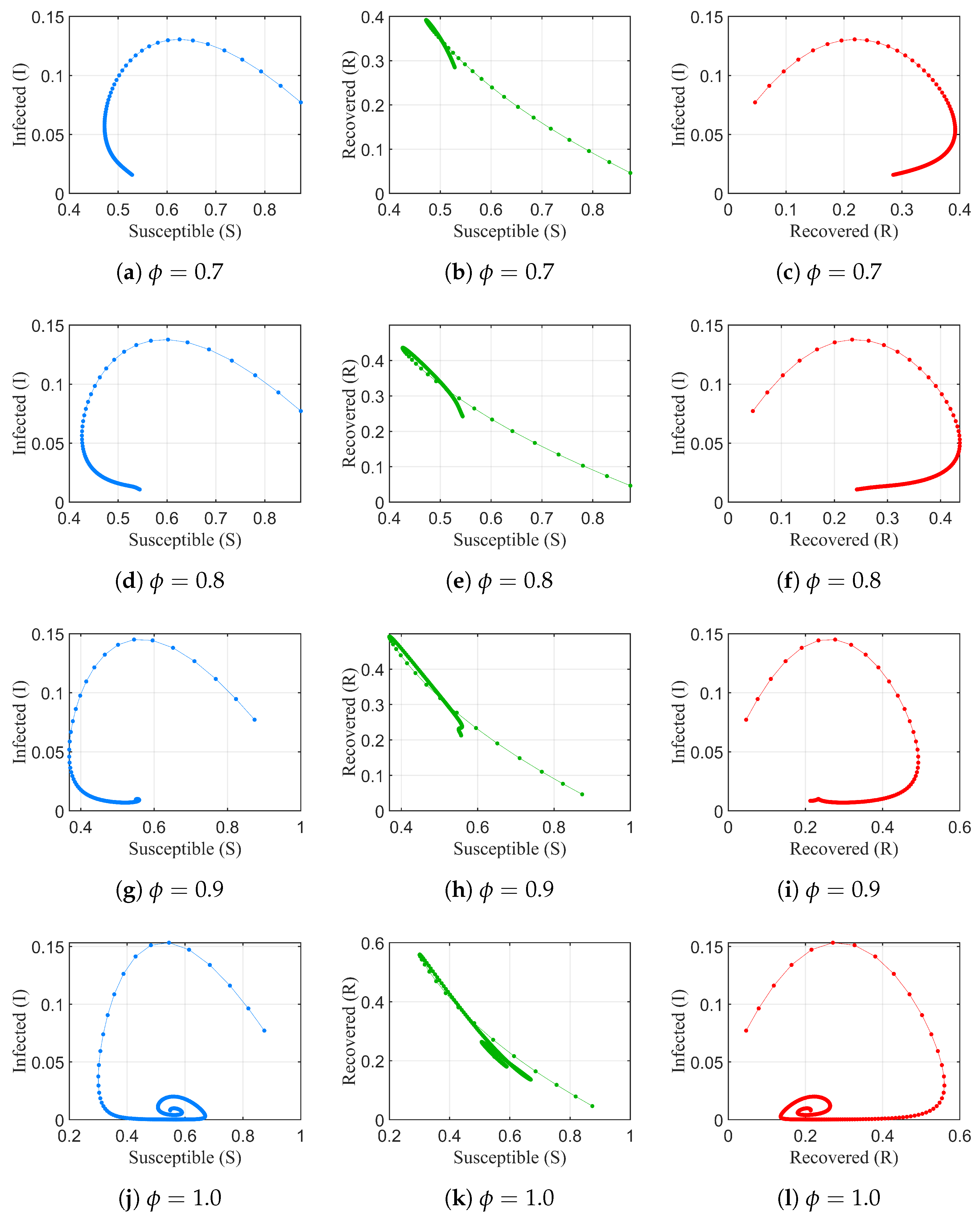
- Case 2. Figure 8 illustrates the dynamics of a highly infectious childhood disease with a high infection rate () and substantial recovery rate (). The figure includes four subplots, each showing a different fractional-order value for : 0.7, 0.8, 0.9, and 1.0. These subplots demonstrate how varying influences the susceptible (), infected (), and recovered () populations over time, while considering the role of immunity loss ().

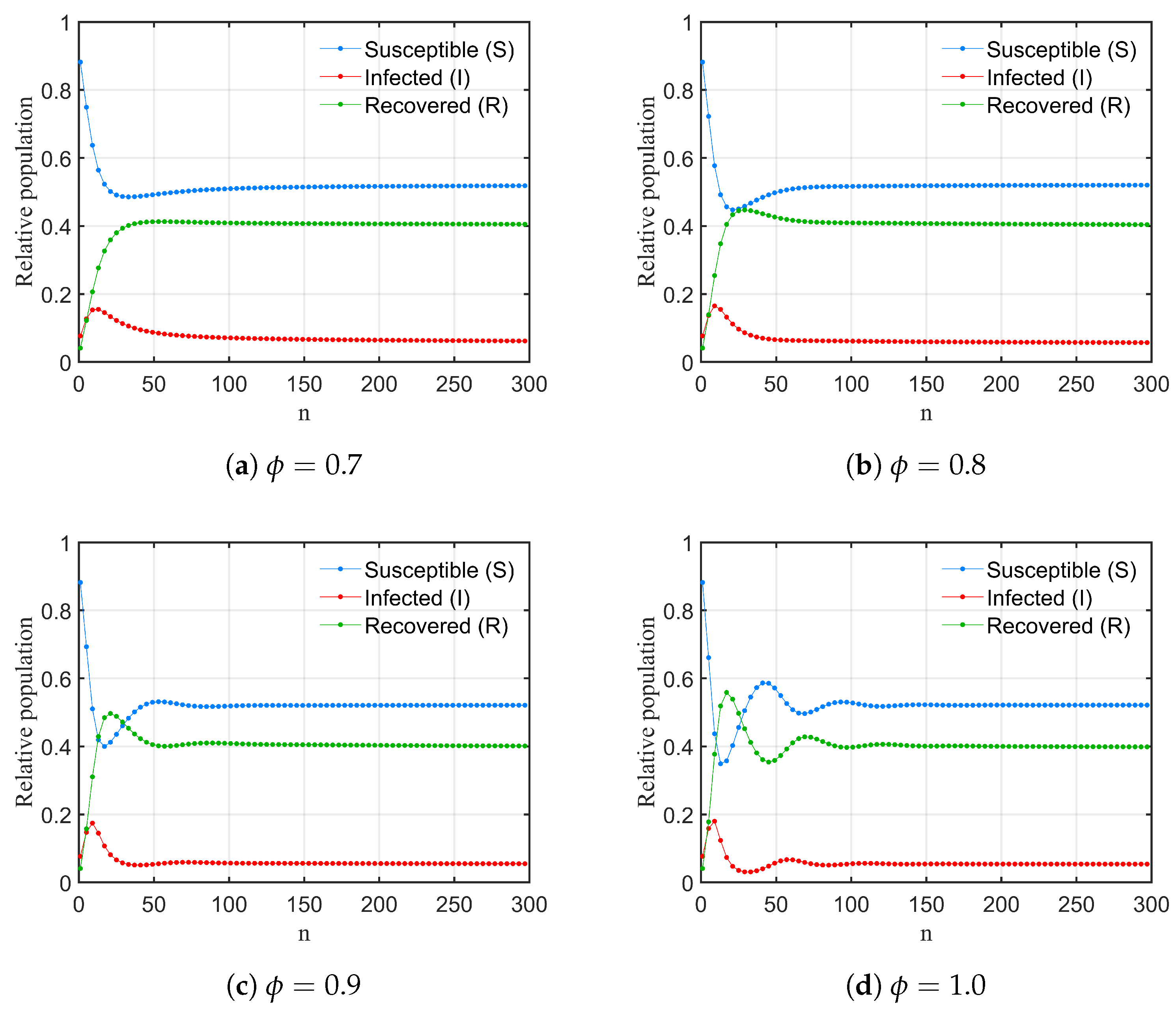
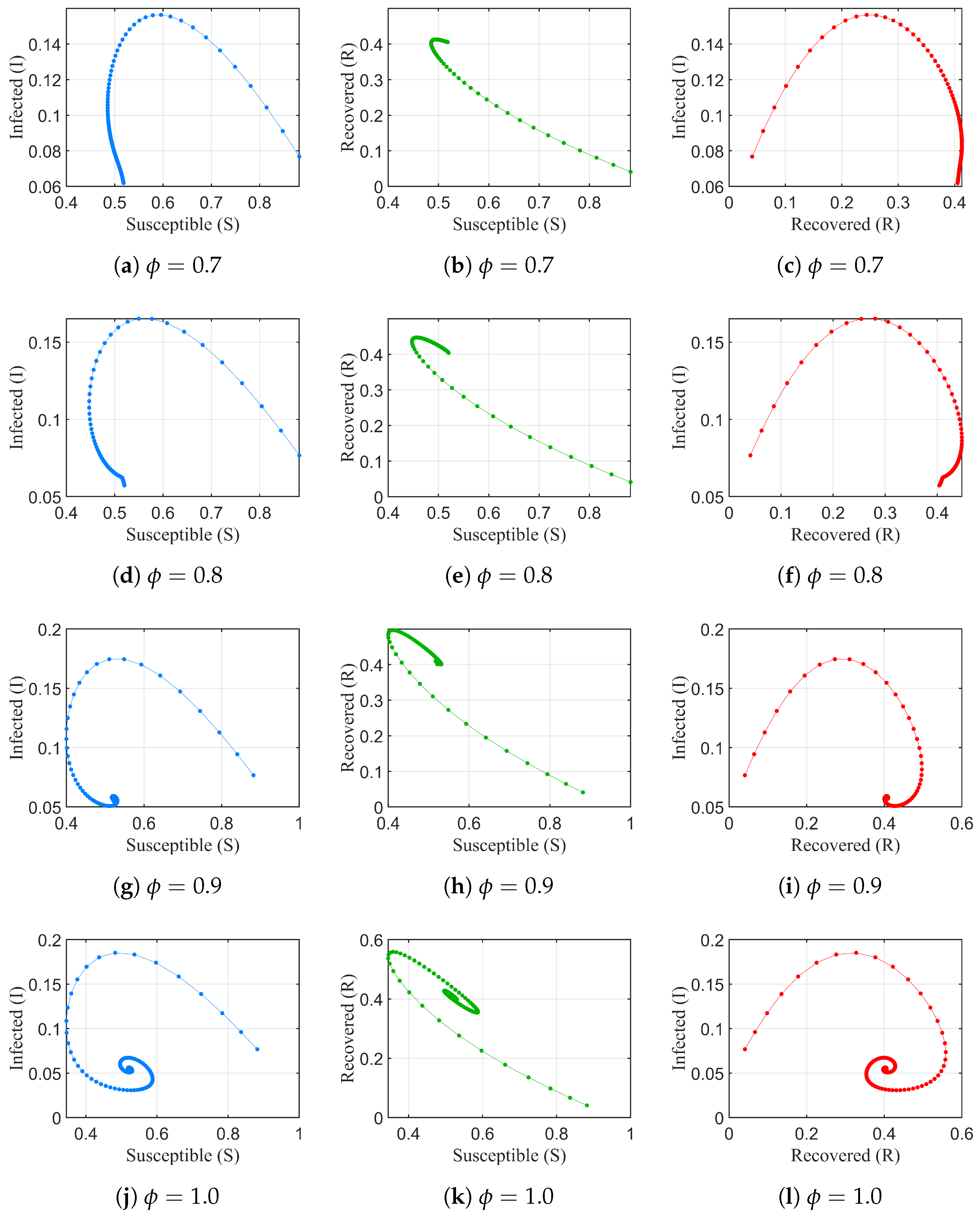
- Case 3. The dynamics of a recurrent disease with high immunity loss (), a moderate infection rate (), a low mortality rate (), and a moderate recovery rate () are illustrated in Figure 10. This figure includes four subplots, each corresponding to a different value of the fractional order : 0.7, 0.8, 0.9, and 1.0. The following parameters remain fixed: vaccination rate (), natural death rate (), and recruitment rate ().
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Martcheva, M. An Introduction to Mathematical Epidemiology; Springer: New York, NY, USA, 2015; Volume 61. [Google Scholar]
- Roberts, M.; Heesterbeek, J. Mathematical Models in Epidemiology; Springer: New York, NY, USA, 2019; Volume 69. [Google Scholar]
- Momani, S.; Kumar, R.; Srivastava, H.M.; Kumar, S.; Hadid, S. A chaos study of fractional SIR epidemic model of childhood diseases. Results Phys. 2021, 27, 104422. [Google Scholar] [CrossRef]
- Darti, I.; Suryanto, A. Dynamics of a SIR Epidemic Model of Childhood Diseases with a Saturated Incidence Rate: Continuous Model and Its Nonstandard Finite Difference Discretization. Mathematics 2020, 8, 1459. [Google Scholar] [CrossRef]
- Alter, S.J.; Bennett, J.S.; Koranyi, K.; Kreppel, A.; Simon, R. Common Childhood Viral Infections. Curr. Probl. Pediatr. Adolesc. Health Care 2015, 45, 21–53. [Google Scholar] [CrossRef] [PubMed]
- Makinde, O.D. Adomian decomposition approach to a SIR epidemic model with constant vaccination strategy. Appl. Math. Comput. 2007, 184, 842–848. [Google Scholar] [CrossRef]
- Platt, L.; Thun, M.; Harriman, K.; Winter, K. A Population-Based Study of Recurrent Symptomatic Bordetella pertussis Infections in Children in California, 2010–2015. Clin. Infect. Dis. 2017, 65, 2099–2104. [Google Scholar] [CrossRef] [PubMed]
- Crawford, S.; Ramani, S.; Tate, J.; Parashar, U.D.; Svensson, L.; Hagbom, M.; Franco, M.A.; Greenberg, H.B.; O’Ryan, M.; Kang, G.; et al. Rotavirus infection. Nat. Rev. Dis. Primers 2017, 3, 17083. [Google Scholar] [CrossRef]
- Streng, A.; Prifert, C.; Weissbrich, B.; Sauerbrei, A.; Krumbholz, A.; Schmidt-Ott, R.; Liese, J.G. Similar severity of influenza primary and re-infections in pre-school children requiring outpatient treatment due to febrile acute respiratory illness: Prospective, multicentre surveillance study (2013–2015). BMC Infect. Dis. 2022, 22, 12. [Google Scholar] [CrossRef] [PubMed]
- Kutsaya, A.; Teros-Jaakkola, T.; Kakkola, L.; Toivonen, L.; Peltola, V.; Waris, M.; Julkunen, I. Prospective clinical and serological follow-up in early childhood reveals a high rate of subclinical RSV infection and a relatively high reinfection rate within the first 3 years of life. Epidemiol. Infect. 2016, 144, 1622–1633. [Google Scholar] [CrossRef] [PubMed]
- Shah, M.P.; Hall, A.J. Norovirus Illnesses in Children and Adolescents. Infect. Dis. Clin. N. Am. 2018, 32, 103–118. [Google Scholar] [CrossRef]
- Selvam, A.G.M.; Vianny, D.A.; Jacintha, M. Stability in a fractional order SIR epidemic model of childhood diseases with discretization. J. Phys. Conf. Ser. 2018, 1139, 012009. [Google Scholar] [CrossRef]
- de Oliveira, E.C.; Machado, J.A.T. A Review of Definitions for Fractional Derivatives and Integral. Math. Probl. Eng. 2014, 2014, 238459. [Google Scholar] [CrossRef]
- Hilfer, R. Applications of Fractional Calculus in Physics; World Scientific: Singapore, 2000. [Google Scholar]
- Chávez-Vázquez, S.; Gómez-Aguilar, J.F.; Lavín-Delgado, J.E.; Escobar-Jiménez, R.F.; Olivares-Peregrino, V.H. Applications of Fractional Operators in Robotics: A Review. J. Intell. Robot. Syst. 2022, 104, 63. [Google Scholar] [CrossRef]
- Das, S. Application of Generalized Fractional Calculus in Electrical Circuit Analysis and Electromagnetics. In Functional Fractional Calculus; Springer: Berlin/Heidelberg, Germany, 2011. [Google Scholar] [CrossRef]
- Magin, R.L. Fractional calculus in bioengineering. Crit. Rev. Biomed. Eng. 2004, 32, 1–104. [Google Scholar] [CrossRef] [PubMed]
- Oldham, K. Fractional differential equations in electrochemistry. Adv. Eng. Softw. 2010, 41, 9–12. [Google Scholar] [CrossRef]
- Ali, Z.; Nia, S.N.; Rabiei, F.; Shah, K.; Tan, M.K. A semi-analytical approach for the solution of time-fractional Navier-Stokes equation. Adv. Math. Phys. 2021, 2021, 5547804. [Google Scholar] [CrossRef]
- Tarasov, V.E. Fractional Dynamics: Applications of Fractional Calculus to Dynamics of Particles, Fields and Media; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2011. [Google Scholar]
- Alqahtani, A.M.; Akram, S.; Ahmad, J.; Aldwoah, K.A.; ur Rahman, M. Stochastic wave solutions of fractional Radhakrishnan-Kundu-Lakshmanan equation arising in optical fibers with their sensitivity analysis. J. Opt. 2024. [Google Scholar] [CrossRef]
- Matušu, R. Application of fractional order calculus to control theory. Int. J. Math. Model. Methods Appl. Sci. 2011, 5, 1162–1169. [Google Scholar]
- Meilanov, R.P.; Magomedov, R.A. Thermodynamics in Fractional Calculus. J. Eng. Phys. Thermophy 2014, 87, 1521–1531. [Google Scholar] [CrossRef]
- Meral, F.; Royston, T.; Magin, R. Fractional calculus in viscoelasticity: An experimental study. Commun. Nonlinear Sci. Numer. Simul. 2010, 15, 939–945. [Google Scholar] [CrossRef]
- Abdulwahhab, O.W.; Abbas, N.H. A new method to tune a fractional-order PID controller for a twin rotor aerodynamic system. Arab. J. Sci. Eng. 2017, 42, 5179–5189. [Google Scholar] [CrossRef]
- Jiang, Y.; Zhang, B.; Shu, X.; Wei, Z. Fractional-order autonomous circuits with order larger than one. J. Adv. Res. 2020, 25, 217–225. [Google Scholar] [CrossRef] [PubMed]
- Ali, Z. Theoretical and Computational Study of Fractional-Order Mathematical Models for Infectious Diseases. Ph.D. Thesis, Monash University, Clayton, VIC, Australia, 2023. [Google Scholar] [CrossRef]
- Shah, N.A.; Vieru, D.; Fetecau, C. Effects of the fractional order and magnetic field on the blood flow in cylindrical domains. J. Magn. Magn. Mater. 2016, 409, 10–19. [Google Scholar] [CrossRef]
- Can, N.H.; Jafari, H.; Ncube, M.N. Fractional calculus in data fitting. Alex. Eng. J. 2020, 59, 3269–3274. [Google Scholar] [CrossRef]
- Madani, Y.A.; Rabih, M.N.A.; Alqarni, F.A.; Ali, Z.; Aldwoah, K.A.; Hleili, M. Existence, Uniqueness, and Stability of a Nonlinear Tripled Fractional Order Differential System. Fractal Fract. 2024, 8, 416. [Google Scholar] [CrossRef]
- Adel, M.; Khader, M.M.; Algelany, S.; Aldwoah, K. An Accurate Approach to Simulate the Fractional Delay Differential Equations. Fractal Fract. 2023, 7, 671. [Google Scholar] [CrossRef]
- Ali, Z.; Rabiei, F.; Shah, K.; Majid, Z.A. Dynamics of SIR mathematical model for COVID-19 outbreak in Pakistan under fractal-fractional derivative. Fractals 2021, 29, 2150120. [Google Scholar] [CrossRef]
- Ostalczyk, P. Discrete Fractional Calculus: Applications in Control and Image Processing; World Scientific: Singapore, 2015; Volume 4. [Google Scholar]
- Al-Khedhairi, A.; Elsadany, A.A.; Elsonbaty, A. On the dynamics of a discrete fractional-order cournot-bertrand competition duopoly game. Math. Probl. Eng. 2022, 2022, 8249215. [Google Scholar] [CrossRef]
- Elsonbaty, A.; Elsadany, A.A. On discrete fractional-order Lotka-Volterra model based on the Caputo difference discrete operator. Math. Sci. 2023, 17, 67–79. [Google Scholar] [CrossRef]
- Huang, L.-L.; Park, J.H.; Wu, G.-C.; Mo, Z.-W. Variable-order fractional discrete-time recurrent neural networks. J. Comput. Appl. Math. 2020, 370, 112633. [Google Scholar] [CrossRef]
- He, Z.-Y.; Abbes, A.; Jahanshahi, H.; Alotaibi, N.D.; Wang, Y. Fractional-Order Discrete-Time SIR Epidemic Model with Vaccination: Chaos and Complexity. Mathematics 2022, 10, 165. [Google Scholar] [CrossRef]
- Tarasov, V.E.; Tarasova, V.V. Long and short memory in economics: Fractional-order difference and differentiation. arXiv 2016, arXiv:1612.07903. [Google Scholar] [CrossRef]
- Peng, Y.; He, S.; Sun, K. Chaos in the discrete memristor-based system with fractional-order difference. Results Phys. 2021, 24, 104106. [Google Scholar] [CrossRef]
- Chen, F.; Zhou, Y. Existence and Ulam Stability of Solutions for Discrete Fractional Boundary Value Problem. Discret. Dyn. Nat. Soc. 2013, 2013, 459161. [Google Scholar] [CrossRef]
- van den Driessche, P. Reproduction Numbers of Infectious Disease Models. Infect. Dis. Model. 2017, 2, 288–303. [Google Scholar] [CrossRef]
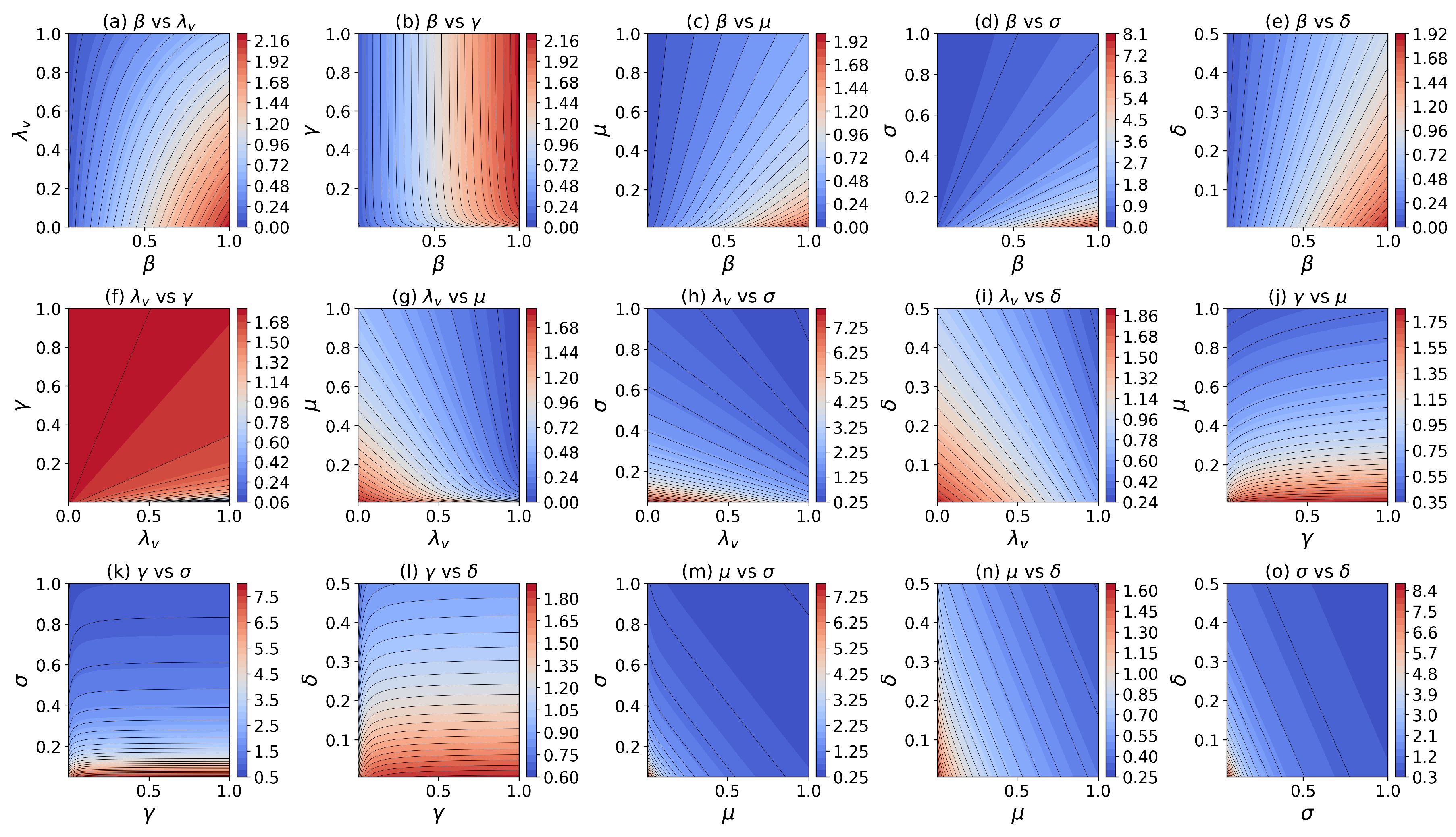
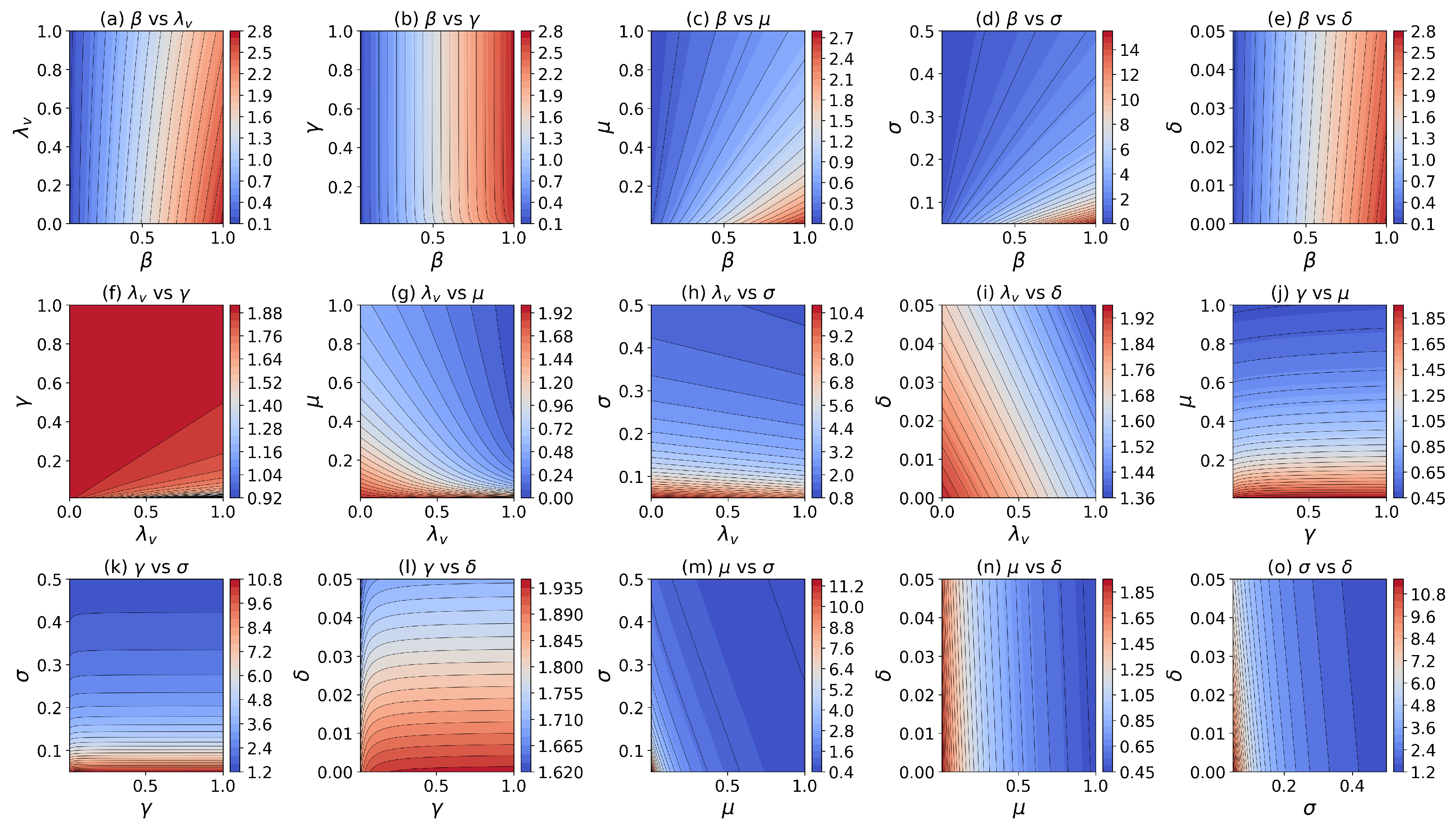


| Parameters | ||||||
|---|---|---|---|---|---|---|
| Case 1 | ||||||
| Case 2 | ||||||
| Case 3 |
| Parameters | |||||||
|---|---|---|---|---|---|---|---|
| Case 1 | 0.15 | 0.01 | 0.6 | 0.3 | 0.015 | 0.01 | 0.02 |
| Case 2 | 0.3 | 0.01 | 0.8 | 0.4 | 0.02 | 0.03 | 0.01 |
| Case 3 | 0.1 | 0.01 | 0.7 | 0.35 | 0.01 | 0.005 | 0.04 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Madani, Y.A.; Ali, Z.; Rabih, M.; Alsulami, A.; Eljaneid, N.H.E.; Aldwoah, K.; Muflh, B. Discrete Fractional-Order Modeling of Recurrent Childhood Diseases Using the Caputo Difference Operator. Fractal Fract. 2025, 9, 55. https://doi.org/10.3390/fractalfract9010055
Madani YA, Ali Z, Rabih M, Alsulami A, Eljaneid NHE, Aldwoah K, Muflh B. Discrete Fractional-Order Modeling of Recurrent Childhood Diseases Using the Caputo Difference Operator. Fractal and Fractional. 2025; 9(1):55. https://doi.org/10.3390/fractalfract9010055
Chicago/Turabian StyleMadani, Yasir A., Zeeshan Ali, Mohammed Rabih, Amer Alsulami, Nidal H. E. Eljaneid, Khaled Aldwoah, and Blgys Muflh. 2025. "Discrete Fractional-Order Modeling of Recurrent Childhood Diseases Using the Caputo Difference Operator" Fractal and Fractional 9, no. 1: 55. https://doi.org/10.3390/fractalfract9010055
APA StyleMadani, Y. A., Ali, Z., Rabih, M., Alsulami, A., Eljaneid, N. H. E., Aldwoah, K., & Muflh, B. (2025). Discrete Fractional-Order Modeling of Recurrent Childhood Diseases Using the Caputo Difference Operator. Fractal and Fractional, 9(1), 55. https://doi.org/10.3390/fractalfract9010055








