High Precision Accelerometer with Integrated Thermal Sensor †
by
Beatrice Bourgeteau-Verlhac
*, 
Raphaël Lévy
,
Pierre Lavenus
,
Claude Chartier
,
Vincent Gaudineau
and
Olivier Le Traon
ONERA, the French Aerospace Lab, Palaiseau, France
*
Author to whom correspondence should be addressed.
†
Presented at the Eurosensors 2017 Conference, Paris, France, 3–6 September 2017.
Proceedings 2017, 1(4), 285; https://doi.org/10.3390/proceedings1040285
Published: 24 August 2017
(This article belongs to the Proceedings of Proceedings of Eurosensors 2017, Paris, France, 3–6 September 2017)
Abstract
:This paper investigates the design of a Vibrating Beam Accelerometer (VBA) with a resolution of 50 nano-g combined with an integrated thermal sensor. Despite quartz intrinsic thermal stability, the time delay between vibrating beam’s temperature and package’s temperature gives way to unwanted transient thermal behavior and thus bias instability. The aim of this study is to include a thermal sensor consisting in a torsional resonator directly at the center of the beam. Previous work demonstrated the feasibility of such integration on a tactical class accelerometer but also highlighted limitations like high motional resistance of the torsional resonator. Benefits of the in-situ temperature sensor are investigated thanks to finite element analysis of the accelerometer transient thermal behavior, which shall be compared to measurements on actual cells.
1. Introduction
1.1. Inertial Grade MEMS Accelerometer for Emerging Applications
Emerging applications require low bias instability (<10 μg), high resolution (<1 μg), miniature (<1 cm3) and low power (<1 mW) accelerometers and allow a limited measurement range: wireless sensor network of Precision MEMS accelerometers based inclinometers dedicated to structural health monitoring (buildings, bridges, …) [1,2], wireless sensor networks of MEMS accelerometers based seismometers to detect and identify intruders in a protected area [3,4], inertial measurement units dedicated to micro UAVs. Although Vibrating Beam Accelerometers (VBA) have demonstrated good performances [5,6], actual accelerometers do not fulfill all these requirements, and are mainly limited by bias instability over temperature.
1.2. Bias Drift over Temperature
Bias drift over temperature is of main concern for inertial sensors performances and especially for Vibrating Beam Accelerometers (VBA). The thermal behavior is usually compensated thanks to an external temperature sensor on the package of the accelerometer itself, with polynomial laws of the temperature or even taking into account its time derivative. Despite those numerical compensations it appears that the time delay between the temperature of the vibrating beam and that of the package gives way to unwanted transient thermal behavior peculiarly for very fast thermal solicitations.
In order to overcome such drawbacks, a torsional resonator can be implemented at the center of the vibrating beam to know instantaneously the temperature of the beam itself. This principle was demonstrated on a strategic accelerometer [7] and is now developed on a very highly sensitive accelerometer with a 50 ng resolution.
The new design, presented in Figure 1 was first elaborated thanks to finite element analysis and some realizations were achieved. In a second phase, the benefits of the use of the in-situ sensor are investigated thanks to finite Element Analysis (FEA) and measurements on actual cells.
2. MEMS Detailed Design
Torsional Mode Design
To integrate the torsional resonator on the vibrating beam, the complete structure was simulated, including the facets due to chemical etching. Figure 2, shows the results of etching facets predicted thanks to MicroCAD® software, the finite element model used and the actual cell with its etching facets.
The frequency shift of the torsional resonator with the dimensions of the etching facets was investigated and showed 0.1% evolution of the frequency for 1 μm evolution which highlights that realization dispersions are very critical for such design. Thus eigen frequencies where thoroughly studied thanks to modal finite element analysis for both the flexural mode of the VBA and the torsional mode to isolate them from other potential parasitic modes.
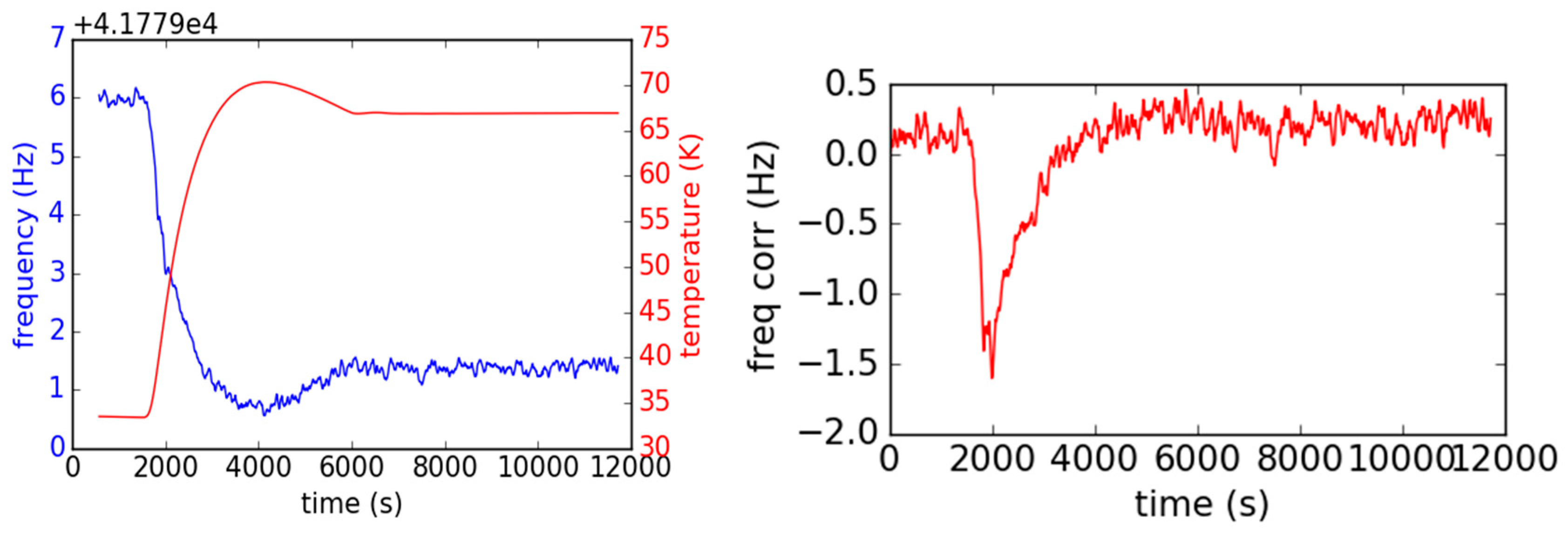
3. Results
In order to improve the performances of the thermal sensor the frequency of the torsional resonator was increased in the design “Candidate B” compared with former version “Candidate A” leading to a lower motional resistance Rm. This frequency increase is also aimed at reducing electrical coupling of the two modes enabling a more efficient signal filtering. The two versions characteristics are described in Table 1 and Candidate B is presented on Figure 3.
Thermal Transient Behavior
The thermal transient behavior of both candidates was studied thanks to the finite element analysis software OOFELIE::Multiphysics©. The finite element analysis includes the thermal transient behavior of the structure chained with static thermomechanical and a prestressed modal analysis. The transient evolution of the vibrating beam frequency is caused by the time delay between the temperature evolution outside and inside the packaging and the thermal gradient which appears in the quartz cell itself.
Usually, the thermal sensitivity of a resonator is modelled thanks to a polynomial law. As an example the evaluation of the temperature behavior was made on an actual cell without torsional sensor on Figure 4.
4. Conclusions
The thermal transient behavior described above evolution produces a bias instability which is partially compensated by the static polynomial model. A residual evolution remains due to transient behavior of 1.5 Hz i.e., 4 mg with the scale factor of Candidate B. Taking into account the time delay between the beam temperature and the packaging presented in Figure 5 should drastically reduce bias instability presented in Figure 4. This is complementary with a correction of the temperature time derivative effects. Experimental measurements on actual cells shall confirm this point soon.
References
- Lynch, J.P.; Loh, K.J. A summary review of wireless sensors and sensor networks for structural health monitoring. Shock Vib. Dig. 2006, 38, 91–130. [Google Scholar] [CrossRef]
- Ha, D.W.; Park, H.S.; Choi, S.W.; Kim, Y. A wireless MEMS-based inclinometer sensor node for structural health monitoring. Sensors 2013, 13, 16090–16104. [Google Scholar] [CrossRef] [PubMed]
- Huang, J.; Zhou, Q.; Zhang, X.; Song, E.; Li, B.; Yuan, X. Seismic target classification using a wavelet packet manifold in unattended ground sensors systems. Sensors 2013, 13, 8534–8550. [Google Scholar] [CrossRef] [PubMed]
- Mehmood, A.; Patel, V.M.; Damarla, T. Discrimination of bipeds from quadrupeds using seismic footstep signatures. In Proceedings of the 2012 IEEE International Geoscience and Remote Sensing Symposium (IGARSS), Munich, Germany, 22–27 July 2012. [Google Scholar]
- Loret, T.; Hardy, G.; Vallée, C.; Demutrecy, V.; Kerrien, T.; Cochain, S.; Blondeau, R. Navigation grade accelerometer with quartz vibrating beam. In Proceedings of the 2014 IEEE DGON Inertial Sensors and Systems Symposium (ISS), Karlsruhe, Germany, 16–17 September 2014. [Google Scholar]
- Levy, R.; Janiaud, D.; Guerard, J.; Taibi, R.; Le Traon, O. A 50 nano-g resolution quartz Vibrating Beam Accelerometer. In Proceedings of the International Symposium on Inertial Sensors and Systems (ISISS), Laguna Beach, CA, USA, 25–26 February 2014. [Google Scholar]
- Levy, R.; Le Traon, O.; Masson, S.; Ducloux, O.; Janiaud, D.; Guerard, J.; Chartier, C. An integrated resonator-based thermal compensation for Vibrating Beam Accelerometers. In Proceedings of the 2012 IEEE Sensors, Taipei, Taiwan, 28–31 October 2012. [Google Scholar]
Figure 1.
Vibrating beam accelerometer MEMS (a), flexural mode of the beam which eigen frequency is proportional to axial load induced by acceleration (b), torsional mode which eigen frequency is proportional to the beam temperature (c).
Figure 1.
Vibrating beam accelerometer MEMS (a), flexural mode of the beam which eigen frequency is proportional to axial load induced by acceleration (b), torsional mode which eigen frequency is proportional to the beam temperature (c).
Figure 2.
Predicted etching facets (a), finite element model taking into account etching facets (b), actual etching facets on the quartz cell (c).
Figure 2.
Predicted etching facets (a), finite element model taking into account etching facets (b), actual etching facets on the quartz cell (c).
Figure 3.
The new accelerometer design and an enlargement of the area with the vibrating beam with the torsional resonator at its centre on the right.
Figure 3.
The new accelerometer design and an enlargement of the area with the vibrating beam with the torsional resonator at its centre on the right.
Figure 4.
Transient behavior measured on an actual cell and residual frequency shift after polynomial correction.
Figure 4.
Transient behavior measured on an actual cell and residual frequency shift after polynomial correction.
Figure 5.
Thermal transient behavior of the accelerometer (FEA).
Table 1.
Characteristics of the two versions of accelerometers “Candidate A” and “Candidate B”.
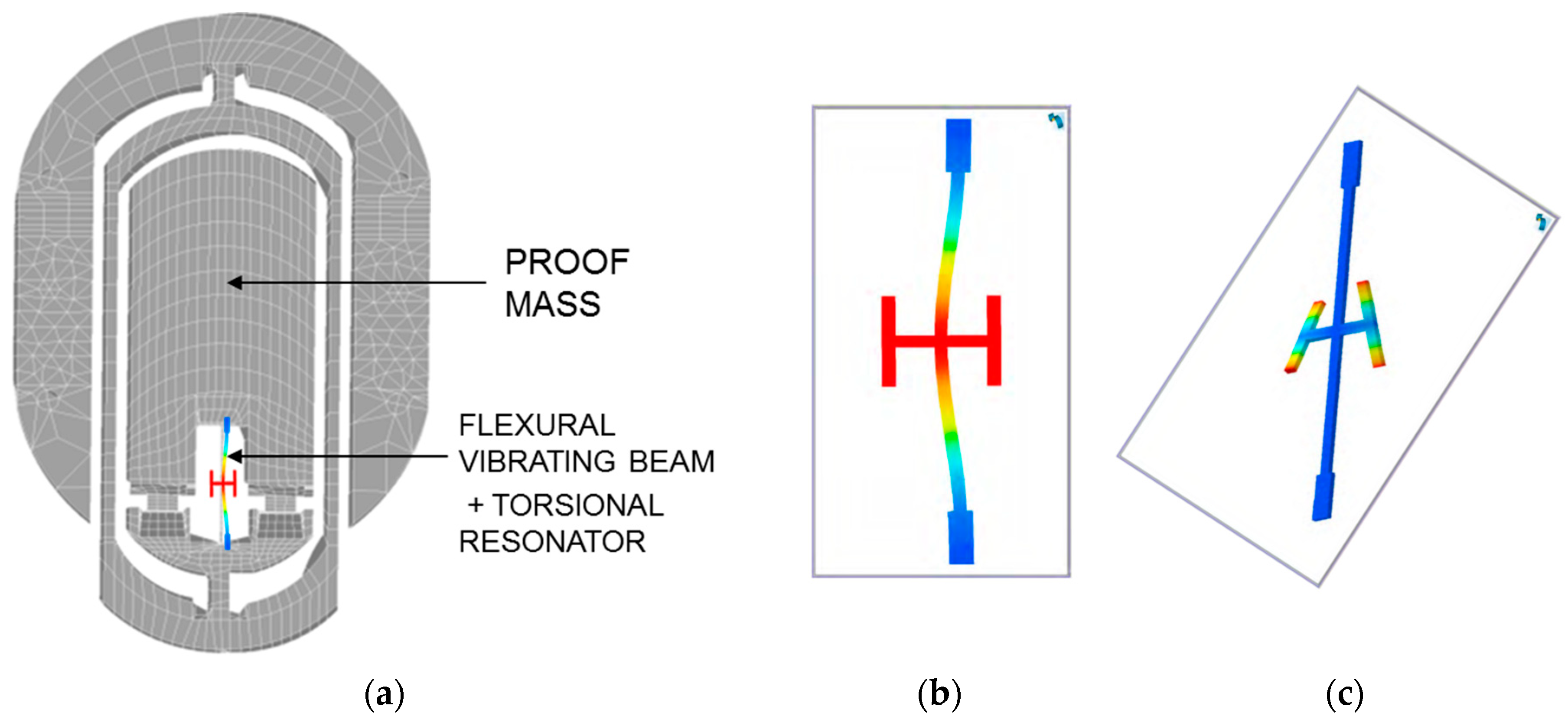
| Flexural Frequency (Hz) | Torsional Frequency (Hz) | Torsional Rm | Scale Factor | |
|---|---|---|---|---|
| Candidate A | 35,020 | 207,210 | 22 MΩ | 211 Hz/g |
| Candidate B | 34,970 | 737,850 | 5 MΩ | 247 Hz/g |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
MDPI and ACS Style
Bourgeteau-Verlhac, B.; Lévy, R.; Lavenus, P.; Chartier, C.; Gaudineau, V.; Traon, O.L. High Precision Accelerometer with Integrated Thermal Sensor. Proceedings 2017, 1, 285. https://doi.org/10.3390/proceedings1040285
AMA Style
Bourgeteau-Verlhac B, Lévy R, Lavenus P, Chartier C, Gaudineau V, Traon OL. High Precision Accelerometer with Integrated Thermal Sensor. Proceedings. 2017; 1(4):285. https://doi.org/10.3390/proceedings1040285
Chicago/Turabian StyleBourgeteau-Verlhac, Beatrice, Raphaël Lévy, Pierre Lavenus, Claude Chartier, Vincent Gaudineau, and Olivier Le Traon. 2017. "High Precision Accelerometer with Integrated Thermal Sensor" Proceedings 1, no. 4: 285. https://doi.org/10.3390/proceedings1040285
APA StyleBourgeteau-Verlhac, B., Lévy, R., Lavenus, P., Chartier, C., Gaudineau, V., & Traon, O. L. (2017). High Precision Accelerometer with Integrated Thermal Sensor. Proceedings, 1(4), 285. https://doi.org/10.3390/proceedings1040285
