Squeezing-Enhanced Phase-Shift-Keyed Binary Communication in Noisy Channels †
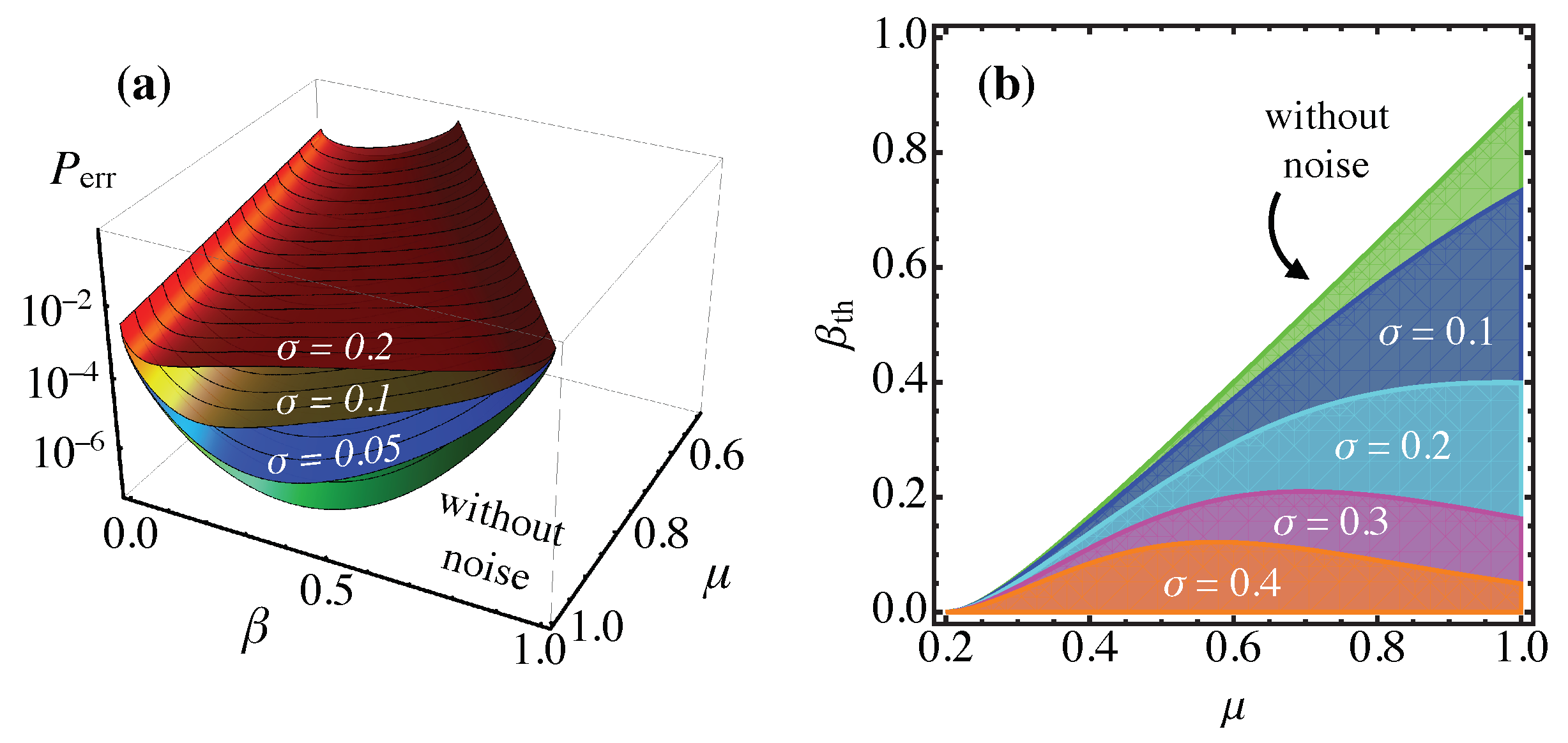
Abstract
:Author Contributions
References
- Yuen, H.P.; Kennedy, R.S.; Lax, M. Optimum testing of multiple hypotheses in quantum detection theory. IEEE Trans. Inf. Theory 1975, 21, 125–134. [Google Scholar] [CrossRef]
- Bergou, J.A. Discrimination of quantum states. J. Mod. Opt. 2010, 57, 160–180. [Google Scholar] [CrossRef]
- Helstrom, C.W. Quantum Detection and Estimation Theory; Academic Press: New York, NY, USA, 1976. [Google Scholar]
- Günthner, K.; Khan, I.; Elser, D.; Stiller, B.; Bayraktar, Ö.; Müller, C.R.; Saucke, K.; Tröndle, D.; Heine, F.; Seel, S.; et al. Quantum-limited measurements of optical signals from a geostationary satellite. Optica 2017, 4, 611–616. [Google Scholar] [CrossRef]
- Diamanti, E.; Lo, H.-K.; Qi, B.; Yuan, Z. Practical challenges in quantum key distribution. NPJ Quantum Inf. 2016, 2, 1–6. [Google Scholar] [CrossRef]
- Shapiro, J.H.; Yuen, H.P.; Mata, J.A.M. Optical Communication with Two-Photon Coherent States—Part II: Photoemissive Detection and Structured Receiver Performance. IEEE Trans. Inf. Theory 1979, IT-25, 179–192. [Google Scholar] [CrossRef]
- Nair, R.; Yen, B.J.; Guha, S.; Shapiro, J.H.; Pirandola, S. Symmetric M-ary phase discrimination using quantum-optical probe states. Phys. Rev. A 2012, 86, 1–12. [Google Scholar] [CrossRef]
- Olivares, S.; Cialdi, S.; Castelli, F.; Paris, M.G.A. Homodyne detection as a near-optimum receiver for phase-shift-keyed binary communication in the presence of phase diffusion. Phys. Rev. A 2013, 87, 1–4. [Google Scholar] [CrossRef]
- Chesi, G.; Olivares, S.; Paris, M.G.A. Squeezing-enhanced phase-shift-keyed binary communication in noisy channels. Phys. Rev. A 2018, 97, 1–6. [Google Scholar] [CrossRef]


© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chesi, G.; Olivares, S.; Paris, M.G.A. Squeezing-Enhanced Phase-Shift-Keyed Binary Communication in Noisy Channels. Proceedings 2019, 12, 58. https://doi.org/10.3390/proceedings2019012058
Chesi G, Olivares S, Paris MGA. Squeezing-Enhanced Phase-Shift-Keyed Binary Communication in Noisy Channels. Proceedings. 2019; 12(1):58. https://doi.org/10.3390/proceedings2019012058
Chicago/Turabian StyleChesi, Giovanni, Stefano Olivares, and Matteo G. A. Paris. 2019. "Squeezing-Enhanced Phase-Shift-Keyed Binary Communication in Noisy Channels" Proceedings 12, no. 1: 58. https://doi.org/10.3390/proceedings2019012058
APA StyleChesi, G., Olivares, S., & Paris, M. G. A. (2019). Squeezing-Enhanced Phase-Shift-Keyed Binary Communication in Noisy Channels. Proceedings, 12(1), 58. https://doi.org/10.3390/proceedings2019012058





