Analytical Modelling of Magnetic Multimedia Control Elements Based on a Single Magnetic Sensor†
1
Carinthian Tech Research AG, Villach 9500, Austria
2
Infineon Technologies Austria, Villach 9500, Austria
3
Systems Design, Carinthia University of Applied Sciences, Villach 9500, Austria
*
Author to whom correspondence should be addressed.
†
Presented at the Eurosensors 2018 Conference, Graz, Austria, 9–12 September 2018.
Proceedings 2018, 2(13), 840; https://doi.org/10.3390/proceedings2130840
Published: 4 December 2018
(This article belongs to the Proceedings of EUROSENSORS 2018)
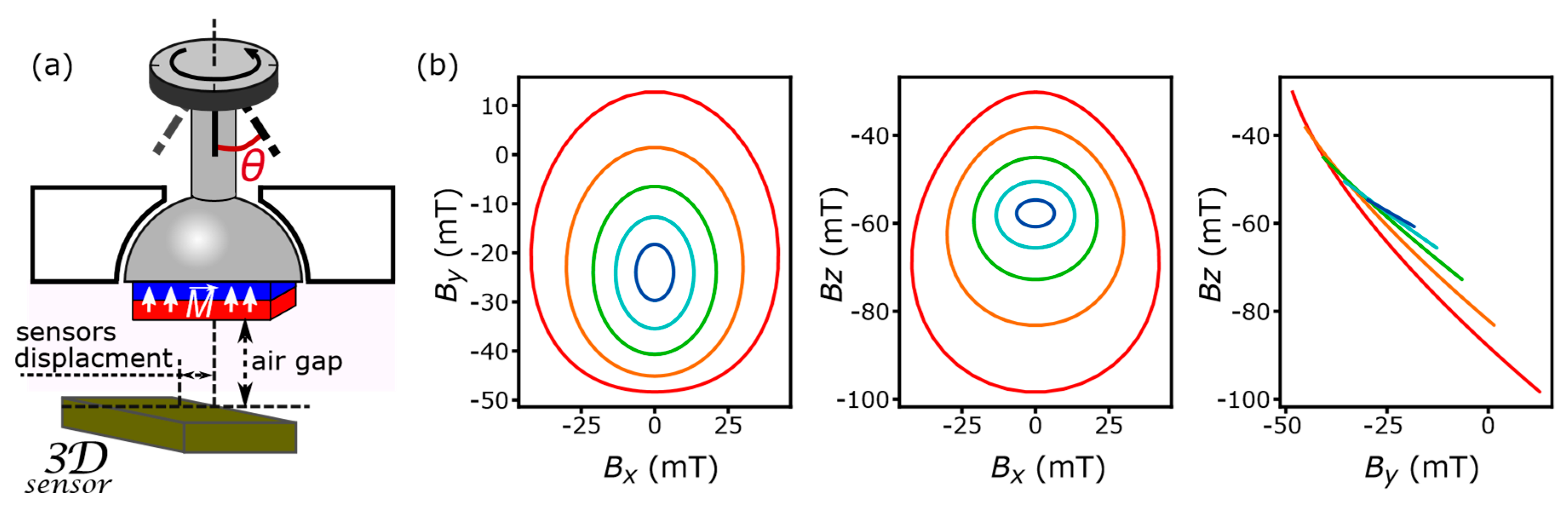
Magnetic multimedia control elements have many advantages in comparison with other systems based on mechanical, electrical, or optical readout. For system layouting, the practicality of analytical models over extensive numerical simulation was demonstrated in the past. In this work, we apply the analytical approach to design magnetic multimedia control elements. Particularly, we focus on a joystick that includes only a single 3D magnetic sensor and can be used in continuous operating mode. Presented results relate to a system based on a single permanent magnet and a single 3D sensor. The scheme was used to build a prototype to perform prove of principle experiments. A semi-analytic method to determine positions of the joystick from the observed magnetic field is discussed.
This is an open access article distributed under the Creative Commons Attribution License which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited
MDPI and ACS Style
Ermakova, A.; Martins, L.; Spitzer, D.; Ortner, M. Analytical Modelling of Magnetic Multimedia Control Elements Based on a Single Magnetic Sensor. Proceedings 2018, 2, 840.
Show more citation formats
Note that from the first issue of 2016, MDPI journals use article numbers instead of page numbers. See further details here.
