Simulation of the Readout Methods for Inductively Coupled High-Frequency Resonance Sensors †
Abstract
:1. Introduction
2. Methods
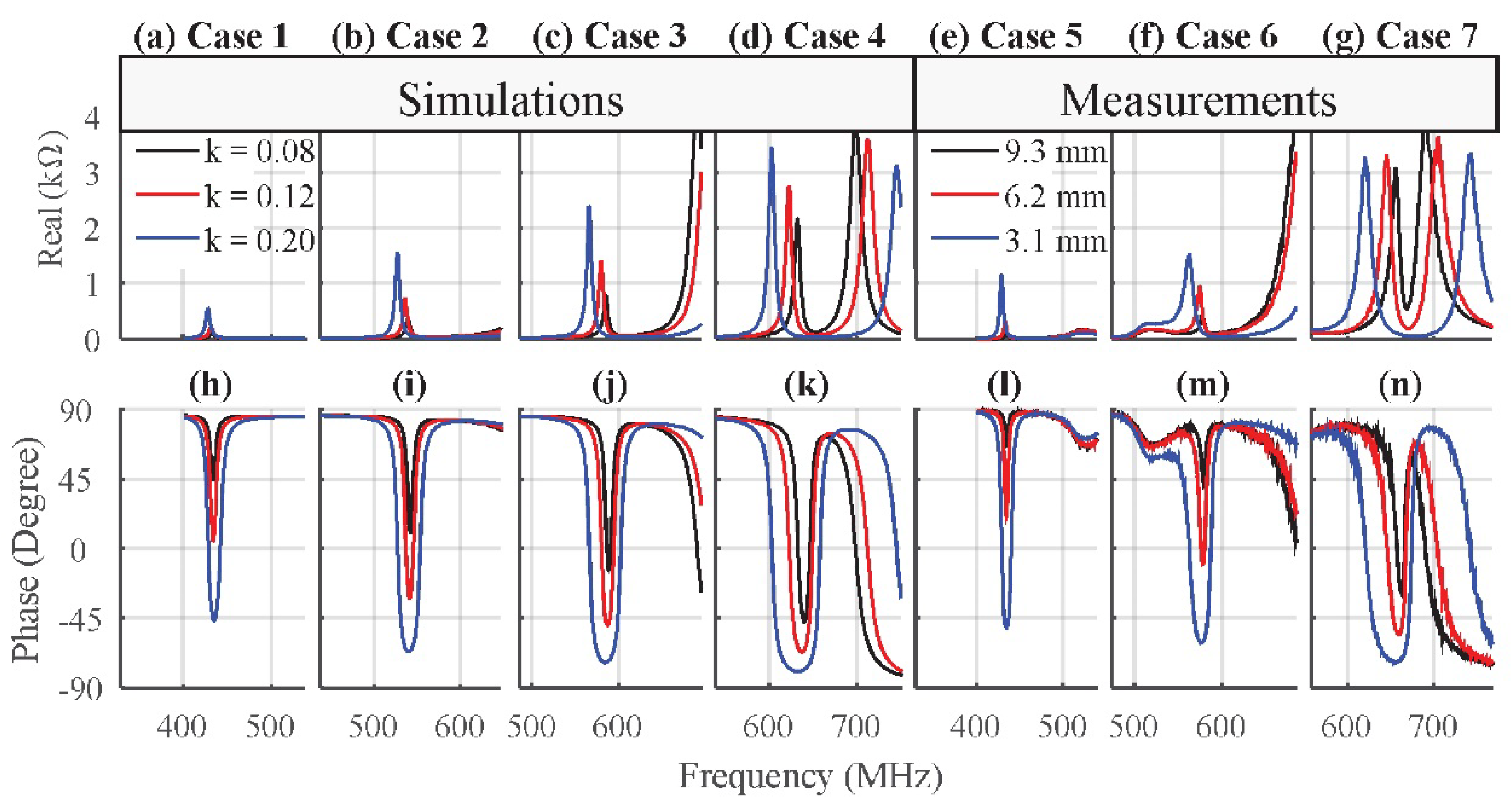
3. Results
4. Discussion
Acknowledgments
References
- Huang, Q.-A.; Dong, L.; Wang, L.-F. LC Passive Wireless Sensors Toward a Wireless Sensing Platform: Status, Prospects, and Challenges. J. Microelectromech. Syst. 2016, 25, 822–841. [Google Scholar] [CrossRef]
- Yousaf, A.; Khan, F.A.; Reindl, L. Passive Wireless Sensing of Micro coil parameters in fluidic environments. Sens. Actuators A Phys. 2012, 186, 69–79. [Google Scholar] [CrossRef]
- Potyrailo, R.A.; Mouquin, H.; Morris, W.G. Position-independent chemical quantitation with passive 13.56- MHz radio frequency identification (RFID) sensors. Talanta 2008, 75, 624–628. [Google Scholar] [CrossRef] [PubMed]
- Salpavaara, T.; Verho, J.; Kumpulainen, P.; Lekkala, J. Readout methods for an inductively coupled resonance sensor used in pressure garment application. Sens. Actuators A Phys. 2011, 172, 109–116. [Google Scholar] [CrossRef]
- Salpavaara, T.; Lekkala, J. A model based analysis of the measurement errors in inductively coupled passive resonance sensors. In Proceedings of the XXI IMEKO World Congress, Pague, Czech Republic, 30 August–4 September 2015. [Google Scholar]
- Salpavaara, T.; Verho, J.; Kumpulainen, P. Performance of a Near-Field Radio-Frequency Pressure Sensing Method in Compression Garment Application. In Proceedings of the Wireless Mobile Communication and Healthcare: Second International ICST Conference, MobiHealth 2011, Cos Island, Greece, 5–7 October 2011. [Google Scholar]
- Salpavaara, T. Inductively Coupled Passive Resonance Sensors: Readout Methods and Applications. Ph.D. Thesis, Tampere University of Technology, Tampere, Finland, 1 March 2018. [Google Scholar]
- Terman, F.E.; Helliwell, R.A.; Pettit, J.M.; Watkins, D.A.; Rambo, W.R. Electronic and Radio Engineering, 4th ed.; McGraw Hill: New York, NY, USA, 1955. [Google Scholar]


| Parameter | Symbol | Value |
|---|---|---|
| Reader and sensor coils | Lr and Ls | 27 nH |
| Parallel capacitance | Cr | 2.01 pF |
| Sensor capacitance | Cs | 4.99, 3.19, 2.69, 2.26 pF (Cases 1–4) |
| Resistance of Lr | Rr | 3 Ω |
| Resistance of the sensor | Rs | 1 Ω |
| Coupling coefficient | k | 0–0.32 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Salpavaara, T.; Hänninen, A.; Lekkala, J.; Kellomäki, M. Simulation of the Readout Methods for Inductively Coupled High-Frequency Resonance Sensors. Proceedings 2018, 2, 923. https://doi.org/10.3390/proceedings2130923
Salpavaara T, Hänninen A, Lekkala J, Kellomäki M. Simulation of the Readout Methods for Inductively Coupled High-Frequency Resonance Sensors. Proceedings. 2018; 2(13):923. https://doi.org/10.3390/proceedings2130923
Chicago/Turabian StyleSalpavaara, Timo, Aleksi Hänninen, Jukka Lekkala, and Minna Kellomäki. 2018. "Simulation of the Readout Methods for Inductively Coupled High-Frequency Resonance Sensors" Proceedings 2, no. 13: 923. https://doi.org/10.3390/proceedings2130923
APA StyleSalpavaara, T., Hänninen, A., Lekkala, J., & Kellomäki, M. (2018). Simulation of the Readout Methods for Inductively Coupled High-Frequency Resonance Sensors. Proceedings, 2(13), 923. https://doi.org/10.3390/proceedings2130923




