Modelling a Holographic Particle Counter †
Abstract
:1. Introduction
2. Materials and Methods
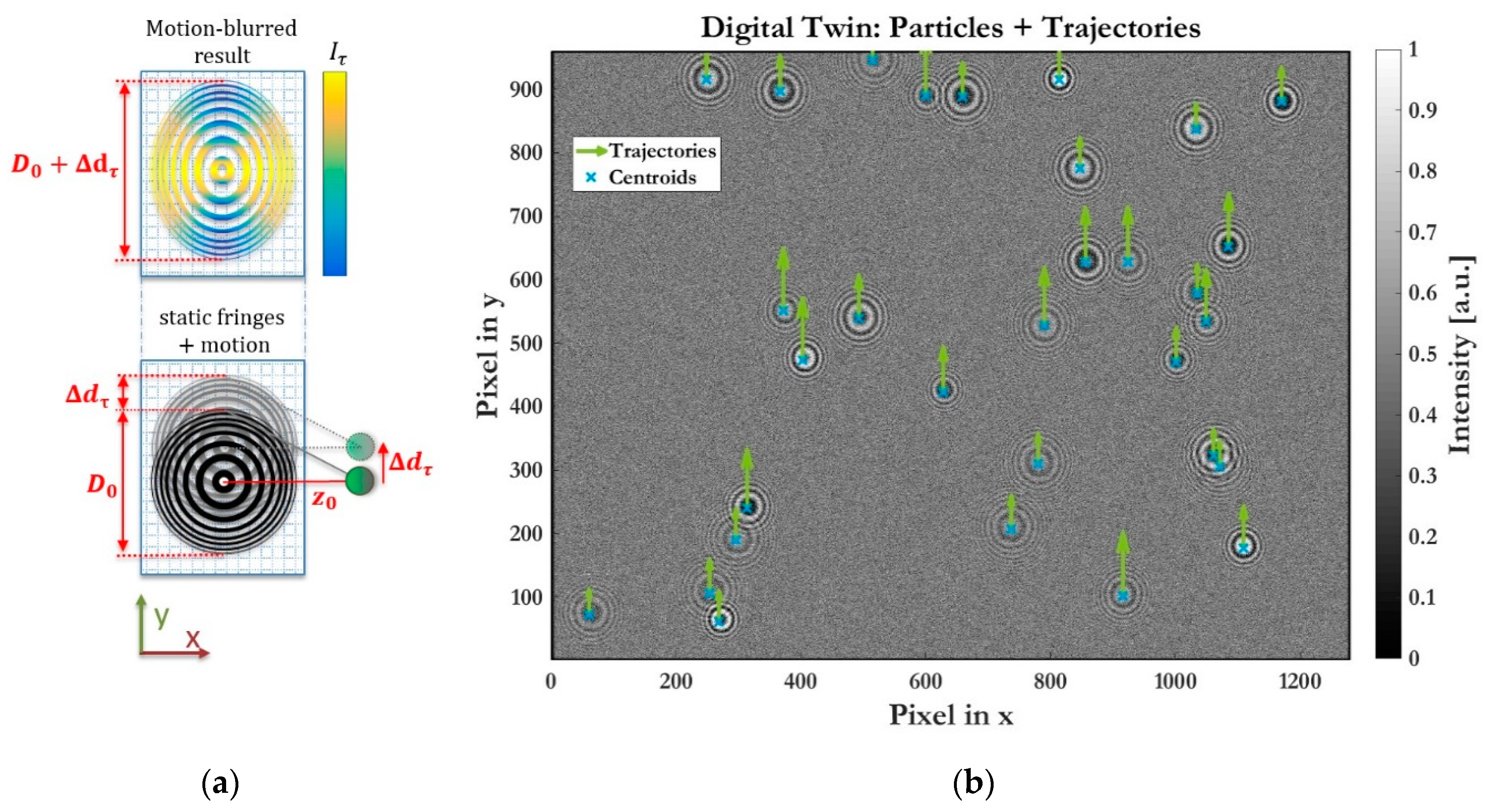
2.1. In-Line Holography Model
2.2. Aerosol Particle Model
3. Results
4. Conclusions
Acknowledgments
Conflicts of Interest
References
- Giechaskiel, B.; Maricq, M.; Ntziachristos, L.; Dardiotis, C.; Wang, X.; Axmann, H.; Bergmann, A.; Schindler, W. Review of motor vehicle particulate emissions sampling and measurement: From smoke and filter mass to particle number. J. Aerosol. Sci. 2014, 67, 48–86. [Google Scholar] [CrossRef]
- Brunnhofer, G.; Bergmann, A.; Kraft, M. Concept for a holographic particle counter. In Proceedings of the 2017 IEEE Photonics Conference (IPC), Orlando, FL, USA, 1–5 October 2017; pp. 581–582. [Google Scholar] [CrossRef]
- Poon, T.C.; Liu, J.P. Introduction to Modern Digital Holography; Cambridge University Press: Cambridge, UK, 2014; p. 223. [Google Scholar]
- Gire, J.; Denis, L.; Fournier, C.; Thiébaut, E.; Soulez, F.; Ducottet, C. Digital holography of particles: Benefits of the ’inverse problem’ approach. Meas. Sci. Technol. 2008, 19, 074005. [Google Scholar] [CrossRef]
- Dixon, L.; Cheong, F.C.; Grier, D.G. Holographic particle-streak velocimetry. Opt. Express 2011, 19, 4393–4398. [Google Scholar] [CrossRef] [PubMed]
- Center for X-ray Optics. Fresnel Zone Plate Theory, Generation, Tolerancing, Fabrication, and Applications. 2014. Available online: http://Zoneplate.Lbl.Gov/Theory (accessed on 19 June 2018).

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Brunnhofer, G.; Bergmann, A. Modelling a Holographic Particle Counter. Proceedings 2018, 2, 967. https://doi.org/10.3390/proceedings2130967
Brunnhofer G, Bergmann A. Modelling a Holographic Particle Counter. Proceedings. 2018; 2(13):967. https://doi.org/10.3390/proceedings2130967
Chicago/Turabian StyleBrunnhofer, Georg, and Alexander Bergmann. 2018. "Modelling a Holographic Particle Counter" Proceedings 2, no. 13: 967. https://doi.org/10.3390/proceedings2130967
APA StyleBrunnhofer, G., & Bergmann, A. (2018). Modelling a Holographic Particle Counter. Proceedings, 2(13), 967. https://doi.org/10.3390/proceedings2130967




