Analysis of Swing Movement in Ballroom Dancing †
Abstract
:1. Introduction
- The music has a fixed tempo for each dance and is constant throughout,
- The movement of dance is based on natural human gait,
- The sequence of dance is not fixed in advance (non-sequential, or non-provisional).
- The man leads the dance movements and the lady follows.
2. Mechanical Energy Consideration and Expression of Rise and Fall as a Mathematical Function
2.1. Potential and Kinetic Energy Exchange in Rise and Fall Movement
Count 1 (first beat in the bar (measure) in the music): Starting height (Down), Commence to rise e/o (end of) 1 (NFR in case of backward step), Count 2 (Second beat in the bar): Continue to rise, and Count 3(Third beat in the bar): Continue to rise, Lower e/o 3.
2.2. Expression of Rise and Fall As a Mathematical Function
3. Rise and Fall in Each Figure
3.1. Rise and Fall in Waltz
Natural Turn type, Spin Turn type, Chassé from PP type, Turning Lock type, Man’s Telemark type, Lady’s Telemark type, Double Reverse Spin type, and No Rise and Fall type. Other figures not included in these types, such as Fallaway Reverse, Bounce Fallaway, Reverse Corté, Wing, Cross Hesitation, and Contra Check have some ambiguity in which the dancer may choose arbitrarily.
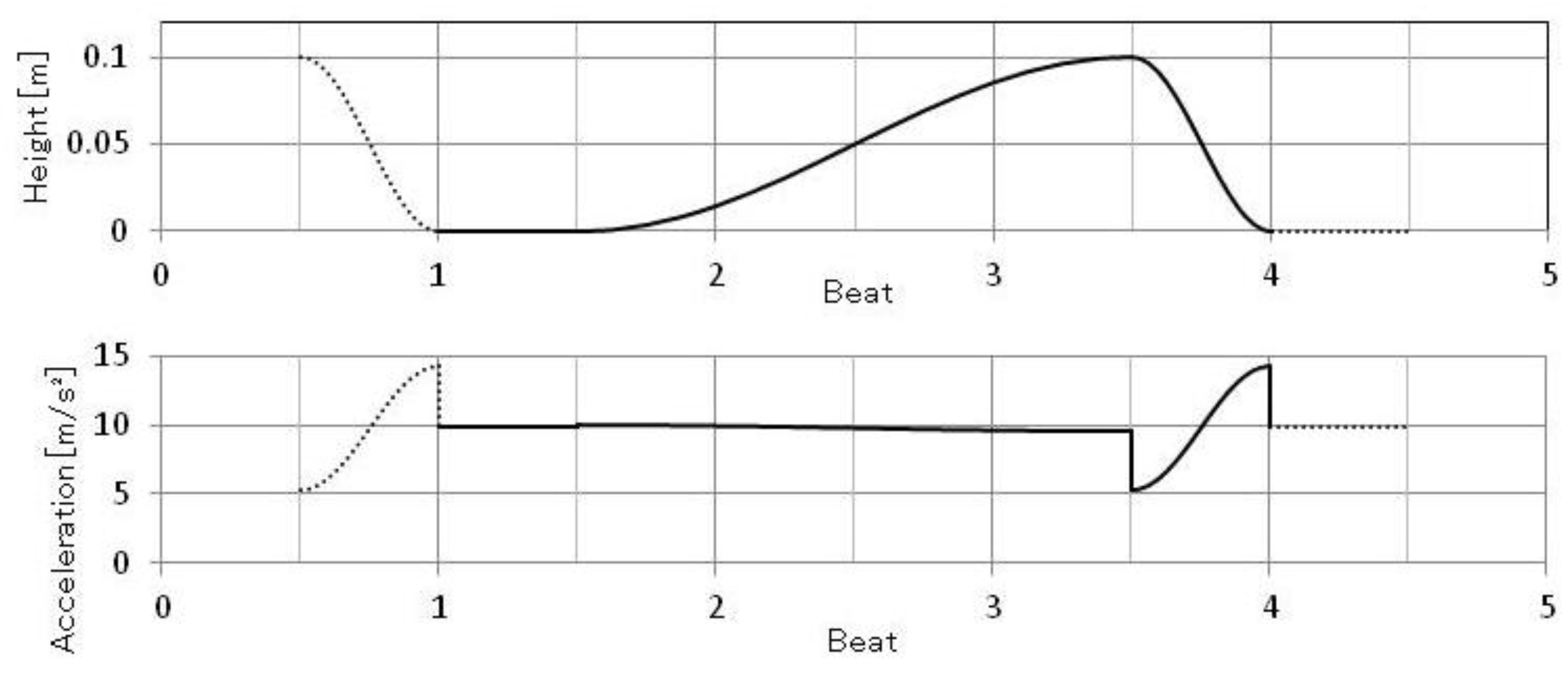
3.1.1. Natural Turn Type
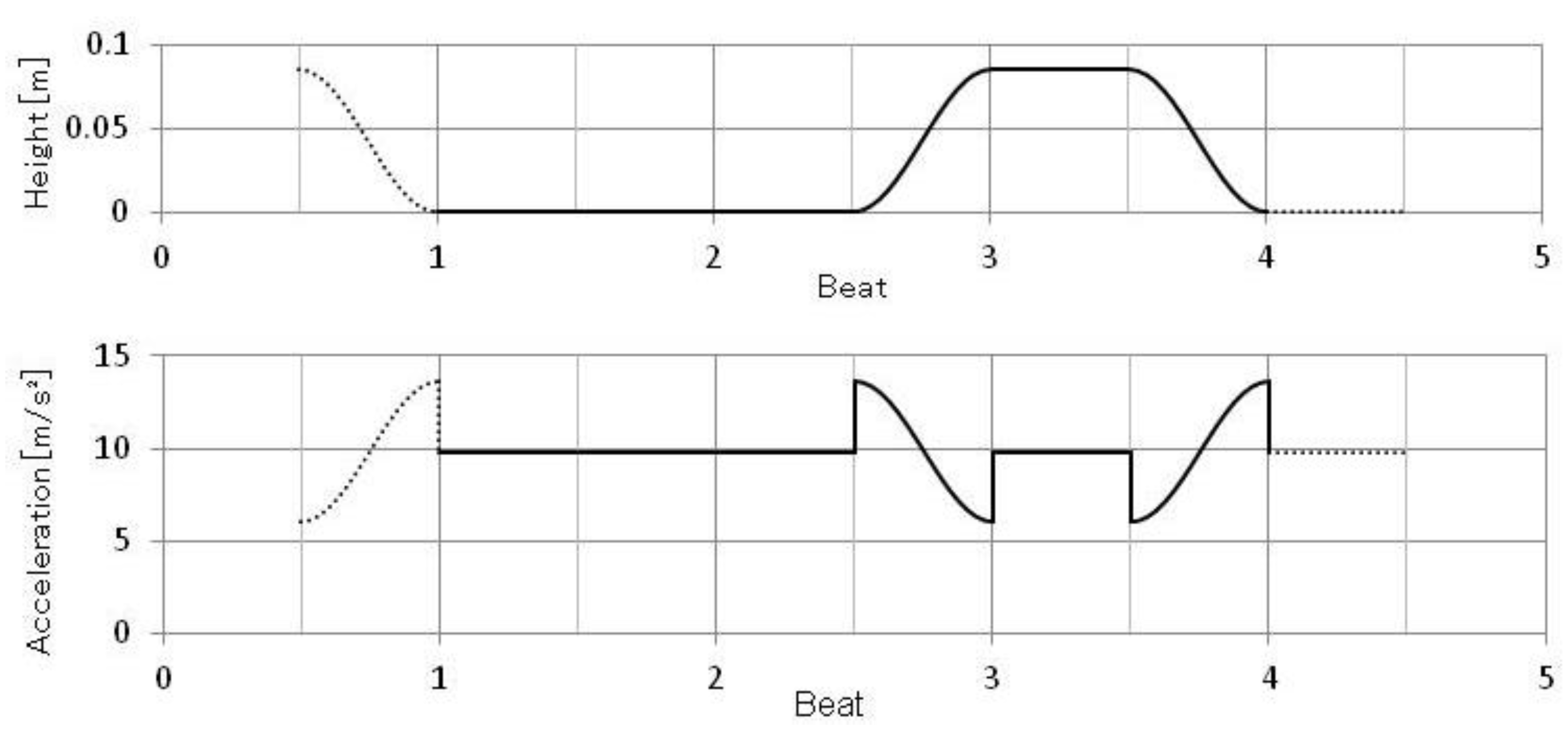
3.1.2. Spin Turn Type
No rise (Down) from the first beat to the middle of second beat; Rise e/o 2, Up on 3, Lower e/o 3. The characteristics of this type are no rise in Count 1, rapid rise e/o Count 2, and “Up” in count 3. The reason of the “Up” is that the step on this count is not closing to the other foot but end in the open position. The height of “Up” is lower than the rising height of Natural turn as explained in the above sub-section. The height and the acceleration are shown in Figure 2. Compared with Natural Turn, the Rise is more rapid and accordingly the change of load (acceleration) is more remarkable. The duration of “Up” in Count 3 becomes shorter if the Turning Lock follows.
3.1.3. Other Type of Rise and Fall
4. Limitation of Rise and Fall in Swing Dances
5. Conclusions
References
- Silvester, V. Modern Ballroom Dancing: History and Practice; Barrie and Jenkins, Ltd.: London, UK, 1977; pp. 1–249. [Google Scholar]
- Moore, A. Ballroom Dancing, 10th ed.; A&C Black Publishers Ltd.: London, UK, 2002; pp. 1–308. [Google Scholar]
- Lavelle, D. Latin & American Dances, 1st ed.; A&C Black Publishers Ltd.: London, UK, 1965; revised ed.; Sportshelf & Soccer Assoc: 1975; pp. 1–194. [Google Scholar]
- ISTD (The Imperial Society of Teachers of Dancing) (Ed.) The Ballroom Technique, 10th ed.; 1982; pp. 1–134. [Google Scholar]
- ISTD (The Imperial Society of Teachers of Dancing) (Ed.) Viennese Waltz, B. D. C., Recommended Version; Code 105; British Dance Council: London, UK, 2001; pp. 1–8. [Google Scholar]
- Howard, G. The Technique of Ballroom Dancing, 1st ed.; IDTA: Brighton, UK, 1976; new ed.; 1995; pp. 1–131. [Google Scholar]
| Waltz | Foxtrot | Viennese Waltz | Quickstep | ||
|---|---|---|---|---|---|
| Tempo (bpm) | 30 | 30 | 60 | 50 | |
| Beat length (s) | 2/3 | 0.5 | 1/3 | 0.3 | |
| Lowering duration | Latter half of count 3 (half beat) | Latter half of count Q (half beat) | Latter half of count 3 (half beat) | Latter half of count Q (half beat) | Latter half of count S (one beat) |
| Maximum height (m) | 0.221 | 0.124 | 0.055 | 0.045 | 0.179 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2018 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shioya, T. Analysis of Swing Movement in Ballroom Dancing. Proceedings 2018, 2, 224. https://doi.org/10.3390/proceedings2060224
Shioya T. Analysis of Swing Movement in Ballroom Dancing. Proceedings. 2018; 2(6):224. https://doi.org/10.3390/proceedings2060224
Chicago/Turabian StyleShioya, Tadashi. 2018. "Analysis of Swing Movement in Ballroom Dancing" Proceedings 2, no. 6: 224. https://doi.org/10.3390/proceedings2060224
APA StyleShioya, T. (2018). Analysis of Swing Movement in Ballroom Dancing. Proceedings, 2(6), 224. https://doi.org/10.3390/proceedings2060224




