A Methodology to Parameterize Wire + Arc Additive Manufacturing: A Case Study for Wall Quality Analysis
Abstract
1. Introduction
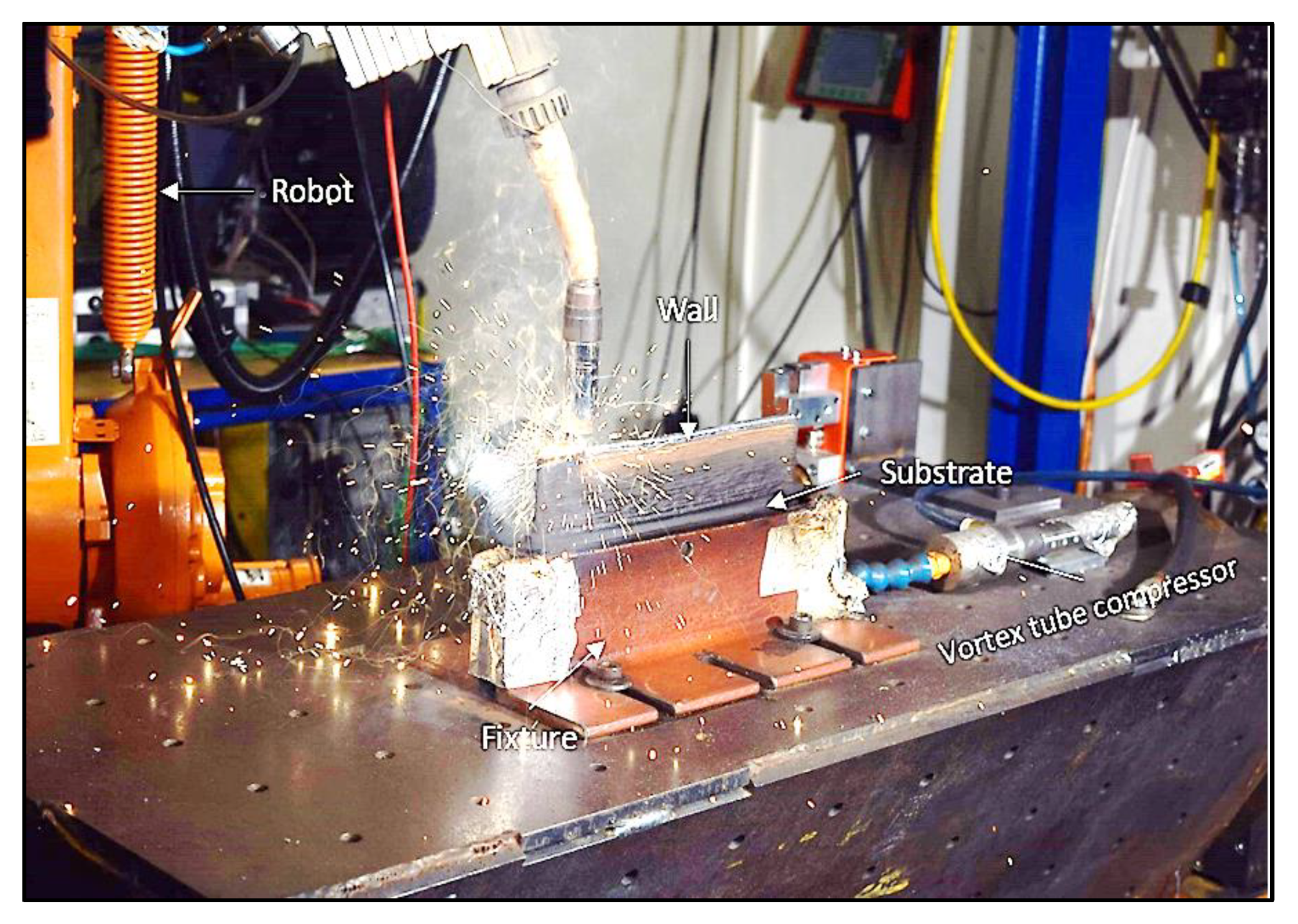
2. Methodology, Materials, and Equipment
- Less material to be removed;
- Higher mechanical properties;
- More robust condition;
- Fast production.
- The stage ‘mock design’: with the objective of finding, without experimentation, a rough operational map (first approximation operational map) to visualize the expected map and reduce further number of experiments;
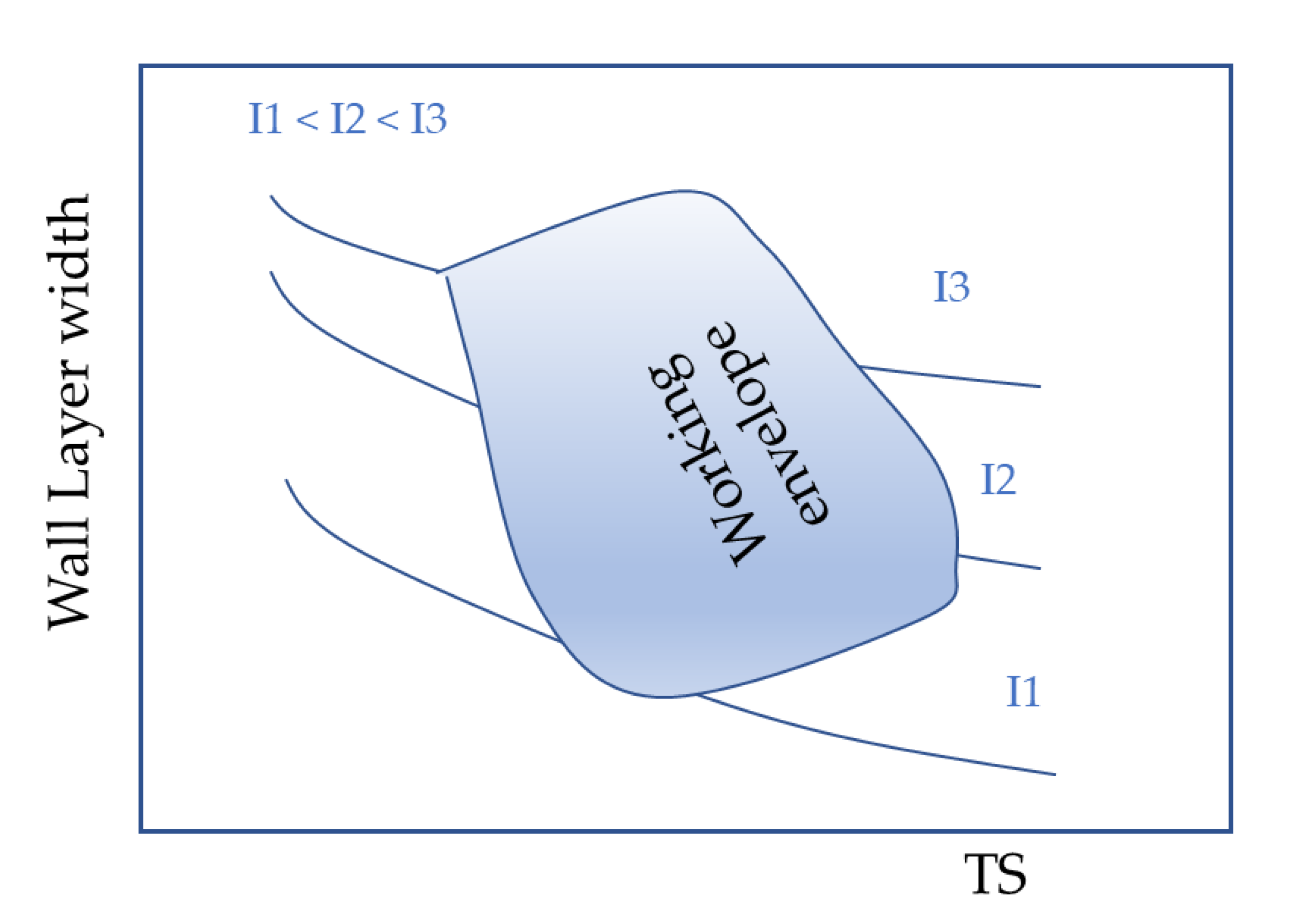
- The stage ‘pre-requisite for realistic operational map’: with the objective of determining the actual WFS, Im and deposition rate (second approximation operational map) and the operating limits of Im and TS towards a second approximation working envelope;
- The stage ‘realistic operational map’: with the objective of raising through a systematic design of experiment the actual working envelope;
- The stage ‘operational map validation’: with the objective of finding corresponding three parametric set (covering the whole range of operational map) to produce walls according to a target wall width.
- Arc pressure should be minimized to avoid pool lateral sag (CMT with low mean current values was employed);
- The pool should present a proper volume for a given deposition rate and current, i.e., a too small pool volume means low heat transferred to the prior layer, and a too large pool is prone to run downward (a range of TSs for a given current that would lead to proper pool volumes was determined);
- The material underneath each layer under deposition should be as cool as possible, so that the heat transfer through the built wall becomes easier (room temperature was adopted as the interlayer temperature, even at the expense of long production time).
3. Results
3.1. Mock Design of Operational Map (First Approximation Operational Map)
- The cross-section of the deposited layer is assumed to be semi-circular;
- The density of the material is constant through-out the material and assumed to be same as that for steels.
- Let,
- 2.
- At the same time, let,
- 3.
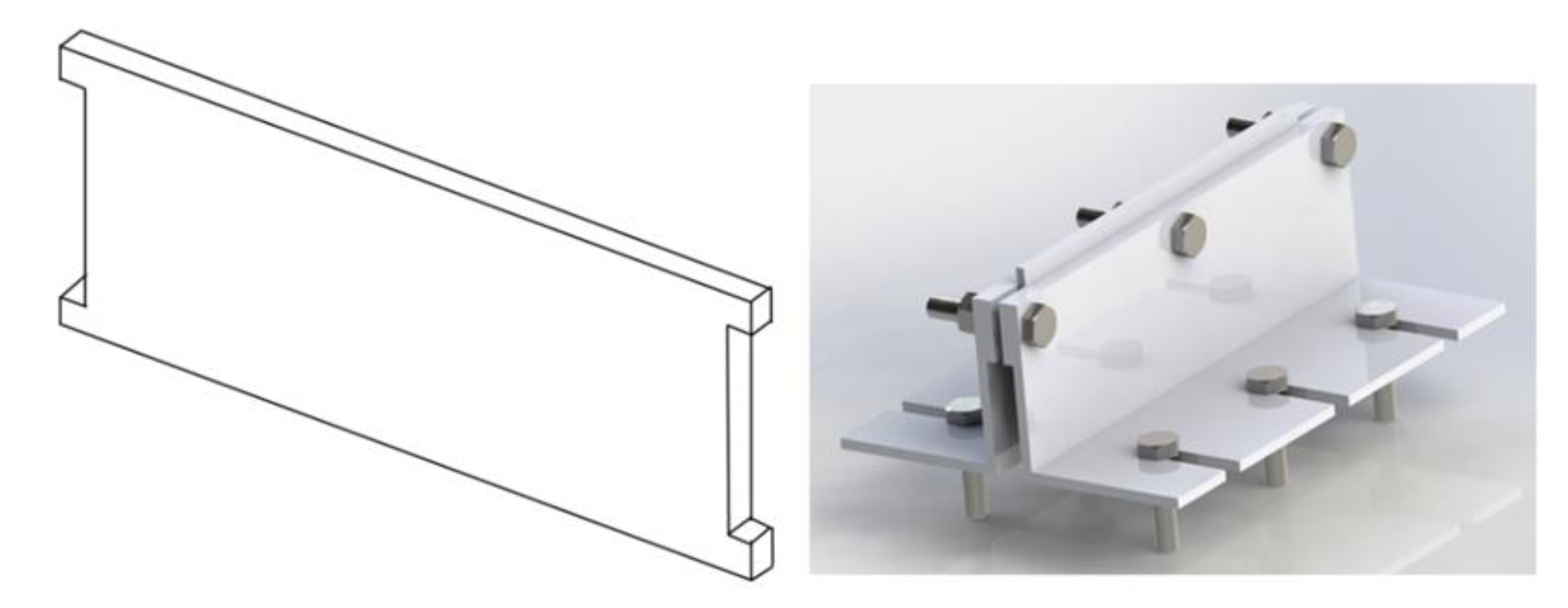
- The cross-section radius (r) would be half of the layer width (LW) and is calculated after equating Equations (1), (2) and (3), that is
- The layer width (LW) in millimeters is reached through Equation (5).
3.2. Pre-Requisite for Realistic Operational Map (Second Approximation Operational Map) and for the Operating Limits of Im and TS (Second Approximation Working Envelope)
3.2.1. Determination of Workable Current Range and Actual Deposition Rate
3.2.2. Determination of Workable TS Range
- a)
- Accepted level of lateral surface waviness: caused by higher current and/or lower travel speed;
- b)
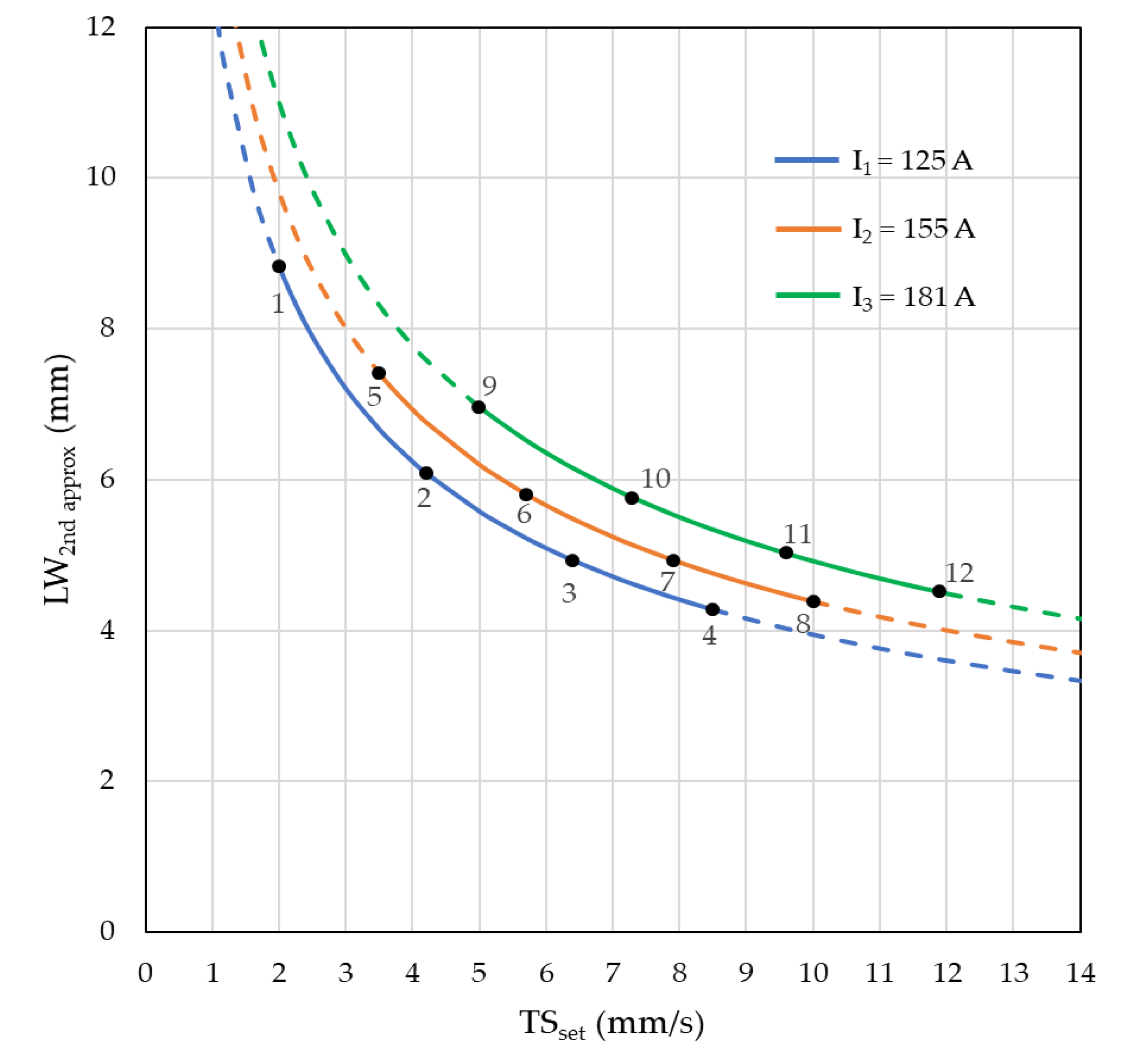
3.2.3. Second Approximation Working Envelope
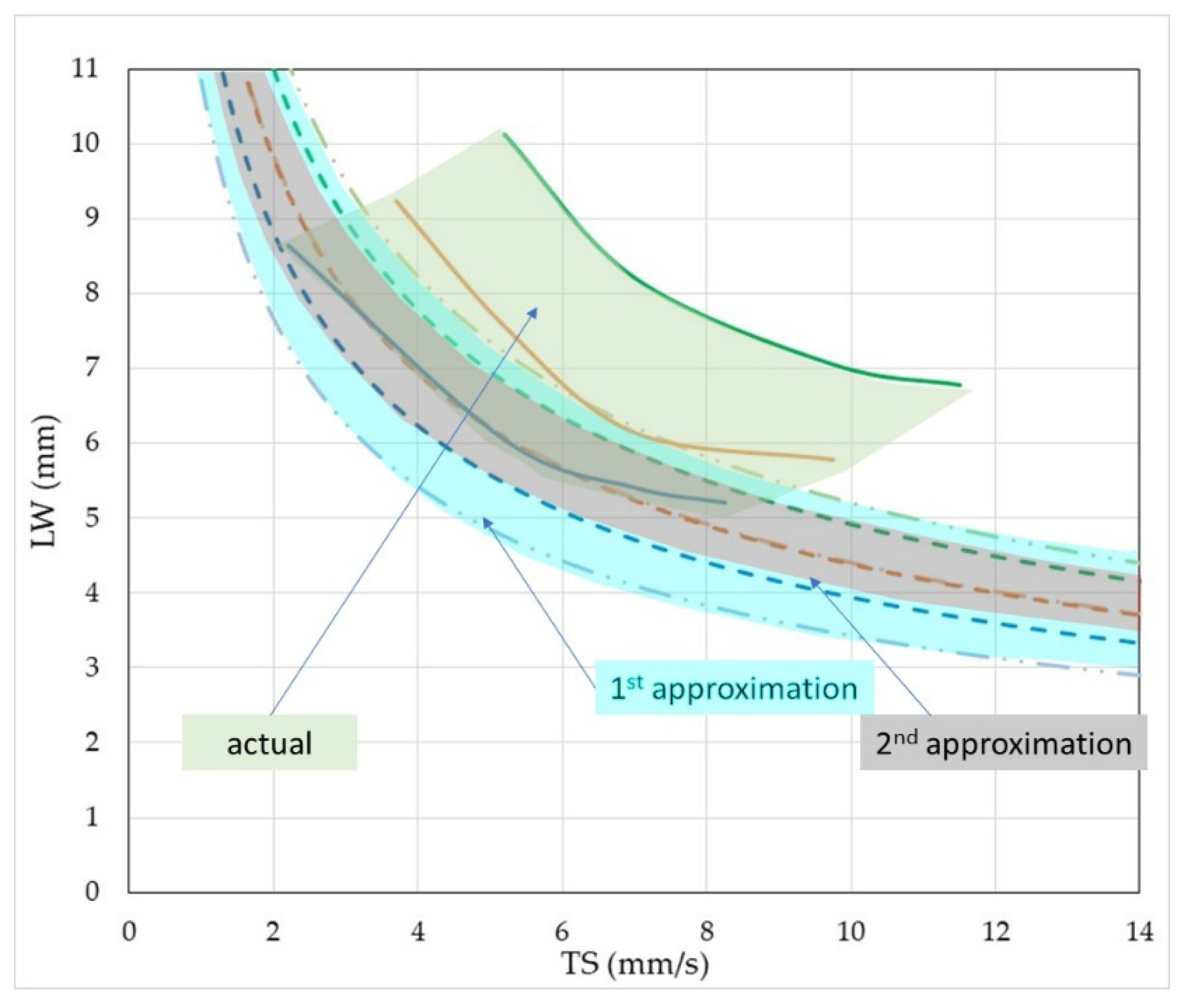
3.3. The ‘Realistic Operational Map’
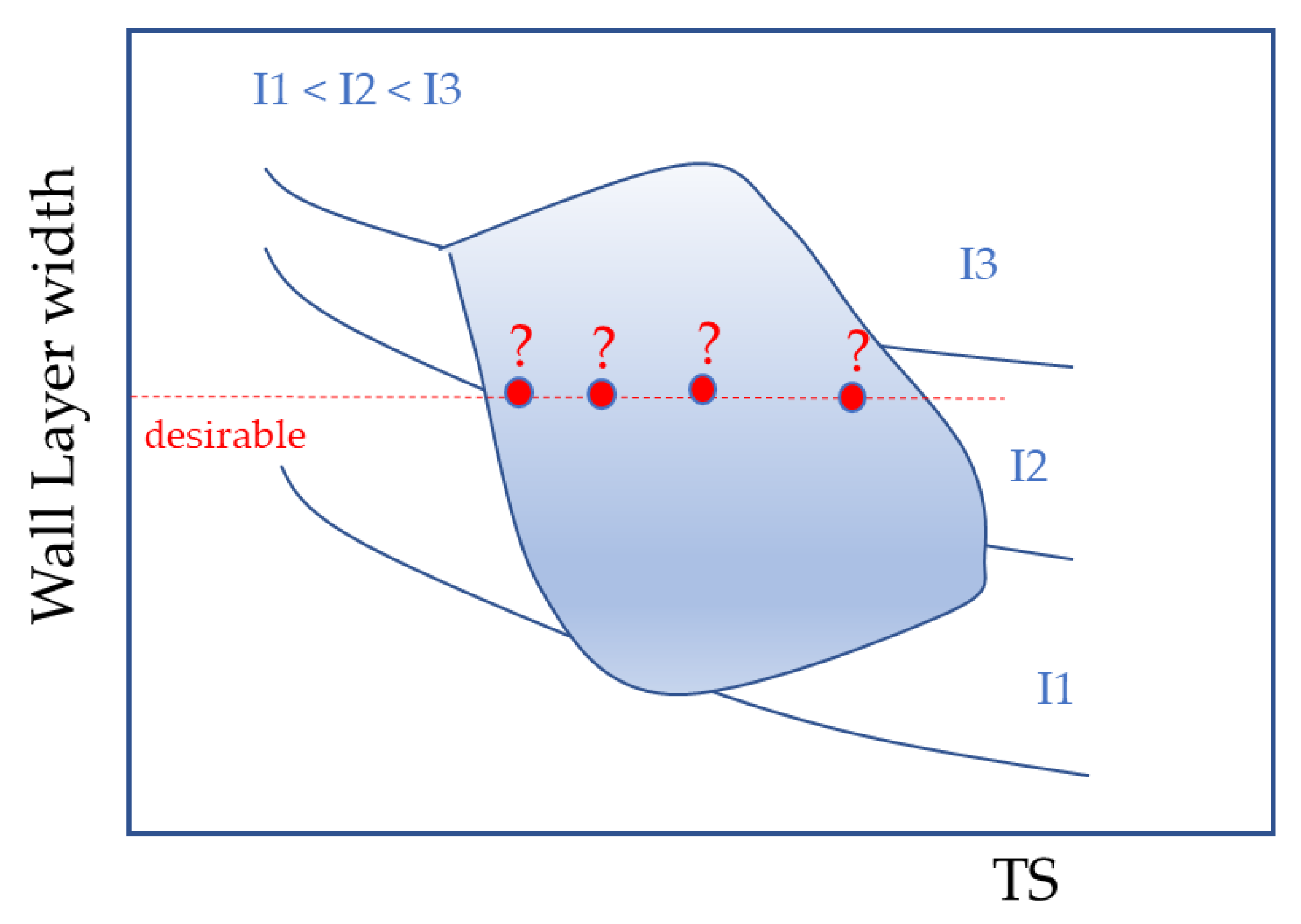
3.3.1. Experiments for the Generation of the Actual Operational Map and the Working Envelope
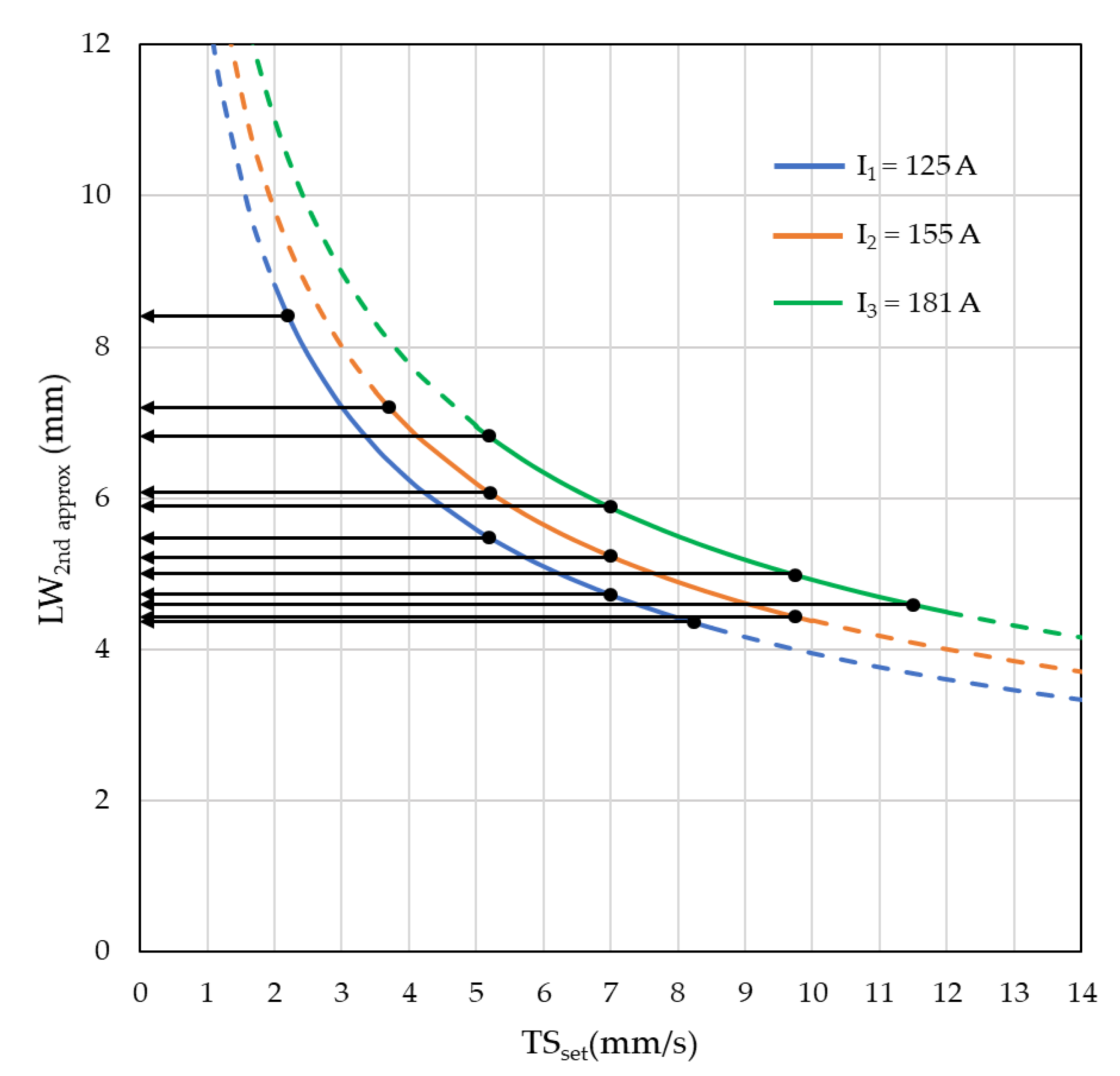
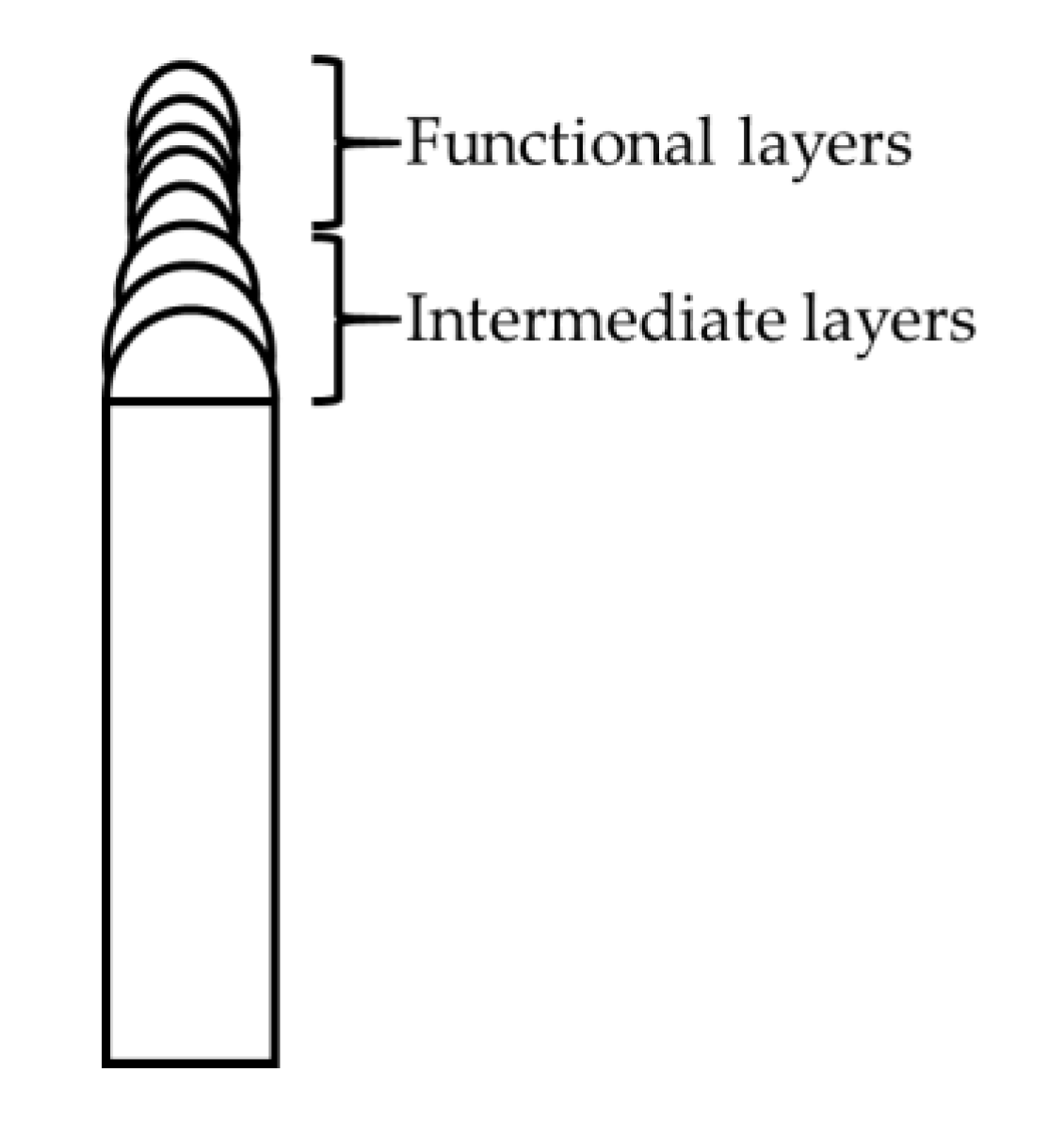
- If the difference between the two-layer thicknesses (or substrate and layer thickness) in the experiments is less than 1 mm, then there is no need to have intermediate layers;
- If the difference between the layers (or substrate and layer thickness) is between 1 mm and 2 mm, then one intermediate layer should be built;
- For layer thickness differences greater than 2 mm, to use arithmetic progression. To do so, keep the difference between the layers as 1 mm (consider negative value while calculating because layer width value is decreasing) and calculate the number of intermediate layers by Equation (6).
- 4.
- If ‘n’ comes out to be fraction, then approximate the value of ‘n’ to a higher whole number;
- 5.
- Recalculate the layer width difference and determine the widths for each intermediate layer.
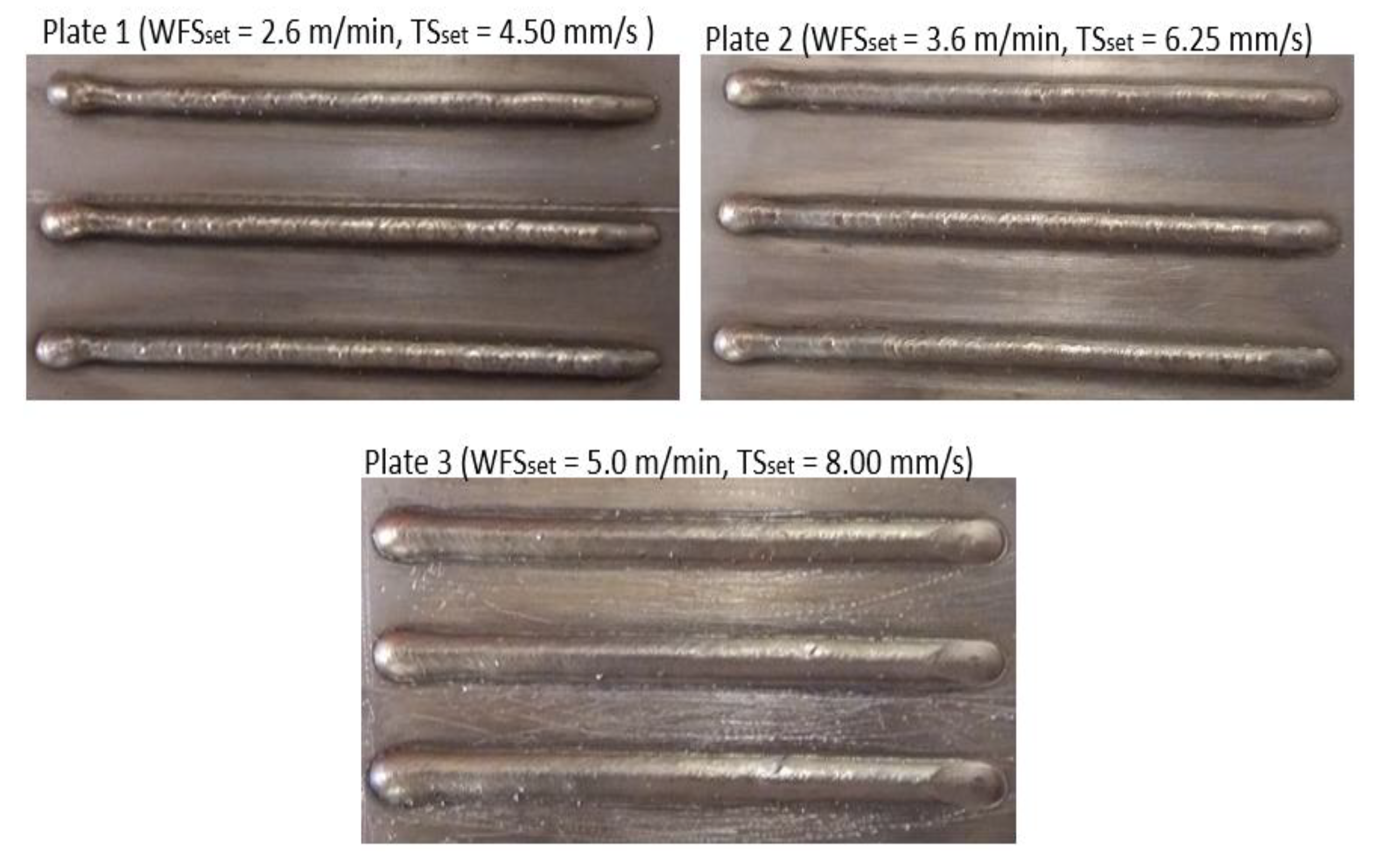
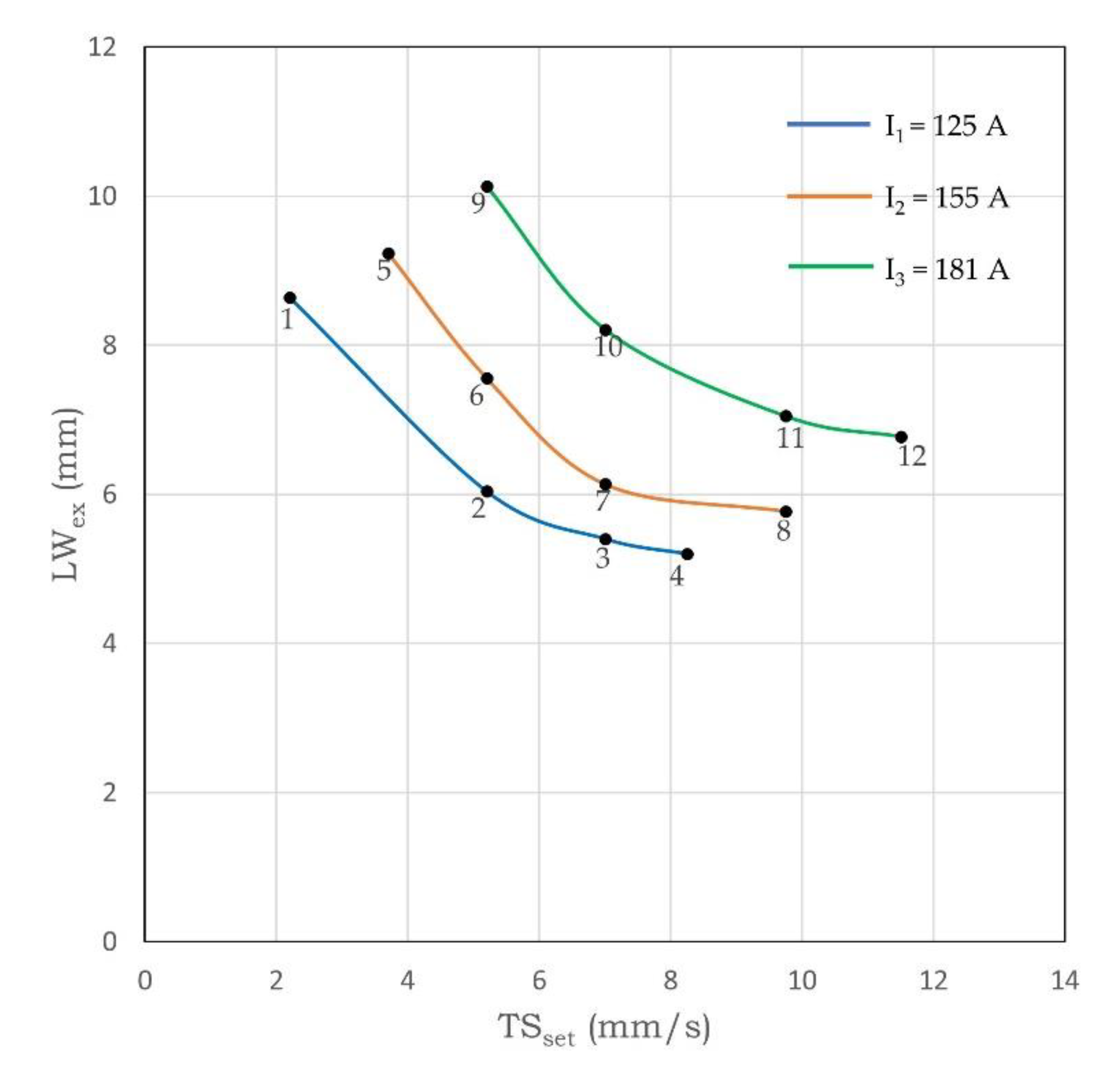
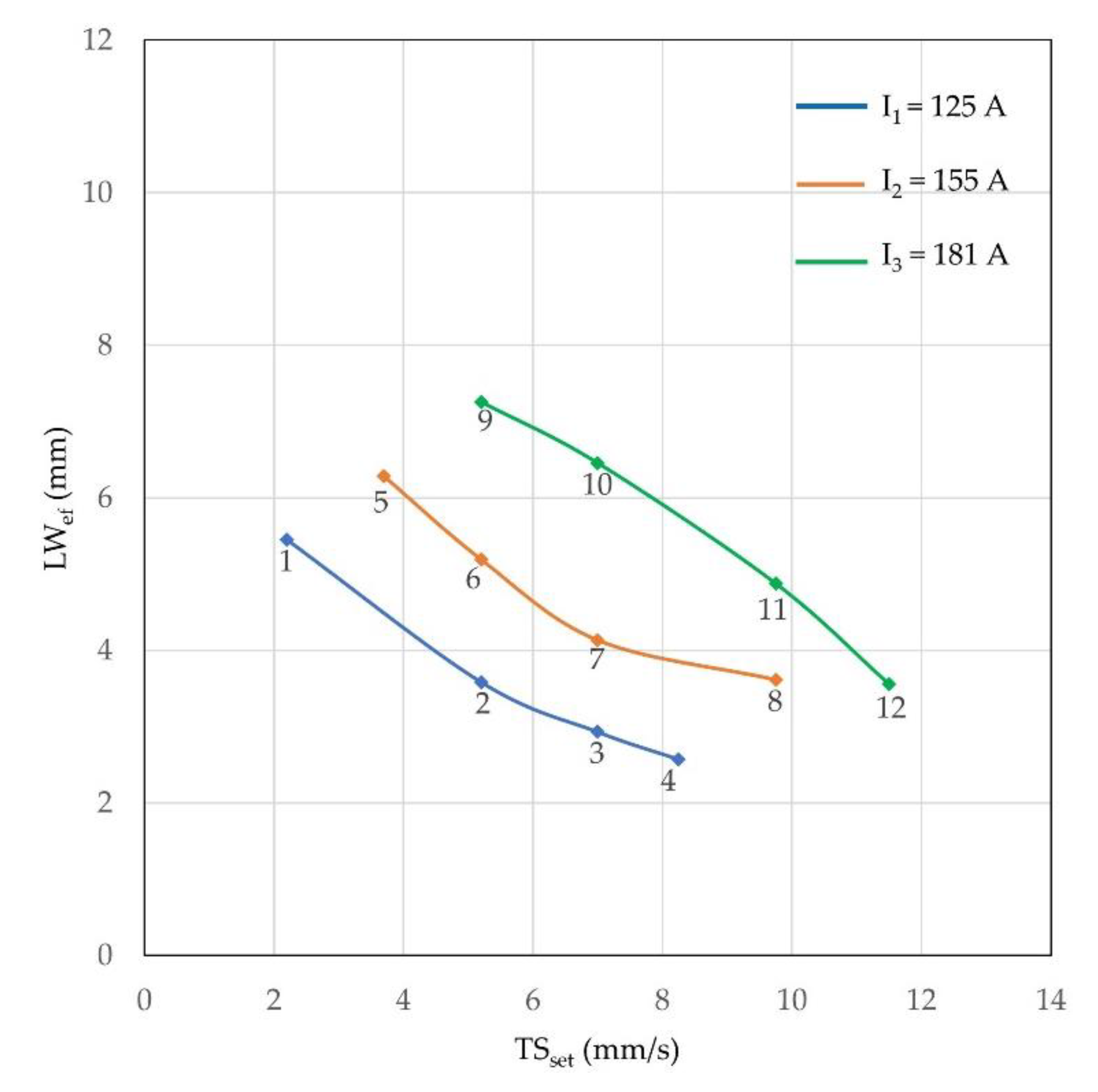
3.3.2. Experimental Responses and the Actual Operational Map
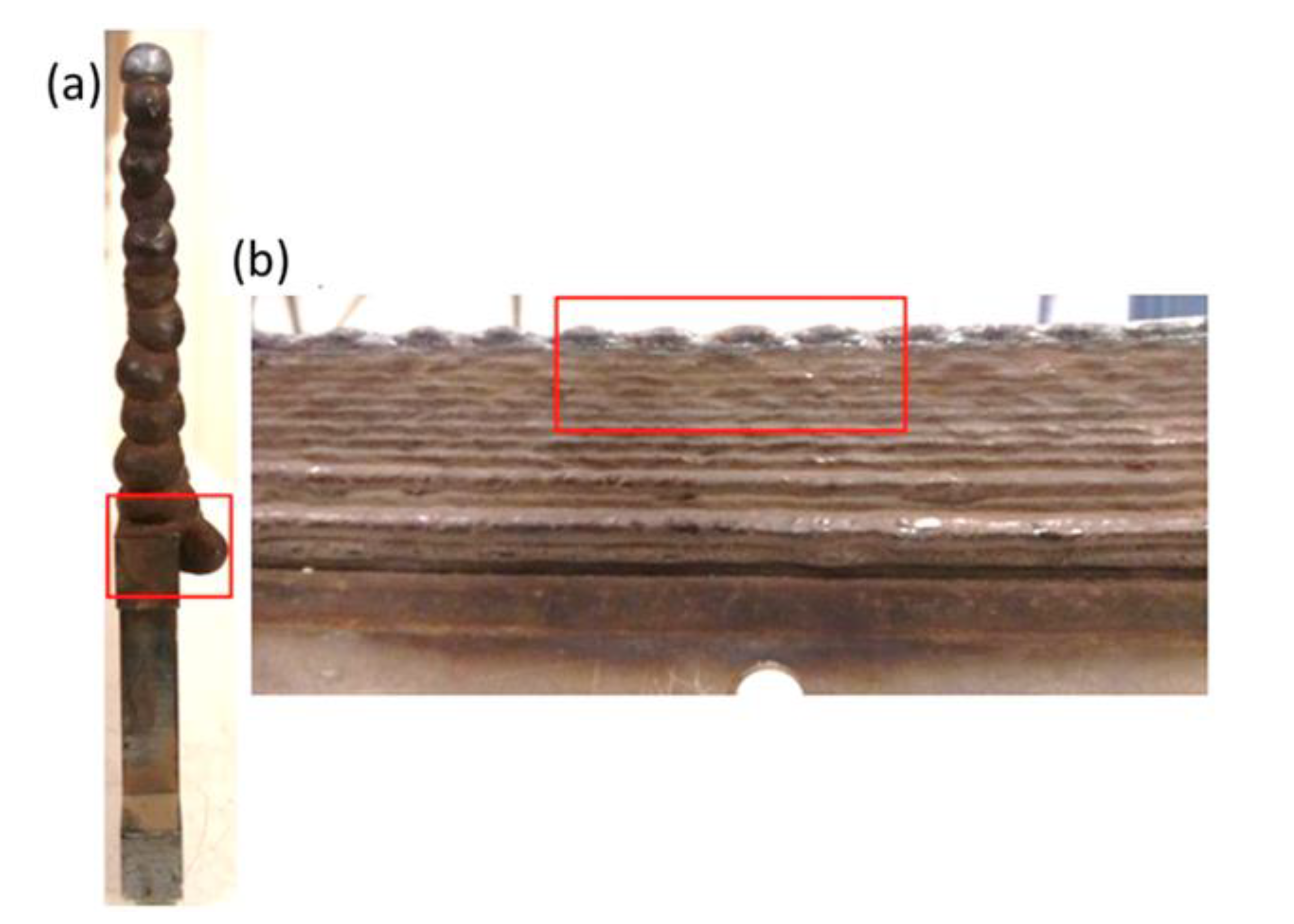
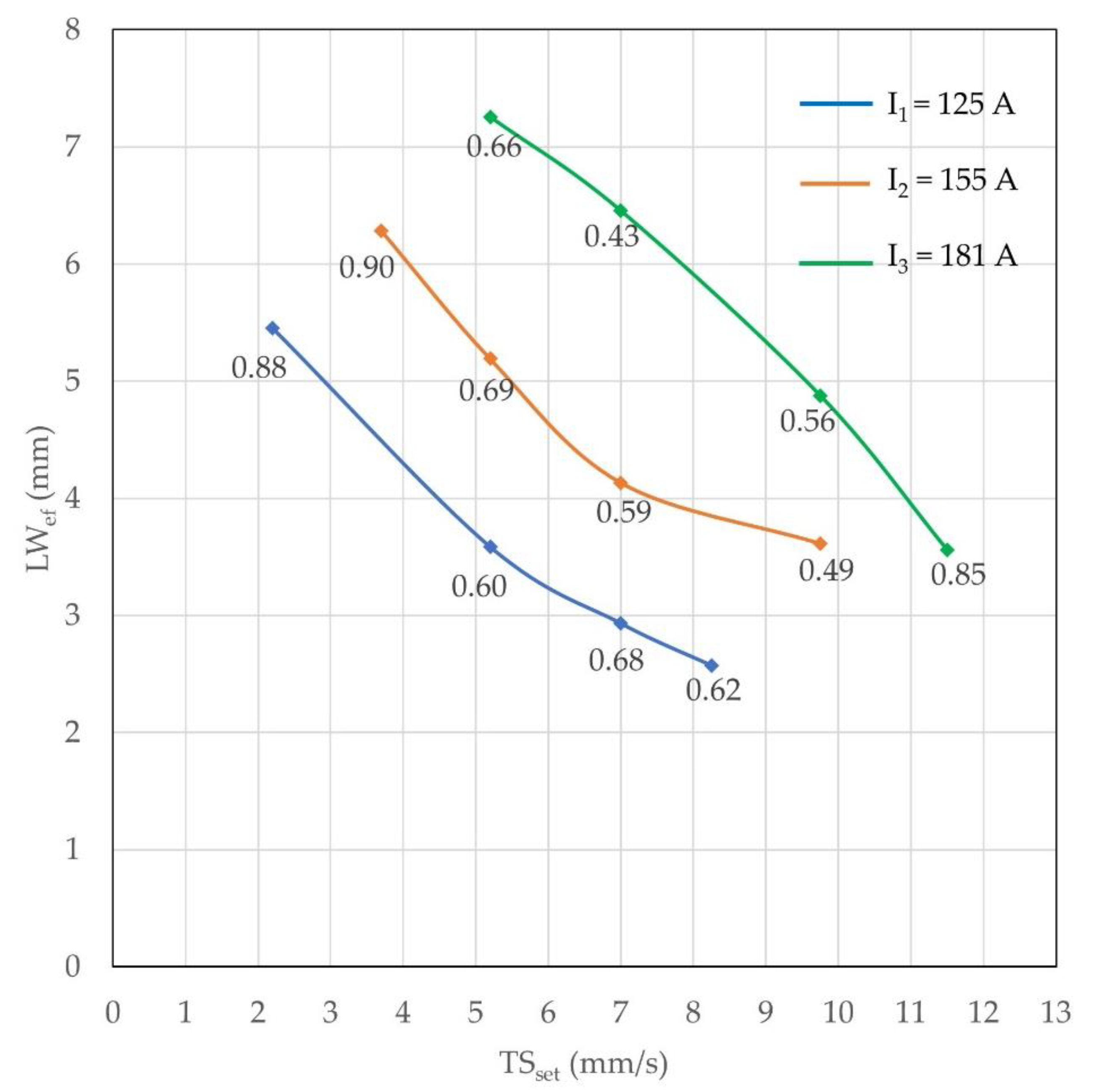
3.3.3. Waviness and the Actual Operational Map
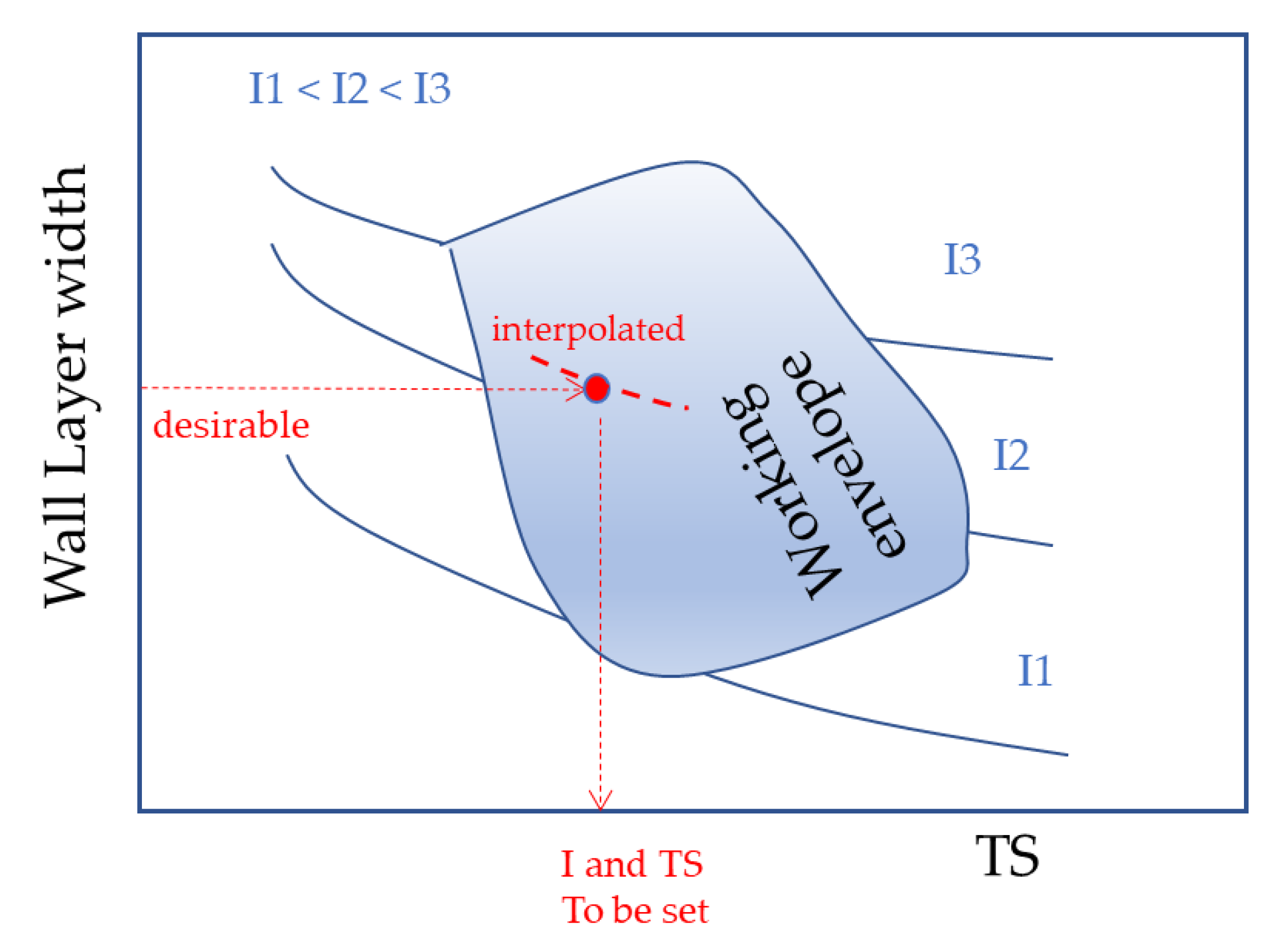
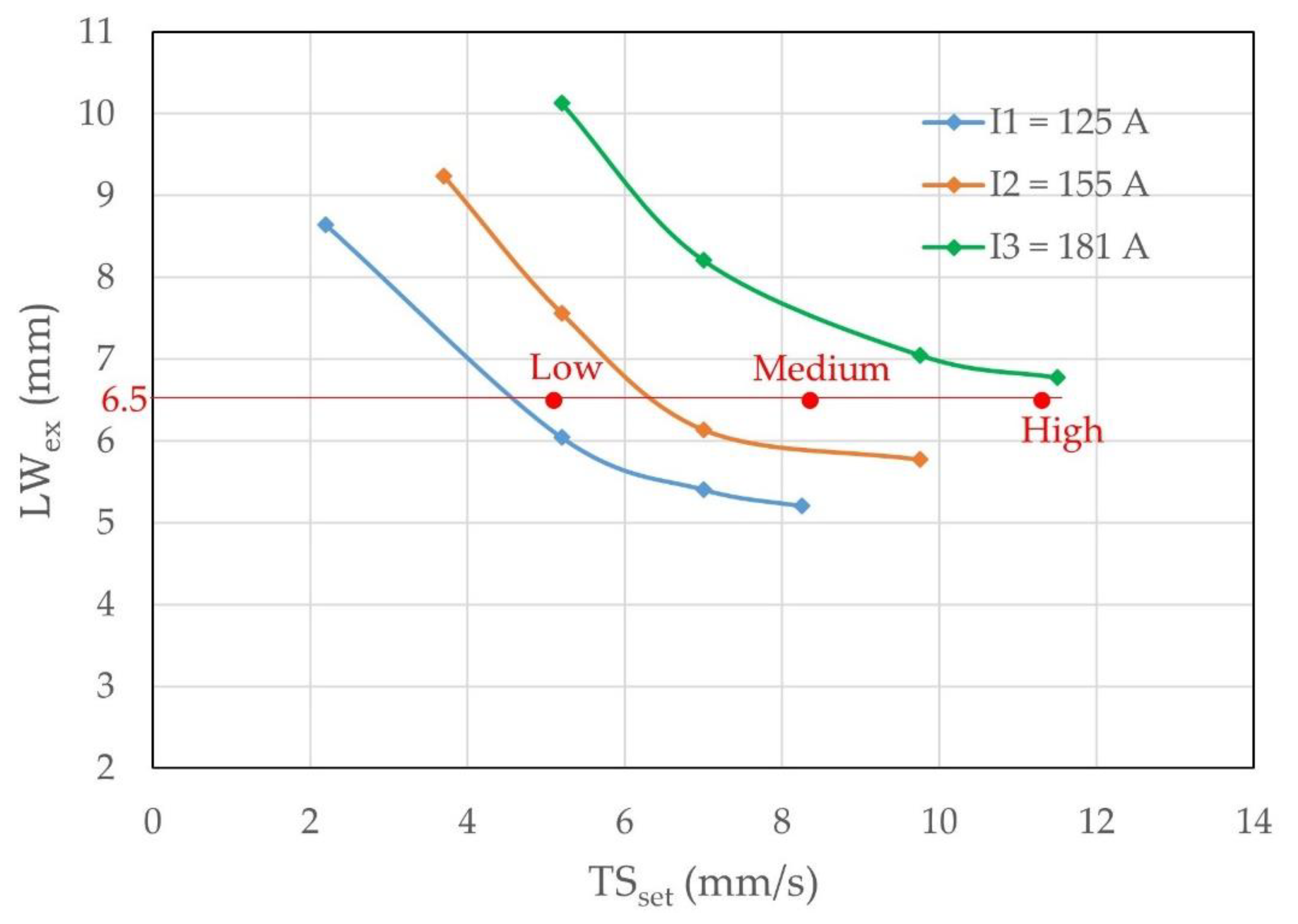
3.4. Operational Map Validation
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- DebRoy, T.; Wei, H.L.; Zuback, J.S.; Mukherjee, T.; Elmer, J.W.; Milewski, J.O.; Beese, A.M.; Wilson-Heid, A.; De, A.; Zhang, W. Additive manufacturing of metallic components—Process, structure and properties. Prog. Mater. Sci. 2018, 92, 112–224. [Google Scholar] [CrossRef]
- Williams, S.W.; Martina, F.; Addison, A.C.; Ding, J.; Pardal, G.; Colegrove, P. Wire + Arc additive manufacturing. Mater. Sci. Technol. 2016, 32, 641–647. [Google Scholar] [CrossRef]
- Milewski, J.O. Additive Manufacturing of Metals: From Fundamental Technology to Rocket Nozzles, Medical Implants, and Custom Jewelry; Springer: Berlin/Heidelberg, Germany, 2017; p. 343. [Google Scholar] [CrossRef]
- Ding, D.; Pan, Z.; Cuiuri, D.; Li, H. Wire-feed additive manufacturing of metal components: Technologies, developments and future interests. Int. J. Adv. Manuf. Technol. 2015, 81, 465–481. [Google Scholar] [CrossRef]
- Yehorov, Y.; da Silva, L.J.; Scotti, A. Balancing WAAM Production Costs and Wall Surface Quality through Parameter Selection: A Case Study of an Al-Mg5 Alloy Multilayer-Non-Oscillated Single Pass Wall. J. Manuf. Mater. Process. 2019, 3, 32. [Google Scholar] [CrossRef]
- Alonso, U.; Veiga, F.; Suárez, A.; Artaza, T. Experimental investigation of the influence of wire arc additive manufacturing on the machinability of titanium parts. Metals 2020, 10, 24. [Google Scholar] [CrossRef]
- Soderstrom, E.; Mendez, P. Humping mechanisms present in high speed welding. Sci. Technol. Weld. Join. 2006, 11, 572–579. [Google Scholar] [CrossRef]
- Nguyen, T.C.; Weckman, D.C.; Johnson, D.A.; Kerr, H.W. The humping phenomenon during high speed gas metal arc welding. Sci. Technol. Weld. Join. 2005, 10, 447–459. [Google Scholar] [CrossRef]
- Selvi, S.; Vishvaksenan, A.; Rajasekar, E. Cold metal transfer (CMT) technology—An overview. Def. Technol. 2018, 4, 28–44. [Google Scholar] [CrossRef]
- Lin, Z.; Goulas, C.; Ya, W.; Hermans, M.J. Microstructure and mechanical properties of medium carbon steel deposits obtained via wire and arc additive manufacturing using metal-cored wire. Metals 2019, 9, 673. [Google Scholar] [CrossRef]
- Purwaningrum, Y.; Triyono; Pu, M.W.; Alfarizi, F. Effect of shielding gas mixture on gas metal arc welding (GMAW) of low carbon steel (LR Grade A). Key Eng. Mater. 2016, 705, 250–254. [Google Scholar] [CrossRef]






| Dimension (mm) | Current (A) | Deposition Rate, DRd (kg/h) | Wire Feed Rate (m/min) | Voltage (V) | ||||
|---|---|---|---|---|---|---|---|---|
| Min | Max | Min | Max | Min | Max | Min | Max | |
| 1.2 | 100 | 320 | 1.3 | 7.5 | 1.8 | 12.0 | 16 | 32 |
| 1.4 | 120 | 380 | 1.6 | 7.5 | 2.0 | 9.0 | 16 | 34 |
| 1.6 | 140 | 450 | 1.6 | 8.0 | 1.5 | 8.5 | 18 | 36 |
| Current Level (A) | DR (kg/hr) |
|---|---|
| 100 | 1.30 |
| 130 | 2.14 |
| 160 | 2.99 |
| Bead | WFSset (m/min) | Iset (A) | TSset (mm/s) | Remarks (Good/Bad) |
|---|---|---|---|---|
| 1 | 4.2 | 160 | 8.00 | good |
| 2 | 1.4 | 100 | 6.00 | bad |
| 3 | 2.0 | 124 | 6.00 | bad |
| 4 | 2.2 | 125 | 6.00 | bad |
| 5 | 2.6 | 130 | 4.50 | good |
| 6 | 5.0 | 180 | 8.00 | good |
| 7 | 3.6 | 155 | 6.25 | good |
| Itarget (A) | WFSset (m/min) | TSset (mm/s) | Iactual (A) | WFSactual (m/min) | DR (kg/h) |
|---|---|---|---|---|---|
| 130 | 2.6 | 4.50 | 125 | 3.6 | 1.72 |
| 155 | 3.6 | 6.25 | 155 | 4.4 | 2.12 |
| 180 | 5.0 | 8.00 | 181 | 5.5 | 2.67 |
| Im (A) | Lower Limit of TSset (mm/s) | Upper Limit of TSset (mm/s) |
|---|---|---|
| 125 | 2.0 | 8.5 |
| 155 | 3.5 | 10.0 |
| 181 | 5.0 | 13.5 |
| Experiment | Factors | Resultant Im (A) | |
|---|---|---|---|
| TSset (mm/s) | WFSset (m/min) | ||
| 1 | 2.20 | 2.6 | 125 |
| 2 | 5.20 | 2.6 | 125 |
| 3 | 7.00 | 2.6 | 125 |
| 4 | 8.25 | 2.6 | 125 |
| 5 | 3.70 | 3.6 | 155 |
| 6 | 5.20 | 3.6 | 155 |
| 7 | 7.00 | 3.6 | 155 |
| 8 | 9.75 | 3.6 | 155 |
| 9 | 5.20 | 5.0 | 181 |
| 10 | 7.00 | 5.0 | 181 |
| 11 | 9.75 | 5.0 | 181 |
| 12 | 11.50 | 5.0 | 181 |
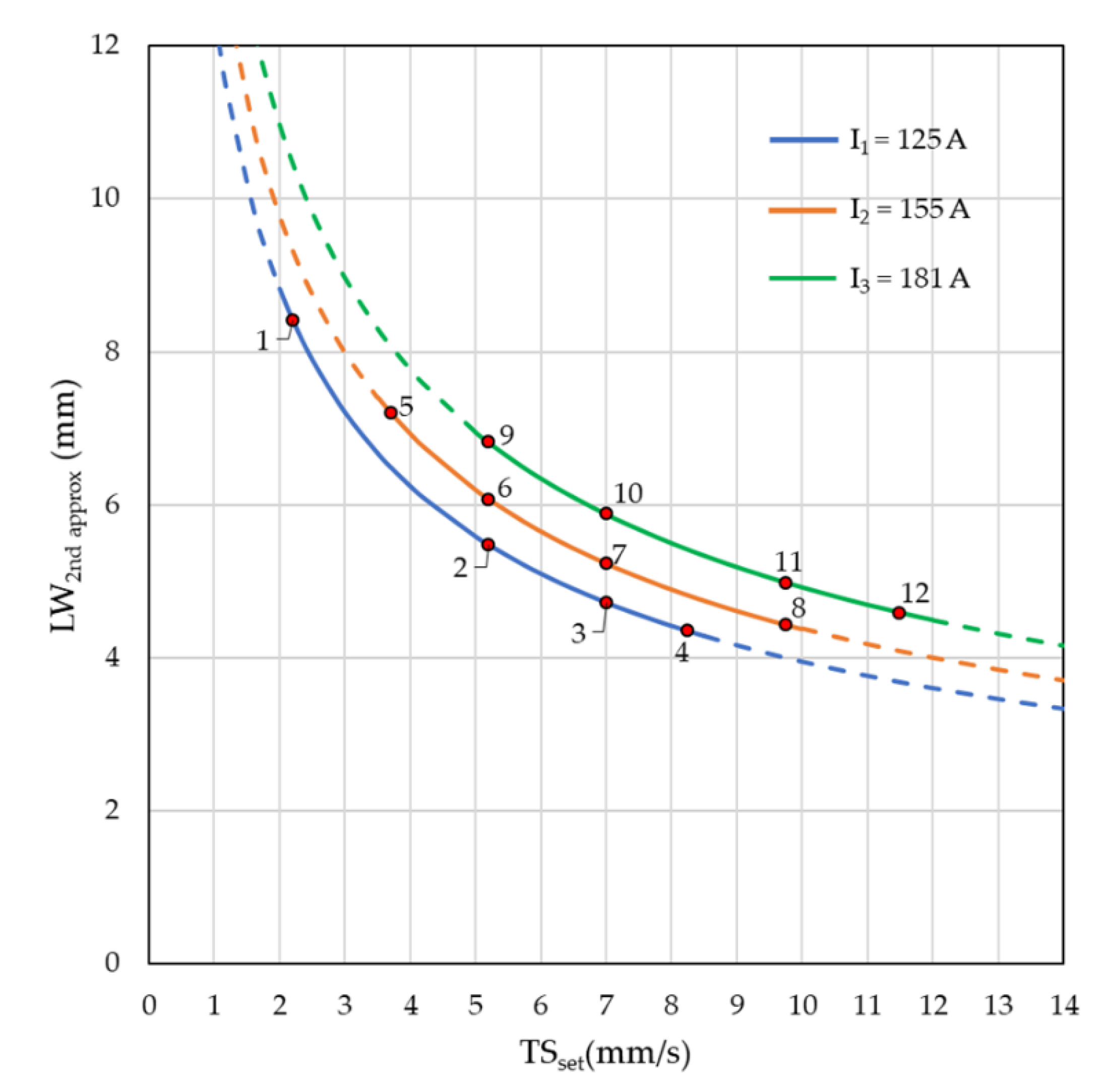
| Experiment Number | Experiments | LW (mm) | Substrate Thickness (mm) | Number of Intermediate Layers | |
|---|---|---|---|---|---|
| TS (mm/s) | I (A) | ||||
| 1 | 2.20 | 125 | 8.42 | 9 | 1 |
| 2 | 5.20 | 125 | 5.47 | 9 | 4 |
| 3 | 7.00 | 125 | 4.72 | 9 | 5 |
| 4 | 8.25 | 125 | 4.35 | 9 | 5 |
| 5 | 3.70 | 155 | 7.20 | 9 | 2 |
| 6 | 5.20 | 155 | 6.08 | 9 | 3 |
| 7 | 7.00 | 155 | 5.24 | 9 | 4 |
| 8 | 9.75 | 155 | 4.44 | 9 | 5 |
| 9 | 5.20 | 181 | 6.82 | 9 | 3 |
| 10 | 7.00 | 181 | 5.88 | 9 | 4 |
| 11 | 9.75 | 181 | 4.98 | 9 | 4 |
| 12 | 11.50 | 181 | 4.59 | 9 | 5 |
| Exp. | Factors | Response | ||
|---|---|---|---|---|
| TSset (mm/s) | Im (A) | LWex (mm) | LWef (mm) | |
| 1 | 2.20 | 125 | 8.6 | 5.5 |
| 2 | 5.20 | 125 | 6.0 | 3.6 |
| 3 | 7.00 | 125 | 5.4 | 2.9 |
| 4 | 8.25 | 125 | 5.2 | 2.6 |
| 5 | 3.70 | 155 | 9.2 | 6.3 |
| 6 | 5.20 | 155 | 7.6 | 5.2 |
| 7 | 7.00 | 155 | 6.1 | 4.1 |
| 8 | 9.75 | 155 | 5.8 | 3.6 |
| 9 | 5.20 | 181 | 10.1 | 7.3 |
| 10 | 7.00 | 181 | 8.2 | 6.5 |
| 11 | 9.75 | 181 | 7.1 | 4.9 |
| 12 | 11.50 | 181 | 6.8 | 3.6 |
| Experiment | Parameter Combinations | Target Values | ||||
|---|---|---|---|---|---|---|
| Im (A) | WFSset (m/min) | TSset (mm/s) | LWex (mm) | LWef (mm) | SWindex (mm) | |
| Low | 133 | 2.7 | 5.10 | 6.5 | 3.8 | 0.60 |
| Medium | 168 | 4.4 | 8.35 | 6.5 | 4.5 | 0.60 |
| High | 177 | 4.9 | 11.30 | 6.5 | 3.5 | 0.60 |
| Exp. | Target Values | Actual Values | Deviation (Target–Actual) | ||||||
|---|---|---|---|---|---|---|---|---|---|
| LWex | LWef | SWindex | LWex | LWef | SWindex | LWex | LWef | SWindex | |
| Low | 6.5 | 3.8 | 0.60 | 6.4 | 3.9 | 0.64 | 0.1 | −0.1 | −0.04 |
| Medium | 6.5 | 4.5 | 0.60 | 6.7 | 4.9 | 0.51 | −0.2 | −0.4 | 0.09 |
| High | 6.5 | 3.5 | 0.60 | 6.3 | 4.6 | 0.60 | 0.2 | −1.1 | 0.00 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dahat, S.; Hurtig, K.; Andersson, J.; Scotti, A. A Methodology to Parameterize Wire + Arc Additive Manufacturing: A Case Study for Wall Quality Analysis. J. Manuf. Mater. Process. 2020, 4, 14. https://doi.org/10.3390/jmmp4010014
Dahat S, Hurtig K, Andersson J, Scotti A. A Methodology to Parameterize Wire + Arc Additive Manufacturing: A Case Study for Wall Quality Analysis. Journal of Manufacturing and Materials Processing. 2020; 4(1):14. https://doi.org/10.3390/jmmp4010014
Chicago/Turabian StyleDahat, Shubham, Kjell Hurtig, Joel Andersson, and Americo Scotti. 2020. "A Methodology to Parameterize Wire + Arc Additive Manufacturing: A Case Study for Wall Quality Analysis" Journal of Manufacturing and Materials Processing 4, no. 1: 14. https://doi.org/10.3390/jmmp4010014
APA StyleDahat, S., Hurtig, K., Andersson, J., & Scotti, A. (2020). A Methodology to Parameterize Wire + Arc Additive Manufacturing: A Case Study for Wall Quality Analysis. Journal of Manufacturing and Materials Processing, 4(1), 14. https://doi.org/10.3390/jmmp4010014






