Meso-Scale Finite Element Model for Rib-Stiffened Composites with Biaxial Weft-Knitted Reinforcements
Abstract
1. Introduction
1.1. Motivation
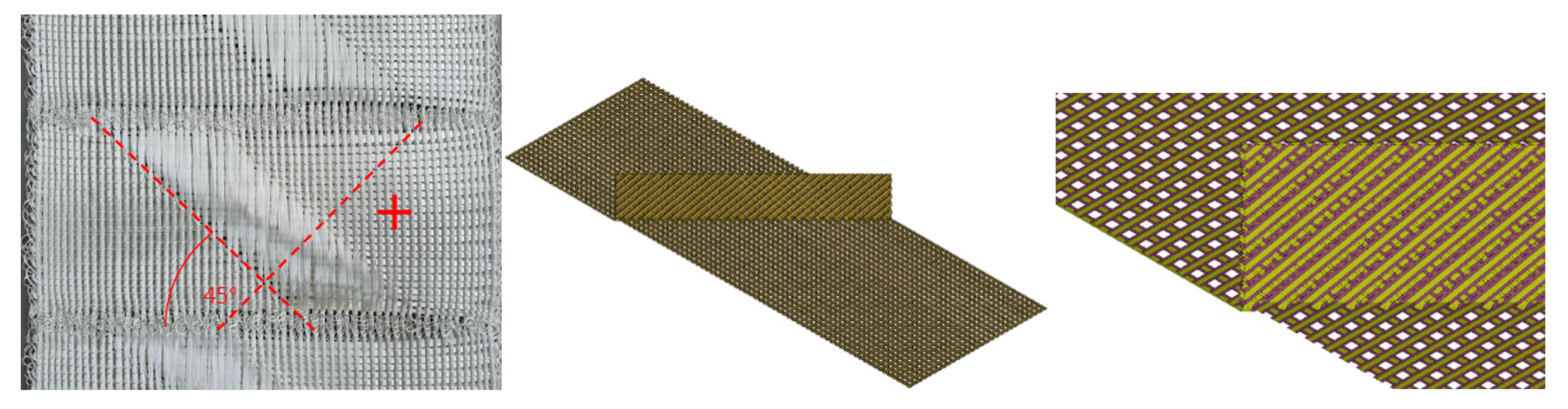
1.2. Production Technique for Biaxial Weft-Knitted Fabrics
1.3. Modeling of Textiles and Textile-Reinforced Composites
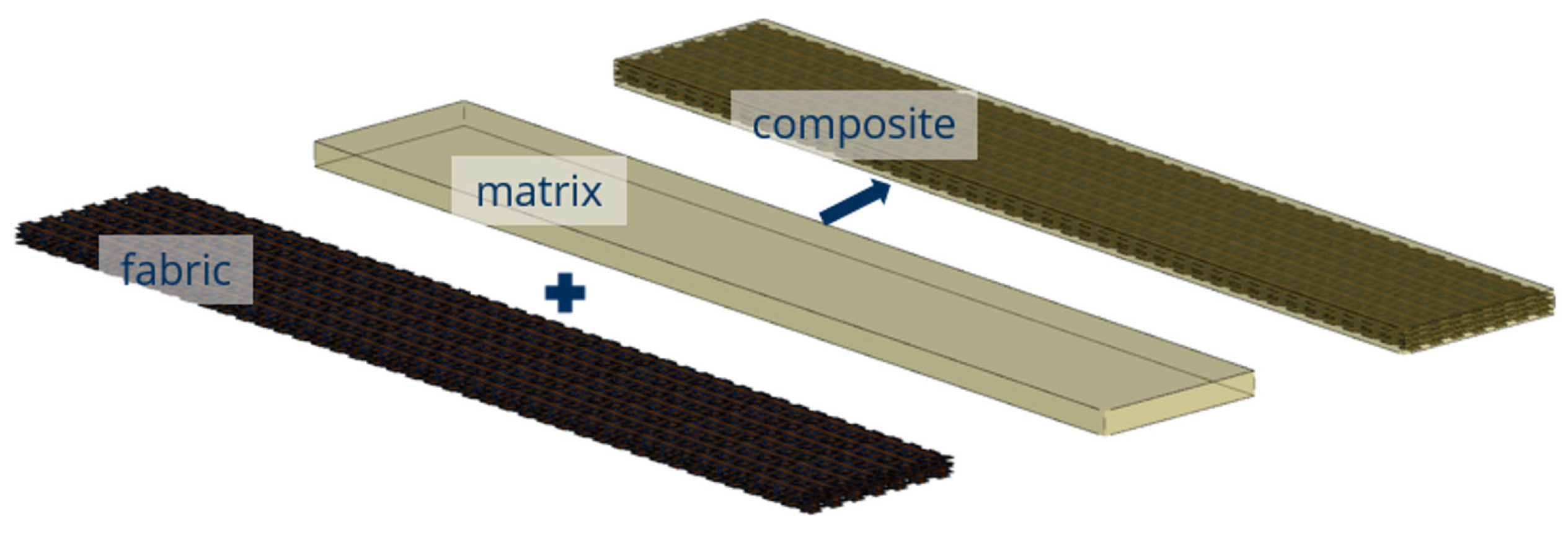
2. Materials and Methods
2.1. Materials
2.2. Manufacturing of Fabrics and Composite
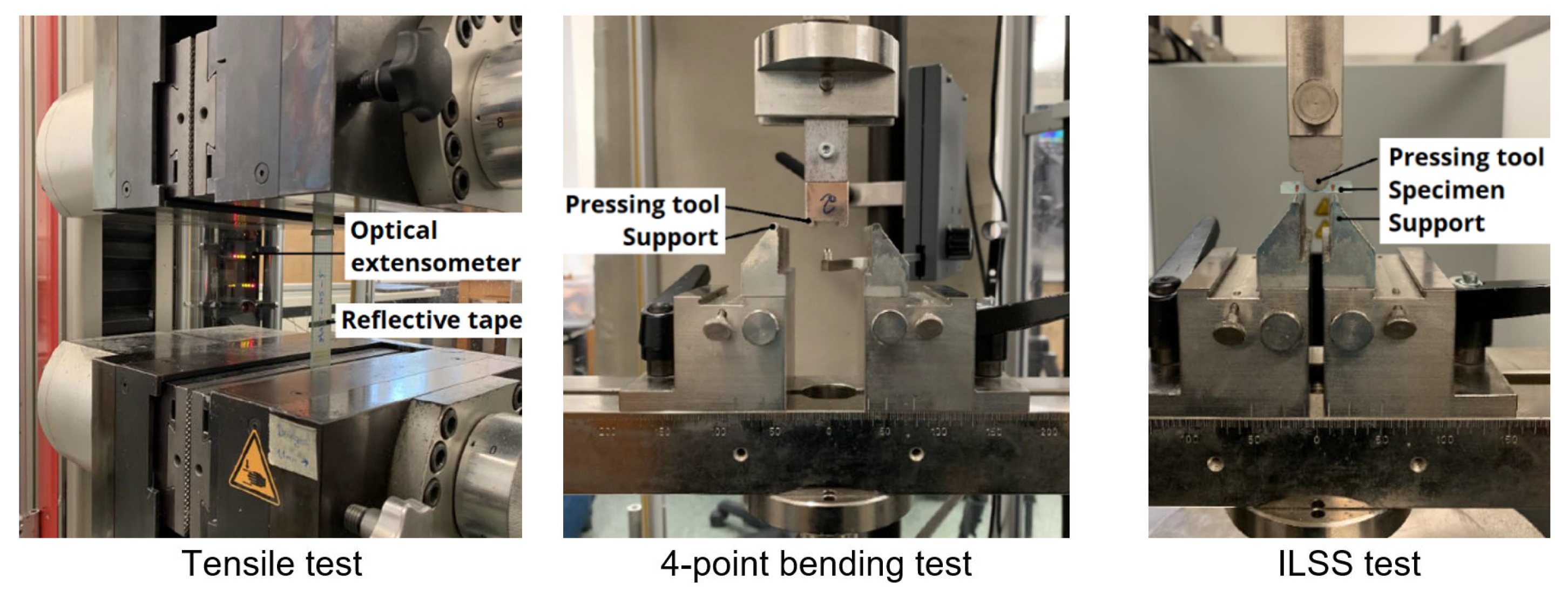
2.3. Testing Methods
2.4. Modelling Methods
3. Results
3.1. Experimental Results
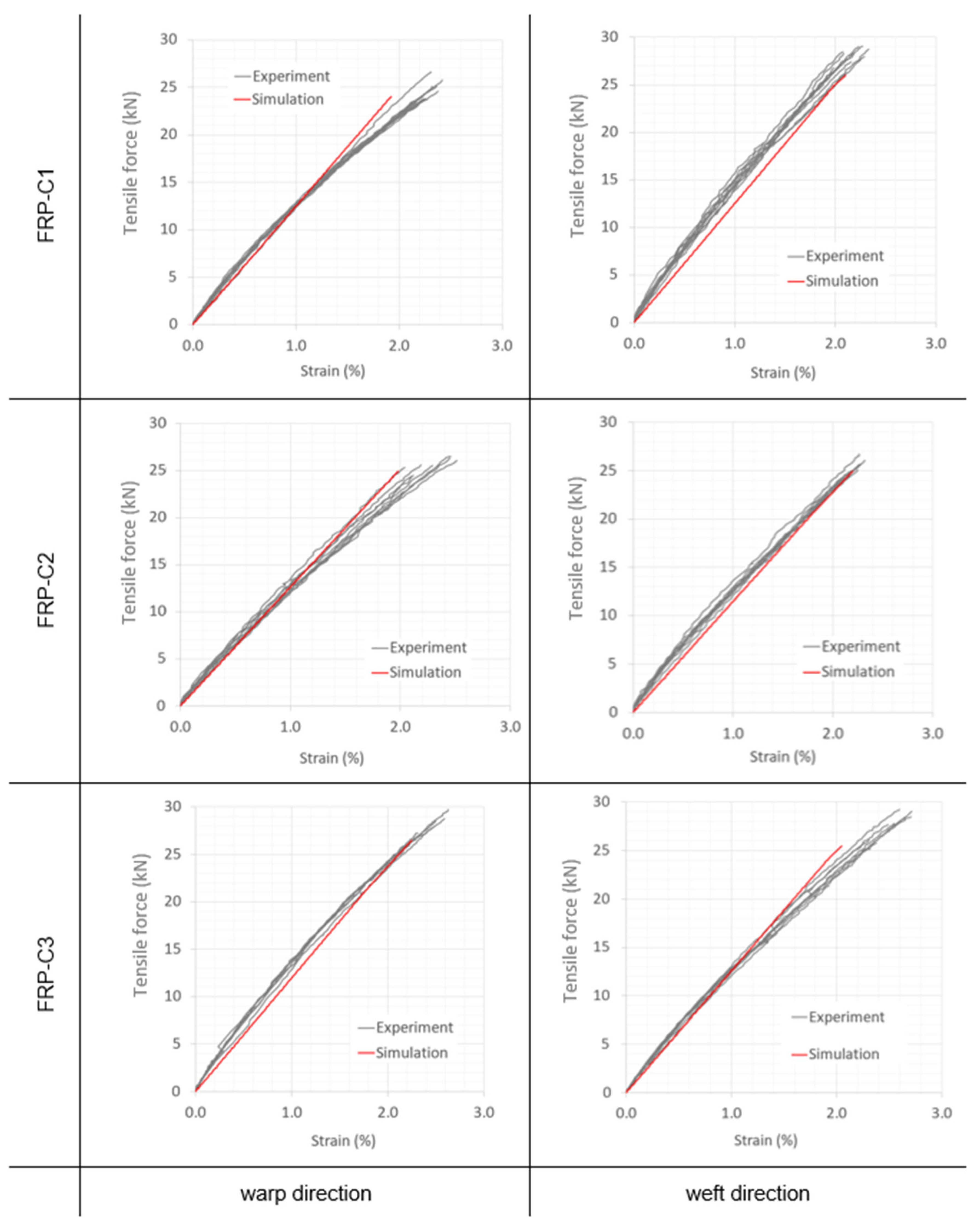
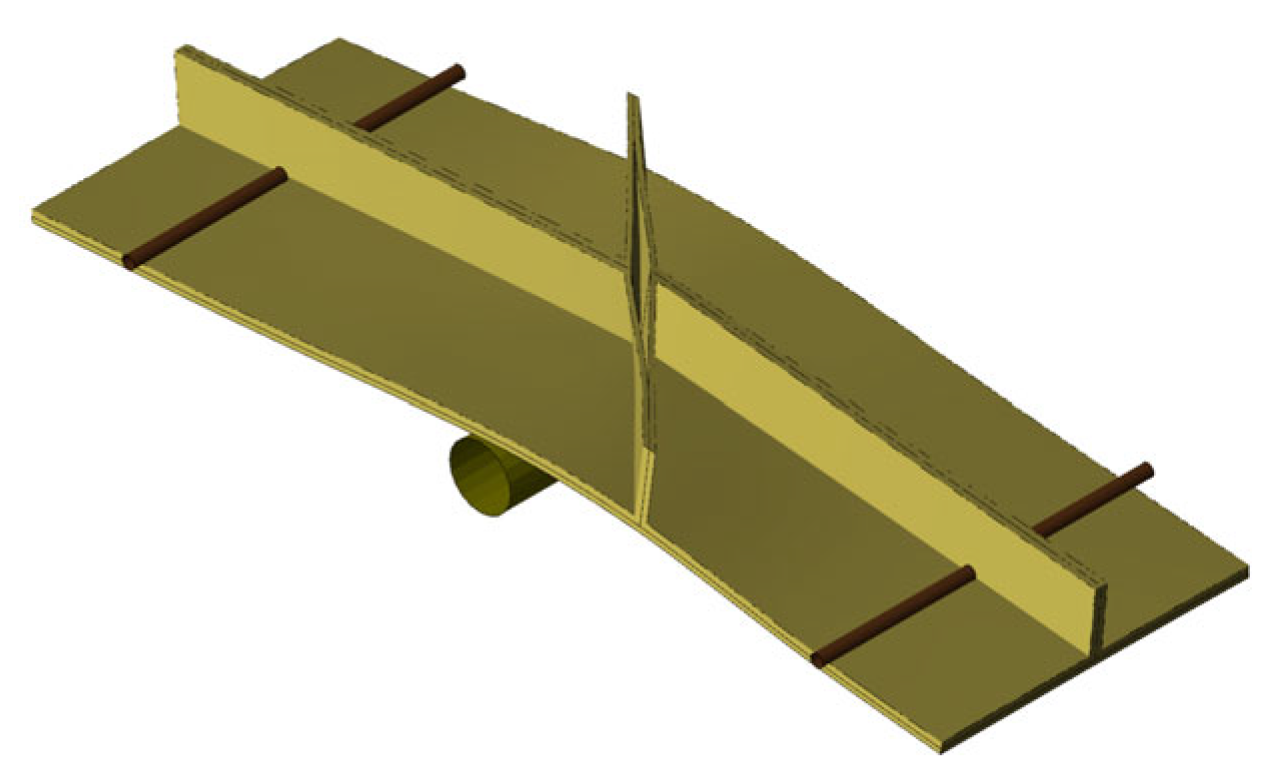
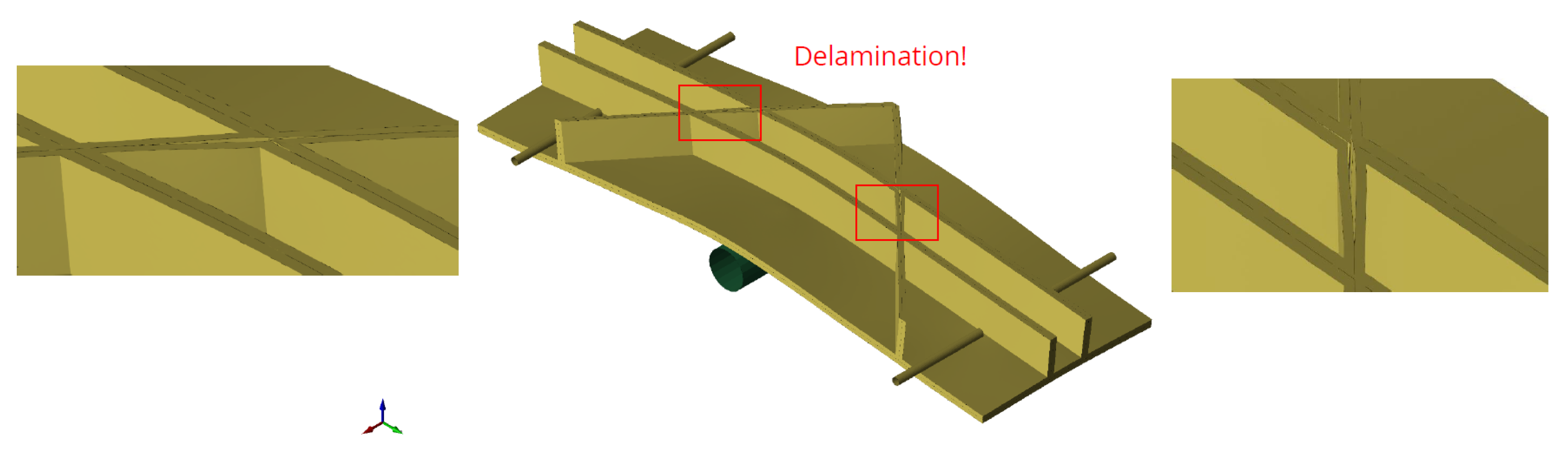
3.2. Modelling Results

- The failure mechanism debonding has not been taken into account.
- Failure of the fiber–matrix interface due to compression is neglected.
- Mode II in-plane fracture toughness in warp and weft direction share the same value.
- Mode III out-of-plane fracture is not included in the model.
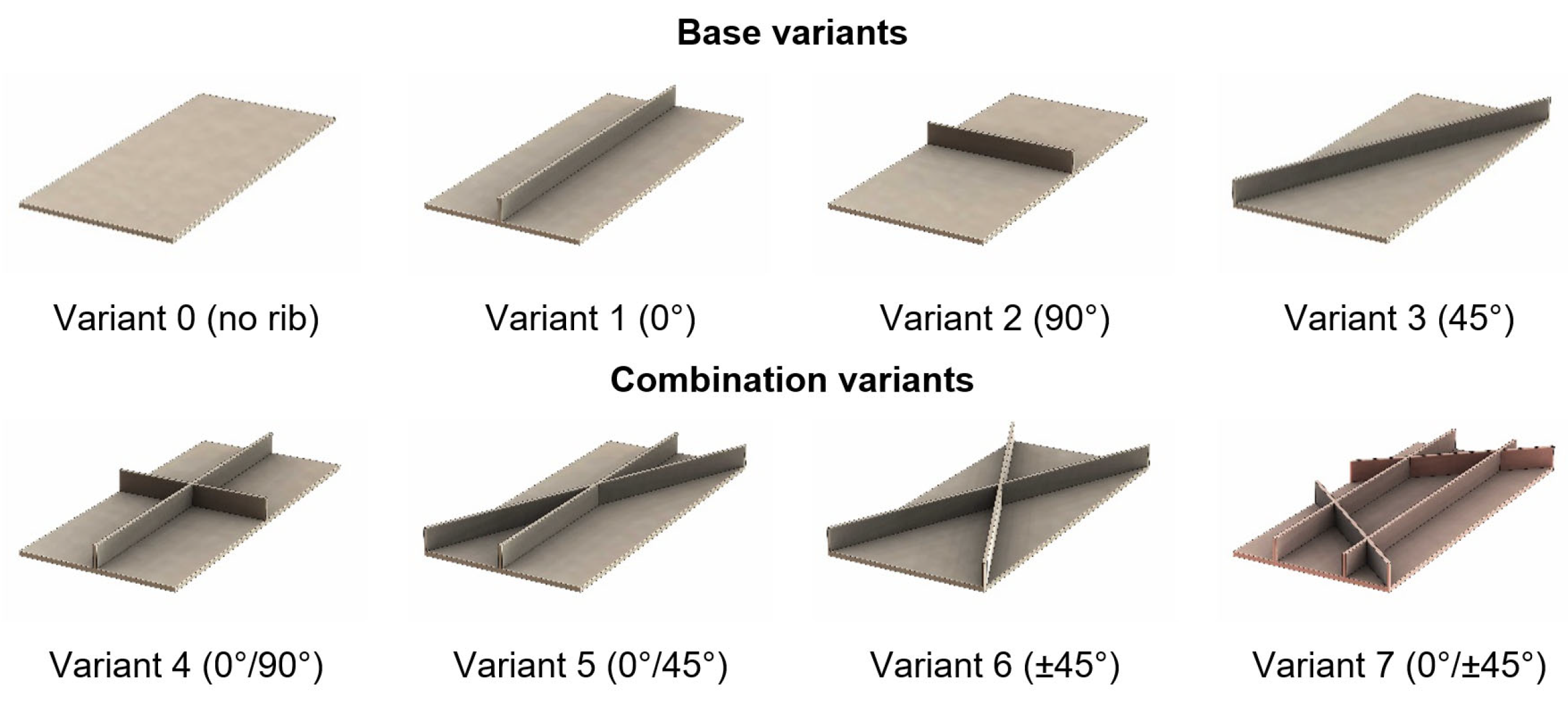
3.3. Application of the Models to the Analysis of Further Variants
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Palmer, R. Techno-Economic Requirements for Composite Aircraft Components: Innovative Composite Aircraft Primary Structure. In Proceedings of the FIBER-TEX 1992: The Sixth Conference on Advanced Engineering Fibers and Textile Structures for Composites, Philadelphia, PA, USA, 27–29 October 1992. [Google Scholar]
- Crosky, A.; Grant, C.; Kelly, D.; Legrand, X.; Pearce, G. 4—Fibre placement processes for composites manufacture. In Advances in Composites Manufacturing and Process Design; Boisse, P., Ed.; Woodhead Publishing: Sawston, UK, 2015; pp. 79–92. ISBN 978-1-78242-307-2. [Google Scholar]
- Hasani, H.; Hassanzadeh, S.; Abghary, M.J.; Omrani, E. Biaxial weft-knitted fabrics as composite reinforcements: A review. J. Ind. Text. 2017, 46, 1439–1473. [Google Scholar] [CrossRef]
- Le Phuc, B. Konstruktive und Technologische Weiterentwicklung der Flachstricktechnik zur Herstellung von Multilayer-Gestricken mit bis zu neun Verstärkungslagen; TUD Press: Dresden, Germany, 2006; ISBN 3-938863-41-2. [Google Scholar]
- Abounaim, M.S.M. Process Development for the Manufacturing of Flat Knitted Innovative 3D Spacer Fabrics for High Performance Composite Applications. Ph.D. Thesis, Technische Universität Dresden, Dresden, Germany, 2010. [Google Scholar]
- Bollengier, Q.; Wieczorek, F.; Hellmann, S.; Trümper, W.; Cherif, C. One-step manufacturing of innovative flat-knitted 3D net-shape preforms for composite applications. IOP Conf. Ser. Mat. Sci. Eng. 2017, 254, 42007. [Google Scholar] [CrossRef]
- Bollengier, Q.; Trümper, W.; Cherif, C. Integral gefertigte 3D-Schale-Rippen-Strukturen auf Basis von Mehrlagengestricken. Tech. Text. 2020, 62, 113–115. [Google Scholar]
- Różyło, P.; Wysmulski, P.; Falkowicz, K. Fem and Experimental Analysis of Thin-Walled Composite Elements Under Compression. Int. J. Appl. Mech. Eng. 2017, 22, 393–402. [Google Scholar] [CrossRef]
- Wysmulski, P.; Debski, H.; Falkowicz, K.; Rozylo, P. The influence of load eccentricity on the behavior of thin-walled compressed composite structures. Compos. Struct. 2019, 213, 98–107. [Google Scholar] [CrossRef]
- Khandan, R.; Noroozi, S.; Sewell, P.; Vinney, J. The development of laminated composite plate theories: A review. J. Mater. Sci. 2012, 47, 5901–5910. [Google Scholar] [CrossRef]
- Hinton, M.; Soden, P.D.; Kaddour, A.-S. Failure Criteria in Fibre Reinforced Polymer Composites: The World-Wide Failure Exercise; Elsevier Ltd.: Kidlington, UK, 2004. [Google Scholar]
- Camanho, P.P.; Hallett, S.R. Numerical Modelling of Failure in Advanced Composite Materials; Woodhead Publishing: Sawston, UK, 2015; ISBN 9780081003428. [Google Scholar]
- Hübner, M.; Staiger, E.; Küchler, K.; Gereke, T.; Cherif, C. Simulation of patched woven fabric composite structures under tensile load. Tekstilec 2016, 59, 175–181. [Google Scholar] [CrossRef]
- Stig, F.; Hallström, S. A modelling framework for composites containing 3D reinforcement. Compos. Struct. 2012, 94, 2895–2901. [Google Scholar] [CrossRef]
- Lomov, S.; Ivanov, D.; Verpoest, I.; Zako, M.; Kurashiki, T.; Nakai, H.; Hirosawa, S. Meso-FE modelling of textile composites: Road map, data flow and algorithms. Compos. Sci. Technol. 2007, 67, 1870–1891. [Google Scholar] [CrossRef]
- Stig, F.; Hallström, S. Spatial modelling of 3D-woven textiles. Compos. Struct. 2012, 94, 1495–1502. [Google Scholar] [CrossRef]
- Wang, Y.; Soutis, C. A Finite Element and Experimental Analysis of Composite T-Joints Used in Wind Turbine Blades. Appl. Compos. Mater. 2018, 25, 953–964. [Google Scholar] [CrossRef]
- Le Page, B.H.; Guild, F.J.; Ogin, S.L.; Smith, P.A. Finite element simulation of woven fabric composites. Compos. Part A Appl. Sci. Manuf. 2004, 35, 861–872. [Google Scholar] [CrossRef]
- LLorca, J.; González, C.; Molina-Aldareguía, J.M.; Segurado, J.; Seltzer, R.; Sket, F.; Rodríguez, M.; Sádaba, S.; Muñoz, R.; Canal, L.P. Multiscale modeling of composite materials: A roadmap towards virtual testing. Adv. Mater. 2011, 23, 5130–5147. [Google Scholar] [CrossRef]
- Barbero, E.J.; Lonetti, P.; Sikkil, K.K. Finite element continuum damage modeling of plain weave reinforced composites. Compos. Part B 2005, 37, 137–147. [Google Scholar] [CrossRef]
- Daggumati, S.; van Paepegem, W.; Degrieck, J.; Xu, J.; Lomov, S.V.; Verpoest, I. Local damage in a 5-harness satin weave composite under static tension: Part II—Meso-FE modelling. Compos. Sci. Technol. 2010, 70, 1934–1941. [Google Scholar] [CrossRef]
- Cox, B.N.; Carter, W.C.; Fleck, N.A. A binary model of textile composites—I. Formulation. Acta Metall. Mater. 1994, 42, 3463–3479. [Google Scholar] [CrossRef]
- Li, D.; Fang, D.; Jiang, N.; Xuefeng, Y. Finite element modeling of mechanical properties of 3D five-directional rectangular braided composites. Compos. Part B 2011, 42, 1373–1385. [Google Scholar] [CrossRef]
- Zhang, C.; Curiel-Sosa, J.L.; Bui, T.Q. Meso-scale finite element analysis of mechanical behavior of 3D braided composites subjected to biaxial tension loadings. Appl. Compos. Mater. 2019, 26, 139–157. [Google Scholar] [CrossRef]
- Pickett, A.K.; Sirtautas, J.; Erber, A. Braiding Simulation and Prediction of Mechanical Properties. Appl. Compos. Mater. 2009, 16, 345–364. [Google Scholar] [CrossRef]
- Liu, Z.; Hou, Y.; Zhao, Q.; Li, C. A novel surrogate modeling strategy of the mechanical properties of 3D braided composites. Chin. J. Aeronaut. 2020, 33, 2589–2601. [Google Scholar] [CrossRef]
- Gholami, A.; Melenka, G.W. Finite element analysis of 2-D tubular braided composite based on geometrical models to study mechanical performances. Mech. Adv. Mater. Struct. 2021, 29, 7542–7558. [Google Scholar] [CrossRef]
- Gu, B. A microstructure model for finite-element simulation of 3D rectangular braided composite under ballistic penetration. Philos. Mag. 2007, 87, 4643–4669. [Google Scholar] [CrossRef]
- Dhimole, V.K.; Chen, Y.; Cho, C. Modeling and Two-Step Homogenization of Aperiodic Heterogenous 3D Four-Directional Braided Composites. J. Compos. Sci. 2020, 4, 179. [Google Scholar] [CrossRef]
- Li, X.; Binienda, W.K.; Goldberg, R.K. Finite-Element Model for Failure Study of Two-Dimensional Triaxially Braided Composite. J. Aerosp. Eng. 2011, 24, 170–180. [Google Scholar] [CrossRef]
- Zheng, H.; Zhou, C.; Yuan, Y. Meso-scale finite element modeling of moisture diffusion in 3D braided composite. Int. J. Heat Mass Transf. 2019, 129, 862–872. [Google Scholar] [CrossRef]
- Xu, K.; Qian, X.; Huang, R. An FEM Analysis with Consideration of Random Void Defects for Predicting the Mechanical Properties of 3D Braided Composites. Adv. Mater. Sci. Eng. 2014, 2014, 439819. [Google Scholar] [CrossRef]
- Yamamoto, T.; Imaoku, A.; Sakakibara, T.; Zako, M. A proposal of conventional FE-modeling for layered braided composites: Comparison of numerical results with experimental results. IOP Conf. Ser. Mater. Sci. Eng. 2018, 406, 12031. [Google Scholar] [CrossRef]
- Song, S.; Waas, A.M.; Shahwan, K.W.; Faruque, O.; Xiao, X. Compression Response of 2D Braided Textile Composites: Single Cell and Multiple Cell Micromechanics Based Strength Predictions. J. Compos. Mater. 2008, 42, 2461–2482. [Google Scholar] [CrossRef]
- Fang, G.; Wang, B.; Liang, J. A coupled FE-FFT multiscale method for progressive damage analysis of 3D braided composite beam under bending load. Compos. Sci. Technol. 2019, 181, 107691. [Google Scholar] [CrossRef]
- Creech, G.; Pickett, A.K. Meso-modelling of Non-Crimp Fabric composites for coupled drape and failure analysis. J. Mater. Sci. 2006, 41, 6725–6736. [Google Scholar] [CrossRef]
- Sirtautas, J.; Pickett, A.K.; Lépicier, P. A mesoscopic model for coupled drape-infusion simulation of biaxial Non-Crimp Fabric. Compos. Part B Eng. 2013, 47, 48–57. [Google Scholar] [CrossRef]
- Drapier, S.; Wisnom, M.R. Finite-element investigation of the compressive strength of non-crimp-fabric-based composites. Compos. Sci. Technol. 1999, 59, 1287–1297. [Google Scholar] [CrossRef]
- Yin, H.; Li, Q.; Iannucci, L. Meso-scale finite element (FE) modelling of biaxial carbon fibre non-crimp-fabric (NCF) based composites under uniaxial tension and in-plane shear. Compos. Struct. 2022, 290, 115538. [Google Scholar] [CrossRef]
- Carvelli, V.; Chi, T.T.; Larosa, M.S.; Lomov, S.V.; Poggi, C.; Angulo, D.R.; Verpoest, I. Experimental and numerical determination of the mechanical properties of multi-axial multi-ply composites. In Proceedings of the 11th European Conference on Composite Materials—ECCM 11, Rhodes, Greece, 31 May—3 June 2004. [Google Scholar]
- Ferreira, L.M.; Graciani, E.; París, F. Predicting failure load of a non-crimp fabric composite by means of a 3D finite element model including progressive damage. Compos. Struct. 2019, 225, 111115. [Google Scholar] [CrossRef]
- González, A.; Graciani, E.; París, F. Prediction of in-plane stiffness properties of non-crimp fabric laminates by means of 3D finite element analysis. Compos. Sci. Technol. 2008, 68, 121–131. [Google Scholar] [CrossRef]
- Mikhaluk, D.S.; Truong, T.C.; Borovkov, A.I.; Lomov, S.V.; Verpoest, I. Experimental observations and finite element modelling of damage initiation and evolution in carbon/epoxy non-crimp fabric composites. Eng. Fract. Mech. 2008, 75, 2751–2766. [Google Scholar] [CrossRef]
- Ravandi, M.; Ahlquist, S.; Banu, M. Numerical modelling of mechanical behaviour of weft-knitted carbon fiber composites. In Proceedings of the 8th European Conference for Aeronautics and Space Sciences (EUCASS), Madrid, Spain, 1–4 July 2019. [Google Scholar]
- Ionesi, S.D.; Ciobanu, L.; Dumitras, C.; Avadanei, M.; Dulgheriu, I.; Ionescu, I.; Loghin, M.C. FEM Analysis of Textile Reinforced Composite Materials Impact Behavior. Materials 2021, 14, 7380. [Google Scholar] [CrossRef]
- Hamedi, S.; Hasani, H.; Dibajian, S.H. Numerical simulating the flexural properties of 3D weft-knitted spacer fabric reinforced composites. J. Compos. Mater. 2017, 51, 1887–1899. [Google Scholar] [CrossRef]
- Haasemann, G.; Ulbricht, V.; Brummund, J. Modelling the mechanical properties of biaxial weft-knitted fabric reinforced. Proc. Appl. Math. Mech. 2004, 4, 193–194. [Google Scholar] [CrossRef]
- Pindera, M.-J.; Khatam, H.; Drago, A.S.; Bansal, Y. Micromechanics of spatially uniform heterogeneous media: A critical review and emerging approaches. Compos. Part B Eng. 2009, 40, 349–378. [Google Scholar] [CrossRef]
- Döbrich, O.; Gereke, T.; Cherif, C. Modelling of fibre-reinforced composites on a near micro scale: Possibilities and benefits. In Proceedings of the 10th European LS-DYNA Conference, Würzburg, Germany, 15–17 June 2015. [Google Scholar]
- Liu, C.; Xie, J.; Sun, Y.; Chen, L. Micro-scale modeling of textile composites based on the virtual fiber embedded models. Compos. Struct. 2019, 230, 111552. [Google Scholar] [CrossRef]
- Raju, K.; Tay, T.-E.; Tan, V.B.C. A review of the FE2 method for composites. Multiscale Multidiscip. Model. Exp. Des. 2021, 4, 1–24. [Google Scholar] [CrossRef]
- De Araújo, M.; Fangueiro, R.; Hong, H. Modelling and simulation of the mechanical behaviour of weft-knitted fabrics for technical applications. Part IV: 3D FEA model with a mesh of tetrahedric elements. Autex Res. J. 2004, 4, 72–80. [Google Scholar]
- De Araújo, M.; Fangueiro, R.; Hong, H. Modelling and simulation of the mechanical behaviour of weft-knitted fabrics for technical applications: Part II: 3D model based on the elastica theory. Autex Res. J. 2003, 3, 166–172. [Google Scholar]
- De Araújo, M.; Fangueiro, R.; Hong, H. Modelling and simulation of the mechanical behaviour of weft-knitted fabrics for technical applications: Part III: 2D hexagonal FEA model with non-linear truss elements. Autex Res. J. 2004, 4, 25–32. [Google Scholar]
- Duhovic, M.; Bhattacharyya, D. Simulating the deformation mechanisms of knitted fabric composites. Compos. Part A Appl. Sci. Manuf. 2006, 37, 1897–1915. [Google Scholar] [CrossRef]
- Döbrich, O.; Gereke, T.; Diestel, O.; Krzywinski, S.; Cherif, C. Decoupling the bending behavior and the membrane properties of finite shell elements for a correct description of the mechanical behavior of textiles with a laminate formulation. J. Ind. Text. 2013, 44, 70–84. [Google Scholar] [CrossRef]
- Pham, M.Q.; Döbrich, O.; Trümper, W.; Gereke, T.; Cherif, C. Numerical modelling of the mechanical behaviour of biaxial weft-knitted fabrics on different length scales. Materials 2019, 12, 3693. [Google Scholar] [CrossRef]
- Pham, M.Q.; Wendt, E.; Häntzsche, E.; Gereke, T.; Cherif, C. Numerical modeling of the mechanical behavior of textile structures on the meso-scale for forming process simulations of composite 3D preforms. Eng. Rep. 2020, 4, e12348. [Google Scholar] [CrossRef]
- Pham, M.Q.; Döbrich, O.; Mersch, J.; Gereke, T.; Cherif, C. Meso-scale model for the forming process of biaxial reinforced weft-knitted fabrics. IOP Conf. Ser. Mat. Sci Eng. 2018, 406, 012026. [Google Scholar] [CrossRef]
- Jiang, W.-G.; Hallett, S.R.; Wisnom, M.R. Development of Domain Superposition Technique for the Modelling of Woven Fabric Composites. In Mechanical Response of Composites; Oñate, E., Camanho, P.P., Dávila, C.G., Pinho, S.T., Remmers, J.J.C., Eds.; Springer: Dordrecht, The Netherlands, 2008; pp. 281–291. ISBN 978-1-4020-8583-3. [Google Scholar]
- Livermore Software Technology Corporation. LS-DYNA Keyword User’s Manual R12.0, Volume I; Livermore Software Technology Corporation: Livermore, CA, USA, 2020. [Google Scholar]
- Bianchi, F.; Koh, T.M.; Zhang, X.; Partridge, I.K.; Mouritz, A.P. Finite element modelling of z-pinned composite T-joints. Compos. Sci. Technol. 2012, 73, 48–56. [Google Scholar] [CrossRef]
- Cartié, D.D.; Dell’Anno, G.; Poulin, E.; Partridge, I.K. 3D reinforcement of stiffener-to-skin T-joints by Z-pinning and tufting. Eng. Fract. Mech. 2006, 73, 2532–2540. [Google Scholar] [CrossRef]
- Liu, Y.; Li, M.; Lu, X.; Zhu, X. Failure Mechanism and Strength Prediction Model of T-Joint of Composite Sandwich Structure. Metals 2021, 11, 1197. [Google Scholar] [CrossRef]
- Chen, J. Simulation of multi-directional crack growth in braided composite T-piece specimens using cohesive models. Fatigue Fract. Eng. Mater. Struct. 2011, 34, 123–130. [Google Scholar] [CrossRef]
- Wang, Z.Q.; Zhou, S.; Zhou, J.S.; Sun, X.Y. Properties Analysis of Bolted Composite T-Joint. Key Eng. Mater. 2011, 467–469, 575–578. [Google Scholar] [CrossRef]













| Density (g/cm3) | Young’s Modulus (GPa) | Tensile Strength (MPa) | Compressive Strength (MPa) | Break Elongation (%) |
|---|---|---|---|---|
| 1.18–1.20 | 2.7–3.2 | 60–75 | 80–90 | 5.0–10.0 |
| Variant | Configuration | Cross-Section along Warp Direction |
|---|---|---|
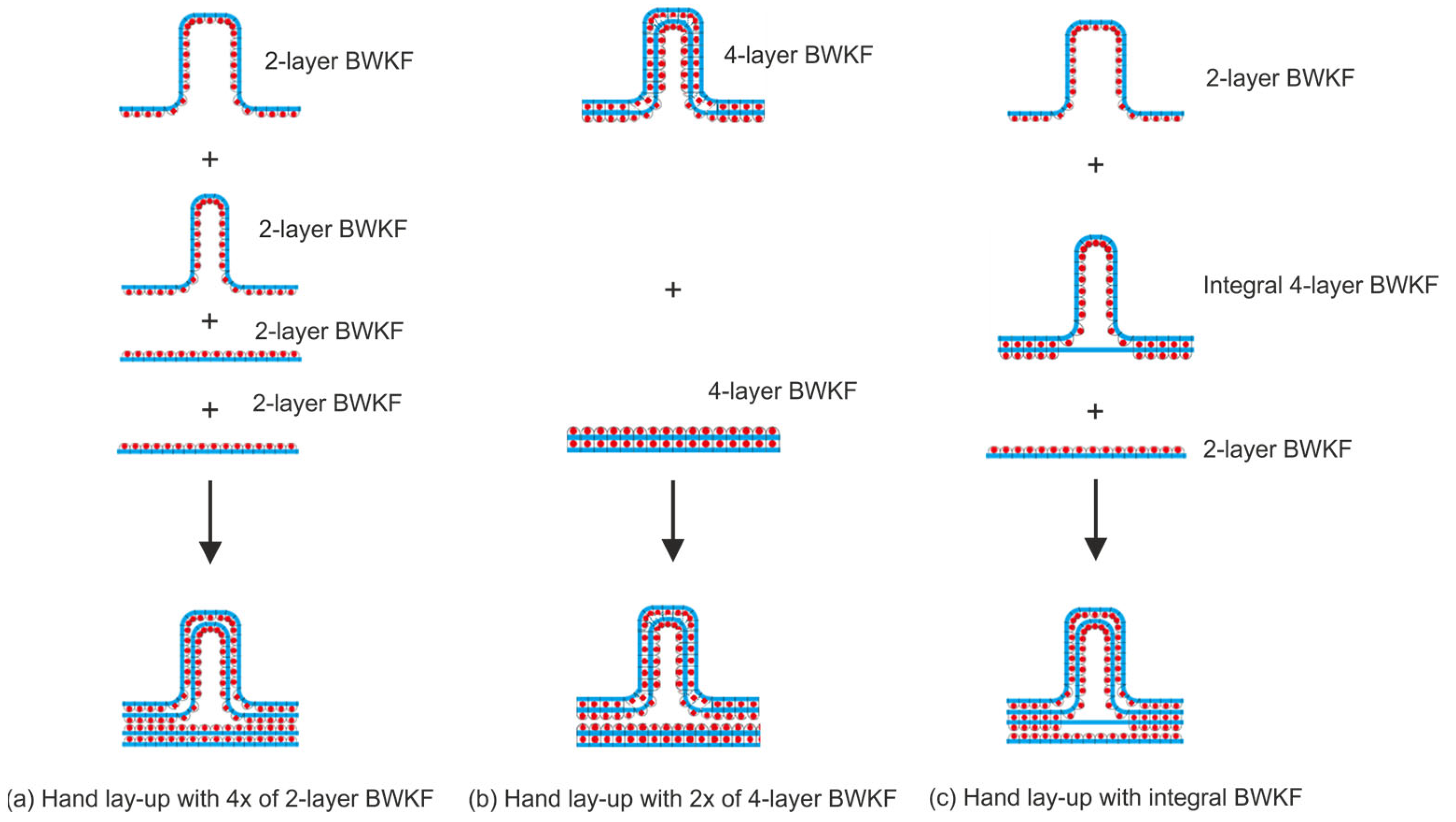
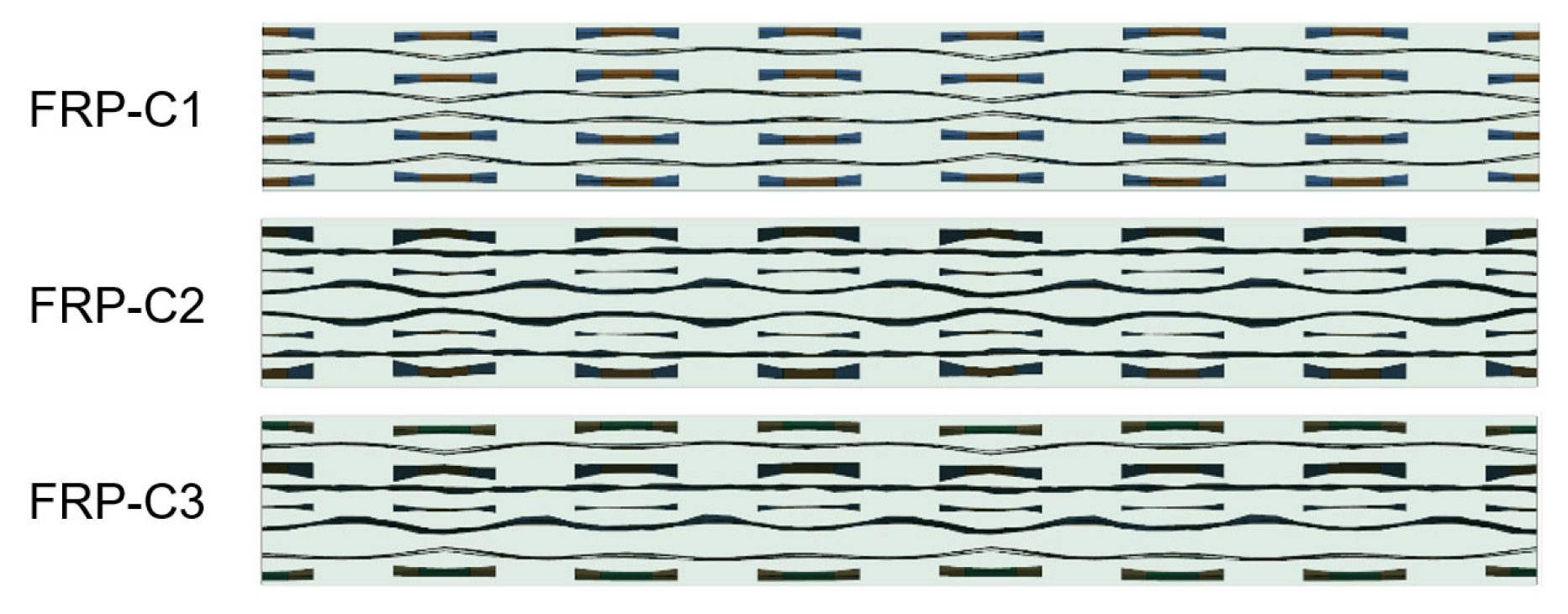
| FRP-C1 | 4 × 2-layer with the symmetric lay-up of [(0°/90°)2]s |  |
| FRP-C2 | 2 × 4-layer with the symmetric lay-up of [(0°/90°/0°/90°)]s |  |
| FRP-C3 | 2-layer/4-layer/2-layer with the lay-up of [(0°/90°)(90°/0°/90°/0°)(90°/0°)] |  |

| Variant | Length L (mm) | Support Length LS (mm) | Width W (mm) | Flange Thickness D (mm) | Rib Height H (mm) | Rib Thickness DR (mm) |
|---|---|---|---|---|---|---|
| T120-0 | 120 | 90 | 50 | 4 | 0 | 0 |
| T120-33 | 120 | 90 | 50 | 4 | 33 | 4 |
| T300-33 | 300 | 225 | 50 | 4 | 33 | 4 |
| T400-33 | 400 | 300 | 50 | 4 | 33 | 4 |
| Variant/Construction | FRP-T1 | FRP-T2 | FRP-T3 |
|---|---|---|---|
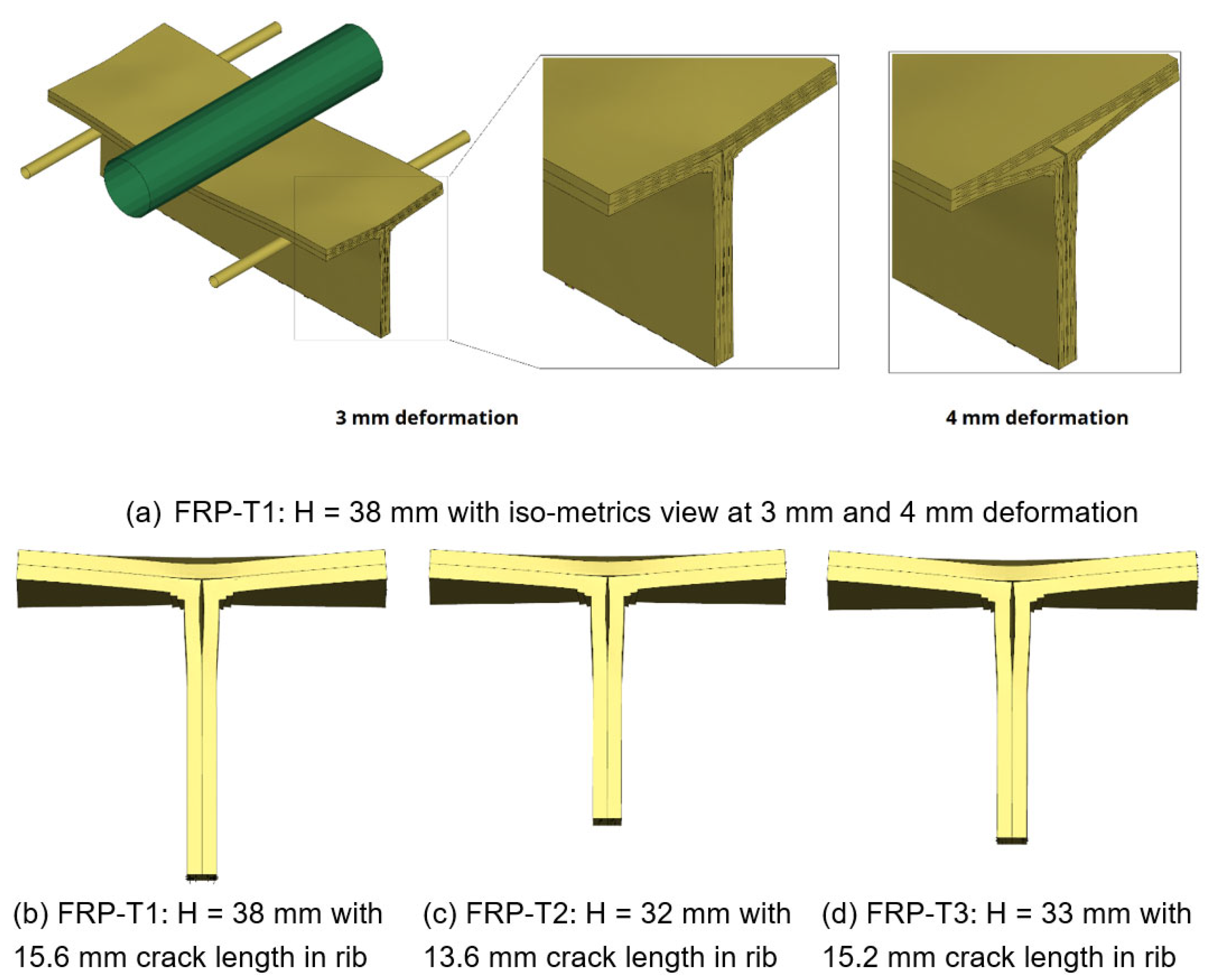
| T120-0 | 3 (0) | 0 | 3 (0) |
| T120-33 | 6 (38.4) | 6 (31.6) | 6 (32.9) |
| T300-33 | 2 (32.1) | 0 | 0 |
| T400-33 | 3 (32.1) | 0 | 0 |
| BWKF | Thickness (mm) | Area Mass Density (g/m2) | Loop Length (mm) | Tensile Strength (N) | Elongation at Break (%) | ||
|---|---|---|---|---|---|---|---|
| Warp | Weft | Warp | Weft | ||||
| 2-layer | 1.6 (0.03) | 1027.8 (33.5) | 13.6 (0.6) | 6744 (573) | 4649 (418) | 1.8 (0.2) | 1.9 (0.2) |
| 4-layer | 2.4 (0.04) | 1721.8 (44.4) | 16.8 (0.5) | 13,247 (575) | 13,556 (856) | 1.6 (0.1) | 1.6 (0.4) |
| Variant | Young’s Modulus (GPa) | Tensile Strength (MPa) | Elongation at Break (%) | Bending Stiffness (MPa) | ILSS (MPa) | |||||
|---|---|---|---|---|---|---|---|---|---|---|
| Warp | Weft | Warp | Weft | Warp | Weft | Warp | Weft | Warp | Weft | |
| FRP-C1 | 17.2 (2.3) | 19.8 (2.4) | 297 (17) | 352 (11) | 2.3 (0.1) | 2.2 (0.1) | 278 (16) | 173 (20) | 31.5 (1.3) | 31.5 (1.6) |
| FRP-C2 | 17.8 (1.8) | 17.9 (1.4) | 322 (13) | 324 (17) | 2.3 (0.2) | 2.2 (0.1) | 385 (26) | 390 (15) | 32.5 (3.5) | 30.9 (1.3) |
| FRP-C3 | 17.6 (1.2) | 16.9 (1.4) | 347 (14) | 340 (13) | 2.3 (0.4) | 2.5 (0.3) | 356 (28) | 277 (21) | 48.1 (11.9) | 31.8 (0.7) |
| Variant | Construction | Max. F (N) | Standardized Max. F (N) | Deformation at Max. F (mm) |
|---|---|---|---|---|
| T120-0 | FRP-T1 | 976 (53) | - | 10.0 (1.6) |
| FRP-T3 | 835 (49) | - | 11.0 (4.2) | |
| T120-33 | FRP-T1 | 11,540 (575) | 9917 (494) | 2.9 (0.2) |
| FRP-T2 | 9301 (431) | 9713 (450) | 3.3 (0.7) | |
| FRP-T3 | 10,689 (1217) | 10,721 (1221) | 3.9 (0.3) | |
| T300-33 | FRP-T1 | 9504 (881) | 9770 (906) | 6.1 (0.7) |
| T400-33 | FRP-T1 | 7649 (940) | 7863 (966) | 8.4 (0.7) |
| Variant | Construction | Error Bending Strength (%) | Error Deformation at Failure (%) |
|---|---|---|---|
| T120-0 | FRP-T1 | 3 | 25 |
| FRP-T3 | 19 | 37 | |
| T120-33 | FRP-T1 | 1 | 21 |
| FRP-T2 | 1 | 39 | |
| FRP-T3 | 4 | 46 | |
| T300-33 | FRP-T1 | 7 | 16 |
| T400-33 | FRP-T1 | 15 | 18 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pham, M.Q.; Bollengier, Q.; Rabe, D.; Lang, T.G.; Häntzsche, E.; Trümper, W.; Cherif, C.; Gereke, T. Meso-Scale Finite Element Model for Rib-Stiffened Composites with Biaxial Weft-Knitted Reinforcements. J. Compos. Sci. 2023, 7, 175. https://doi.org/10.3390/jcs7050175
Pham MQ, Bollengier Q, Rabe D, Lang TG, Häntzsche E, Trümper W, Cherif C, Gereke T. Meso-Scale Finite Element Model for Rib-Stiffened Composites with Biaxial Weft-Knitted Reinforcements. Journal of Composites Science. 2023; 7(5):175. https://doi.org/10.3390/jcs7050175
Chicago/Turabian StylePham, Minh Quang, Quentin Bollengier, David Rabe, Tobias Georg Lang, Eric Häntzsche, Wolfgang Trümper, Chokri Cherif, and Thomas Gereke. 2023. "Meso-Scale Finite Element Model for Rib-Stiffened Composites with Biaxial Weft-Knitted Reinforcements" Journal of Composites Science 7, no. 5: 175. https://doi.org/10.3390/jcs7050175
APA StylePham, M. Q., Bollengier, Q., Rabe, D., Lang, T. G., Häntzsche, E., Trümper, W., Cherif, C., & Gereke, T. (2023). Meso-Scale Finite Element Model for Rib-Stiffened Composites with Biaxial Weft-Knitted Reinforcements. Journal of Composites Science, 7(5), 175. https://doi.org/10.3390/jcs7050175









