A Numerical Method for Unstable Propagation of Damage in Fiber-Reinforced Plastics with an Implicit Static FE Solver
Abstract
1. Introduction
2. Numerical Method
2.1. A Method of Failure Path Tracking
2.2. Modification of Program Flow of a Finite Element Code
2.3. Constitutive Models of the Material
3. Numerical Examples
3.1. Open-Hole Compression Tests
3.1.1. FEA Model
3.1.2. Results and Discussion
3.2. Double-Cantilever Beam Tests
3.2.1. FEA Model
3.2.2. Results and Discussion
3.3. Discussion on Characteristics of the Present Methodology
3.3.1. Applicability to Unstable Damage Propagation
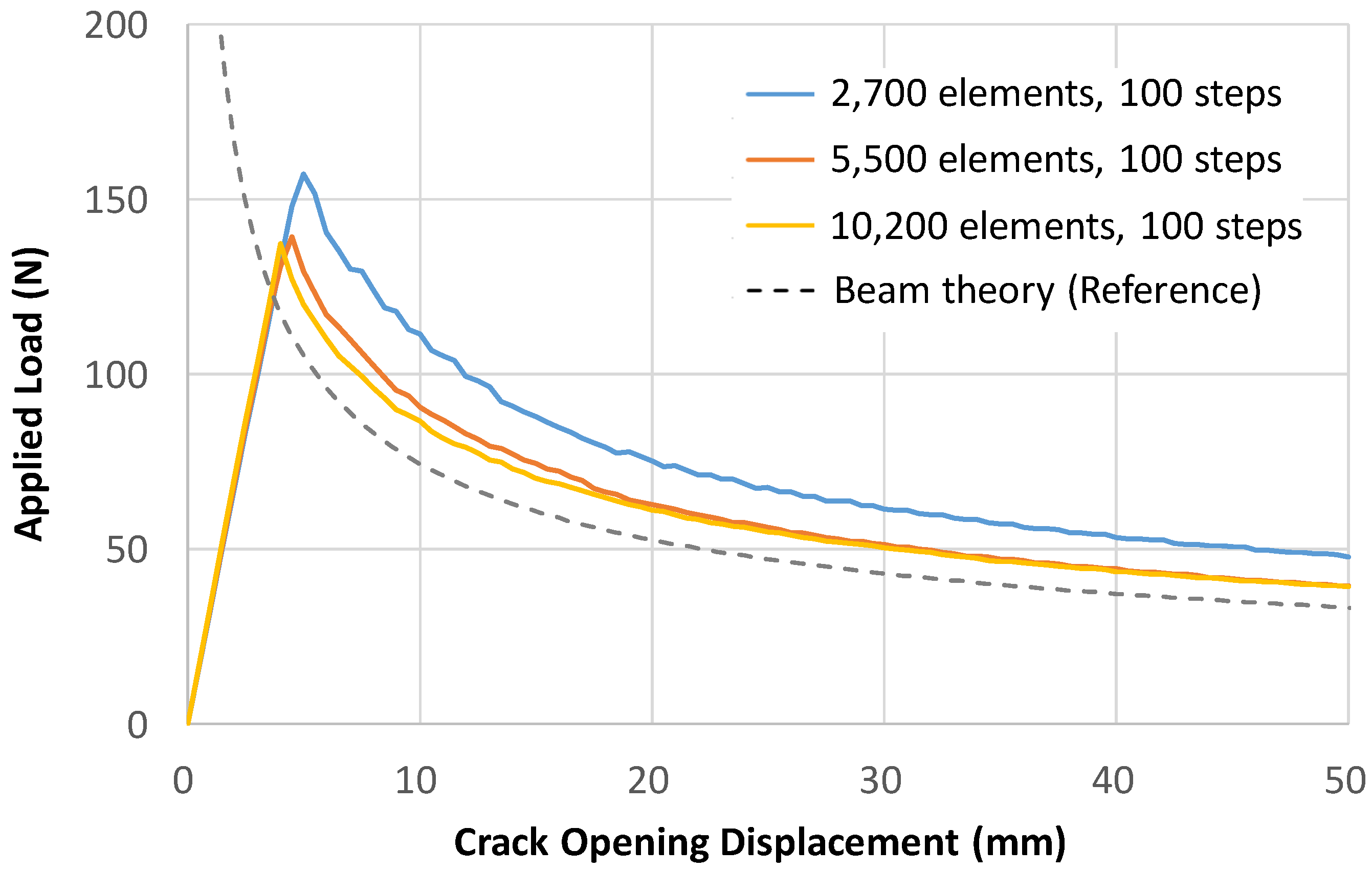
3.3.2. Dependency on Mesh Density
3.3.3. Dependency on Loading History
4. Conclusions
- The method is applicable to the problems of unstable damage propagation, which are usually difficult to solve by an implicit static FE solver.
- The dependency of solutions on mesh density with the present method is so small that it is applicable to analyses with a wide variety of element sizes without losing accuracy.
- A solution by the present method reaches a single state of mechanical equilibrium in analyses without dependency on loading history.
- In further research, this method is going to be applied to analyses of Filled-Hole Compression tests in which one of the major challenges is modelling the contact between the hole and a fastener inserted in the hole. The present method will contribute toward coping with this challenge because it enables an implicit FE solver to calculate unstable damage propagation with reasonable consideration of the contact conditions. In addition, the mesoscopic constitutive law for fiber compressive damage at the contact point is going to be further studied.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Jones, R.M. Mechanics of Composite Materials, 2nd ed.; Taylor and Francis Book; CRC Press: Boca Raton, FL, USA, 1998. [Google Scholar]
- Morais, A.B. Open-hole tensile strength of quasi-isotropic laminates. Compos. Sci. Technol. 2000, 60, 1997–2004. [Google Scholar] [CrossRef]
- Wisnom, M.R.; Hallett, S.R. The role of delamination in strength, failure mechanism and hole size effect in open hole tensile tests on quasi-isotropic laminates. Compos. Part A 2009, 40, 335–342. [Google Scholar] [CrossRef]
- Soutis, C. Damage Tolerance of Open-Hole CFRP Laminates Loaded in Compression. Compos. Eng. 1994, 4, 317–327. [Google Scholar] [CrossRef]
- Schltheis, C.R.; Waas, A.M. Compressive failure of composites, Part I: Testing and micromechanical theories. Prog. Aerosp. Sci. 1996, 32, 1–42. [Google Scholar] [CrossRef]
- Chang, F.K.; Lessard, L.B. Damage Tolerance of Laminated Composites Containing an Open Hole and Subjected to Compressive Loadings: Part I—Analysis. J. Compos. Mater. 1991, 25, 2–43. [Google Scholar] [CrossRef]
- Pinho, S.T.; Iannucci, L.; Robinson, P. Physically based failure models ad criteria for laminated fibre-reinforced composites with emphasis on fibre kinking. Part I: Development. Compos. Part A 2006, 37, 67–73. [Google Scholar]
- Hallett, S.R.; Green, B.G.; Jiang, W.G.; Wisnom, M.R. An experimental and numerical investigation into the damage mechanisms in notched composites. Compos. Part A 2009, 40, 613–624. [Google Scholar] [CrossRef]
- Camanho, P.P.; Erçin, G.H.; Catalanotti, G.; Mahdi, S.; Linde, P. A finite fracture mechanics model for the prediction of the open-hole strength of composite laminates. Compos. Part A 2012, 43, 1219–1225. [Google Scholar] [CrossRef]
- Suemasu, H.; Naito, Y.; Gozu, K.; Aoki, Y. Damage initiation and growth in composite laminates during open hole compression tests. Adv. Compos. Mater. 2012, 21, 209–220. [Google Scholar] [CrossRef]
- Hashin, Z. Failure criteria for unidirectional fibre composites. J. Appl. Mech. 1980, 47, 329–334. [Google Scholar] [CrossRef]
- Pinho, S.T.; Davila, C.G.; Camanho, P.P.; Iannucci, L.; Robinson, P. Failure Models and Criteria for FRP under In-Plane or Three-Dimensional Stress States Including Shear Non-Linearity; NASA Technical Memorandum TM-2005-213530; NASA: Washington, DC, USA, 2005. [Google Scholar]
- Eshelby, J.D. The determination of the elastic field of an ellipsoidal inclusion, and related problems. Proc. R. Soc. A 1957, 241, 376–396. [Google Scholar]
- Laws, N. A note on interaction energies associated with cracks in anisotropic solids. Philos. Mag. 1977, 36, 367–372. [Google Scholar] [CrossRef]
- Orifici, A.C.; Thomson, R.S. Review of methodologies for composite material modelling incorporating failure. Compos. Struct. 2008, 86, 194–210. [Google Scholar] [CrossRef]
- Liu, P.F.; Zheng, J.Y. Recent developments on damage modeling and finite element analysis for composite laminates: A review. Mater. Des. 2010, 31, 3825–3834. [Google Scholar] [CrossRef]
- Maneval, V.; Vedvik, N.-P.; Echtermeyer, A.T. Progressive Fatigue Modelling of Open-Hole Glass-Fibre Epoxy Laminates. J. Compos. Sci. 2023, 7, 516. [Google Scholar] [CrossRef]
- Yang, F.; Li, Y.; Li, B. Characterization of Failure Behavior in Unidirectional Fiber-Reinforced Polymer via Off-Axis Compression on Small Block Specimens. Polymers 2023, 15, 4699. [Google Scholar] [CrossRef]
- Deng, J.; Zhou, J.; Wu, T.; Liu, Z.; Wu, Z. Review and Assessment of Fatigue Delamination Damage of Laminated Composite Structures. Materials 2023, 16, 7677. [Google Scholar] [CrossRef] [PubMed]
- Ravinath, G.; Wessley, J.J. LS-Dyna Impact Modelling on Carbon Fibre Reinforced Polymers (CFRP) Composite Aircraft Panel with Various Impactors. Eng. Proc. 2023, 59, 26. [Google Scholar] [CrossRef]
- Liu, B.; Lai, J.; Liu, H.; Huang, Z.; Liu, T.; Xia, Y.; Zhang, W. Finite Element Analysis of the Effect for Different Thicknesses and Stitching Densities under the Low-Velocity Impact of Stitched Composite Laminates. Polymers 2023, 15, 4628. [Google Scholar] [CrossRef]
- Tsivouraki, N.; Tserpes, K.; Sioutis, I. Modelling of Fatigue Delamination Growth and Prediction of Residual Tensile Strength of Thermoplastic Coupons. Materials 2024, 17, 362. [Google Scholar] [CrossRef]
- Murakami, S. Continuum Damage Mechanics; Springer: Dordrecht, The Netherland, 2014. [Google Scholar]
- Pinho, S.T.; Iannucci, L.; Robinson, P. Physically based failure models ad criteria for laminated fibre-reinforced composites with emphasis on fibre kinking. Part II: FE implementation. Compos. Part A 2006, 37, 766–777. [Google Scholar] [CrossRef]
- Hallett, S.; Jiang, W.G.; Khan, B.; Wisnom, M. Modelling the interaction between matrix cracks and delamination damage in scaled quasi-isotropic specimens. Compos. Sci. Technol. 2008, 68, 80–89. [Google Scholar] [CrossRef]
- Su, Z.C.; Tay, T.E.; Ridha, M.; Chen, B.Y. Progressive damage modeling of open-hole composite laminates under compression. Compos. Struct. 2015, 122, 507–517. [Google Scholar] [CrossRef]
- Shivakumar, K.N.; Tan, P.W.; Newman, J.C. A virtual crack-closure technique for calculating stress intensity factors for cracked three dimensional bodies. Int. J. Fract. 1988, 36, 43–50. [Google Scholar] [CrossRef]
- Qian, Q.; Xie, D. Analysis of mixed mode dynamic crack propagation by interface element based on virtual crack closure technique. Eng. Fract. Mech. 2007, 74, 807–814. [Google Scholar] [CrossRef]
- Camanho, P.P.; Dávila, C.G. Mixed-Mode Decohesion Finite Elements for the Simulation of Delamination in Composite Materials; NASA Technical Memorandum TM-2002-211737; NASA: Washington, DC, USA, 2002. [Google Scholar]
- Karihaloo, B.L.; Xiao, Q.Z. Modelling of stationary and growing cracks in FE framework without remeshing: A state-of-the-art review. Comput. Struct. 2003, 81, 119–129. [Google Scholar] [CrossRef]
- Giner, E.; Sukumar, N.; Tarancón, J.E.; Fuenmayor, F.J. An Abaqus implementation of the extended finite element method. Eng. Fract. Mech. 2009, 76, 347–368. [Google Scholar] [CrossRef]
- Higuchi, R.; Warabi, S.; Yoshimura, A.; Nagashima, T.; Yokozeki, T.; Okabe, T. Experimental and numerical study on progressive damage and failure in composite laminates during open-hole compression tests. Compos. Part A 2021, 145, 106300. [Google Scholar] [CrossRef]
- Courant, R.; Friedrichs, K.; Lewy, H. On the partial difference equations of mathematical physics. IBM J. Res. Dev. 1967, 11, 215–234. [Google Scholar] [CrossRef]
- Marc Volume A: Theory and User Information, version 2017; 880-881; MSC Software: Newport Beach, CA, USA, 2017.
- Kondo, A.; Takahashi, M.; Watanabe, Y.; Iwahori, Y.; Hara, E.; Katoh, H. Numerical analysis of filled-hole compression tests with different materials of inclusions. In Proceedings of the 23rd International Conference on Composite Materials, Belfast, UK, 30 July–4 August 2023. [Google Scholar]
- Ripling, E.J.; Mostovoy, S.; Patrick, R.L. Measuring fracture toughness of adhesive joints. Mater. Res. Stand. 1964, 4, 129–134. [Google Scholar]




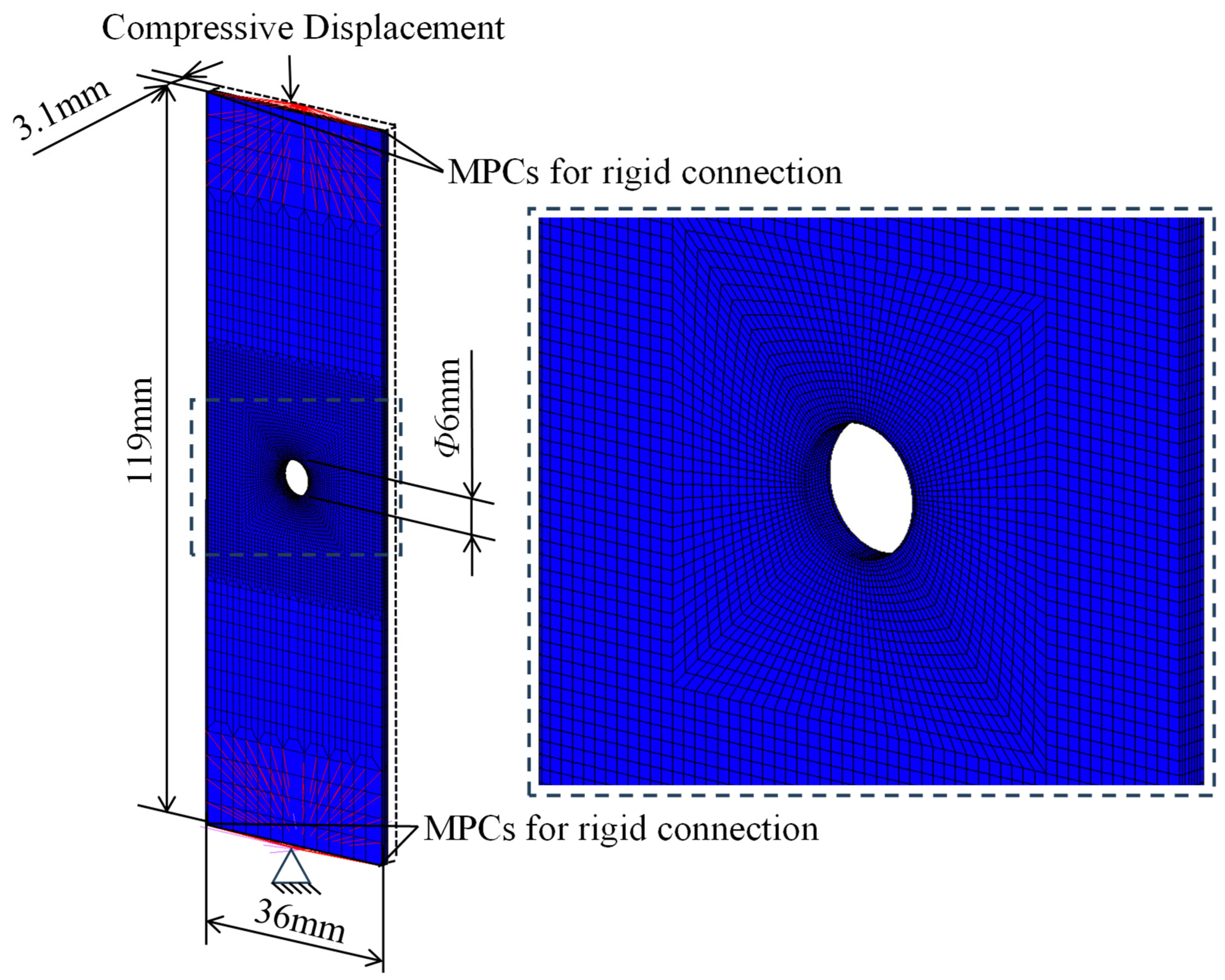
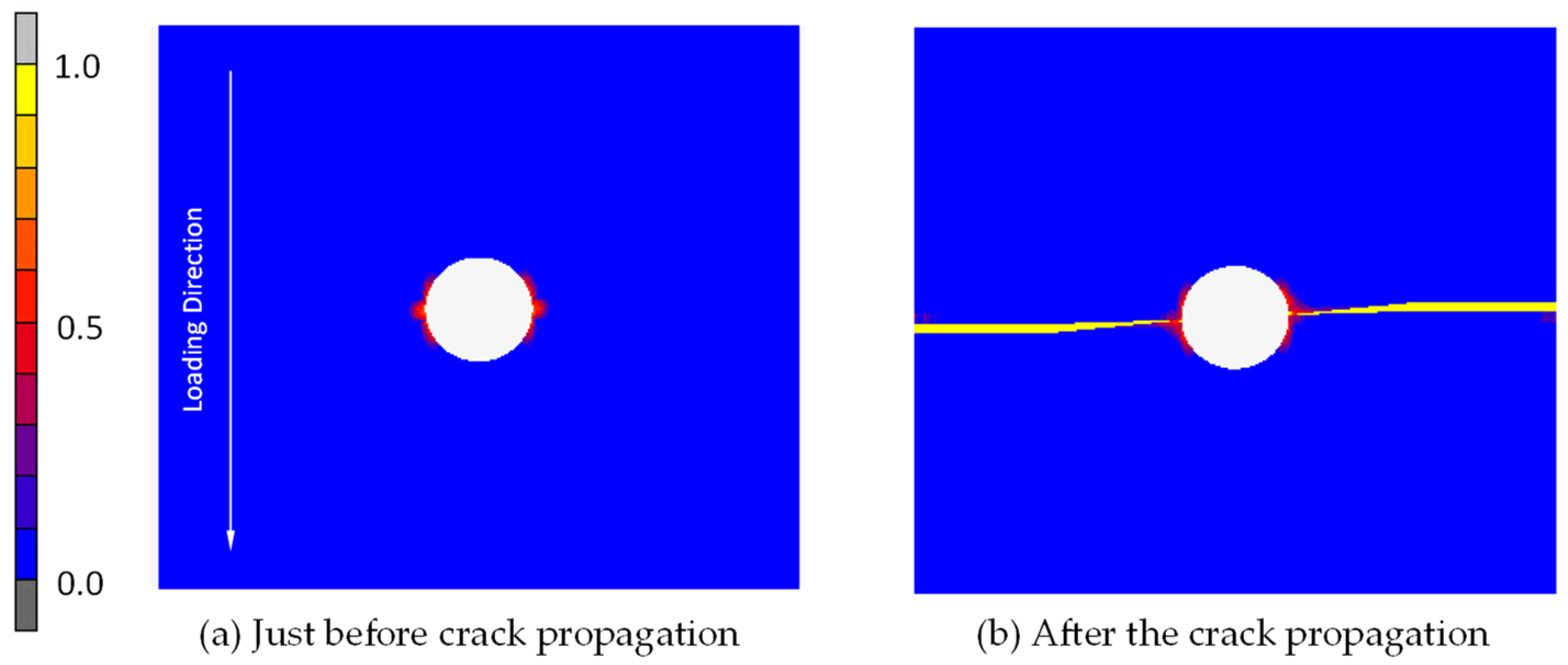
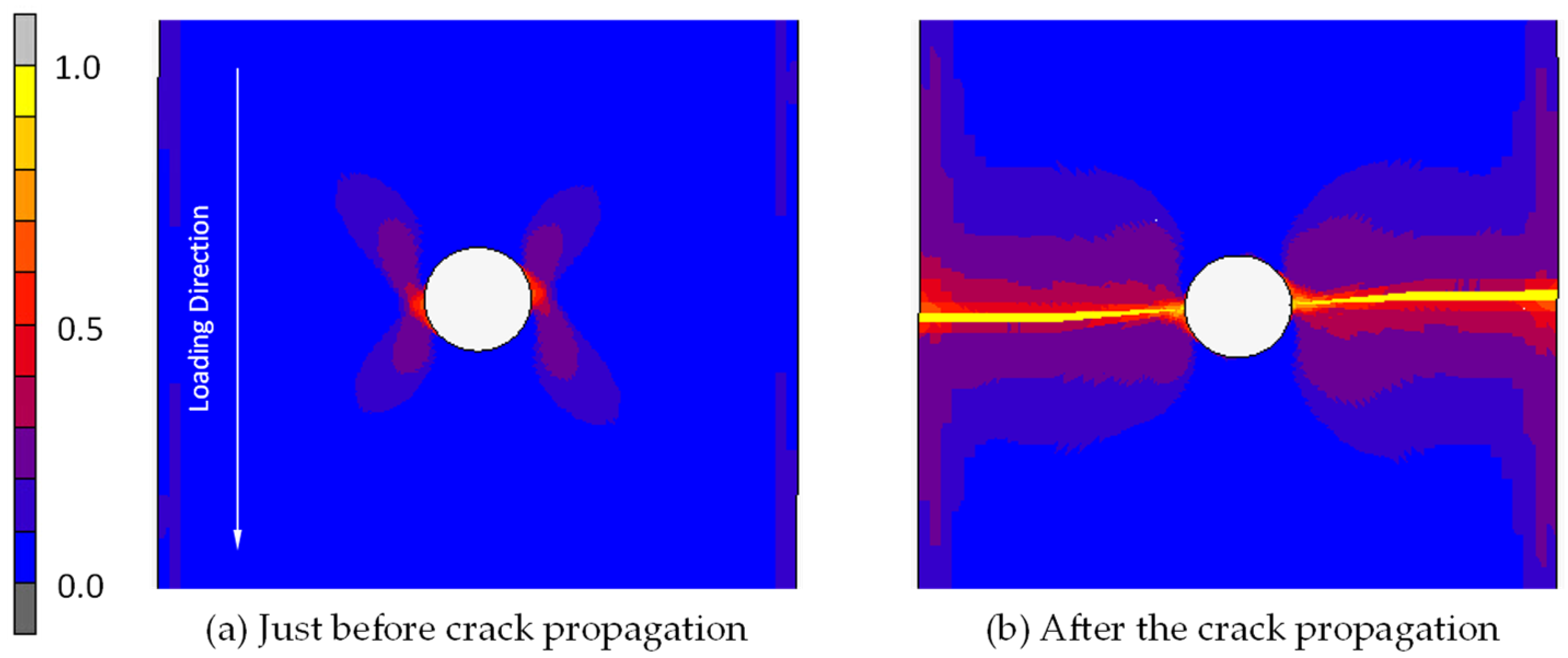



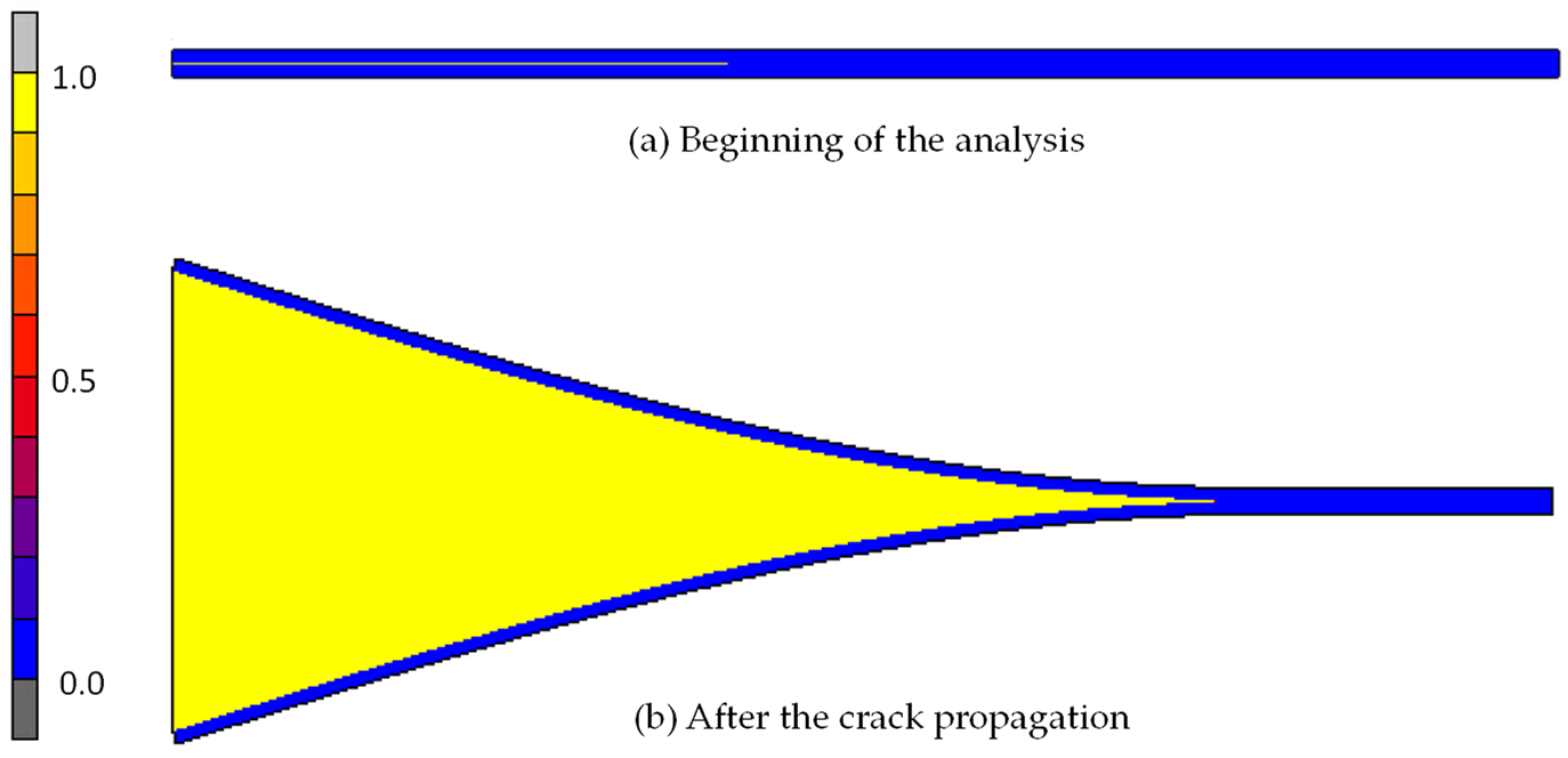

| EL | ET | EZ | νLT | νLZ | νTZ | GLT | GLZ | GTZ |
|---|---|---|---|---|---|---|---|---|
| 153 GPa | 8.00 GPa | 8.00 GPa | 0.340 | 0.344 | 0.544 | 4.82 GPa | 3.56 GPa | 2.30 GPa |
| Analysis Cases | Damage Mode | GcL (kJ/m2) | GcT (kJ/m2) | Iteration (Total) | Iteration (Unstable) | CPU Time (s) |
|---|---|---|---|---|---|---|
| 1 | L | 10.0 | N.A. 1 | 720 | 517 | 19,437.47 |
| 2 | L | 20.0 | N.A. 1 | 2158 | 897 | 57,782.35 |
| 3 | L, T | 10.0 | 2.0 | 318 | 212 | 9014.30 |
| 4 | L, T | 20.0 | 2.0 | 552 | 443 | 14,796.60 |
| Analysis Cases | Number of Elements | Number of Load Steps | Initial Crack (mm) | Iterations | CPU Time (s) |
|---|---|---|---|---|---|
| 1 | 2700 | 100 | 30 | 1548 | 1855.68 |
| 2 | 5500 | 100 | 30 | 1455 | 3419.63 |
| 3 | 10,200 | 100 | 30 | 2213 | 9550.84 |
| 4 | 2700 | 25 | 30 | 1263 | 1354.59 |
| 5 | 5500 | 25 | 30 | 1569 | 3413.81 |
| 6 | 10,200 | 25 | 30 | 1463 | 6094.47 |
| 7 | 2700 | 25 | 60 | 840 | 925.51 |
| 8 | 5500 | 25 | 60 | 1128 | 2494.51 |
| 9 | 10,200 | 25 | 60 | 1182 | 4970.65 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kondo, A.; Watanabe, Y.; Sakai, K.; Iwahori, Y.; Hara, E.; Katoh, H. A Numerical Method for Unstable Propagation of Damage in Fiber-Reinforced Plastics with an Implicit Static FE Solver. J. Compos. Sci. 2024, 8, 130. https://doi.org/10.3390/jcs8040130
Kondo A, Watanabe Y, Sakai K, Iwahori Y, Hara E, Katoh H. A Numerical Method for Unstable Propagation of Damage in Fiber-Reinforced Plastics with an Implicit Static FE Solver. Journal of Composites Science. 2024; 8(4):130. https://doi.org/10.3390/jcs8040130
Chicago/Turabian StyleKondo, Atsushi, Yutaro Watanabe, Kentaro Sakai, Yutaka Iwahori, Eiichi Hara, and Hisaya Katoh. 2024. "A Numerical Method for Unstable Propagation of Damage in Fiber-Reinforced Plastics with an Implicit Static FE Solver" Journal of Composites Science 8, no. 4: 130. https://doi.org/10.3390/jcs8040130
APA StyleKondo, A., Watanabe, Y., Sakai, K., Iwahori, Y., Hara, E., & Katoh, H. (2024). A Numerical Method for Unstable Propagation of Damage in Fiber-Reinforced Plastics with an Implicit Static FE Solver. Journal of Composites Science, 8(4), 130. https://doi.org/10.3390/jcs8040130





