Feedback-Driven Plasmonic-Thermal Route to Femtosecond-Laser-Induced Periodic Surface Structures in Silicon Indicated by Pump-Probe Scattering and Diffraction
Abstract
1. Introduction
2. Experimental Techniques
3. Material Properties
3.1. Crystalline Silicon
3.2. Amorphous Silicon
4. Spatial Frequencies and Plasmon Coupling
5. Results and Discussion
5.1. Dynamic Initial Feedback Mechanism
5.1.1. Micro-craters
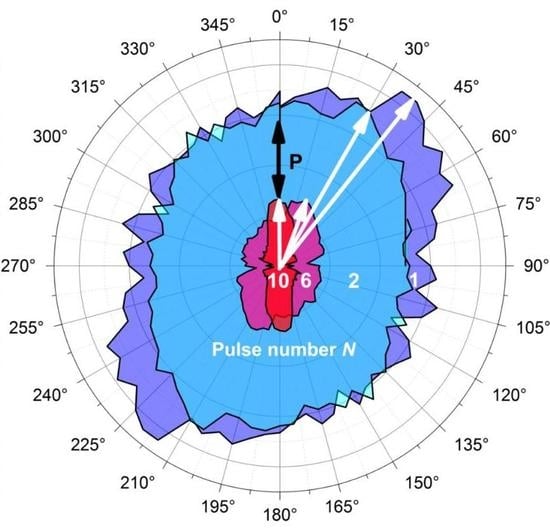
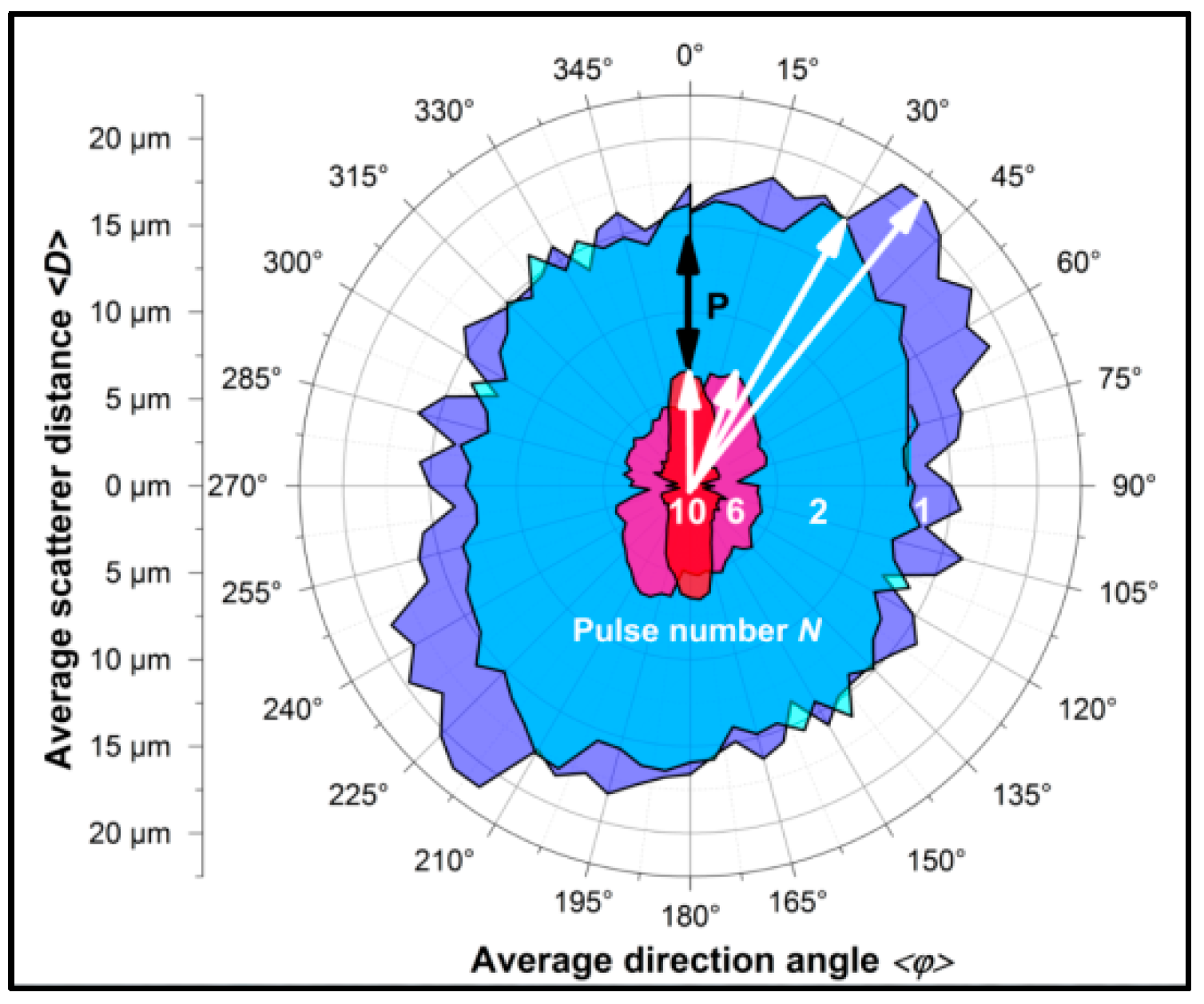
5.1.2. Distance Function and Angular Characteristics
5.2. Pump-Probe Scattering and Diffraction
5.2.1. Measuring Conditions
5.2.2. Initial Pulse (N = 1)
5.2.3. Pulse Number N = 2
5.2.4. Pulse Number N = 3
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A. Pump-Probe Scattering and Diffraction for N = 1

Appendix B. Pump-Probe Scattering and Diffraction for N = 2

Appendix C. Pump-Probe Scattering and Diffraction for N = 3

References
- Birnbaum, M. Semiconductor surface damage produced by Ruby lasers. J. Appl. Phys. 1965, 36, 3688–3689. [Google Scholar] [CrossRef]
- Messaoudi, H.; Das, S.K.; Lange, J.; Heinrich, F.; Schrader, S.; Frohme, M.; Grunwald, R. Femtosecond-laser induced periodic surface structures for surface enhanced Raman spectroscopy of biomolecules. In Progress in Nonlinear Nano-Optics; Sakabe, M., Lienau, C., Grunwald, R., Eds.; Springer: Berlin, Germany, 2015; pp. 207–219. [Google Scholar]
- Lübcke, A.; Andreev, A.A.; Höhm, S.; Grunwald, R.; Ehrentraut, L.; Schnürer, M. Prospects of target nanostructuring for laser proton acceleration. Sci. Rep. 2017, 7, 44030. [Google Scholar] [CrossRef]
- Khan, M.E.; Khan, M.M.; Cho, M.H. Recent progress of metal–graphene nanostructures in photocatalysis. Nanoscale 2018, 10, 9427–9440. [Google Scholar] [CrossRef]
- Khan, M.E.; Han, T.H.; Khan, M.M.; Karim, M.R.; Cho, M.H. Environmentally Sustainable Fabrication of Ag@g-C3N4 Nanostructures and Their Multifunctional Efficacy as Antibacterial Agents and Photocatalysts. ACS Appl. Nano Mater. 2018, 1, 2912–2922. [Google Scholar] [CrossRef]
- Bonse, J.; Höhm, S.; Kirner, S.V.; Rosenfeld, A.; Krüger, J. Laser-induced periodic surface structures—A scientific evergreen. IEEE J. Sel. Top. Quantum Electron. 2017, 23, 9000615. [Google Scholar] [CrossRef]
- Sipe, J.E.; Young, J.F.; Preston, J.S.; van Driel, H.M. Laser-induced periodic surface structure. I. Theory. Phys. Rev. B 1983, 27, 1141–1154. [Google Scholar] [CrossRef]
- Young, J.F.; Preston, J.S.; van Driel, H.M.; Sipe, J.E. Laser-induced periodic surface structure. II Experiments on Ge, Si, Al, and brass. Phys. Rev. B 1983, 27, 1155–1172. [Google Scholar] [CrossRef]
- Young, J.F.; Sipe, J.E.; Driel, H.M. Laser-induced periodic surface structure. III. Fluence regimes, the role of feedback, and details of the induced topography in germanium. Phys. Rev. B 1984, 30, 2001–2015. [Google Scholar] [CrossRef]
- Brueck, S.R.; Ehrlich, D.J. Simulated surface plasma-wave scattering and growth of a periodic structure in laser-photodeposited metal films. Phys. Rev. Lett. 1982, 48, 1678–1681. [Google Scholar] [CrossRef]
- Kaplan, A.E.; Miyazaki, K. Laser-induced surface nano-ripples as manifestation of Wigner excitons. In Proceedings of the Quantum Electronics and Laser Science Conference (QELS), Baltimore, MD, USA, 6–11 May 2007. [Google Scholar]
- Tsibidis, G.D.; Fotakis, C.; Stratakis, E. From ripples to spikes: A hydrodynamical mechanism to interpret femtosecond laser-induced self-assembles structures. Phys. Rev. B 2015, 92, 041405(R). [Google Scholar] [CrossRef]
- Costache, F.; Eckert, S.; Reif, J. On ultra-short laser pulse induced instabilities at the surface of non-metallic solids. Proc. SPIE 2006, 6261, 626107. [Google Scholar]
- Varlamova, O. Self-Organized Surface Patterns Originating from Femtosecond Laser-Induced Instability. Ph.D. Thesis, Technical University, Cottbus, Germany, November 2013. [Google Scholar]
- Reif, J.; Costache, F.; Henyk, M.; Pandelov, S.V. Ripples revisited: Non-classical morphology at the bottom of femtosecond laser ablation craters in transparent dielectrics. Appl. Surf. Sci. 2002, 197–198, 891–895. [Google Scholar] [CrossRef]
- Csete, M.; Hild, S.; Plettl, A.; Ziemann, P.; Bor, Z.; Marti, O. The role of original surface roughness in laser-induced periodic surface structure formation process on polycarbonate films. Thin Solid Films 2004, 453–454, 114–120. [Google Scholar] [CrossRef]
- Obara, G.; Maeda, N.; Miyanishi, T.; Terakawa, M.; Nedyalkov, N.N.; Obara, M. Plasmonic and Mie scattering control of far-field interference for regular ripple formation on various material substrates. Opt. Express 2011, 19, 19093–19103. [Google Scholar] [CrossRef]
- Kudryashov, S.I.; Makarov, S.V.; Ionin, A.A.; Nathala, C.S.R.; Ajami, A.; Ganz, T.; Assion, A.; Husinsky, W. Dynamic polarization flip in nanoripples on photoexcited Ti surface near its surface plasmon resonance. Opt. Lett. 2015, 40, 4967–4970. [Google Scholar] [CrossRef]
- Dufft, D.; Rosenfeld, A.; Das, S.K.; Grunwald, R.; Bonse, J. Femtosecond laser-induced periodic surface structures revisited: A comparative study on ZnO. J. Appl. Phys. 2009, 105, 034908. [Google Scholar] [CrossRef]
- Lübcke, A.; Schnürer, M.; Ehrentraut, L.; McGlynn, E.; Byrne, D.; Lowry, S.; Wehner, R.; Grunwald, R. Interaction of ultrafast laser pulses with nanostructure surfaces. In Encyclopedia of Interfacial Chemistry: Surface Science and Electrochemistry; Wandelt, K., Ed.; Elsevier: Oxford, UK, 2018; Volume 2, pp. 420–432. [Google Scholar]
- Bonse, J.; Rosenfeld, A.; Krüger, J. Femtosecond laser-induced periodic surface structures: Recent approaches to explain, their sub-wavelength periodicities. Proc. SPIE 2012, 7994, 79940M-7. [Google Scholar]
- Van Driel, H.M.; Dworschak, K. Locking of optical and thermodynamical length scales in laser-induced melt-solid patterns on silicon. Phys. Rev. Lett. 1992, 69, 3487–3490. [Google Scholar] [CrossRef]
- Jia, T.Q.; Chen, H.X.; Huang, M.; Zhao, F.L.; Qiu, J.R.; Li, R.X.; Xu, Z.Z.; He, X.K.; Zhang, J.; Kuroda, H. Formation of nanogratings on the surface of a ZnSe crystal irradiated by femtosecond laser pulses. Phys. Rev. B 2005, 72, 125429. [Google Scholar] [CrossRef]
- Wagner, R.; Gottmann, J.; Horn, A.; Kreutz, E.W. Subwavelength ripple formation induced by tightly focused femtosecond laser radiation. Appl. Surf. Sci. 2006, 252, 8576–8579. [Google Scholar] [CrossRef]
- Zhao, Q.Z.; Malzer, S.; Wang, L.J. Formation of subwavelength periodic structures on tungsten induced by ultrashort laser pulses. Opt. Lett. 2007, 32, 1932–1934. [Google Scholar] [CrossRef]
- Ganeev, R.A. Formation of different periodic nanostructures on semiconductors. Opt. Spectrosc. 2009, 106, 142–146. [Google Scholar] [CrossRef]
- LeHarzig, R.; Schuck, H.; Sauer, D.; Anhut, T.; Riemann, I.; König, K. Sub-100 nm nanostructuring of silicon by ultrashort laser pulses. Opt. Express 2005, 13, 6651–6656. [Google Scholar] [CrossRef]
- Das, S.K.; Messaoudi, H.; Debroy, A.; McGlynn, E.; Grunwald, R. Multiphoton excitation of surface plasmon-polaritons and scaling of nanoripple formation in large bandgap materials. Opt. Mater. Express 2013, 3, 1705–1715. [Google Scholar] [CrossRef]
- Das, S.K.; Dufft, D.; Rosenfeld, A.; Bonse, J.; Bock, M.; Grunwald, R. Femtosecond-laser-induced quasiperiodic nanostructures on TiO2 surfaces. J. Appl. Phys. 2009, 105, 084912. [Google Scholar] [CrossRef]
- Huang, M.; Zhao, F.; Cheng, Y.; Xu, N.; Xu, Z. Origin of laser-induced near-subwavelength ripples: Interference between surface plasmons and incident laser. ACS Nano 2009, 3, 4062–4070. [Google Scholar] [CrossRef]
- Huang, M.; Cheng, Y.; Zhao, F.; Xu, Z. The significant role of plasmonic effects in femtosecond laser-induced grating fabrication on the nanoscale. Ann. Phys. 2013, 525, 74–86. [Google Scholar] [CrossRef]
- Garrelie, F.; Colombier, J.P.; Pigeon, F.; Tonchev, S.; Faure, N.; Bounhalli, M.; Reynaud, S.; Parriaux, O. Evidence of surface plasmon resonance in ultrafast laser-induced ripples. Opt. Express 2008, 19, 9035–9043. [Google Scholar] [CrossRef]
- Robitaille, A.; Boulais, E.; Meunier, M. Mechanisms of plasmon-enhanced femtosecond laser nanoablation of silicon. Opt. Express 2013, 21, 9703–9710. [Google Scholar] [CrossRef]
- Derrien, T.J.-Y.; Itina, T.E.; Torres, R.; Sarnet, T.; Sentis, M. Possible surface plasmon polariton excitation under femtosecond laser irradiation of silicon. J. Appl. Phys. 2013, 114, 083104. [Google Scholar] [CrossRef]
- Derrien, T.J.-Y.; Krüger, J.; Itina, T.E.; Höhm, S.; Rosenfeld, A.; Bonse, J. Rippled area formed by surface plasmon polaritons upon femtosecond laser double-pulse irradiation of silicon. Appl. Phys. A 2014, 117, 77–81. [Google Scholar] [CrossRef]
- Straub, M.; Afshar, M.; Feili, D.; Seidel, H.; König, K. Surface plasmon polariton model of high-spatial frequency laser-induced periodic surface structure generation in silicon. J. Appl. Phys. 2012, 111, 124315. [Google Scholar] [CrossRef]
- Zhu, J.-T.; Shen, Y.-F.; Li, W.; Chen, X.; Yin, G.; Chen, D.-Y.; Zhao, L. Effect of polarization on femtosecond laser pulses structuring silicon surface. Appl. Surf. Sci. 2006, 252, 2752–2756. [Google Scholar] [CrossRef]
- Song, H.-Y.; Liu, S.-B.; Liu, H.Y.; Wang, Y.; Chen, T.; Dong, X.-M. Subwavelength topological structures resulting from surface two-plasmon resonance by femtosecond laser exposure solid surface. Opt. Express 2016, 24, 12151–12165. [Google Scholar] [CrossRef]
- Ionin, A.A.; Kudryashov, S.I.; Makarov, S.V. Nanoscale surface boiling in sub-threshold spallation of bulk aluminum and gold by single femtosecond laser pulses. Laser Phys. Lett. 2016, 13, 025603. [Google Scholar] [CrossRef]
- Nathala, C.C.S.; Ali, A.; Ionin, A.A.; Kudryashov, S.I.; Makarov, S.V.; Ganz, T.; Assion, A.; Hussinsky, W. Experimental study of fs-laser induced sub-100-nm periodic surface structures on titanium. Opt. Express 2015, 23, 5915–5929. [Google Scholar] [CrossRef]
- Mermillaud-Blondin, A.; Bonse, J.; Rosenfeld, A.; Hertel, I.V.; Meshcheryakov, Y.P.; Bulgakova, N.M.; Audouard, E.; Stoian, R. Dynamics of femtosecond laser induced voidlike structures in fused silica. Appl. Phys. Lett. 2009, 94, 041911. [Google Scholar] [CrossRef]
- Zimmermann, F.; Plech, A.; Richter, S.; Tünnermann, A.; Nolte, S. The onset of ultrashort pulse-induced nanogratings. Laser-Photonics Rev. 2016, 10, 327–334. [Google Scholar] [CrossRef]
- Puerto, D.; Garcia-Lechuga, M.; Solis, J.; Siegel, J. Study of phase change LIPSS formation in Si by fs-resolved microscopy. In Proceedings of the 2016 Conference on Lasers and Electro-Optics (CLEO), San Jose, CA, USA, 5–10 June 2016. [Google Scholar]
- Garcia-Lechuga, M.; Puerto, D.; Fuentes-Edfuf, Y.; Solis, J.; Siegel, J. Ultrafast moving-spot microscopy: Birth and growth of laser of laser-induced periodic surface surface structures. ACS Photonics 2016, 3, 1961–1967. [Google Scholar] [CrossRef]
- Bonse, J.; Rosenfeld, A.; Krüger, J. On the role of surface plasmon polaritons in the formation of laser-induced periodic surface structures upon irradiation of silicon by femtosecond laser pulses. J. Appl. Phys. 2009, 106, 104910. [Google Scholar] [CrossRef]
- Satapathy, P.; Panda, R.; Sahoo, R.; Shukla, M.K.; Khatua, M.K.; Sahoo, P.K.; Das, R.; Das, S.K. Observation of continuous and non-continuous laser-induced periodic structures on silicon. J. Laser Micro Nanoeng. 2018, 13, 146–149. [Google Scholar]
- Höhm, S.; Herzlieb, M.; Rosenfeld, A.; Krüger, J.; Bonse, J. Femtosecond laser-induced periodic surface structures on silicon upon polarization controlled two-color double-pulse irradiation. Opt. Express 2015, 23, 61–71. [Google Scholar] [CrossRef]
- Tan, B.; Venkatakrishnan, K. A femtosecond laser-induced periodical surface structure on crystalline silicon. J. Micromech. Microeng. 2006, 16, 1080–1085. [Google Scholar] [CrossRef]
- Hamad, S.; Moram, S.S.B.; Yendeti, B.; Podagatlapalli, G.K. Femtosecond laser-induced, nanoparticle-embedded periodic surface structures on crystalline silicon for reproducible and multiutility SERS platforms. ACS Omega 2018, 3, 18420–18432. [Google Scholar] [CrossRef]
- Cheng, K.; Liu, J.; Cao, K.; Chen, L.; Zhang, Y.; Jiang, Q.; Feng, D.; Zhang, S.; Sun, Z.; Jia, T. Ultrafast dynamics of single-pulse femtosecond laser-induced periodic ripples on the surface of a gold film. Phys. Rev. B 2018, 98, 184106. [Google Scholar] [CrossRef]
- Wehner, R.; Grunwald, R. Combined plasmonic and thermal mechanism of laser-induced nanostructure formation in silicon. In Proceedings of the 2017 Conference on Lasers and Electro-Optics Europe & European Quantum Electronics Conference (CLEO/Europe-EQEC), Munich, Germany, 25–29 June 2017. [Google Scholar]
- Sokolowski-Tinten, K.; von der Linde, D. Generation of dense electron-hole plasmas in silicon. Phys. Rev. B 2000, 61, 2643–2650. [Google Scholar] [CrossRef]
- Gamaly, E.G.; Rode, A.V. Transient optical properties of dielectrics and semiconductors excited by an ultrashort laser pulse. J. Opt. Soc. Am. B 2014, 31, C36–C43. [Google Scholar] [CrossRef]
- Jellison, G.E., Jr. Optical functions of silicon determined by two-channel polarization modulation ellipsometry. Opt. Mater. 1992, 1, 41–47. [Google Scholar] [CrossRef]
- Sabbah, A.J.; Riffe, D.M. Femtosecond pump-probe reflectivity study of silicon carrier dynamics. Phys. Rev. B 2002, 66, 165217. [Google Scholar] [CrossRef]
- Palik, E. Handbook of Optical Constants of Solids, 4th ed.; Academic Press: Cambridge, MD, USA, 1998. [Google Scholar]
- Kittel, C. Introduction to Solid State Physics, 5th ed.; John Wiley and Sons Inc.: New York, NY, USA, 2005; p. 396. [Google Scholar]
- Shazad, M. Infrared Surface Plasmon Polaritons on Semiconductor, Semimetal and Conducting Polymer. Ph.D. Thesis, University of Central Florida, Orlando, FL, USA, 2012; p. 15. [Google Scholar]
- Gnilitskyi, I.; Grudzev, V.; He, X.; Sergaeva, O.; Ji, P.; White, T.; Zhang, Y. Sub-surface layer of silicon singe crystal periodically nanostructured by near-infrared femtosecond laser pulses. In Proceedings of the Conference on Lasers and Electro-Optics/Pacific Rim, Hong Kong, China, 29 July–3 August 2018. [Google Scholar]
- He, S.; Nivas, J.J.J.; Vecchione, A.; Hu, M.; Amoruso, S. On the generation of grooves on crystalline silicon irradiated by femtosecond laser pulses. Opt. Express 2016, 24, 3238–3247. [Google Scholar] [CrossRef]
- Bonse, J. All-optical characterization of single femtosecond laser-pulse-induced amorphization in silicon. Appl. Phys. A 2006, 84, 63–66. [Google Scholar] [CrossRef]
- Piers, D.; Spicer, W. Electronic structure of amorphous Si from photoemission and optical studies. Phys. Rev. B 1972, 5, 3017–3029. [Google Scholar] [CrossRef]
- Choi, T.; Hwang, D.; Grigoropoulos, C. Ultrafast laser-induced crystallization of amorphous silicon films. Opt. Eng. 2003, 42, 3383–3389. [Google Scholar]
- Pitarke, J.M.; Silkin, V.M.; Chulkov, E.V.; Echenique, P.M. Theory of surface plasmons and surface-plasmon polaritons. Rep. Prog. Phys. 2007, 70, 1–87. [Google Scholar] [CrossRef]
- Teng, Y.-Y.; Stern, E.A. Plasma Radiation from Metal Grating Surfaces. Phys. Rev. Lett. 1967, 19, 511–514. [Google Scholar] [CrossRef]
- Sprafke, A. Optische Nahfeld-Wechselwirkungen von Plasmonen mit Ihrer Umgebung. Ph.D. Thesis, University Aachen, Aachen, Germany, 2014. [Google Scholar]
- Tomita, T.; Kinoshita, K.; Matsuo, S.; Hashimoto, S. Effect of surface roughening on femtosecond laser-induced ripple structures. Appl. Phys. Lett. 2007, 90, 153115. [Google Scholar] [CrossRef]
- Roeterdink, W.G.; Juurlink, L.B.F.; Vaughan, O.P.H.; Dura Diez, J.; Bonn, M.; Kleyn, A.W. Coulomb explosion in femtosecond laser ablation of Si (111). Appl. Phys. Lett. 2003, 82, 4190. [Google Scholar] [CrossRef]
- Höhm, S.; Rosenfeld, A.; Krüger, J.; Bonse, J. Femtosecond diffraction dynamics of laser-induced periodic surface structures on fused silica. Appl. Phys. Lett. 2013, 102, 054102. [Google Scholar] [CrossRef]
- Qi, L.; Nishii, K.; Namba, Y. Regular sub-wavelength ripples formation by femtosecond laser pulses on silicon. Optik 2015, 126, 4905–4909. [Google Scholar] [CrossRef]
- Cvecek, K.; Miyamoto, I.; Schmidt, M. Gas bubble formation in fused silica generated by ultra-short laser pulses. Opt. Express 2014, 22, 15877–15893. [Google Scholar] [CrossRef]
- Liang, F.; Vallée, R.; Chin, S.L. Physical evolution of nanograting inscription on the surface of fused silica. Opt. Express 2012, 2, 900–906. [Google Scholar] [CrossRef]
- Jia, X.; Yuan, Y.; Yang, D.; Jia, T. Ultrafast time-resolved imaging of femtosecond laser-induced periodic surface structures on GaAs. Chin. Opt. Lett. 2014, 12, 113203. [Google Scholar]
- Gemini, L.; Hashida, M.; Shimizu, M.; Miyasaka, Y.; Inoue, S.; Tokita, S.; Limpouch, J.; Mocek, T.; Sakabe, S. Periodic nanostructures self-formed on silicon and silicon carbide by femtosecond laser irradiation. Appl. Phys. A 2014, 117, 49–54. [Google Scholar] [CrossRef]



© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wehner, R.; Grunwald, R. Feedback-Driven Plasmonic-Thermal Route to Femtosecond-Laser-Induced Periodic Surface Structures in Silicon Indicated by Pump-Probe Scattering and Diffraction. Surfaces 2019, 2, 277-294. https://doi.org/10.3390/surfaces2020021
Wehner R, Grunwald R. Feedback-Driven Plasmonic-Thermal Route to Femtosecond-Laser-Induced Periodic Surface Structures in Silicon Indicated by Pump-Probe Scattering and Diffraction. Surfaces. 2019; 2(2):277-294. https://doi.org/10.3390/surfaces2020021
Chicago/Turabian StyleWehner, Robin, and Ruediger Grunwald. 2019. "Feedback-Driven Plasmonic-Thermal Route to Femtosecond-Laser-Induced Periodic Surface Structures in Silicon Indicated by Pump-Probe Scattering and Diffraction" Surfaces 2, no. 2: 277-294. https://doi.org/10.3390/surfaces2020021
APA StyleWehner, R., & Grunwald, R. (2019). Feedback-Driven Plasmonic-Thermal Route to Femtosecond-Laser-Induced Periodic Surface Structures in Silicon Indicated by Pump-Probe Scattering and Diffraction. Surfaces, 2(2), 277-294. https://doi.org/10.3390/surfaces2020021