Ultrasonic Study of Longitudinal Critically Refracted and Bulk Waves of the Heat-Affected Zone of a Low-Carbon Steel Welded Joint under Fatigue

Abstract

Currently, ultrasonic methods for assessing the fatigue lifetime of various structural materials are being actively developed. Many steel constructions are made by welding. The weld heat-affected zone is the weak point of the construction, as it is most susceptible to destruction. Therefore, it is actually important to search for acoustic parameters that uniquely characterize the structural damage accumulation in the heat-affected zone of a welded joint in order to predict failure. In this work, the specimens were made from the base metal and the welded joint’s heat-affected zone. The specimens were subjected to uniaxial tension–compression under a symmetrical cycle in the region of low-cycle fatigue with control of the strain amplitude. The propagation bulk velocities of longitudinal, shear waves and subsurface longitudinal critically refracted (LCR) waves during cyclic loading were studied. The acoustic birefringence of shear waves was calculated, and a similar parameter was proposed for longitudinal and LCR waves. The dependence of the elastic modulus ratio on the cycle ratio was obtained. It was shown that the acoustic parameters change most intensively in the heat-affected zone. According to the data of the C₃₃/C₅₅ ratio changes measured through the ultrasonic method, a formula for calculating the remaining fatigue life in the heat-affected zone was proposed.

1. Introduction

The fatigue of metal components is a common problem in the oil, gas, chemical, nuclear, aerospace, marine, hydropower, and building industries. For load-bearing elements of welded structures, hot-rolled, low-carbon steel is widely used in many industries due to its machinability, weldability, and mechanical properties. Welded joints are weakened areas due to the inhomogeneity of the materials’ microstructure and mechanical properties [1,2,3]. Furthermore, during welding, there is usually a heat-affected zone (HAZ). The HAZ is the weakest point, so material destruction most often occurs there [2,3].

Predicting the fatigue behavior of welded joints is a difficult problem. Theoretical approaches to the fatigue analysis of welded joints are complicated by many influencing factors [1]. Therefore, it is important to carry out in-service monitoring of the welded joint material state for timely maintenance and repair work in order to prevent failures and accidents in these constructions. To monitor the damage of components experiencing fatigue, including welded joints, nondestructive evaluation techniques can be used [4]. Ultrasonic testing is one of the most commonly used NDT methods [5,6].

The fatigue process usually consists of several stages: incubation, initiation, and, further, the development of microcracks, the emergence of a main crack, and the main crack’s growth into a final fracture. The early stages of fatigue are rarely monitored using nondestructive testing methods, in contrast to the stage of main crack growth. Meanwhile, the accumulation of microstructural damage begins immediately at the incubation stage [7,8,9] and can take up about 90% of the fatigue lifetime [8,9,10]. In this context, monitoring material degradation in the early stages of fatigue seems to be an exceedingly common engineering task. Ultrasonic nondestructive evaluation techniques have the ability to define the amount of damage through the monitoring of microstructure-sensitive parameters [10,11,12]. Due to their sensitivity to microstructures, ultrasonic techniques can be used to control the fatigue behavior of welded joints [13].

Surface and internal microstructural damage must be monitored separately, as they can accumulate at different rates.

For internal damage evaluation, it is advisable to use an ultrasonic method based on bulk wave propagation analysis [14,15,16,17]. The most promising techniques are those based on acoustic birefringence measurements and the elastic modulus ratio. These techniques have an important advantage: there is no need to measure the thickness of the material since relative values are determined by measuring the ultrasonic waves’ propagation times through the material thickness.

To determine the characteristics of the microstructure in the near-surface layer and to evaluate stresses, LCR waves are often used [18,19,20]. Waves of this type propagate in the subsurface layer at a velocity close to the velocity of a longitudinal wave. LCR waves (also called lateral or creeping waves in NDT) radiate a bulk shear wave into the material at an angle of under 33°, approximately, which leads to a strong attenuation [21,22,23]. Head waves are widely used in TOFD [24,25].

They are not sensitive to the state of the surface, and the depth of penetration can be changed using different wave frequencies. There are two primary aims of this study. The first aim is to investigate the effect of fatigue on the bulk and LCR waves’ velocities in the heat-affected zone and the base metal of the low-carbon steel welded joint. The second aim is to investigate changes in nondimensional parameters, defined as the ratio of velocities or time propagation, to determine the most sensitive of them to the evolution of the microstructure during fatigue. Understanding how acoustic parameters change in the different zones of a welded joint under fatigue can be utilized in structural health-monitoring applications based on ultrasonic techniques.

2. Theoretical Background

2.1. Bulk Waves

The bulk elastic wave velocities propagating through a polycrystalline material are related to its elastic properties and can be determined by the Christoffel eigenvalues equation [26]:

$$\left(〈C_{ijkl}〉{\widehat{n}}_j{\widehat{n}}_k-\rho v^2{\delta }_{il}\right)u_i=0,$$

(1)

where $\widehat{n}$ is the unit vector normal to the wavefront, indicating, therefore, the direction of propagation of the wave, ρ is the density of the medium, v is the phase velocity, $〈C_{ijkl}〉$ represents the second-order elastic constants, u is the displacement or polarization vector, and δ_il is the Kronecker delta.

Equation (1) corresponds to three homogeneous equations, the solution of which results in three different velocity values for each considered wave propagation direction by taking the determinant of the coefficient matrix equal to zero. These values correspond to the phase velocities of three ultrasonic waves with mutually perpendicular polarization vectors. The following relations are obtained for an anisotropic material:

$$C_{33}=\rho V_{33}^2, C_{44}=\rho V_{32}^2, C_{55}=\rho V_{31}^2,$$

(2)

where $V_{33}$ is the longitudinal wave velocity and $V_{32}$ and $V_{31}$ are the shear wave velocities; the first index shows the wave propagation direction, and the second index indicates the direction of polarization.

The difference between the elastic constants C₄₄ and C₅₅ gives rise to the acoustic birefringence:

$$B=2\frac{t_{32}-t_{31}}{t_{31}+t_{32}}=2\frac{V_{31}-V_{32}}{V_{32}+V_{31}},$$

(3)

where t₃₁ and t₃₂ are the time propagations of shear waves (the first index corresponds to the direction of propagation, and the second index corresponds to the direction of polarization).

Previous studies have shown the effectiveness of using the modulus ratios C₃₃/C₄₄ and C₃₃/C₅₅ in assessing steel under fatigue [27]. They follow from Equation (2):

$$\frac{C_{33}}{C_{44}}=\frac{V_{33}^2}{V_{32}^2}=\frac{t_{32}^2}{t_{33}^2} , \frac{C_{33}}{C_{55}}=\frac{V_{33}^2}{V_{31}^2}=\frac{t_{31}^2}{t_{33}^2},$$

(4)

where t₃₃ is the time propagation of a longitudinal wave.

2.2. Longitudinal Critically Refracted Wave

The longitudinal critically refracted technique, as described by Bray [28], releases a longitudinal wave traveling just beneath the surface. To generate such waves, the slope of an incident longitudinal wave hitting the surface of the material should be close to the first critical angle. We can calculate the angle of incidence using Snell’s law. The first critical angle is given by

$$\frac{V_1}{sin\alpha }=\frac{V_2}{sin\beta },$$

(5)

where V₁ is the longitudinal velocity in the wedge material (generally polymethyl methacrylate or polystyrene), V₂ is the longitudinal velocity in the steel, α is the first critical angle, and β = 90° for an LCR wave.

The surface layer depth, δ, in which the LCR wave propagates is approximately equal to one wavelength, λ [29]:

$$\delta \approx \lambda =\frac{V}{f},$$

(6)

where V is the wave velocity (5900 m/s) and f is the frequency.

The dependence of LCR waves’ penetration depth into the subsurface layer on frequency was studied experimentally [19]. A correlation model of the LCR wave propagation depth and frequency was also obtained [30]. The results of these studies are in good agreement with each other, as shown in Figure 1. Taking into account the results of these two works, we decided to calculate the LCR wave penetration depth using the following formula:

$$\delta =\frac{k}{f},$$

(7)

where k = 5000 m/s is the coefficient. The dependence of the LCR waves’ penetration depth on frequency, calculated according to Formula (7), is shown in Figure 1.

Similar to the shear waves’ acoustic birefringence (3), we can propose the parameter D for the velocities of LCR ($V_{11}^{LCR}$) and longitudinal waves using the following formula:

$$D=2\frac{V_{11}^{LCR}-V_{33}}{V_{11}^{LCR}+V_{33}}.$$

(8)

This parameter can characterize acoustic anisotropy in the X(1)Z(3) plane, provided that the LCR wave frequency is selected corresponding to the propagation depth equal to the sample along the thickness of axis 3.

3. Materials and Methods

3.1. Material and Specimens

Hot-rolled, low-carbon steel for load-bearing elements of welded and nonwelded structures and parts (analog of ASTM 1020 steel) was chosen for this study. Such steel is widely used for various industrial applications. Its actual chemical composition, determined with a Spectral Laboratory MCA II V5 optical emission spectrometer, is given in

Table 1. Chemical composition of investigated steel (weight %).

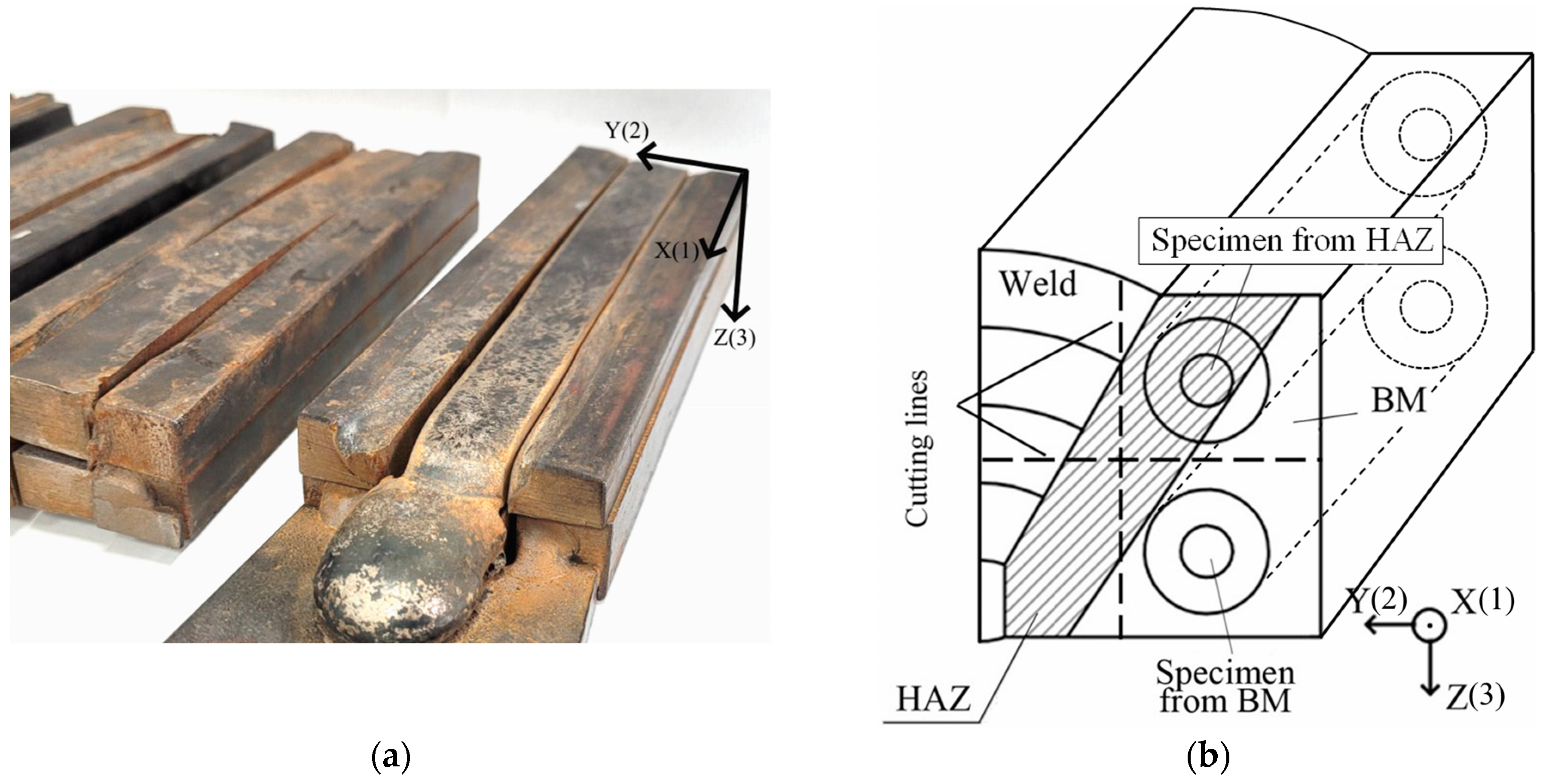
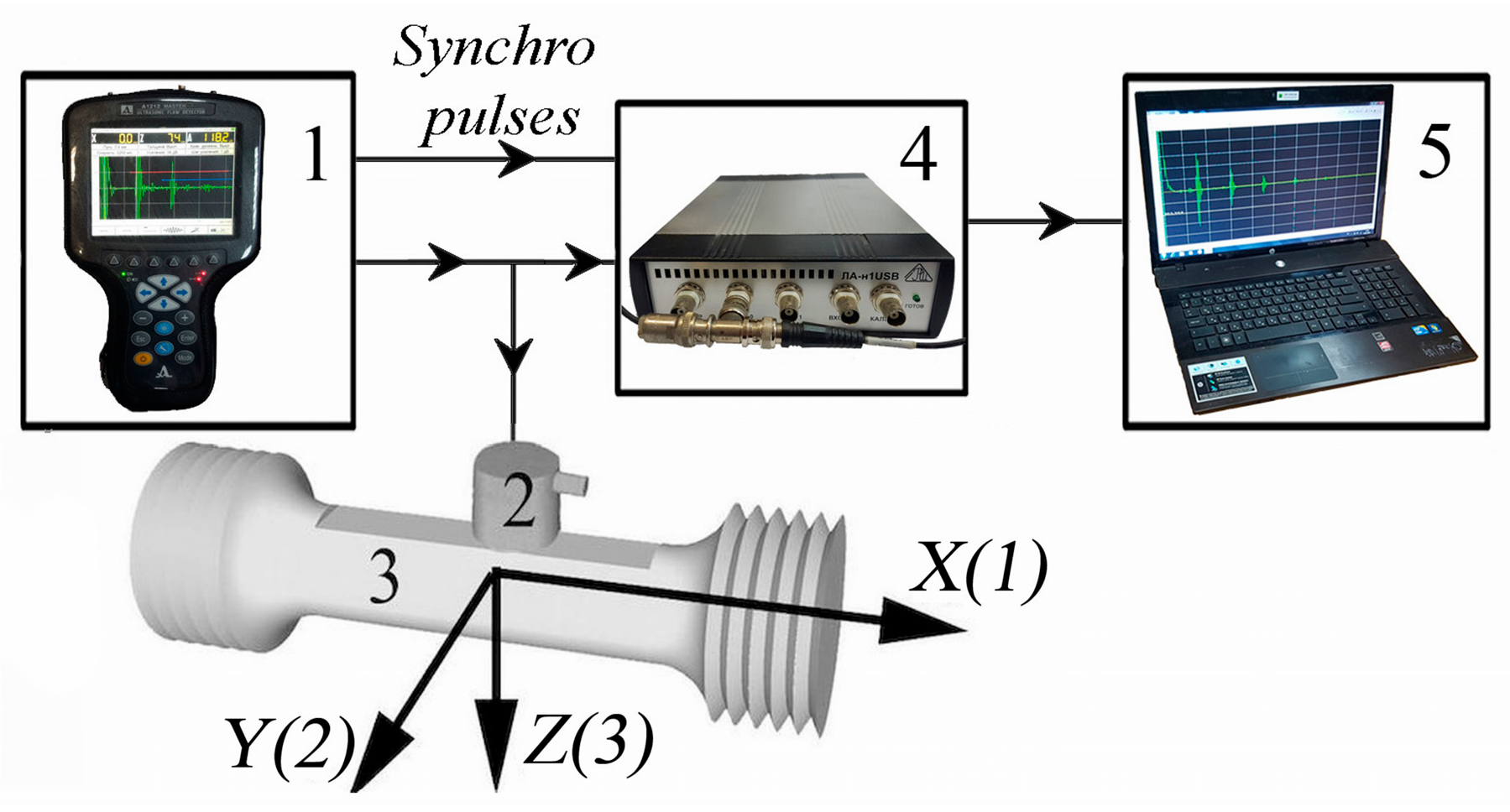
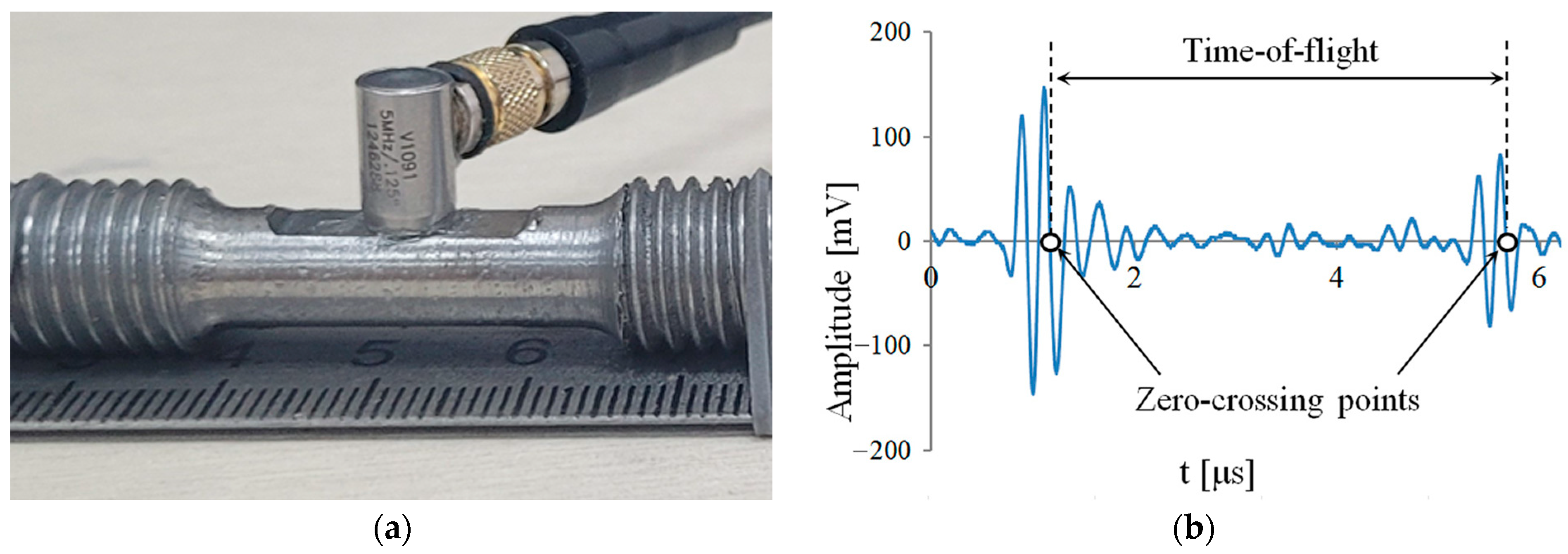
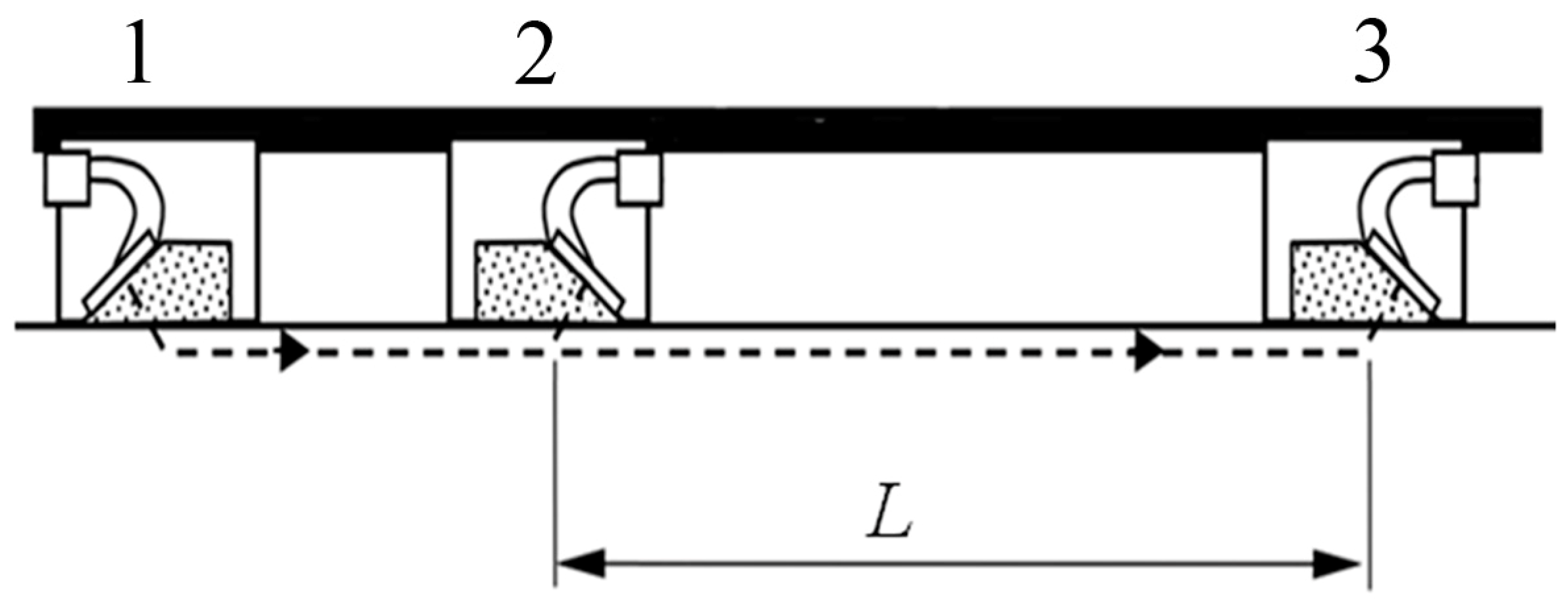
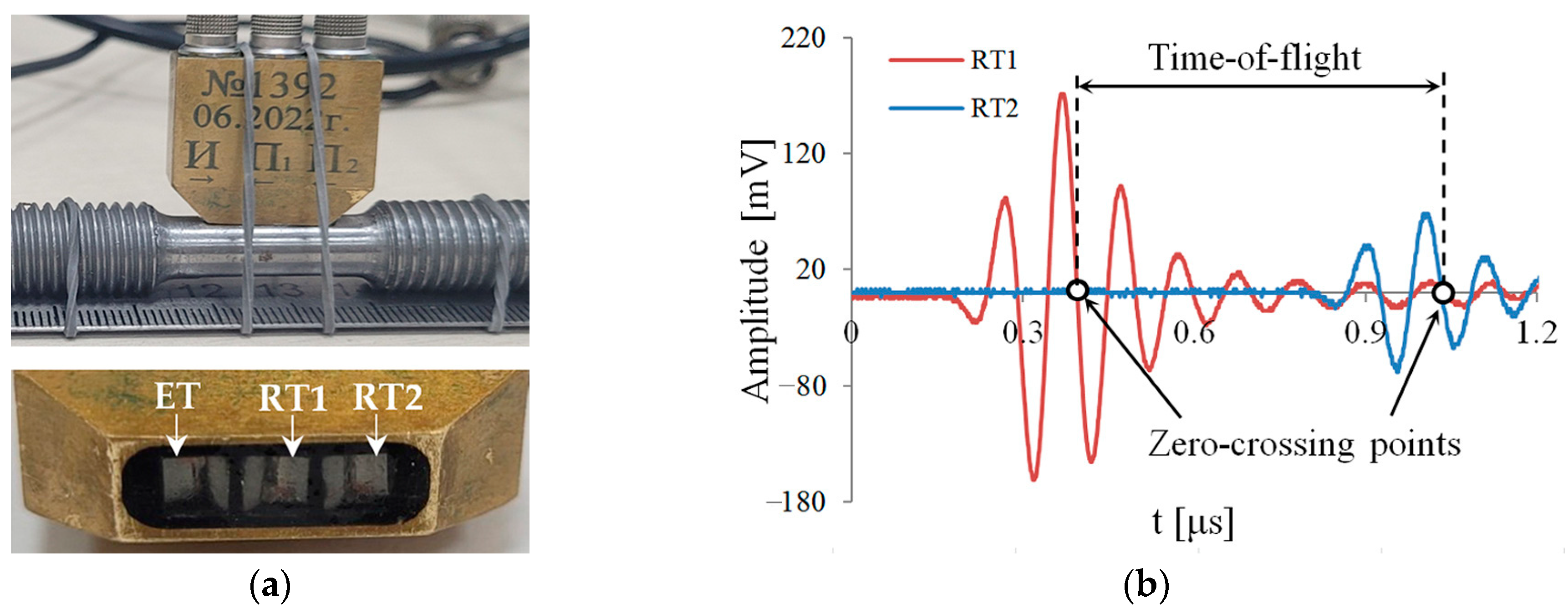
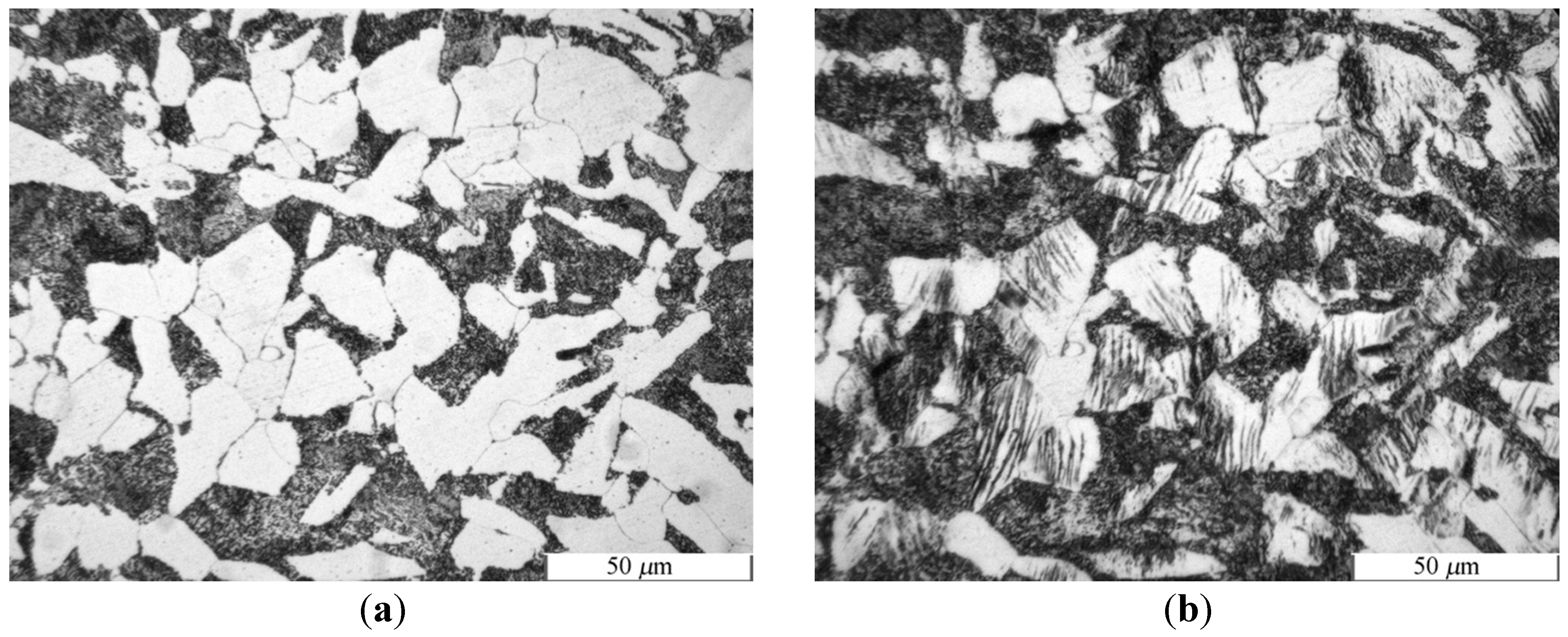
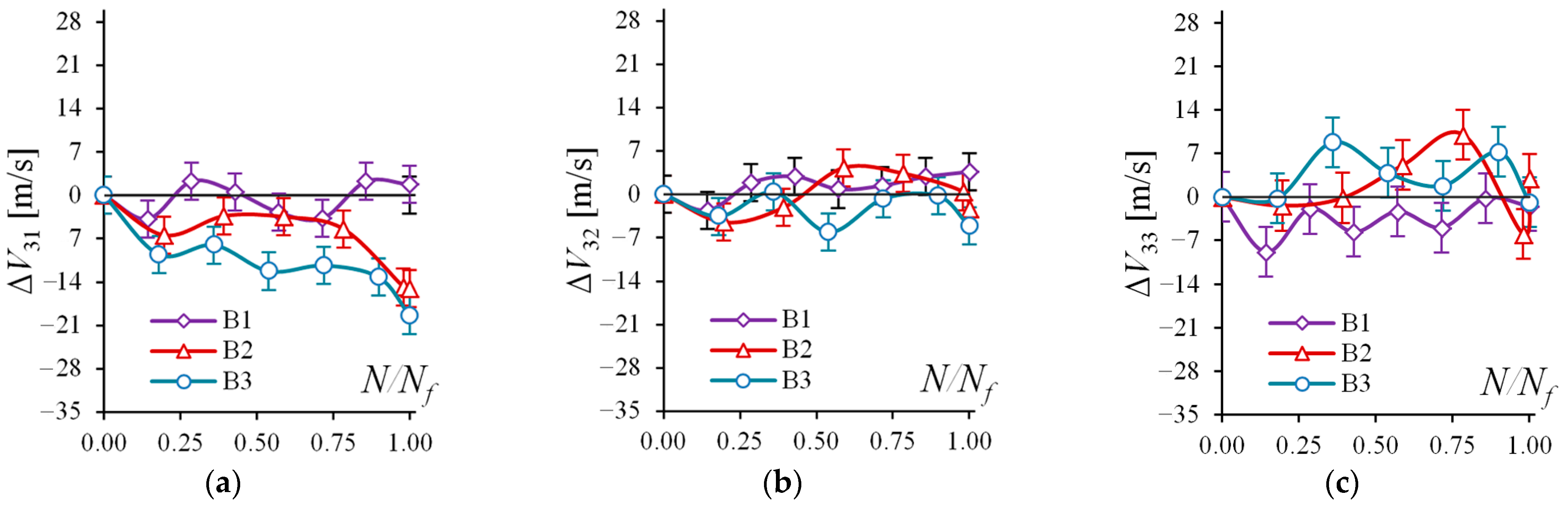
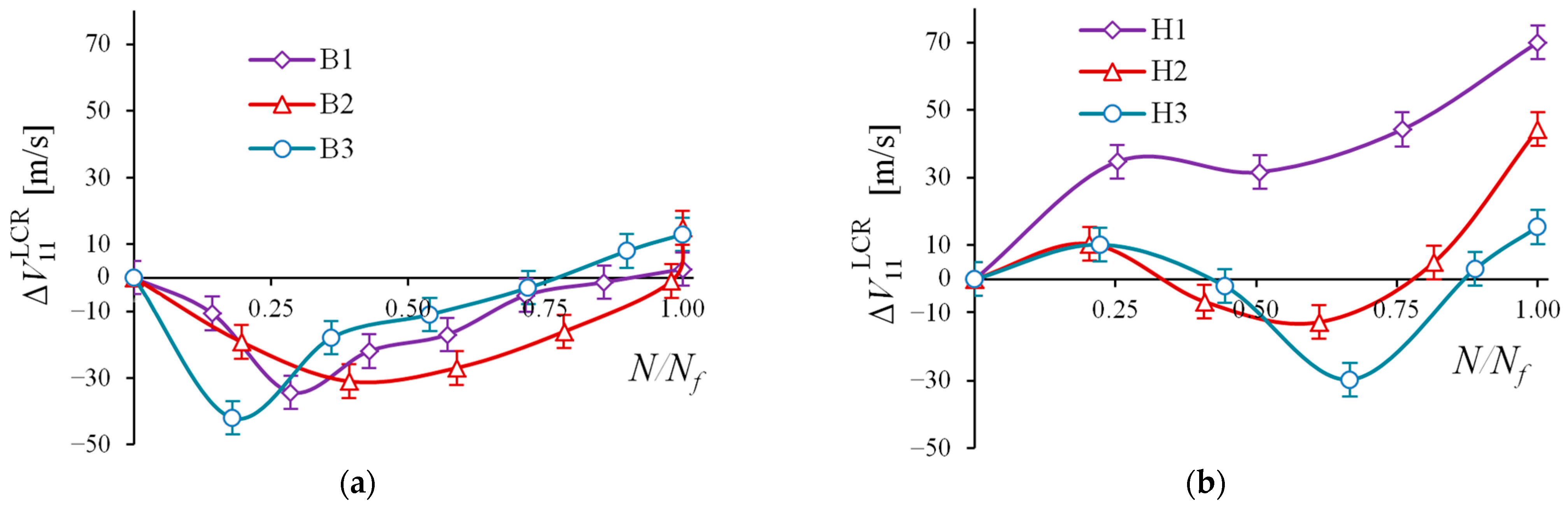
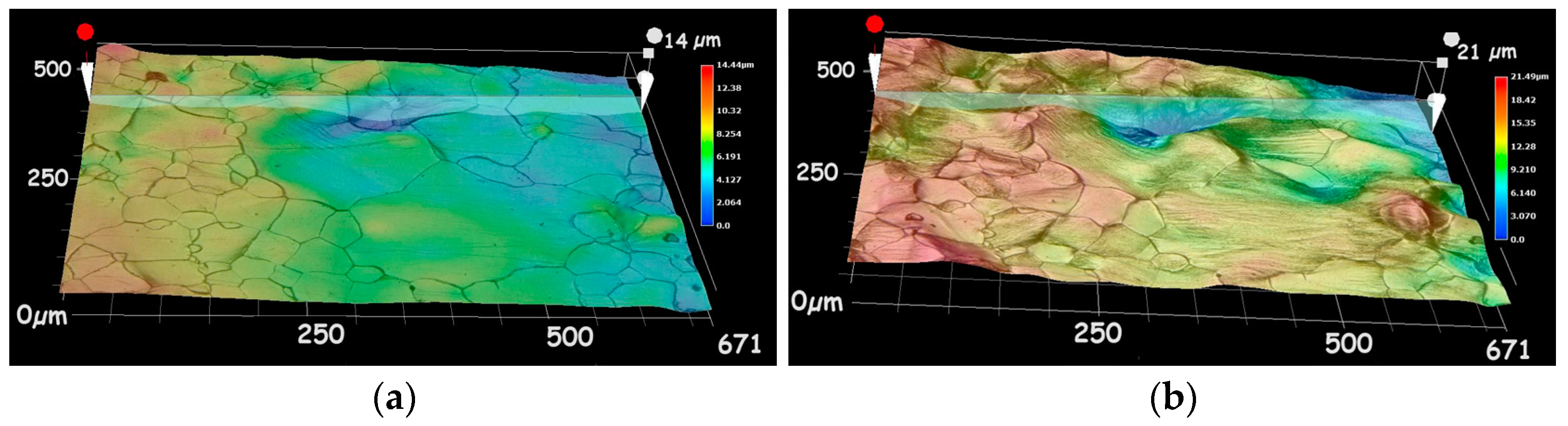
Fe	C	Si	Mn	Ni	Cr	Cu	As	S	P
>98.7	0.18	0.21	0.56	0.08	0.11	0.08	0.04	<0.01	<0.02

.

Table 2. Mechanical properties of investigated steel as received.

Yield Tensile Strength, MPa	Ultimate Tensile Strength, MPa	Elongation at Break, %
270 ± 10	450 ± 10	28 ± 2

shows the mechanical properties determined according to the ASTM E8 standard [31].

Two hot-rolled steel workpieces with a square cross-section of 30 × 30 mm² (Figure 2a) were welded by manual arc welding on argon shielding gas in several passes. A specific welding mode was selected to ensure the heat-affected zone had a width of about 15 mm. Test specimens were made from the base metal (BM) and from the heat-affected zone (HAZ) of a welded joint (Figure 2b). Blanks were cut parallel to the weld through hydro-abrasive erosion. Next, we made round-cross-section specimens from blanks by lathing them with a special cooling liquid. Generally, the final specimens were not thermally affected.

Figure 3a shows the geometric dimensions (in mm) of the test specimens. Two plane-parallel platforms 10 × 3 mm² in size were cut out to enable ultrasonic measurements.

3.2. Low-Cycle Fatigue Tests

The specimens were subjected to cyclic tension–compression loading in strain-control mode using a BISS Nano UT-01-0025 servo-hydraulic testing machine (BISS, Bangalore, India) at a frequency of 1 Hz. Fatigue tests were carried out at a temperature of +22 °C. The strain ratio was equal to −1. Each specimen was loaded at a specific total strain amplitude, Δε, in accordance with the ASTM E1823 standard [32] until the formation of a 1 mm crack occurred, as visible to the naked eye. The stress amplitude, Δσ, and the number of cycles to failure, N_f, were determined.

Table 3. Mechanical test data.

Test No.	Type	Δε, %	Δσ, MPa	Nf
B1	Base Metal	0.3	306	10,500
B2		0.4	347	5100
B3		0.5	379	2780
H1	Heat-affected zone	0.3	308	5900
H2		0.4	314	4900
H3		0.5	348	2250

shows the mechanical test data.

According to Table 3, specimens cut from the HAZ were destroyed, on average, 1.5 times faster compared to the specimens from the base metal.

The strain was controlled with a BISS AC-07-1005 extensometer (BISS, Bangalore, India) (Figure 3b). The applied stress was automatically calculated by the machine software as the ratio of the applied force to the cross-sectional area. The specimens were tested in stages. For each stage, after interrupting the test and unloading the specimen, ultrasonic measurements were carried out. The stage was 1500 cycles for a strain amplitude of 0.3%, 1000 cycles for a strain amplitude of 0.4, and 500 cycles for a strain amplitude of 0.5%.

For each tested specimen (for base metal and heat-affected zone), stress–strain hysteresis loops were automatically recorded for all loading cycles. There was no significant evolution of the hysteresis loops during fatigue. No redistribution between the elastic and plastic components of the strain was observed. As an example, Figure 4 shows the mid-life stress–strain hysteresis loops for the HAZ.

The results of the fatigue tests showed that the investigated steel actually underwent neither cyclic hardening nor cyclic softening. For example, the stress amplitude remained almost constant for the HAZ under fatigue, as shown in Figure 4b.

3.3. Ultrasonic Investigations

Ultrasonic measurements were carried out on the specimens in the unloaded state before the fatigue test and after each test stage in laboratory conditions at a temperature of +22 °C. The study was carried out with longitudinal, shear, and LCR waves.

The ultrasonic data were obtained with a specially designed setup, as schematically shown in Figure 5. An ACS A1212 MASTER ultrasonic flaw detector (ACS Group, Saarbrücken, Germany) was used to generate the electrical pulses. An LA-n1USB digital oscilloscope (LLC “Rudnev-Shilyaev”, Moscow, Russia) and ADCLabSE (v.2.1.21344.2042) software were used to record an amplitude–time diagram of the pulses from the piezoelectric transducer on a PC. The sampling frequency was 1 GHz, and the time resolution was 1 ns.

The times of flight of one longitudinal, t₃₃, and two shear (polarization in the mutually perpendicular directions along t₃₁ and across t₃₂, the loading axis) waves propagating through the thickness of the specimen between two plane-parallel platforms were measured using the echo method.

Olympus V1091 and V157 (Evident, Tokyo, Japan) wideband piezoelectric transducers were attached to the plane-parallel platform in the gauge section of the specimen to excite and receive longitudinal and shear waves, respectively. Figure 6a shows a method of installing a bulk wave transducer on a specimen. These transducers have a central frequency of 5 MHz and element sizes of 3.2 mm. Times of flight were measured between the first and second echo pulses at the zero-crossing points. A shear wave diagram is shown in Figure 6b.

Water-based ultrasound gel was used to ensure the acoustic contact of the longitudinal wave transducer, and epoxy resin was used for the shear wave transducer.

The times of flight of the LCR waves propagating along the specimen were determined through a differential measurement scheme (Figure 7).

This scheme consists of an emission transducer (ET), a first receiving transducer (RT1), and a second receiving transducer (RT2). The transducers are piezoceramic plates glued to a wedge.

An experimental LCR transducer was manufactured, consisting of three transducers in one casing, in accordance with the scheme (Figure 8a). The piezoelectric plates’ frequency was 10 MHz, and the wedges were made of polymethyl methacrylate. Since the longitudinal wave velocity in polymethyl methacrylate is 2700 m/s, according to Formula (7), the wedge angle (first critical angle) is 27°. The emission and receiving of LCR waves were carried out at the specimens’ surfaces through the contact method using ultrasound gel. The differential measurement scheme made it possible to compensate for the influence of the thermal expansion of the wedge and significantly reduce the layer contact fluid influence and delay lines in the cables [33,34]. The calibration of the LCR transducer was carried out on samples with a known longitudinal wave propagation velocity. From the calibration results and taking into account the choice of in-phase points, the measuring base, L, between the two receiving transducers was 3.760 ± 0.005 mm. In accordance with Formula (7), the propagation depth of the LCR waves at 10 MHz was 0.5 mm.

The times of flight of the LCR waves, $t_{11}^{LCR},$ were measured between the signals from the first and second receiving transducers. The zero-crossing points at the transition from the minimum to the maximum in the signal were taken as in-phase points in the pulses (Figure 8b).

At each stage, the ultrasonic measurements were repeated ten times and then averaged. High accuracy was ensured by maintaining stable contact and exact positioning of the transducer. The absolute error in measuring the time of flight of shear, longitudinal, and LCR waves was no more than 1 ns.

The velocities of longitudinal, V₃₃, and shear waves, polarized along V₃₁ and across the V₃₂ loading axis, are calculated using the following formulas:

$$V_{33}=\frac{2h}{t_{33}}, V_{31}=\frac{2h}{t_{31}}, V_{32}=\frac{2h}{t_{32}}.$$

(9)

where h is the thickness of the specimens (measured using a micrometer).

The LCR wave propagation velocity was calculated as follows:

$$V_{11}^{LCR}=\frac{L}{t_{11}^{LCR}}$$

(10)

The main contribution to the velocity error comes from measuring the distance traveled by the elastic wave. The absolute error in the shear waves’ velocity was 3 m/s, and that of the longitudinal and LCR waves was 5 m/s.

The acoustic birefringence, the module ratios, and the parameter D were determined using Formulas (3), (4) and (8), respectively. The absolute error in determining the acoustic birefringence did not exceed 4 × 10⁻⁴; in determining the module ratios, it did not exceed 2 × 10⁻⁴; and in determining parameter D, it did not exceed 1 × 10⁻³.

The changes in the velocities, ΔV, acoustic birefringence, ΔB, parameter, ΔD, and the module ratios, Δ(C₃₃/C₅₅) and Δ(C₃₃/C₄₄), were calculated as the difference between the subsequent value and the initial one.

3.4. Metallographic Investigations

For each tested specimen (for the base metal and heat-affected zone), microstructure images were obtained. The surface was first mechanically polished and then chemically etched within 10 s using a 5% nitric acid water solution to reveal the grain boundaries. The microstructure was observed using a ZEISS Axio Observer optical microscope (ZEISS AG, Oberkochen, Germany). The microstructures of the base material and the heat-affected zone before and after testing are shown in Figure 9 and Figure 10.

The structure of the HAZ differs from that of the BM and corresponds to the welded joint’s overheating area. The grain size of the base metal is 27 µm, and that of the heat-affected zone is 52 µm. It follows from observations that persistent slip bands (PSBs) appear in the ferrite grains already in the first stage of the fatigue test. PSBs have a prevalent orientation across the axis of loading.

A more detailed study of changes in surface topography was carried out using a Keyence VHX 1000 universal digital microscope (KEYENCE CORPORATION OF AMERICA, Itasca, IL, USA). The results are presented in Section 5.

4. Results

The velocities of the longitudinal and shear waves, calculated by Formula (9), and the LCR wave velocities, calculated by Formula (10), in accordance with the number of cycles, N, are presented in

Table 4. Ultrasonic waves’ velocities.

Test No.	N, Cycles	${\mathit{V}}_{31}$, m/s	${\mathit{V}}_{32}$, m/s	${\mathit{V}}_{33}$, m/s	${\mathit{V}}_{11}^{\mathit{L}\mathit{C}\mathit{R}}$, m/s
B1	0	3272	3268	5984	5941
	1500	3268	3266	5975	5931
	3000	3274	3270	5982	5907
	4500	3272	3271	5978	5919
	6000	3269	3269	5982	5924
	7500	3268	3270	5979	5936
	9000	3274	3271	5984	5940
	10,500	3273	3272	5983	5944
B2	0	3279	3277	6001	5930
	1000	3273	3273	6000	5911
	2000	3276	3275	6001	5902
	3000	3276	3281	6006	5903
	4000	3274	3281	6011	5914
	5000	3265	3278	5995	5929
	5100	3264	3275	6004	5945
B3	0	3275	3272	5978	5939
	500	3265	3268	5978	5897
	1000	3267	3272	5987	5921
	1500	3262	3265	5982	5928
	2000	3263	3271	5980	5936
	2500	3261	3271	5986	5947
	2780	3255	3266	5978	5952
H1	0	3259	3274	5984	5869
	1500	3252	3275	5990	5904
	3000	3252	3277	5997	5901
	4500	3249	3276	5998	5914
	5900	3238	3278	6009	5992
H2	0	3258	3270	5990	5853
	1000	3253	3263	5994	5863
	2000	3248	3262	5999	5846
	3000	3245	3268	6005	5840
	4000	3244	3265	6016	5858
	4900	3240	3265	6010	5897
H3	0	3255	3271	5972	5874
	500	3254	3277	5974	5884
	1000	3241	3269	5977	5872
	1500	3236	3271	5985	5832
	2000	3226	3263	5982	5877
	2250	3224	3268	5987	5889

.

The shear wave velocities, V₃₁, V₃₂, and V₃₃ for tests B1, B2, and B3, do not change (Figure 11). For tests B2 and B3, a monotonic decrease in the velocity of the shear waves, V₃₁, is observed. The intensity of the V₃₁ velocity change increases with increasing amplitude deformation.

Compared to the BM, the shear and longitudinal waves’ velocities in the HAZ change more intensively (Figure 12).

A monotonous velocity decrease in V₃₁ is observed in the HAZ (Figure 12a). The velocity of V₃₂ for the H1 test remains almost unchanged (Figure 12b). A monotonous velocity decrease in V₃₂ is observed for tests H2 and H3. The velocity of the longitudinal waves V₃₃ increases monotonically (Figure 12c).

The changes in the velocities of the LCR waves, $V_{11}^{LCR},$ in the BM can be divided into two stages: first, there is a decrease; then, after N/N_f = 0.2–0.3, there is an increase (Figure 13a).

A gradual change in the LCR wave velocity, $V_{11}^{LCR},$ is observed for the HAZ: a slight increase in the initial stage (N/N_f = 0.25), then a decrease (N/N_f = 0.6–0.7), and then rapid growth, as shown in Figure 13b.

Thus, the shear wave velocities V₃₁ and the velocities $V_{11}^{LCR}$ for both the BM and the HAZ turned out to be the most sensitive to fatigue.

5. Discussion

Metallographic studies indicate the active formation of PSBs (Figure 3), especially in the HAZ (Figure 4), which are a consequence of the movement of active dislocations. We carried out more detailed studies in our work [35]. An increase in dislocation density reduces the velocity of bulk ultrasonic waves [36].

The PSBs detected on the surface were predominantly oriented across the loading axis. The intrusion–extrusion relief observed on the surface displays the movement of dislocations in certain crystallographic directions in the volume of the material, as shown in Figure 14. Accordingly, defects (mesodefects, dislocations, etc.) formed inside the material have a prevalent orientation and affect the shear waves’ velocity polarized along the loading axis [37]. We suppose that the formation of such oriented defects leads to a monotonic decrease in the shear wave velocity polarized along the loading axis (Figure 11a and Figure 12a).

There was no change in the velocity of the longitudinal waves, V₃₃, in the BM. For the HAZ, a linear increase in the velocity of the longitudinal waves is observed, which may be due to the same influence of texture changes.

The decrease in the LCR wave velocities, $V_{11}^{LCR}$ (for BM up to N/N_f = 0.25 and for HAZ from N/N_f = 0.25 to N/N_f = 0.6–0.7), may be associated with PSB formation. Accordingly, these prevalent orientation defects influence the LCR wave velocity in the same way as the shear wave velocity becomes polarized along the loading axis.

Another factor that can affect the decrease in the propagation velocities of LCR waves is an increase in the material surface roughness. The intensity of the relief change is related to the magnitude of the strain amplitude. For example, for test H3, the relief changed significantly after N = 1500, and the maximum depression was from 14 µm to 21 µm (Figure 14). An increase in the effective acoustic path with a constant measurement base, L, for the LCR wave sensor leads to a decrease in velocity when calculated according to formula (10).

The subsequent increase in the LCR wave velocity (for the BM from N/N_f = 0.25 to N/N_f = 1 and for the HAZ from N/N_f = 0.6–0.7) could be associated with several factors. One of the factors may be the influence of changes in the metal’s crystallographic texture. Another factor may be the partial transformation of the LCR wave into a longitudinal wave due to structural transformations in the surface layer, the velocity of which is approximately 10% higher [38]. It is known that in the later stages of fatigue, grain fragmentation and grinding can occur. The authors in [39] showed that the velocity of LCR waves increases with decreasing grain size.

For the HAZ, the slight increase in the LCR wave velocities in the initial stage (from N/N_f = 0 to N/N_f = 0.25) may be associated with internal stress relaxation.

To assess the material’s condition, it is advisable to use dimensionless parameters, for the calculation of which there is no need to measure the material thickness. Therefore, dimensionless parameters (except for parameter D) can be determined with one-sided access to the structure and with less error, in contrast to the elastic wave velocities.

The acoustic birefringence, B, calculated by Formula (3), the parameter D, calculated by Formula (8), and the module ratios C₃₃/C₄₄ and C₃₃/C₅₅, calculated by Formula (4), are presented in

Table 5. Dimensionless acoustic parameters.

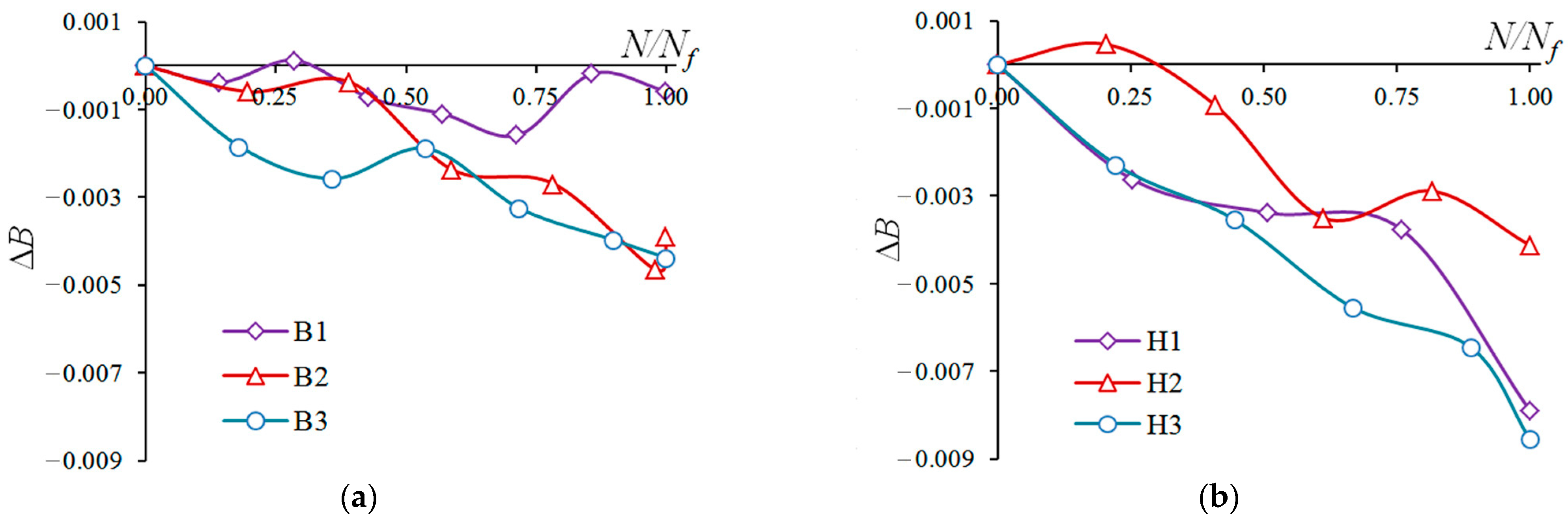
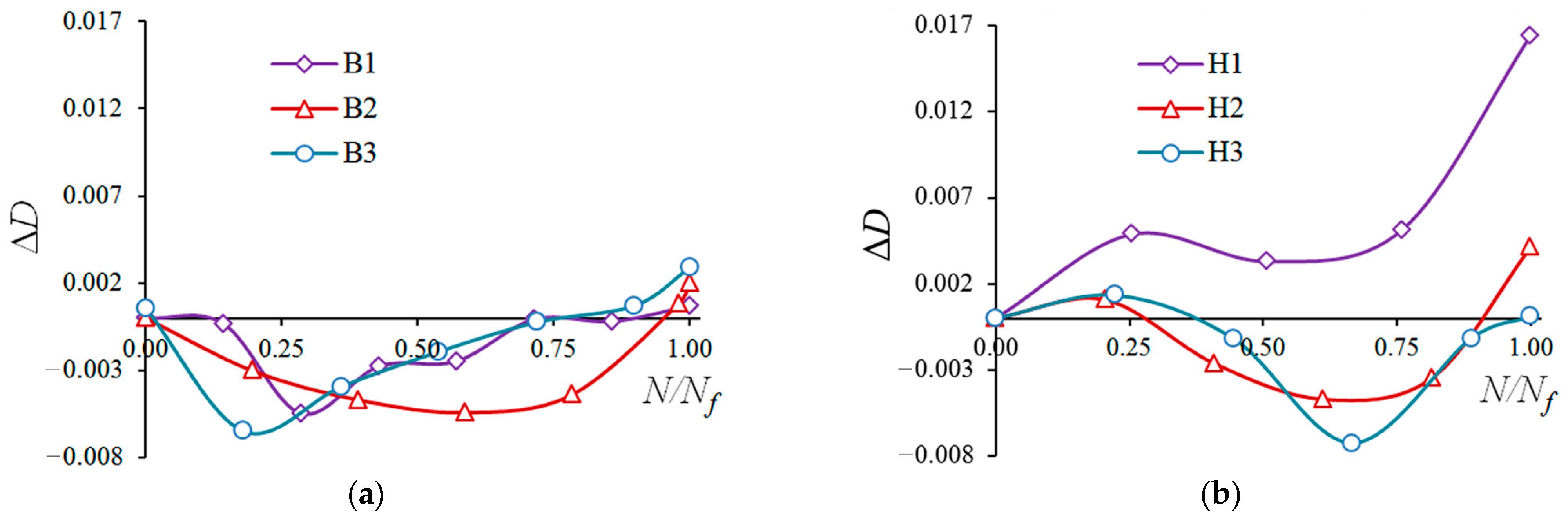
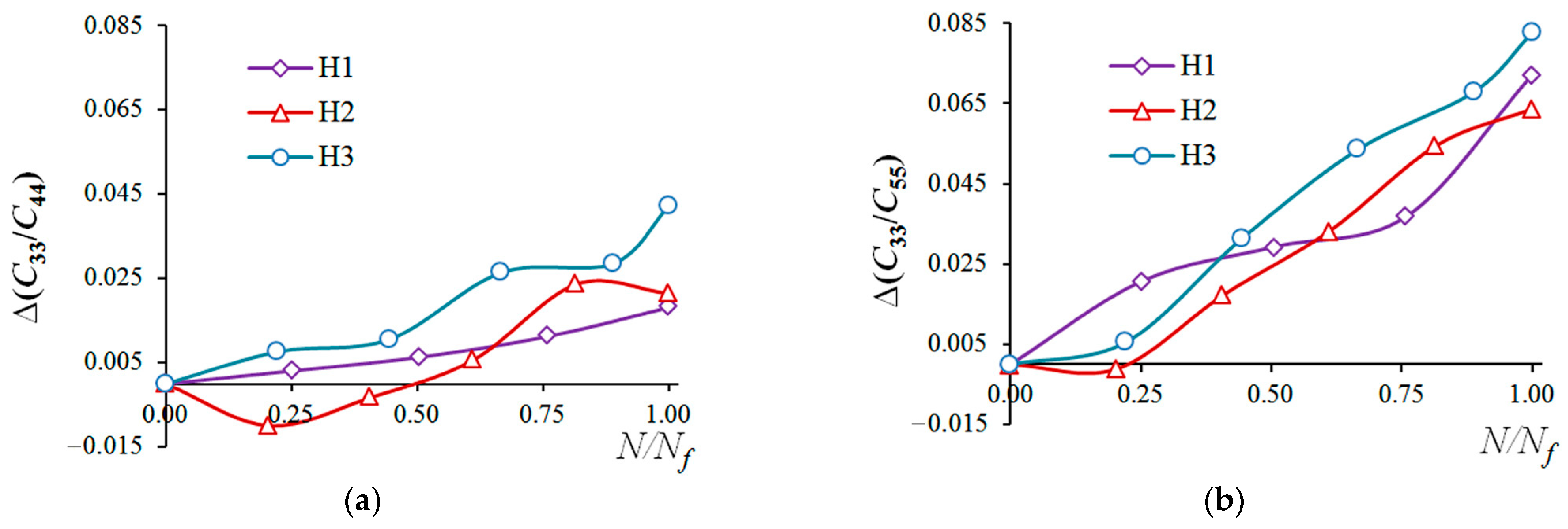
Test No	N, Cycles	B	D	C33/C44	C33/C55
B1	0	0.0011	−0.007	3.353	3.345
	1500	0.0007	−0.008	3.348	3.343
	3000	0.0012	−0.013	3.347	3.339
	4500	0.0004	−0.010	3.340	3.338
	6000	0.0000	−0.010	3.348	3.348
	7500	−0.0005	−0.007	3.344	3.347
	9000	0.0009	−0.007	3.347	3.341
	10,500	0.0005	−0.006	3.344	3.340
B2	0	0.0007	−0.012	3.354	3.349
	1000	0.0001	−0.015	3.361	3.361
	2000	0.0003	−0.017	3.358	3.356
	3000	−0.0017	−0.017	3.350	3.362
	4000	−0.0020	−0.016	3.358	3.371
	5000	−0.0040	−0.011	3.346	3.373
	5100	−0.0032	−0.010	3.362	3.383
B3	0	0.0009	−0.007	3.339	3.333
	500	−0.0009	−0.014	3.346	3.353
	1000	−0.0016	−0.011	3.348	3.360
	1500	−0.0010	−0.009	3.356	3.363
	2000	−0.0023	−0.007	3.343	3.358
	2500	−0.0030	−0.006	3.348	3.369
	2780	−0.0035	−0.004	3.349	3.372
H1	0	−0.0045	−0.019	3.342	3.372
	1500	−0.0071	−0.014	3.345	3.393
	3000	−0.0079	−0.016	3.348	3.401
	4500	−0.0083	−0.014	3.353	3.409
	5900	−0.0124	−0.003	3.360	3.444
H2	0	−0.0036	−0.023	3.362	3.386
	1000	−0.0032	−0.022	3.352	3.385
	2000	−0.0045	−0.026	3.359	3.404
	3000	−0.0071	−0.028	3.367	3.419
	4000	−0.0065	−0.027	3.385	3.441
	4900	−0.0078	−0.019	3.383	3.450
H3	0	−0.0050	−0.017	3.333	3.366
	500	−0.0073	−0.015	3.341	3.372
	1000	−0.0085	−0.017	3.343	3.398
	1500	−0.0105	−0.024	3.359	3.420
	2000	−0.0114	−0.017	3.361	3.434
	2250	−0.0135	−0.016	3.375	3.449

.

The acoustic birefringence changes in a similar way with velocity, V₃₁ (Figure 15). The monotonous decrease in ΔB is due to the formation of predominantly oriented microdefects.

The behavior of the parameter D is due to the change in velocity $V_{11}^{LCR}$ and is associated with the same factors (Figure 16).

A monotonic decrease in ΔB over the fatigue lifetime and a monotonic growth in ΔD after the half-life can be used to determine the material state of a welded joint under fatigue.

The module ratios, C₃₃/C₄₄, for the base metal do not change (Figure 17a). For test B1, the C₃₃/C₅₅ ratio also does not change (Figure 17b). For tests B2 and B3, C₃₃/C₅₅ grows monotonically with Δε.

For the HAZ, Δ(C₃₃/C₄₄) and Δ(C₃₃/C₅₅) grow monotonically, as shown in Figure 18. The module ratios in the HAZ Δ(C₃₃/C₅₅) increase according to a linear law, and the intensity of their change is the greatest here.

For the HAZ, the module ratio Δ(C₃₃/C₅₅) does not depend on the strain amplitude, which can be used to calculate the remaining service life, R, during material fatigue:

$$R=1-\frac{N}{N_f}=1-14.2\mathsf{\Delta }\left(\frac{C_{33}}{C_{55}}\right)$$

(11)

As a result of ultrasonic studies, it was found that the acoustic parameters change most intensively in the specimens cut from the HAZ. This effect can be explained by the large grain size in the heat-affected zone (overheating area) compared to the base material. It is known that, in accordance with the Hall–Petch law [40,41], as the grain increases, the yield strength decreases. The increased plasticity of the HAZ causes more intense movement of dislocations and changes in the microstructure. It is also necessary to take into account that in large grains more extended microdefects are formed.

The proposed method is based on determining dimensionless first-order acoustic parameters. Acoustic birefringence is insensitive to the coupling layer between the sensor and the test object and is not sensitive to the waviness or roughness of a surface. Therefore, there is no need to know the acoustical patch or thickness, as it can be implemented with single-sided access. This increases the reliability of using this method in industrial facilities, in contrast, for example, to nonlinear ultrasonic coefficient methods [42], the main problem of which is a change in the waveform due to many factors.

6. Conclusions

In this work, the influence of fatigue on the acoustic parameters of the base metal and the heat-affected zone of a welded joint made of ASTM 1020 carbon steel was experimentally investigated. According to the mechanical test data, it was found that the specimens from the overheating zone of the welded joint were destroyed approximately 1.5 times faster than the specimens from the base metal.

It was established that the elastic waves’ velocities change most intensively in the heat-affected zone. The linear decrease in the shear wave velocities polarized along the specimen’s loading axis is explained by the formation of linear microdefects predominantly oriented across the loading axis. The nonmonotonic change in the LCR wave velocity is explained by two factors: microdefect formation and the change in the texture of the subsurface layer. It was found that a monotonic decrease in acoustic birefringence occurs more intensely in the heat-affected zone. It was observed that parameter D, calculated through the ratio of the longitudinal and LCR waves’ velocities (similar to the acoustic birefringence of shear waves), changes states. It was found that the ratios of modules C₃₃/C₄₄ and C₃₃/C₅₅ increase most intensively in the heat-affected zone. The linear relationship between the cycle ratio and the change in C₃₃/C₅₅ is proposed to be used to calculate the remaining fatigue lifetime and predict the moment of failure using the acoustic method.