Performance Improvement of Active Suspension System Collaborating with an Active Airfoil Based on a Quarter-Car Model
Abstract
1. Introduction
- Initially, simulations were conducted solely for the airfoil case to examine the aerodynamic effects of an active aerodynamic surface.
- Subsequently, simulations were carried out to assess the aerodynamic impact on the active suspension of the quarter-car model.
- Finally, comparative analyses with other suspension systems were performed to evaluate the performance of the proposed study.
2. Modeling of the Quarter-Car
2.1. Aerodynamic Force
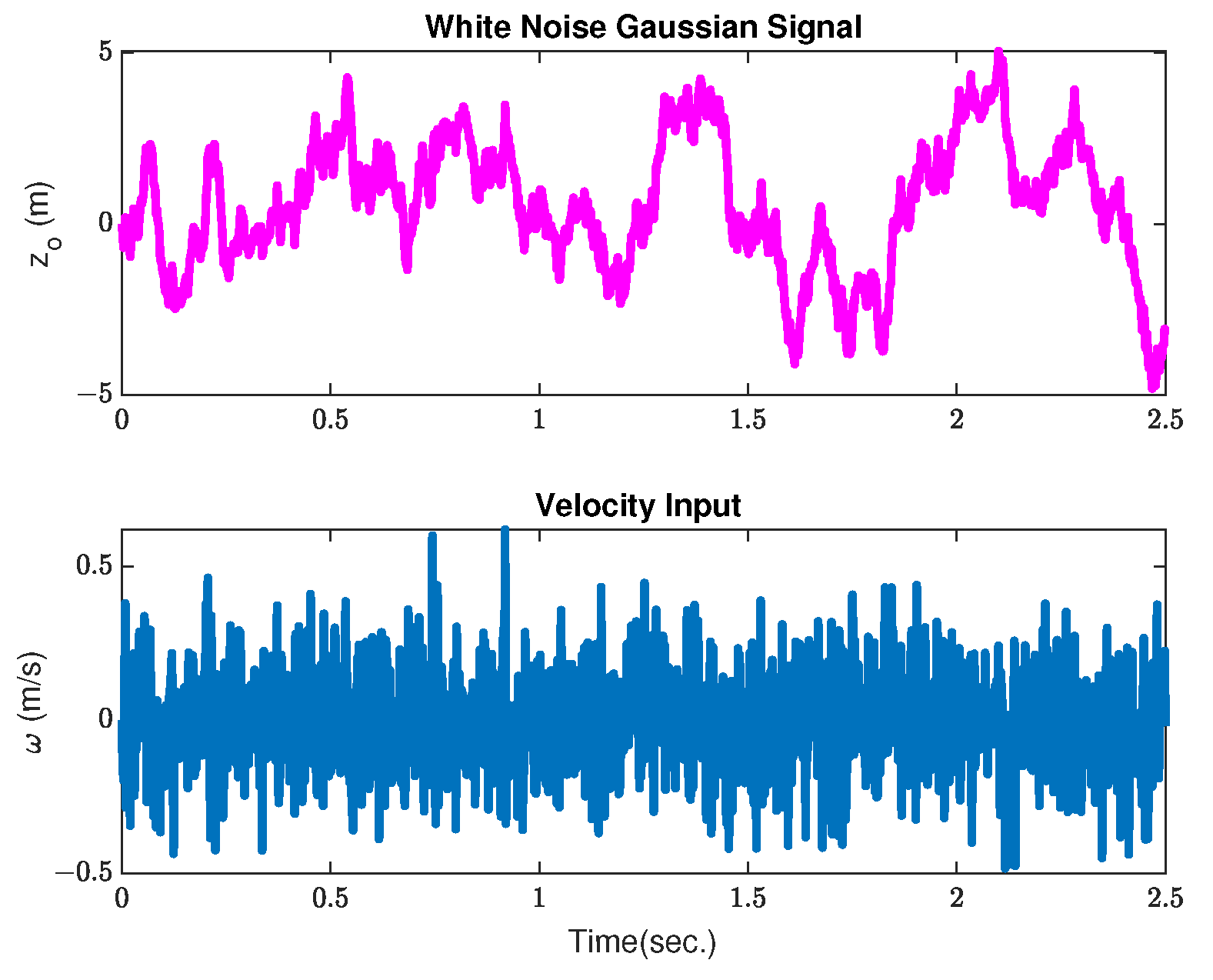
2.2. Road Excitation Model
3. Problem Formulation
- The tire damping coefficient, representing the energy loss due to tire deformation, is considered too small to significantly affect the vehicle dynamics. Thus, it is excluded from the model to simplify the analysis.
- The model assumes that all state variables, such as the vehicle’s absolute velocity, suspension deflection, and dynamic tire load deflection, can be directly measured or detected from the controller output. This eliminates the need for complex estimations or additional sensor data.
- In the simulation, only the vertical lift force produced by the airfoil is taken into account to affect the vehicle’s vertical dynamics. Being small, the horizontal drag force is neglected.
- For simplicity, the model assumes that there are no physical limits on the actuator force and the lift force generated by the active aerodynamic surface. This allows the simulation to focus on and investigate the effects of these forces without considering the complex, constrained dynamics.
4. Optimal Controller Design
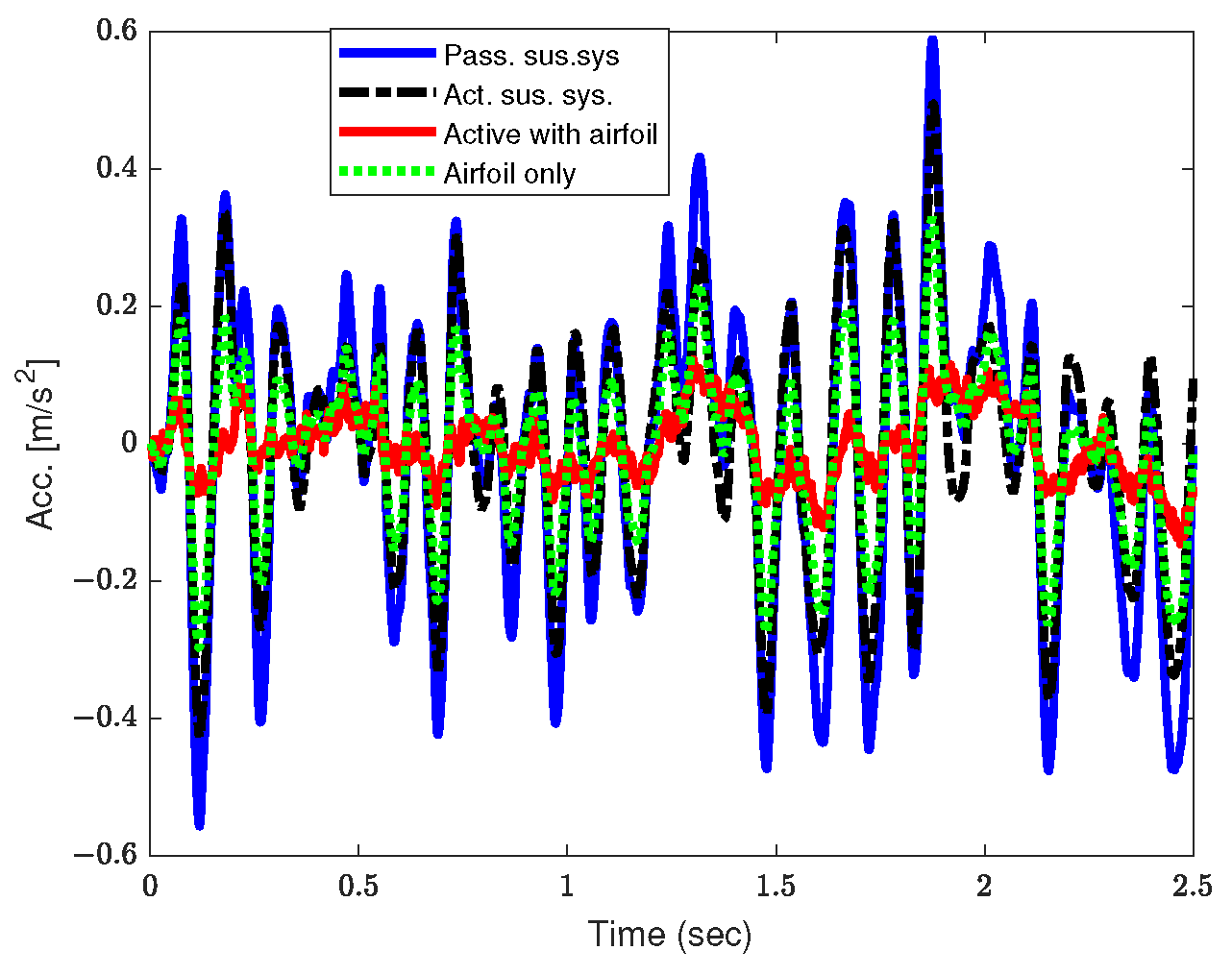
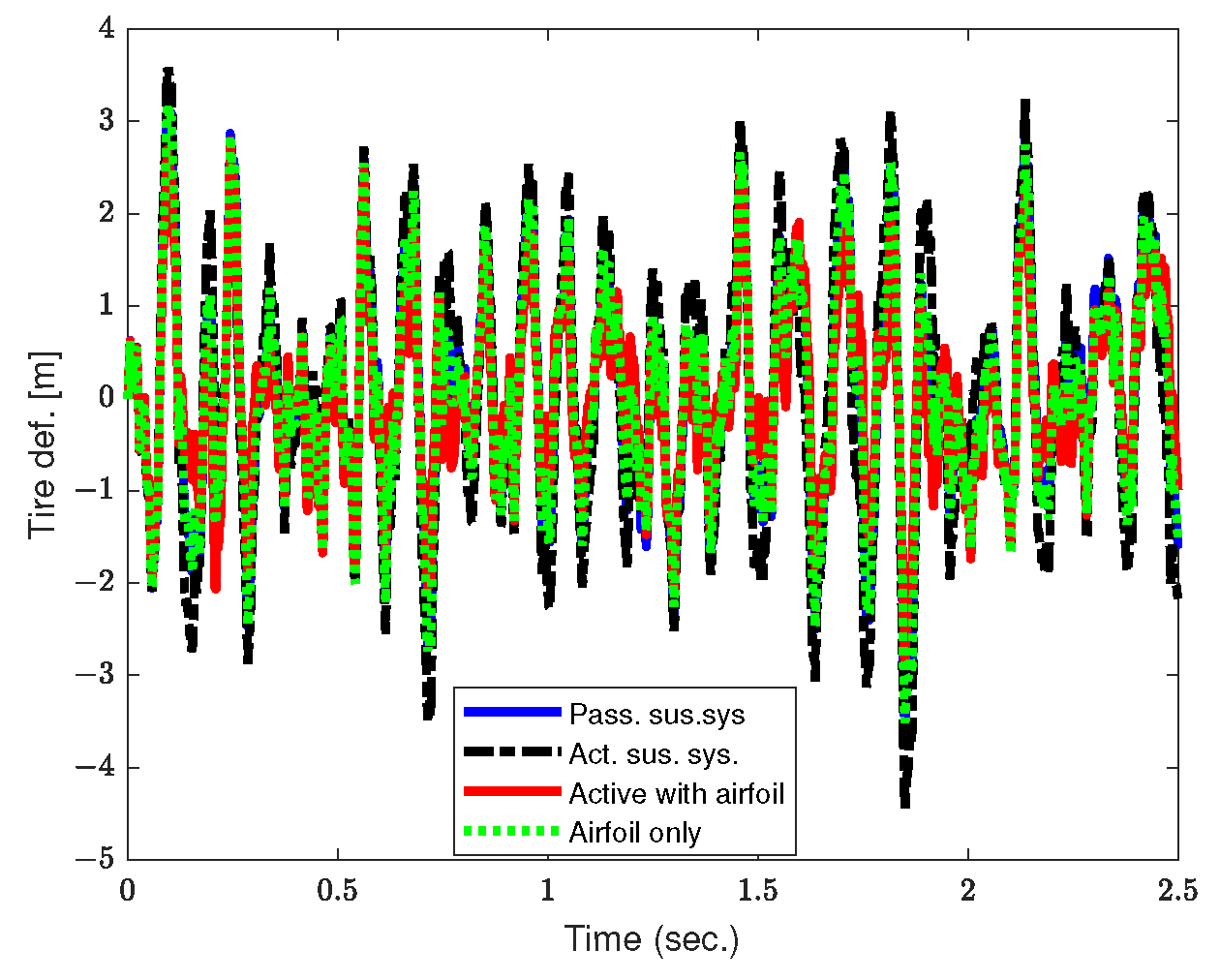
5. Results and Discussion
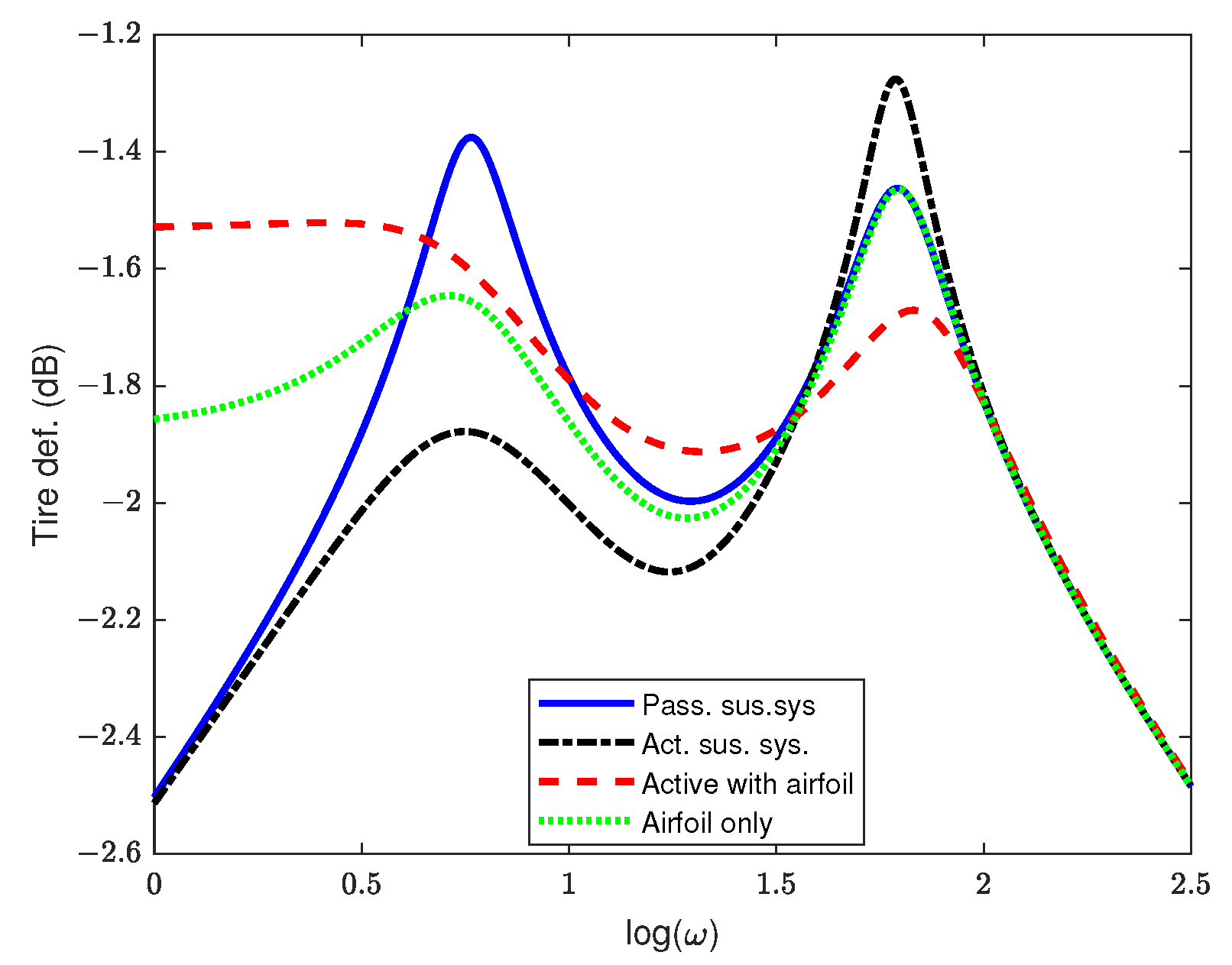
5.1. Frequency Domain Analysis
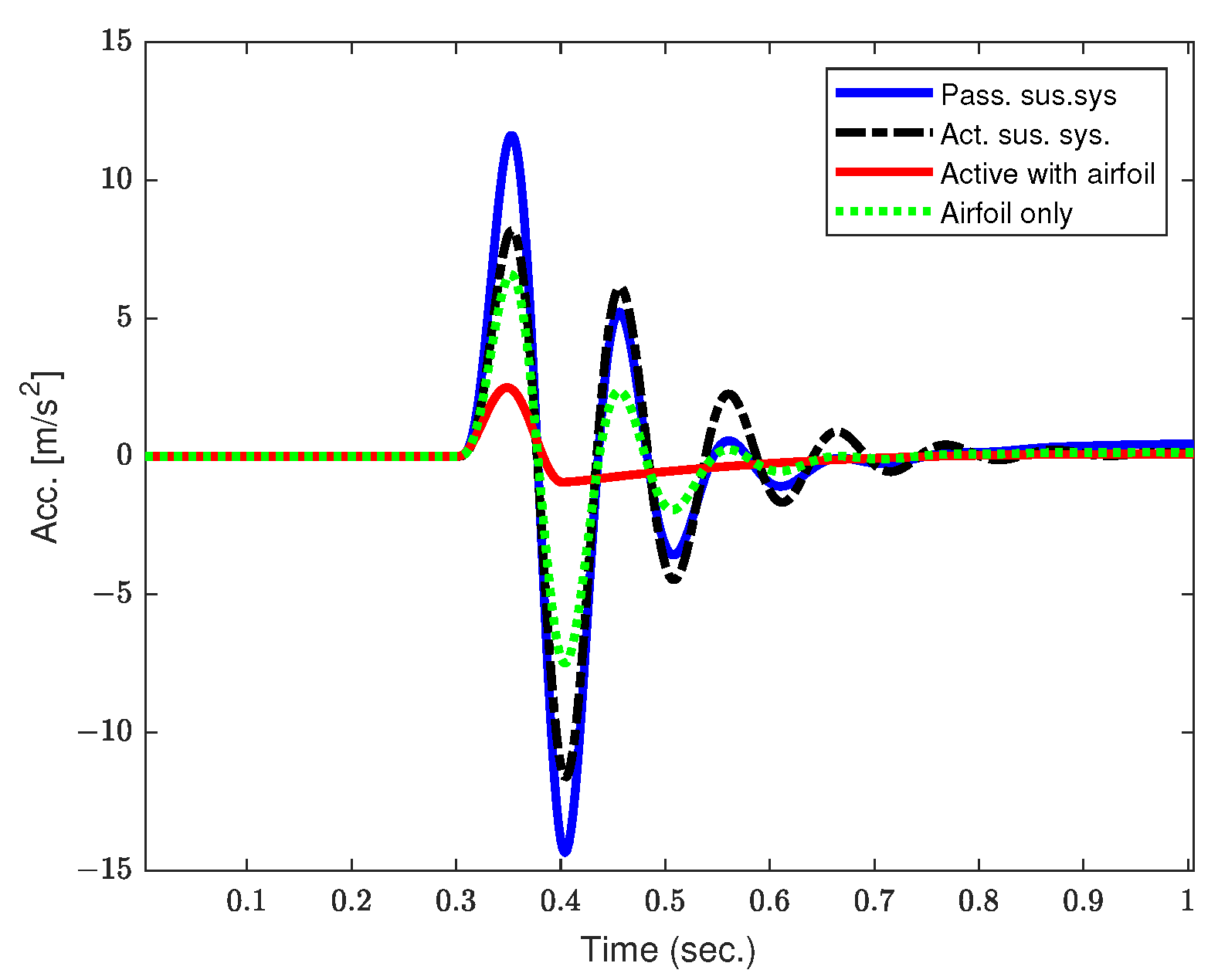
5.2. Time Domain Analysis
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| LQR | Linear Quadratic Regulator |
| AAS | Active Aerodynamic Surface |
| DOF | Degrees of Freedom |
| PSD | Power Spectral Density |
Appendix A
| Suspension System | Weight () | Weight () | Weight () | Weight () |
|---|---|---|---|---|
| Active Sus. System | 0.1 | 0.001 | ||
| Active Sus. with Airfoil | 0.1 | 0.001 | ||
| Airfoil only | 0.1 | 1 |
| Suspension System | Weight () | Weight () | Weight () | Weight () |
|---|---|---|---|---|
| Active Sus. System | 0.1 | 0.001 | ||
| Active Sus. with Airfoil | 0.1 | 0.001 | ||
| Airfoil only | 0.1 | 1 |
References
- He, D.; He, W.; Song, X. Efficient predictive cruise control of autonomous vehicles with improving ride comfort and safety. Meas. Control 2020, 53, 18–28. [Google Scholar] [CrossRef]
- Cvok, I.; Hrgetić, M.; Hoić, M.; Deur, J.; Ivanovic, V. Design of a linear motor-based shaker rig for testing driver’s perceived ride comfort. Mechatronics 2021, 75, 102521. [Google Scholar] [CrossRef]
- Mata-Carballeira, Ó.; del Campo, I.; Asua, E. An eco-driving approach for ride comfort improvement. IET Intell. Transp. Syst. 2022, 16, 186–205. [Google Scholar] [CrossRef]
- Tang, X.; Duan, Z.; Hu, X.; Pu, H.; Cao, D.; Lin, X. Improving Ride Comfort and Fuel Economy of Connected Hybrid Electric Vehicles Based on Traffic Signals and Real Road Information. IEEE Trans. Veh. Technol. 2021, 70, 3101–3112. [Google Scholar] [CrossRef]
- Sadeghi, J.; Rabiee, S.; Khajehdezfuly, A. Effect of Rail Irregularities on Ride Comfort of Train Moving Over Ballast-Less Tracks. Int. J. Struct. Stab. Dyn. 2019, 19, 1950060. [Google Scholar] [CrossRef]
- Liu, C.; Chen, L.; Yang, X.; Zhang, X.; Yang, Y. General theory of skyhook control and its application to semi-active suspension control strategy design. IEEE Access 2019, 7, 101552–101560. [Google Scholar] [CrossRef]
- Desai, R.M.; Jamadar, M.E.H.; Kumar, H.; Joladarashi, S.; Rajasekaran, S.; Amarnath, G. Evaluation of a commercial MR damper for application in semi-active suspension. SN Appl. Sci. 2019, 1, 1–10. [Google Scholar] [CrossRef]
- Youn, I.; Ahmad, E. Anti-jerk optimal preview control strategy to enhance performance of active and semi-active suspension systems. Electronics 2022, 11, 1657. [Google Scholar] [CrossRef]
- Hosseinian Ahangarnejad, A.; Melzi, S. Numerical analysis of the influence of an actively controlled spoiler on the handling of a sports car. J. Vib. Control 2018, 24, 5437–5448. [Google Scholar] [CrossRef]
- Diba, F.; Barari, A.; Esmailzadeh, E. Handling and safety enhancement of race cars using active aerodynamic systems. Veh. Syst. Dyn. 2014, 52, 1171–1190. [Google Scholar] [CrossRef]
- Piechna, J. A Review of Active Aerodynamic Systems for Road Vehicles. Energies 2021, 14, 7887. [Google Scholar] [CrossRef]
- Corno, M.; Bottelli, S.; Panzani, G.; Spelta, C.; Tanelli, M.; Savaresi, S.M. Performance Assessment of Active Aerodynamic Surfaces for Comfort and Handling Optimization in Sport Cars. IEEE Trans. Control Syst. Technol. 2015, 24, 189–199. [Google Scholar] [CrossRef]
- Wu, Y.; Chen, Z. Improving road holding and ride comfort of vehicle using dual active aerodynamic surfaces. In Proceedings of the 2018 2nd International Conference on Robotics and Automation Sciences (ICRAS), Wuhan, China, 23–25 June 2018; IEEE: New York, NY, USA, 2018; pp. 1–5. [Google Scholar]
- Ahmad, E.; Iqbal, J.; Arshad Khan, M.; Liang, W.; Youn, I. Predictive control using active aerodynamic surfaces to improve ride quality of a vehicle. Electronics 2020, 9, 1463. [Google Scholar] [CrossRef]
- Ahmad, E.; Youn, I. Performance Improvement of a Vehicle Equipped with Active Aerodynamic Surfaces Using Anti-Jerk Preview Control Strategy. Sensors 2022, 22, 8057. [Google Scholar] [CrossRef] [PubMed]
- Gao, W.; Kong, X.; Deng, Z.; Yu, W.; Wu, Y.; Luo, J. Review of state of the art in active aerodynamic control research for vehicles. In Proceedings of the Journal of Physics: Conference Series; IOP Publishing: Bristol, UK, 2021; Volume 1985, p. 012040. [Google Scholar]
- Gu, X.; Zhao, B.; Liu, Y. Optimal nonlinear polynomial control of a quarter-vehicle suspension system under harmonic and random road excitations. J. Low Freq. Noise Vib. Act. Control 2023, 14613484231189971. [Google Scholar] [CrossRef]
- Kim, Y.-J.; Sohn, Y.; Chang, S.; Choi, S.-B.; Oh, J.-S. Vibration Control of Car Body and Wheel Motions for In-Wheel Motor Vehicles Using Road Type Classification. Actuators 2024, 13, 80. [Google Scholar] [CrossRef]
- Karim Afshar, K.; Korzeniowski, R.; Konieczny, J. Evaluation of Ride Performance of Active Inerter-Based Vehicle Suspension System with Parameter Uncertainties and Input Constraint via Robust H∞ Control. Energies 2023, 16, 4099. [Google Scholar] [CrossRef]
- Ganesan, S.; Thiyagarajan, R.; Khan, P.A.K.M. Aerodynamic Performance Assessment on Typical SUV Car Model by On-Road Surface Pressure Mapping Method; Technical Report; SAE Technical Paper; SAE International: Warrendale, PA, USA, 2021. [Google Scholar]
- Stadler, M.; Nordin, J.; Rama, K.; Sjöstrand, P.; Falkovén, A.; Rask, A. CFD-analysis of the aerodynamic properties of a Mercedes-Benz 300SLR. 2015. [Google Scholar]
- Corno, M.; Bottelli, S.; Tanelli, M.; Spelta, C.; Savaresi, S.M. Active control of aerodynamic surfaces for ride control in sport vehicles. IFAC Proc. Vol. 2014, 47, 7553–7558. [Google Scholar] [CrossRef]
- Benhiba, A.; Bybi, A.; Alla, R.; Drissi, H.; Nabti, M.; Chater, E.A. Modeling and simulation of vibrational energy harvesting from passive car suspension subjected to random excitations. In Proceedings of the 2022 2nd International Conference on Innovative Research in Applied Science, Engineering and Technology (IRASET), Meknes, Morocco, 3–4 March 2022; IEEE: New York, NY, USA, 2022; pp. 1–9. [Google Scholar]
- Lenkutis, T.; Čerškus, A.; Šešok, N.; Dzedzickis, A.; Bučinskas, V. Road surface profile synthesis: Assessment of suitability for simulation. Symmetry 2020, 13, 68. [Google Scholar] [CrossRef]
- Tyan, F.; Hong, Y.-F.; Tu, S.-H.; Jeng, W.S. Generation of random road profiles. J. Adv. Eng. 2009, 4, 1373–1378. [Google Scholar]
- Mastinu, G.; Plöchl, M. Road and Off-Road Vehicle System Dynamics Handbook; CRC Press: Boca Raton, FL, USA, 2014. [Google Scholar]
- Youn, I. Optimal design of discrete time preview controllers for semi-active and active suspension systems. KSME Int. J. 2000, 14, 807–815. [Google Scholar] [CrossRef]
- Babawuro, A.; Tahir, N.; Muhammed, M.; Sambo, A. Optimized state feedback control of quarter car active suspension system based on LMI algorithm. In Proceedings of the Journal of Physics: Conference Series; IOP Publishing: Bristol, UK, 2020; Volume 1502, p. 012019. [Google Scholar]
- Rodriguez-Guevara, D.; Favela-Contreras, A.; Beltran-Carbajal, F.; Sotelo, C.; Sotelo, D. A Differential Flatness-Based Model Predictive Control Strategy for a Nonlinear Quarter-Car Active Suspension System. Mathematics 2023, 11, 1067. [Google Scholar] [CrossRef]
- Cao, Z.; Zhao, W.; Hou, X.; Chen, Z. Multi-objective robust control for vehicle active suspension systems via parameterized controller. IEEE Access 2019, 8, 7455–7465. [Google Scholar] [CrossRef]
- Abut, T.; Salkim, E. Control of Quarter-Car Active Suspension System Based on Optimized Fuzzy Linear Quadratic Regulator Control Method. Appl. Sci. 2023, 13, 8802. [Google Scholar] [CrossRef]
- Manna, S.; Mani, G.; Ghildiyal, S.; Stonier, A.A.; Peter, G.; Ganji, V.; Murugesan, S. Ant colony optimization tuned closed-loop optimal control intended for vehicle active suspension system. IEEE Access 2022, 10, 53735–53745. [Google Scholar] [CrossRef]
- Akgul, T.; Unluturk, A. Comparison of PSO-LQR and PSO-PID Controller Performances on a Real Quarter Vehicle Suspension. In Proceedings of the 2023 Innovations in Intelligent Systems and Applications Conference (ASYU), Sivas, Turkiye, 11–13 October 2023; IEEE: New York, NY, USA, 2023; pp. 1–6. [Google Scholar]
- Aljarbouh, A.; Fayaz, M. Hybrid modelling and sliding mode control of semi-active suspension systems for both ride comfort and road-holding. Symmetry 2020, 12, 1286. [Google Scholar] [CrossRef]
- Uddin, N.; Manurung, A.; Wijaya, R.N.A. Optimal state feedback control design of half-car active suspension system. IAENG Int. J. Appl. Math. 2021, 51, 1–9. [Google Scholar]
- Rami, M.A.; Moore, J.B.; Zhou, X.Y. Indefinite stochastic linear quadratic control and generalized differential Riccati equation. SIAM J. Control Optim. 2002, 40, 1296–1311. [Google Scholar] [CrossRef]
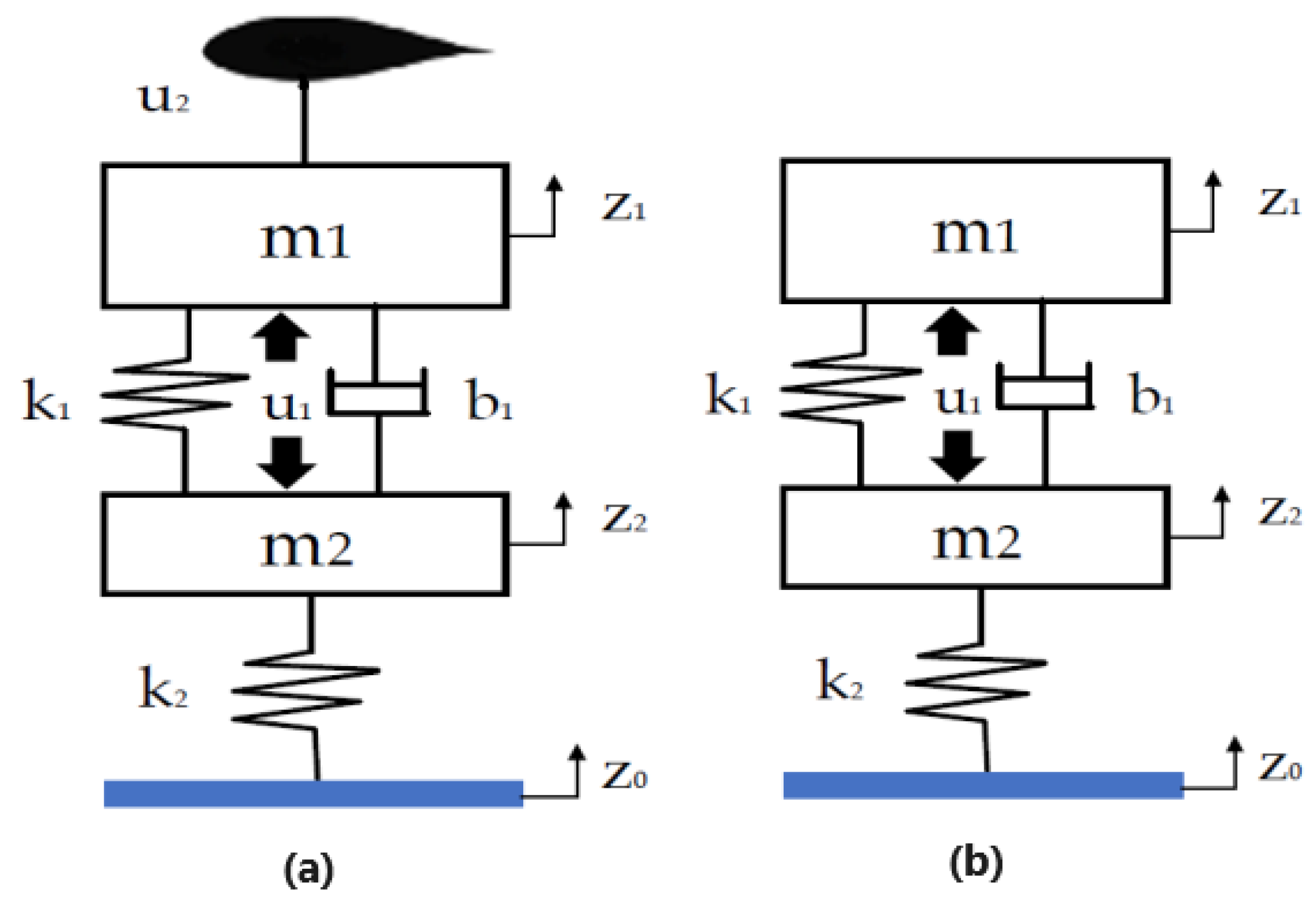







| Parameters | Description | Unit | Typical Values |
|---|---|---|---|
| Sprung mass | |||
| Unsprung mass | |||
| Suspension Stiffness | |||
| Tire stiffness | |||
| Passive damping coefficient |
| Sus. System | Body Acc. (%) | Tire Def. (%) | Sus. Def. (%) | Per. Index. (%) |
|---|---|---|---|---|
| Passive Suspension System | 100 | 100 | 100 | 100 |
| Active Suspension System | ||||
| Active Sus. With Airfoil | ||||
| Airfoil only |
| Sus. System | Body Acc. (%) | Tire Def. (%) | Sus. Def. (%) | Per. Index. (%) |
|---|---|---|---|---|
| Passive Suspension System | 100 | 100 | 100 | 100 |
| Active Suspension System | ||||
| Active Sus. With Airfoil | ||||
| Airfoil only |
| Sus. System | Body Acc. (%) | Tire Def. (%) | Sus. Def. (%) | Per. Index. (%) |
|---|---|---|---|---|
| Passive Suspension System | 100 | 100 | 100 | 100 |
| Active Suspension System | ||||
| Active Sus. With Airfoil | ||||
| Airfoil only |
| Sus. System | Body Acc. (%) | Tire Def. (%) | Sus. Def. (%) | Per. Index. (%) |
|---|---|---|---|---|
| Passive Suspension System | 100 | 100 | 100 | 100 |
| Active Suspension System | ||||
| Active Sus. With Airfoil | ||||
| Airfoil only |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Abbas, S.B.; Youn, I. Performance Improvement of Active Suspension System Collaborating with an Active Airfoil Based on a Quarter-Car Model. Vehicles 2024, 6, 1268-1283. https://doi.org/10.3390/vehicles6030060
Abbas SB, Youn I. Performance Improvement of Active Suspension System Collaborating with an Active Airfoil Based on a Quarter-Car Model. Vehicles. 2024; 6(3):1268-1283. https://doi.org/10.3390/vehicles6030060
Chicago/Turabian StyleAbbas, Syed Babar, and Iljoong Youn. 2024. "Performance Improvement of Active Suspension System Collaborating with an Active Airfoil Based on a Quarter-Car Model" Vehicles 6, no. 3: 1268-1283. https://doi.org/10.3390/vehicles6030060
APA StyleAbbas, S. B., & Youn, I. (2024). Performance Improvement of Active Suspension System Collaborating with an Active Airfoil Based on a Quarter-Car Model. Vehicles, 6(3), 1268-1283. https://doi.org/10.3390/vehicles6030060








