Digital Quantum Simulation of Linear and Nonlinear Optical Elements
Abstract
:1. Digitalization of Bosonic Hamiltonians
1.1. Mapping Bosons to Qubits
1.2. Trotter–SuzukiDecomposition
- The Hamiltonian is decomposed into a number m of terms:
- Time is discretized, namely divided into s steps of duration :
- The exponential of a sum of operators is approximated by the exponential of a product of operators. The approximation is exact only in the case that all the Hamiltonian terms commute. Otherwise, it neglects all the commutators in the Baker–Campbell–Hausdorff formula.
1.3. Gate Decomposition
2. Examples
2.1. Boson Sampling and Boson Sampling Hamiltonian
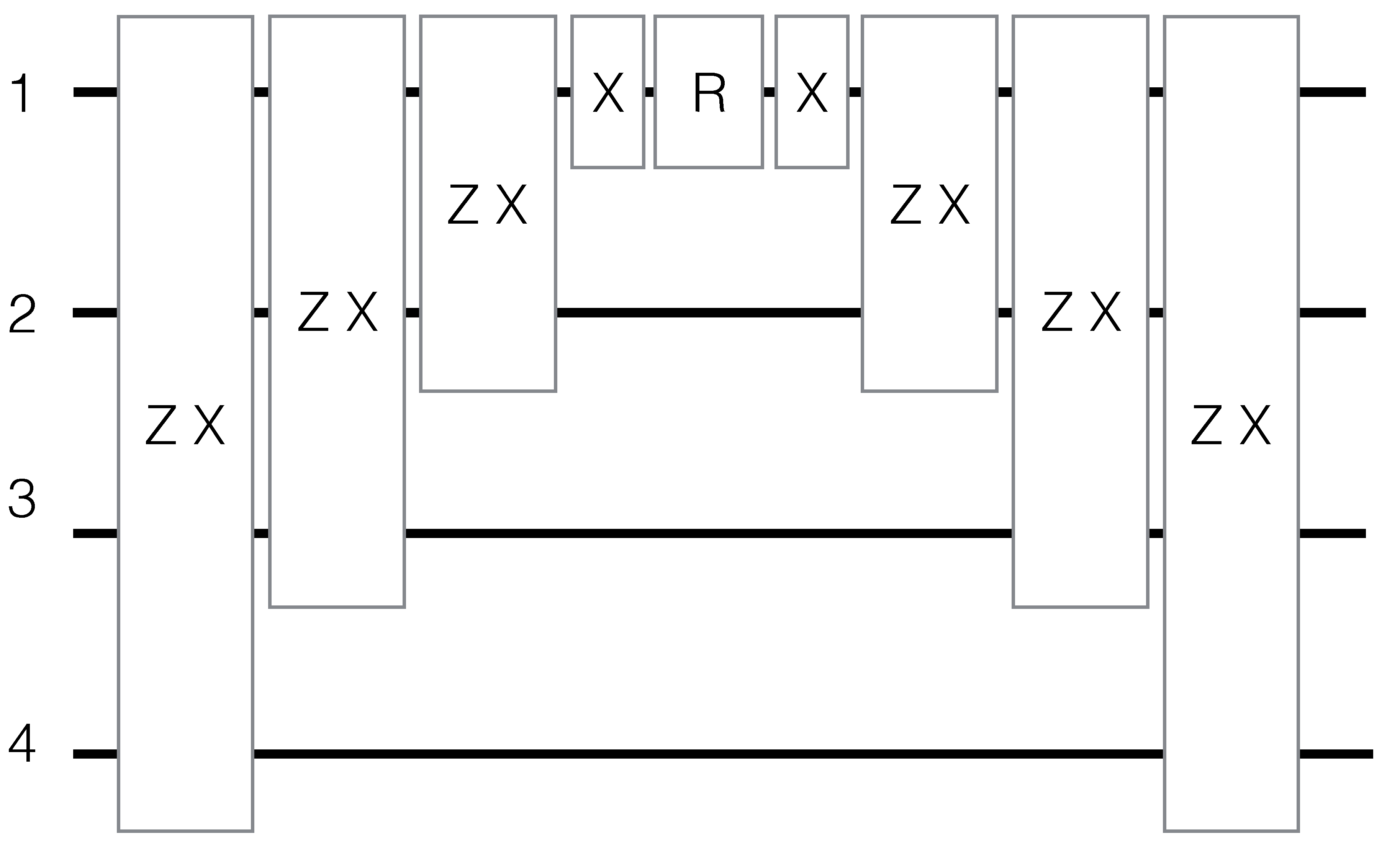
2.2. Beam-Splitters
2.3. Sequence of Beam Splitters
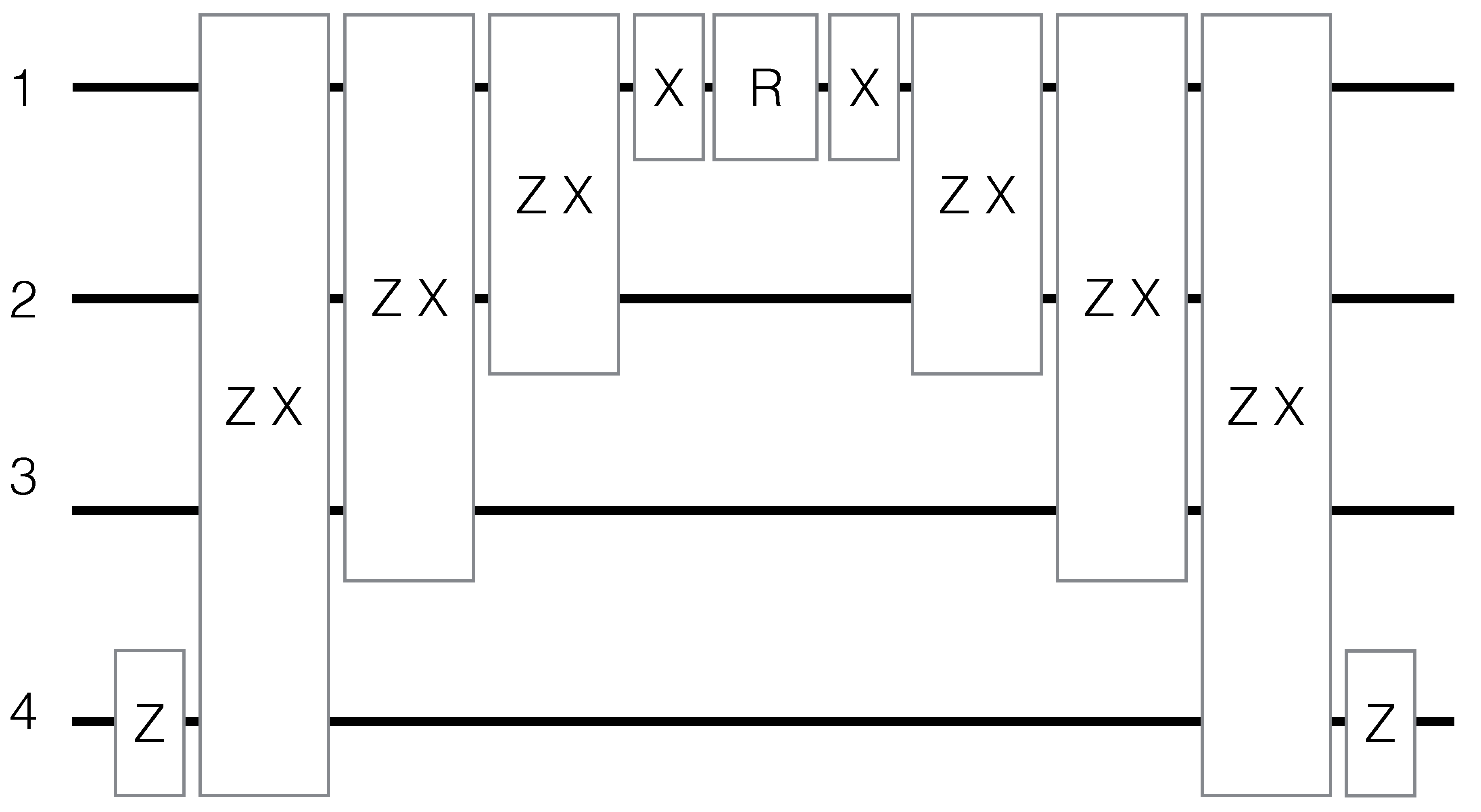
2.4. Two-Mode Squeezing
2.5. Bogoliubov Transformations
2.6. Quantum Information Processing and Quantum Computing Gates
2.7. Molecular Force Fields
3. Conclusions
Funding
Acknowledgments
Conflicts of Interest
References
- Preskill, J. Quantum Computing and the entanglement frontier. In Proceedings of the 25th Solvay Conference on Physics (“The Theory of the Quantum World”), Brussels, Belgium, 19–25 October 2011; World Scientific: Singapore, 2012. [Google Scholar]
- Boixo, S.; Isakov, S.V.; Smelyanskiy, V.N.; Babbush, R.; Ding, N.; Jiang, Z.; Bremner, M.J.; Martinis, J.M.; Neven, H. Characterizing quantum supremacy in near-term devices. Nat. Phys. 2018, 14, 595–600. [Google Scholar] [CrossRef]
- Harrow, A.; Montanaro, A. Quantum computational supremacy. Nature 2017, 549, 203–209. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Aaronson, S.; Arkhipov, A. The computational complexity of linear optics. In Proceedings of the 34th Annual ACM Symposium on Theory of Computing, Montreal, QC, Canada, 19–21 May 2002. [Google Scholar]
- Spring, J.B.; Metcalf, B.J.; Humphreys, P.C.; Kolthammer, W.S.; Jin, X.M.; Barbieri, M.; Datta, A.; Thomas-Peter, N.; Langford, N.K.; Kundys, D.; et al. Boson sampling on a photonic chip. Science 2013, 339, 798–801. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Broome, M.A.; Fedrizzi, A.; Rahimi-Keshari, S.; Dove, J.; Aaronson, S.; Ralph, T.C.; White, A.G. Photonic boson sampling in a tunable circuit. Science 2013, 339, 794–798. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Crespi, A.; Osellame, R.; Ramponi, R.; Brod, D.J.; Galvao, E.F.; Spagnolo, N.; Vitelli, C.; Maiorino, E.; Mataloni, P.; Sciarrino, F. Integrated multimode interferometers with arbitrary designs for photonic boson sampling. Nat. Photonics 2013, 7, 545. [Google Scholar] [CrossRef]
- Tillmann, M.; Dakić, B.; Heilmann, R.; Nolte, S.; Szameit, A.; Walther, P. Experimental boson sampling. Nat. Photonics 2013, 7, 540–544. [Google Scholar] [CrossRef]
- Peropadre, B.; Guerreschi, G.G.; Huh, J.; Aspuru-Guzik, A. Proposal for microwave boson sampling. Phys. Rev. Lett. 2016, 117, 140505. [Google Scholar] [CrossRef]
- Peropadre, B.; Huh, J.; Sabín, C. Dynamical Casimir effect for Gaussian boson sampling. Sci. Rep. 2018, 8, 1–8. [Google Scholar] [CrossRef]
- Gao, Y.Y.; Lester, B.J.; Zhang, Y.; Wang, C.; Rosenblum, S.; Frunzio, L.; Jiang, L.; Girvin, S.M.; Schoelkopf, R.J. Programmable interference between two microwave quantum memories. Phys. Rev. X 2018, 8, 021073. [Google Scholar] [CrossRef] [Green Version]
- Zhang, Y.; Lester, B.J.; Gao, Y.Y.; Jiang, L.; Schoelkopf, R.J.; Girvin, S.M. Engineering bilinear mode coupling in circuit QED: Theory and experiment. Phys. Rev. A 2019, 99, 012314. [Google Scholar] [CrossRef] [Green Version]
- Gao, Y.Y.; Lester, B.J.; Chou, K.S.; Frunzio, L.; Devoret, M.H.; Jiang, L.; Girvin, S.M.; Schoelkopf, R.J. Entanglement of bosonic modes through an engineered exchange interaction. Nature 2019, 566, 509–512. [Google Scholar] [CrossRef] [Green Version]
- Napolitano, M.; Koschorreck, M.; Dubost, B.; Behbood, N.; Sewell, R.J.; Mitchell, M.W. Interaction-based quantum metrology showing scaling beyond the Heisenberg limit. Nature 2011, 471, 486–489. [Google Scholar] [CrossRef]
- Lloyd, S. Universal quantum simulators. Science 1996, 23, 1073–1078. [Google Scholar] [CrossRef]
- Somma, R.; Ortiz, G.; Knill, E.; Gubernatis, J. Quantum simulations of physics problems. Available online: https://www.spiedigitallibrary.org/conference-proceedings-of-spie/5105.toc?SSO=1 (accessed on 3 March 2020).
- Somma, R.D. Quantum Computation, Complexity and Many-Body Physics. Ph.D. Thesis, Cornell University, Ithaca, NY, USA, 2005. [Google Scholar]
- Whitfield, J.D.; Biamonte, J.; Aspuru-Guzik, A. Simulation of electronic structure Hamiltonians using quantum computers. Mol. Phys. 2011, 109, 735–750. [Google Scholar] [CrossRef] [Green Version]
- Macridin, A.; Spentzouris, P.; Amundson, J.; Harnik, R. Electron-phonon systems on a universal quantum computer. Phys. Rev. Lett. 2018, 121, 110504. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Macridin, A.; Spentzouris, P.; Amundson, J.; Harnik, R. Digital quantum computation of fermion-boson interacting systems. Phys. Rev. A 2018, 98, 042312. [Google Scholar] [CrossRef] [Green Version]
- Rungger, I. Dynamical Mean Field Theory Algorithm and Experiment on Quantum Computers. Available online: https://arxiv.org/abs/1910.04735 (accessed on 3 March 2020).
- Huerta Alderete, C. Quantum walks and Dirac cellular automata on a programmable trapped-ion quantum computer. Available online: https://arxiv.org/abs/2002.02537 (accessed on 3 March 2020).
- Wilson, C.M.; Johansson, G.; Pourkabirian, A.; Simoen, M.; Johansson, J.R.; Duty, T.; Nori, F.; Delsing, P. Observation of the dynamical Casimir effect in a superconducting circuit. Nature 2011, 479, 376–379. [Google Scholar] [CrossRef] [PubMed]
- Johansson, J.R.; Johansson, G.; Wilson, C.M.; Delsing, P.; Nori, F. Nonclassical microwave radiation from the dynamical Casimir effect. Phys. Rev. A 2013, 87, 043804. [Google Scholar] [CrossRef] [Green Version]
- Sabín, C.; Fuentes, I.; Johansson, G. Quantum discord in the dynamical Casimir effect. Phys. Rev. A 2015, 92, 012314. [Google Scholar] [CrossRef] [Green Version]
- Sabín, C.; Adesso, G. Generation of quantum steering and interferometric power in the dynamical Casimir effect. Phys. Rev. A 2015, 92, 042107. [Google Scholar] [CrossRef] [Green Version]
- De Buruaga, D.S.; Sabín, C. Quantum coherence in the dynamical Casimir effect. Phys. Rev. A 2017, 95, 022307. [Google Scholar] [CrossRef] [Green Version]
- Felicetti, S.; Sanz, M.; Lamata, L.; Romero, G.; Johansson, G.; Delsing, P.; Solano, E. Dynamical Casimir effect entangles artificial atoms. Phys. Rev. Lett. 2014, 113, 093602. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Agustí, A.; Solano, E.; Sabín, C. Entanglement through qubit motion and the dynamical Casimir effect. Phys. Rev. A 2019, 99, 052328. [Google Scholar] [CrossRef] [Green Version]
- Jaskula, J.C.; Partridge, G.B.; Bonneau, M.; Lopes, R.; Ruaudel, J.; Boiron, D.; Westbrook, C.I. Acoustic analog to the dynamical Casimir effect in a Bose-Einstein condensate. Phys. Rev. Lett. 2012, 109, 220401. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wittemer, M.; Hakelberg, F.; Kiefer, P.; Schröder, J.P.; Fey, C.; Schützhold, R.; Warring, U.; Schaetz, T. Phonon Pair Creation by Inflating Quantum Fluctuations in an Ion Trap. Phys. Rev. Lett. 2019, 123, 180502. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Huh, J. Unified Description of Vibronic Transitions with Coherent States. Ph.D. Thesis, Sungkyunkwan University, Seoul, Korea, 2011. [Google Scholar]
- Olivares, D.G.; Peropadre, B.; Huh, J.; García-Ripoll, J.J. Quantum emulation of molecular force fields: A blueprint for a superconducting architecture. Phys. Rev. Appl. 2017, 8, 064008. [Google Scholar] [CrossRef] [Green Version]
- Cao, Y.; Romero, J.; Olson, J.P.; Degroote, M.; Johnson, P.D.; Kieferová, M.; Kivlichan, I.D.; Menke, T.; Peropadre, B.; Sawaya, N.P.; et al. Quantum chemistry in the age of quantum computing. Chem. Rev. 2019, 119, 10856–10915. [Google Scholar] [CrossRef] [Green Version]
- Lanyon, B.P.; Hempel, C.; Nigg, D.; Müller, M.; Gerritsma, R.; Zähringer, F.; Schindler, P.; Barreiro, J.T.; Rambach, M.; Kirchmair, G.; et al. Universal digital quantum simulation with trapped ions. Science 2011, 334, 57–61. [Google Scholar] [CrossRef] [Green Version]
- Barends, R.; Lamata, L.; Kelly, J.; García-Álvarez, L.; Fowler, A.G.; Megrant, A.; Jeffrey, E.; White, T.C.; Sank, D.; Mutus, J.Y.; et al. Digital quantum simulation of fermionic models with a superconducting circuit. Nat. Commun. 2015, 6, 1–7. [Google Scholar] [CrossRef]
- Barends, R.; Shabani, A.; Lamata, L.; Kelly, J.; Mezzacapo, A.; Las Heras, U.; Babbush, R.; Fowler, A.G.; Campbell, B.; Chen, Y.; et al. Digitized adiabatic quantum computing with a superconducting circuit. Nature 2016, 534, 222–226. [Google Scholar] [CrossRef] [Green Version]
- Geller, M.R.; Martinis, J.M.; Sornborger, A.T.; Stancil, P.C.; Pritchett, E.J.; You, H.; Galiautdinov, A. Universal quantum simulation with prethreshold superconducting qubits: Single-excitation subspace method. Phys. Rev. A 2015, 91, 062309. [Google Scholar] [CrossRef] [Green Version]
- Somma, R.; Ortiz, G.; Gubernatis, J.E.; Knill, E.; Laflamme, R. Simulating physical phenomena by quantum networks. Phys. Rev. A 2002, 65, 042323. [Google Scholar] [CrossRef] [Green Version]
- Neville, A.; Sparrow, C.; Clifford, R.; Johnston, E.; Birchall, P.M.; Montanaro, A.; Laing, A. Classical boson sampling algorithms with superior performance to near-term experiments. Nat. Phys. 2017, 13, 1153–1157. [Google Scholar] [CrossRef]
- Peropadre, B.; Aspuru-Guzik, A.; García-Ripoll, J.J. Equivalence between spin Hamiltonians and boson sampling. Phys. Rev. A 2017, 95, 032327. [Google Scholar] [CrossRef] [Green Version]
- Lund, A.P.; Laing, A.; Rahimi-Keshari, S.; Rudolph, T.; O’Brien, J.L.; Ralph, T.C. Boson sampling from a Gaussian state. Phys. Rev. Lett. 2014, 113, 100502. [Google Scholar] [CrossRef] [Green Version]
- Caves, C.M.; Zhu, C.; Milburn, G.J.; Schleich, W. Photon statistics of two-mode squeezed states and interference in four-dimensional phase space. Phys. Rev. A 1991, 43, 3854. [Google Scholar] [CrossRef] [Green Version]
- Zhang, D.; Li, C.; Zhang, Z.; Zhang, Y.; Zhang, Y.; Xiao, M. Enhanced intensity-difference squeezing via energy-level modulations in hot atomic media. Phys. Rev. A 2017, 96, 043847. [Google Scholar] [CrossRef] [Green Version]
- Li, X.; Zhang, D.; Zhang, D.; Hao, L.; Chen, H.; Wang, Z.; Zhang, Y. Dressing control of biphoton waveform transitions. Phys. Rev. A 2018, 97, 053830. [Google Scholar] [CrossRef]
- Li, C.; Jiang, Z.; Zhang, Y.; Zhang, Z.; Wen, F.; Chen, H.; Zhang, Y.; Xiao, M. Controlled correlation and squeezing in pr 3+: Y 2 sio 5 to yield correlated light beams. Phys. Rev. Appl. 2017, 7, 014023. [Google Scholar] [CrossRef]
- Cheng, L.; Wang, K.; Mao, J.; Goh, X.M.; Chu, Z.; Zhang, Y.; Zhang, L. Extrinsic Polarization-Enabled Covert Plasmonic Colors Using Aluminum Nanostructures. Annalen der Physik 2019, 531, 1900073. [Google Scholar] [CrossRef]
- Chang, C.S.; Sabín, C.; Forn-Díaz, P.; Quij, ría, F.; Vadiraj, A.M.; Nsanzineza, I.; Johansson, G.; Wilson, C.M. Observation of Three-Photon Spontaneous Parametric Down-Conversion in a Superconducting Parametric Cavity. Phys. Rev. X 2020, 10, 011011. [Google Scholar]
- Agustí, A. Tripartite genuine non-gaussian entanglement in three-mode spontaneous parametric downconversion. Available online: https://arxiv.org/abs/2001.07050 (accessed on 3 March 2020).


© 2020 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sabín, C. Digital Quantum Simulation of Linear and Nonlinear Optical Elements. Quantum Rep. 2020, 2, 208-220. https://doi.org/10.3390/quantum2010013
Sabín C. Digital Quantum Simulation of Linear and Nonlinear Optical Elements. Quantum Reports. 2020; 2(1):208-220. https://doi.org/10.3390/quantum2010013
Chicago/Turabian StyleSabín, Carlos. 2020. "Digital Quantum Simulation of Linear and Nonlinear Optical Elements" Quantum Reports 2, no. 1: 208-220. https://doi.org/10.3390/quantum2010013
APA StyleSabín, C. (2020). Digital Quantum Simulation of Linear and Nonlinear Optical Elements. Quantum Reports, 2(1), 208-220. https://doi.org/10.3390/quantum2010013




