A Dyson Brownian Motion Model for Weak Measurements in Chaotic Quantum Systems
Abstract
1. Introduction
2. Preliminaries on Unravelings and Trajectories
3. The Model
4. The Case
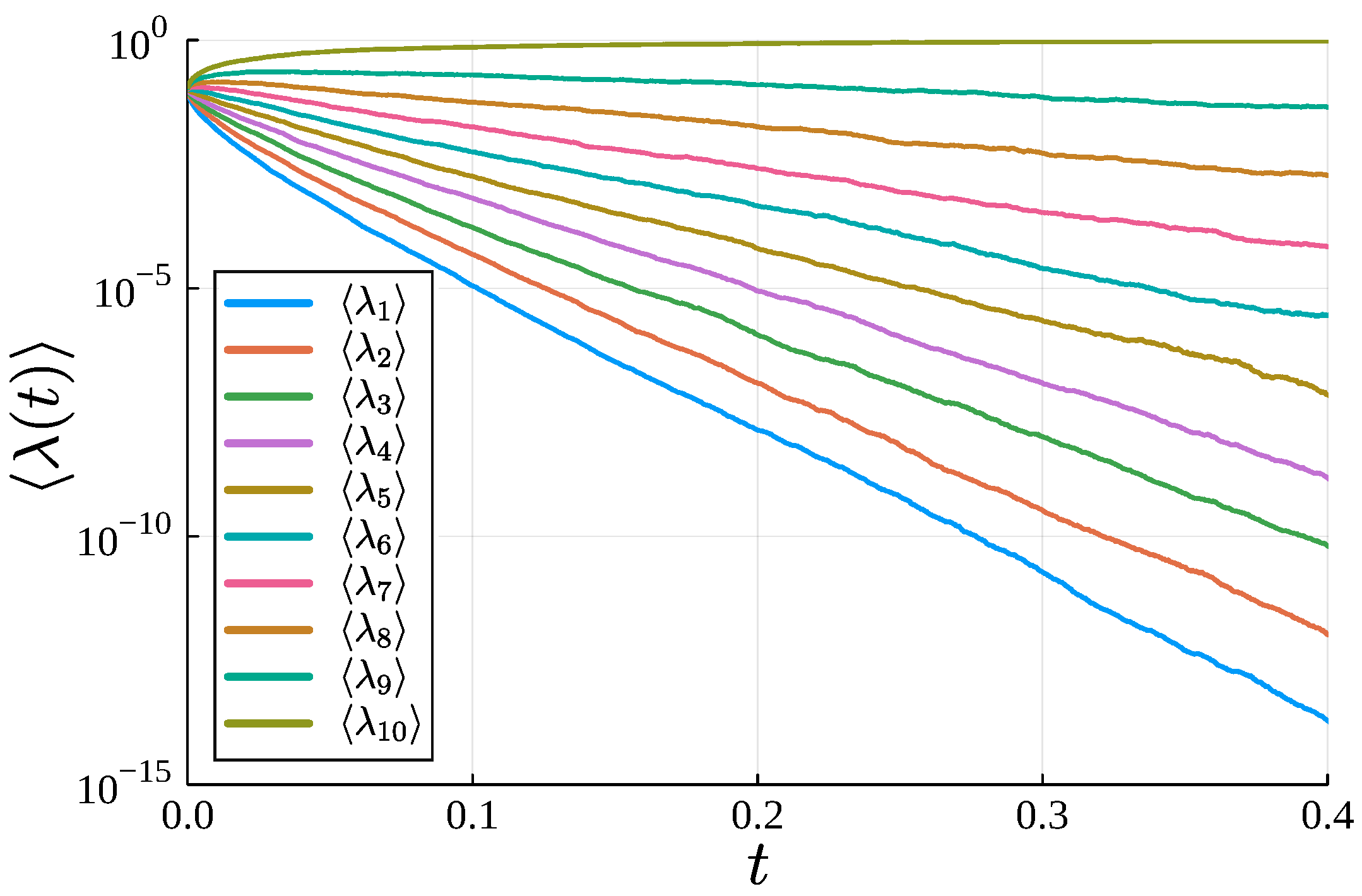
5. Dynamics of the Spectrum
5.1. Stochastic Evolution of the Eigenvalues
5.2. Mapping to Unconstrained Variables
6. Stationary State at and
6.1. Finite Time Dynamics
7. The Perfect Measurement Dynamics
7.1. Exact Solution at Finite Time
7.2. Relation between the Two Averages
8. Exact Results for the Unbiased Ensemble
8.1. Average of Schur’s Polynomials
8.2. Power-Law Symmetric Polynomials
8.3. Calculation of the Moments
8.4. Equivalent Formulations
8.5. Coulomb Gas Regime
8.6. Universal Regime
8.6.1. Scaling of the Edge
8.6.2. Asymptotics at Large
9. Entanglement Entropies for Continuous Monitoring
9.1. Short Time Regime
9.2. Universal Regime
10. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Identities
Appendix B. Finite-Time Von Neumann Entropy for the n = 2 Case
Appendix C. Inverse-Wishart Ensemble
Appendix D. Equivalent Dyson Brownian Motion
Appendix E. Kernel
Appendix F. Large Time Moments from a Saddle Point
Appendix G. Long-Time Entanglement Entropy
References
- Bonderson, P.; Freedman, M.; Nayak, C. Measurement-Only Topological Quantum Computation. Phys. Rev. Lett. 2008, 101, 010501. [Google Scholar] [CrossRef] [PubMed]
- Leung, D.W. Quantum computation by measurements. Int. J. Quantum Inf. 2004, 2, 33–43. [Google Scholar] [CrossRef]
- Skinner, B.; Ruhman, J.; Nahum, A. Measurement-Induced Phase Transitions in the Dynamics of Entanglement. Phys. Rev. X 2019, 9, 031009. [Google Scholar] [CrossRef]
- Li, Y.; Chen, X.; Fisher, M.P.A. Quantum Zeno effect and the many-body entanglement transition. Phys. Rev. B 2018, 98, 205136. [Google Scholar] [CrossRef]
- Li, Y.; Chen, X.; Fisher, M.P.A. Measurement-driven entanglement transition in hybrid quantum circuits. Phys. Rev. B 2019, 100, 134306. [Google Scholar] [CrossRef]
- Christopoulos, A.; Le Doussal, P.; Bernard, D.; De Luca, A. Universal Out-of-Equilibrium Dynamics of 1D Critical Quantum Systems Perturbed by Noise Coupled to Energy. Phys. Rev. X 2023, 13, 011043. [Google Scholar] [CrossRef]
- Hruza, L.; Bernard, D. Coherent Fluctuations in Noisy Mesoscopic Systems, the Open Quantum SSEP, and Free Probability. Phys. Rev. X 2023, 13, 011045. [Google Scholar] [CrossRef]
- Bernard, D. Can the macroscopic fluctuation theory be quantized? J. Phys. A Math. Theor. 2021, 54, 433001. [Google Scholar] [CrossRef]
- Zhou, T.; Nahum, A. Emergent statistical mechanics of entanglement in random unitary circuits. Phys. Rev. B 2019, 99, 174205. [Google Scholar] [CrossRef]
- Gullans, M.J.; Huse, D.A. Scalable Probes of Measurement-Induced Criticality. Phys. Rev. Lett. 2020, 125, 070606. [Google Scholar] [CrossRef]
- D’Alessio, L.; Kafri, Y.; Polkovnikov, A.; Rigol, M. From quantum chaos and eigenstate thermalization to statistical mechanics and thermodynamics. Adv. Phys. 2016, 65, 239–362. [Google Scholar] [CrossRef]
- Nahum, A.; Ruhman, J.; Vijay, S.; Haah, J. Quantum Entanglement Growth under Random Unitary Dynamics. Phys. Rev. X 2017, 7, 031016. [Google Scholar] [CrossRef]
- Kim, H.; Huse, D.A. Ballistic Spreading of Entanglement in a Diffusive Nonintegrable System. Phys. Rev. Lett. 2013, 111, 127205. [Google Scholar] [CrossRef]
- Bardarson, J.H.; Pollmann, F.; Moore, J.E. Unbounded Growth of Entanglement in Models of Many-Body Localization. Phys. Rev. Lett. 2012, 109, 017202. [Google Scholar] [CrossRef] [PubMed]
- Abanin, D.A.; Altman, E.; Bloch, I.; Serbyn, M. Colloquium: Many-body localization, thermalization, and entanglement. Rev. Mod. Phys. 2019, 91, 021001. [Google Scholar] [CrossRef]
- Calabrese, P.; Cardy, J. Evolution of entanglement entropy in one-dimensional systems. J. Stat. Mech. Theory Exp. 2005, 2005, P04010. [Google Scholar] [CrossRef]
- Chan, A.; De Luca, A.; Chalker, J.T. Solution of a Minimal Model for Many-Body Quantum Chaos. Phys. Rev. X 2018, 8, 041019. [Google Scholar] [CrossRef]
- Bertini, B.; Kos, P.; Prosen, T.c.v. Entanglement Spreading in a Minimal Model of Maximal Many-Body Quantum Chaos. Phys. Rev. X 2019, 9, 021033. [Google Scholar] [CrossRef]
- Fisher, M.P.; Khemani, V.; Nahum, A.; Vijay, S. Random Quantum Circuits. Annu. Rev. Condens. Matter Phys. 2023, 14, 335–379. [Google Scholar] [CrossRef]
- Lunt, O.; Szyniszewski, M.; Pal, A. Measurement-induced criticality and entanglement clusters: A study of one-dimensional and two-dimensional Clifford circuits. Phys. Rev. B 2021, 104, 155111. [Google Scholar] [CrossRef]
- Jian, C.M.; You, Y.Z.; Vasseur, R.; Ludwig, A.W.W. Measurement-induced criticality in random quantum circuits. Phys. Rev. B 2020, 101, 104302. [Google Scholar] [CrossRef]
- Zabalo, A.; Gullans, M.J.; Wilson, J.H.; Vasseur, R.; Ludwig, A.W.W.; Gopalakrishnan, S.; Huse, D.A.; Pixley, J.H. Operator Scaling Dimensions and Multifractality at Measurement-Induced Transitions. Phys. Rev. Lett. 2022, 128, 050602. [Google Scholar] [CrossRef] [PubMed]
- Nahum, A.; Wiese, K.J. Renormalization group for measurement and entanglement phase transitions. Phys. Rev. B 2023, 108, 104203. [Google Scholar] [CrossRef]
- Minato, T.; Sugimoto, K.; Kuwahara, T.; Saito, K. Fate of Measurement-Induced Phase Transition in Long-Range Interactions. Phys. Rev. Lett. 2022, 128, 010603. [Google Scholar] [CrossRef] [PubMed]
- Zhou, Y.N. Generalized Lindblad master equation for measurement-induced phase transition. SciPost Phys. Core 2023, 6, 023. [Google Scholar] [CrossRef]
- Tang, Q.; Zhu, W. Measurement-induced phase transition: A case study in the nonintegrable model by density-matrix renormalization group calculations. Phys. Rev. Res. 2020, 2, 013022. [Google Scholar] [CrossRef]
- Willsher, J.; Liu, S.W.; Moessner, R.; Knolle, J. Measurement-induced phase transition in a chaotic classical many-body system. Phys. Rev. B 2022, 106, 024305. [Google Scholar] [CrossRef]
- Li, Y.; Zou, Y.; Glorioso, P.; Altman, E.; Fisher, M.P.A. Cross Entropy Benchmark for Measurement-Induced Phase Transitions. Phys. Rev. Lett. 2023, 130, 220404. [Google Scholar] [CrossRef] [PubMed]
- Yang, Z.C.; Li, Y.; Fisher, M.P.A.; Chen, X. Entanglement phase transitions in random stabilizer tensor networks. Phys. Rev. B 2022, 105, 104306. [Google Scholar] [CrossRef]
- Choi, S.; Bao, Y.; Qi, X.L.; Altman, E. Quantum Error Correction in Scrambling Dynamics and Measurement-Induced Phase Transition. Phys. Rev. Lett. 2020, 125, 030505. [Google Scholar] [CrossRef]
- Gullans, M.J.; Huse, D.A. Dynamical Purification Phase Transition Induced by Quantum Measurements. Phys. Rev. X 2020, 10, 041020. [Google Scholar] [CrossRef]
- Ticozzi, F.; Viola, L. Quantum resources for purification and cooling: Fundamental limits and opportunities. Sci. Rep. 2014, 4, 5192. [Google Scholar] [CrossRef] [PubMed]
- Lóio, H.; De Luca, A.; De Nardis, J.; Turkeshi, X. Purification timescales in monitored fermions. Phys. Rev. B 2023, 108, L020306. [Google Scholar] [CrossRef]
- Kelly, S.P.; Poschinger, U.; Schmidt-Kaler, F.; Fisher, M.; Marino, J. Coherence requirements for quantum communication from hybrid circuit dynamics. SciPost Phys. 2023, 15, 250. [Google Scholar] [CrossRef]
- Vidal, G. Efficient Classical Simulation of Slightly Entangled Quantum Computations. Phys. Rev. Lett. 2003, 91, 147902. [Google Scholar] [CrossRef]
- Vidal, G. Efficient Simulation of One-Dimensional Quantum Many-Body Systems. Phys. Rev. Lett. 2004, 93, 040502. [Google Scholar] [CrossRef]
- Claeys, P.W.; Henry, M.; Vicary, J.; Lamacraft, A. Exact dynamics in dual-unitary quantum circuits with projective measurements. Phys. Rev. Res. 2022, 4, 043212. [Google Scholar] [CrossRef]
- Nahum, A.; Skinner, B. Entanglement and dynamics of diffusion-annihilation processes with Majorana defects. Phys. Rev. Res. 2020, 2, 023288. [Google Scholar] [CrossRef]
- Cao, X.; Tilloy, A.; Luca, A.D. Entanglement in a fermion chain under continuous monitoring. SciPost Phys. 2019, 7, 024. [Google Scholar] [CrossRef]
- Fidkowski, L.; Haah, J.; Hastings, M.B. How Dynamical Quantum Memories Forget. Quantum 2021, 5, 382. [Google Scholar] [CrossRef]
- Coppola, M.; Tirrito, E.; Karevski, D.; Collura, M. Growth of entanglement entropy under local projective measurements. Phys. Rev. B 2022, 105, 094303. [Google Scholar] [CrossRef]
- Santini, A.; Solfanelli, A.; Gherardini, S.; Giachetti, G. Observation of partial and infinite-temperature thermalization induced by repeated measurements on a quantum hardware. J. Phys. Commun. 2023, 7, 065007. [Google Scholar] [CrossRef]
- Piccitto, G.; Russomanno, A.; Rossini, D. Entanglement transitions in the quantum Ising chain: A comparison between different unravelings of the same Lindbladian. Phys. Rev. B 2022, 105, 064305. [Google Scholar] [CrossRef]
- Turkeshi, X.; Dalmonte, M.; Fazio, R.; Schirò, M. Entanglement transitions from stochastic resetting of non-Hermitian quasiparticles. Phys. Rev. B 2022, 105, L241114. [Google Scholar] [CrossRef]
- Turkeshi, X.; Biella, A.; Fazio, R.; Dalmonte, M.; Schiró, M. Measurement-induced entanglement transitions in the quantum Ising chain: From infinite to zero clicks. Phys. Rev. B 2021, 103, 224210. [Google Scholar] [CrossRef]
- Alberton, O.; Buchhold, M.; Diehl, S. Entanglement Transition in a Monitored Free–Fermion Chain: From Extended Criticality to Area Law. Phys. Rev. Lett. 2021, 126, 170602. [Google Scholar] [CrossRef] [PubMed]
- Buchhold, M.; Minoguchi, Y.; Altland, A.; Diehl, S. Effective Theory for the Measurement-Induced Phase Transition of Dirac Fermions. Phys. Rev. X 2021, 11, 041004. [Google Scholar] [CrossRef]
- Müller, T.; Diehl, S.; Buchhold, M. Measurement-Induced Dark State Phase Transitions in Long-Ranged Fermion Systems. Phys. Rev. Lett. 2022, 128, 010605. [Google Scholar] [CrossRef] [PubMed]
- Ladewig, B.; Diehl, S.; Buchhold, M. Monitored open fermion dynamics: Exploring the interplay of measurement, decoherence, and free Hamiltonian evolution. Phys. Rev. Res. 2022, 4, 033001. [Google Scholar] [CrossRef]
- Lucas, M.; Piroli, L.; De Nardis, J.; De Luca, A. Generalized deep thermalization for free fermions. Phys. Rev. A 2023, 107, 032215. [Google Scholar] [CrossRef]
- Fava, M.; Piroli, L.; Swann, T.; Bernard, D.; Nahum, A. Nonlinear Sigma Models for Monitored Dynamics of Free Fermions. Phys. Rev. X 2023, 13, 041045. [Google Scholar] [CrossRef]
- Poboiko, I.; Pöpperl, P.; Gornyi, I.V.; Mirlin, A.D. Theory of Free Fermions under Random Projective Measurements. Phys. Rev. X 2023, 13, 041046. [Google Scholar] [CrossRef]
- Mézard, M.; Parisi, G.; Virasoro, M.A. Spin Glass Theory and Beyond; World Scientific Publishing Company: Singapore, 1987; Volume 9. [Google Scholar]
- Bray, A.J.; Moore, M.A. Replica theory of quantum spin glasses. J. Phys. C Solid State Phys. 1980, 13, L655. [Google Scholar] [CrossRef]
- Lopez-Piqueres, J.; Ware, B.; Vasseur, R. Mean-field entanglement transitions in random tree tensor networks. Phys. Rev. B 2020, 102, 064202. [Google Scholar] [CrossRef]
- Vasseur, R.; Potter, A.C.; You, Y.Z.; Ludwig, A.W.W. Entanglement transitions from holographic random tensor networks. Phys. Rev. B 2019, 100, 134203. [Google Scholar] [CrossRef]
- Bentsen, G.S.; Sahu, S.; Swingle, B. Measurement-induced purification in large-N hybrid Brownian circuits. Phys. Rev. B 2021, 104, 094304. [Google Scholar] [CrossRef]
- Jian, S.K.; Liu, C.; Chen, X.; Swingle, B.; Zhang, P. Measurement-Induced Phase Transition in the Monitored Sachdev-Ye-Kitaev Model. Phys. Rev. Lett. 2021, 127, 140601. [Google Scholar] [CrossRef] [PubMed]
- Nahum, A.; Roy, S.; Skinner, B.; Ruhman, J. Measurement and Entanglement Phase Transitions in All-To-All Quantum Circuits, on Quantum Trees, and in Landau-Ginsburg Theory. PRX Quantum 2021, 2, 010352. [Google Scholar] [CrossRef]
- Fisher, R.A. The wave of advance of advantageous genes. Ann. Eugen. 1937, 7, 355–369. [Google Scholar] [CrossRef]
- Kolmogorov, A.N.; Petrovskii, I.; Piskunov, N.S. A Study of the Diffusion Equation with Increase in the Amount of Substance, and its Application to a Biological Problem. Mosc. Univ. Math. Bull. 1991, 6, 1–25. [Google Scholar]
- Derrida, B.; Spohn, H. Polymers on disordered trees, spin glasses, and traveling waves. J. Stat. Phys. 1988, 51, 817–840. [Google Scholar] [CrossRef]
- Giachetti, G.; De Luca, A. Elusive phase transition in the replica limit of monitored systems. arXiv 2023, arXiv:2306.12166. [Google Scholar] [CrossRef]
- Schomerus, H. Noisy monitored quantum dynamics of ergodic multi-qubit systems. J. Phys. A Math. Theor. 2022, 55, 214001. [Google Scholar] [CrossRef]
- Caves, C.M.; Milburn, G.J. Quantum-mechanical model for continuous position measurements. Phys. Rev. A 1987, 36, 5543–5555. [Google Scholar] [CrossRef] [PubMed]
- Nielsen, M.A.; Chuang, I. Quantum Computation and Quantum Information; Cambridge University Press: Cambridge, UK, 2002. [Google Scholar]
- Choi, M.D. Completely positive linear maps on complex matrices. Linear Algebra Its Appl. 1975, 10, 285–290. [Google Scholar] [CrossRef]
- Kraus, K. General state changes in quantum theory. Ann. Phys. 1971, 64, 311–335. [Google Scholar] [CrossRef]
- Diósi, L.; Gisin, N.; Strunz, W.T. Non-Markovian quantum state diffusion. Phys. Rev. A 1998, 58, 1699–1712. [Google Scholar] [CrossRef]
- Gisin, N.; Percival, I.C. The quantum-state diffusion model applied to open systems. J. Phys. A Math. Gen. 1992, 25, 5677. [Google Scholar] [CrossRef]
- Dyson, F.J. A Brownian-Motion Model for the Eigenvalues of a Random Matrix. J. Math. Phys. 2004, 3, 1191–1198. [Google Scholar] [CrossRef]
- Gautié, T.; Bouchaud, J.P.; Le Doussal, P. Matrix Kesten recursion, inverse-Wishart ensemble and fermions in a Morse potential. J. Phys. A Math. Theor. 2021, 54, 255201. [Google Scholar] [CrossRef]
- Karlin, S.; McGregor, J. Coincidence probabilities. Pac. J. Math. 1959, 9, 1141–1164. [Google Scholar] [CrossRef]
- Smith, N.R.; Doussal, P.L.; Majumdar, S.N.; Schehr, G. Full counting statistics for interacting trapped fermions. SciPost Phys. 2021, 11, 110. [Google Scholar] [CrossRef]
- Ipsen, J.R.; Schomerus, H. Isotropic Brownian motions over complex fields as a solvable model for May–Wigner stability analysis. J. Phys. A Math. Theor. 2016, 49, 385201. [Google Scholar] [CrossRef]
- Mergny, P.; Majumdar, S.N. Stability of large complex systems with heterogeneous relaxation dynamics. J. Stat. Mech. Theory Exp. 2021, 2021, 123301. [Google Scholar] [CrossRef]
- Derrida, B.; Hilhorst, H.J. Singular Behaviour of Certain Infinite Products of Random 2 × 2 Matrices. J. Phys. A Math. Gen. 1983, 16, 2641–2654. [Google Scholar] [CrossRef]
- Bouchard, J.P.; Georges, A.; Hansel, D.; Doussal, P.L.; Maillard, J.M. Rigorous Bounds and the Replica Method for Products of Random Matrices. J. Phys. A Math. Gen. 1986, 19, L1145–L1152. [Google Scholar] [CrossRef]
- Ipsen, J.R. Products of Independent Gaussian Random Matrices. arXiv 2015, arXiv:1510.06128. [Google Scholar] [CrossRef]
- Haake, F. Quantum Signatures of Chaos; Springer: Berlin/Heidelberg, Germany, 1991. [Google Scholar]
- Forrester, P.J. Global and local scaling limits for the β = 2 Stieltjes–Wigert random matrix ensemble. arXiv 2020, arXiv:math-ph/2011.11783. [Google Scholar] [CrossRef]
- Macdonald, I.G. Symmetric Functions and Hall Polynomials; Oxford University Press: Oxford, UK, 1998. [Google Scholar]
- Forrester, P.J. Meet Andréief, Bordeaux 1886, and Andreev, Kharkov 1882–1883. Random Matrices Theory Appl. 2019, 8, 1930001. [Google Scholar] [CrossRef]
- Jonnadula, B.; Keating, J.P.; Mezzadri, F. Symmetric function theory and unitary invariant ensembles. J. Math. Phys. 2021, 62, 093512. [Google Scholar] [CrossRef]
- Wikipedia Contributors. Hypergeometric Function—Wikipedia, the Free Encyclopedia. 2023. Available online: https://en.wikipedia.org/w/index.php?title=Hypergeometric_function&oldid=1177949416 (accessed on 22 December 2023).
- Brézin, E.; Hikami, S. Correlations of nearby levels induced by a random potential. Nucl. Phys. B 1996, 479, 697–706. [Google Scholar] [CrossRef]
- Brézin, E.; Hikami, S. Level spacing of random matrices in an external source. Phys. Rev. E 1998, 58, 7176–7185. [Google Scholar] [CrossRef]
- Johansson, K.J. Universality of the Local Spacing Distribution in Certain Ensembles of Hermitian Wigner Matrices. Commun. Math. Phys. 2001, 215, 683–705. [Google Scholar] [CrossRef]
- Claeys, T.; Wang, D. Random matrices with equispaced external source. Commun. Math. Phys. 2014, 328, 1023–1077. [Google Scholar] [CrossRef]
- Krajenbrink, A.; Le Doussal, P.; O’Connell, N. Tilted elastic lines with columnar and point disorder, non-Hermitian quantum mechanics, and spiked random matrices: Pinning and localization. Phys. Rev. E 2021, 103, 042120. [Google Scholar] [CrossRef]
- Claeys, T.; Kuijlaars, A.B.; Liechty, K.; Wang, D. Propagation of singular behavior for Gaussian perturbations of random matrices. Commun. Math. Phys. 2018, 362, 1–54. [Google Scholar] [CrossRef]
- Forrester, P.J. Properties of an exact crystalline many-body ground state. J. Stat. Phys. 1994, 76, 331–346. [Google Scholar] [CrossRef]
- Akemann, G.; Kieburg, M.; Wei, L. Singular value correlation functions for products of Wishart random matrices. J. Phys. A Math. Theor. 2013, 46, 275205. [Google Scholar] [CrossRef]
- De Luca, A.; Liu, C.; Nahum, A.; Zhou, T. Universality classes for purification in nonunitary quantum processes. arXiv 2023, arXiv:2312.17744. [Google Scholar]
- Flack, A.; Le Doussal, P.; Majumdar, S.N.; Schehr, G. Out-of-equilibrium dynamics of repulsive ranked diffusions: The expanding crystal. Phys. Rev. E 2023, 107, 064105. [Google Scholar] [CrossRef]
- Le Doussal, P. Ranked diffusion, delta Bose gas, and Burgers equation. Phys. Rev. E 2022, 105, L012103. [Google Scholar] [CrossRef] [PubMed]
- Bulchandani, V.B.; Sondhi, S.L.; Chalker, J.T. Random-matrix models of monitored quantum circuits. J. Stat. Phys. 2024, 191, 55. [Google Scholar] [CrossRef]
- Livan, G.; Novaes, M.; Vivo, P. Introduction to random matrices theory and practice. Monogr. Award 2018, 63, 54–57. [Google Scholar]
- Potters, M.; Bouchaud, J.P. A First Course in Random Matrix Theory: For Physicists, Engineers and Data Scientists; Cambridge University Press: Cambridge, UK, 2020. [Google Scholar]
- Liu, D.Z.; Wang, D.; Wang, Y. Lyapunov Exponent, Universality and Phase Transition for Products of Random Matrices. Commun. Math. Phys. 2023, 399, 1811–1855. [Google Scholar] [CrossRef]
- Akemann, G.; Burda, Z.; Kieburg, M. Universality of local spectral statistics of products of random matrices. Phys. Rev. E 2020, 102, 052134. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gerbino, F.; Le Doussal, P.; Giachetti, G.; De Luca, A. A Dyson Brownian Motion Model for Weak Measurements in Chaotic Quantum Systems. Quantum Rep. 2024, 6, 200-230. https://doi.org/10.3390/quantum6020016
Gerbino F, Le Doussal P, Giachetti G, De Luca A. A Dyson Brownian Motion Model for Weak Measurements in Chaotic Quantum Systems. Quantum Reports. 2024; 6(2):200-230. https://doi.org/10.3390/quantum6020016
Chicago/Turabian StyleGerbino, Federico, Pierre Le Doussal, Guido Giachetti, and Andrea De Luca. 2024. "A Dyson Brownian Motion Model for Weak Measurements in Chaotic Quantum Systems" Quantum Reports 6, no. 2: 200-230. https://doi.org/10.3390/quantum6020016
APA StyleGerbino, F., Le Doussal, P., Giachetti, G., & De Luca, A. (2024). A Dyson Brownian Motion Model for Weak Measurements in Chaotic Quantum Systems. Quantum Reports, 6(2), 200-230. https://doi.org/10.3390/quantum6020016





