Effect of Adhesive Thickness on Fatigue Disbonding Through a Cohesive Zone Modelling Approach †
Abstract
:1. Introduction
2. Cohesive Zone Models (CZMs)
2.1. Numerical Implementation of Cohesive Zone Models for Quasi-Static Loading
2.2. Numerical Implementation of Cohesive Zone Models for Fatigue Loading
Cycle Jump Strategy
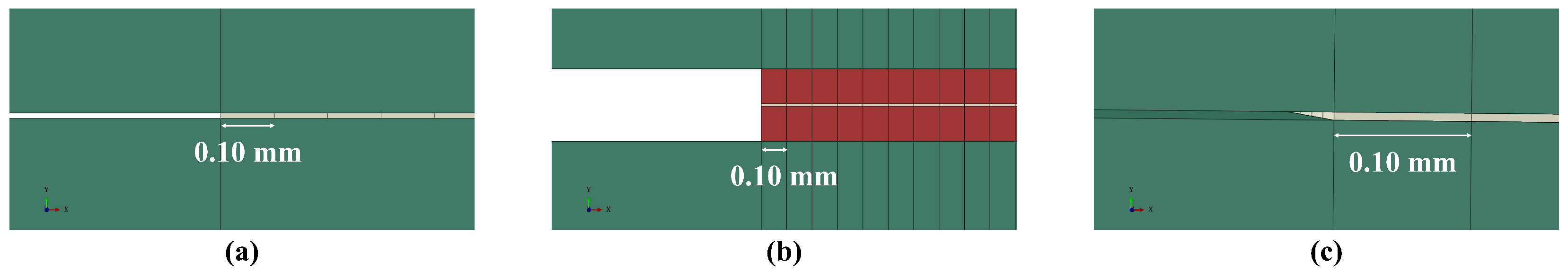
3. Methods
- Figure 2a—CPE4 elements of 1.00 mm length for the aluminum arms and COH2D4 elements of 0.10 mm length for the cohesive zone;
- Figure 2b—CPE4 elements of 1.00 mm length for the aluminum arms, CPE4 elements of 0.10 mm length for the adhesive and COH2D4 elements of 0.10 mm length for the cohesive zone;
- Figure 2c—SC8R elements of 1.00 mm length with a refinement of 0.10 mm on the crack growth zone for the aluminum arms and COH3D8 elements of 0.10 mm length for the cohesive zone.
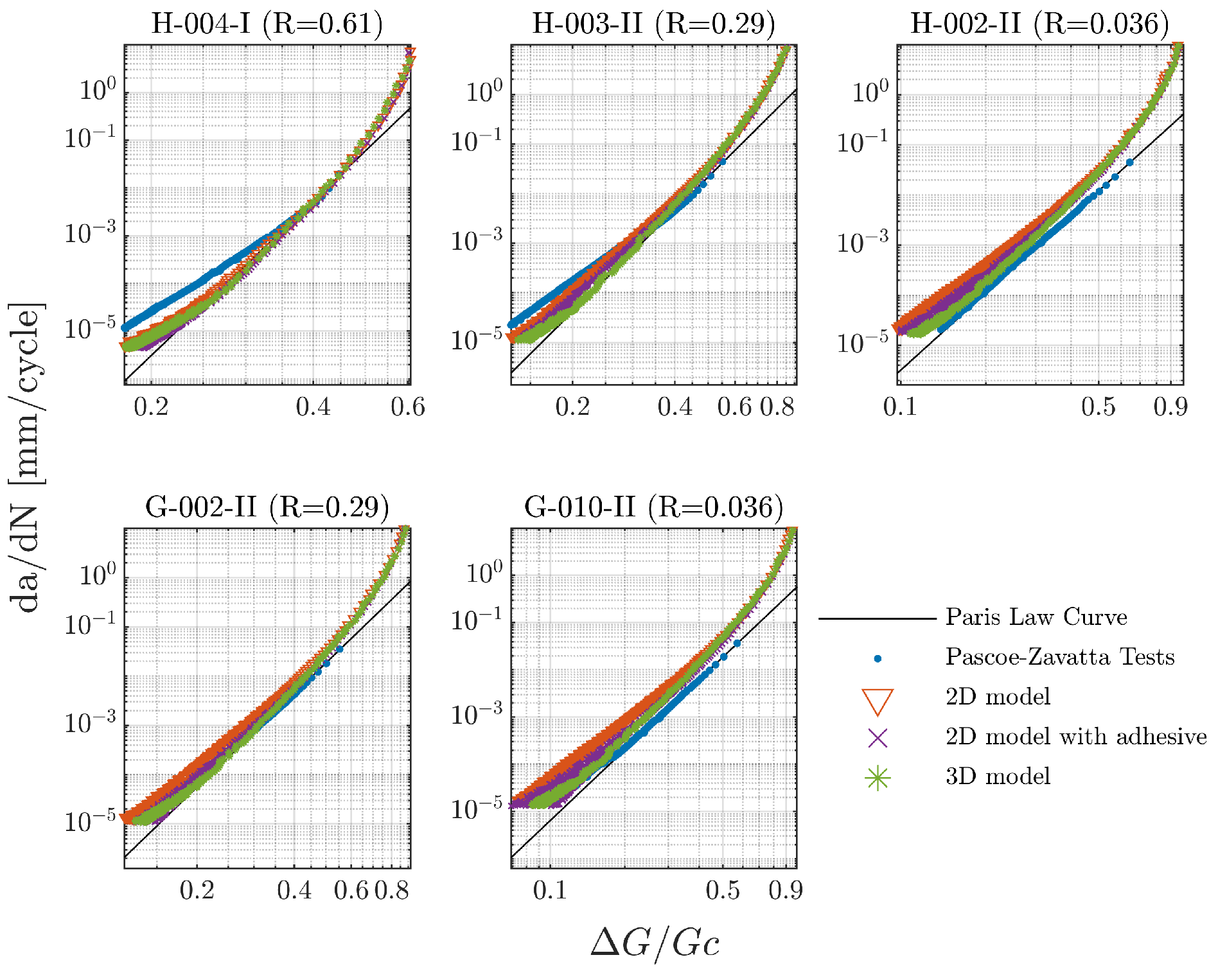
4. Results
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Clean Aviation Joint Undertaking. Who we are | Clean Aviation. Clean Aviation Joint Undertaking: Brussels, Belgium. Available online: https://www.clean-aviation.eu/about-us/who-we-are (accessed on 29 July 2024).
- Kupski, J.; Teixeira de Freitas, S. Design of adhesively bonded lap joints with laminated CFRP adherends: Review, challenges and new opportunities for aerospace structures. Compos. Struct. 2021, 268, 113923. [Google Scholar] [CrossRef]
- Pascoe, J.A. Characterisation of Fatigue Crack Growth in Adhesive Bonds. Ph.D. Thesis, TU Delft, Delft, The Netherlands, 2016. [Google Scholar]
- Tserpes, K.; Floros, I. Fatigue crack growth simulation in adhesively bonded composite joints. Fatigue Fract. Eng. Mater. Struct. 2019, 42, 1430–1440. [Google Scholar] [CrossRef]
- Rocha, A.V.M.; Akhavan-Safar, A.; Carbas, R.; Marques, E.A.S.; Goyal, R.; El-zein, M.; da Silva, L.F.M. Numerical analysis of mixed-mode fatigue crack growth of adhesive joints using CZM. Theor. Appl. Fract. Mech. 2020, 106, 102493. [Google Scholar] [CrossRef]
- Khoramishad, H.; Crocombe, A.D.; Katnam, K.B.; Ashcroft, I.A. Predicting fatigue damage in adhesively bonded joints using a cohesive zone model. Int. J. Fatigue 2010, 32, 1146–1158. [Google Scholar] [CrossRef]
- Zavatta, N. Crack growth in adhesively bonded joints under quasi-static and fatigue loading. Doctoral Thesis, Alma Mater Studiorum-Università di Bologna, Bologna, Italy, 2020. [Google Scholar] [CrossRef]
- Pascoe, J.A.; Zavatta, N.; Troiani, E.; Alderliesten, R.C. The effect of bond-line thickness on fatigue crack growth rate in adhesively bonded joints. Eng. Fract. Mech. 2020, 229, 106959. [Google Scholar] [CrossRef]
- Geubelle, P.H.; Baylor, J.S. Impact-induced delamination of composites: A 2D simulation. Compos. Part B Eng. 1998, 29, 589–602. [Google Scholar] [CrossRef]
- Turon, A.; Costa, J.; Camanho, P.P.; Dávila, C.G. Simulation of delamination in composites under high-cycle fatigue. Compos. Part A Appl. Sci. Manuf. 2007, 38, 2270–2282. [Google Scholar] [CrossRef]
- Pirondi, A.; Moroni, F. Improvement of a Cohesive Zone Model for Fatigue Delamination Rate Simulation. Materials 2019, 12, 181. [Google Scholar] [CrossRef] [PubMed]
- Harper, P.W.; Hallett, S.R. Cohesive zone length in numerical simulations of composite delamination. Eng. Fract. Mech. 2008, 75, 4774–4792. [Google Scholar] [CrossRef]
- Kawashita, L.F.; Hallett, S.R. A crack tip tracking algorithm for cohesive interface element analysis of fatigue delamination propagation in composite materials. Int. J. Solids Struct. 2012, 49, 2898–2913. [Google Scholar] [CrossRef]
- Li, G.; Li, C. Assessment of debond simulation and cohesive zone length in a bonded composite joint. Compos. Part B Eng. 2015, 69, 359–368. [Google Scholar] [CrossRef]
- Dassault Systèmes SE. Abaqus User Subroutines Guide; Dassault Systèmes: Vélizy-Villacoublay, France, 2024. [Google Scholar]
- Schijve, J. Fatigue of Structures and Materials; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2009. [Google Scholar]



| Specimen | Adhesive Thickness [ml | Displacement Ratio (R) | C | m | Maximum Displacement [ml |
|---|---|---|---|---|---|
| G-002-II | 0.275 | 0.29 | 1.4775 | 6.3322 | 4.083 |
| G-010-II | 0.285 | 0.036 | 0.58133 | 4.9545 | 2.893 |
| H-002-II | 0.195 | 0.036 | 0.4185 | 5.1144 | 6.27 |
| H-003-II | 0.135 | 0.29 | 2.4102 | 6.8236 | 5.13 |
| H-004-I | 0.245 | 0.61 | 72.4202 | 10.3649 | 2.3275 |
| Properties | Symbol | Value | Units |
|---|---|---|---|
| Young’s Modulus | E | 3000 | MPa |
| Normal Stiffness | N/mm3 | ||
| Tangential Stiffness | N/mm3 | ||
| Cohesive Strength (Mode I) | 50 | MPa | |
| Fracture Toughness (Mode I) | N/mm |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Birnie, J.; Falaschetti, M.P.; Troiani, E. Effect of Adhesive Thickness on Fatigue Disbonding Through a Cohesive Zone Modelling Approach. Eng. Proc. 2025, 90, 65. https://doi.org/10.3390/engproc2025090065
Birnie J, Falaschetti MP, Troiani E. Effect of Adhesive Thickness on Fatigue Disbonding Through a Cohesive Zone Modelling Approach. Engineering Proceedings. 2025; 90(1):65. https://doi.org/10.3390/engproc2025090065
Chicago/Turabian StyleBirnie, Johan, Maria Pia Falaschetti, and Enrico Troiani. 2025. "Effect of Adhesive Thickness on Fatigue Disbonding Through a Cohesive Zone Modelling Approach" Engineering Proceedings 90, no. 1: 65. https://doi.org/10.3390/engproc2025090065
APA StyleBirnie, J., Falaschetti, M. P., & Troiani, E. (2025). Effect of Adhesive Thickness on Fatigue Disbonding Through a Cohesive Zone Modelling Approach. Engineering Proceedings, 90(1), 65. https://doi.org/10.3390/engproc2025090065








